CRMISM Colloquium Universit Laval The New World of
















![Dense sets in C[0, 1] (AJQ, 17+) • piecewise linear functions and polynomials – Dense sets in C[0, 1] (AJQ, 17+) • piecewise linear functions and polynomials –](https://slidetodoc.com/presentation_image_h2/0a9ef8c398b0f5feba5d571e795310f6/image-50.jpg)
![Isomorphism in C[0, 1] Theorem (AJQ, 17+) 1. Smoothly dense sets give rise to Isomorphism in C[0, 1] Theorem (AJQ, 17+) 1. Smoothly dense sets give rise to](https://slidetodoc.com/presentation_image_h2/0a9ef8c398b0f5feba5d571e795310f6/image-51.jpg)
![Questions • “almost all” countable sets in C[0, 1] are Rado? – need a Questions • “almost all” countable sets in C[0, 1] are Rado? – need a](https://slidetodoc.com/presentation_image_h2/0a9ef8c398b0f5feba5d571e795310f6/image-53.jpg)


- Slides: 55

CRM-ISM Colloquium Université Laval The New World of Infinite Random Geometric Graphs Anthony Bonato Ryerson University

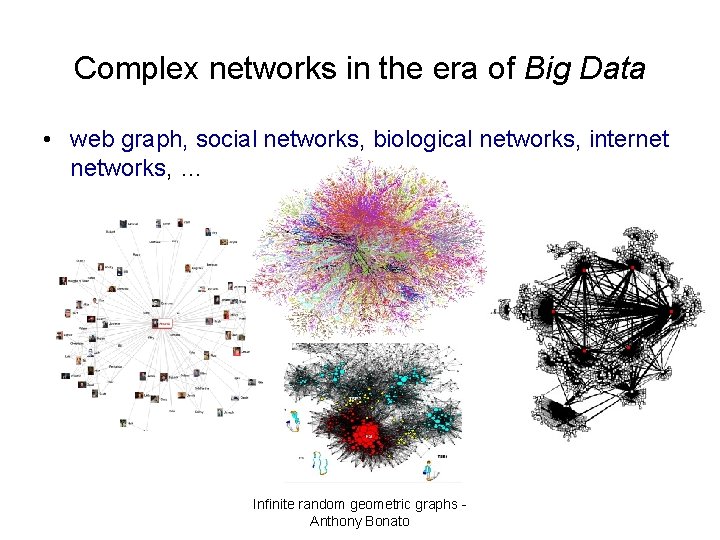
Complex networks in the era of Big Data • web graph, social networks, biological networks, internet networks, … Infinite random geometric graphs Anthony Bonato

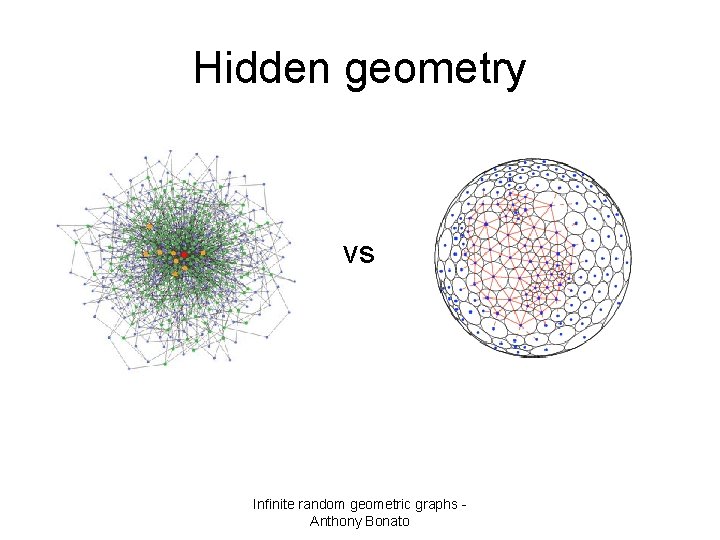
Hidden geometry vs Infinite random geometric graphs Anthony Bonato

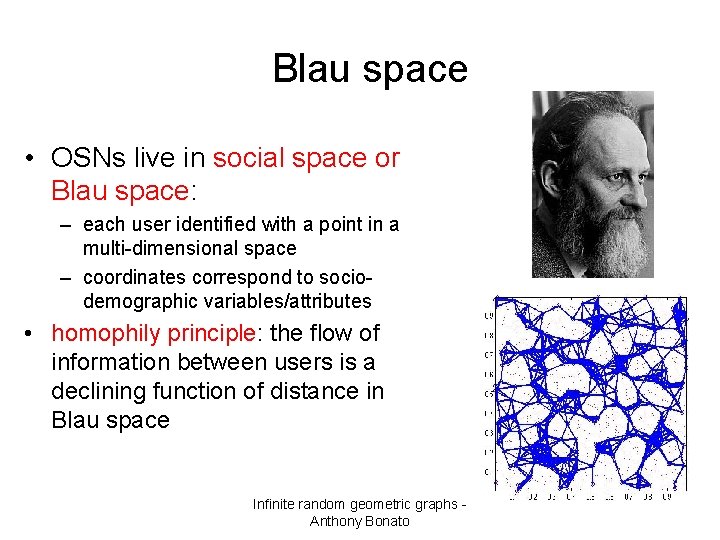
Blau space • OSNs live in social space or Blau space: – each user identified with a point in a multi-dimensional space – coordinates correspond to sociodemographic variables/attributes • homophily principle: the flow of information between users is a declining function of distance in Blau space Infinite random geometric graphs Anthony Bonato

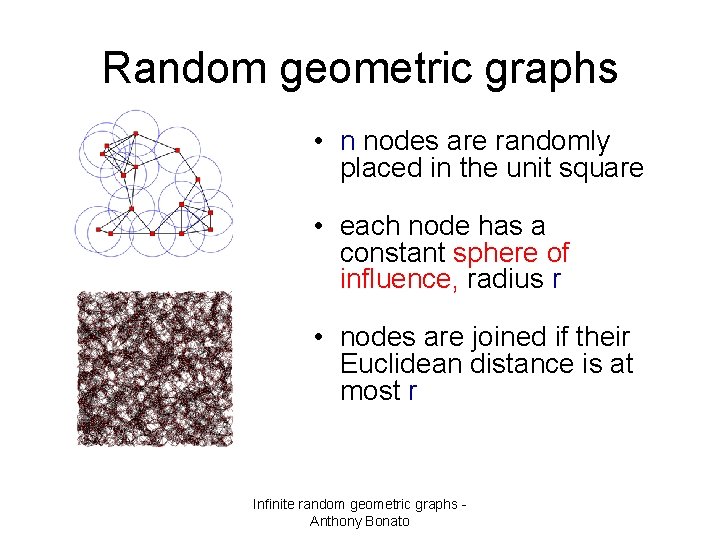
Random geometric graphs • n nodes are randomly placed in the unit square • each node has a constant sphere of influence, radius r • nodes are joined if their Euclidean distance is at most r Infinite random geometric graphs Anthony Bonato

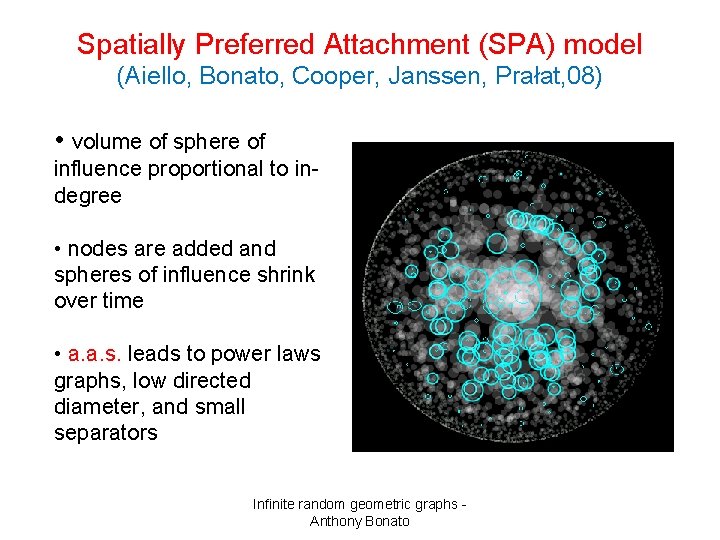
Spatially Preferred Attachment (SPA) model (Aiello, Bonato, Cooper, Janssen, Prałat, 08) • volume of sphere of influence proportional to indegree • nodes are added and spheres of influence shrink over time • a. a. s. leads to power laws graphs, low directed diameter, and small separators Infinite random geometric graphs Anthony Bonato

Into the infinite

R 111 110 101 011 100 010 Infinite random geometric graphs Anthony Bonato 001 000

Properties of R • limit graph is countably infinite • every finite graph gets added eventually – infinitely often – holds also for countable graphs • add an exponential number of vertices at each time-step Infinite random geometric graphs Anthony Bonato

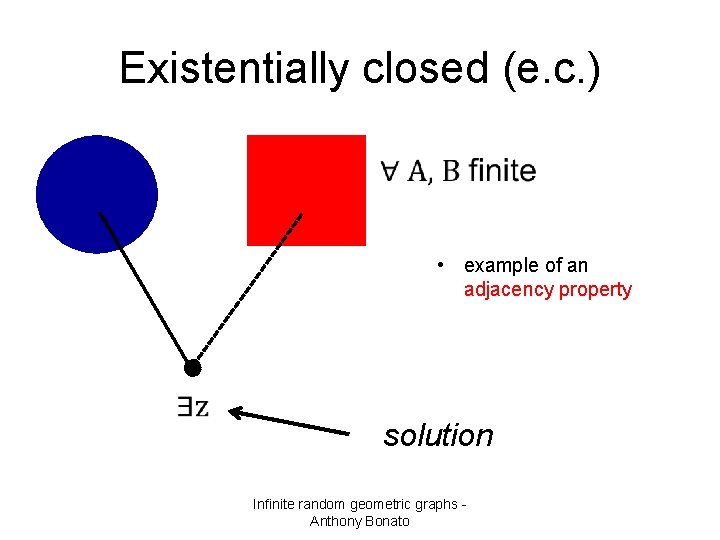
Existentially closed (e. c. ) • example of an adjacency property solution Infinite random geometric graphs Anthony Bonato

Categoricity • e. c. captures R in a strong sense Theorem (Fraïssé, 53) Any two countable e. c. graphs are isomorphic. Proof: back-and-forth argument. Infinite random geometric graphs Anthony Bonato

Explicit construction • Infinite random geometric graphs Anthony Bonato

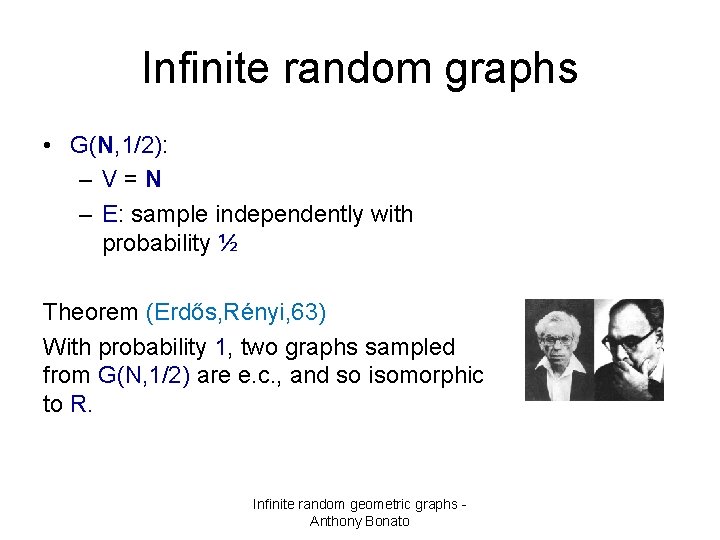
Infinite random graphs • G(N, 1/2): – V=N – E: sample independently with probability ½ Theorem (Erdős, Rényi, 63) With probability 1, two graphs sampled from G(N, 1/2) are e. c. , and so isomorphic to R. Infinite random geometric graphs Anthony Bonato

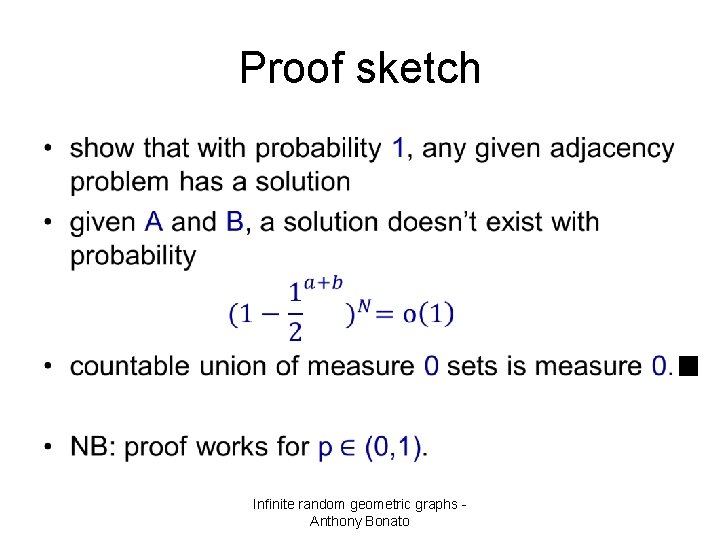
Proof sketch • Infinite random geometric graphs Anthony Bonato

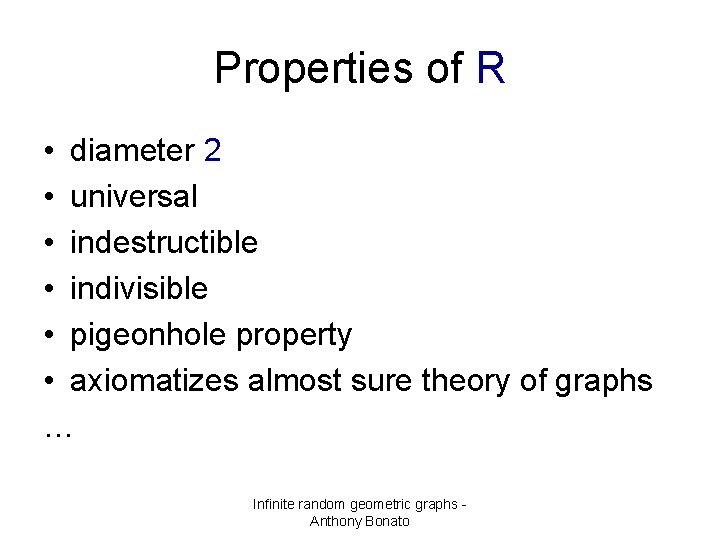
Properties of R • diameter 2 • universal • indestructible • indivisible • pigeonhole property • axiomatizes almost sure theory of graphs … Infinite random geometric graphs Anthony Bonato

More on R • A. Bonato, A Course on the Web Graph, AMS, 2008. • P. J. Cameron, The random graph, In: Algorithms and Combinatorics 14 (R. L. Graham and J. Nešetřil, eds. ), Springer Verlag, New York (1997) 333 -351. • P. J. Cameron, The random graph revisited, In: European Congress of Mathematics Vol. I (C. Casacuberta, R. M. Miró-Roig, J. Verdera and S. Xambó-Descamps, eds. ), Birkhauser, Basel (2001) 267 -274. Infinite random geometric graphs Anthony Bonato

And now for something completely different

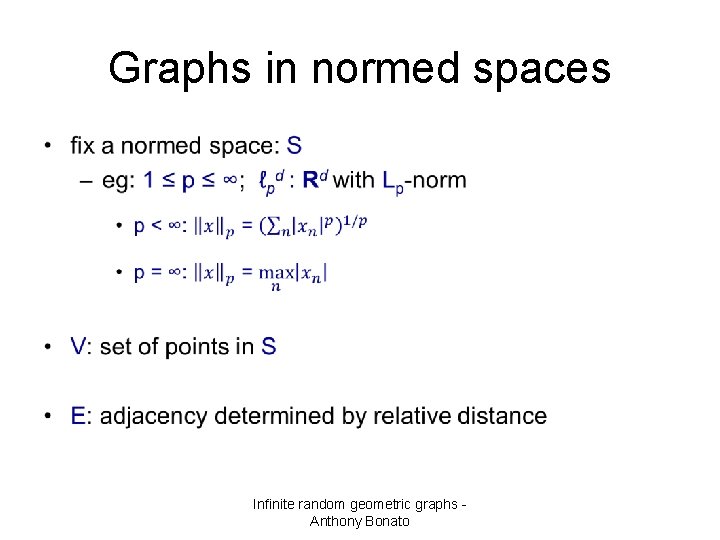
Graphs in normed spaces • Infinite random geometric graphs Anthony Bonato

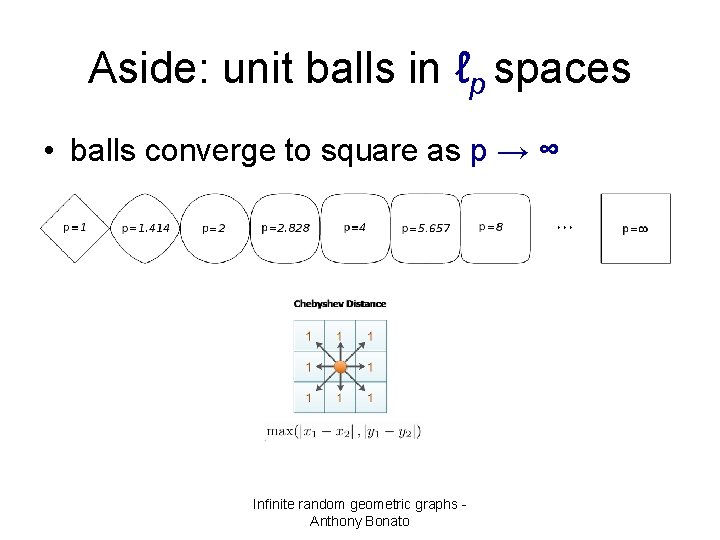
Aside: unit balls in ℓp spaces • balls converge to square as p → ∞ Infinite random geometric graphs Anthony Bonato

Random geometric graphs Infinite random geometric graphs Anthony Bonato

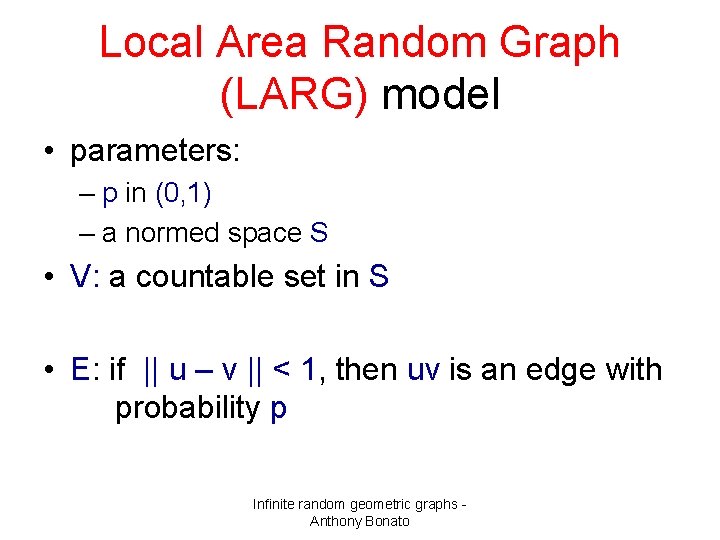
Local Area Random Graph (LARG) model • parameters: – p in (0, 1) – a normed space S • V: a countable set in S • E: if || u – v || < 1, then uv is an edge with probability p Infinite random geometric graphs Anthony Bonato

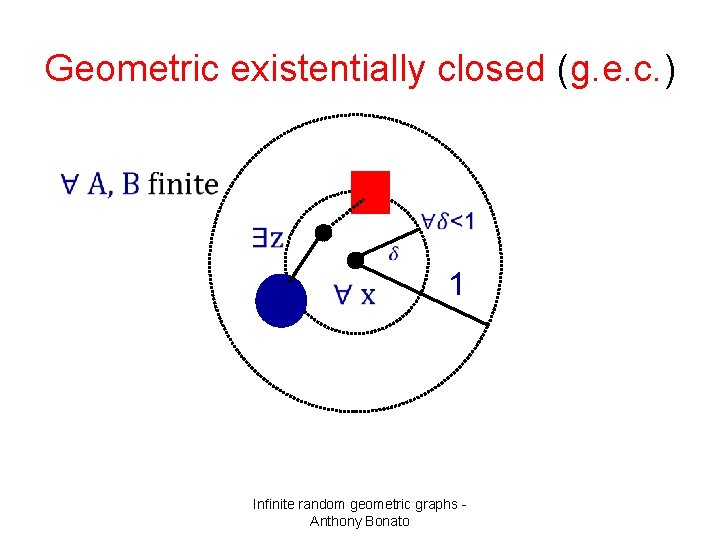
Geometric existentially closed (g. e. c. ) 1 Infinite random geometric graphs Anthony Bonato

Properties following from g. e. c • locally R • vertex sets are dense Infinite random geometric graphs Anthony Bonato

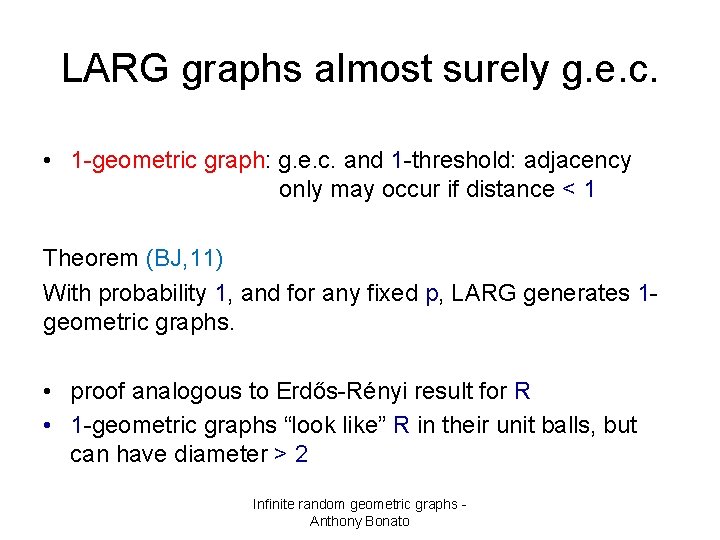
LARG graphs almost surely g. e. c. • 1 -geometric graph: g. e. c. and 1 -threshold: adjacency only may occur if distance < 1 Theorem (BJ, 11) With probability 1, and for any fixed p, LARG generates 1 geometric graphs. • proof analogous to Erdős-Rényi result for R • 1 -geometric graphs “look like” R in their unit balls, but can have diameter > 2 Infinite random geometric graphs Anthony Bonato

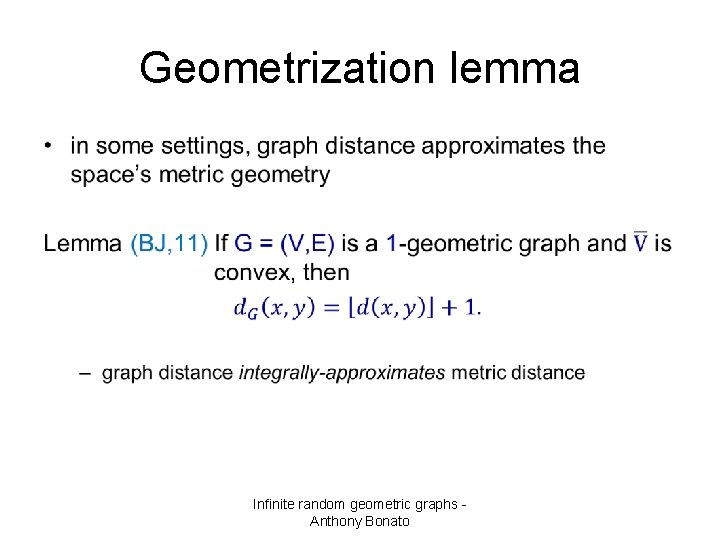
Geometrization lemma • Infinite random geometric graphs Anthony Bonato

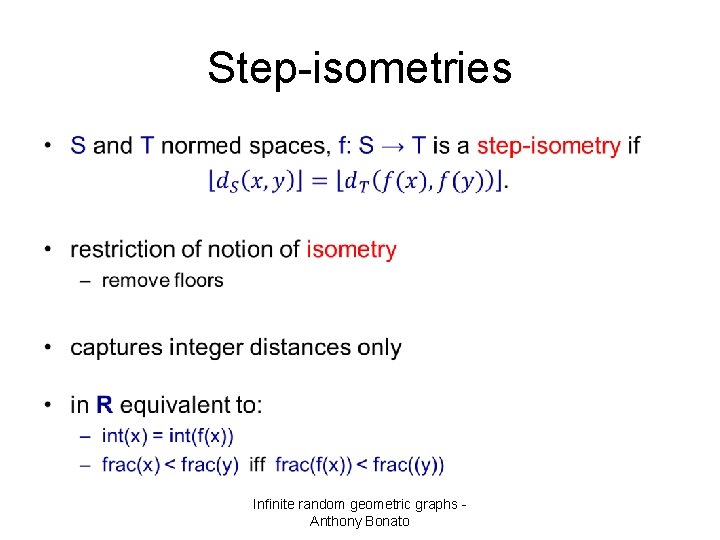
Step-isometries • Infinite random geometric graphs Anthony Bonato

Example: ℓ∞ • V: dense countable set in R • E: LARG model • integer distance free (IDF) set – pairwise ℓ∞ distance non-integer • dense sets contain idf dense sets • “random” countable dense sets are idf Infinite random geometric graphs Anthony Bonato

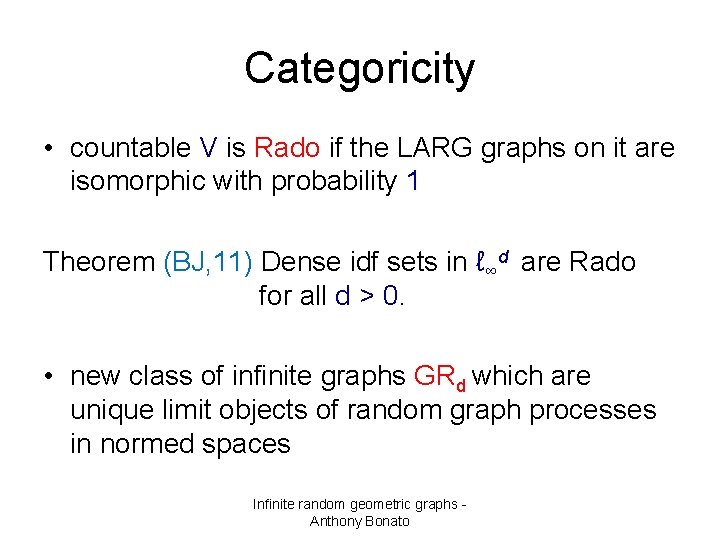
Categoricity • countable V is Rado if the LARG graphs on it are isomorphic with probability 1 Theorem (BJ, 11) Dense idf sets in ℓ∞d are Rado for all d > 0. • new class of infinite graphs GRd which are unique limit objects of random graph processes in normed spaces Infinite random geometric graphs Anthony Bonato

Sketch of proof for d = 1 • Infinite random geometric graphs Anthony Bonato

The new world

Properties of GRd • symmetry: – step-isometric isomorphisms of finite induced subgraphs extend to automorphisms • indestructible • locally R, but infinite diameter Infinite random geometric graphs Anthony Bonato

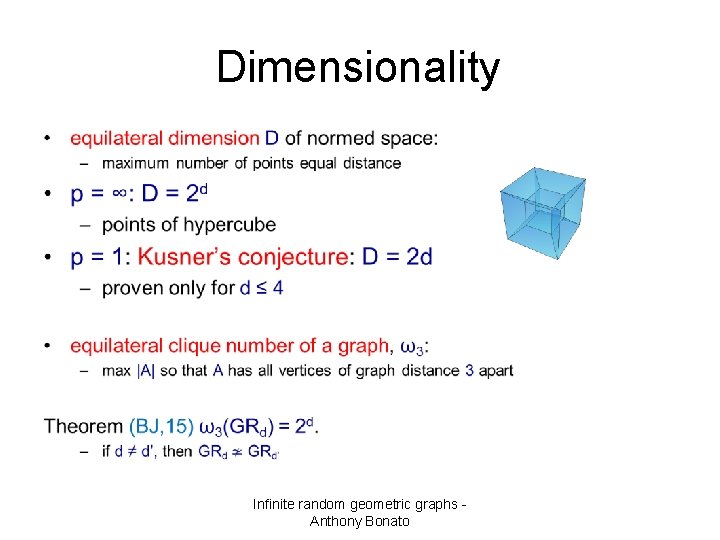
Dimensionality • Infinite random geometric graphs Anthony Bonato

Euclidean distance Lemma (BJ, 11) In ℓ 22, every step-isometry is an isometry. • countable dense V is strongly non-Rado if any two such LARG graphs on V are with probability 1 not isomorphic Corollary (BJ, 11) All countable dense sets in ℓ 22 are strongly non-Rado. • non-trivial proof, but ad hoc Infinite random geometric graphs Anthony Bonato

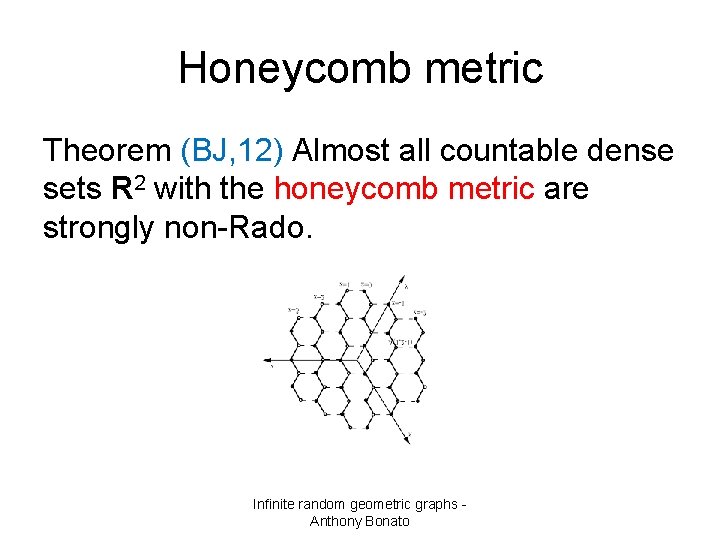
Honeycomb metric Theorem (BJ, 12) Almost all countable dense sets R 2 with the honeycomb metric are strongly non-Rado. Infinite random geometric graphs Anthony Bonato

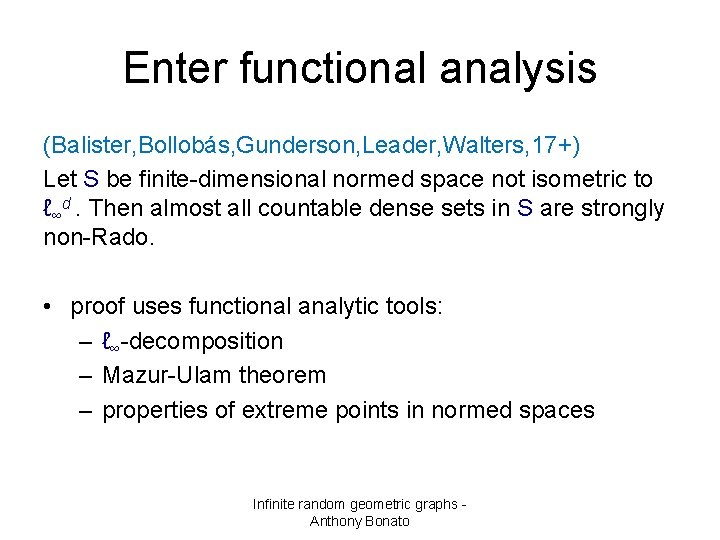
Enter functional analysis (Balister, Bollobás, Gunderson, Leader, Walters, 17+) Let S be finite-dimensional normed space not isometric to ℓ∞d. Then almost all countable dense sets in S are strongly non-Rado. • proof uses functional analytic tools: – ℓ∞-decomposition – Mazur-Ulam theorem – properties of extreme points in normed spaces Infinite random geometric graphs Anthony Bonato

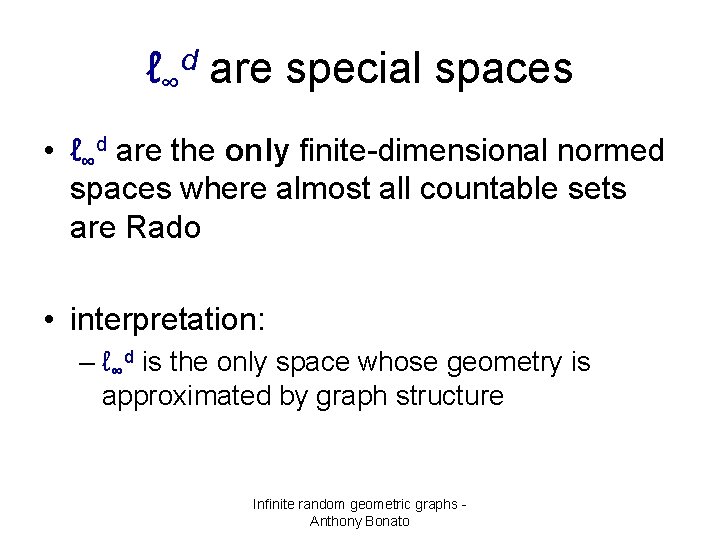
ℓ∞d are special spaces • ℓ∞d are the only finite-dimensional normed spaces where almost all countable sets are Rado • interpretation: – ℓ∞d is the only space whose geometry is approximated by graph structure Infinite random geometric graphs Anthony Bonato

Questions • classify which countable dense sets are Rado in ℓ∞d • same question, but for finite-dimensional normed spaces. • what about infinite dimensional spaces? Infinite random geometric graphs Anthony Bonato

Infinitely many parallel universes

Classical Banach spaces • C(X): continuous function on a compact Hausdorff space X – eg: C[0, 1] • ℓ∞ bounded sequences • c: convergent sequences • c 0: sequences convergent to 0 Infinite random geometric graphs Anthony Bonato

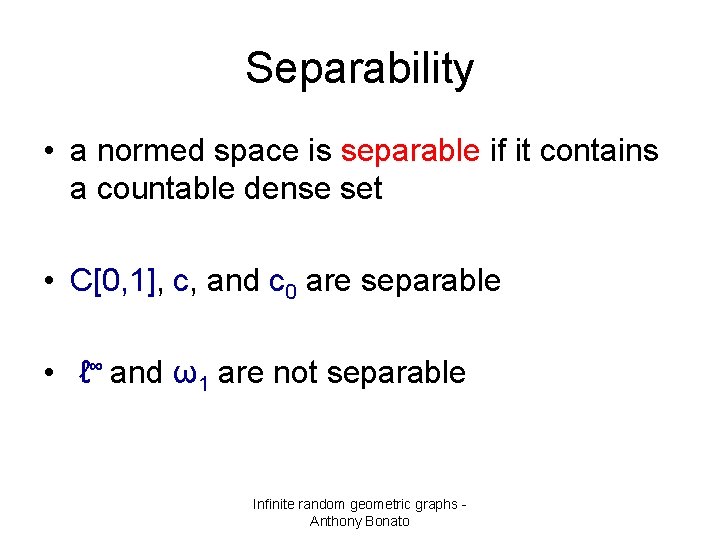
Separability • a normed space is separable if it contains a countable dense set • C[0, 1], c, and c 0 are separable • ℓ∞ and ω1 are not separable Infinite random geometric graphs Anthony Bonato

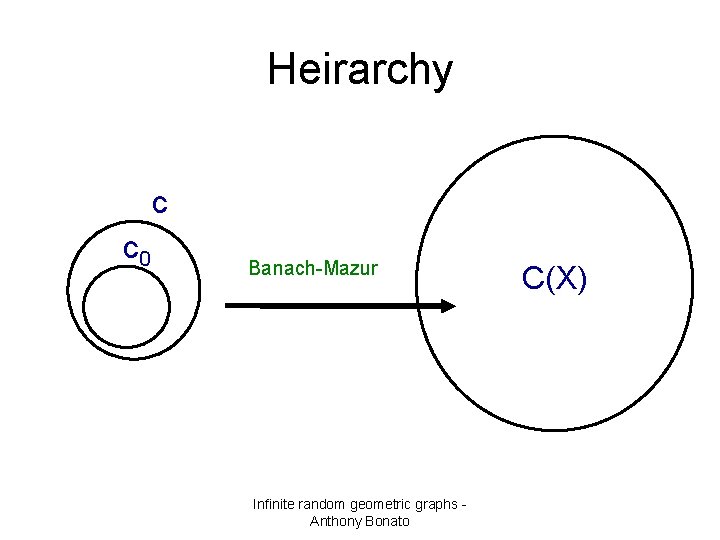
Heirarchy c c 0 Banach-Mazur Infinite random geometric graphs Anthony Bonato C(X)

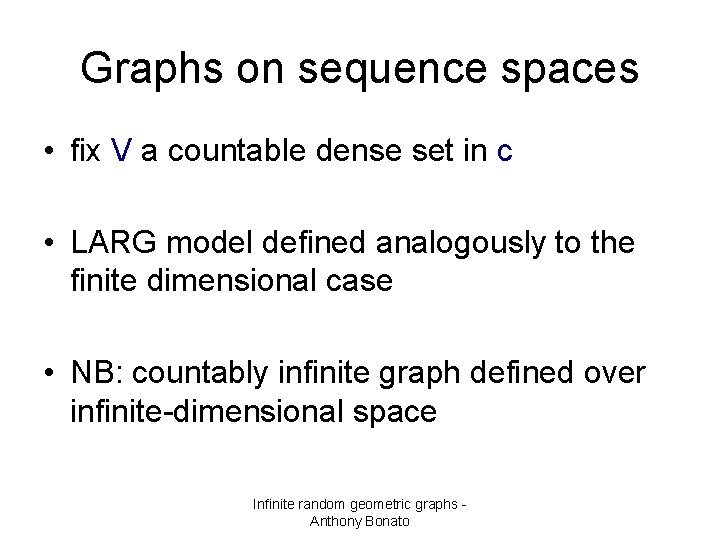
Graphs on sequence spaces • fix V a countable dense set in c • LARG model defined analogously to the finite dimensional case • NB: countably infinite graph defined over infinite-dimensional space Infinite random geometric graphs Anthony Bonato

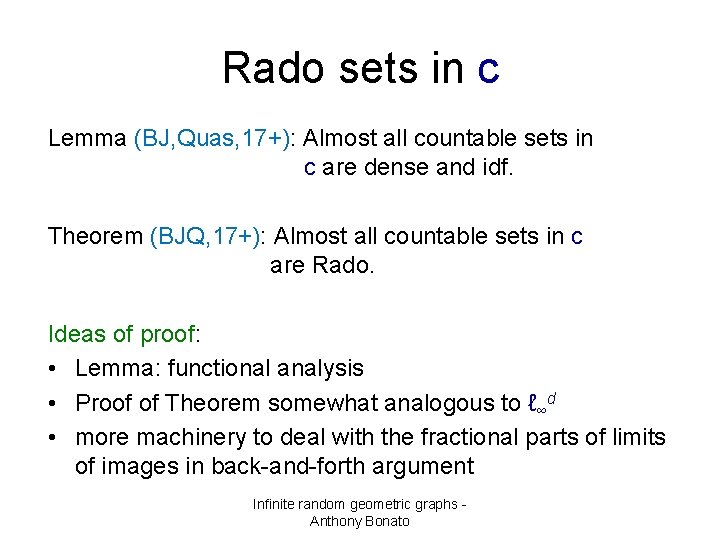
Rado sets in c Lemma (BJ, Quas, 17+): Almost all countable sets in c are dense and idf. Theorem (BJQ, 17+): Almost all countable sets in c are Rado. Ideas of proof: • Lemma: functional analysis • Proof of Theorem somewhat analogous to ℓ∞d • more machinery to deal with the fractional parts of limits of images in back-and-forth argument Infinite random geometric graphs Anthony Bonato

Rado sets in c 0 Theorem (BJ, 17+): There exist countable dense in c 0 that are Rado. • idea: consider the subspace of sequences which are eventually 0 – almost all countable sets in this subspace are dense and idf Infinite random geometric graphs Anthony Bonato

The curious geometry of sequence spaces

Geometric structure: c vs c 0 • c vs c 0 are isomorphic as vector spaces • not isometrically isomorphic: – c contains extreme points • eg: (1, 1, …) – unit ball of c 0 contains no extreme points Infinite random geometric graphs Anthony Bonato

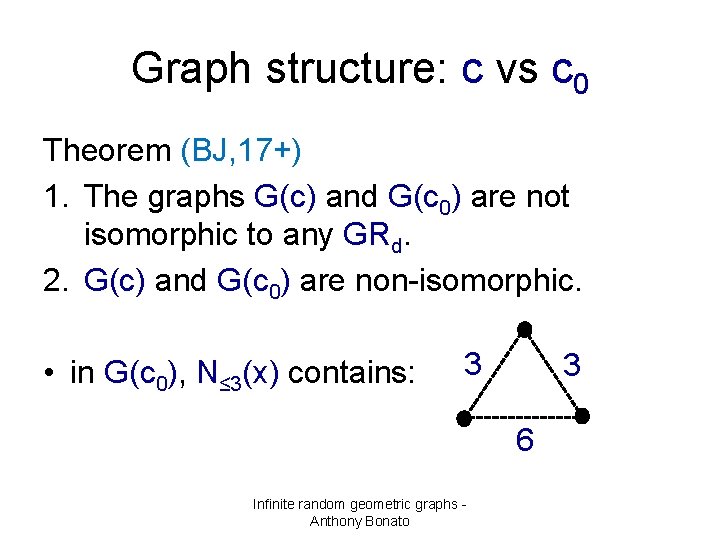
Graph structure: c vs c 0 Theorem (BJ, 17+) 1. The graphs G(c) and G(c 0) are not isomorphic to any GRd. 2. G(c) and G(c 0) are non-isomorphic. • in G(c 0), N≤ 3(x) contains: 3 3 6 Infinite random geometric graphs Anthony Bonato

Interpolating the space from the graph Theorem (BJQ, 17+) Suppose V and W are Banach spaces with dense sets X and Y. If G and H are the 1 -geometric graphs on X and Y (resp) and are isomorphic, then there is a surjective isometry from V to W. • hidden geometry: if we know LARG graphs almost surely, then we can recover the Banach space! Idea - use Dilworth’s theorem: δ-surjective ε-isometries of Banach spaces are uniformly approximated by genuine isometries Infinite random geometric graphs Anthony Bonato

Continuous functions
![Dense sets in C0 1 AJQ 17 piecewise linear functions and polynomials Dense sets in C[0, 1] (AJQ, 17+) • piecewise linear functions and polynomials –](https://slidetodoc.com/presentation_image_h2/0a9ef8c398b0f5feba5d571e795310f6/image-50.jpg)
Dense sets in C[0, 1] (AJQ, 17+) • piecewise linear functions and polynomials – almost all sets are smoothly dense • Brownian motion path functions – almost all sets are IC-dense Infinite random geometric graphs Anthony Bonato
![Isomorphism in C0 1 Theorem AJQ 17 1 Smoothly dense sets give rise to Isomorphism in C[0, 1] Theorem (AJQ, 17+) 1. Smoothly dense sets give rise to](https://slidetodoc.com/presentation_image_h2/0a9ef8c398b0f5feba5d571e795310f6/image-51.jpg)
Isomorphism in C[0, 1] Theorem (AJQ, 17+) 1. Smoothly dense sets give rise to a unique isotype of LARG graphs: GR(SD). 2. Almost surely IC-sets give rise to a unique isotype of LARG graphs: GR(ICD). Infinite random geometric graphs Anthony Bonato

Non-isomorphism Theorem (AJQ, 17+) The graphs GR(SD) and GR(ICD) are non-isomorphic. Idea: Dilworth’s theorem and Banach-Stone theorem: isometries on C[0, 1] induce homeomorphisms on [0, 1] Infinite random geometric graphs Anthony Bonato
![Questions almost all countable sets in C0 1 are Rado need a Questions • “almost all” countable sets in C[0, 1] are Rado? – need a](https://slidetodoc.com/presentation_image_h2/0a9ef8c398b0f5feba5d571e795310f6/image-53.jpg)
Questions • “almost all” countable sets in C[0, 1] are Rado? – need a suitable measure of random continuous function • which Banach spaces have Rado sets? • program: interplay of graph structure and the geometry of Banach spaces Infinite random geometric graphs Anthony Bonato

Contact • Web: http: //www. math. ryerson. ca/~abonato/ • Blog: https: //anthonybonato. com/ • • @Anthony_Bonato https: //www. facebook. com/anthony. bonato. 5

Merci!
 Onsc 7179
Onsc 7179 Universit
Universit Erasmus universit
Erasmus universit Universit of london
Universit of london Nanterre universit
Nanterre universit Universit sherbrooke
Universit sherbrooke Alfa laval sustainability report
Alfa laval sustainability report [email protected]
[email protected] Quentin martin laval
Quentin martin laval Abyssinian crisis map
Abyssinian crisis map Primate traits
Primate traits New world to old world columbian exchange
New world to old world columbian exchange Hình ảnh bộ gõ cơ thể búng tay
Hình ảnh bộ gõ cơ thể búng tay Frameset trong html5
Frameset trong html5 Bổ thể
Bổ thể Tỉ lệ cơ thể trẻ em
Tỉ lệ cơ thể trẻ em Gấu đi như thế nào
Gấu đi như thế nào Chụp tư thế worms-breton
Chụp tư thế worms-breton Chúa yêu trần thế
Chúa yêu trần thế Các môn thể thao bắt đầu bằng từ đua
Các môn thể thao bắt đầu bằng từ đua Thế nào là hệ số cao nhất
Thế nào là hệ số cao nhất Các châu lục và đại dương trên thế giới
Các châu lục và đại dương trên thế giới Công của trọng lực
Công của trọng lực Trời xanh đây là của chúng ta thể thơ
Trời xanh đây là của chúng ta thể thơ Mật thư anh em như thể tay chân
Mật thư anh em như thể tay chân Phép trừ bù
Phép trừ bù Phản ứng thế ankan
Phản ứng thế ankan Các châu lục và đại dương trên thế giới
Các châu lục và đại dương trên thế giới Thể thơ truyền thống
Thể thơ truyền thống Quá trình desamine hóa có thể tạo ra
Quá trình desamine hóa có thể tạo ra Một số thể thơ truyền thống
Một số thể thơ truyền thống Cái miệng nó xinh thế
Cái miệng nó xinh thế Vẽ hình chiếu vuông góc của vật thể sau
Vẽ hình chiếu vuông góc của vật thể sau Thế nào là sự mỏi cơ
Thế nào là sự mỏi cơ đặc điểm cơ thể của người tối cổ
đặc điểm cơ thể của người tối cổ Giọng cùng tên là
Giọng cùng tên là Vẽ hình chiếu đứng bằng cạnh của vật thể
Vẽ hình chiếu đứng bằng cạnh của vật thể Fecboak
Fecboak Thẻ vin
Thẻ vin đại từ thay thế
đại từ thay thế điện thế nghỉ
điện thế nghỉ Tư thế ngồi viết
Tư thế ngồi viết Diễn thế sinh thái là
Diễn thế sinh thái là Các loại đột biến cấu trúc nhiễm sắc thể
Các loại đột biến cấu trúc nhiễm sắc thể Số nguyên tố là gì
Số nguyên tố là gì Tư thế ngồi viết
Tư thế ngồi viết Lời thề hippocrates
Lời thề hippocrates Thiếu nhi thế giới liên hoan
Thiếu nhi thế giới liên hoan ưu thế lai là gì
ưu thế lai là gì Hươu thường đẻ mỗi lứa mấy con
Hươu thường đẻ mỗi lứa mấy con Sự nuôi và dạy con của hươu
Sự nuôi và dạy con của hươu Sơ đồ cơ thể người
Sơ đồ cơ thể người Từ ngữ thể hiện lòng nhân hậu
Từ ngữ thể hiện lòng nhân hậu Thế nào là mạng điện lắp đặt kiểu nổi
Thế nào là mạng điện lắp đặt kiểu nổi Split direct speech worksheet
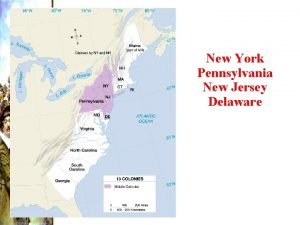
Split direct speech worksheet New york, new jersey, pennsylvania, and delaware
New york, new jersey, pennsylvania, and delaware