MATH 2401 Harrell Curves A lengthy story Lecture













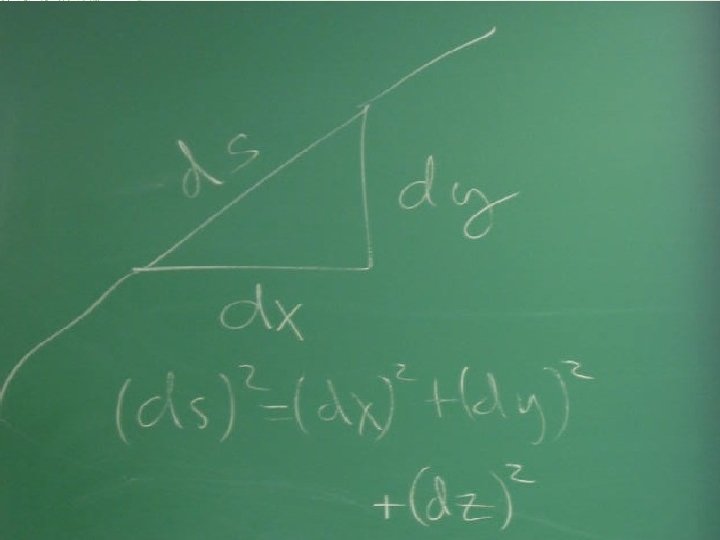

















































- Slides: 97

MATH 2401 - Harrell Curves - A lengthy story Lecture 4 Copyright 2007 by Evans M. Harrell II.

Reminder… No class on Monday, but ….

Reminder… No class on Monday, but …. There’s a test on Thursday!


a h W y l e n o l a t ! e v i arch


In our previous episode: We encountered tangent vectors The velocity vector is tangent to the curve. All tangents (velocities) are proportional. They may have different lengths but their directions are the same. Or exactly opposite (negative multiple). Exceptional case: v = 0.

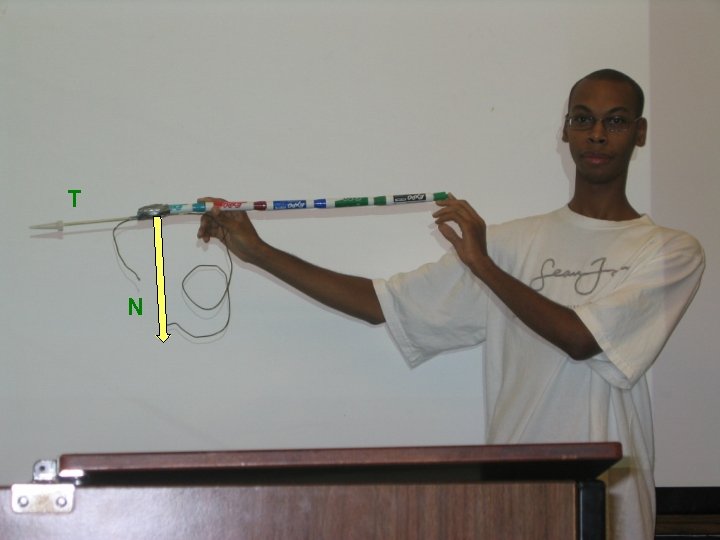
Normal vectors N = T /|T |. Unless the curve is straight at position P, N is defined as a unit vector perpendicular to T.

Who in the cast of characters might show up on the test? Curves r(t), velocity v(t). Tangent and normal lines. Angles at which curves cross. T, N, B, and the curvature and torsion t. The arc length s. The osculating plane.

Let’s vote! Choice #1…. Charge ahead and learn about curvature and osculating planes. Choice #2…. Spend more time on curves.

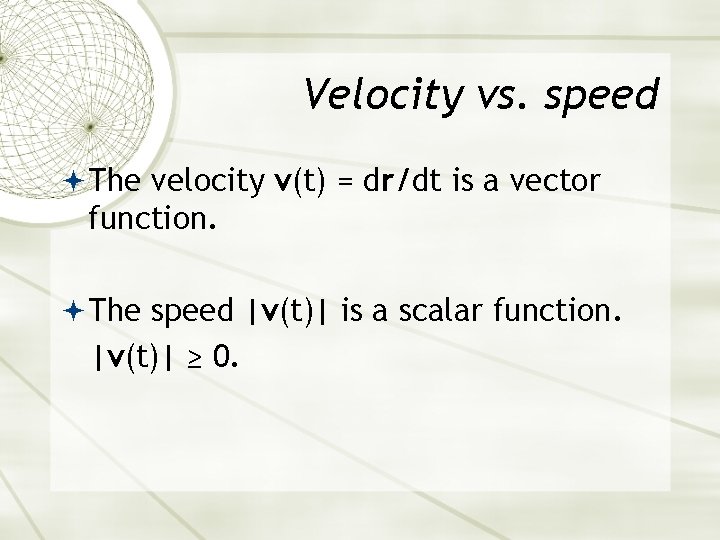
Velocity vs. speed The velocity v(t) = dr/dt is a vector function. The speed |v(t)| is a scalar function. |v(t)| ≥ 0.

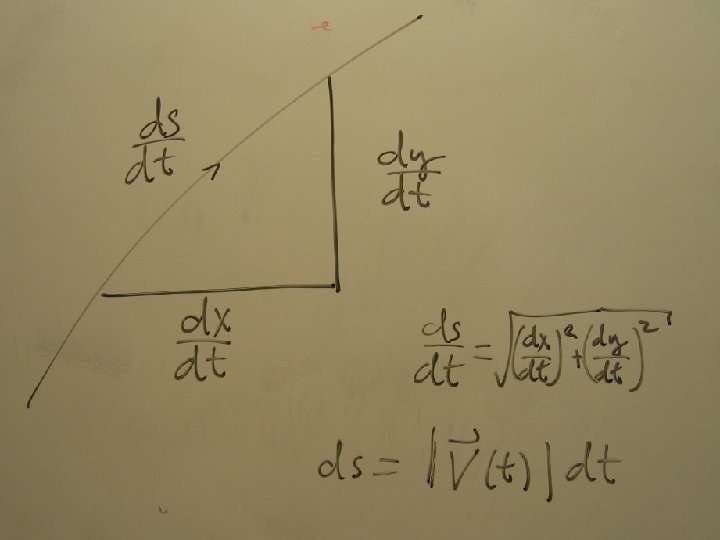
Arc length If an ant crawls at 1 cm/sec along a curve, the time it takes from a to b is the arc length from a to b.



Arc length If an ant crawls at 1 cm/sec along a curve, the time it takes from a to b is the arc length from a to b. More generally, ds = |v(t)| dt

Arc length If an ant crawls at 1 cm/sec along a curve, the time it takes from a to b is the arc length from a to b. More generally, ds = |v(t)| dt In 2 -D ds = (1 + y 2)1/2 dx, or ds 2 = dx 2 + dy 2 or…
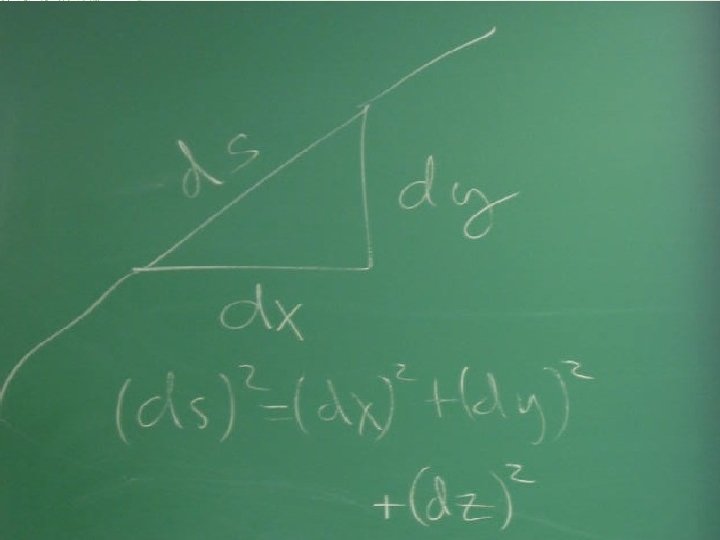


Arc length

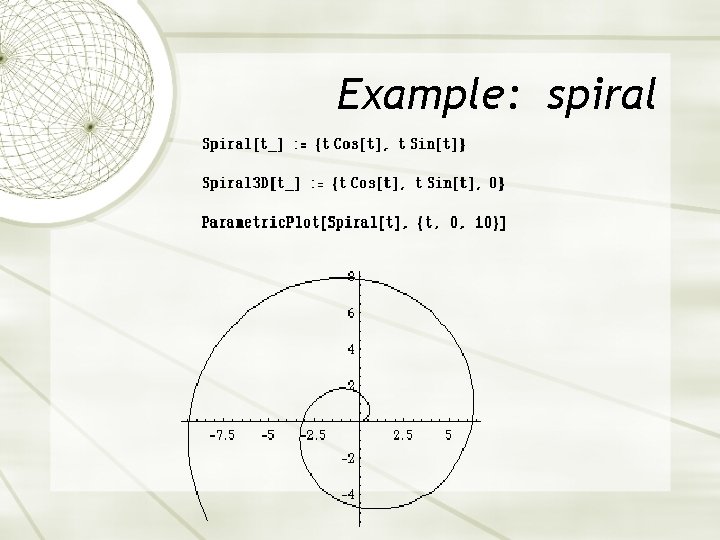
Example: spiral

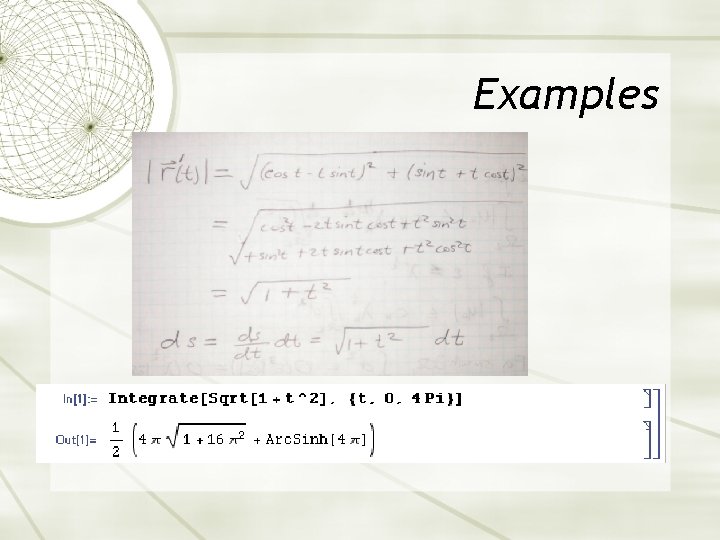
Examples

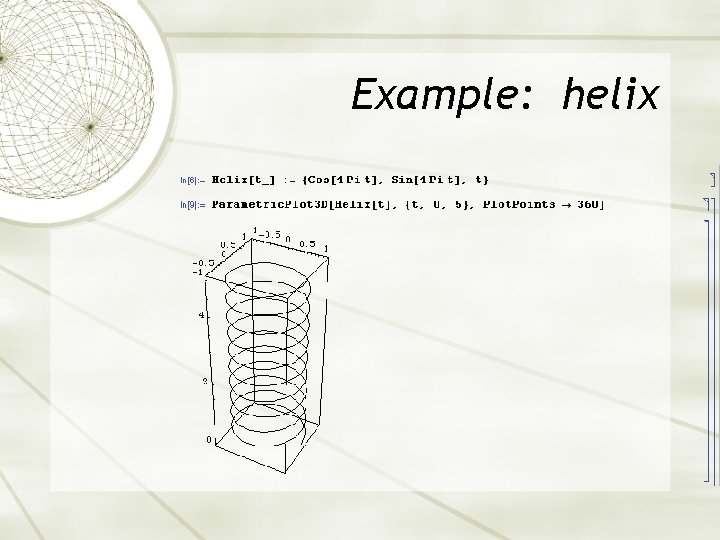
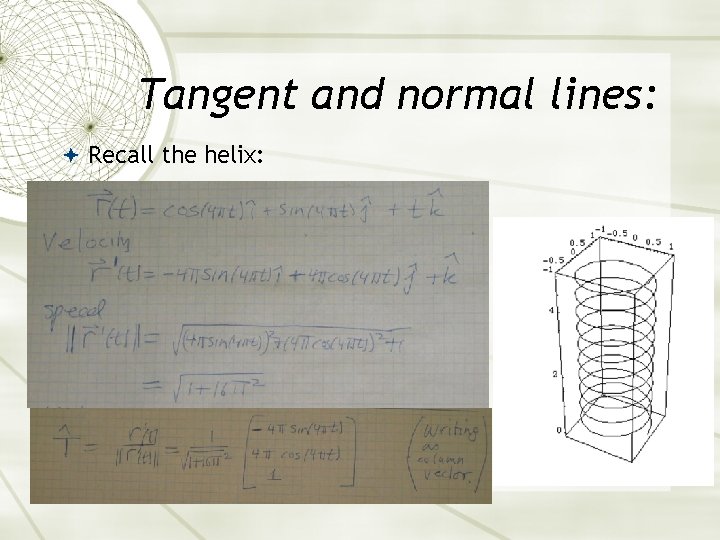
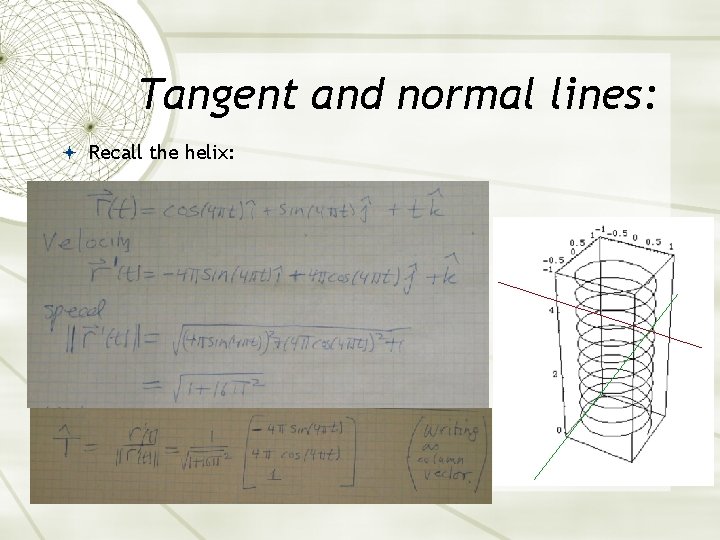
Example: helix

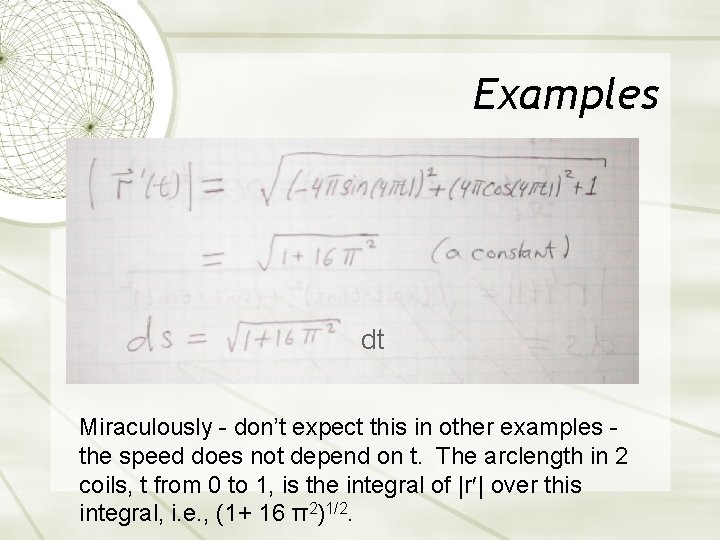
Examples dt Miraculously - don’t expect this in other examples the speed does not depend on t. The arclength in 2 coils, t from 0 to 1, is the integral of |r | over this integral, i. e. , (1+ 16 π2)1/2.

Unit tangent vectors Not only useful for arc length, also for understanding the curve ‘from the inside. ’ Move on curve with speed 1. T(t) = r (t)/ |r (t)|


T N

Normal vectors N = T /|T |. Unless the curve is straight at position P, N is defined as a unit vector perpendicular to T.

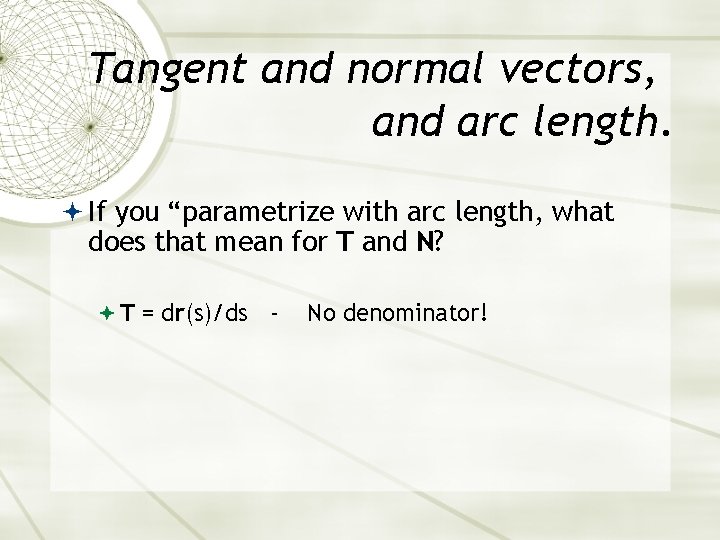
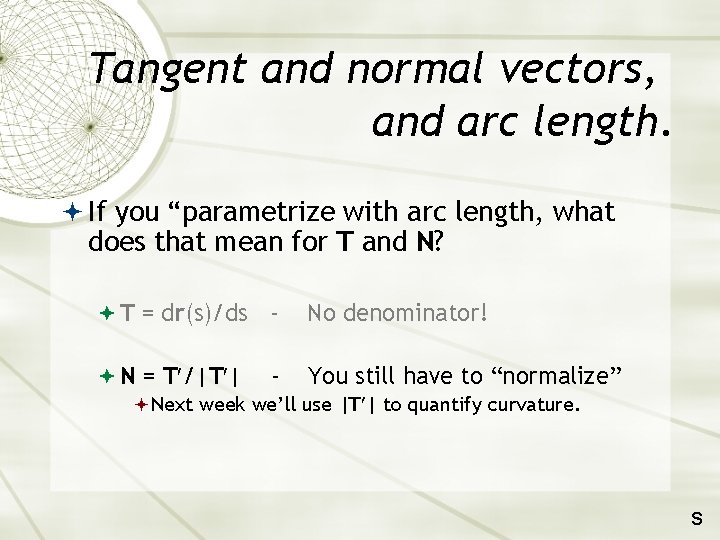
Tangent and normal vectors, and arc length. If you “parametrize with arc length, ” what does that mean for T and N?

Tangent and normal vectors, and arc length. If you “parametrize with arc length, what does that mean for T and N? T = dr(s)/ds - No denominator!

Tangent and normal vectors, and arc length. If you “parametrize with arc length, what does that mean for T and N? T = dr(s)/ds - No denominator! N = T /|T | You still have to “normalize” -

Tangent and normal vectors, and arc length. If you “parametrize with arc length, what does that mean for T and N? T = dr(s)/ds - No denominator! N = T /|T | You still have to “normalize” - Next week we’ll use |T | to quantify curvature. s



Admittedly…. You can really get tangled up in these calculations!


Tangent and normal lines: Recall the helix:

Tangent and normal lines: Recall the helix:

Tangent and normal lines: Recall the helix:

Tangent and normal lines: Ways to describe a line: slope-intercept y = m x + b 2 points, point-slope These are not so useful in 3 -D. Better: parametric form: r(t) = r 0 + u v (call parameter something other than t)

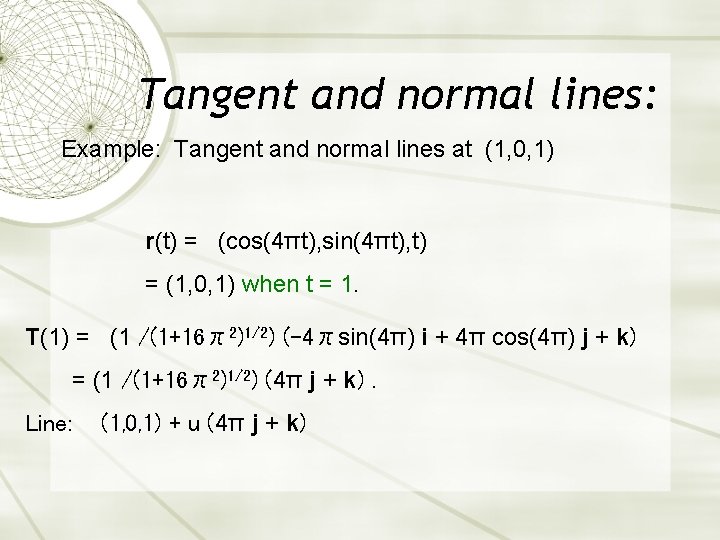
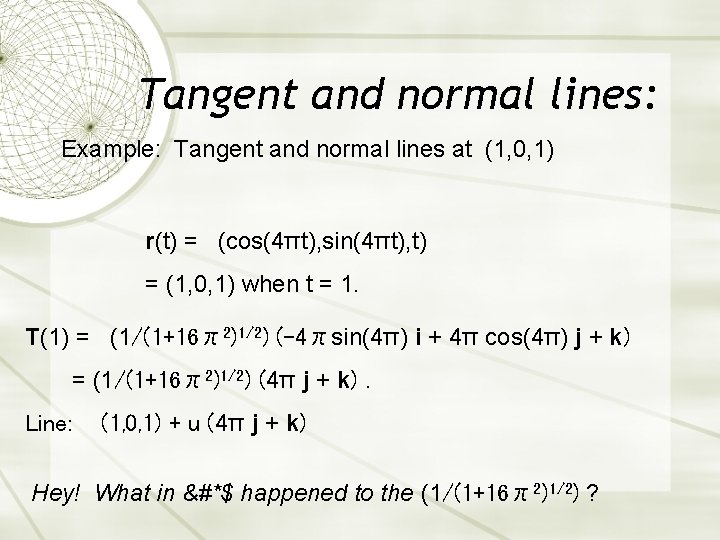
Tangent and normal lines: The essential facts about the helix: r(t) = cos(4πt) i + sin(4πt) j + t k T(t) = (1 /(1+16π2)1/2) (-4πsin(4πt) i + 4π cos(4πt) j + k) N(t) = - cos(4πt) i - sin(4πt) j Example: Tangent and normal lines at (1, 0, 1)

Tangent and normal lines: Example: Tangent and normal lines at (1, 0, 1) r(t) = (cos(4πt), sin(4πt), t) = (1, 0, 1) when t = 1. T(1) = (1 /(1+16π2)1/2) (-4πsin(4π) i + 4π cos(4π) j + k) = (1 /(1+16π2)1/2) (4π j + k). Line: (1, 0, 1) + u (4π j + k)

Tangent and normal lines: Example: Tangent and normal lines at (1, 0, 1) r(t) = (cos(4πt), sin(4πt), t) = (1, 0, 1) when t = 1. T(1) = (1/(1+16π2)1/2) (-4πsin(4π) i + 4π cos(4π) j + k) = (1/(1+16π2)1/2) (4π j + k). Line: (1, 0, 1) + u (4π j + k) Hey! What in &#*$ happened to the (1/(1+16π2)1/2) ?

Tangent and normal lines: Example: Tangent and normal lines at (1, 0, 1) r(t) = (cos(4πt), sin(4πt), t) = (1, 0, 1) when t = 1. N(1) = - cos(4π) i - sin(4π) j =- i. Line: (1, 0, 1) + u i. Wait a minute! What about the sign ?

The osculating plane Bits of curve have a “best plane. ” stickies on wire.

The osculating plane Bits of curve have a “best plane. ” stickies on wire. Each stickie contains T and N.



The osculating plane Bits of curve have a “best plane. ” One exception - a straight line lies in infinitely many planes.

The osculating plane What’s the formula, for example for the helix? 1. Parametric form 2. Single equation

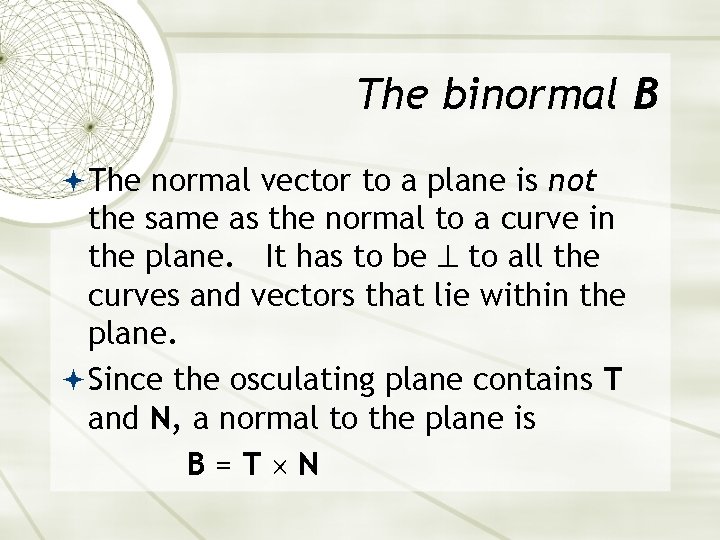
The binormal B The normal vector to a plane is not the same as the normal to a curve in the plane. It has to be ^ to all the curves and vectors that lie within the plane.

The binormal B The normal vector to a plane is not the same as the normal to a curve in the plane. It has to be ^ to all the curves and vectors that lie within the plane. Since the osculating plane contains T and N, a normal to the plane is B=T N


Close-up

The osculating plane Bits of curve have a “best plane. ” One exception - a straight line lies in infinitely many planes.

The osculating plane What’s the formula, for example for the helix? 1. Parametric form 2. Single equation

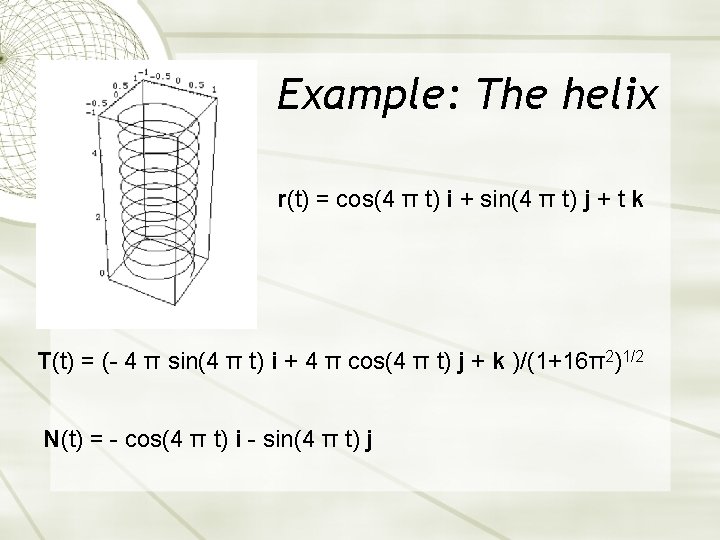
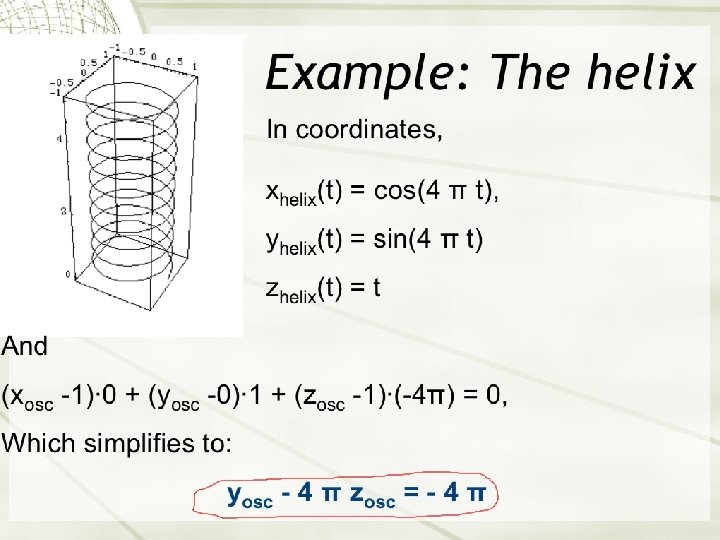
Example: The helix r(t) = cos(4 π t) i + sin(4 π t) j + t k T(t) = (- 4 π sin(4 π t) i + 4 π cos(4 π t) j + k )/(1+16π2)1/2 N(t) = - cos(4 π t) i - sin(4 π t) j

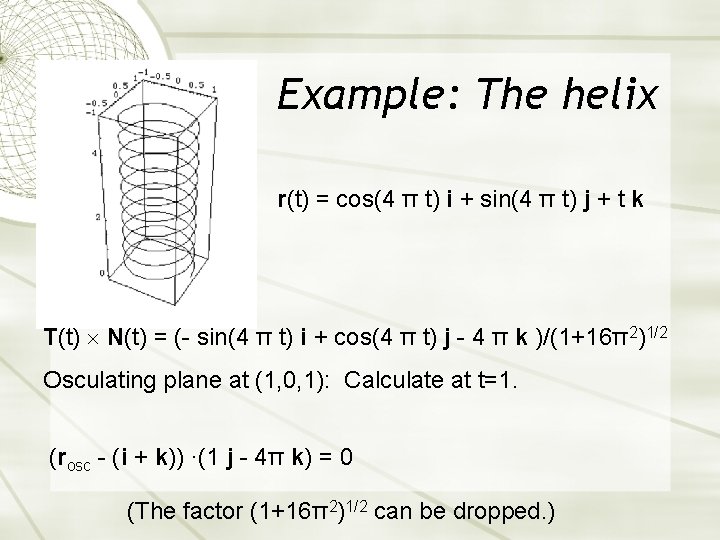
Example: The helix r(t) = cos(4 π t) i + sin(4 π t) j + t k T(t) N(t) = (- sin(4 π t) i + cos(4 π t) j - 4 π k )/(1+16π2)1/2 Osculating plane at (1, 0, 1): Calculate at t=1. (rosc - (i + k)) ·(1 j - 4π k) = 0 (The factor (1+16π2)1/2 can be dropped. )


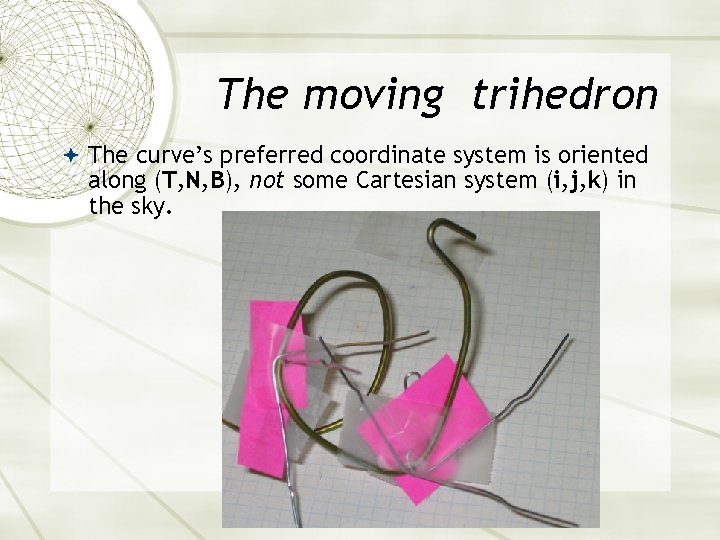
The moving trihedron The curve’s preferred coordinate system is oriented along (T, N, B), not some Cartesian system (i, j, k) in the sky.

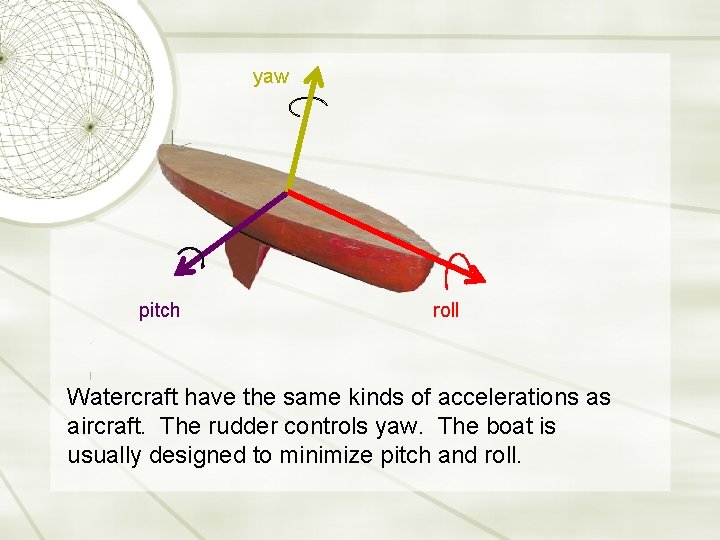
The moving trihedron A vehicle can rotate around any of these axes. A rotation around T is known as roll. If the vehicle has wings (or a hull) it may prefer a second direction over N. For example, the wing direction may correlate with N when the airplane turns without raising or lowering the nose. Such an acceleration is called yaw.

Figure from JPL/NASA

The moving trihedron However, when the aircraft soars or dives (this kind of acceleration is called pitch), the normal vector N is perpendicular to the wing axis, which in this case correlates with the binormal B. An aircraft can accelerate, roll, yaw, and pitch all at once.

The moving trihedron However, when the aircraft soars or dives (this kind of acceleration is called pitch), the normal vector N is perpendicular to the wing axis, which in this case correlates with the binormal B. An aircraft can accelerate, roll, yaw, and pitch all at once. Fasten your seatbelt!

yaw pitch roll Watercraft have the same kinds of accelerations as aircraft. The rudder controls yaw. The boat is usually designed to minimize pitch and roll.

Just what is curvature? How do you know a curve is curving? And how much?

Just what is curvature? How do you know a curve is curving? And how much? The answer should depend just on the shape of the curve, not on the speed at which it is drawn.

Just what is curvature? How do you know a curve is curving? And how much? The answer should depend just on the shape of the curve, not on the speed at which it is drawn. So it connects with arclength s, not with a time-parameter t.

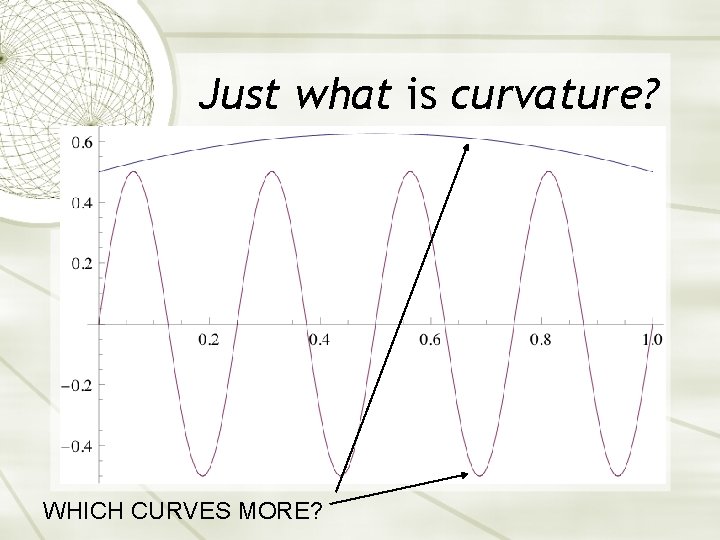
Just what is curvature? WHICH CURVES MORE?

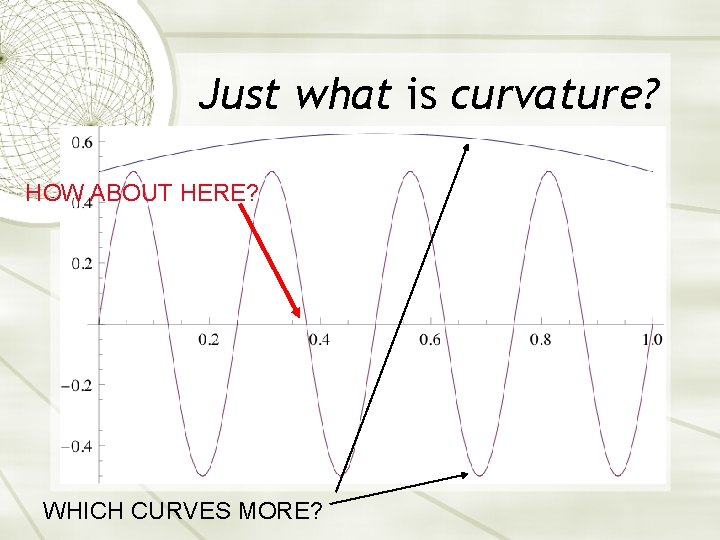
Just what is curvature? HOW ABOUT HERE? WHICH CURVES MORE?

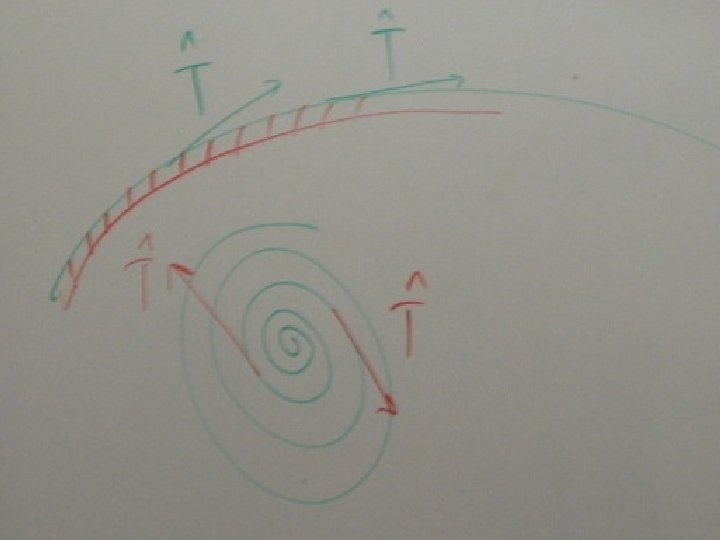
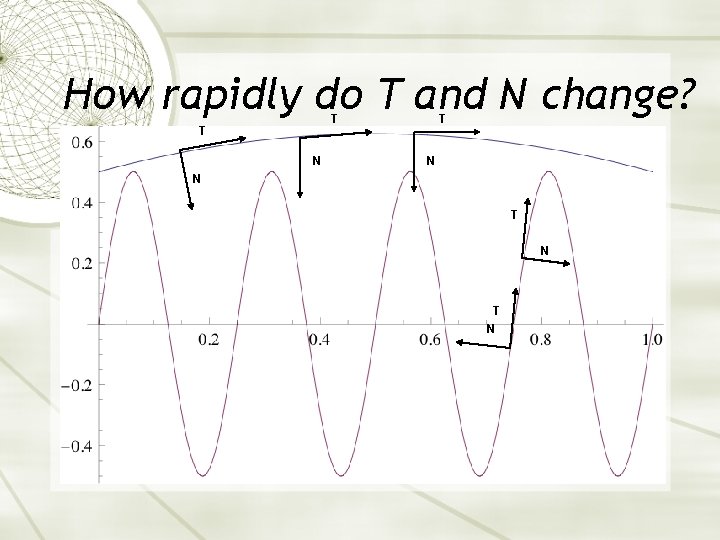
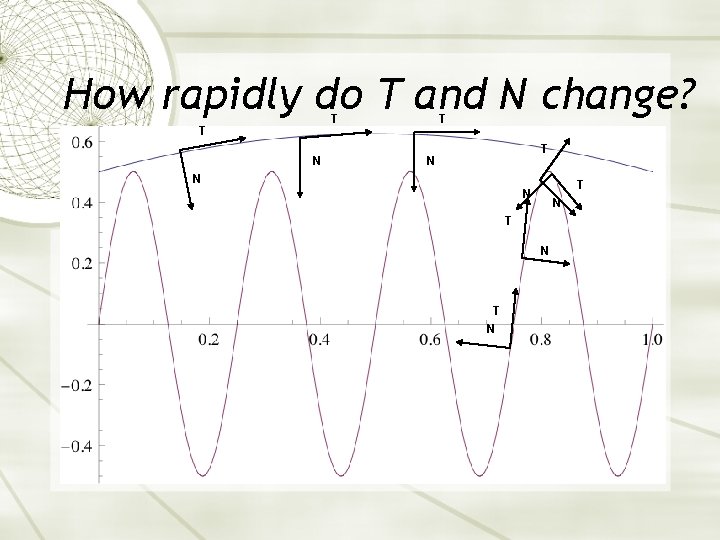
How rapidly do T and N change? T T N N T N

How rapidly do T and N change? T T N N T N

How rapidly do T and N change? T T N N N T N

How rapidly do T and N change? T T N N T N T N

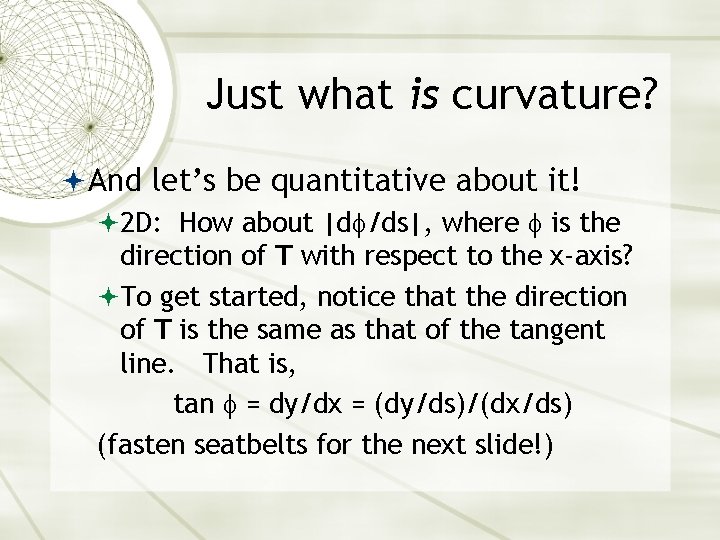
Just what is curvature? And let’s be quantitative about it! 2 D: How about |d /ds|, where is the direction of T with respect to the x-axis?

Just what is curvature? And let’s be quantitative about it! 2 D: How about |d /ds|, where is the direction of T with respect to the x-axis? Conceptually that’s reasonable. In practice it is just a bit involved…

Just what is curvature? And let’s be quantitative about it! 2 D: How about |d /ds|, where is the direction of T with respect to the x-axis? To get started, notice that the direction of T is the same as that of the tangent line. That is, tan = dy/dx = (dy/ds)/(dx/ds) (fasten seatbelts for the next slide!)


It’s our old friend the chain rule, used in a creative way!



Different expressions for = |d /ds|

Different expressions for = |d /ds| = |(d /dt)/(ds/dt)|

Different expressions for = |d /ds| = |(d /dt)/(ds/dt)| = |x (s) y (s) - y (s) x (s)|

Different expressions for = |d /ds| = |(d /dt)/(ds/dt)| = |x (s) y (s) - y (s) x (s)| = |x (t) y (t) - y (t) x (t)| |(x (t))2 + (y (t))2|3/2 Huh? ?

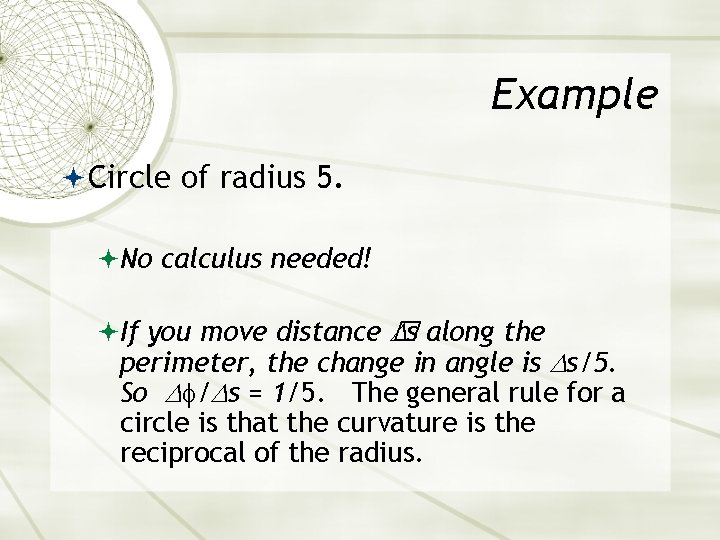
Example Circle of radius 5.

Example Circle of radius 5. No calculus needed!

Example Circle of radius 5. No calculus needed! If you move distance � s along the perimeter, the change in angle is s/5. So / s = 1/5. The general rule for a circle is that the curvature is the reciprocal of the radius.

Example Spiral: The formula for curvature is complicated, but the spiral is simple, so the curvature should be simple.

Example Spiral: The formula for curvature is complicated, but the spiral is simple, so the curvature should be simple. Still, we’ll be lazy and use Mathematica:

Example

In our previous episode: A spaceship doesn’t see a big Cartesian grid in the sky. Looked at from the inside, a better basis for vectors will use the unit tangent T, the principal normal N. , and the binormal B.

Dimensional analysis What units do you use to measure curvature?

Dimensional analysis What units do you use to measure curvature? Hint: angles are considered dimensionless, since radian measure is a ratio of arclength (cm) to radius (also cm)

Dimensional analysis What units do you use to measure curvature? Answer: 1/distance, for instance 1/cm. 1/ is known as the radius of curvature. It’s the radius of the circle that best matches the curve at a given contact point.



 Msursd
Msursd Math 2401
Math 2401 Math 2401
Math 2401 Which mode is used to write lengthy programs in python
Which mode is used to write lengthy programs in python Accounting final exam review
Accounting final exam review Cits2401
Cits2401 Motor equipment utilization record
Motor equipment utilization record Biol 2401
Biol 2401 Cs 2401
Cs 2401 Cs 2401
Cs 2401 01:640:244 lecture notes - lecture 15: plat, idah, farad
01:640:244 lecture notes - lecture 15: plat, idah, farad Math game math hit the button
Math game math hit the button Find the domain of the vector function
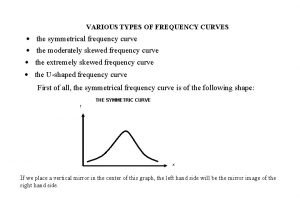
Find the domain of the vector function Different types of frequency curves
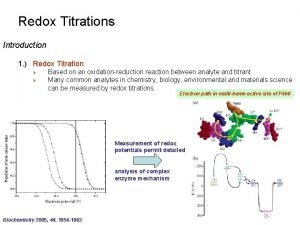
Different types of frequency curves Titration curve of alanine
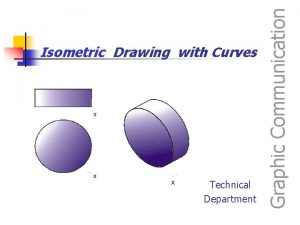
Titration curve of alanine Isometric curve
Isometric curve Some animals are dangerous *
Some animals are dangerous * What are solutions
What are solutions Area between two curves formula
Area between two curves formula Titration curve of permanganometric
Titration curve of permanganometric Railway curves iricen
Railway curves iricen Creating production possibilities schedules and curves
Creating production possibilities schedules and curves Carrying capacity population
Carrying capacity population Mirror that curves outward
Mirror that curves outward Glycine titration curve
Glycine titration curve Intersection of square prism and cylinder
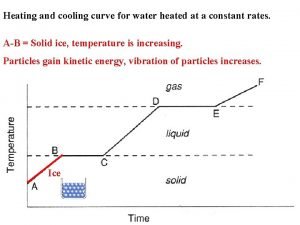
Intersection of square prism and cylinder Construction of the cooling curve for water
Construction of the cooling curve for water Gear terminology
Gear terminology Rectangular hyperbola in engineering drawing
Rectangular hyperbola in engineering drawing Is cycloid a conic section
Is cycloid a conic section Area of polar curves practice
Area of polar curves practice Learning curves 2003
Learning curves 2003 Highway curves banked and unbanked
Highway curves banked and unbanked S and j curves
S and j curves Advantages of balanced occlusion
Advantages of balanced occlusion Asymptote of a curve
Asymptote of a curve Acid base titration curve
Acid base titration curve Conductometric titration of weak acid and strong base
Conductometric titration of weak acid and strong base 18 curves com
18 curves com Area between two curves formula
Area between two curves formula Extra clearance on curves
Extra clearance on curves Drivers ed module 5 topic 3
Drivers ed module 5 topic 3 Physical behavior of matter
Physical behavior of matter How to read solubility curves
How to read solubility curves 13 curves
13 curves Uses of concave mirror
Uses of concave mirror Steep grade sharp curves
Steep grade sharp curves Indifference curve inferior good
Indifference curve inferior good Nelson curve htha
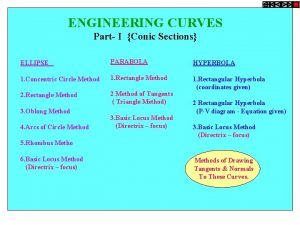
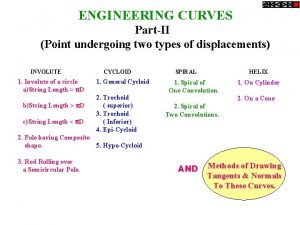
Nelson curve htha Engineering curves
Engineering curves Elliptic curves number theory and cryptography
Elliptic curves number theory and cryptography Hermite curve in computer graphics
Hermite curve in computer graphics What are curves in computer graphics
What are curves in computer graphics Chapter 1 section 3 production possibilities curves
Chapter 1 section 3 production possibilities curves Blending curve
Blending curve Learning curves in sport
Learning curves in sport Why do titration curves flatten out
Why do titration curves flatten out Why do titration curves flatten out
Why do titration curves flatten out 18curves
18curves Physical behavior of matter heating and cooling curves
Physical behavior of matter heating and cooling curves Slutsky vs hicks
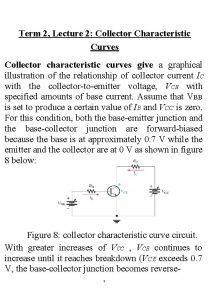
Slutsky vs hicks Collector characteristic curves
Collector characteristic curves Graph of polar equation
Graph of polar equation Determinants of pes
Determinants of pes Solubility curve calculator
Solubility curve calculator Noaa idf curves
Noaa idf curves Heating curve
Heating curve When two curves coincide the two objects have the same
When two curves coincide the two objects have the same A 2d area enclosed by lines or curves
A 2d area enclosed by lines or curves Fills in gaps in data and fit data into curves
Fills in gaps in data and fit data into curves Normal curves and sampling distributions
Normal curves and sampling distributions Drive-in diffusion
Drive-in diffusion Plotting curves in matlab
Plotting curves in matlab A graph made of connected lines or curves.
A graph made of connected lines or curves. Acid base titration equivalence point
Acid base titration equivalence point When both curves shift
When both curves shift Elee curves
Elee curves Steep incisal guidance
Steep incisal guidance Risky curves clothing
Risky curves clothing Msn quiz
Msn quiz Interesting curves
Interesting curves Curves gym
Curves gym Seinfeld
Seinfeld The sine and cosine curves intersect infinitely
The sine and cosine curves intersect infinitely Table g solubility curves
Table g solubility curves Lysine titration
Lysine titration Curves of quickest descent bernoulli
Curves of quickest descent bernoulli How to read a solubility curve
How to read a solubility curve Learning curve coefficient table
Learning curve coefficient table The secondary winding of a pth
The secondary winding of a pth Interpolation and approximation of curves in cad
Interpolation and approximation of curves in cad Standard deviation normal curve
Standard deviation normal curve Heating curve graph
Heating curve graph 6 phase changes
6 phase changes Adas graph
Adas graph Quiz curves
Quiz curves Reading fan curves
Reading fan curves Curves and surfaces for computer graphics
Curves and surfaces for computer graphics