Chapter 5 Relationships within Triangles In this chapter





















































- Slides: 135

Chapter 5 Relationships within Triangles In this chapter you will learn how special lines and segments in triangles relate.

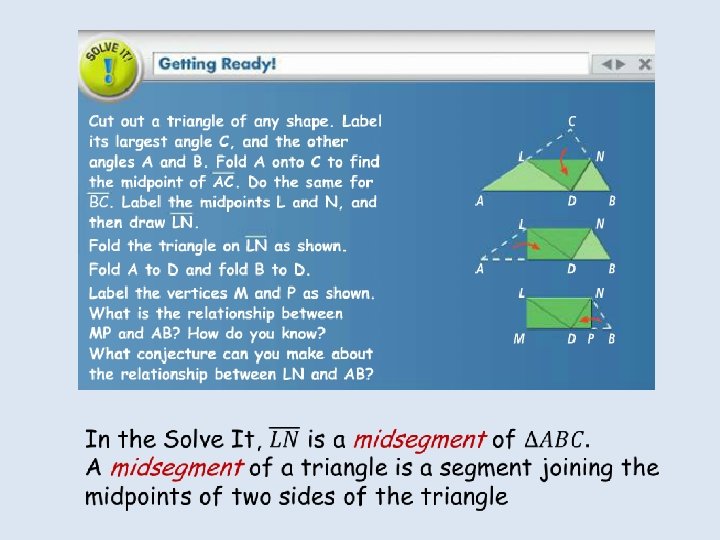
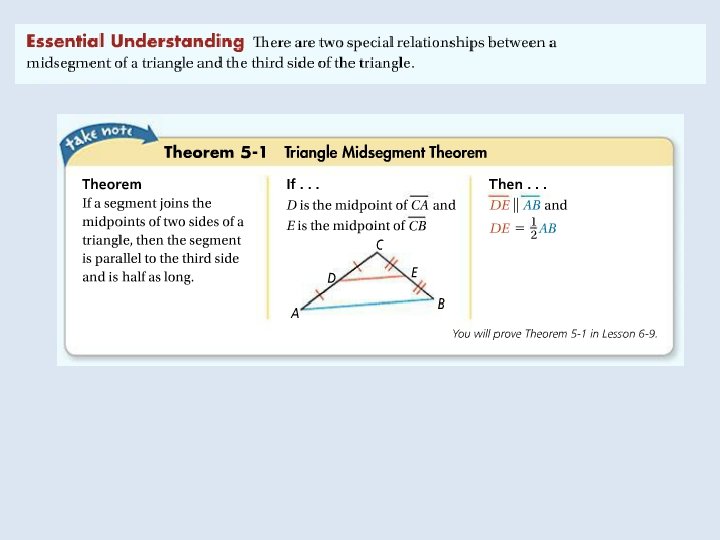
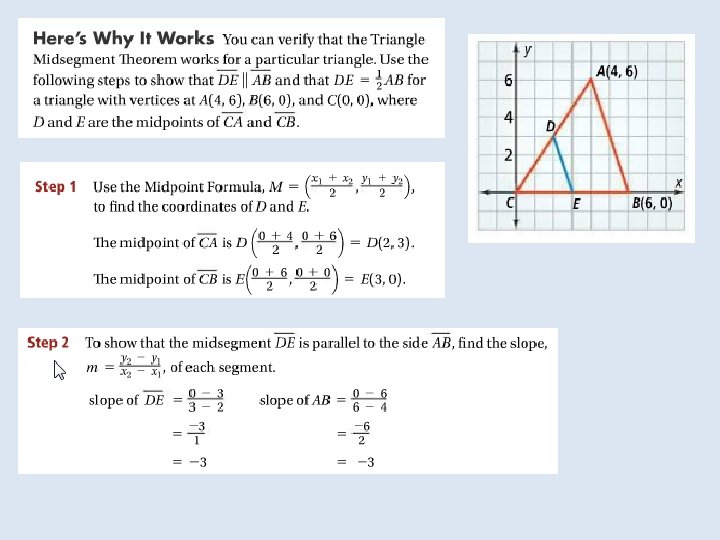
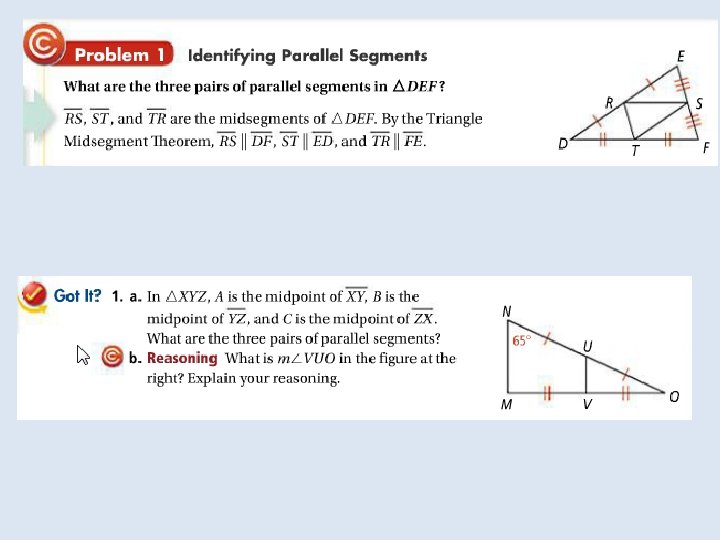
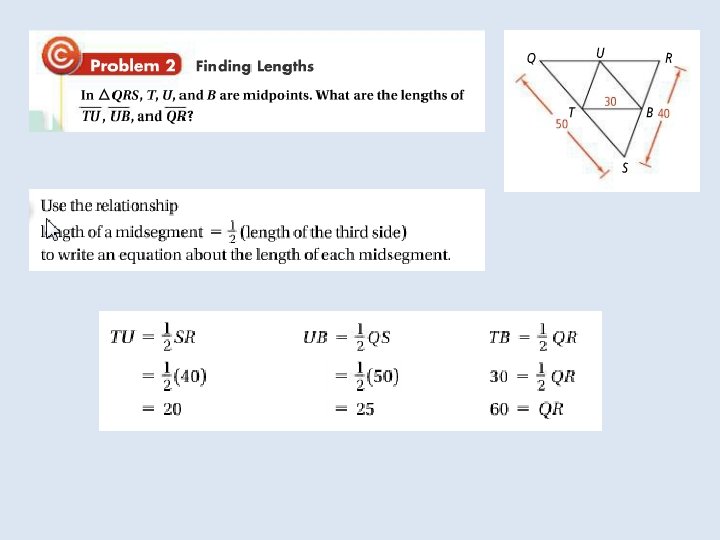
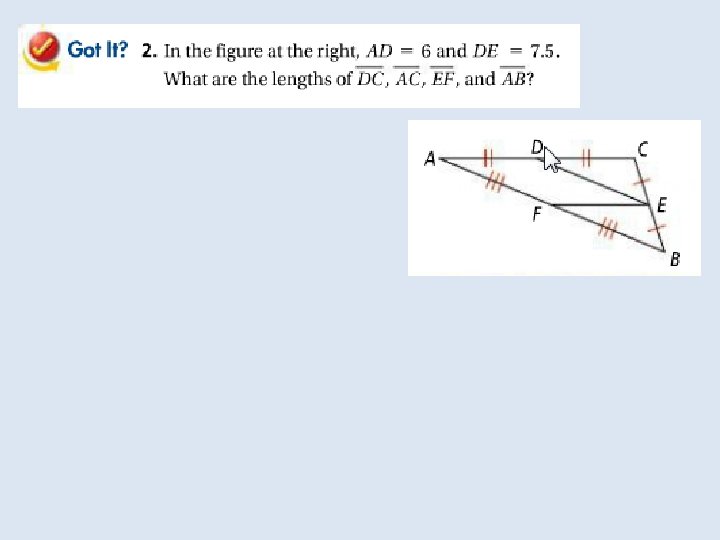
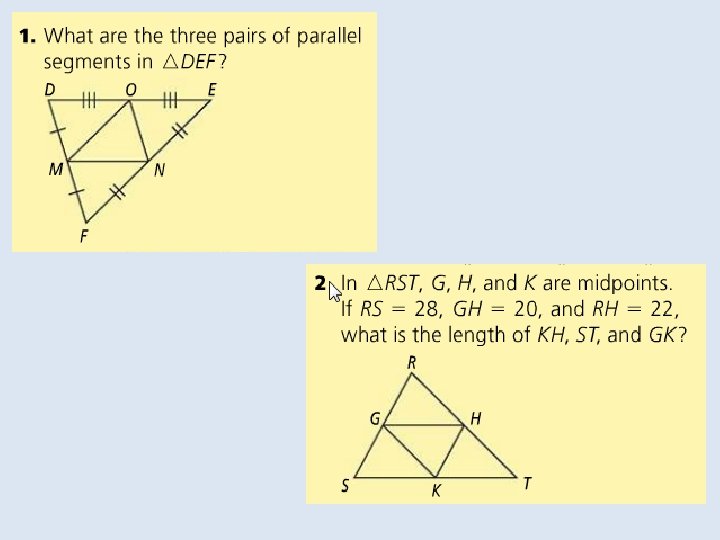
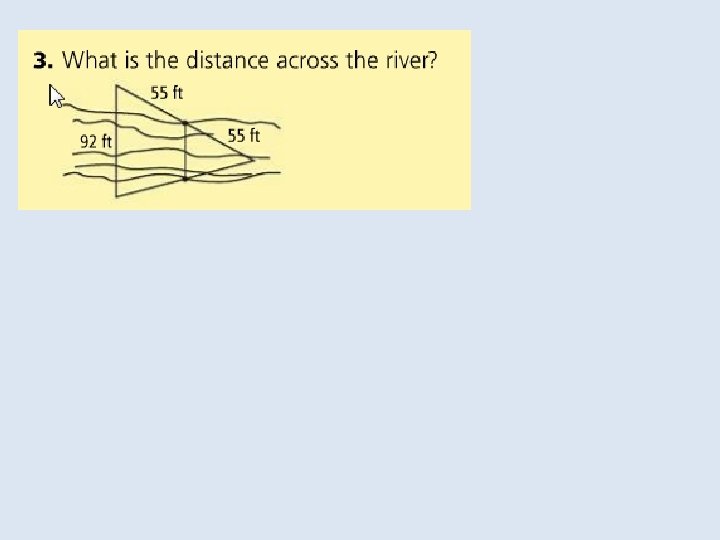
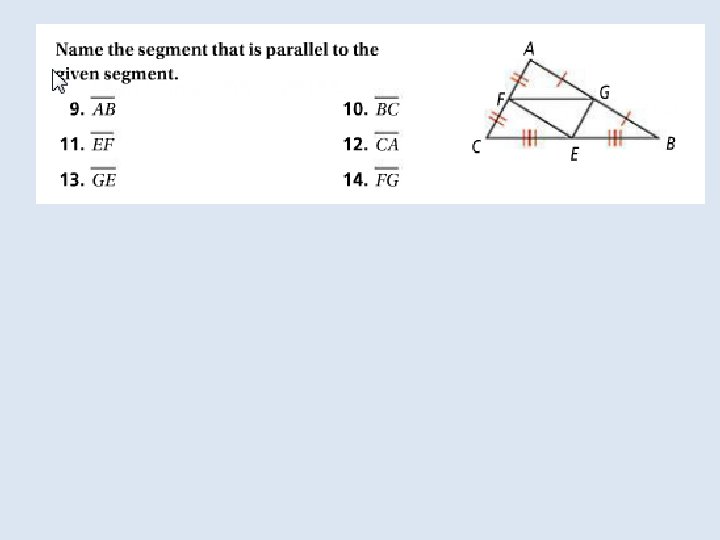
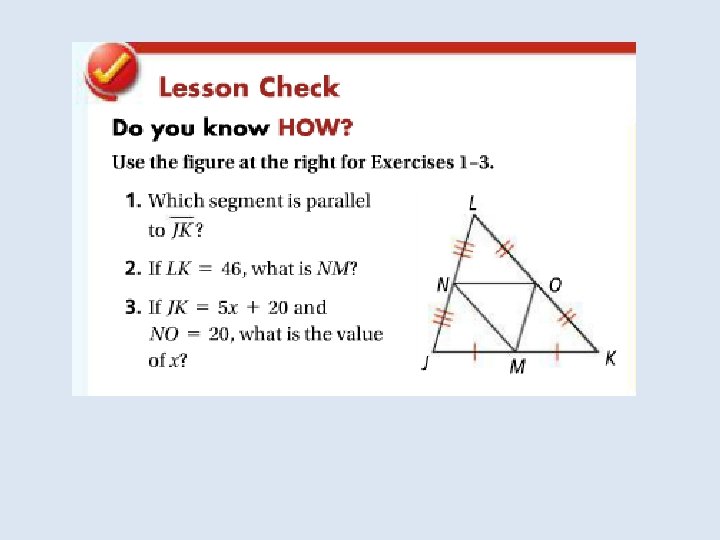
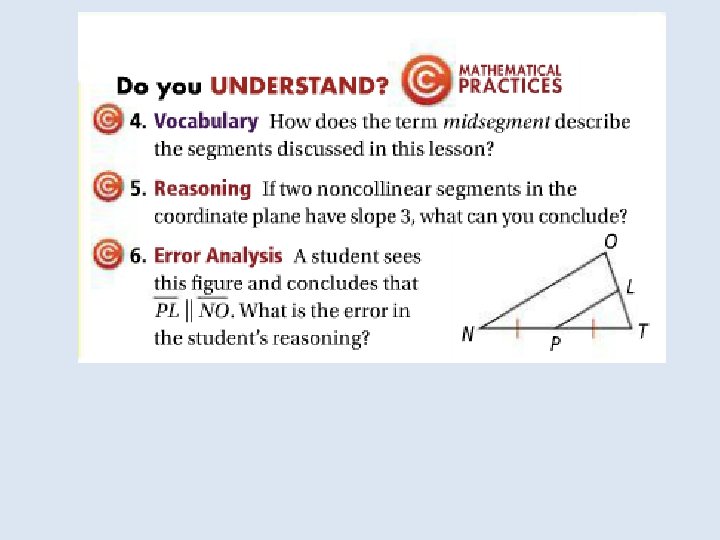
Objective: To use properties of midsegments to solve problems Vocabulary: Midsegment of a triangle


















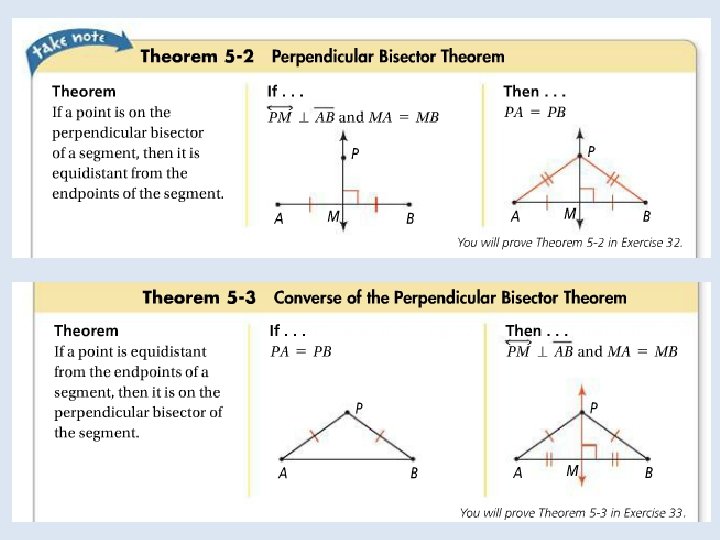
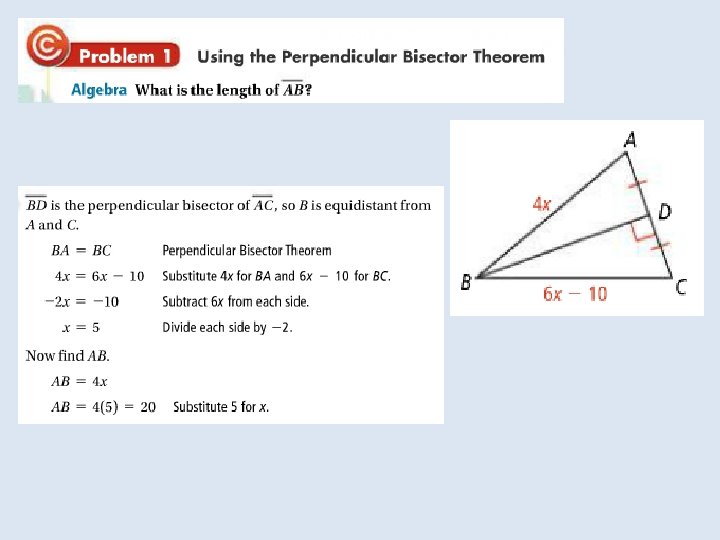
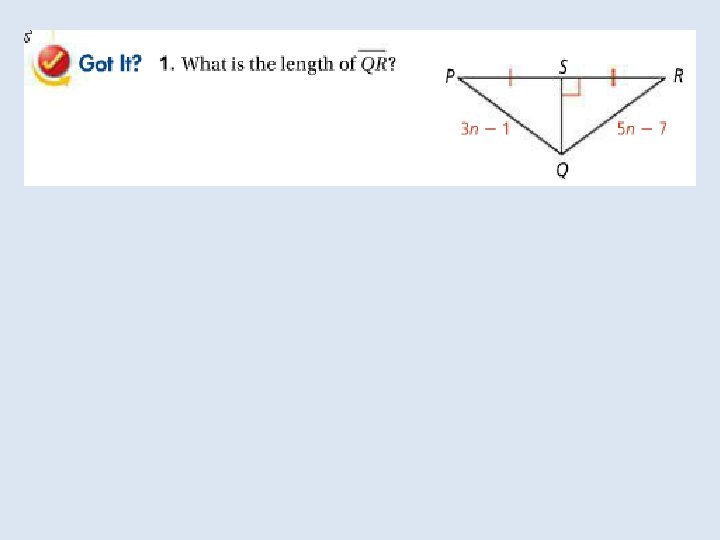
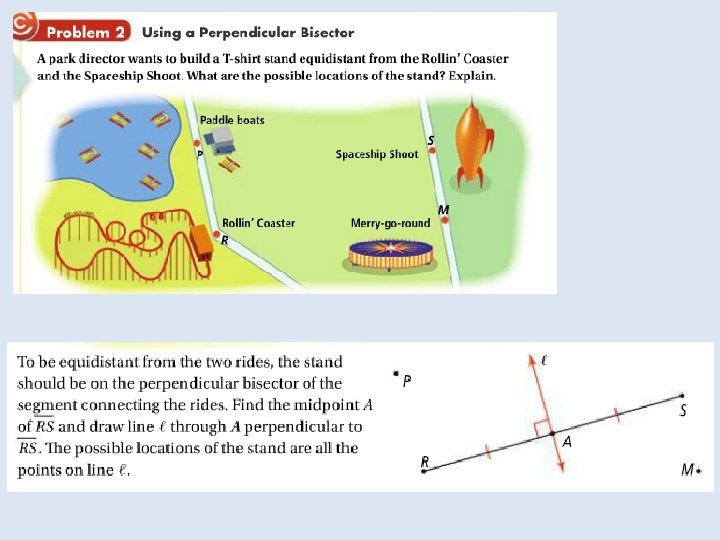
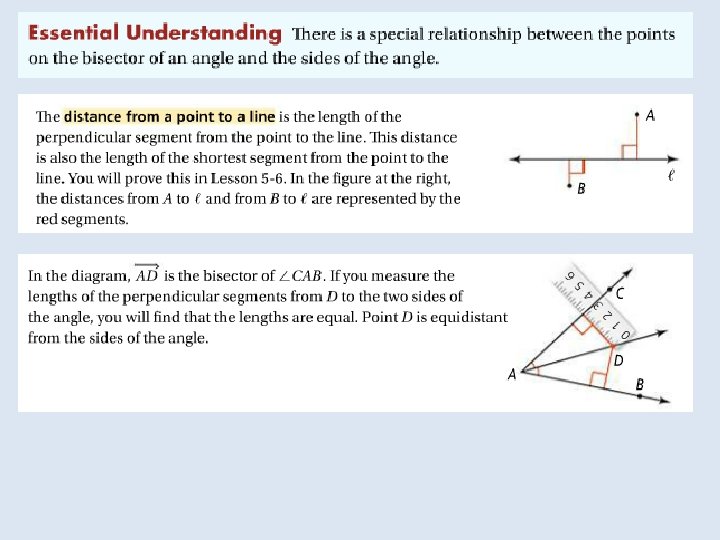
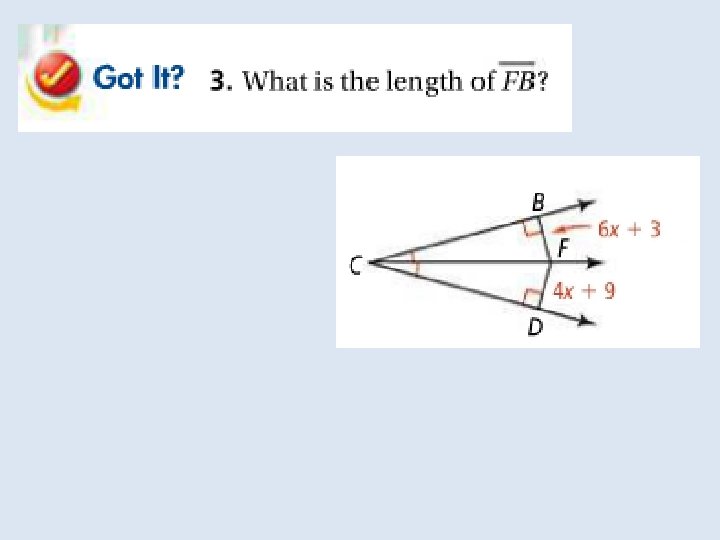
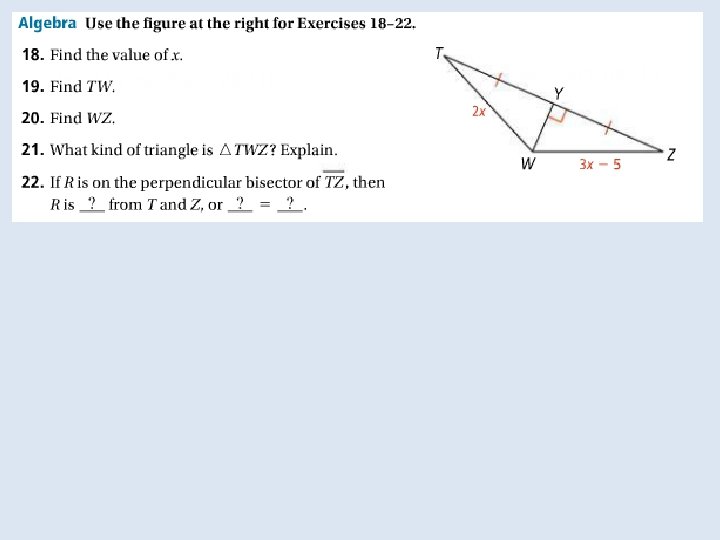
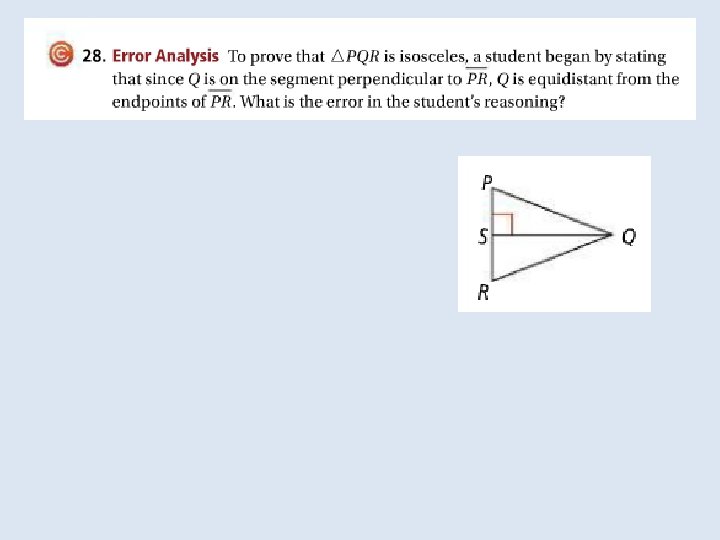
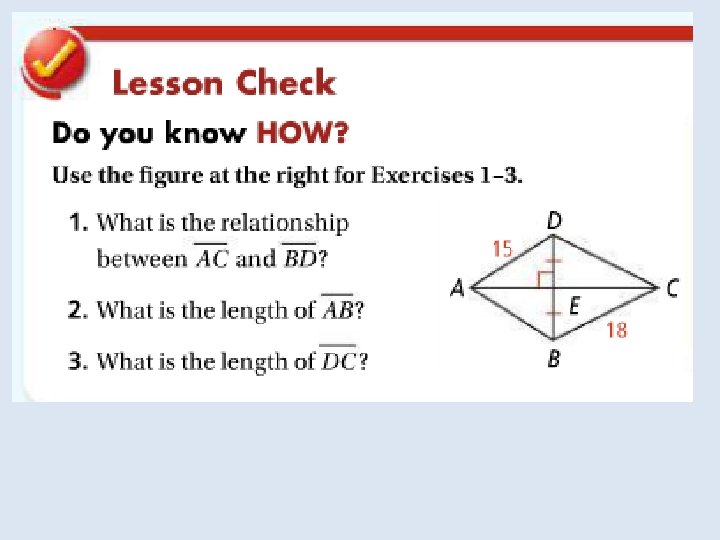
Objective: To use properties of bisectors Vocabulary: Equidistant Distance from a point to a line





















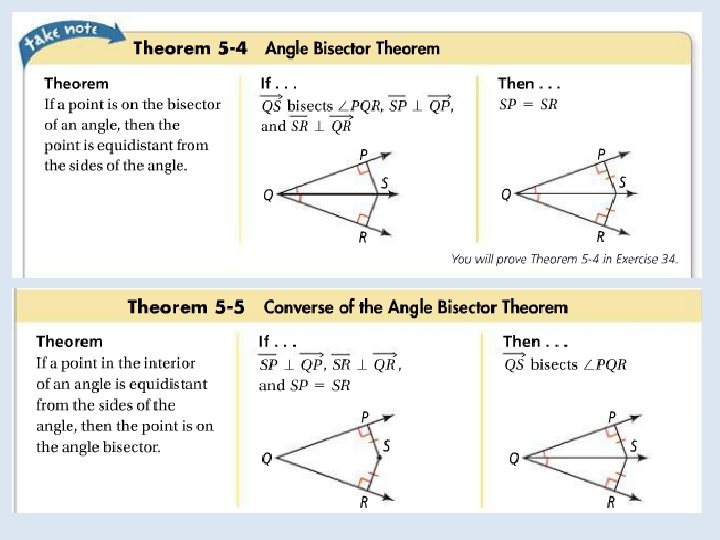
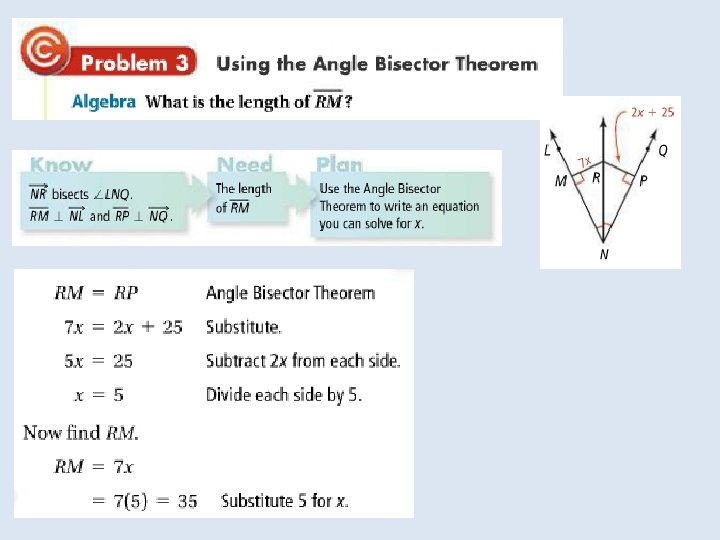
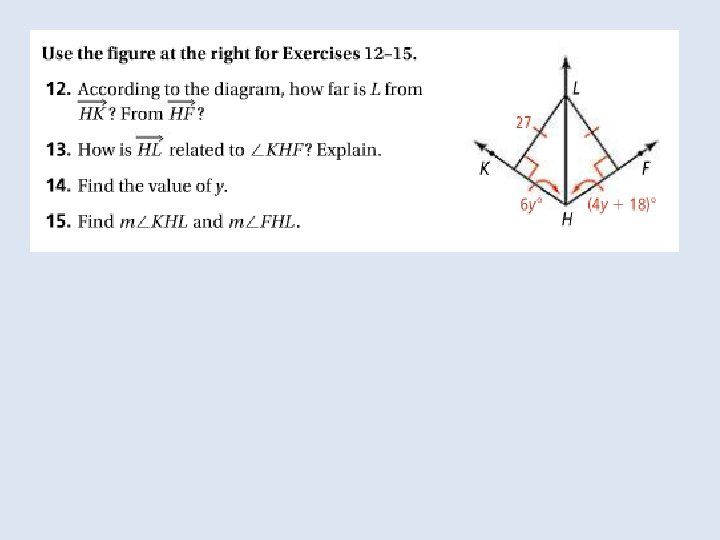
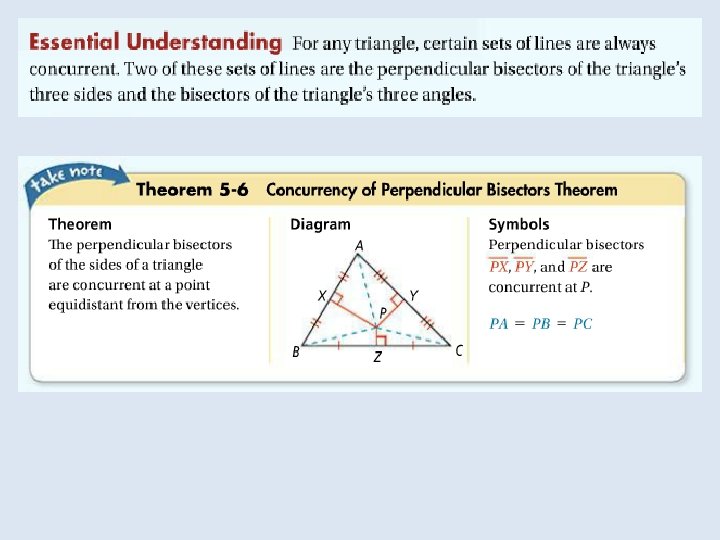
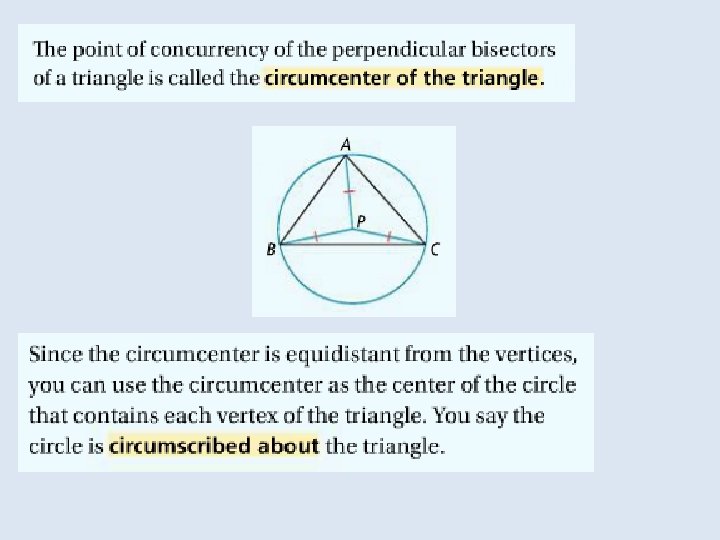
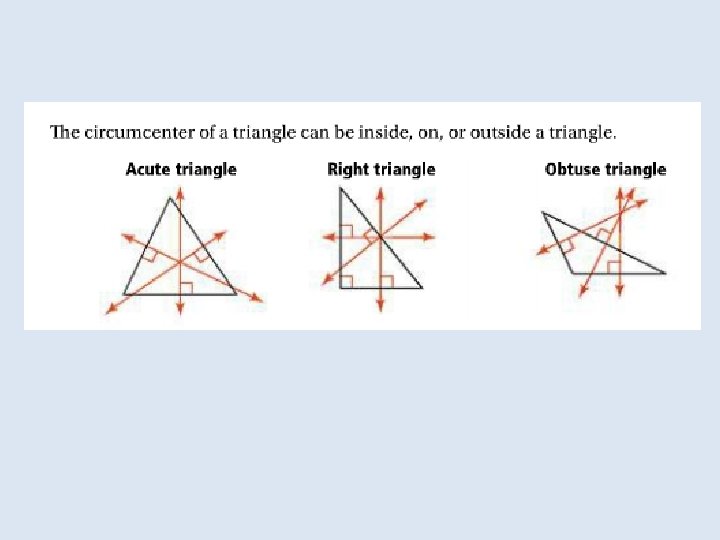
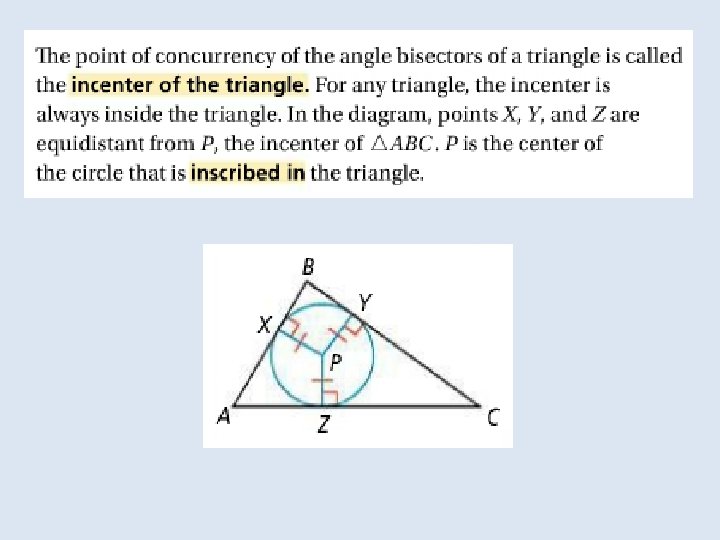
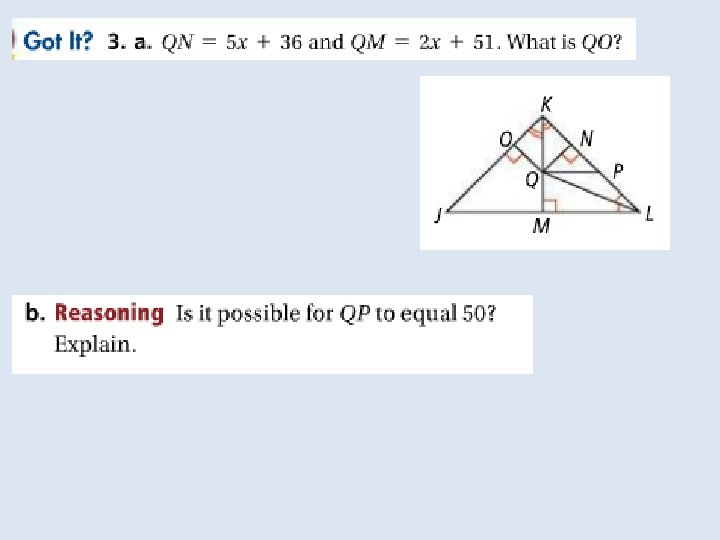
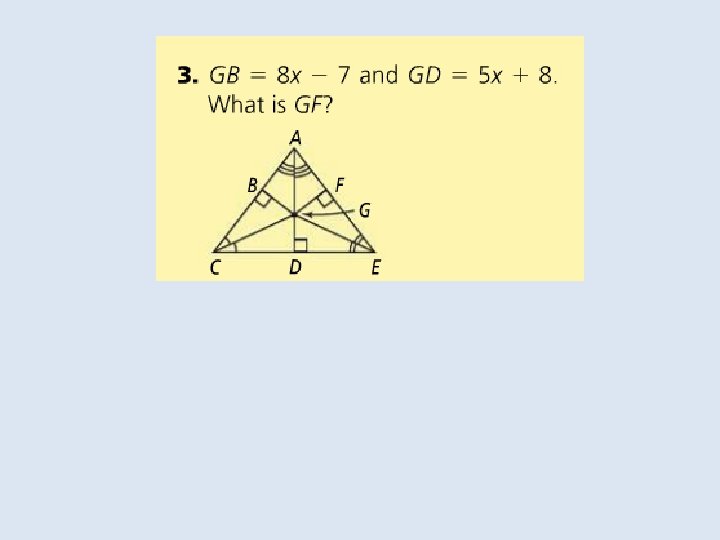
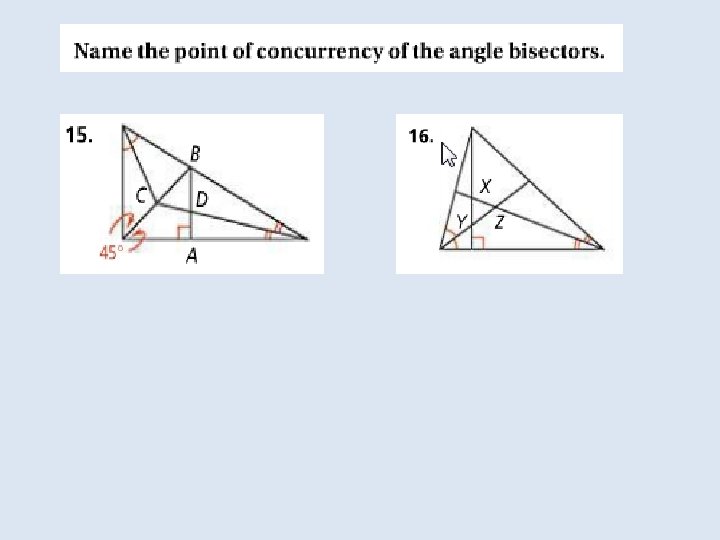
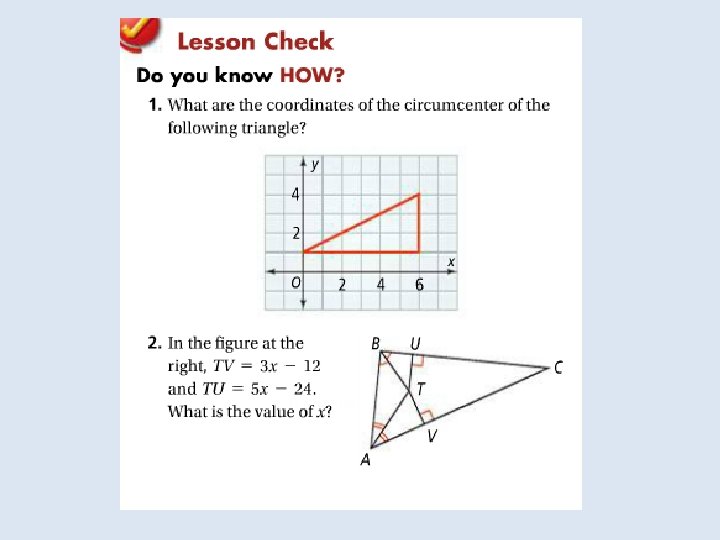
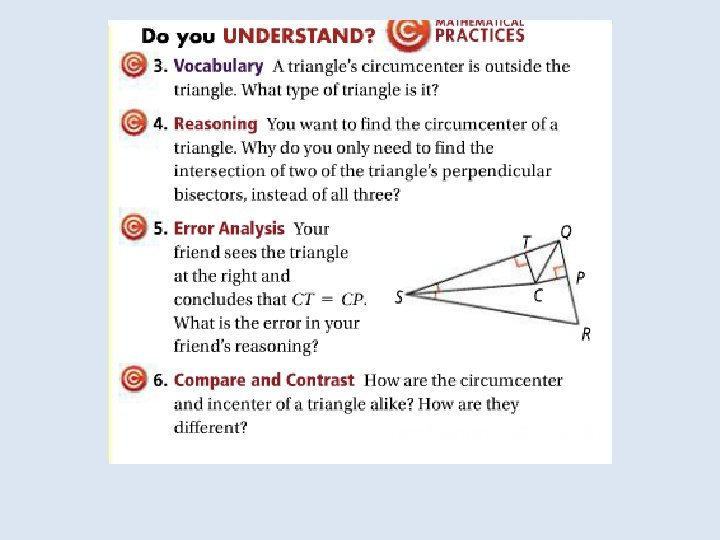
Objective: to identify properties of bisectors and angle bisectors Vocabulary: • Concurrent • Point of concurrency • Circumcenter of a triangle • Circumscribed about • Incenter of a triangle • Inscribed in






















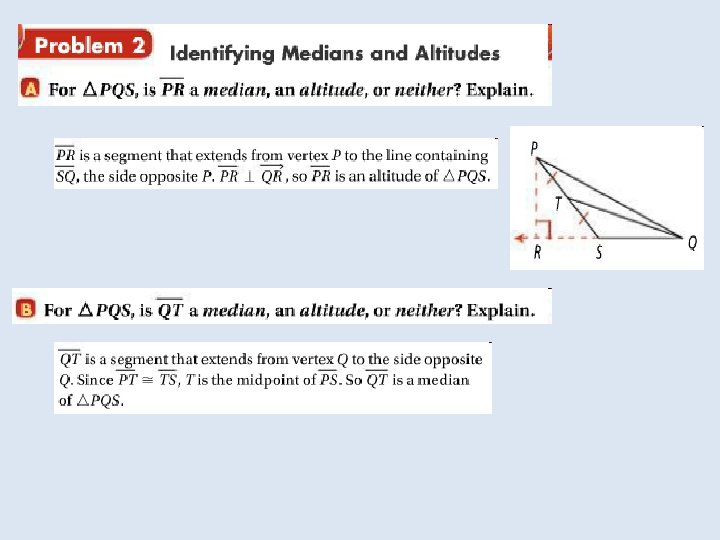
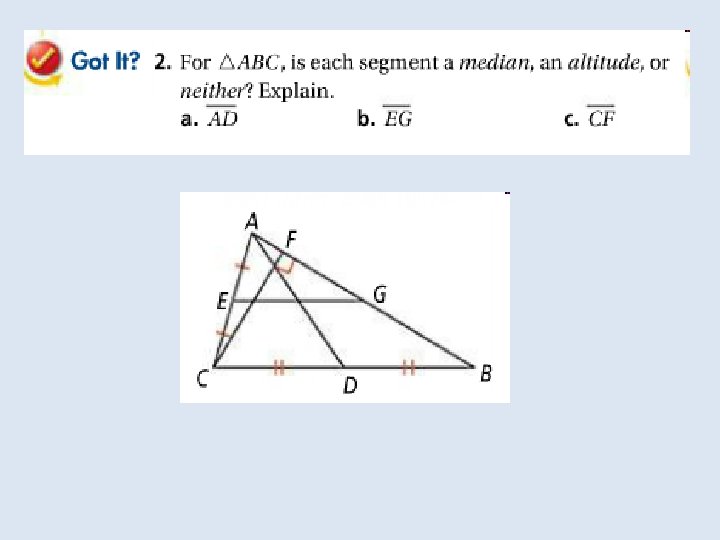
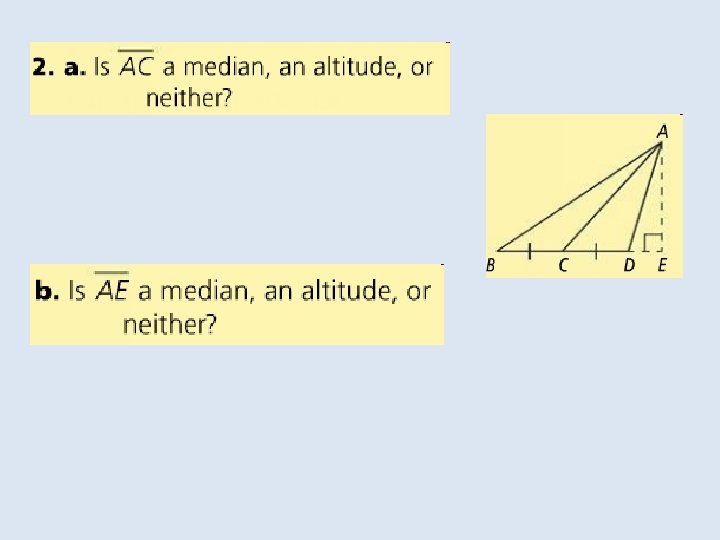
Objective: To identify properties of medians and altitudes of a triangle Vocabulary: Median of a triangle Centroid of a triangle Altitude of a triangle Orthocenter of a triangle

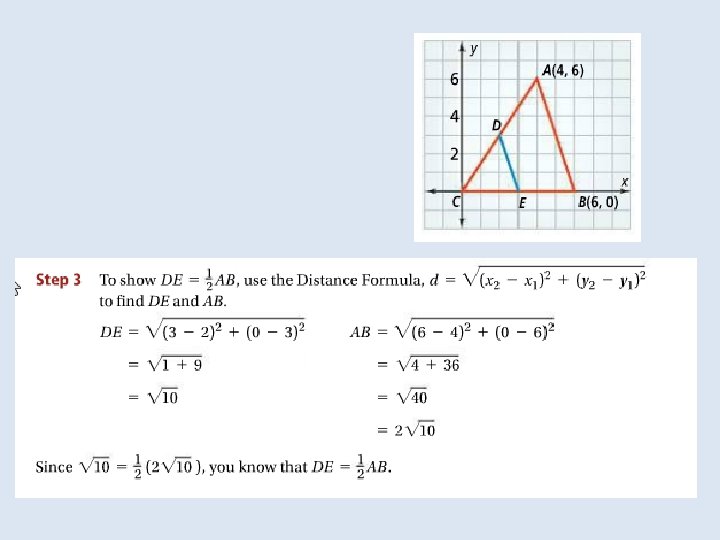
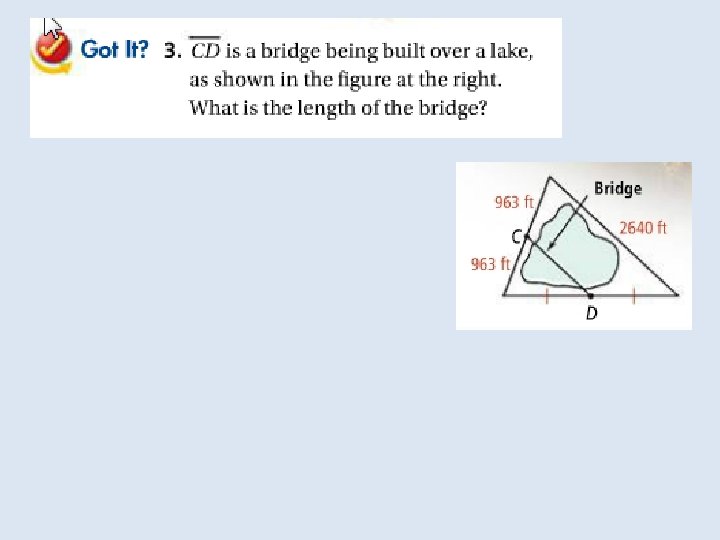
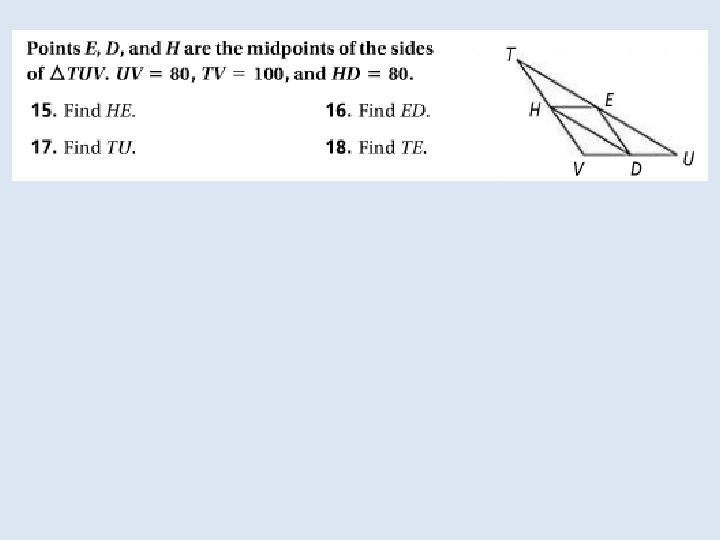
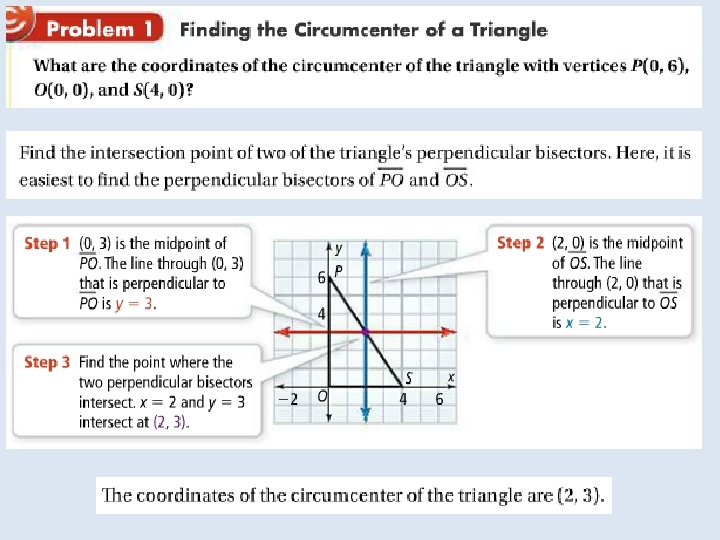
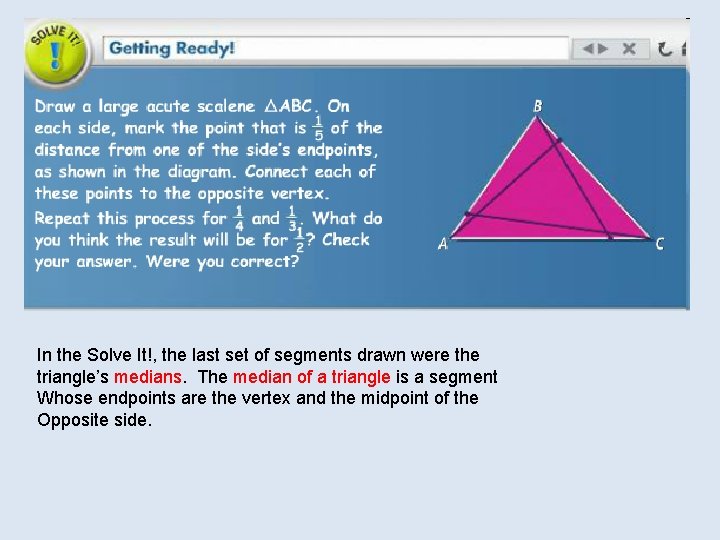
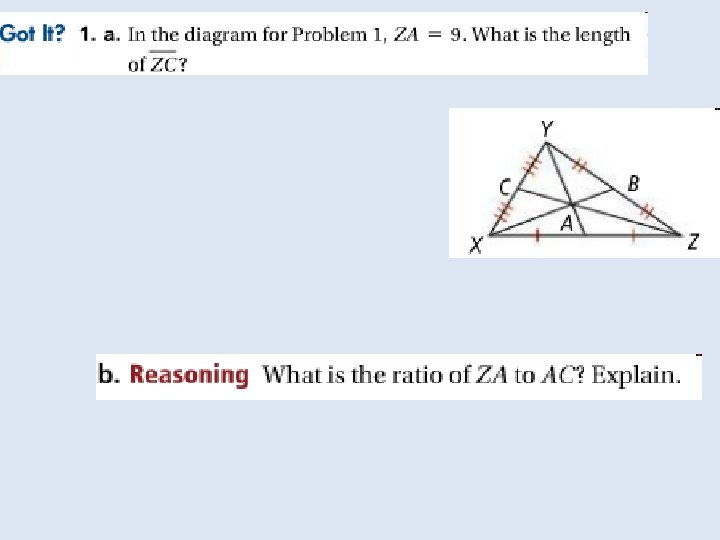
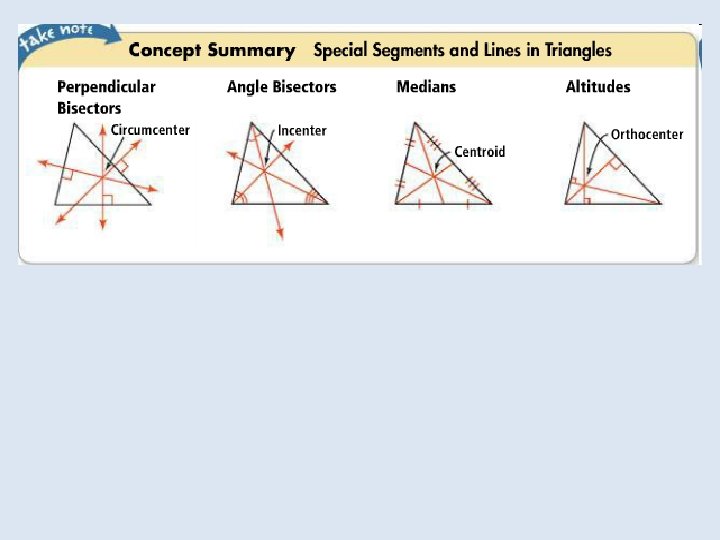
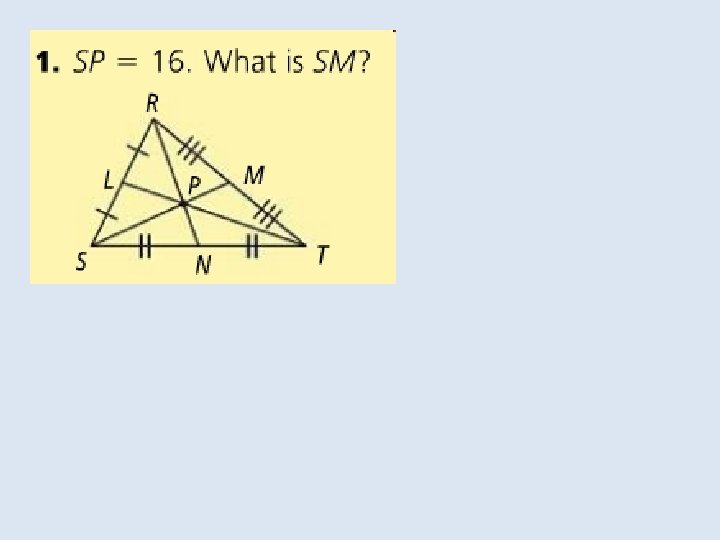
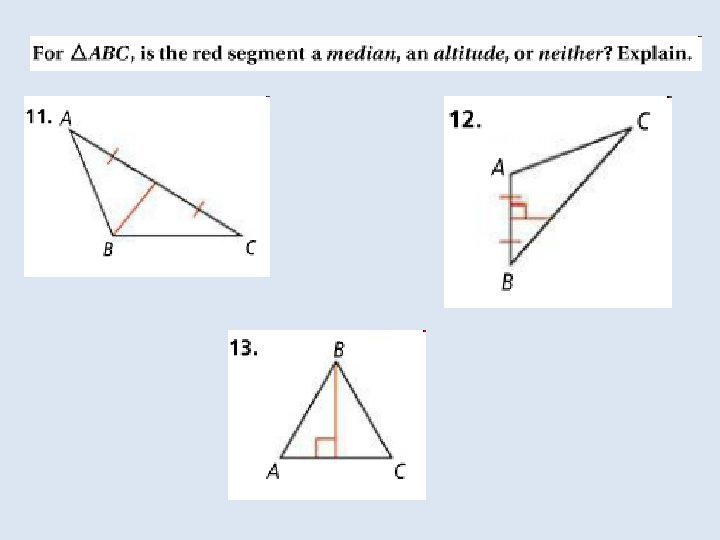
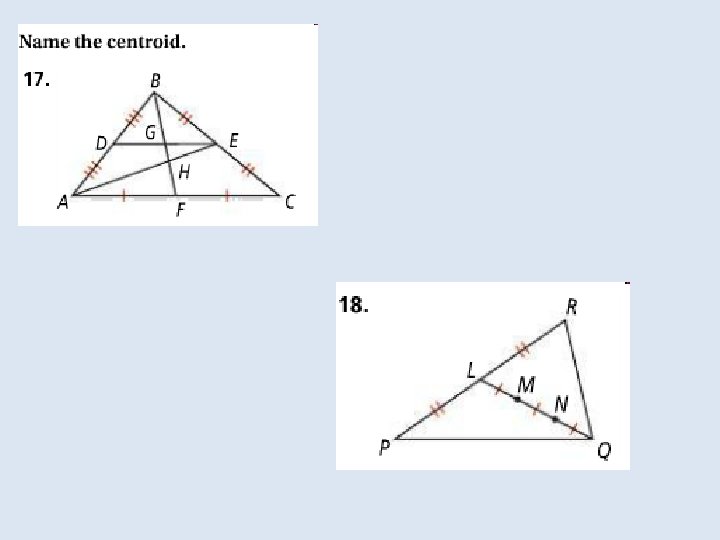
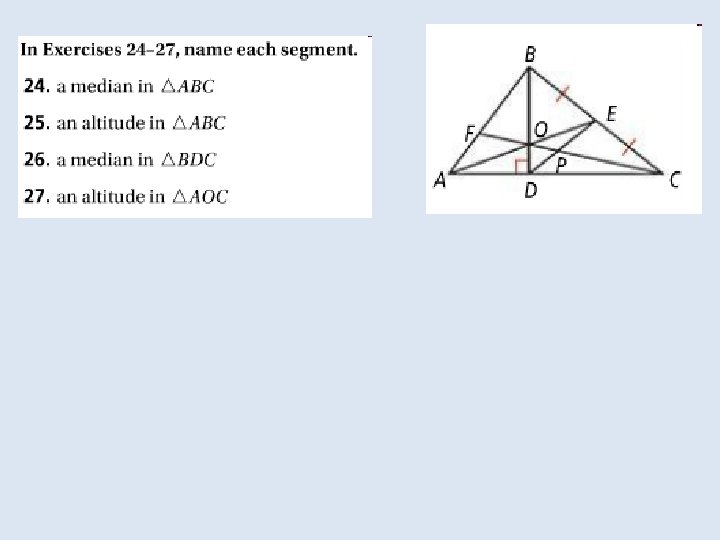
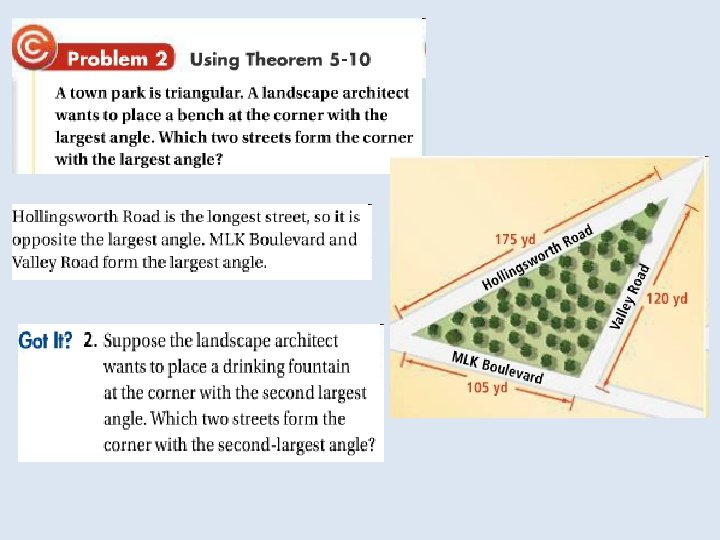
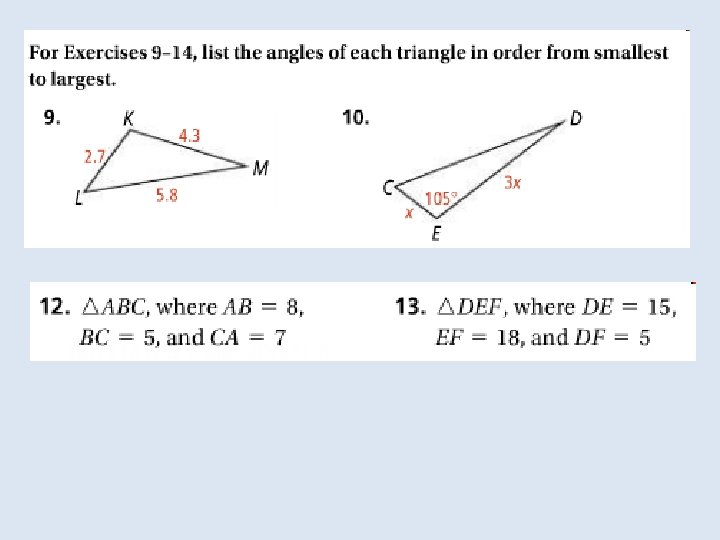
In the Solve It!, the last set of segments drawn were the triangle’s medians. The median of a triangle is a segment Whose endpoints are the vertex and the midpoint of the Opposite side.

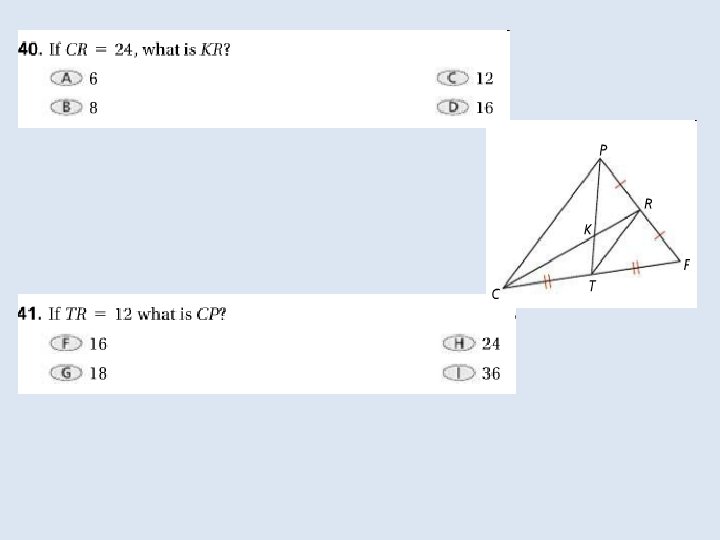
In a triangle the point of concurrency of the medians is the centroid of the triangle. The point is also called the center of gravity of a triangle because it is a point where the triangular shape will balance. For any triangle the centroid is always inside the triangle.



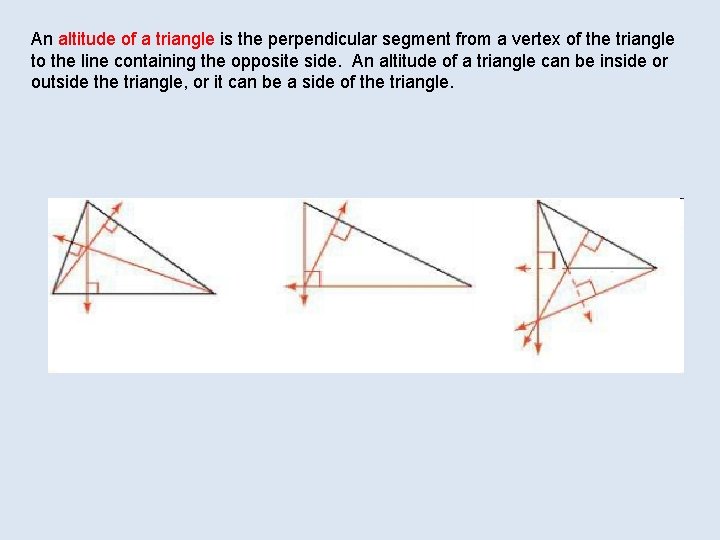
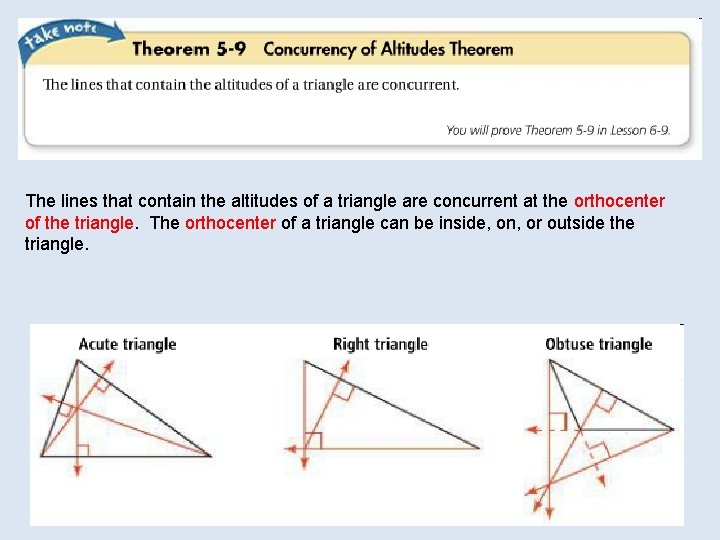
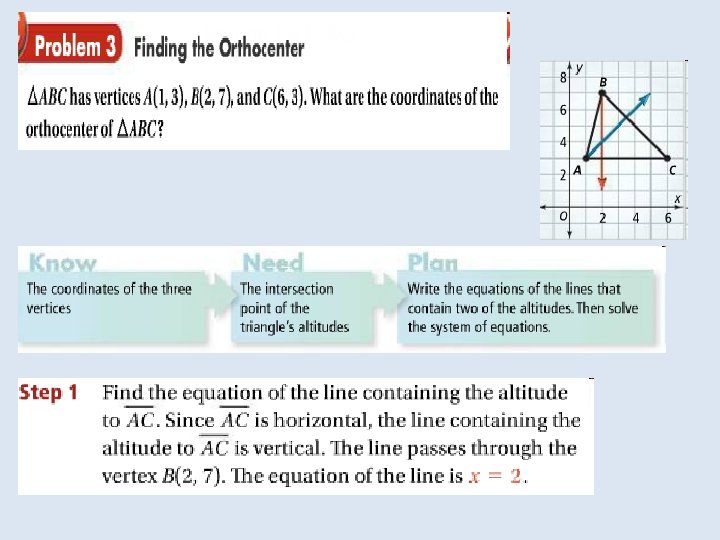
An altitude of a triangle is the perpendicular segment from a vertex of the triangle to the line containing the opposite side. An altitude of a triangle can be inside or outside the triangle, or it can be a side of the triangle.



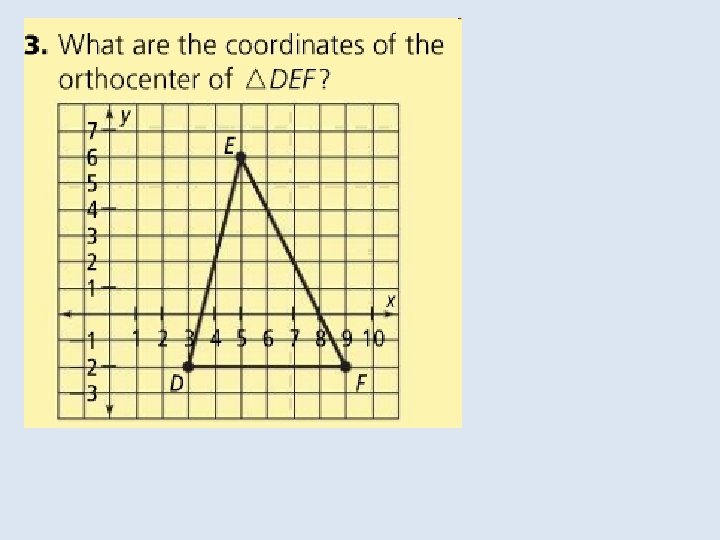
The lines that contain the altitudes of a triangle are concurrent at the orthocenter of the triangle. The orthocenter of a triangle can be inside, on, or outside the triangle.












Objective: To use indirect reasoning to write proofs Vocabulary: Indirect reasoning Indirect proof

In the Solve It!, you can conclude that a square must contain a certain number if you can eliminate the other three numbers as possibilities. In indirect reasoning, all possibilities are considered and then all but one are proved false.

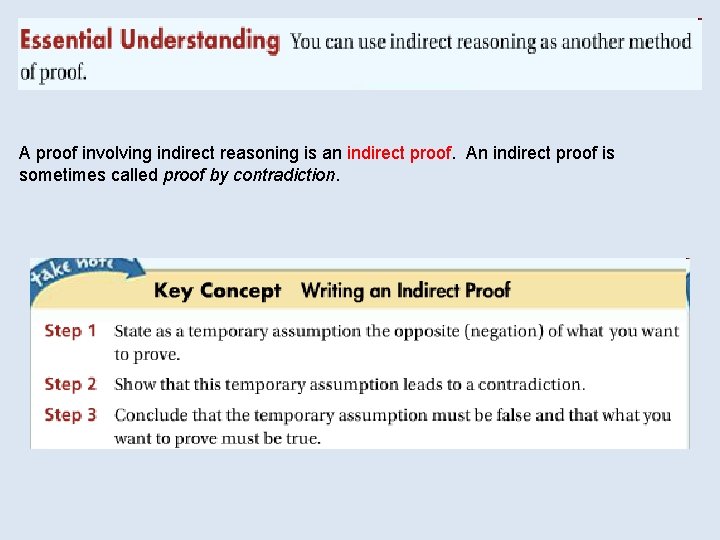
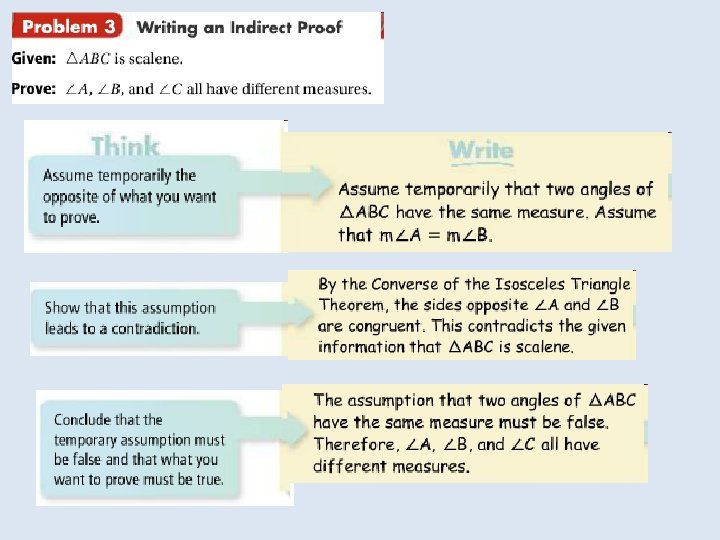
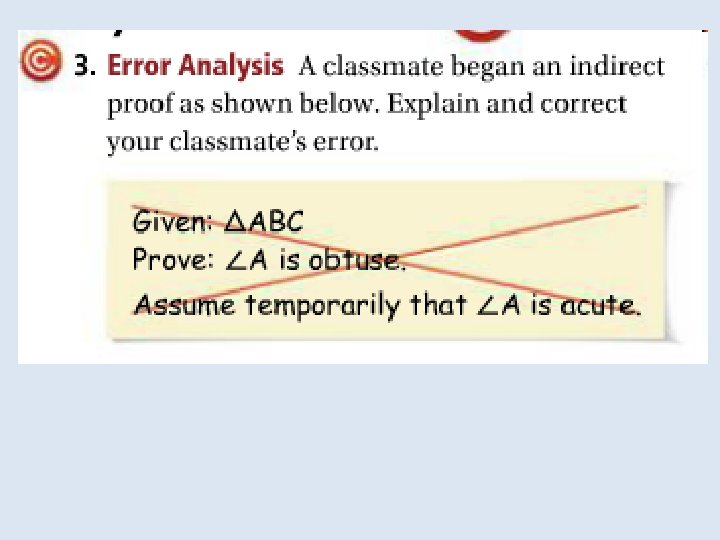
A proof involving indirect reasoning is an indirect proof. An indirect proof is sometimes called proof by contradiction.

In the first step of an indirect proof you assume as true the opposite of what you want to prove.


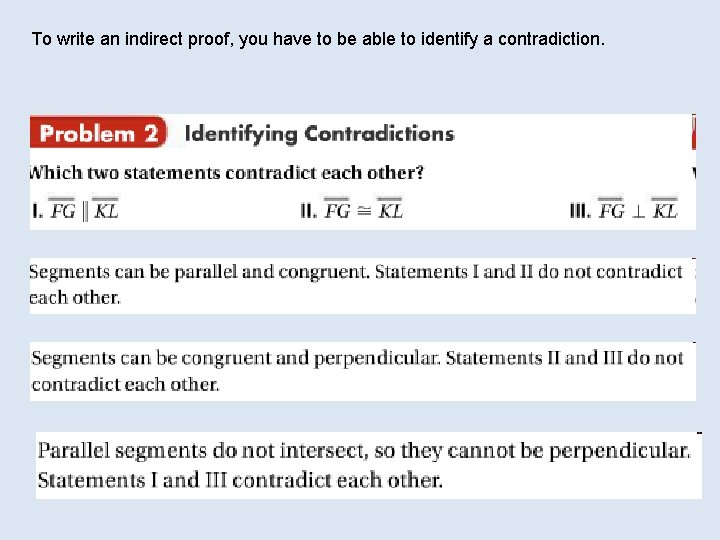
To write an indirect proof, you have to be able to identify a contradiction.











Objective: To use inequalities involving angles and sides of triangles Vocabulary: No new vocabulary

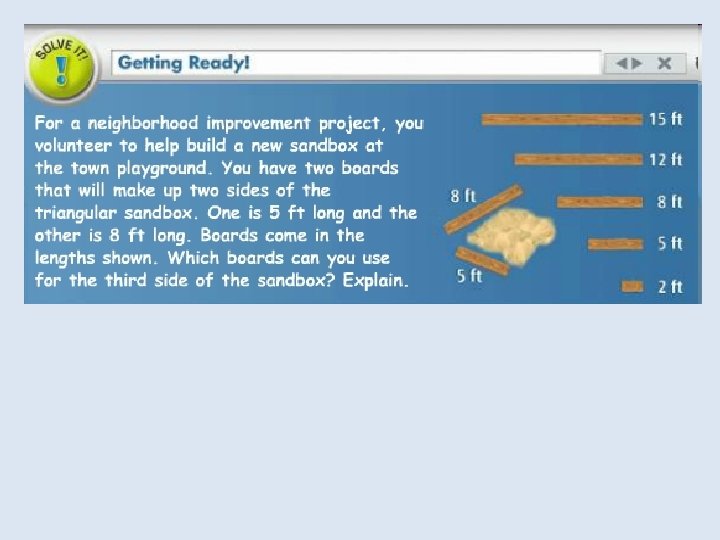
In the Solve It!, you explored triangles formed by various lengths of board. You may have noticed that changing the angle formed by the two sides of the sandbox changes the length of the third side.


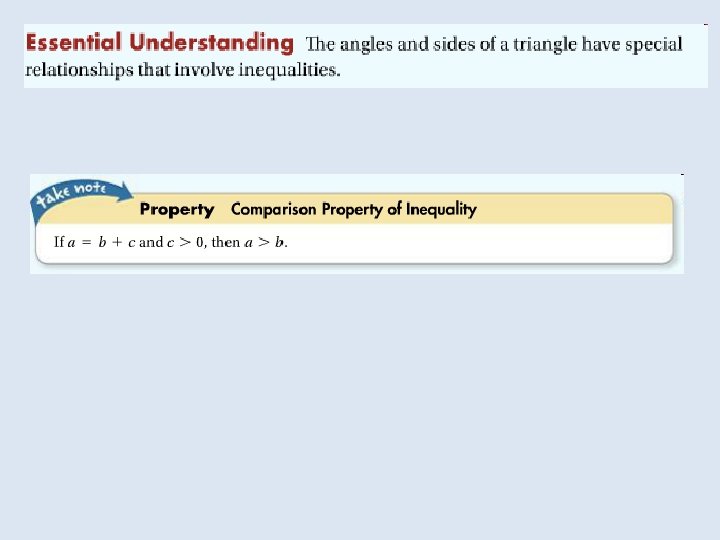
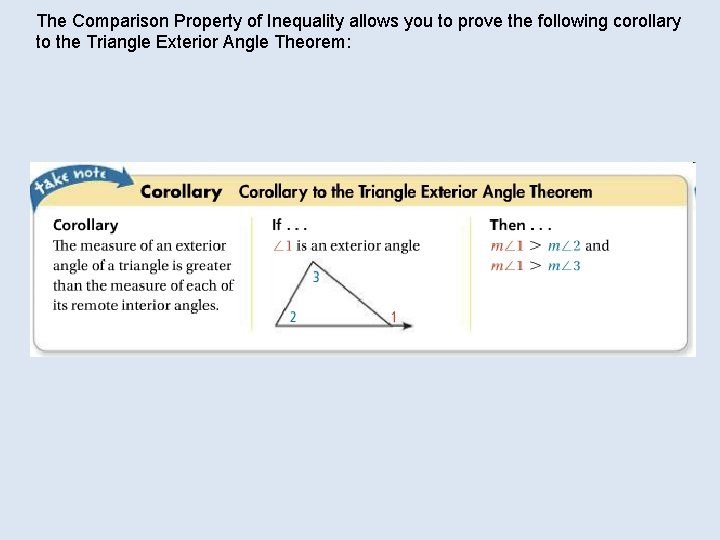
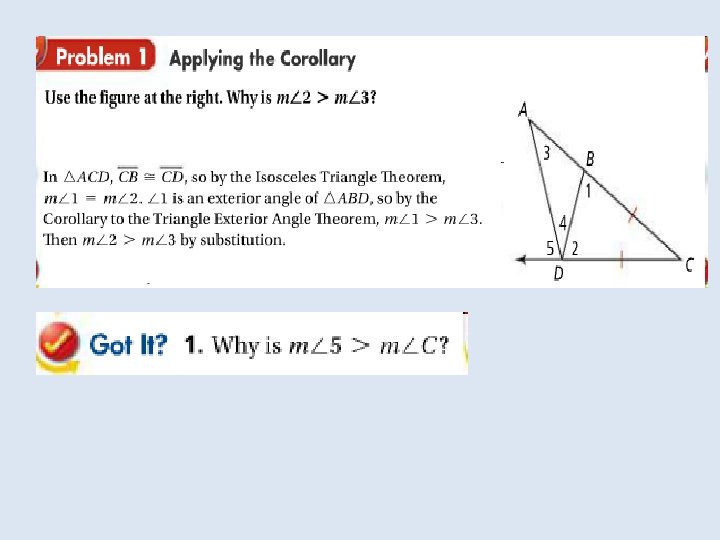
The Comparison Property of Inequality allows you to prove the following corollary to the Triangle Exterior Angle Theorem:






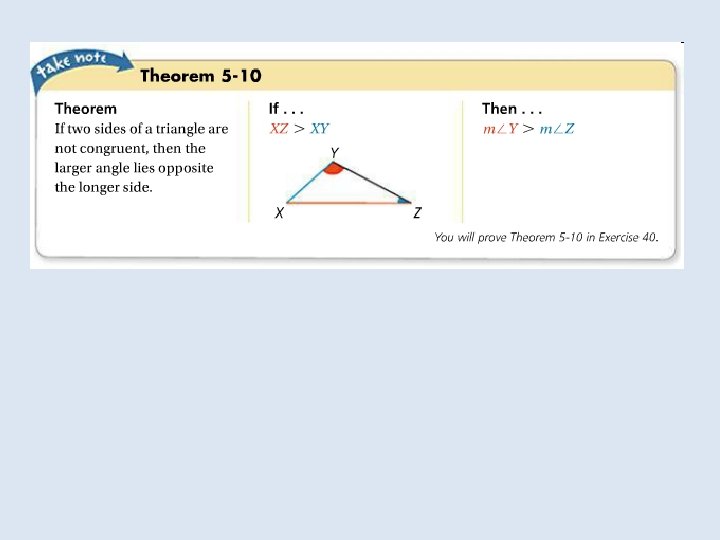
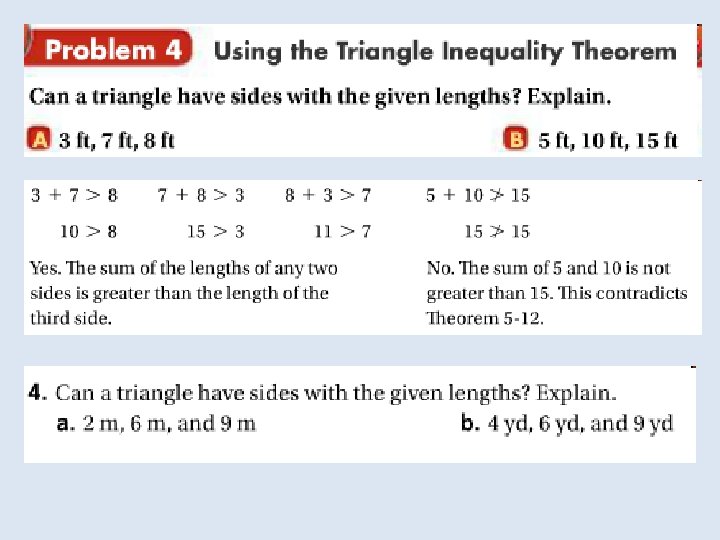
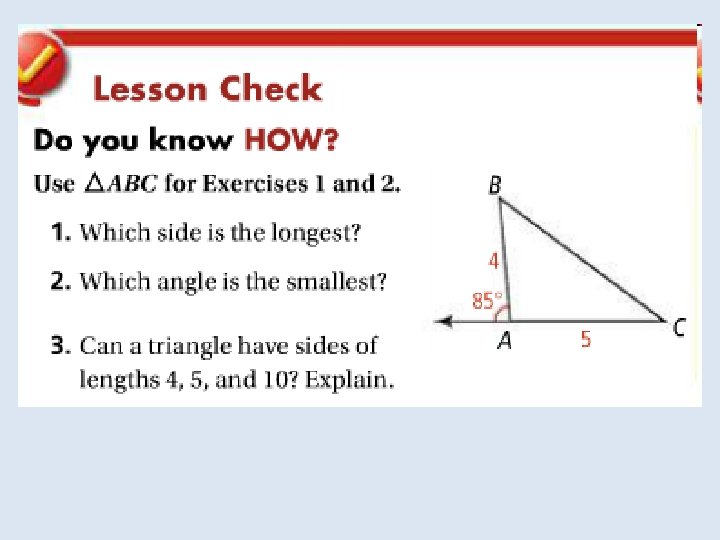
For three segments to form a triangle, their lengths must be related in a certain way. Notice that only one of the sets of segments below can form a triangle. The sum of the smallest two lengths must be greater than the greatest length.









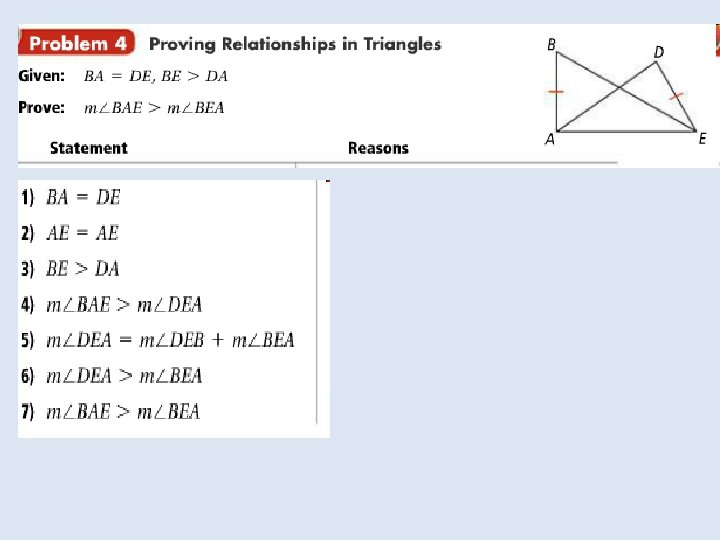
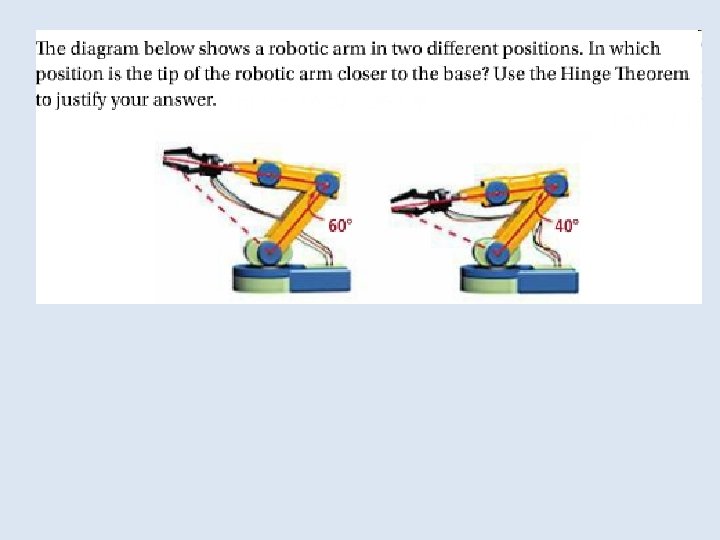
Objective: To apply inequalities in two triangles Vocabulary: No new vocabulary

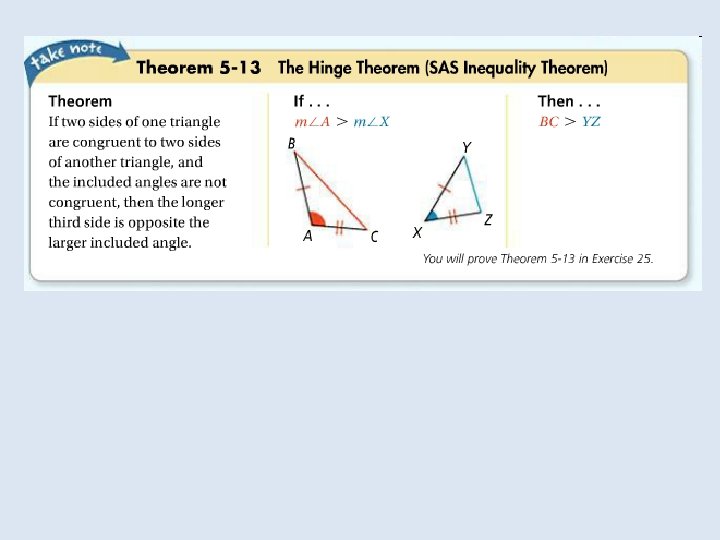
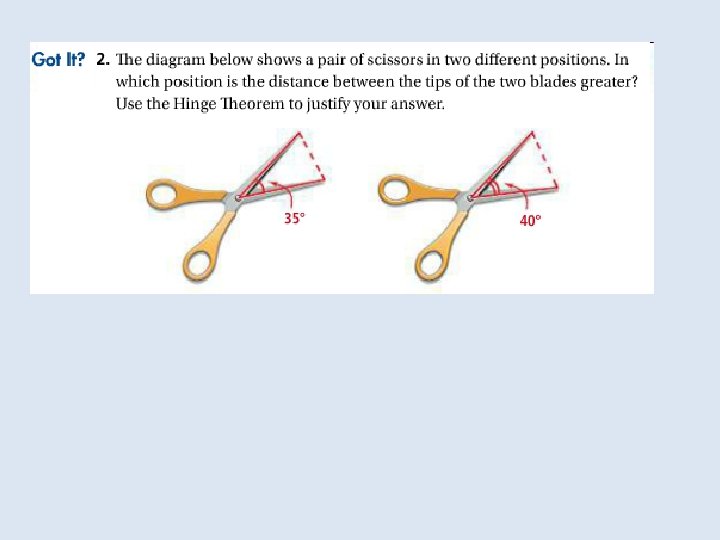
In the Solve It!, the hands of the clock and the segment labeled x form a triangle. As the time changes, the shape of the triangle changes, but the lengths of two of its sides do not change.







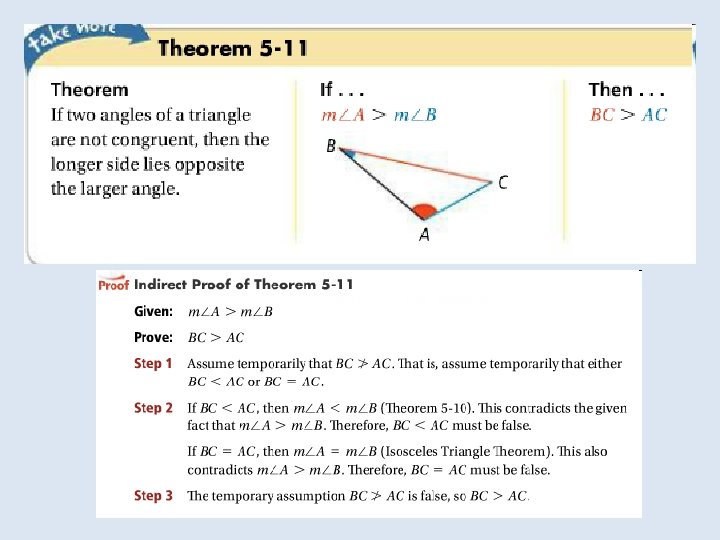
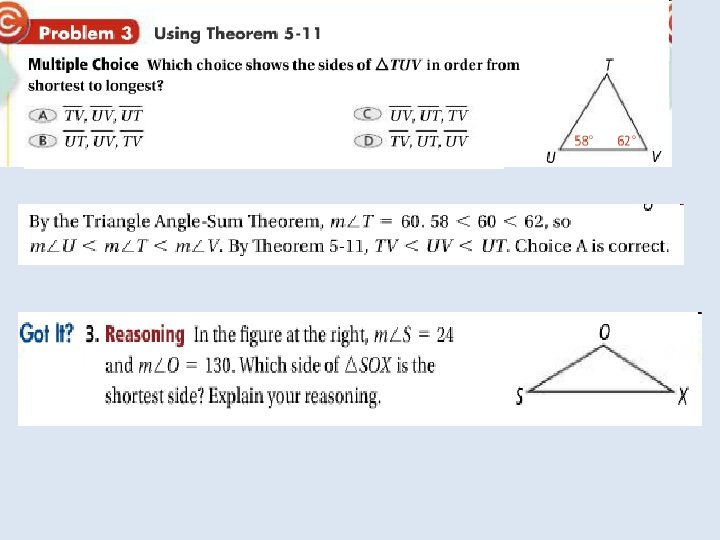
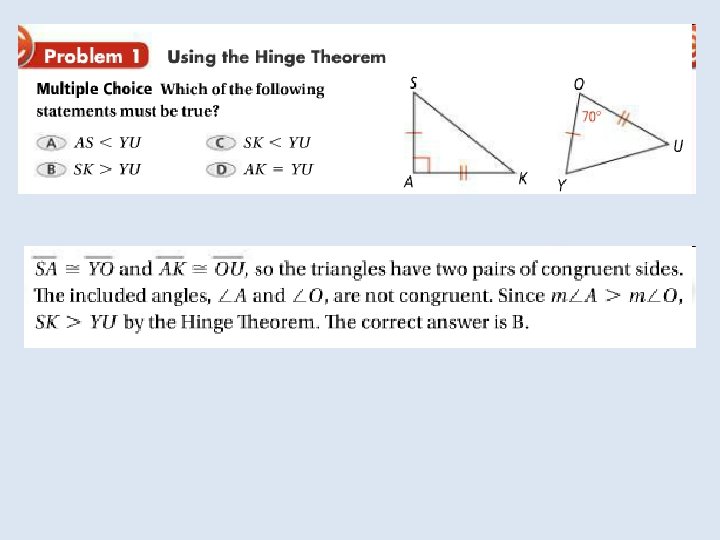
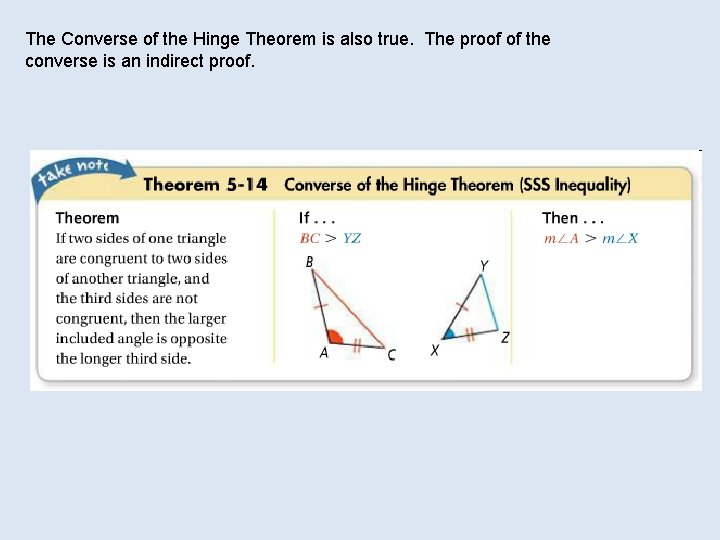
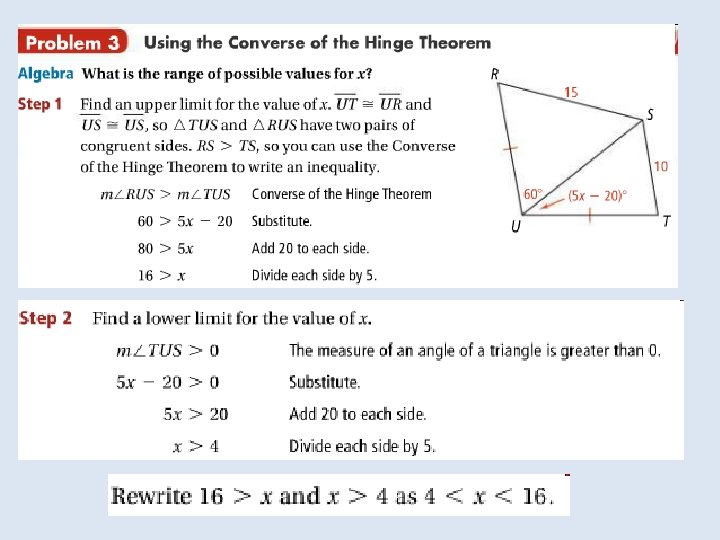
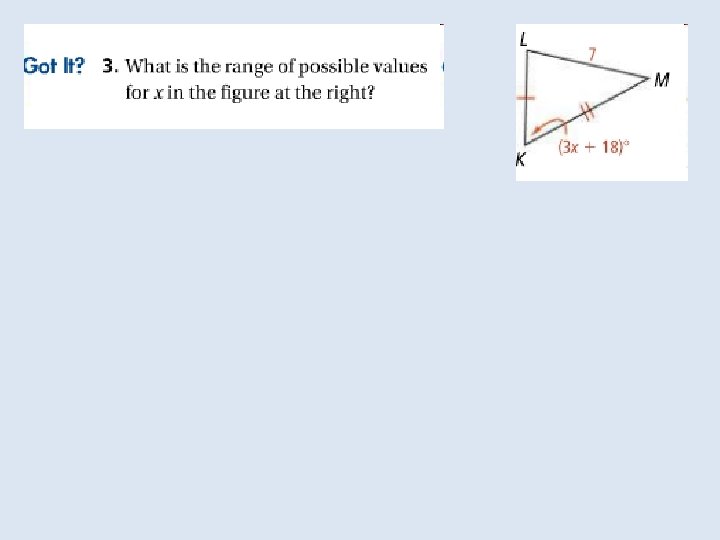
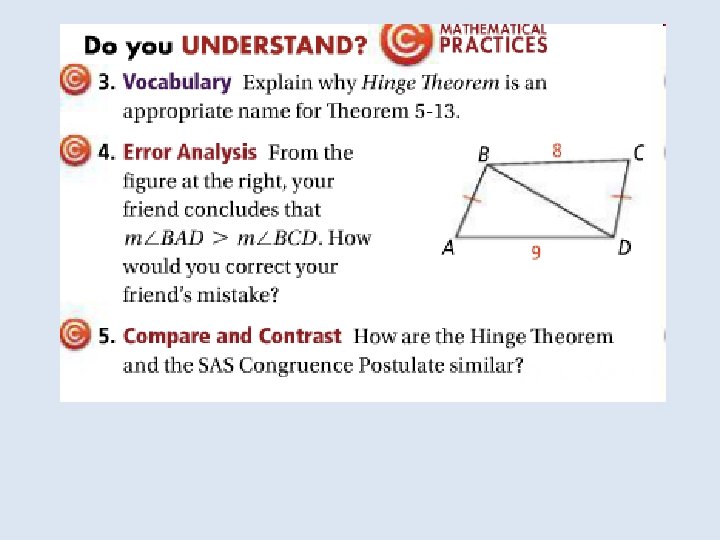
The Converse of the Hinge Theorem is also true. The proof of the converse is an indirect proof.










 Relationships within triangles
Relationships within triangles Chapter 5 relationships within triangles
Chapter 5 relationships within triangles Relationships within triangles
Relationships within triangles Module 11 angle relationships in parallel
Module 11 angle relationships in parallel Unit 5 homework 1 triangle midsegments
Unit 5 homework 1 triangle midsegments 4-3 angle relationships in triangles
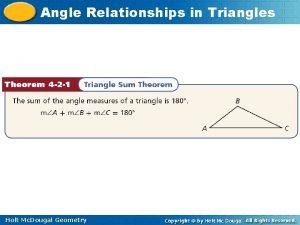
4-3 angle relationships in triangles Lesson 4-2 problem solving angle relationships in triangles
Lesson 4-2 problem solving angle relationships in triangles Angle relationships in triangles
Angle relationships in triangles Congruent triangles isosceles and equilateral triangles
Congruent triangles isosceles and equilateral triangles Chapter 7 lesson 1 healthy family relationships
Chapter 7 lesson 1 healthy family relationships Chapter 8 peer relationships answer key
Chapter 8 peer relationships answer key Chapter 7 family relationships
Chapter 7 family relationships Chapter 2 section 1 organisms and their relationships
Chapter 2 section 1 organisms and their relationships Chapter 2 section 1 organisms and their relationships
Chapter 2 section 1 organisms and their relationships Principles of ecology section 2.1 worksheet answers
Principles of ecology section 2.1 worksheet answers Chapter 2 principles of ecology answer key
Chapter 2 principles of ecology answer key Friendly relationship chapter 8
Friendly relationship chapter 8 Chapter 6 skills for healthy relationships
Chapter 6 skills for healthy relationships Chapter 5 family relationships answers
Chapter 5 family relationships answers Chapter 18 healthy family and peer relationships
Chapter 18 healthy family and peer relationships Geometry lesson 1-5 angle relationships answers
Geometry lesson 1-5 angle relationships answers Chapter 7 family relationships
Chapter 7 family relationships Jokes about communication
Jokes about communication The three r's for stopping domestic violence
The three r's for stopping domestic violence Describing scatterplots
Describing scatterplots Chapter 6 skills for healthy relationships
Chapter 6 skills for healthy relationships Acquaintance vs friend
Acquaintance vs friend Chapter 2 section 1 organisms and their relationships
Chapter 2 section 1 organisms and their relationships Segment fg is the angle bisector of
Segment fg is the angle bisector of  Chapter 7 right triangles and trigonometry answer key
Chapter 7 right triangles and trigonometry answer key Chapter 9 right triangles and trigonometry
Chapter 9 right triangles and trigonometry Chapter 8 right triangles and trigonometry answer key
Chapter 8 right triangles and trigonometry answer key 4-4 using congruent triangles cpctc
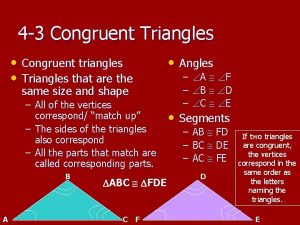
4-4 using congruent triangles cpctc Chapter 4 congruent triangles
Chapter 4 congruent triangles Congruent figures 4-1
Congruent figures 4-1 Within word pattern
Within word pattern Between group design adalah
Between group design adalah Describes how a software communicates within itself.
Describes how a software communicates within itself. Expericorr design
Expericorr design Words with the stem pugn
Words with the stem pugn Word within the word stems
Word within the word stems Word within the word list 21
Word within the word list 21 A consistent, orderly or pleasing arrangement of parts
A consistent, orderly or pleasing arrangement of parts Group of lines in a poem
Group of lines in a poem What is the first rule in social correspondence
What is the first rule in social correspondence What is shapping
What is shapping Category 5 interdependence within environmental systems
Category 5 interdependence within environmental systems Category 5 interdependence within environmental systems
Category 5 interdependence within environmental systems Between within design
Between within design Between and within subjects
Between and within subjects Unpacking and storing guest luggage
Unpacking and storing guest luggage What are the two focal points of a fingerprint
What are the two focal points of a fingerprint Market form of meat
Market form of meat A meaningful, authentic good life is based on
A meaningful, authentic good life is based on Communication with oneself
Communication with oneself Interdependence within environmental systems
Interdependence within environmental systems Within group variance vs between group
Within group variance vs between group Interactions within ecosystems grade 7
Interactions within ecosystems grade 7 Film positions descriptions
Film positions descriptions Factorial designs
Factorial designs Between measures design
Between measures design Dependent variable
Dependent variable Between subjects factorial design
Between subjects factorial design Mixed factorial design
Mixed factorial design How is energy transferred within the water cycle
How is energy transferred within the water cycle During for difference
During for difference Between subjects vs within subjects
Between subjects vs within subjects Large whirl of water within an ocean basin
Large whirl of water within an ocean basin Effect size for t test
Effect size for t test Labouvie-vief pragmatic thought
Labouvie-vief pragmatic thought Nesting of member function in c++
Nesting of member function in c++ Multiple authors apa format
Multiple authors apa format Circle theorems a level
Circle theorems a level Multirule
Multirule Within subjects design
Within subjects design Str
Str Shaping across response topographies
Shaping across response topographies Developing the leader within you summary
Developing the leader within you summary Angles within a circle
Angles within a circle 2x3 within subjects design
2x3 within subjects design Anova within group and between group
Anova within group and between group Word within the word list 9
Word within the word list 9 Pearson uncertainty map
Pearson uncertainty map What is the main function of xylem within a plant brainpop
What is the main function of xylem within a plant brainpop Management is responsible for unguarded moving parts
Management is responsible for unguarded moving parts Is a cornerstone in the protection of information assets
Is a cornerstone in the protection of information assets Contribution margin
Contribution margin Crazys 3
Crazys 3 Implicatures within legal language:
Implicatures within legal language: Frog heart
Frog heart It brings together a composition with similar units
It brings together a composition with similar units What is a pyramid of biomass
What is a pyramid of biomass When you group subcategories within broader concepts
When you group subcategories within broader concepts Within clementini
Within clementini Process indicators enable a software project manager to
Process indicators enable a software project manager to 90 of the canadian population lives within
90 of the canadian population lives within Awaken the force within
Awaken the force within Live out thy life within me hymn
Live out thy life within me hymn Incomplete counterbalancing
Incomplete counterbalancing Multoor.
Multoor. Revolution within band
Revolution within band Taming the tiger within
Taming the tiger within What is markov analysis
What is markov analysis Design is achieving goals within constraints
Design is achieving goals within constraints Psiformis
Psiformis Thinking, language and intelligence psychology summary
Thinking, language and intelligence psychology summary A code surrounded within italicized, or slanted, brackets
A code surrounded within italicized, or slanted, brackets Key issue 2: where do people migrate within a country?
Key issue 2: where do people migrate within a country? Problem 3-11 describing business transactions
Problem 3-11 describing business transactions Within the inis baeg, marital sexual relations:
Within the inis baeg, marital sexual relations: Partial quotes ap style
Partial quotes ap style 신일녀 스캇
신일녀 스캇 Electric forces within an atomic nucleus tend to
Electric forces within an atomic nucleus tend to Within subject design definition
Within subject design definition What is meant by physical diversity
What is meant by physical diversity Reaching out from within
Reaching out from within A prestige grading position or rank within a group
A prestige grading position or rank within a group Levels of organization within an ecosystem
Levels of organization within an ecosystem 250 words
250 words You may not park within ____ of a crosswalk.
You may not park within ____ of a crosswalk. Pursuit tackling drills
Pursuit tackling drills The smallest living unit within the human body is
The smallest living unit within the human body is Bless the lord oh my soul and all that is within me
Bless the lord oh my soul and all that is within me From michael, all the group arrived on time.
From michael, all the group arrived on time. Ablative of respect
Ablative of respect Cvit sia licence
Cvit sia licence Sororicide definition
Sororicide definition Word within the word list 8
Word within the word list 8 Captious in a sentence
Captious in a sentence Gozago
Gozago Within the meninges cerebrospinal fluid occupies the
Within the meninges cerebrospinal fluid occupies the Open your eyes look within
Open your eyes look within Design process outline
Design process outline Foresight is deeply rooted within intuitive mind
Foresight is deeply rooted within intuitive mind Within walking distance
Within walking distance Woman within maternity
Woman within maternity