Recent Developments in Inverse Scattering for Maxwells Equations



































- Slides: 75

Recent Developments in Inverse Scattering for Maxwell's Equations and Applications Gang Bao Interdisciplinary Center for Appl. & Comput. Math, Zhejiang U Michigan Center for Industrial & Applied Math, Michigan State U

Acknowledgements Collaborators: Peijun Li, Ying Li, Junshan Lin, Hai Zhang Faouzi Triki, Mickey S. Hou, Ki. Hyun Yun S-N Chow, H-M Zhou, J. Zou Research supported in parts by: NSF Appl. /Comput. Math. Programs NSF Theoretical Foundation Programs NSF Collaboration in Math. & Geo. Science Programs NSF Focused Research Group Programs Office of Naval Research Applied Analysis Programs

Outline Motivation and problem formulation Ill-posedness for inverse BVP Inverse scattering for Maxwell’s equations: multiple frequency data, convergence Inverse source problems Ongoing and future research


3 -D Inverse Medium Scattering Applications Non-destructive testing, material characterization and design Ultra sound tomography Seismic imaging Submarine detection Remote sensing Near-field and nano optics, diffraction limit, super-resolution, nano-technology

Inverse Boundary Value Problems: Challenges Nonlinear, ill-posedness, global versus local (initial guess) Incomplete or partial data Uncertainty, indistinguishability Large-scale computation: complexity, iterative, memory, CPU, choice of methods Stochastic versus deterministic

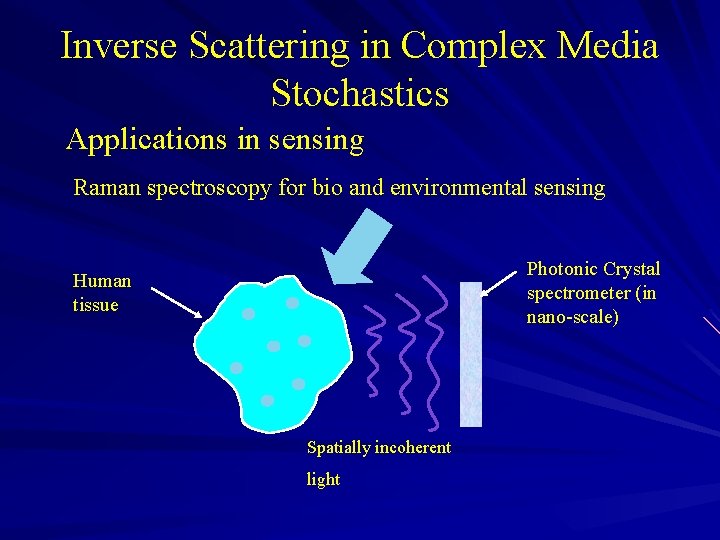
Inverse Scattering in Complex Media Stochastics Applications in sensing Raman spectroscopy for bio and environmental sensing Photonic Crystal spectrometer (in nano-scale) Human tissue Spatially incoherent light

Inverse Boundary Value Problems: theory The inverse conductivity problem: Calderon’s Problem: To determine the conductivity function from the boundary data (Dt. N or voltage to current map) Ref: On an inverse boundary value problem, in ``Seminar on Numerical Analysis and its Applications to Continuum Physics’’, pp. 65 -73, Soc. Brasilerira de Matematica, Rio de Haneiro, 1980 Calderon, Kohn-Vogelius, Uhlmann-Sylvester, Nachman, Isakov, Alessandrini, …. (Uniqueness and stability)…

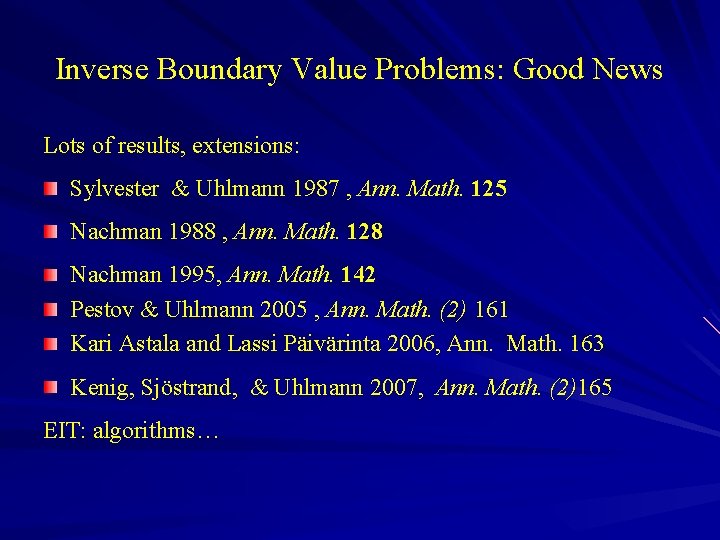
Inverse Boundary Value Problems: Good News Lots of results, extensions: Sylvester & Uhlmann 1987 , Ann. Math. 125 Nachman 1988 , Ann. Math. 128 Nachman 1995, Ann. Math. 142 Pestov & Uhlmann 2005 , Ann. Math. (2) 161 Kari Astala and Lassi Päivärinta 2006, Ann. Math. 163 Kenig, Sjöstrand, & Uhlmann 2007, Ann. Math. (2)165 EIT: algorithms…

Inverse Boundary Value Problems: Bad News Ill-posedness Severely (exponentially) ill-posed: Alessandrini’ 87, Mandache’ 01 More questions than answers! Various means to overcome the ill-posedness: Seo, Nachman, … What is wrong? ! More data…

Objective To develop a data driven reconstruction method for solving the inverse problems. Objective: Stable reconstruction methods Engineering practice: broad band data, frequency sweeping. Some hints from the corresponding hyperbolic inverse problems: B. & Yun (2008) (also Sun ’ 90, Alessandrini &Sylvester ’ 90)

Scattering Model: Maxwell’s Equations in Unbounded Domains Boundary conditions Regularity analysis Numerical solution Refs: Muller (1969) , Abboud and Nedelec (1992), Costabel (1991), Birman and Solomyak (1987), Leis (1986), Kirsch and Monk (1995, 1998) Lp estimates, B. , Minut, Zhou (CPDE, 2007) B. , Li, Zhou (JDE, 2008)

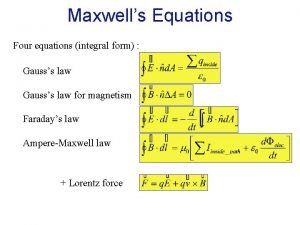
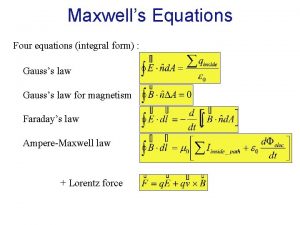
Scattering: Time Hramonic Maxwell’s Equations Where Ei is the incident field E is the scattered field

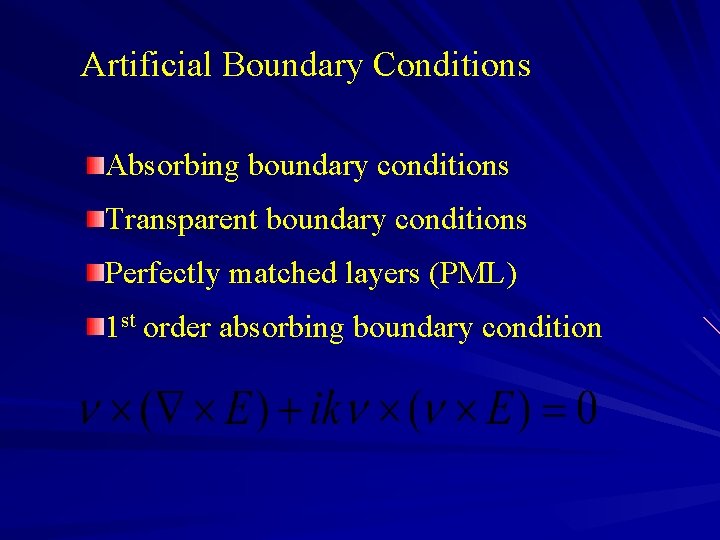
Artificial Boundary Conditions Absorbing boundary conditions Transparent boundary conditions Perfectly matched layers (PML) 1 st order absorbing boundary condition

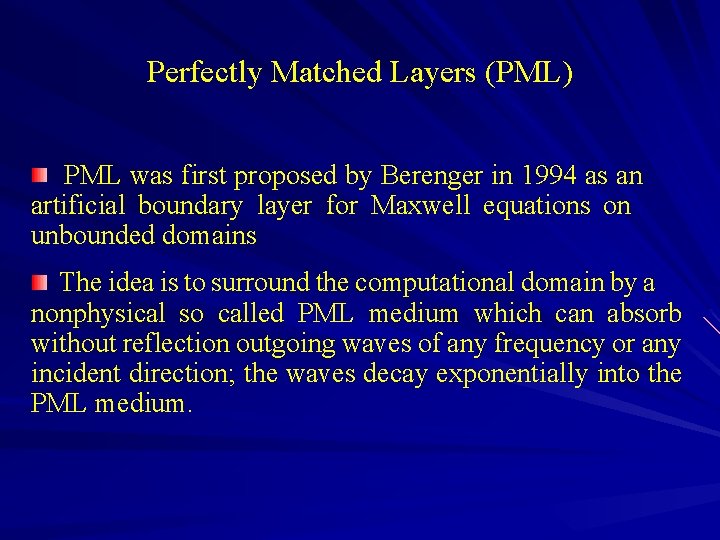
Perfectly Matched Layers (PML) PML was first proposed by Berenger in 1994 as an artificial boundary layer for Maxwell equations on unbounded domains The idea is to surround the computational domain by a nonphysical so called PML medium which can absorb without reflection outgoing waves of any frequency or any incident direction; the waves decay exponentially into the PML medium.

PML: Issues In practice, the PML medium must be truncated and the new (PML) boundary generates reflected waves which can pollute the solution in the computational domain. Error estimates in the computational domain between the solutions of the original scattering and the truncated PML problems. Related works: Helmholtz scattering problems: Lassas and Somersalo (98, 01), Hohage, Schmidt, and Zschiedrich (2001), Chen and Liu (2005). 3 -D EM scattering: little is known!

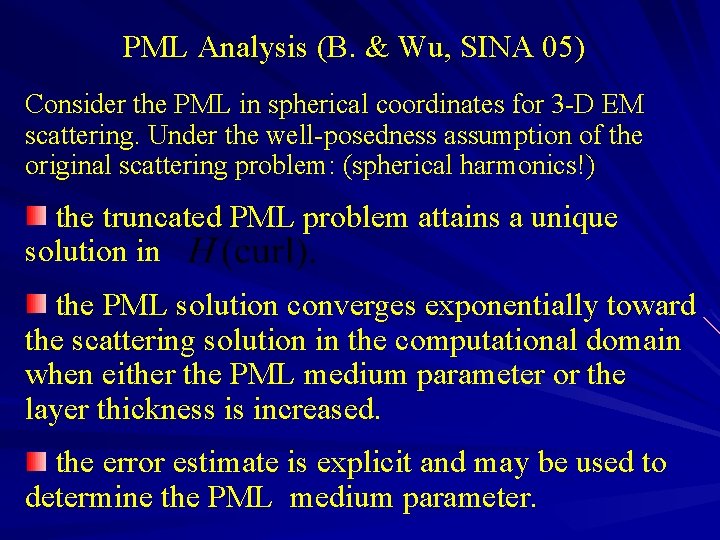
PML Analysis (B. & Wu, SINA 05) Consider the PML in spherical coordinates for 3 -D EM scattering. Under the well-posedness assumption of the original scattering problem: (spherical harmonics!) the truncated PML problem attains a unique solution in the PML solution converges exponentially toward the scattering solution in the computational domain when either the PML medium parameter or the layer thickness is increased. the error estimate is explicit and may be used to determine the PML medium parameter.

Variational Problem

Compact Imbedding

More General Scattering Model Consider the following general form: With jump coefficients and smooth interfaces Applications include: Modeling of nonlinear optics Scattering by nonlinear media

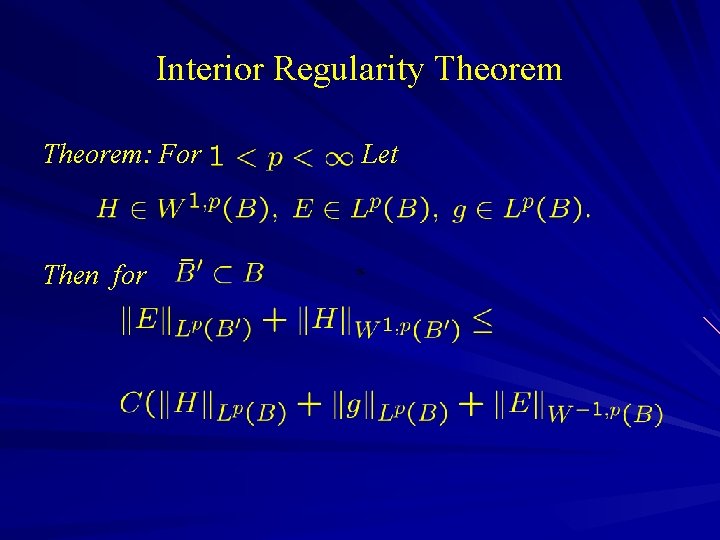
Interior Regularity Theorem: For Then for Let

Global Estimates Interior estimates. Boundary estimates: a new version of Maximum Principle. Decompose the fields into propagating modes and evanescent modes Scattering by periodic structures (B. , Minut, and Zhou, CPDE ‘ 07) and general bounded media (B. , Li, Zhou, JDE, ‘ 08) Many open problems!

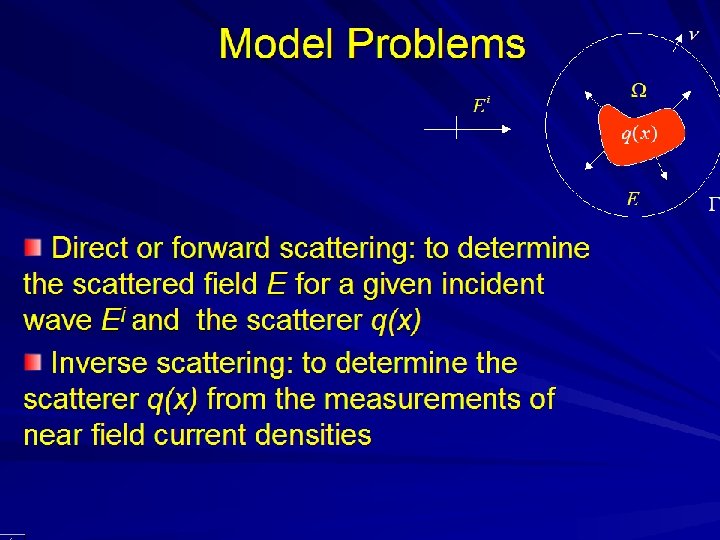
3 -D Inverse Medium Scattering To reconstruct the medium from boundary easurements: Dorn, Bertete-Aguirre, Berryman, Papanicolaou (1999), Haddar and Monk (2002), Hohage (2003), Colton and Kress, W. Chew et al (2003, 2004) Theoretical studies…: Dt. N data

Measured Data Boundary measurements: far-field (a few wavelength) or near-field (subwavelength) Multiple frequency data Fixed frequency Incomplete or partial data A reconstruction should be no more precise than that the data it represents.

Uncertainty Principle At the end of 19 th century, Abbe and Rayleigh showed: there is a limit to the sharpness of details that could be observed by an optical microscope Heisenberg later used the optical experiment to illustrate that the smallest details of the medium that can be detected are always larger than a half wavelength! Far field versus near-field!

Uncertainty Principle(cont’d) Recently, it is confirmed that the loss is related to the nonradiative components of the field -- evanescent waves confined on the surface of the scatterer (a few nanometers) and decaying outside the smallest distance between two solved points in the spatial or frequency domain, and the approximate spread in wave number Good news: It does not limit the resolution rather offers a limit on the accuracy of the reconstruction at a given wave number. To increase the resolution, a larger wave number is

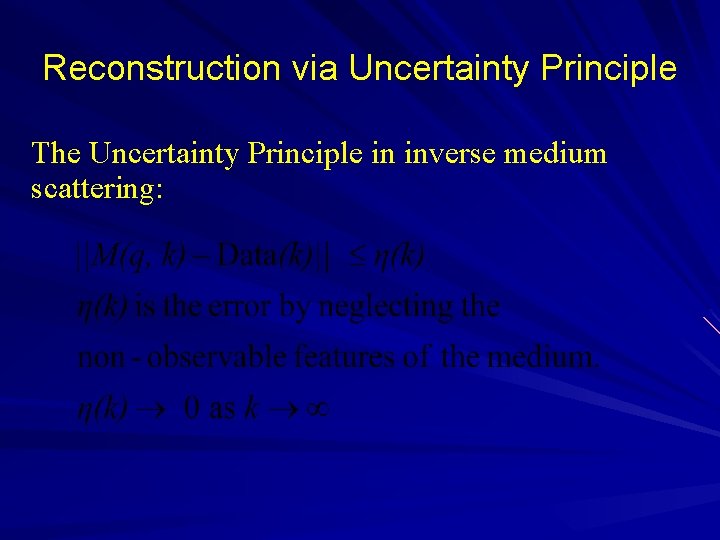
Reconstruction via Uncertainty Principle The Uncertainty Principle in inverse medium scattering:

Continuation Method 2 -D Inverse medium scattering – Full aperture, Chen & Rokhlin (97), Chen (97 a, 97 b), Analysis, B. , Chen, & Ma(00) – Limited aperture, B. & Liu (03), B. & Li (07) – Fixed frequency, B. & Li (05, 06, 07) – Convergence, B. & Triki (08) Inverse obstacle scattering problem – Coifman, Goldberg, Hrycak, & Rokhlin (99) – B. , Hou, & Li (07) 3 -D Inverse medium scattering problem

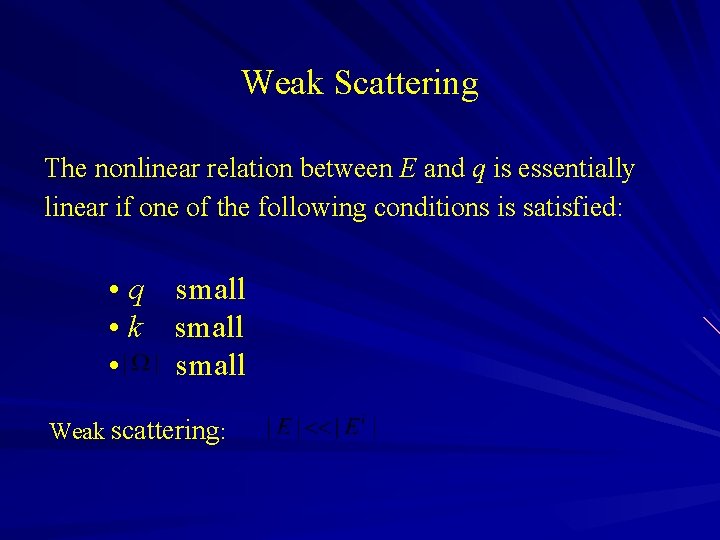
Weak Scattering The nonlinear relation between E and q is essentially linear if one of the following conditions is satisfied: • q small • k small • small Weak scattering:

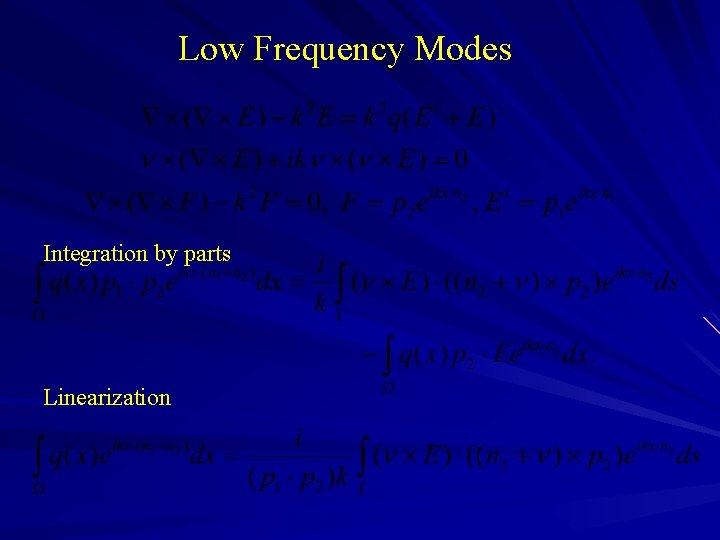
Low Frequency Modes Integration by parts Linearization

Kaczmarz method (Natterer, 86) In short

Recursive Linearization

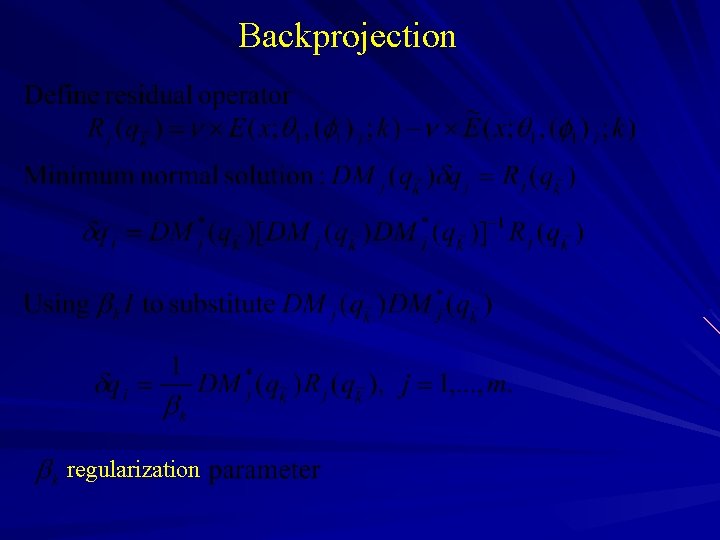
Backprojection regularization

Adjoint State Method

Reconstruction Algorithm: Beyond Born

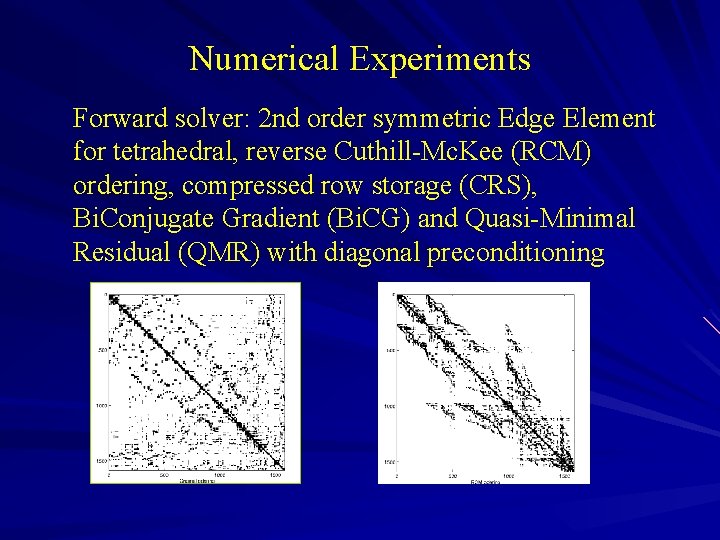
Numerical Experiments Forward solver: 2 nd order symmetric Edge Element for tetrahedral, reverse Cuthill-Mc. Kee (RCM) ordering, compressed row storage (CRS), Bi. Conjugate Gradient (Bi. CG) and Quasi-Minimal Residual (QMR) with diagonal preconditioning

Numerical Experiments Forward solver: 2 nd order symmetric Edge Element for tetrahedral, reverse Cuthill-Mc. Kee (RCM) ordering, compressed row storage (CRS), Bi. Conjugate Gradient (Bi. CG) and Quasi-Minimal Residual (QMR) with diagonal preconditioning


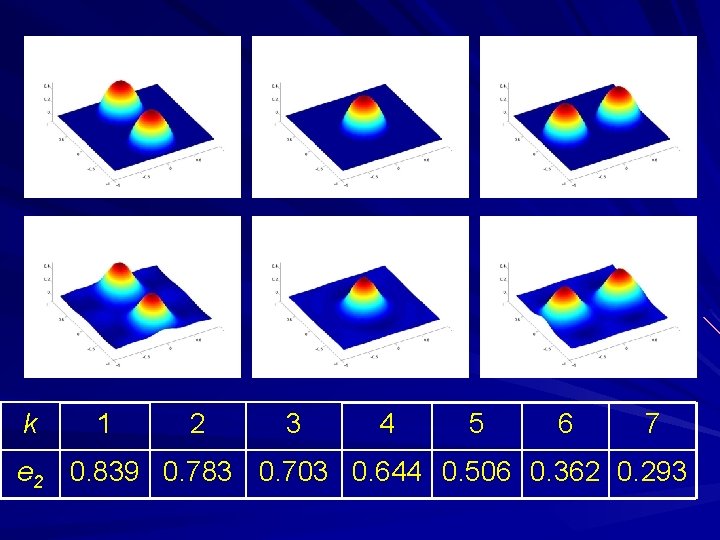
k 1 2 3 4 5 6 7 e 2 0. 839 0. 783 0. 703 0. 644 0. 506 0. 362 0. 293

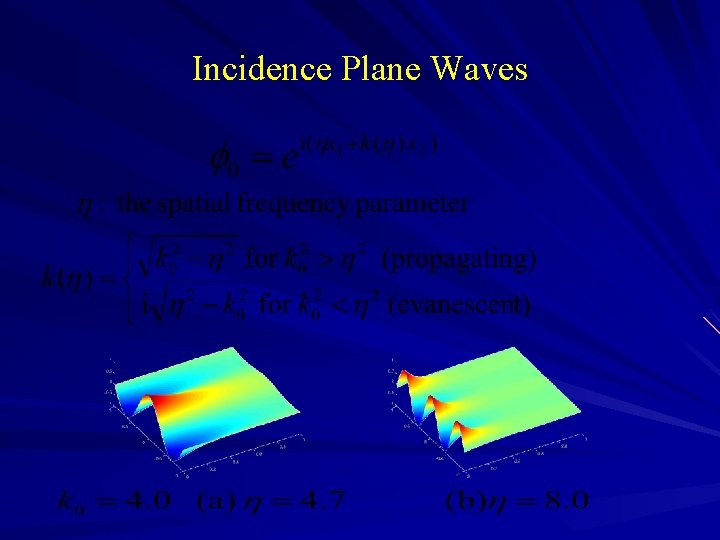
Recursive Linearization for Fixed Frequency IMP: Evanescent Incident Fields Choose a large spatial frequency parameter Divide the interval Computer the initial guess via Born (weak scattering) Recursive linearization Subwavelength resolution

Incidence Plane Waves

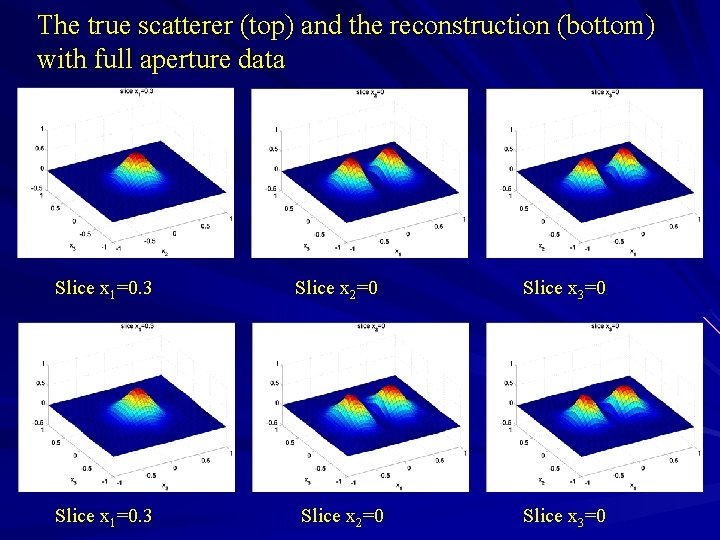
The true scatterer (top) and the reconstruction (bottom) with full aperture data Slice x 1=0. 3 Slice x 2=0 Slice x 3=0

The true scatterer (top) and the reconstruction (bottom) with limited aperture data Slice x 1=0. 3 Slice x 2=0 Slice x 3=0






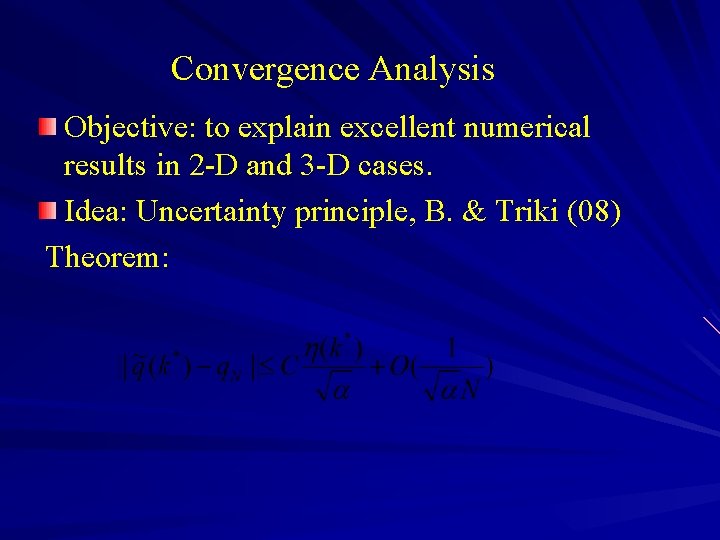
Convergence Analysis Objective: to explain excellent numerical results in 2 -D and 3 -D cases. Idea: Uncertainty principle, B. & Triki (08) Theorem:

Convergence Analysis: Discussions Linear convergence as the wave number goes to the infinity For a bounded sequence of wave numbers convergence to the observable part of the medium The regularization parameter indicates the computational cost How certain is Uncertainty Principle ? ? ?

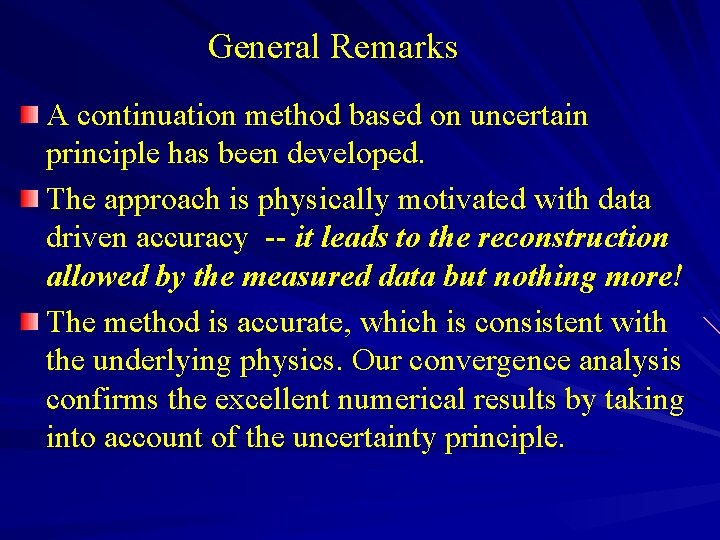
General Remarks A continuation method based on uncertain principle has been developed. The approach is physically motivated with data driven accuracy -- it leads to the reconstruction allowed by the measured data but nothing more! The method is accurate, which is consistent with the underlying physics. Our convergence analysis confirms the excellent numerical results by taking into account of the uncertainty principle.

(II) Inverse Source Problems (joint with Lin & Triki, JDE, 2010) Linear but with many common features Complete stability analysis with fixed frequency or multiple frequency data; Promising numerical results Previous results: Bleistein & Cohen`77, He & Romanov`98, Ammari, B. , Fleming `02; Albanese&Monk `06, Davaney et al `04, 07 Difficulties: Nonuniqueness and ill-posedness.

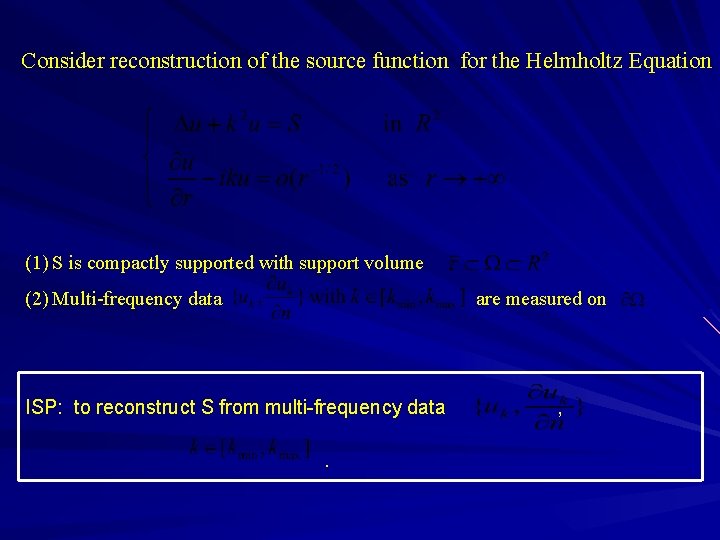
Consider reconstruction of the source function for the Helmholtz Equation (1) S is compactly supported with support volume (2) Multi-frequency data are measured on ISP: to reconstruct S from multi-frequency data. ,

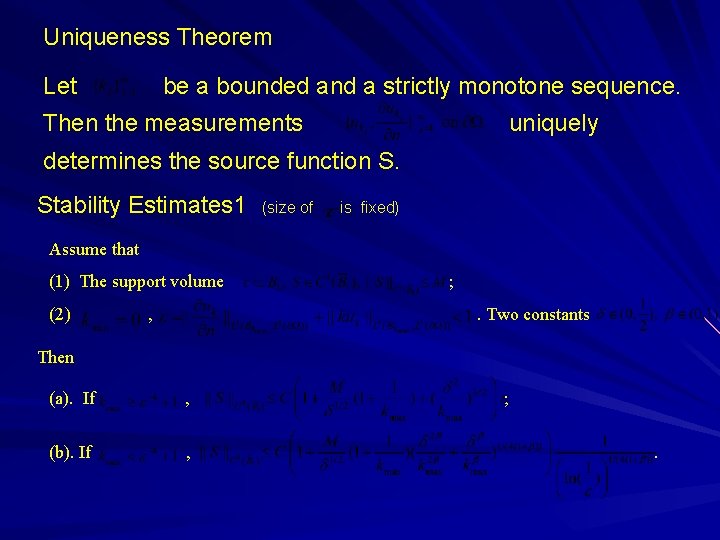
Uniqueness Theorem Let be a bounded and a strictly monotone sequence. Then the measurements uniquely determines the source function S. Stability Estimates 1 (size of is fixed) Assume that (1) The support volume (2) , ; . Two constants Then (a). If , (b). If , ; .

Stability Estimates 2 ( is fixed, ) Assume that (1) The support volume (2) ; , , . Two constants (3) Then (a). If (b). If , ; , .

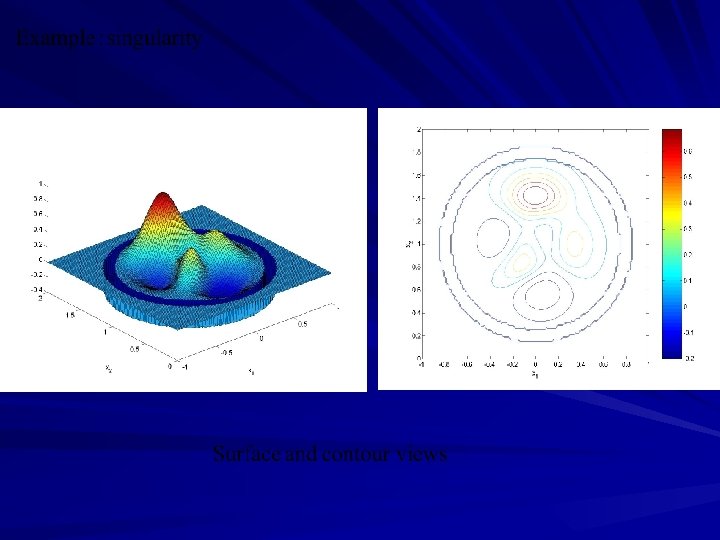
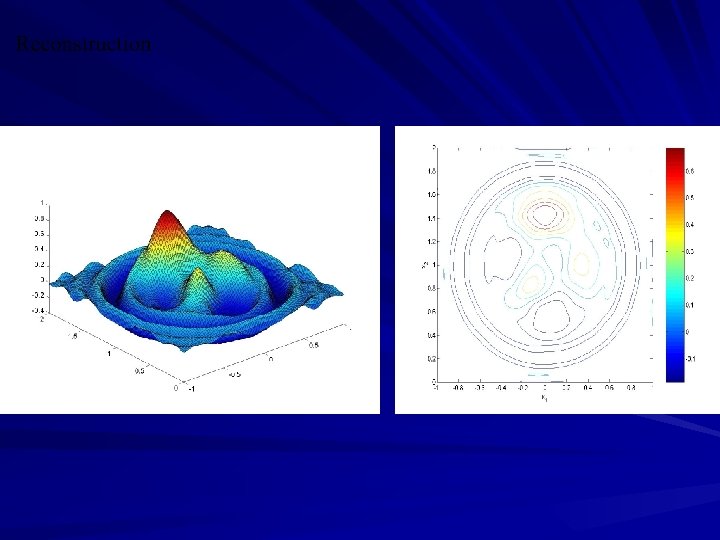
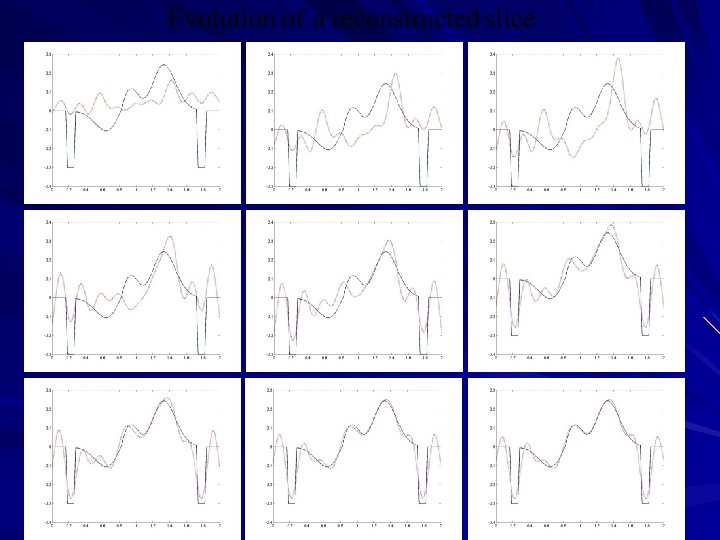
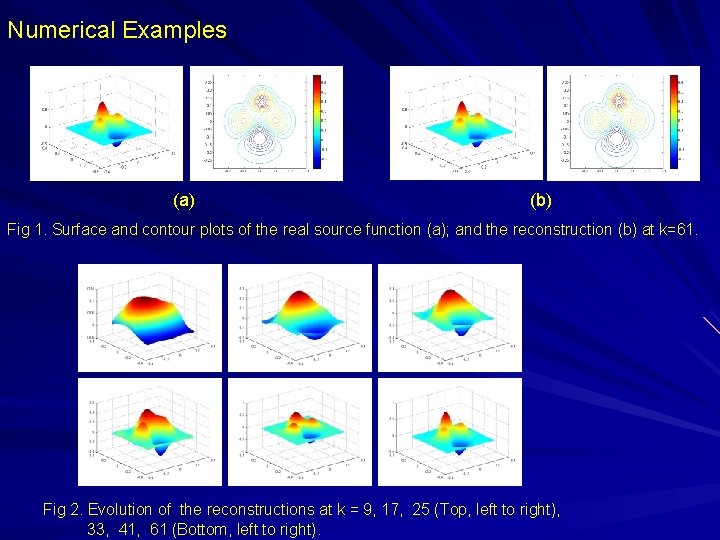
Numerical Examples (a) (b) Fig 1. Surface and contour plots of the real source function (a); and the reconstruction (b) at k=61. Fig 2. Evolution of the reconstructions at k = 9, 17, 25 (Top, left to right), 33, 41, 61 (Bottom, left to right).

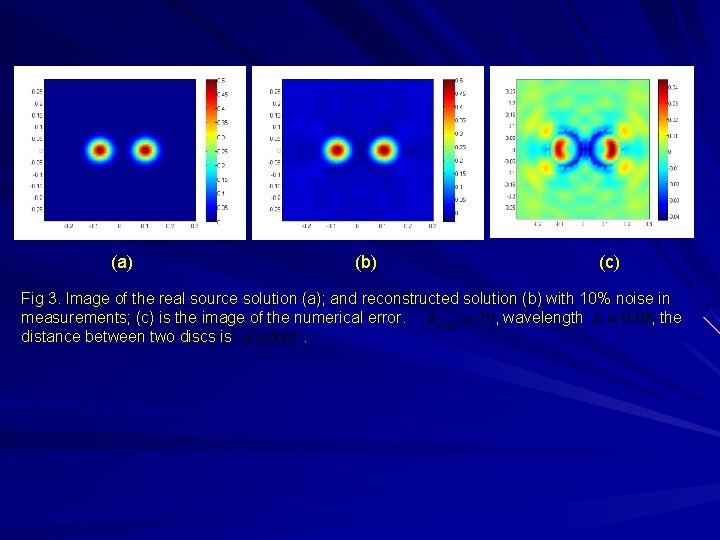
(a) (b) (c) Fig 3. Image of the real source solution (a); and reconstructed solution (b) with 10% noise in measurements; (c) is the image of the numerical error. , wavelength , the distance between two discs is.

Inverse Source Problems Limited aperture Inhomogeneous media ISP in a (weakly) random medium Determination of a stochastic source Cloak a source?

Concluding Remarks:Stability Calderon’s problem: bad/optimal estimate Almost Lipschitz type estimate (B. & Yun’ 08) Velocity inversion? Estimates for the multiple frequency case --completely open for inverse medium problem! ISP Convergence study of recursive linearization --- rigorous characterization of the uncertainty principle

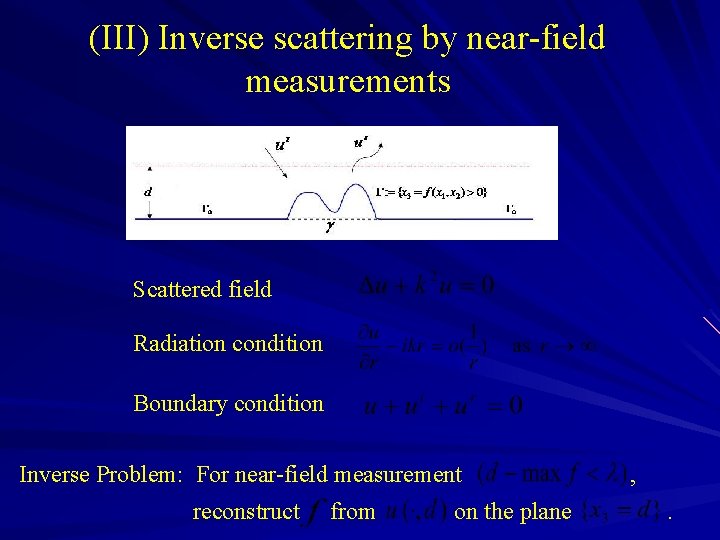
(III) Inverse scattering by near-field measurements Scattered field Radiation condition Boundary condition Inverse Problem: For near-field measurement reconstruct from on the plane , .

Recent studies A. Willers, (1987) well-posedness study of the forward problem. G. Derveaux, G. Papanicolaou and C. Tsogka, (2006) linearized model ( ), denoising using broadband signals. S. Chandler-wilde et al, scattering by unbounded rough surface. H. Ammari, G. Bao, A. Wood, analysis of the cavity problem (local downward surface displacement) Challenges : nonlinearity, ill-posedness,diffraction limit Opportunity : evanescent mode becomes significant in near field regime.

Analysis of the scattered wave Boundary integral formulation: Plane wave decomposition of Green’s function where Scattered field consists of propagation and evanescent mode!

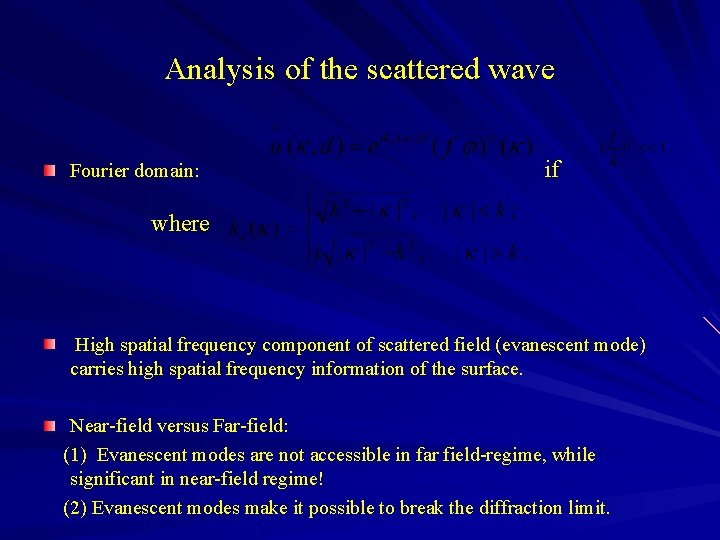
Analysis of the scattered wave Fourier domain: if where High spatial frequency component of scattered field (evanescent mode) carries high spatial frequency information of the surface. Near-field versus Far-field: (1) Evanescent modes are not accessible in far field-regime, while significant in near-field regime! (2) Evanescent modes make it possible to break the diffraction limit.

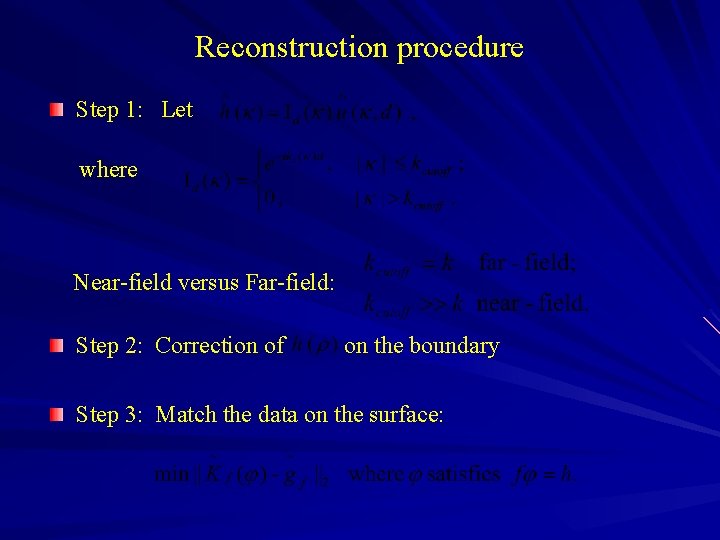
Reconstruction procedure Step 1: Let where Near-field versus Far-field: Step 2: Correction of on the boundary Step 3: Match the data on the surface:

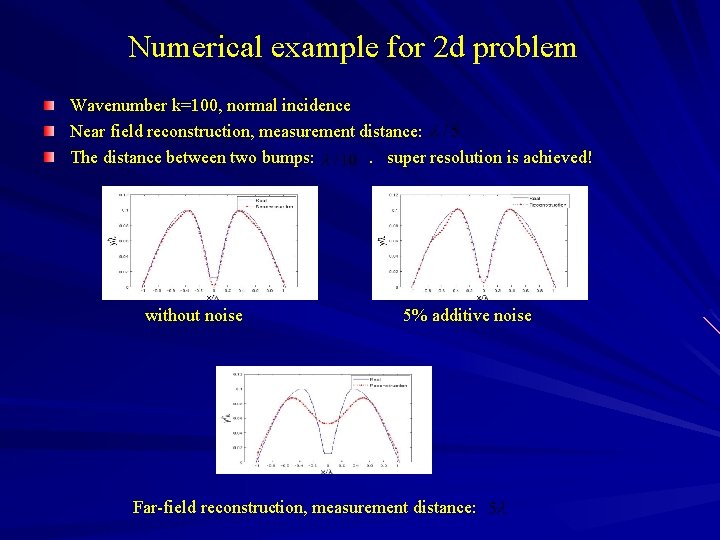
Numerical example for 2 d problem Wavenumber k=100, normal incidence Near field reconstruction, measurement distance: The distance between two bumps: . super resolution is achieved! without noise 5% additive noise Far-field reconstruction, measurement distance:

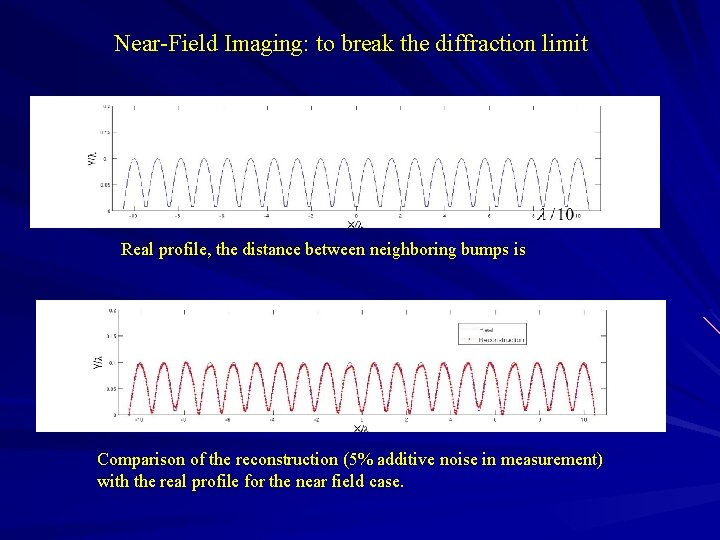
Near-Field Imaging: to break the diffraction limit Real profile, the distance between neighboring bumps is Comparison of the reconstruction (5% additive noise in measurement) with the real profile for the near field case.

Incomplete or Partial Data Limited aperture Phaseless data Poor initial guess Mathematical issues: to redefine uniqueness & stability

Inverse Backscattering with Limited Aperture Data

Inverse Scattering in Complex Media Dispersive Medium Applications include breast cancer detections and imaging of human tissues Multi-frequency data but the dielectric coefficients (medium) are now frequency dependent: the Debye model To determine two functions instead of one! Large scale inverse problems

Inverse Scattering in Complex Media Stochastics Applications in sensing Raman spectroscopy for bio and environmental sensing Photonic Crystal spectrometer (in nano-scale) Human tissue Spatially incoherent light

Micro Diffractive Optics Maxwell’s Eqns in periodic complicated media (optical diffraction gratings) Applications areas: optical comm. , optical storage, sensors, displays, corrective lenses, scanners, laser technology, security, … Optical medium: --- Classical --- Chiral --- Nonlinear: second harmonic generation --- Anisotropic Issues: Modeling, simulation, analysis, & optimal design

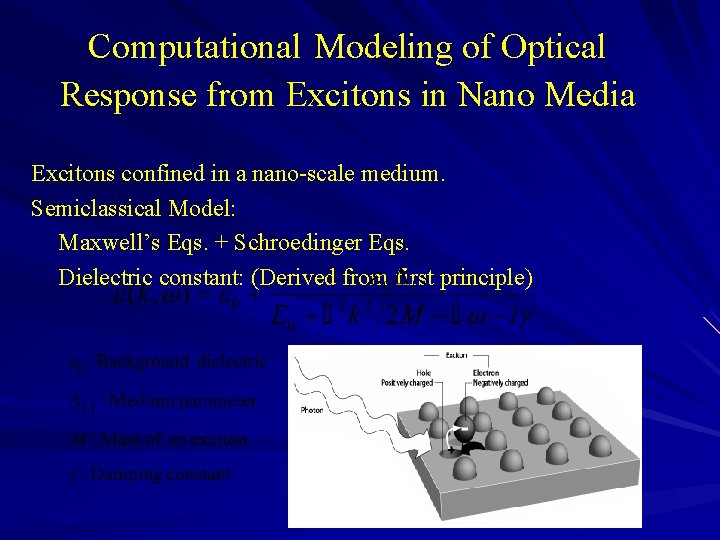
Computational Modeling of Optical Response from Excitons in Nano Media Excitons confined in a nano-scale medium. Semiclassical Model: Maxwell’s Eqs. + Schroedinger Eqs. Dielectric constant: (Derived from first principle)

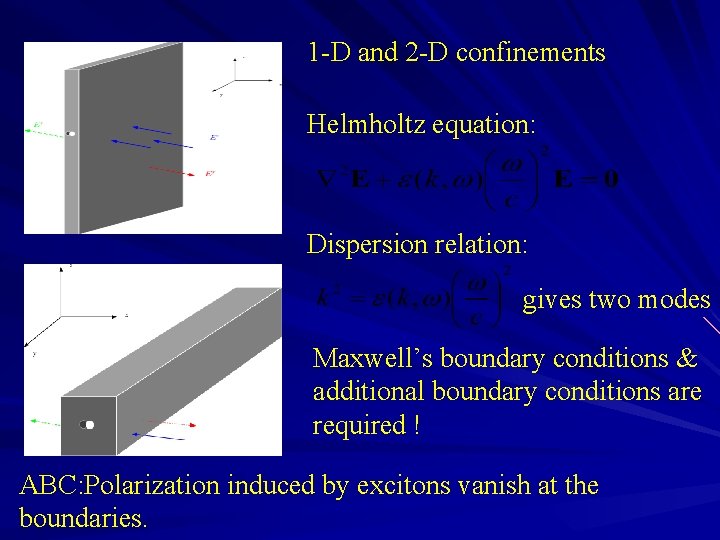
1 -D and 2 -D confinements Helmholtz equation: Dispersion relation: gives two modes Maxwell’s boundary conditions & additional boundary conditions are required ! ABC: Polarization induced by excitons vanish at the boundaries.

Nano Optical Modeling/Imaging ● An emerging area in optical science dealing with scales small but not yet atomic ● Microscopic versus macroscopic Maxwell’s equations ● Multiscale approaches are essential Long term objective: characterizations of nano materials/structures Other related topics: Uniqueness for IPs with minimum amount of data: 3 -D gratings (joint with Zhang, and Zou, TAMS) Stability estimates for high wave number problems (joint with Yun, Zhou)

 Recent developments in ict
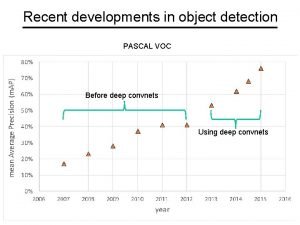
Recent developments in ict Recent developments in object detection
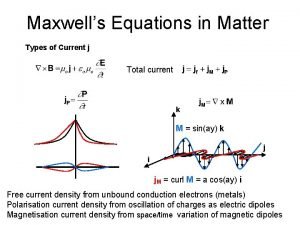
Recent developments in object detection Maxwells equations in matter
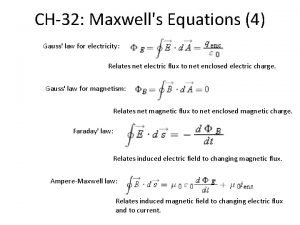
Maxwells equations in matter Maxwells equations
Maxwells equations Maxwells equations
Maxwells equations Maxwell equations
Maxwell equations Gauss law
Gauss law Blger
Blger Ampère's circuital law (with maxwell's addition)
Ampère's circuital law (with maxwell's addition) Target developments
Target developments Cultural development of sahelanthropus tchadensis brainly
Cultural development of sahelanthropus tchadensis brainly Democratic developments in england
Democratic developments in england Chapter 11 political developments in the early republic
Chapter 11 political developments in the early republic American grassfed association standards
American grassfed association standards Determine the different patterns of development
Determine the different patterns of development Chartered developments
Chartered developments Cplim
Cplim Transmission developments
Transmission developments What is surface development
What is surface development How did parliament emerged victorious in medieval england
How did parliament emerged victorious in medieval england Truncated cylinder development
Truncated cylinder development Political developments in the early republic
Political developments in the early republic Surface developments
Surface developments In the colonial era developments such as the new england
In the colonial era developments such as the new england Asis atca
Asis atca Huron creek developments reviews
Huron creek developments reviews Medical developments international penthrox
Medical developments international penthrox Inverse circular functions
Inverse circular functions Use inverse of matrix to solve linear system
Use inverse of matrix to solve linear system Solving equations inverse operations
Solving equations inverse operations How are inverse operations used to solve equations
How are inverse operations used to solve equations Rayleigh theory of light scattering
Rayleigh theory of light scattering Contoh refraksi gelombang
Contoh refraksi gelombang Scattering cross section in nuclear physics
Scattering cross section in nuclear physics Zimm plot excel
Zimm plot excel Compton scattering
Compton scattering Double parton scattering
Double parton scattering Scattering of light
Scattering of light Rayleigh theory of light scattering
Rayleigh theory of light scattering Scattering matrix for a reciprocal network is:
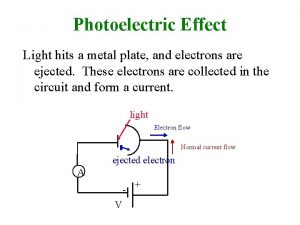
Scattering matrix for a reciprocal network is: Compton scattering vs photoelectric effect
Compton scattering vs photoelectric effect Back scattering
Back scattering Rayleigh theory of light scattering
Rayleigh theory of light scattering Compton scattering
Compton scattering Scattering matrix
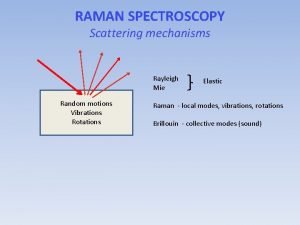
Scattering matrix Raman scattering definition
Raman scattering definition Reciprocal network
Reciprocal network Scattering of light in suspension
Scattering of light in suspension Vertical
Vertical Diffusione thomson
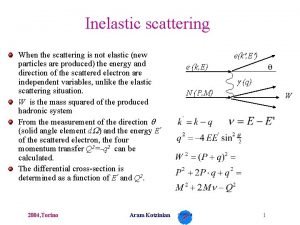
Diffusione thomson Elastic scattering vs inelastic
Elastic scattering vs inelastic Coherent vs incoherent scattering
Coherent vs incoherent scattering Polarisation by scattering
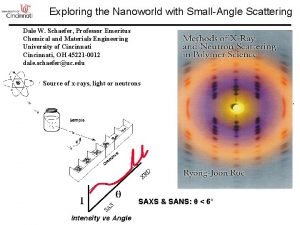
Polarisation by scattering Small angle scattering
Small angle scattering Rayleigh vs raman scattering
Rayleigh vs raman scattering Compton scattering
Compton scattering Scattering efficiency
Scattering efficiency Pauli blocking of light scattering in degenerate fermions
Pauli blocking of light scattering in degenerate fermions Coherent scattering
Coherent scattering Dynamic scattering type lcd
Dynamic scattering type lcd Scattering in central force field
Scattering in central force field Boltzmann law
Boltzmann law Diffraction and scattering
Diffraction and scattering Rutherford scattering
Rutherford scattering Compton scattering formula derivation
Compton scattering formula derivation Double scattering
Double scattering Advances in mri
Advances in mri Elastic energy
Elastic energy Scattering matrix
Scattering matrix Scattering matrix
Scattering matrix Dynamic light scattering 원리
Dynamic light scattering 원리 Scattering reaction
Scattering reaction Area of cross section
Area of cross section Horizontal
Horizontal Phase doppler anemometry
Phase doppler anemometry News passive voice
News passive voice