Lattices and Symmetry Scattering and Diffraction Physics James




















![Definitions [hkl] <hkl> (hkl) {hkl} hkl indices of a lattice direction indices of a Definitions [hkl] <hkl> (hkl) {hkl} hkl indices of a lattice direction indices of a](https://slidetodoc.com/presentation_image_h2/48838d42d8ed1c6550d5df0d94ae08be/image-36.jpg)


















- Slides: 87

Lattices and Symmetry Scattering and Diffraction (Physics) James A. Kaduk INEOS Technologies Analytical Science Research Services Naperville IL 60566 James. Kaduk@innovene. com 1

Harry Potter and the Sorcerer’s (Philosopher’s) Stone Ron: Seeker? But first years never make the house team. You must be the youngest Quiddich player in … Harry: … a century. According to Mc. Gonagall. Fred/George: Well done, Harry. Wood’s just told us. Ron: Fred and George are on the team, too. Beaters. Fred/George: Our job is to make sure you don’t get bloodied up too bad. 2

Alastor “Mad-Eye” Moody – “Constant Vigilance” Harry Potter and the Goblet of Fire (2005) 3

The crystallographer’s world view Reality can be more complex! 4

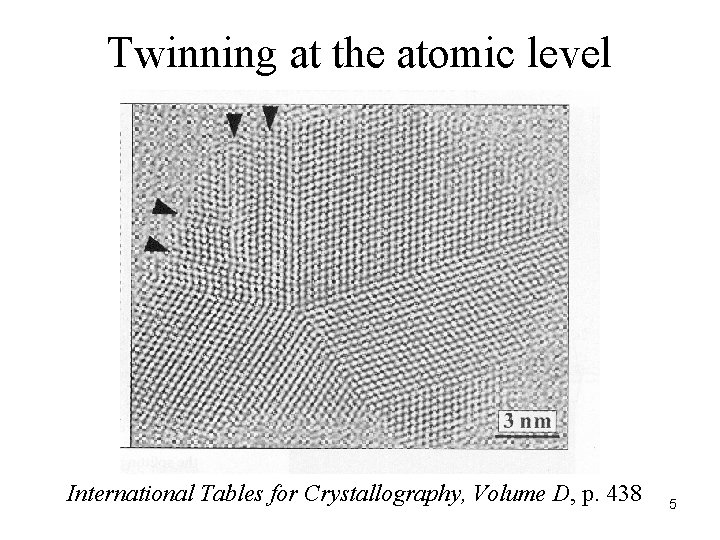
Twinning at the atomic level International Tables for Crystallography, Volume D, p. 438 5

PDB entry 1 eqg = ovine COX-1 complexed with Ibuprofen 6

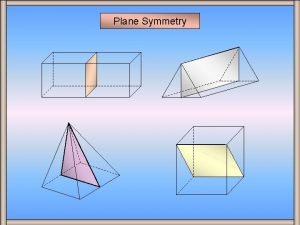
Atoms (molecules) pack together in a regular pattern to form a crystal. There are two aspects to this pattern: Periodicity Symmetry First, consider the periodicity… 7

To describe the periodicity, we superimpose (mentally) on the crystal structure a lattice. A lattice is a regular array of geometrical points, each of which has the same environment (they are all equivalent). 8

A Primitive Cubic Lattice (Cs. Cl) 9

A unit cell of a lattice (or crystal) is a volume which can describe the lattice using only translations. In 3 dimensions (for crystallographers), this volume is a parallelepiped. Such a volume can be defined by six numbers – the lengths of the three sides, and the angles between them – or three basis vectors. 10

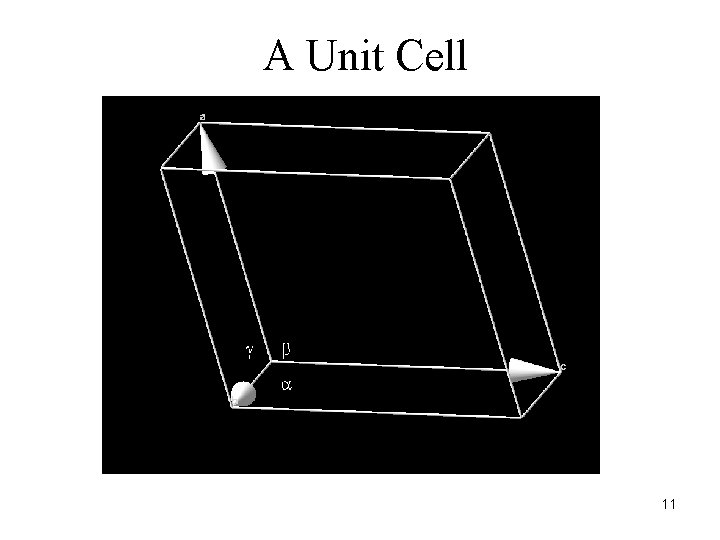
A Unit Cell 11

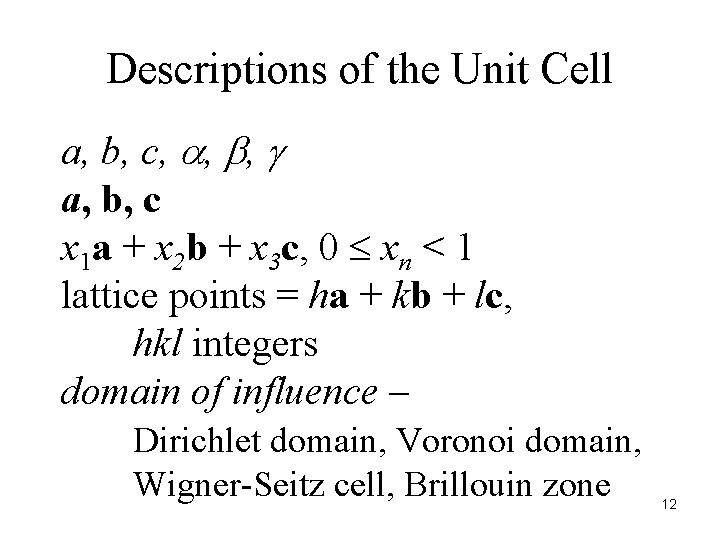
Descriptions of the Unit Cell a, b, c, , , a, b, c x 1 a + x 2 b + x 3 c, 0 xn < 1 lattice points = ha + kb + lc, hkl integers domain of influence – Dirichlet domain, Voronoi domain, Wigner-Seitz cell, Brillouin zone 12

A Brillouin Zone C. Kittel, Introduction to Solid State Physics, 6 th Edition, p. 41 (1986) 13

The unit cell is not unique (c: My. FilesClinicindex 2. wrl) 14

15

16

17

How do I pick the unit cell? • Axis system (basis set) is right-handed • Symmetry defines natural directions and boundaries • Angles close to 90° • Standard settings of space groups • To make structural similarities clearer 18

The Reduced Cell • 3 shortest non-coplanar translations • Main Conditions (shortest vectors) • Special Conditions (unique) • May not exhibit the true symmetry 19

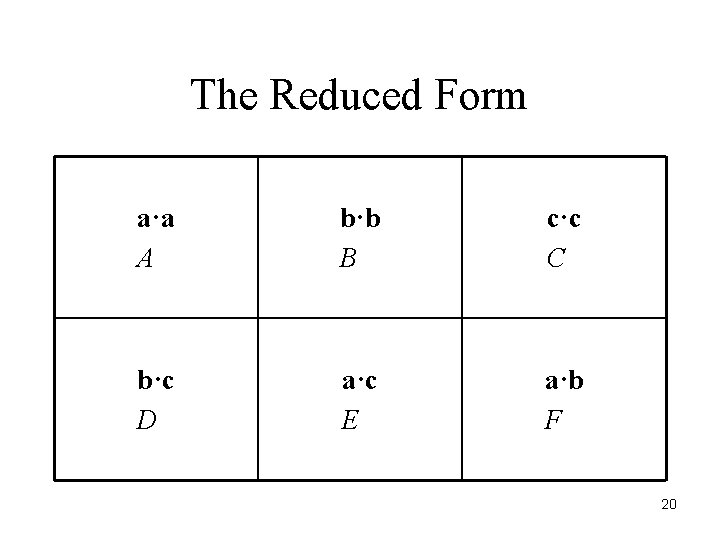
The Reduced Form a·a A b·b B c·c C b·c D a·c E a·b F 20

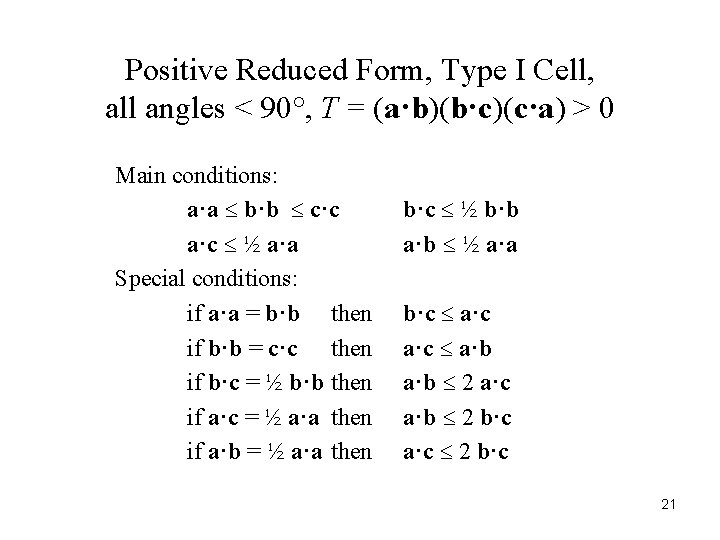
Positive Reduced Form, Type I Cell, all angles < 90°, T = (a·b)(b·c)(c·a) > 0 Main conditions: a·a b·b c·c a·c ½ a·a Special conditions: if a·a = b·b then if b·b = c·c then if b·c = ½ b·b then if a·c = ½ a·a then if a·b = ½ a·a then b·c ½ b·b a·b ½ a·a b·c a·c a·b 2 a·c a·b 2 b·c a·c 2 b·c 21

Negative reduced Form, Type II Cell angles 90°, T = (a·b)(b·c)(c·a) ≤ 0 Main Conditions: a·a b·b c·c b·c| ½ b·b |a·c| ½ a·a |a·b| a·a ( |b·c| + |a·b| ) ½ ( a·a + b·b ) Special Conditions: if a·a = b·b then |b·c| |a·c| if b·b = c·c then |a·c| |a·b| if |b·c| = ½ b·b then a·b = 0 if |a·c| = ½ a·a then a·b = 0 if |a·b| = ½ a·a then a·c = 0 if ( |b·c| + |a·b| ) = ½ ( a·a + b·b ) then a·a 2 |a·c| + |a·b| 22

There are 44 reduced forms. The relationships among the six terms determine the Bravais lattice of the crystal. J. K. Stalick and A. D. Mighell, NBS Technical Note 1229, 1986. A. D. Mighell and J. R. Rodgers, Acta Cryst. , A 36, 321 -326 (1980). 23

“The Normalized Reduced Form and Cell: Mathematical Tools for Lattice Analysis – Symmetry and Similarity”, Alan D. Mighell, J. Res. Nat. Inst. Stand. Tech. , 108(6), 447 -452 (2003). 24

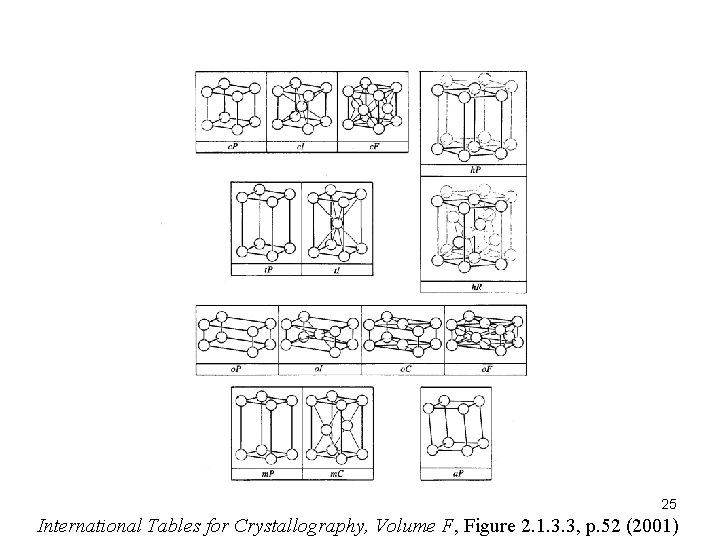
25 International Tables for Crystallography, Volume F, Figure 2. 1. 3. 3, p. 52 (2001)

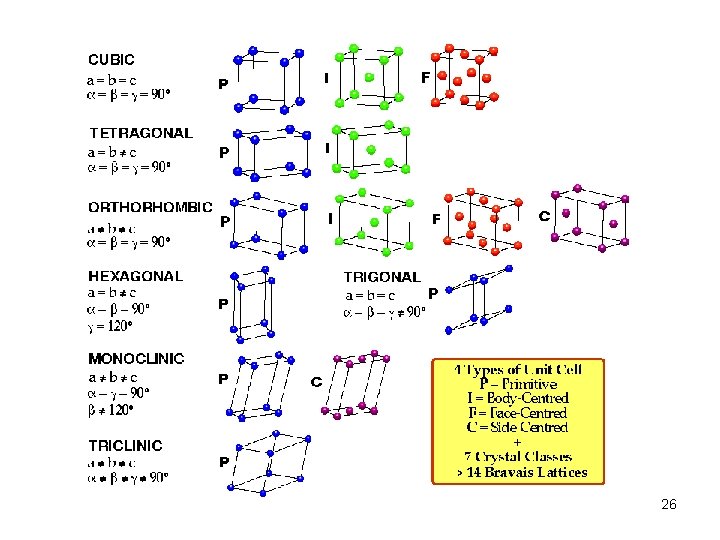
26

“The mystery of the fifteenth Bravais lattice”, A. Nussbaum, Amer. J. Phys. , 68(10), 950 -954 (2000). http: //ojps. aip. org/ajp/ 27

Symmetry Groups and Their Applications, W. Miller, Jr. , Academic Press, New York (1972), Chapter 2. 28

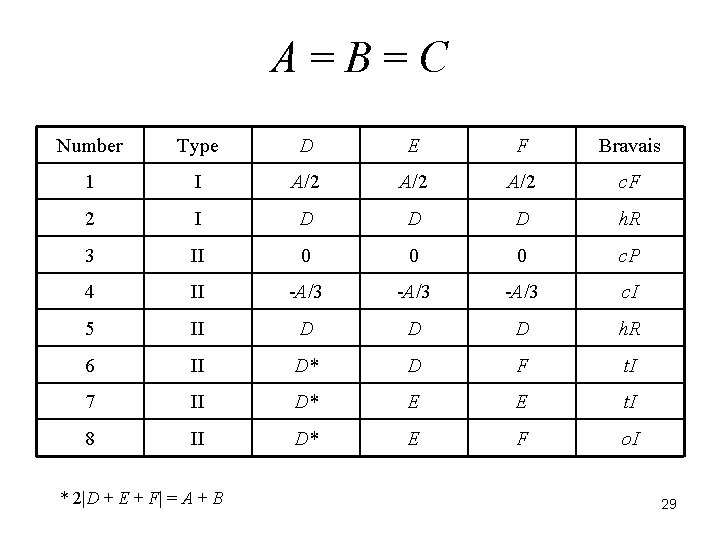
A=B=C Number Type D E F Bravais 1 I A/2 A/2 c. F 2 I D D D h. R 3 II 0 0 0 c. P 4 II -A/3 c. I 5 II D D D h. R 6 II D* D F t. I 7 II D* E E t. I 8 II D* E F o. I * 2|D + E + F| = A + B 29

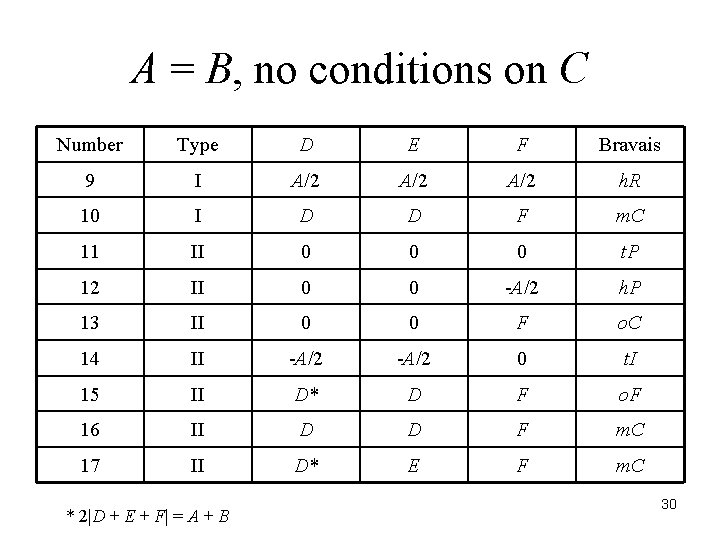
A = B, no conditions on C Number Type D E F Bravais 9 I A/2 A/2 h. R 10 I D D F m. C 11 II 0 0 0 t. P 12 II 0 0 -A/2 h. P 13 II 0 0 F o. C 14 II -A/2 0 t. I 15 II D* D F o. F 16 II D D F m. C 17 II D* E F m. C * 2|D + E + F| = A + B 30

B = C, no conditions on A Number Type D E F Bravais 18 I A/4 A/2 t. I 19 I D A/2 o. I 20 I D E E m. C 21 II 0 0 0 t. P 22 II -B/2 0 0 h. P 23 II D 0 0 o. C 24 II D* -A/3 h. R 25 II D E E m. C * 2|D + E + F| = A + B 31

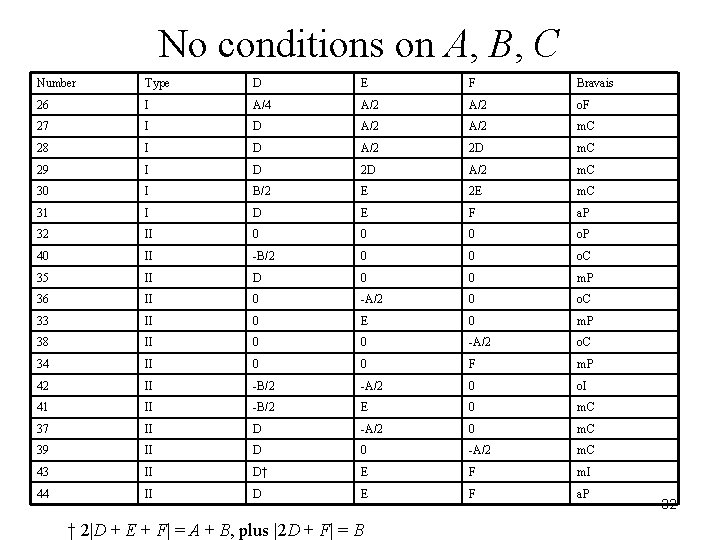
No conditions on A, B, C Number Type D E F Bravais 26 I A/4 A/2 o. F 27 I D A/2 m. C 28 I D A/2 2 D m. C 29 I D 2 D A/2 m. C 30 I B/2 E 2 E m. C 31 I D E F a. P 32 II 0 0 0 o. P 40 II -B/2 0 0 o. C 35 II D 0 0 m. P 36 II 0 -A/2 0 o. C 33 II 0 E 0 m. P 38 II 0 0 -A/2 o. C 34 II 0 0 F m. P 42 II -B/2 -A/2 0 o. I 41 II -B/2 E 0 m. C 37 II D -A/2 0 m. C 39 II D 0 -A/2 m. C 43 II D† E F m. I 44 II D E F a. P † 2|D + E + F| = A + B, plus |2 D + F| = B 32

Indexing programs can get “caught” in a reduced cell, and miss the (higher) true symmetry. It’s always worth a manual check of your cell. 33

The metric symmetry can be higher than the crystallographic symmetry! (A monoclinic cell can have β = 90°) 34

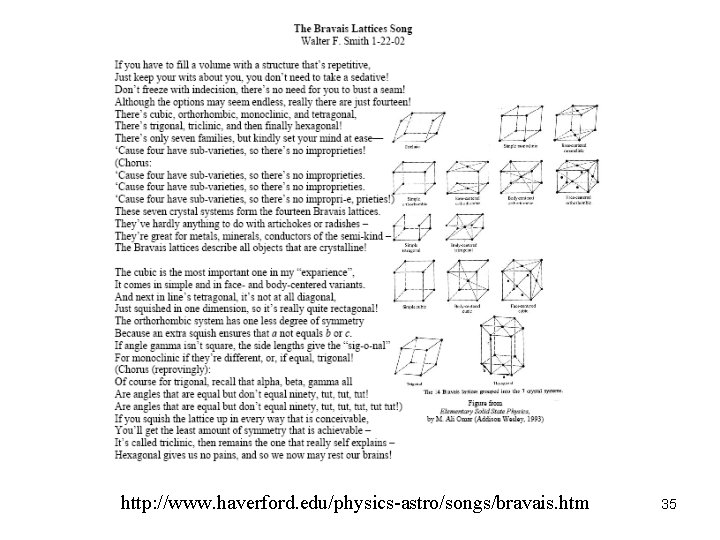
http: //www. haverford. edu/physics-astro/songs/bravais. htm 35
![Definitions hkl hkl hkl hkl hkl indices of a lattice direction indices of a Definitions [hkl] <hkl> (hkl) {hkl} hkl indices of a lattice direction indices of a](https://slidetodoc.com/presentation_image_h2/48838d42d8ed1c6550d5df0d94ae08be/image-36.jpg)
Definitions [hkl] <hkl> (hkl) {hkl} hkl indices of a lattice direction indices of a set of symmetryequivalent lattice directions indices of a single crystal face indices of a set of all symmetryequivalent crystal faces indices of a Bragg reflection 36

Now consider the symmetry… 37

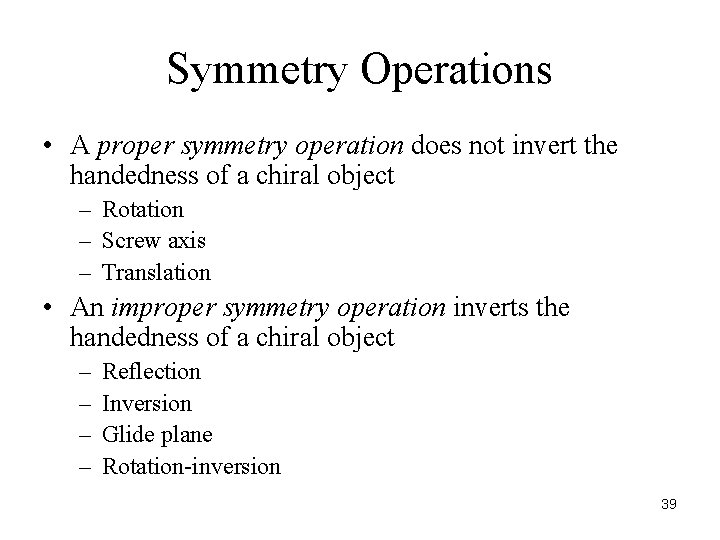
Point Symmetry Elements • A point symmetry operation does not alter at least one point upon which it operates – Rotation axes – Mirror planes – Rotation-inversion axes (rotation-reflection) – Center Screw axes and glide planes are not point symmetry elements! 38

Symmetry Operations • A proper symmetry operation does not invert the handedness of a chiral object – Rotation – Screw axis – Translation • An improper symmetry operation inverts the handedness of a chiral object – – Reflection Inversion Glide plane Rotation-inversion 39

Not all combinations of symmetry elements are possible. In addition, some point symmetry elements are not possible if there is to be translational symmetry as well. There are only 32 crystallographic point groups consistent with periodicity in three dimensions. 40

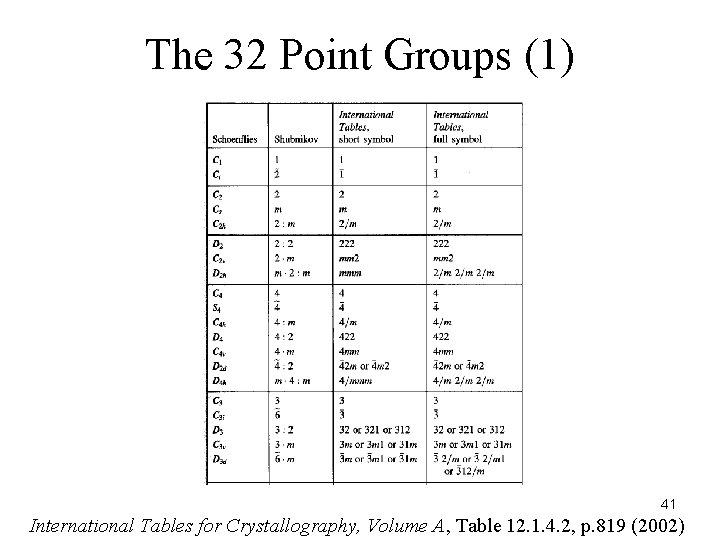
The 32 Point Groups (1) 41 International Tables for Crystallography, Volume A, Table 12. 1. 4. 2, p. 819 (2002)

The 32 Point Groups (2) 42 International Tables for Crystallography, Volume A, Table 12. 1. 4. 2, p. 819 (2002)

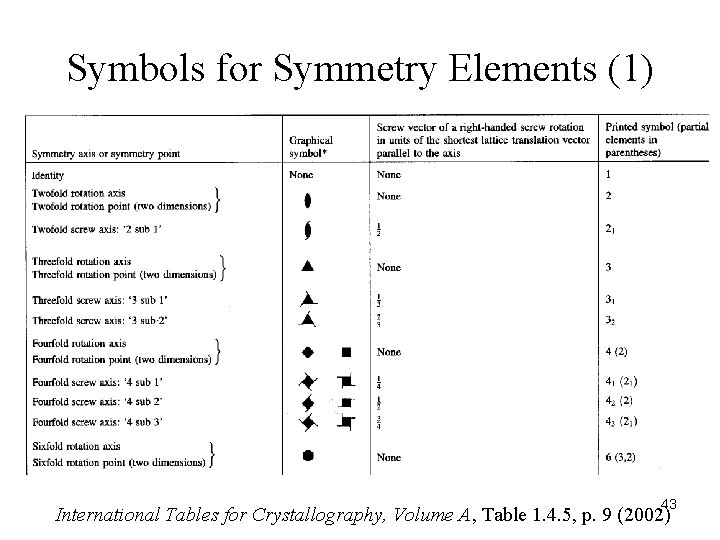
Symbols for Symmetry Elements (1) 43 International Tables for Crystallography, Volume A, Table 1. 4. 5, p. 9 (2002)

Symbols for Symmetry Elements (2) International Tables for Crystallography, Volume A, Table 1. 4. 5, p. 9 (2002)44

Symbols for Symmetry Elements (3) 45 International Tables for Crystallography, Volume A, Table 1. 4. 2, p. 7 (2002)

2 Rotation Axis (ZINJAH) 46

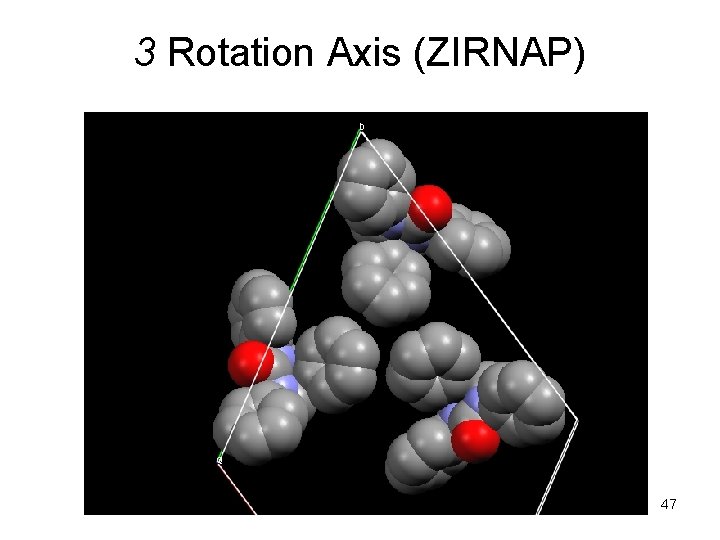
3 Rotation Axis (ZIRNAP) 47

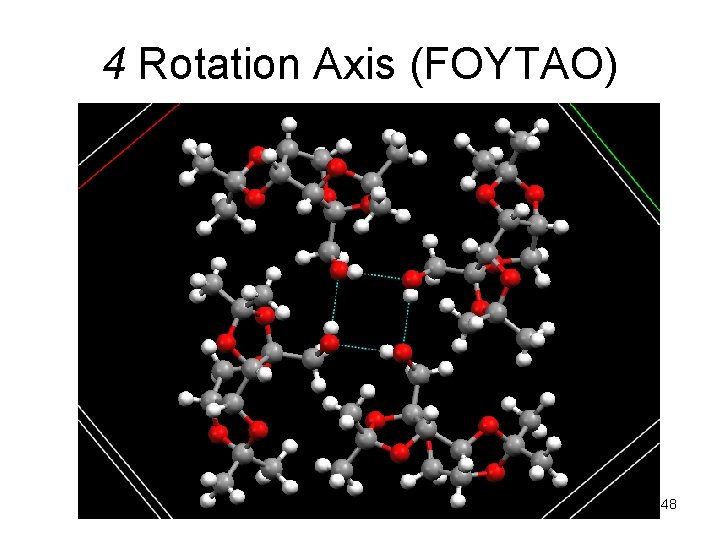
4 Rotation Axis (FOYTAO) 48

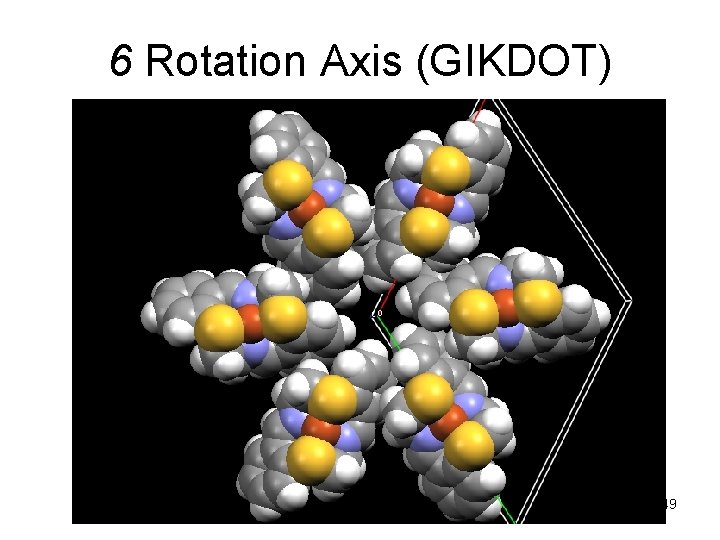
6 Rotation Axis (GIKDOT) 49

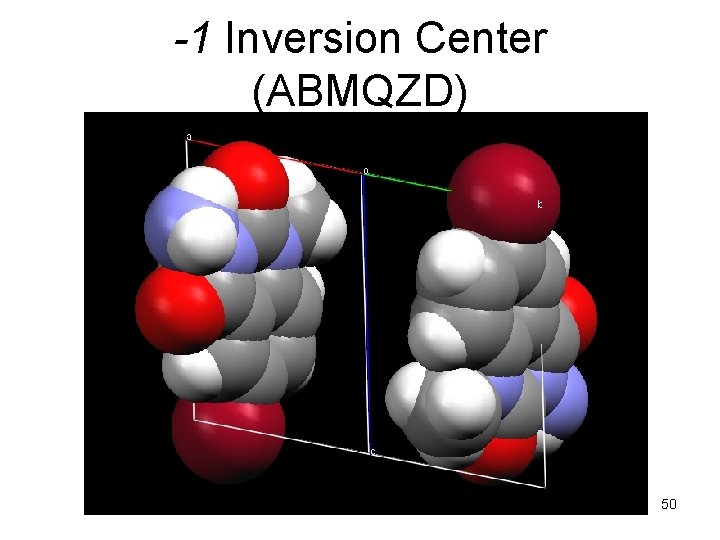
-1 Inversion Center (ABMQZD) 50

-2 Rotary Inversion Axis? 51

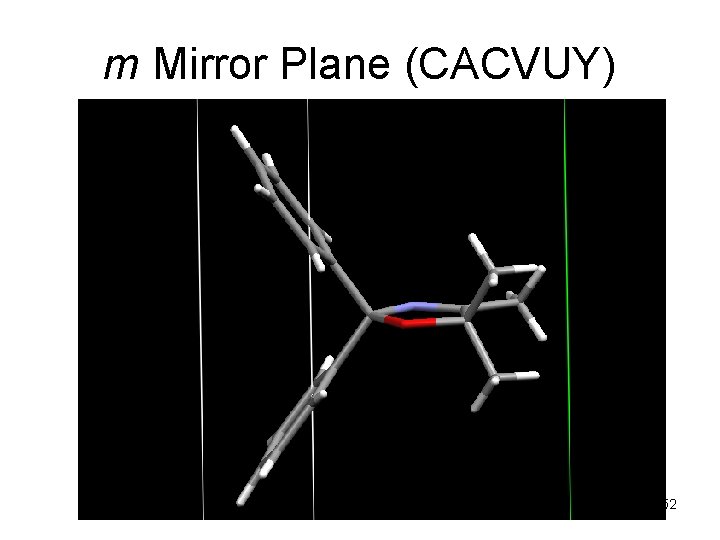
m Mirror Plane (CACVUY) 52

-3 Rotary Inversion Axis (DOXBOH) 53

-4 Rotary Inversion Axis (MEDBUS) 54

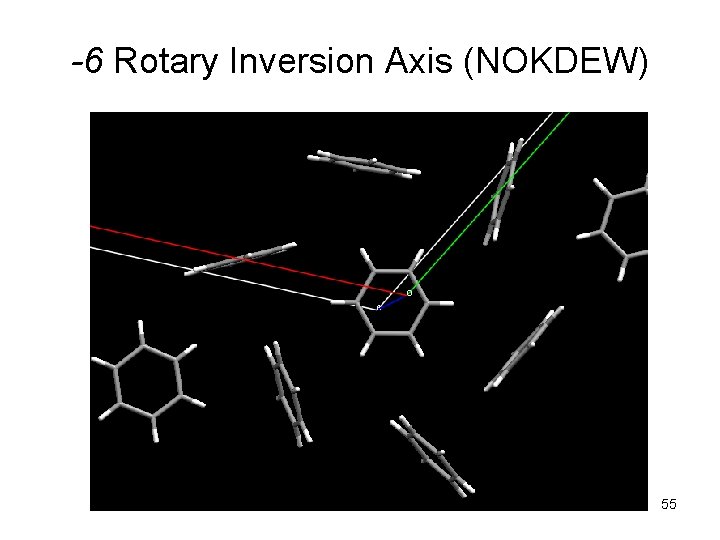
-6 Rotary Inversion Axis (NOKDEW) 55

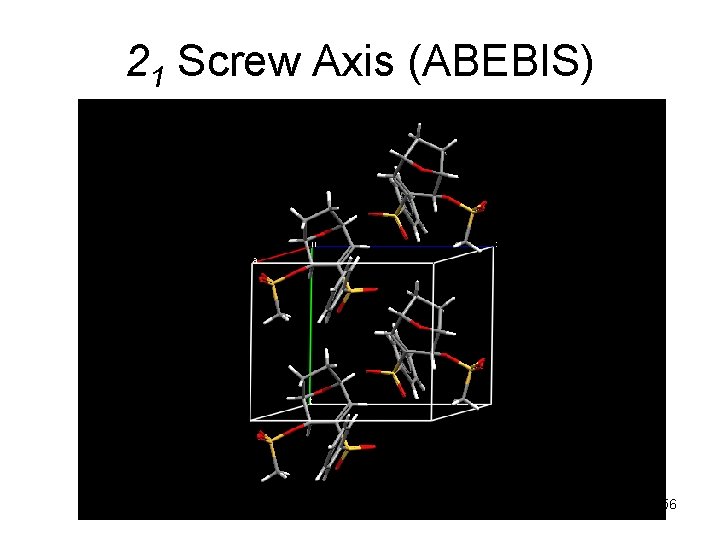
21 Screw Axis (ABEBIS) 56

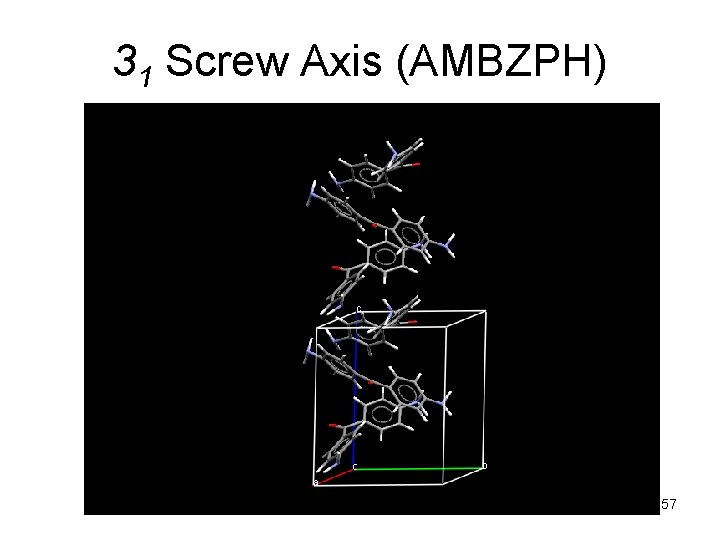
31 Screw Axis (AMBZPH) 57

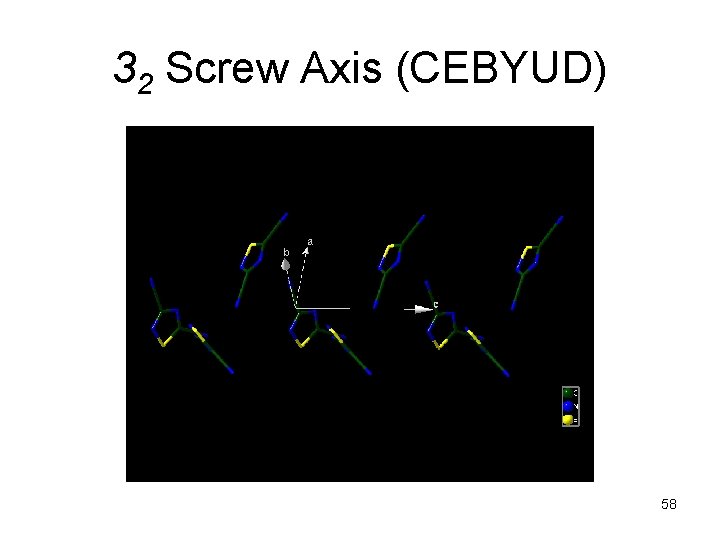
32 Screw Axis (CEBYUD) 58

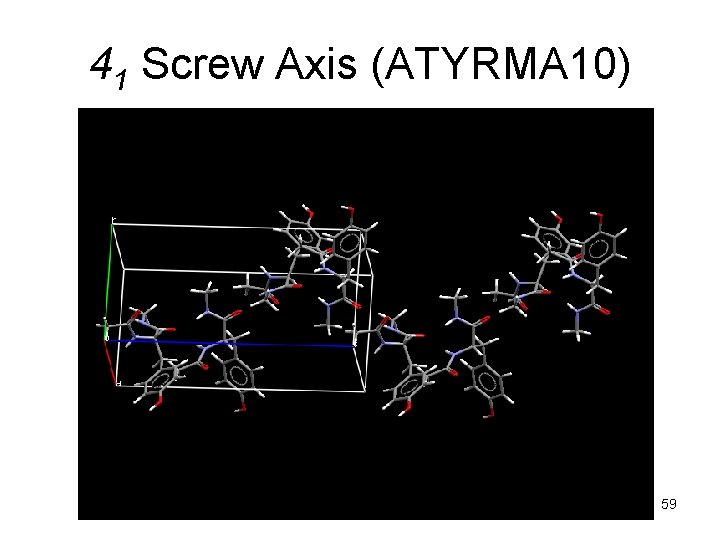
41 Screw Axis (ATYRMA 10) 59

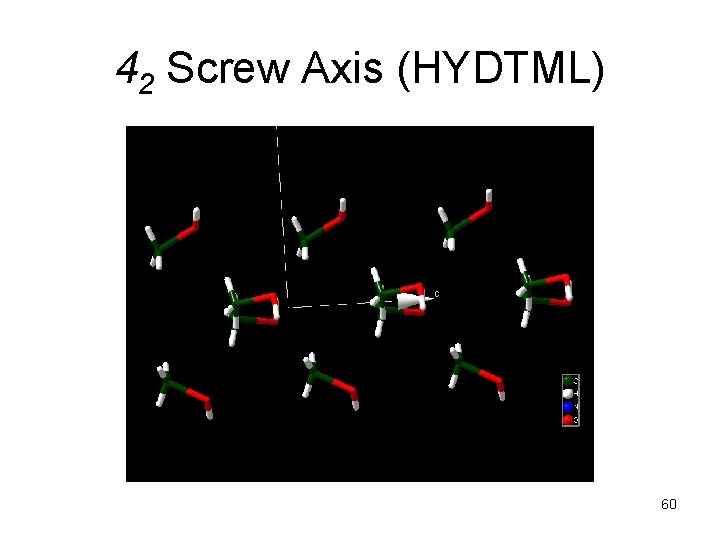
42 Screw Axis (HYDTML) 60

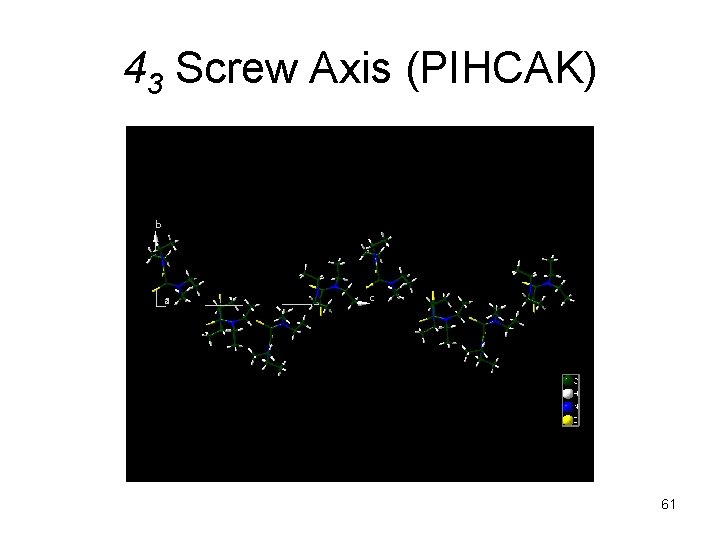
43 Screw Axis (PIHCAK) 61

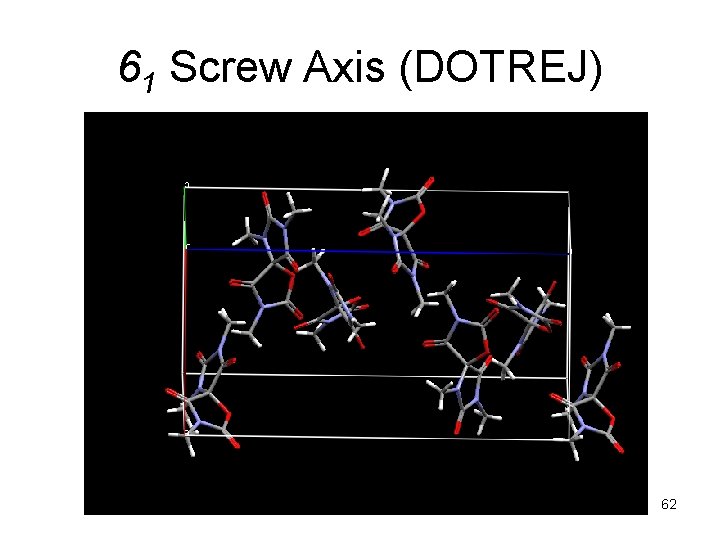
61 Screw Axis (DOTREJ) 62

62 Screw Axis (BHPETS 10) 63

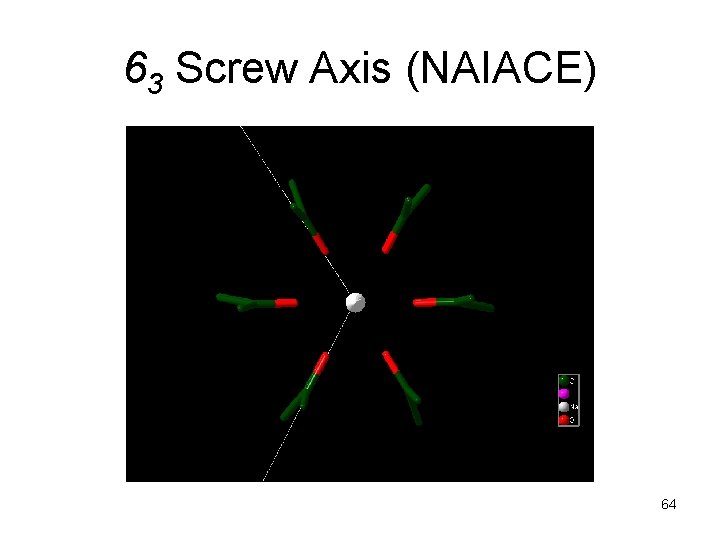
63 Screw Axis (NAIACE) 64

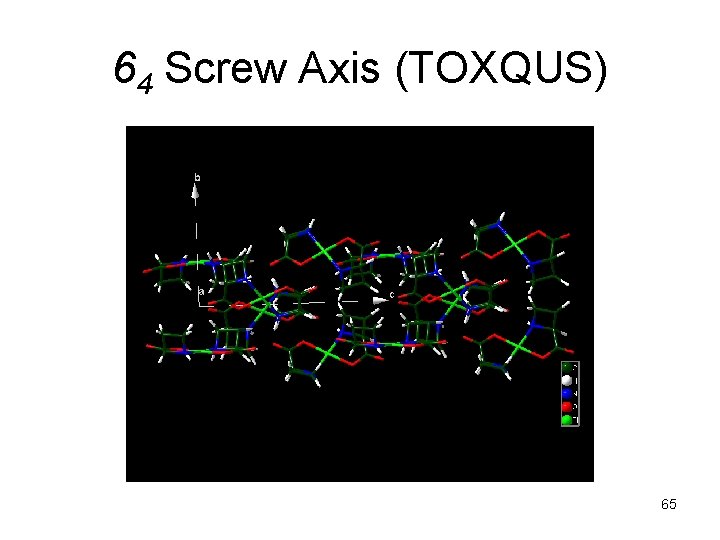
64 Screw Axis (TOXQUS) 65

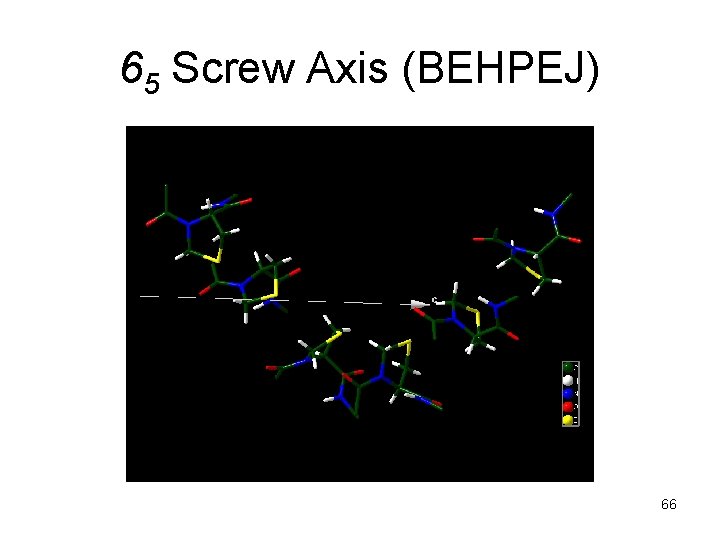
65 Screw Axis (BEHPEJ) 66

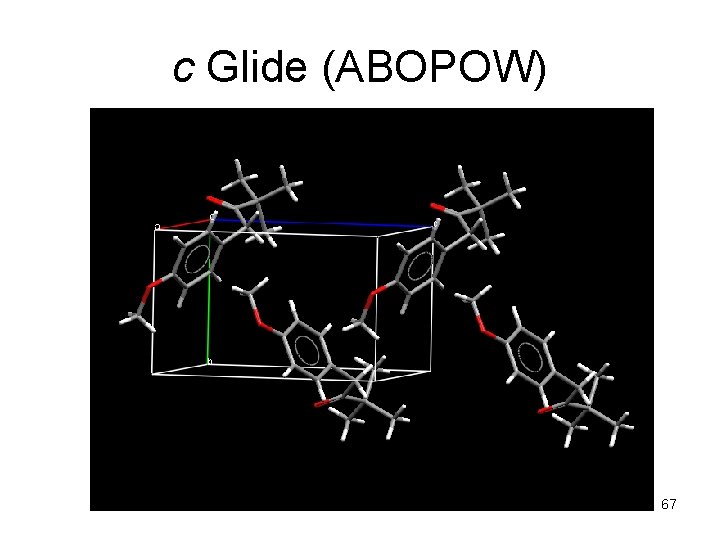
c Glide (ABOPOW) 67

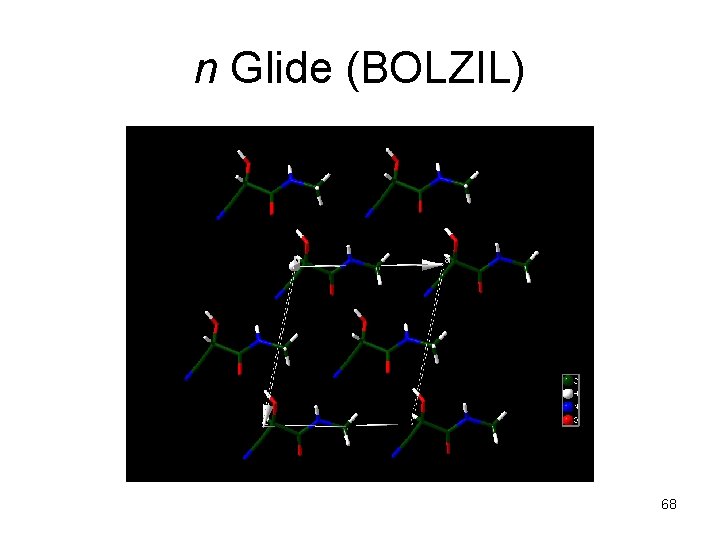
n Glide (BOLZIL) 68

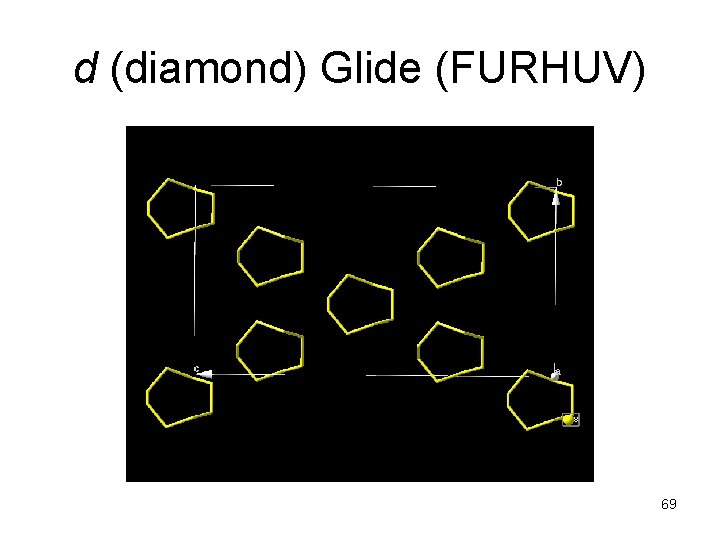
d (diamond) Glide (FURHUV) 69

What does all this mean? 70

Symmetry information is tabulated in International Tables for Crystallography, Volume A edited by Theo Hahn Fifth Edition 2002 71

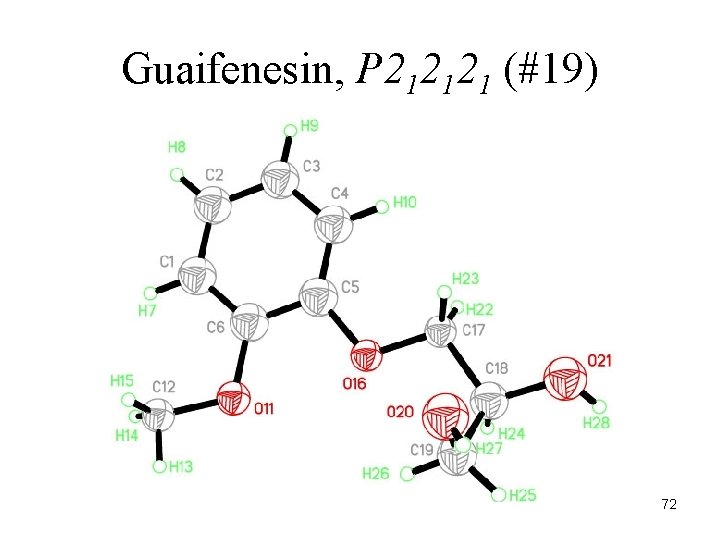
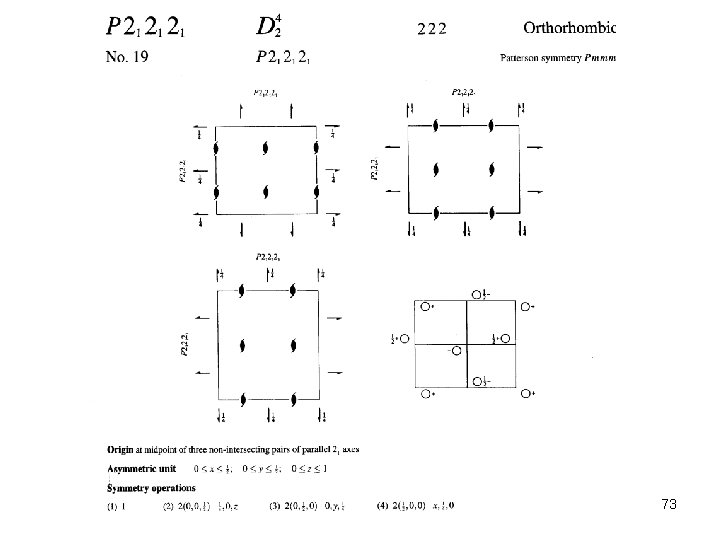
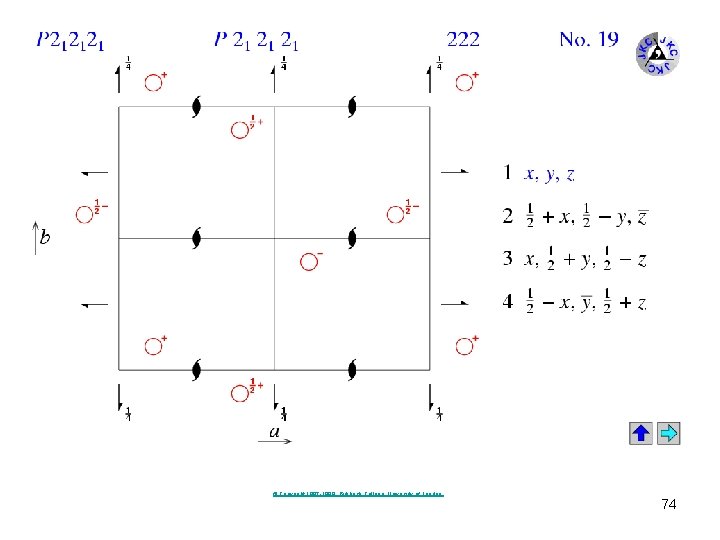
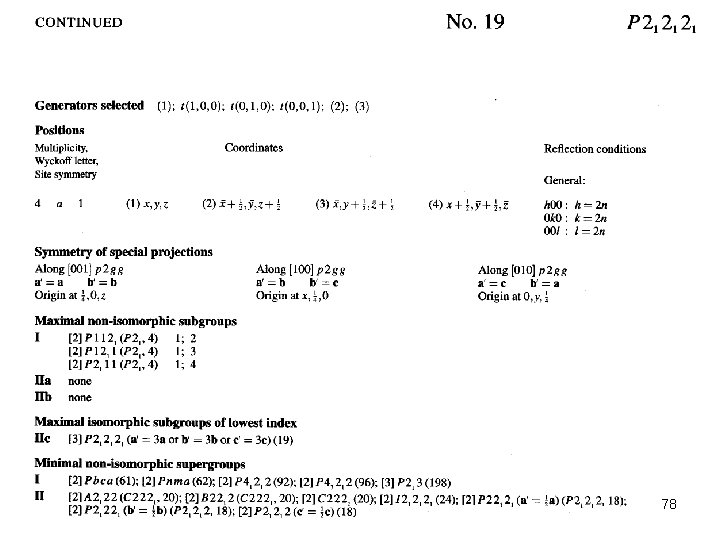
Guaifenesin, P 212121 (#19) 72

73

© Copyright 1997 -1999. Birkbeck College, University of London. 74

Hermann-Mauguin Space Group Symbols the centering, and then a set of characters indicating the symmetry elements along the symmetry directions Lattice Primary Triclinic None Monoclinic unique (b or c) Orthorhombic [100] Tetragonal [001] Hexagonal [001] Rhom. (hex) [001] Rhom. (rho) [111] Cubic {100} Secondary Tertiary [010] {100} {1 -10} {111} [001] {110} 75

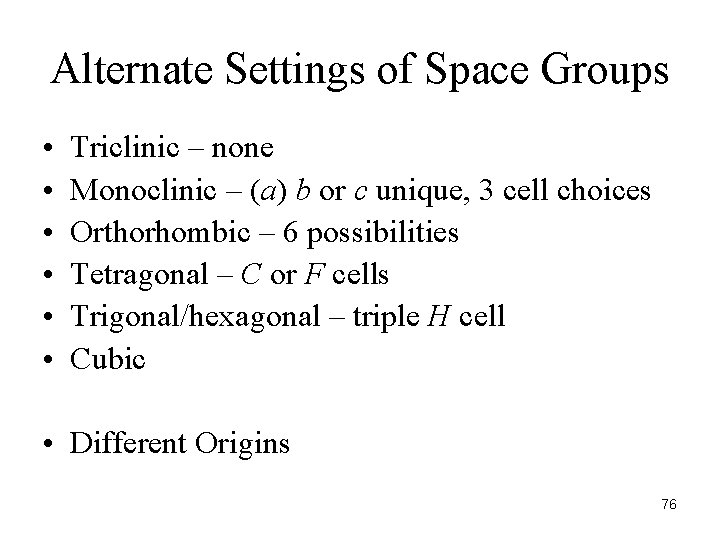
Alternate Settings of Space Groups • • • Triclinic – none Monoclinic – (a) b or c unique, 3 cell choices Orthorhombic – 6 possibilities Tetragonal – C or F cells Trigonal/hexagonal – triple H cell Cubic • Different Origins 76

An Asymmetric Unit A simply-connected smallest closed volume which, by application of all symmetry operations, fills all space. It contains all the information necessary for a complete description of the crystal structure. 77

78

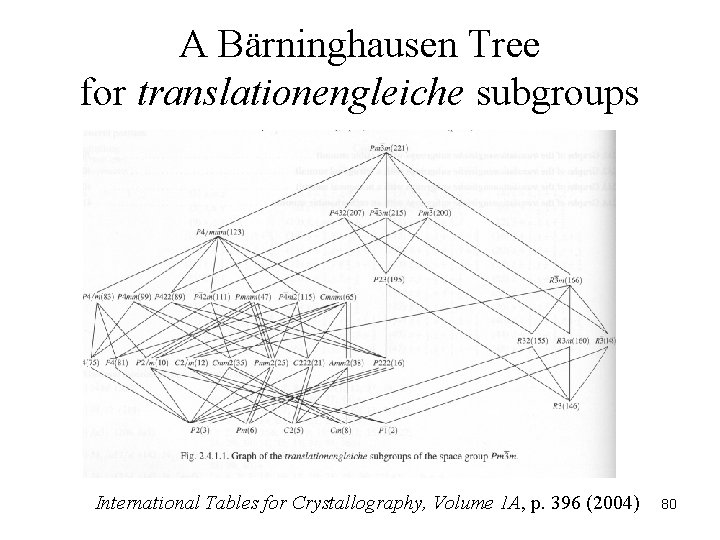
Sub- and Super-Groups • • Phase transitions (second-order) Overlooked symmetry Relations between crystal structures Subgroups – Translationengleiche (keep translations, lose class) – Klassengleiche (lose translations, keep class) – General (lose translations and class) 79

A Bärninghausen Tree for translationengleiche subgroups International Tables for Crystallography, Volume 1 A, p. 396 (2004) 80

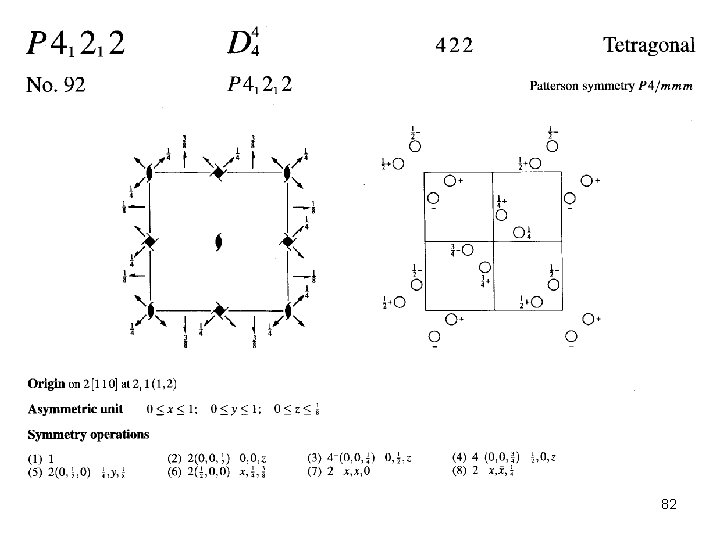
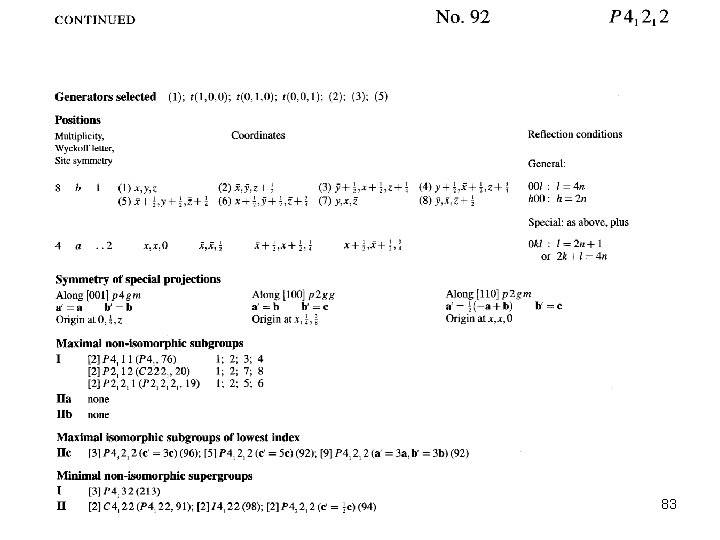
Mercury/ETGUAN (P 41212 #92) 81

82

83

Not all space groups are possible for protein crystals. 84

85

86

Some Classifications of Space Groups • Enantiomorphic, chiral, or dissymmetric – absence of improper rotations (including , = m, and ) • Polar – two directional senses are geometrically or physically different 87
 Diffraction and scattering
Diffraction and scattering Scattering cross section in nuclear physics
Scattering cross section in nuclear physics 7 crystal systems and 14 bravais lattices
7 crystal systems and 14 bravais lattices Cubic void
Cubic void State and prove isotonicity property in a lattice
State and prove isotonicity property in a lattice Bravais lattices
Bravais lattices What is bravais lattice
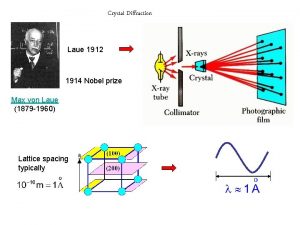
What is bravais lattice Bragg's equation class 12
Bragg's equation class 12 Glide reflection
Glide reflection Giant ionic crystal lattice structure
Giant ionic crystal lattice structure Empty lattice model
Empty lattice model 14 bravais lattices
14 bravais lattices Oded regev
Oded regev Oded regev lattices
Oded regev lattices Ionic bonds hardness
Ionic bonds hardness Lattices definition
Lattices definition Rotational symmetry diagram
Rotational symmetry diagram David carpenter serial killer
David carpenter serial killer James russell odom and james clayton lawson
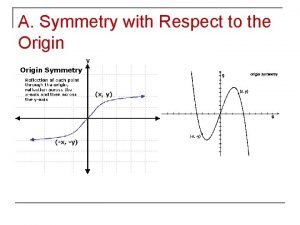
James russell odom and james clayton lawson With respect to the origin
With respect to the origin Brightness and contrast
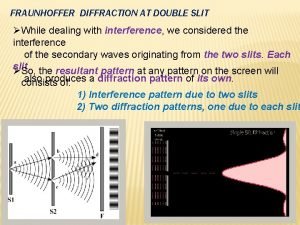
Brightness and contrast Fraunhofer and fresnel diffraction
Fraunhofer and fresnel diffraction Fresnel and fraunhofer diffraction difference
Fresnel and fraunhofer diffraction difference Diffraction pattern
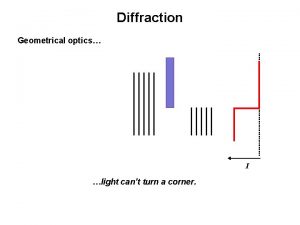
Diffraction pattern How are refraction and diffraction similar behaviors
How are refraction and diffraction similar behaviors Fresnel and fraunhofer diffraction difference
Fresnel and fraunhofer diffraction difference Difference between fresnel and fraunhofer diffraction
Difference between fresnel and fraunhofer diffraction Bragg's law in reciprocal lattice formula
Bragg's law in reciprocal lattice formula Zimm plot excel
Zimm plot excel Scattering of light in suspension
Scattering of light in suspension Scattering in a central force field
Scattering in a central force field Rayleigh vs mie
Rayleigh vs mie Mie scattering vs rayleigh
Mie scattering vs rayleigh Coherent scattering
Coherent scattering Abcd matrix transmission line
Abcd matrix transmission line Dynamic scattering type lcd
Dynamic scattering type lcd Scattering reaction
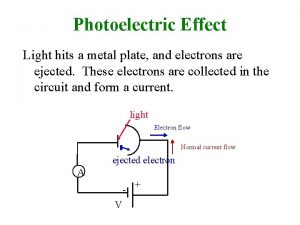
Scattering reaction Photoelectric effect vs compton scattering
Photoelectric effect vs compton scattering Polarisation by scattering
Polarisation by scattering Conservation of momentum
Conservation of momentum Reciprocal network
Reciprocal network Scattering of light definition
Scattering of light definition Double scattering
Double scattering Contoh refraksi gelombang
Contoh refraksi gelombang Raman scattering definition
Raman scattering definition Coherent scattering
Coherent scattering Dynamic light scattering 원리
Dynamic light scattering 원리 Rayleigh theory of light scattering
Rayleigh theory of light scattering Elastic scattering vs inelastic
Elastic scattering vs inelastic Rutherford scattering
Rutherford scattering Rayleigh theory of light scattering
Rayleigh theory of light scattering Scattering matrix
Scattering matrix Pauli blocking of light scattering in degenerate fermions
Pauli blocking of light scattering in degenerate fermions Magic t
Magic t Double parton scattering
Double parton scattering Scattering efficiency
Scattering efficiency Scattering matrix
Scattering matrix Scattering of light
Scattering of light Scattering di rutherford
Scattering di rutherford Phase doppler anemometry
Phase doppler anemometry Golite trig 2
Golite trig 2 Photoelectric effect สรุป
Photoelectric effect สรุป Medium energy ion scattering
Medium energy ion scattering Compton scattering
Compton scattering Vertical
Vertical Boltzmann law
Boltzmann law Rayleigh scattering intensity formula
Rayleigh scattering intensity formula Free space optics
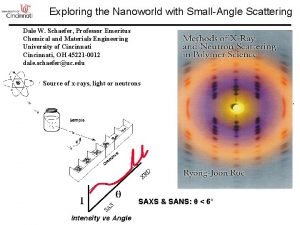
Free space optics Small angle scattering
Small angle scattering Coherent and incoherent sources of light
Coherent and incoherent sources of light Reflection refraction diffraction interference
Reflection refraction diffraction interference Reflection refraction diffraction
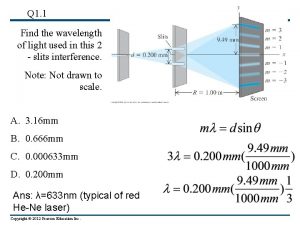
Reflection refraction diffraction Multiple slits diffraction
Multiple slits diffraction Magnets for neutron diffraction
Magnets for neutron diffraction Fraunhoffer diffraction
Fraunhoffer diffraction Dispersive power of grating is
Dispersive power of grating is Sound diffraction
Sound diffraction Diffraction of light
Diffraction of light Missing order in diffraction
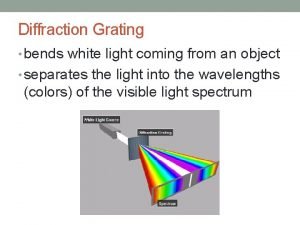
Missing order in diffraction Diffraction grating
Diffraction grating Diffraction in a sentence
Diffraction in a sentence Hkl
Hkl For viewing tiny objects in a microscope, diffraction is
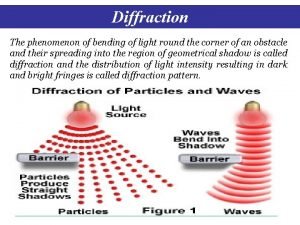
For viewing tiny objects in a microscope, diffraction is Diffraction examples
Diffraction examples Powder diffraction database
Powder diffraction database Laser diffraction spectroscopy
Laser diffraction spectroscopy Diffraction of waves
Diffraction of waves Diffraction grating
Diffraction grating