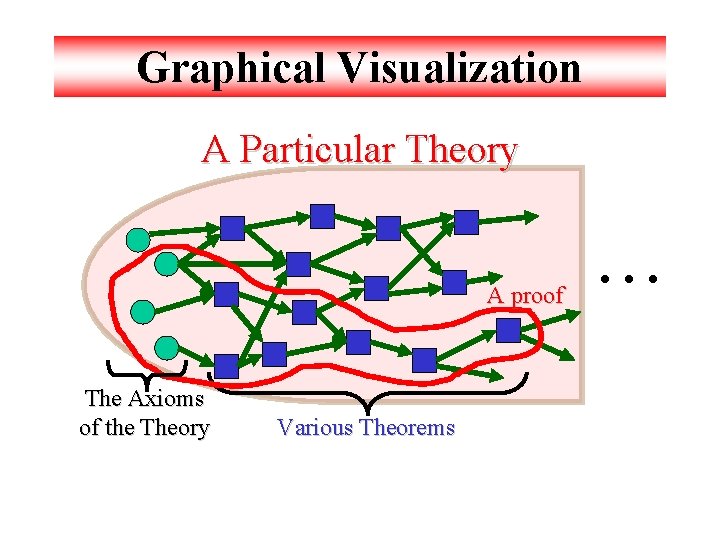
Graphical Visualization A Particular Theory A proof The


















- Slides: 38







Graphical Visualization A Particular Theory A proof The Axioms of the Theory Various Theorems …




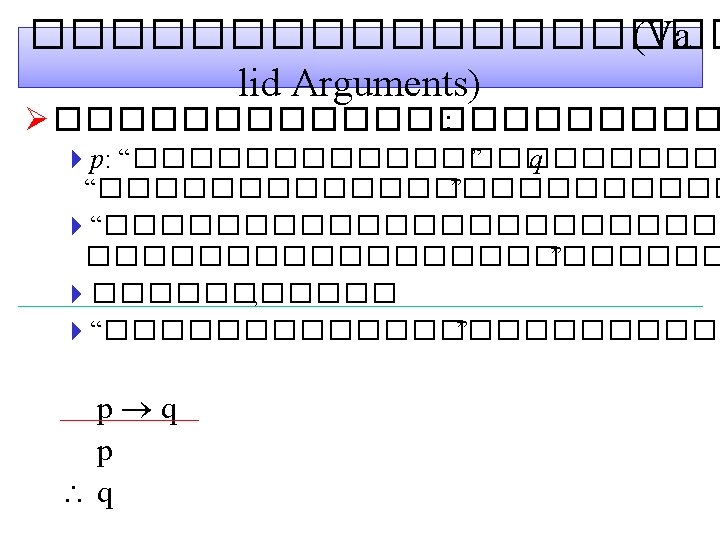
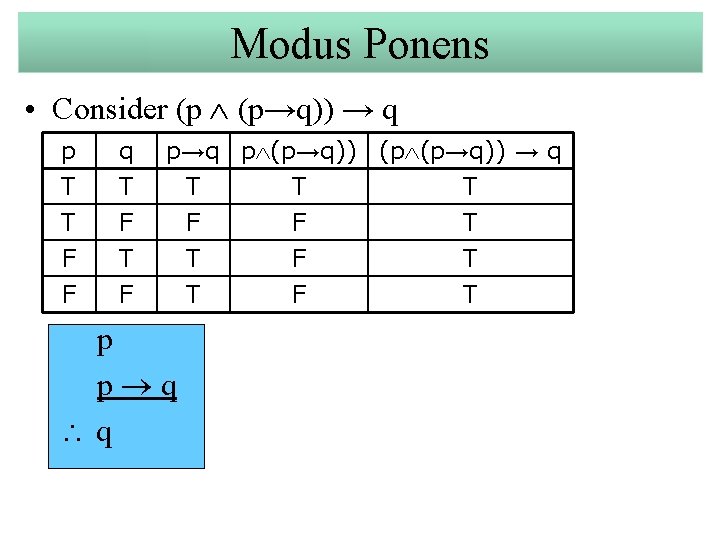
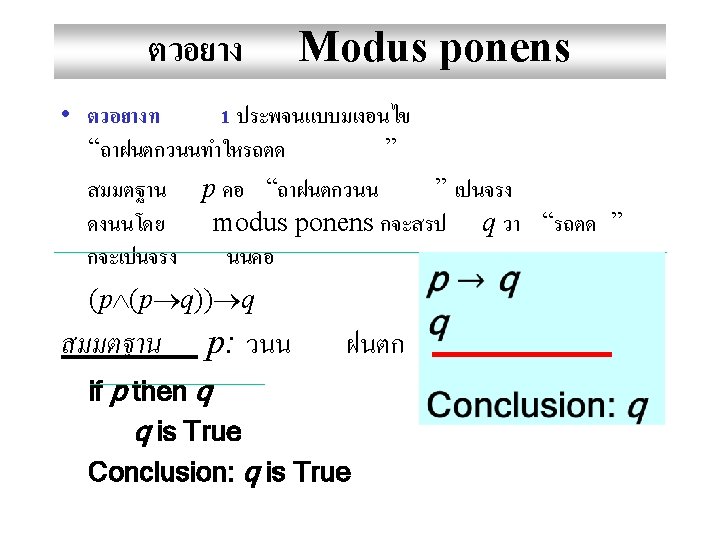
Modus Ponens • Consider (p (p→q)) → q p T T F q T F F p→q p (p→q)) (p (p→q)) → q T T T F F T T F T p p q q T F T


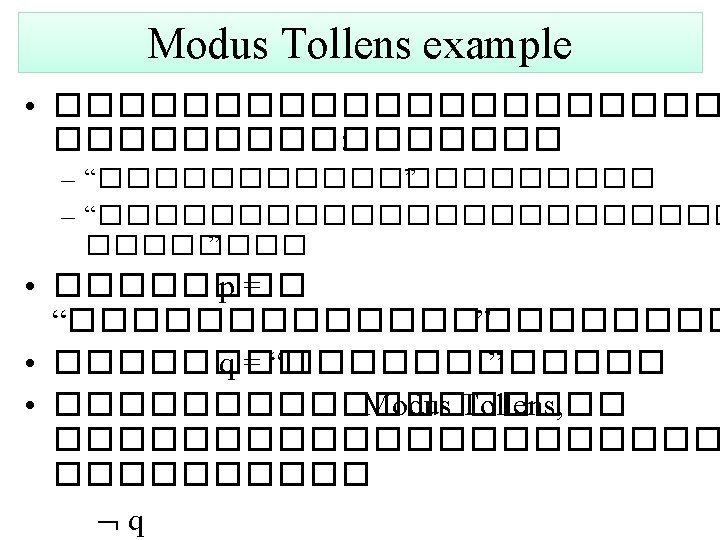
Modus Tollens • Assume that we know: ¬q and p → q – Recall that p → q ¬q → ¬p • Thus, we know ¬q and ¬q → ¬p • We can conclude ¬p q p


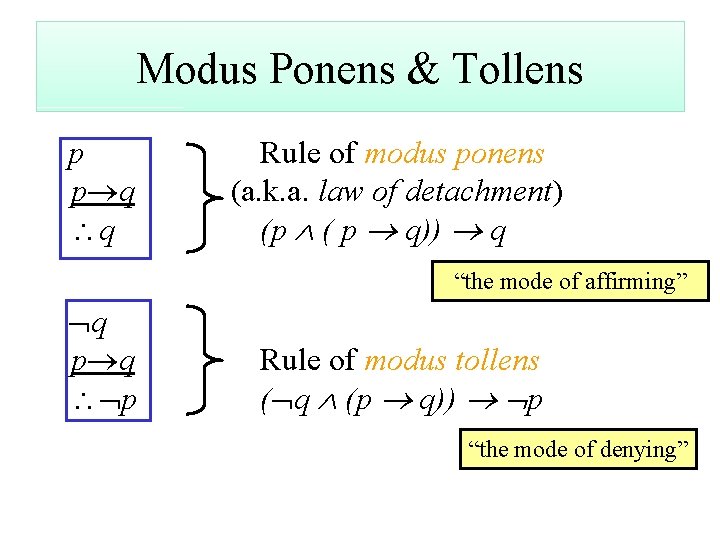
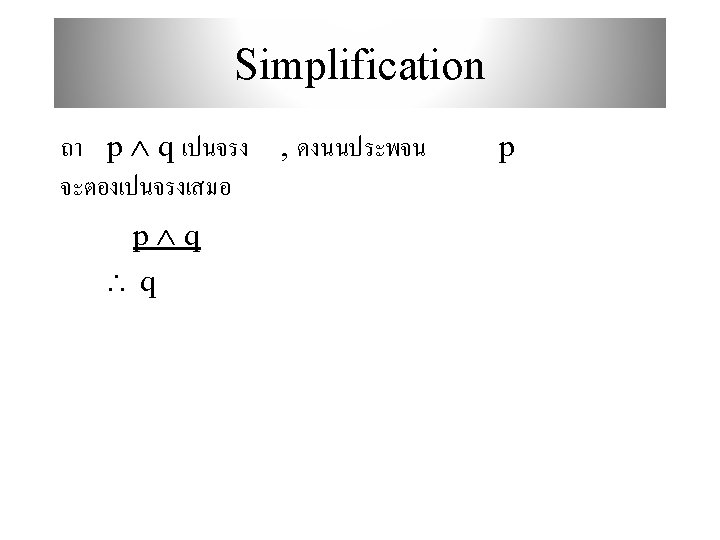
Modus Ponens & Tollens p p q q Rule of modus ponens (a. k. a. law of detachment) (p ( p q)) q “the mode of affirming” q p q p Rule of modus tollens ( q (p q)) p “the mode of denying”




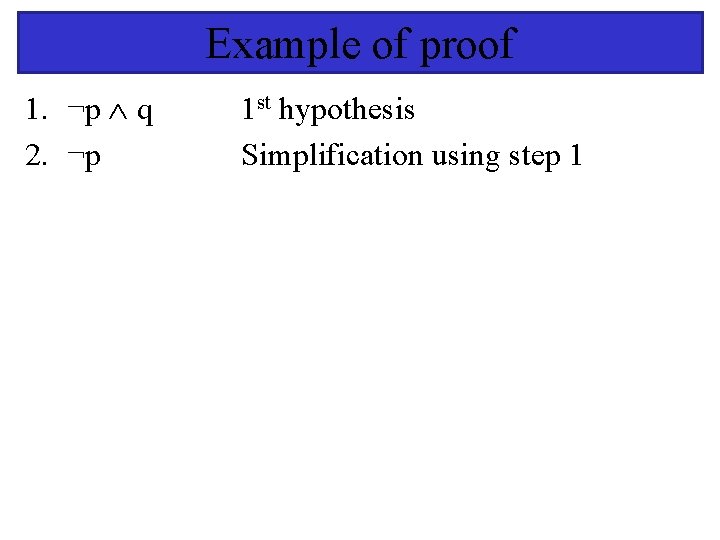
Example of proof 1. ¬p q 2. ¬p 1 st hypothesis Simplification using step 1

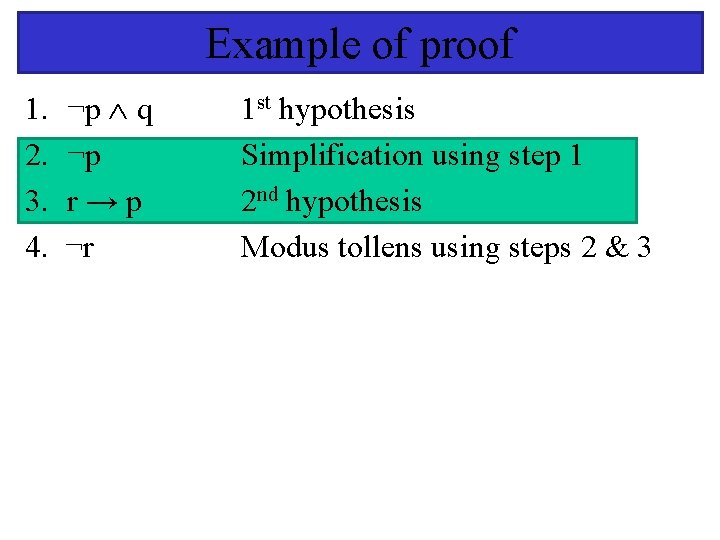
Example of proof 1. ¬p q 2. ¬p 3. r → p 4. ¬r 1 st hypothesis Simplification using step 1 2 nd hypothesis Modus tollens using steps 2 & 3

Example of proof 1. 2. 3. 4. 5. 6. ¬p q ¬p r → p ¬r ¬r → s s 1 st hypothesis Simplification using step 1 2 nd hypothesis Modus tollens using steps 2 & 3 3 rd hypothesis Modus ponens using steps 4 & 5

Example of proof 1. 2. 3. 4. 5. 6. 7. 8. ¬p q ¬p r → p ¬r ¬r → s s s → t t 1 st hypothesis Simplification using step 1 2 nd hypothesis Modus tollens using steps 2 & 3 3 rd hypothesis Modus ponens using steps 4 & 5 4 th hypothesis Modus ponens using steps 6 & 7

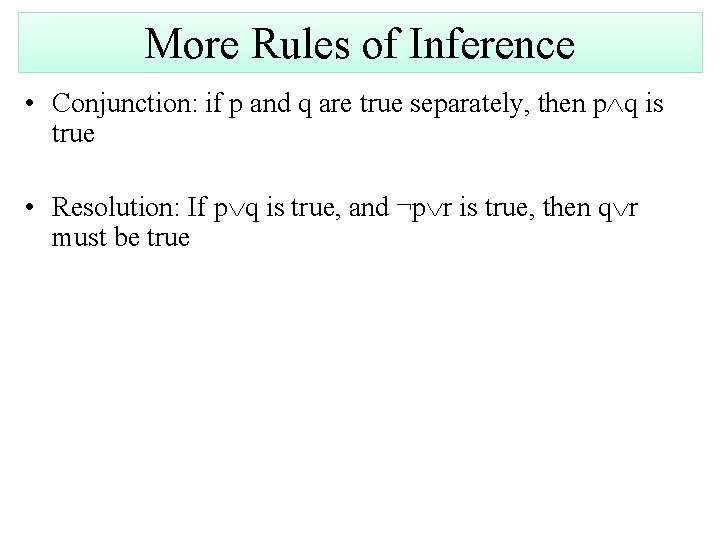
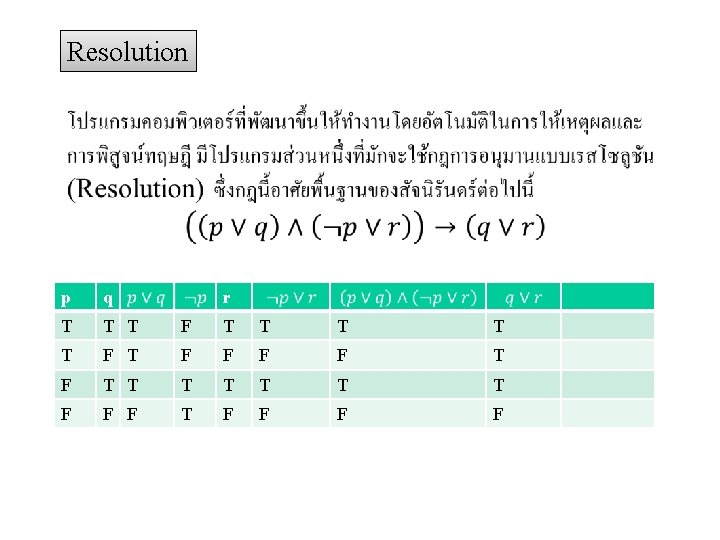
More Rules of Inference • Conjunction: if p and q are true separately, then p q is true • Resolution: If p q is true, and ¬p r is true, then q r must be true

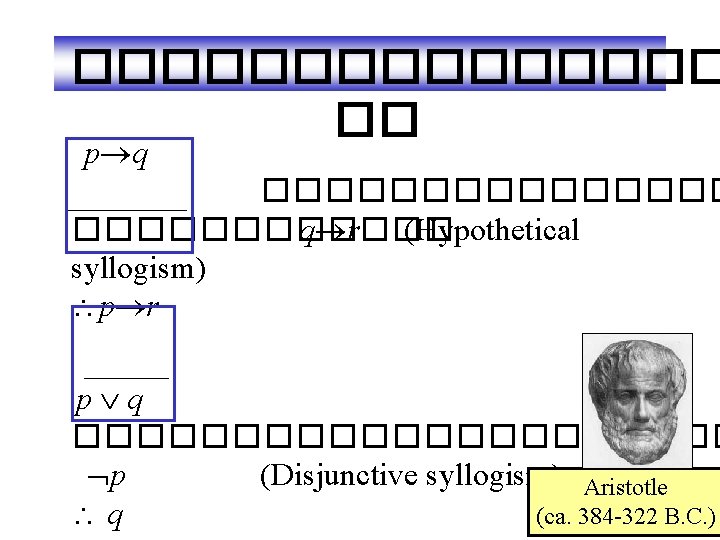
�������� �� p q �������� q r (Hypothetical syllogism) p r p q ����������� p (Disjunctive syllogism) Aristotle (ca. 384 -322 B. C. ) q

ตารางกฎการอนมาน p Modus ponens p q q Hypothetical syllogism p q q r p r Addition p p q Conjunction p q Modus tollens q p q p Disjunctive syllogism p q Simplification p q p Resolution p q p r q r

Resolution p q r T T T F F F F T T T T F F F T F F


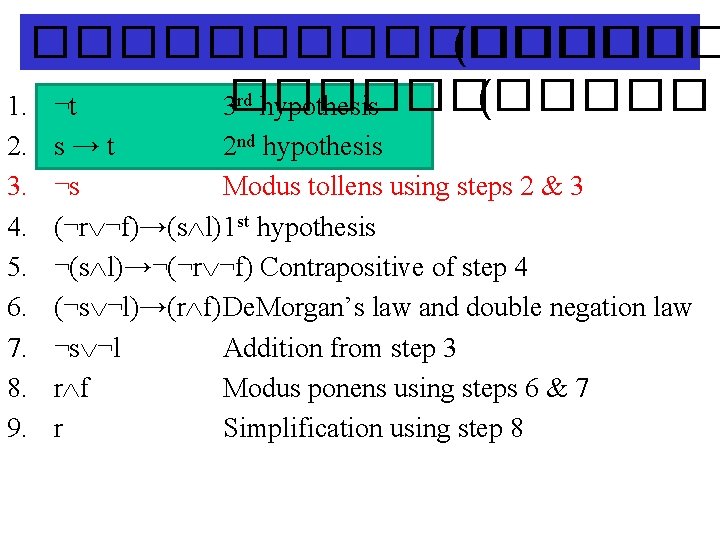
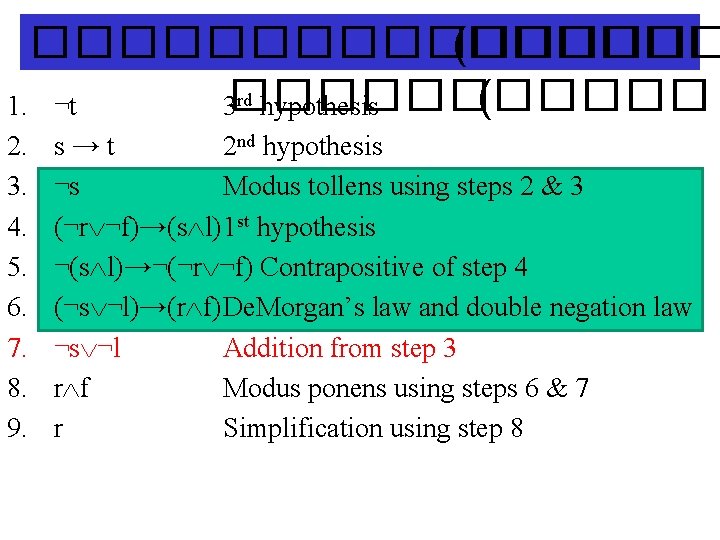
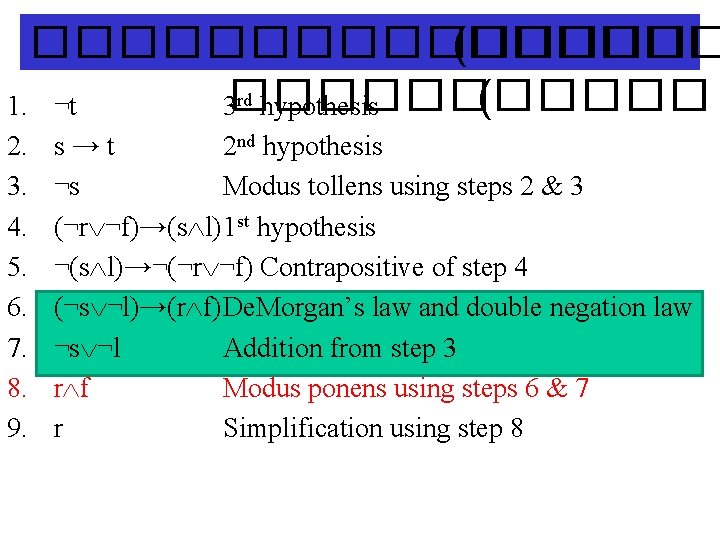
�������� (������ rd hypothesis ( 1. ¬t 3������ 2. 3. 4. 5. 6. 7. 8. 9. s → t 2 nd hypothesis ¬s Modus tollens using steps 2 & 3 (¬r ¬f)→(s l)1 st hypothesis ¬(s l)→¬(¬r ¬f) Contrapositive of step 4 (¬s ¬l)→(r f)De. Morgan’s law and double negation law ¬s ¬l Addition from step 3 r f Modus ponens using steps 6 & 7 r Simplification using step 8

�������� (������ rd hypothesis ( 1. ¬t 3������ 2. 3. 4. 5. 6. 7. 8. 9. s → t 2 nd hypothesis ¬s Modus tollens using steps 2 & 3 (¬r ¬f)→(s l)1 st hypothesis ¬(s l)→¬(¬r ¬f) Contrapositive of step 4 (¬s ¬l)→(r f)De. Morgan’s law and double negation law ¬s ¬l Addition from step 3 r f Modus ponens using steps 6 & 7 r Simplification using step 8

�������� (������ rd hypothesis ( 1. ¬t 3������ 2. 3. 4. 5. 6. 7. 8. 9. s → t 2 nd hypothesis ¬s Modus tollens using steps 2 & 3 (¬r ¬f)→(s l)1 st hypothesis ¬(s l)→¬(¬r ¬f) Contrapositive of step 4 (¬s ¬l)→(r f)De. Morgan’s law and double negation law ¬s ¬l Addition from step 3 r f Modus ponens using steps 6 & 7 r Simplification using step 8

�������� (������ rd hypothesis ( 1. ¬t 3������ 2. 3. 4. 5. 6. 7. 8. 9. s → t 2 nd hypothesis ¬s Modus tollens using steps 2 & 3 (¬r ¬f)→(s l)1 st hypothesis ¬(s l)→¬(¬r ¬f) Contrapositive of step 4 (¬s ¬l)→(r f)De. Morgan’s law and double negation law ¬s ¬l Addition from step 3 r f Modus ponens using steps 6 & 7 r Simplification using step 8




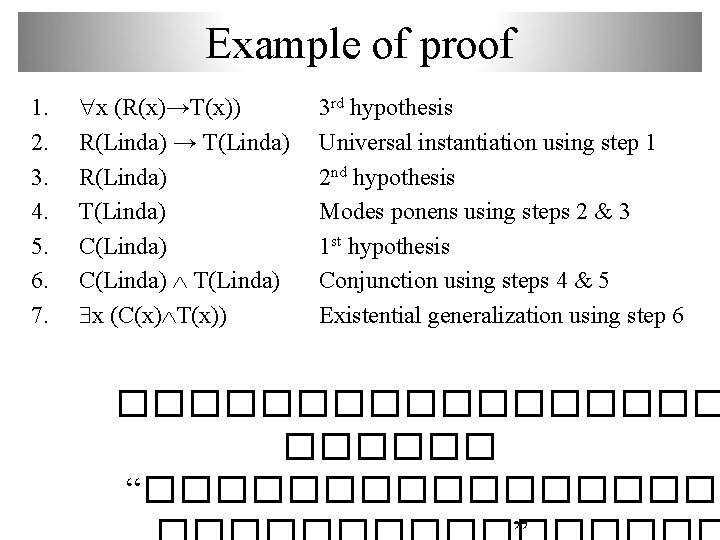
Example of proof 1. 2. 3. 4. 5. 6. 7. x (R(x)→T(x)) R(Linda) → T(Linda) R(Linda) T(Linda) C(Linda) T(Linda) x (C(x) T(x)) 3 rd hypothesis Universal instantiation using step 1 2 nd hypothesis Modes ponens using steps 2 & 3 1 st hypothesis Conjunction using steps 4 & 5 Existential generalization using step 6 ��������� “���������

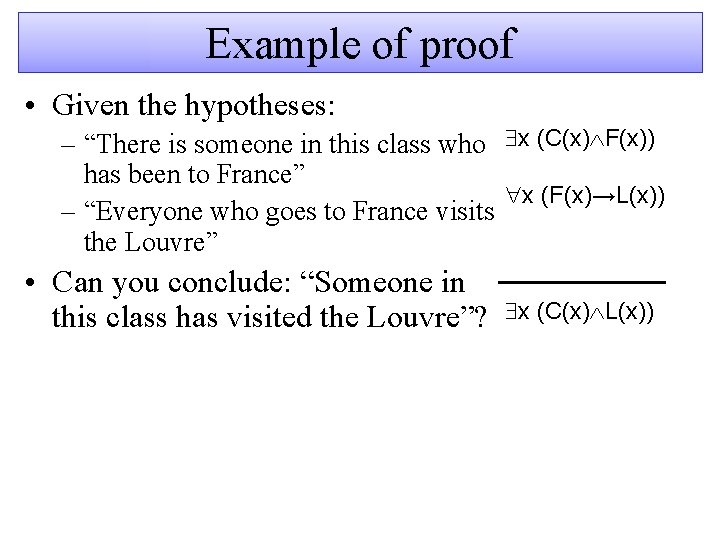
Example of proof • Given the hypotheses: – “There is someone in this class who x (C(x) F(x)) has been to France” x (F(x)→L(x)) – “Everyone who goes to France visits the Louvre” • Can you conclude: “Someone in this class has visited the Louvre”? x (C(x) L(x))

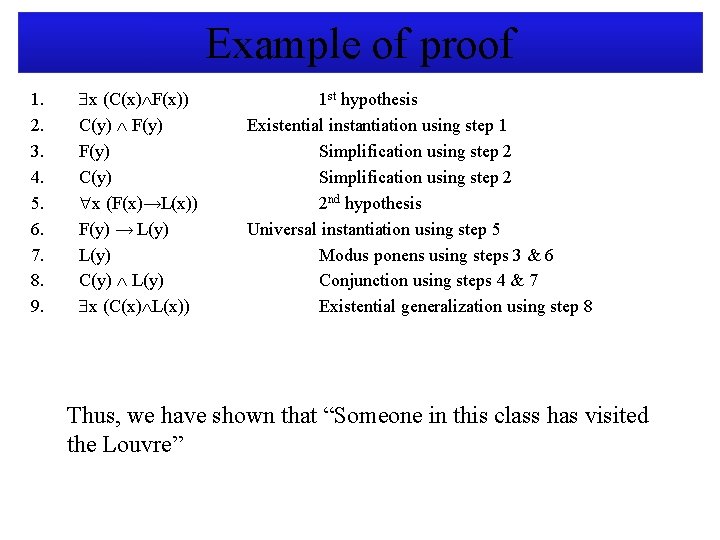
Example of proof 1. 2. 3. 4. 5. 6. 7. 8. 9. x (C(x) F(x)) C(y) F(y) C(y) x (F(x)→L(x)) F(y) → L(y) C(y) L(y) x (C(x) L(x)) 1 st hypothesis Existential instantiation using step 1 Simplification using step 2 2 nd hypothesis Universal instantiation using step 5 Modus ponens using steps 3 & 6 Conjunction using steps 4 & 7 Existential generalization using step 8 Thus, we have shown that “Someone in this class has visited the Louvre”

How do you know which one to use? • Experience! • In general, use quantifiers with statements like “for all” or “there exists”
 Direct proof and indirect proof
Direct proof and indirect proof Direct proof and indirect proof
Direct proof and indirect proof How to write an indirect proof
How to write an indirect proof Direct proof and indirect proof
Direct proof and indirect proof Unit 2 logic and proof algebraic proof worksheet
Unit 2 logic and proof algebraic proof worksheet Unit 2 logic and proof homework 6 algebraic proof day 1
Unit 2 logic and proof homework 6 algebraic proof day 1 Graphical models for game theory
Graphical models for game theory Set identities table
Set identities table Proof of continental drift theory
Proof of continental drift theory Proof of continental drift theory
Proof of continental drift theory Hát kết hợp bộ gõ cơ thể
Hát kết hợp bộ gõ cơ thể Ng-html
Ng-html Bổ thể
Bổ thể Tỉ lệ cơ thể trẻ em
Tỉ lệ cơ thể trẻ em Chó sói
Chó sói Tư thế worm breton
Tư thế worm breton Chúa sống lại
Chúa sống lại Các môn thể thao bắt đầu bằng tiếng nhảy
Các môn thể thao bắt đầu bằng tiếng nhảy Thế nào là hệ số cao nhất
Thế nào là hệ số cao nhất Các châu lục và đại dương trên thế giới
Các châu lục và đại dương trên thế giới Cong thức tính động năng
Cong thức tính động năng Trời xanh đây là của chúng ta thể thơ
Trời xanh đây là của chúng ta thể thơ Cách giải mật thư tọa độ
Cách giải mật thư tọa độ Phép trừ bù
Phép trừ bù Phản ứng thế ankan
Phản ứng thế ankan Các châu lục và đại dương trên thế giới
Các châu lục và đại dương trên thế giới Thơ thất ngôn tứ tuyệt đường luật
Thơ thất ngôn tứ tuyệt đường luật Quá trình desamine hóa có thể tạo ra
Quá trình desamine hóa có thể tạo ra Một số thể thơ truyền thống
Một số thể thơ truyền thống Cái miệng xinh xinh thế chỉ nói điều hay thôi
Cái miệng xinh xinh thế chỉ nói điều hay thôi Vẽ hình chiếu vuông góc của vật thể sau
Vẽ hình chiếu vuông góc của vật thể sau Biện pháp chống mỏi cơ
Biện pháp chống mỏi cơ đặc điểm cơ thể của người tối cổ
đặc điểm cơ thể của người tối cổ V cc
V cc Vẽ hình chiếu đứng bằng cạnh của vật thể
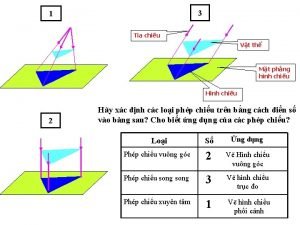
Vẽ hình chiếu đứng bằng cạnh của vật thể Tia chieu sa te
Tia chieu sa te Thẻ vin
Thẻ vin đại từ thay thế
đại từ thay thế điện thế nghỉ
điện thế nghỉ