UNIT 2 Algebraic Proofs A proof is an

UNIT 2 Algebraic Proofs A proof is an argument that uses logic, definitions, properties, and previously proven statements to show that a conclusion is true. An important part of writing a proof is giving justifications to show that every step is valid. 2. 5

UNIT 2 Algebraic Proofs Properties of Equality 2. 5 see page 104 Addition Property………If a=b, then a+c = b+c Subtraction Property…. . If a=b, then a–c = b–c Multiplication Property. . . If a=b, then a • c = b • c Division Property……. . If a=b and c 0, then a c = b c Reflexive Property……. . a = a Symmetric Property……If a = b, then b = a Transitive Property……. If a = b and b = c, then a = c Substitution Property…. If a=b, then b can replace a in any expression. Distributive Property…. . a(b + c) = a • b + a • c not on 104

UNIT 2 Algebraic Proofs 2. 5 Earlier we said geometric figures, like segment and angles are congruent instead of equal (XY WV). Since numbers are different than figures, we must have some properties of congruence for those figures. Properties of Congruence see page 106 Reflexive Property of Congruence……EF EF Symmetric Property of Congruence…. if 1 2 then 2 1 Transitive Property of Congruence…. . . if AB CD and CD EF, then AB EF

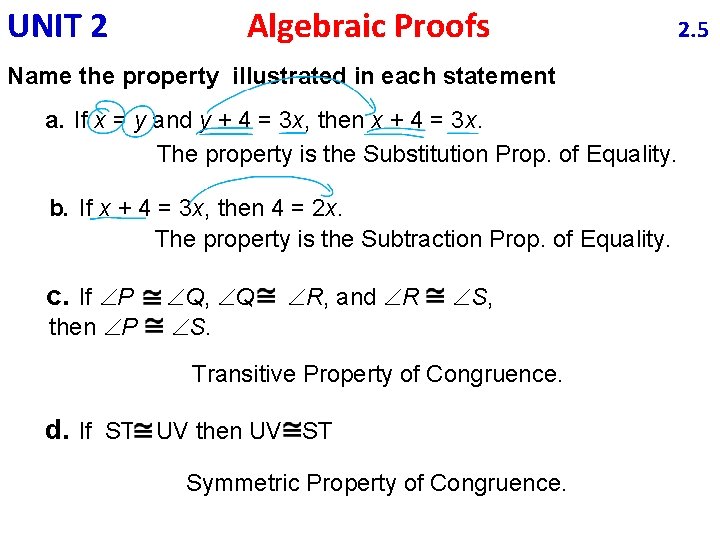
UNIT 2 Algebraic Proofs Name the property illustrated in each statement a. If x = y and y + 4 = 3 x, then x + 4 = 3 x. The property is the Substitution Prop. of Equality. b. If x + 4 = 3 x, then 4 = 2 x. The property is the Subtraction Prop. of Equality. c. If P then P Q, Q S. R, and R S, Transitive Property of Congruence. d. If ST UV then UV ST Symmetric Property of Congruence. 2. 5

UNIT 2 Geometric Reasoning Review properties on page 104 and 106. Then name the property that justifies each statement. 1. If AB = CD, then AB + XY = CD + XY 2. If XD = FY and FY = 12, then XD = 12 3. If XY + JM = GT + XY, then JM = GT 4. If 2(m ABC) = 180, then m ABC = 90 5. RS = RS 6. If m ABC = 25, then 25 = m ABC Use the given property to complete each statement. 7. Symmetric Prop of Eq. If MN = UT, then ____ 8. Div. Prop of Eq. If 4 m QWR= 120, then ____ 9. Transitive Prop of Eq. If SB=VT and VT=MN, then _______ 10. Add. Prop of Eq. If y-15 = 36, then y-10 = _____ 11. Reflexive Prop of Eq. JL ≌ _____ 12. Substitution Prop of Eq. If 2 x+3 a=7 and 3 a=2, then____

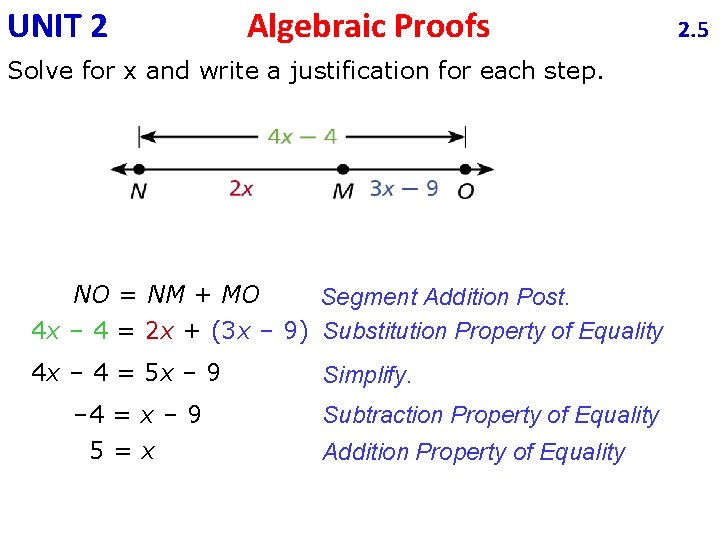
UNIT 2 Algebraic Proofs Solve for x and write a justification for each step. NO = NM + MO Segment Addition Post. 4 x – 4 = 2 x + (3 x – 9) Substitution Property of Equality 4 x – 4 = 5 x – 9 – 4 = x – 9 5=x Simplify. Subtraction Property of Equality Addition Property of Equality 2. 5

UNIT 2 Algebraic Proofs Solve for x and write a justification for each step. ABD + DBC = ABC AAP (3 x + 5) + (6 x – 16) = 8 x Subst 9 x - 11= 8 x Simplify x - 11= 0 SPE x = 11 APE 2. 5

Homework: 2. 5(107): 16 -23(odds), 25 -35(omit 29), 37