Section 7 2 Inverse Transforms and Transforms of




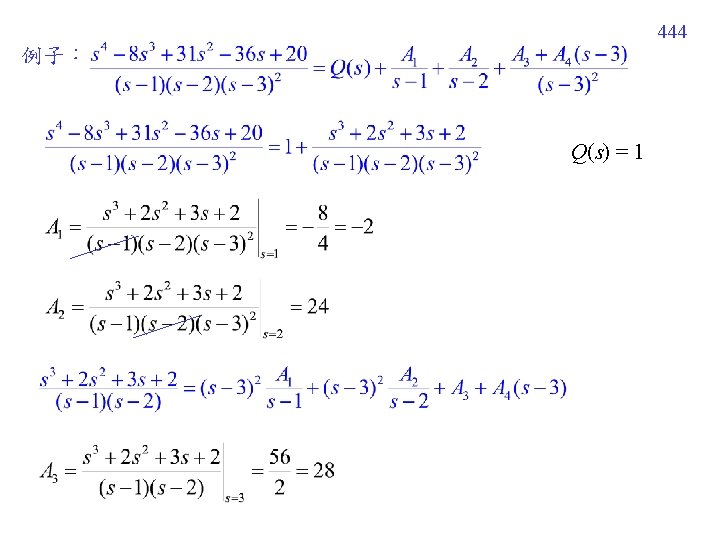




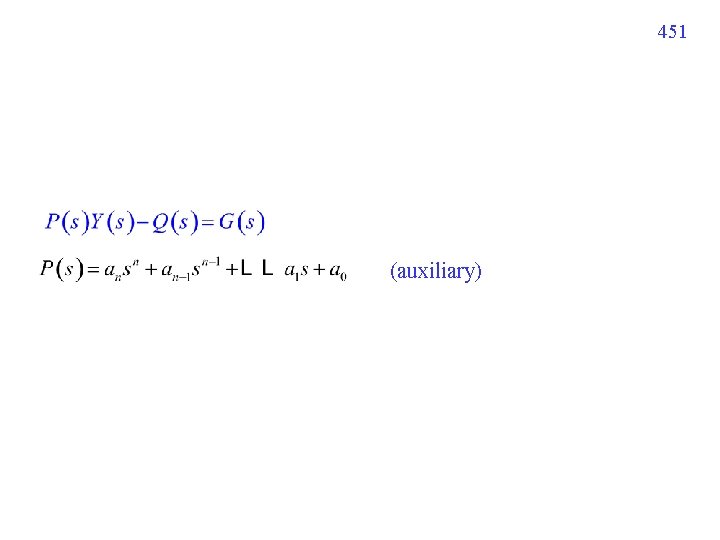
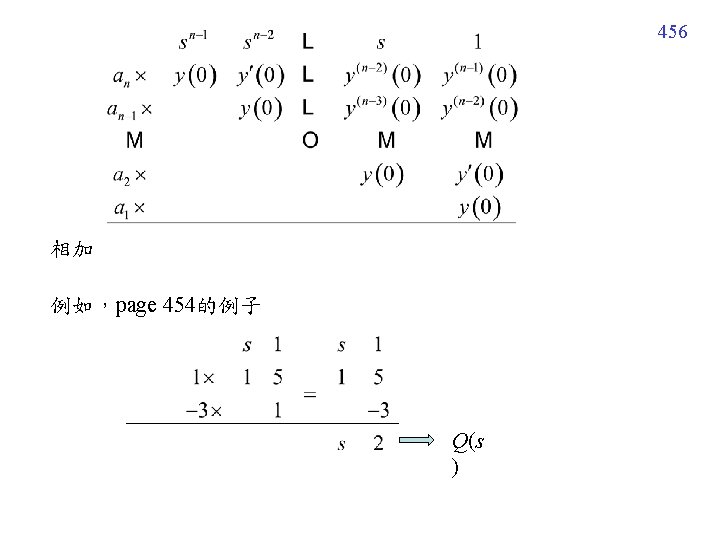



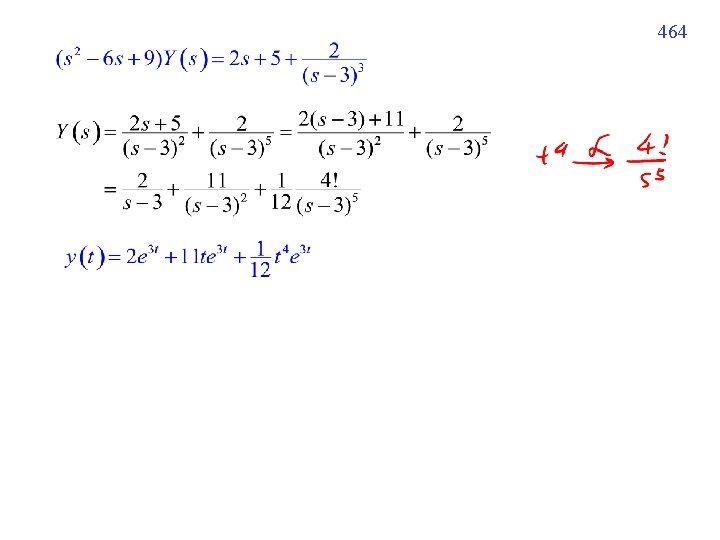


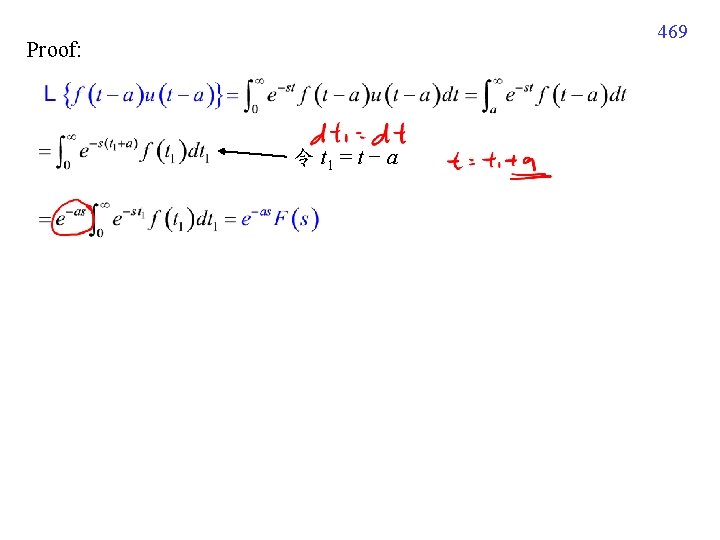





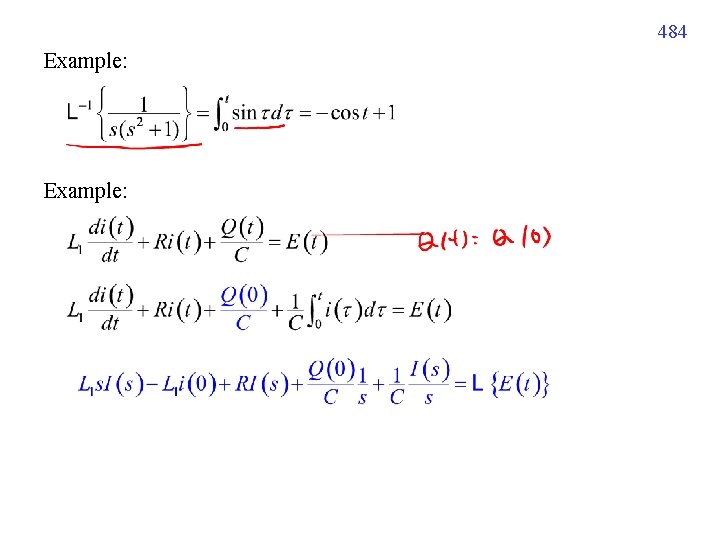
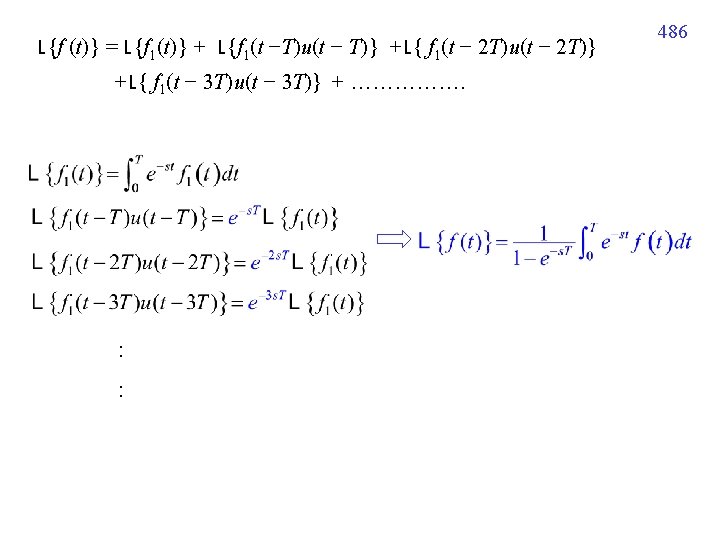
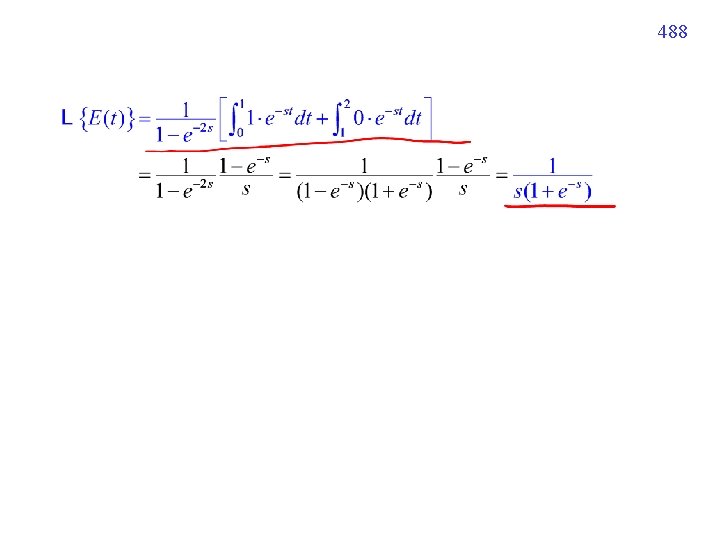






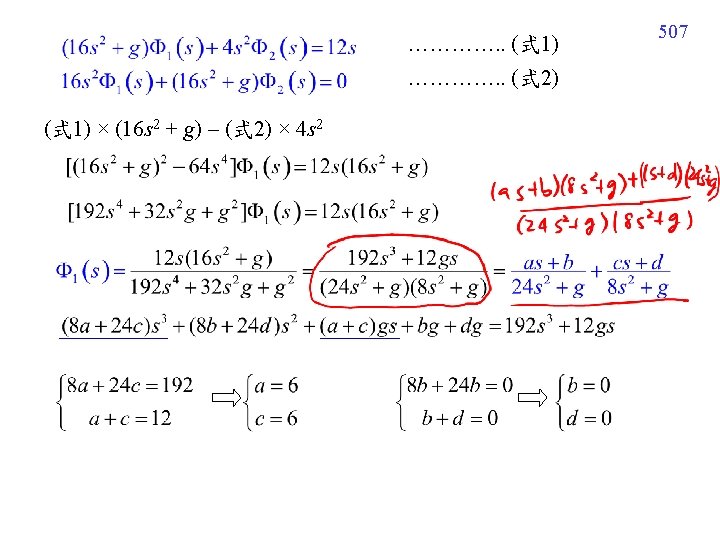
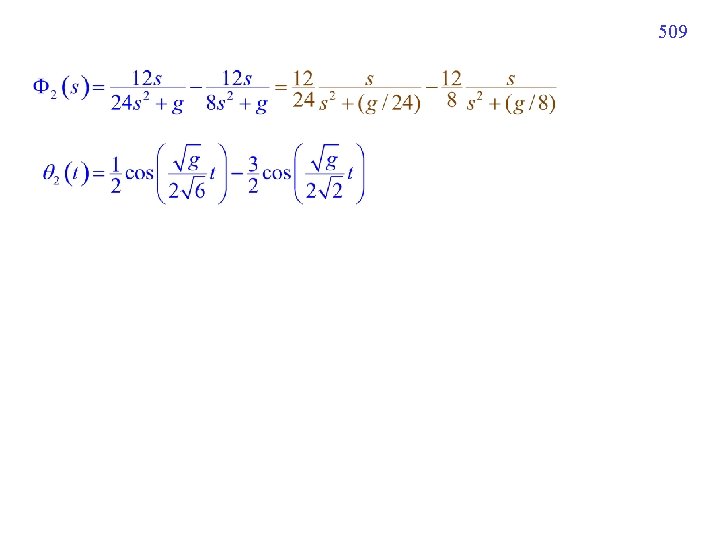






- Slides: 84

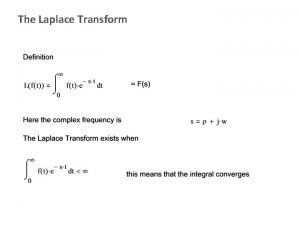
Section 7 -2 Inverse Transforms and Transforms of Derivatives 本節有兩大部分: (1) inverse Laplace transform 的計算 (7 -2 -1 ~ 7 -2 -3) (2) 將微分變成 Laplace transform 當中的乘法 (7 -2 -4 ~ 7 -2 -6) 437

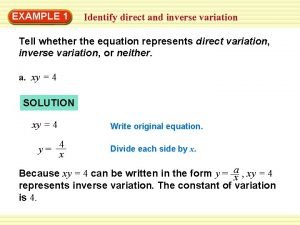
438 7 -2 -1 Inverse 方法一: One-to-One Relation When (1) f 1(t) and f 2(t) are piecewise continuous on [0, ), and (2) f 1(t) and f 2(t) are of exponential order, then if f 1(t) f 2(t) then F 1(s) F 2(s) 換句話說,在這種情形下,Laplace transform 是 one-to-one 的運算。 If the Laplace transform of f 1(t) is F 1(s), then the inverse Laplace transform of F 1(s) must be f 1(t).

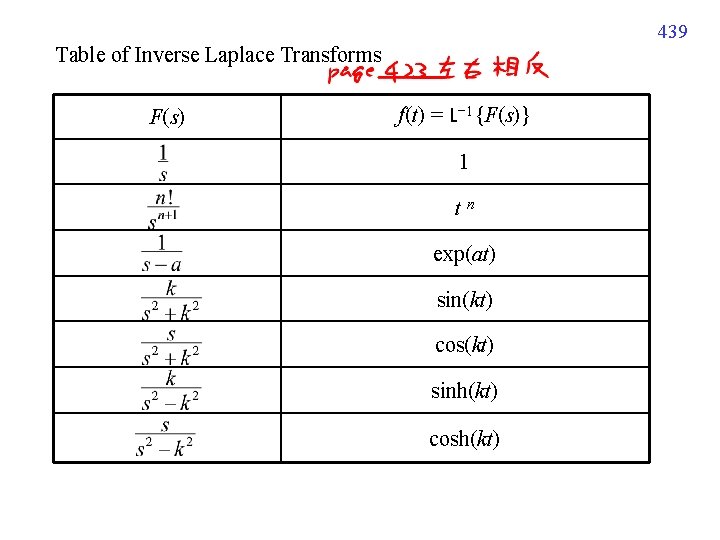
439 Table of Inverse Laplace Transforms F(s) f(t) = L− 1{F(s)} 1 tn exp(at) sin(kt) cos(kt) sinh(kt) cosh(kt)

440 Example 1 (text page 287) (a) Example 2 (text page 287)

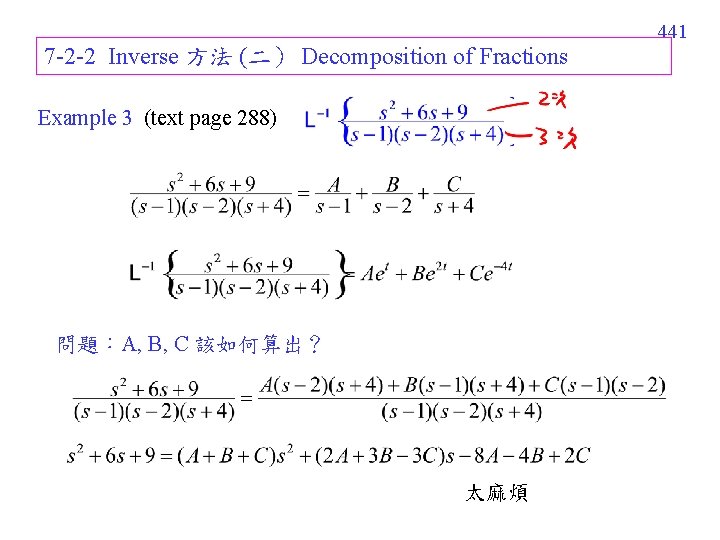
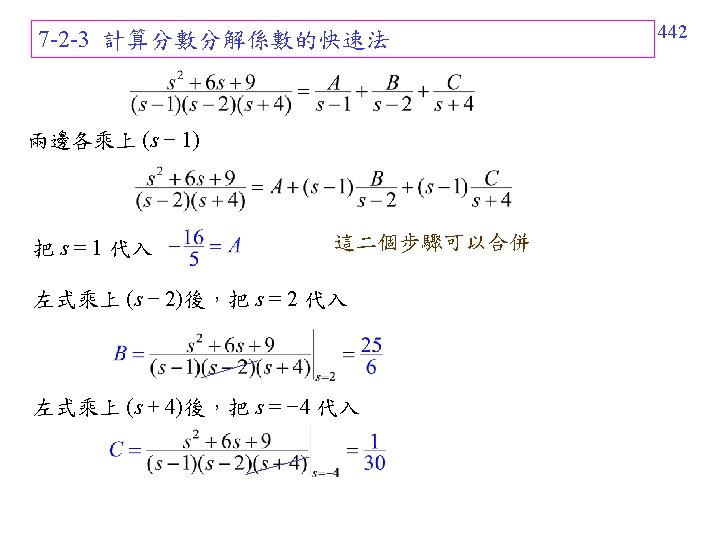
441 7 -2 -2 Inverse 方法 (二) Decomposition of Fractions Example 3 (text page 288) 問題:A, B, C 該如何算出? 太麻煩


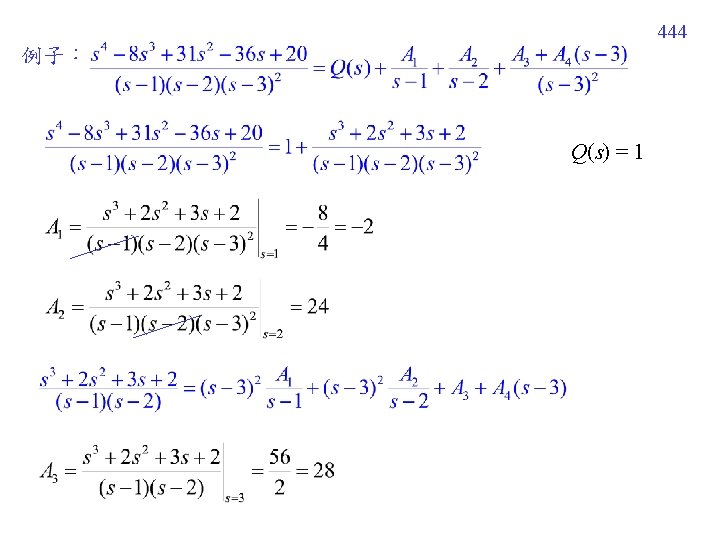

445



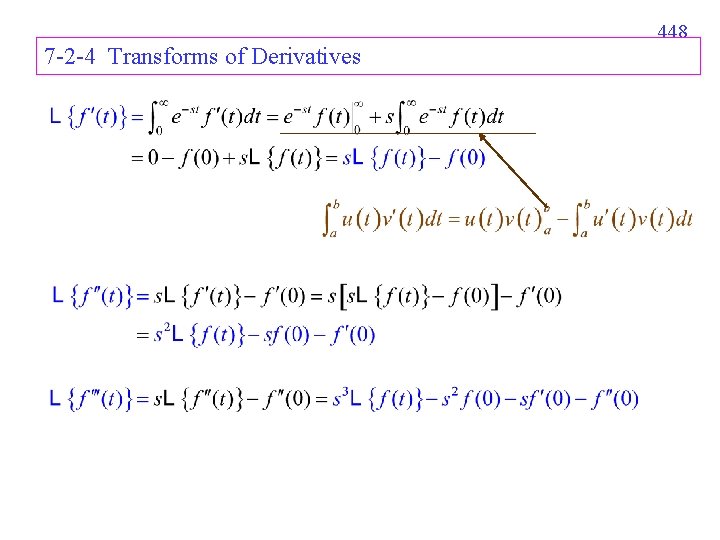
448 7 -2 -4 Transforms of Derivatives

449 Theorem 7. 2. 2 Derivative Property of the Laplace Transform

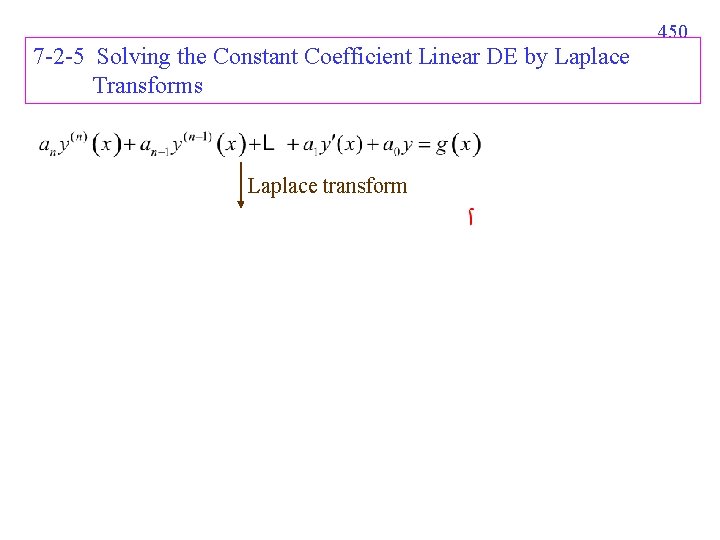
450 7 -2 -5 Solving the Constant Coefficient Linear DE by Laplace Transforms Laplace transform
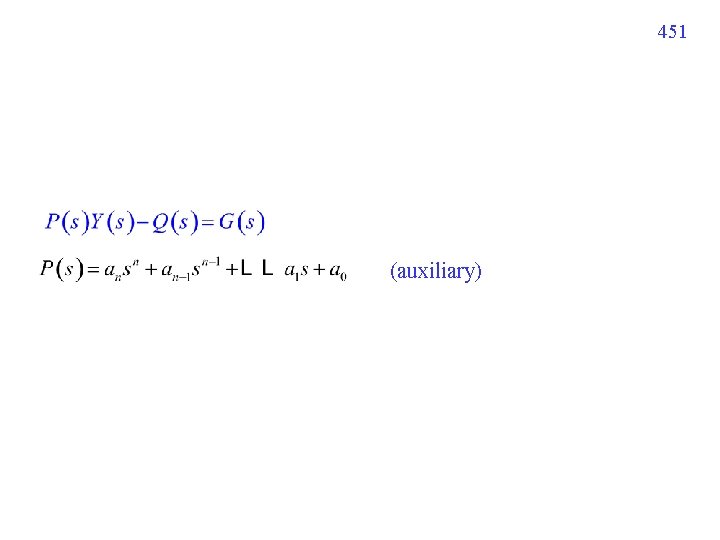
451 (auxiliary)

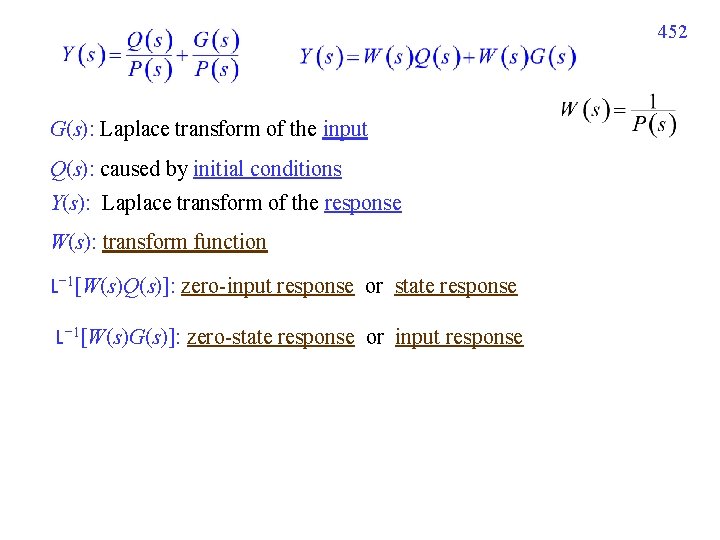
452 G(s): Laplace transform of the input Q(s): caused by initial conditions Y(s): Laplace transform of the response W(s): transform function L− 1[W(s)Q(s)]: zero-input response or state response L− 1[W(s)G(s)]: zero-state response or input response

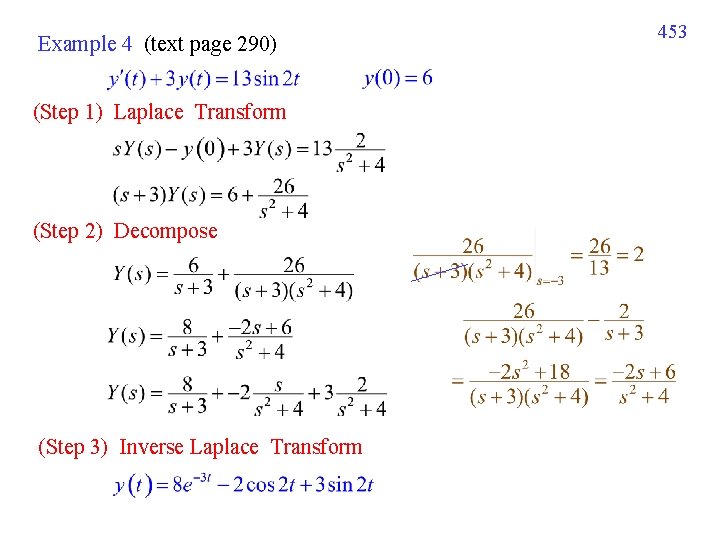
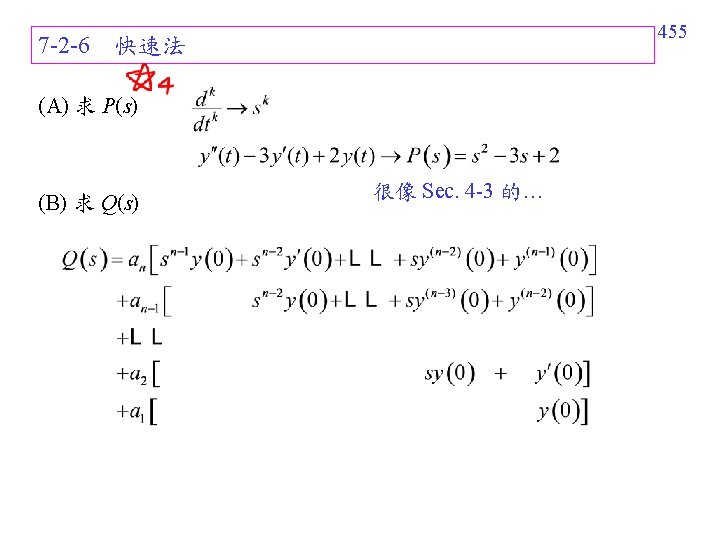
Example 4 (text page 290) (Step 1) Laplace Transform (Step 2) Decompose (Step 3) Inverse Laplace Transform 453

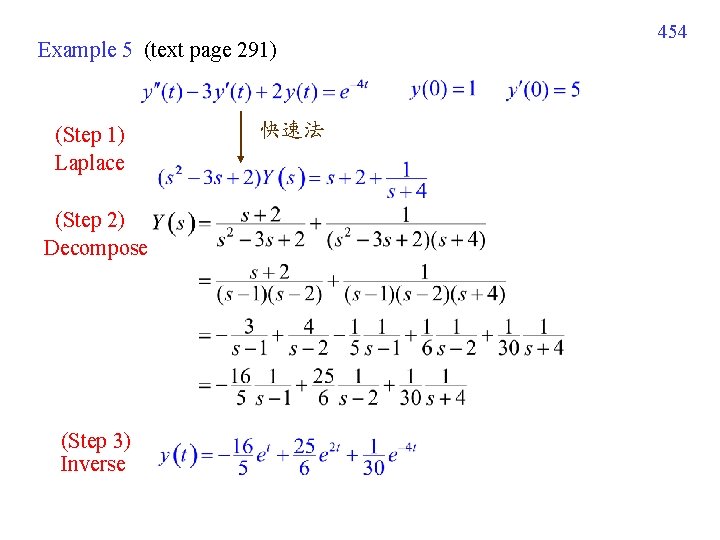
Example 5 (text page 291) (Step 1) Laplace (Step 2) Decompose (Step 3) Inverse 快速法 454

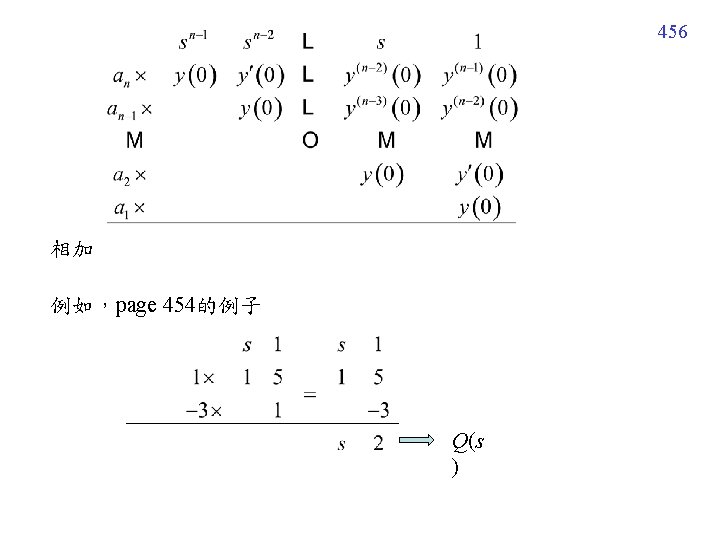


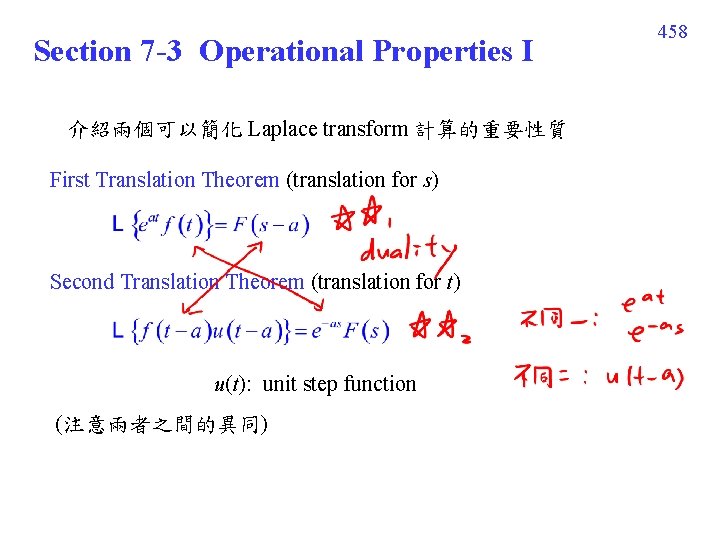
Section 7 -3 Operational Properties I 介紹兩個可以簡化 Laplace transform 計算的重要性質 First Translation Theorem (translation for s) Second Translation Theorem (translation for t) u(t): unit step function (注意兩者之間的異同) 458

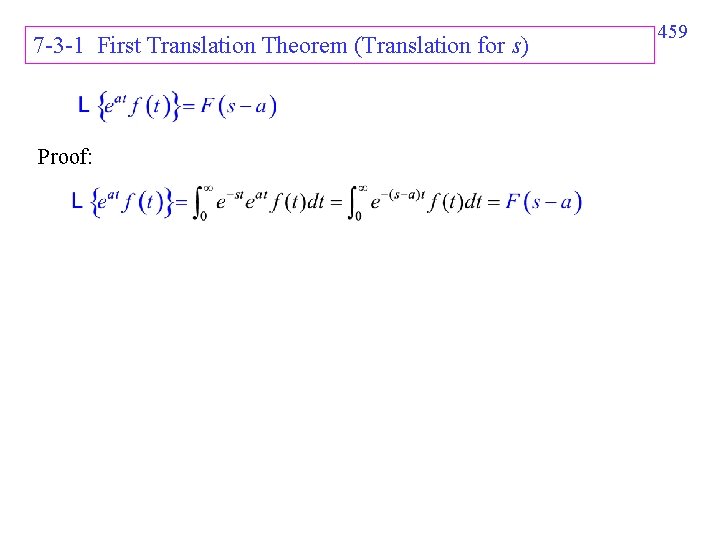
7 -3 -1 First Translation Theorem (Translation for s) Proof: 459

7 -3 -1 -1 Inverse of “Translation for s” When f(t) is piecewise continuous and of exponential order (一對一) 註: Sections 7 -3 和 7 -4 其他的定理亦如此 460

7 -3 -1 -2 Examples Example 1 (text page 295) (a) (b) 461

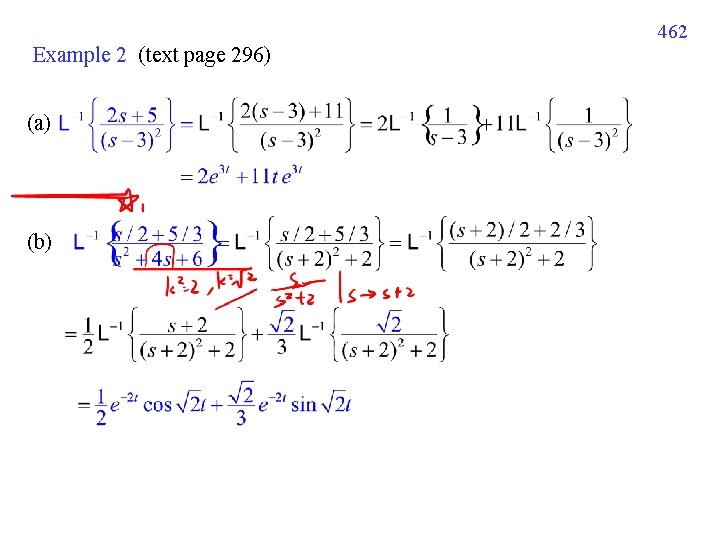
462 Example 2 (text page 296) (a) (b)

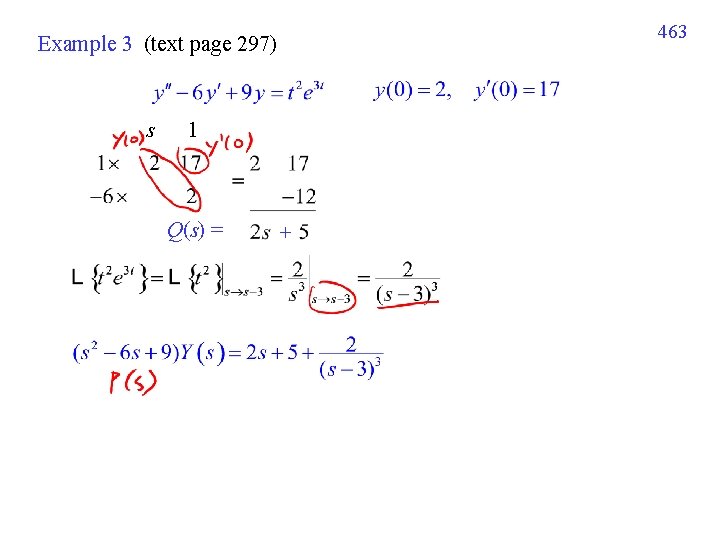
463 Example 3 (text page 297) s 1 Q(s) = +
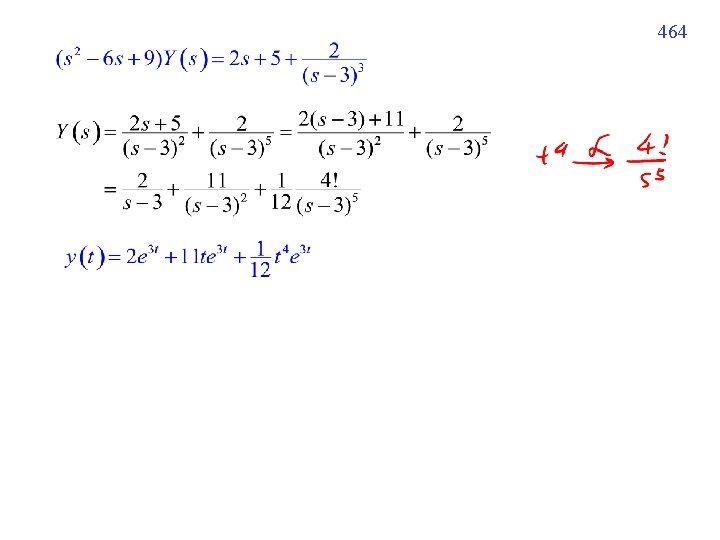
464

465 7 -3 -2 Step Function u(t): unit step function u(t) = 1 for t > 0 u(t) = 0 for t < 0 t-axis t=0 u(t−a) = 1 for t > a u(t−a) = 0 for t < a t=a The unit step function acts as a switch (開關). t-axis

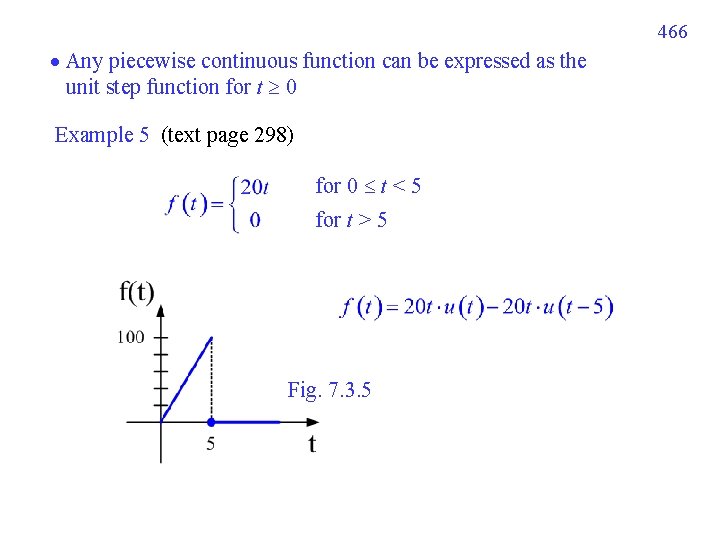
466 Any piecewise continuous function can be expressed as the unit step function for t 0 Example 5 (text page 298) for 0 t < 5 for t > 5 Fig. 7. 3. 5

467 In general, for 0 t < a for t > a

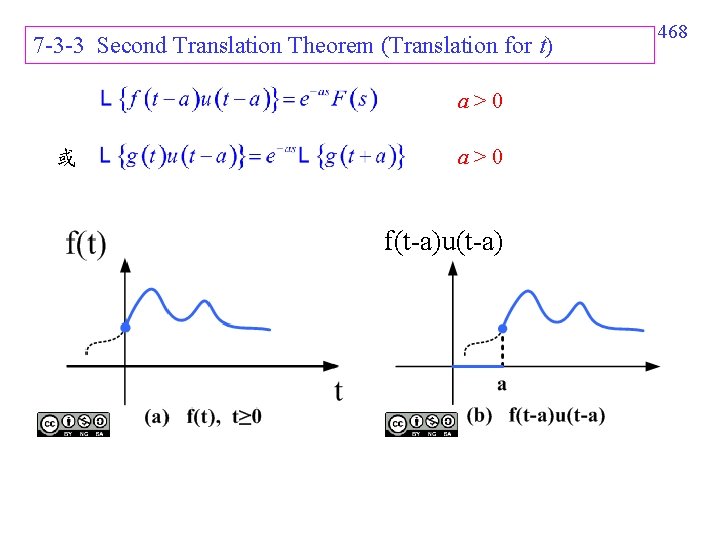
7 -3 -3 Second Translation Theorem (Translation for t) a>0 或 a>0 f(t-a)u(t-a) 468
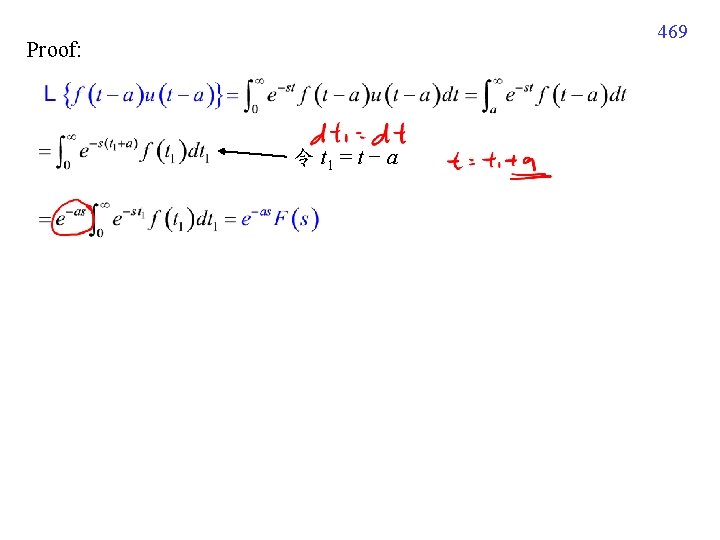
469 Proof: 令 t 1 = t − a

470 Example 7(a) (text page 300) Example 8 (text page 300)

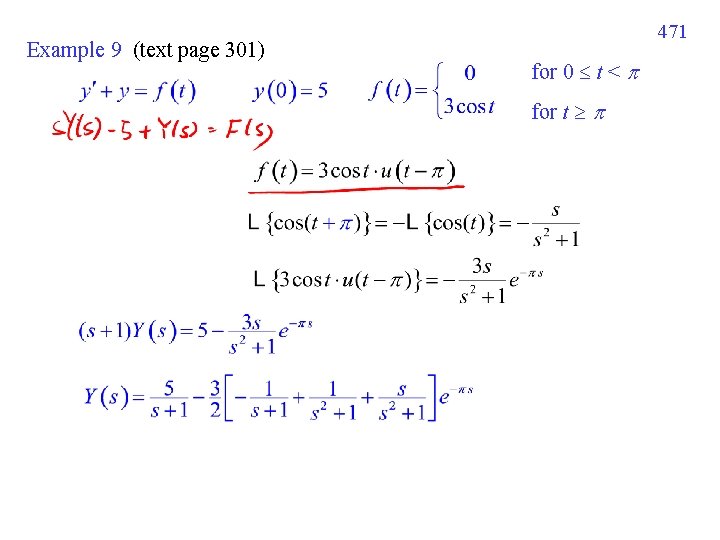
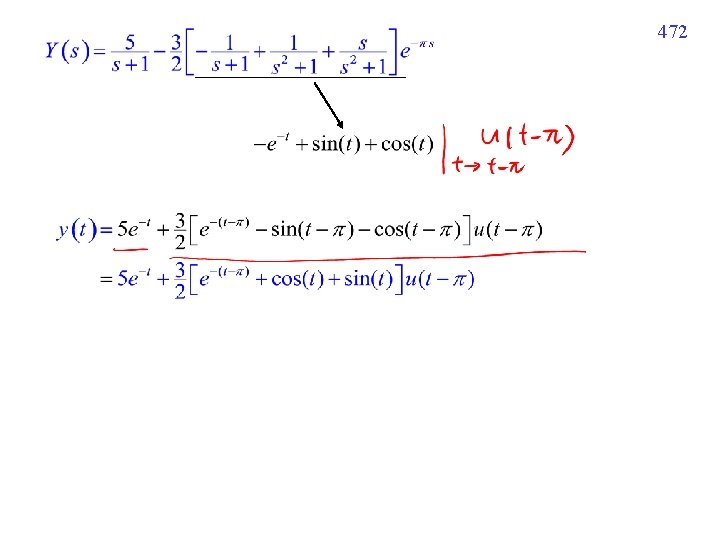
Example 9 (text page 301) 471 for 0 t < for t

472

7 -3 -4 本節需要注意的地方 (1) 套用 “translation for t” 的公式時, 先將 input 變成 g( t + a ) 再作 Laplace transform (例如 Example 7) (2) Second translation theorem (translation for t) 當 a > 0 時才適用 (3) 套用公式時,注意「順序」 473

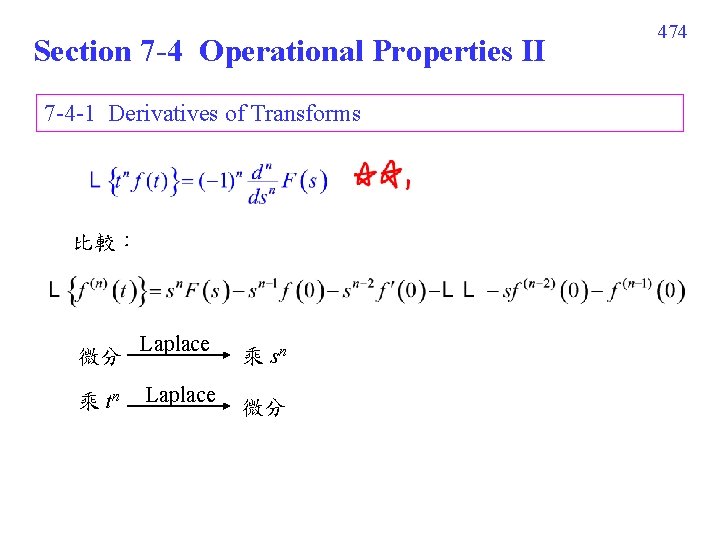
Section 7 -4 Operational Properties II 7 -4 -1 Derivatives of Transforms 比較: 微分 Laplace 乘 tn Laplace 乘 sn 微分 474

Proof of the Theorem of Derivatives of Transforms: 475

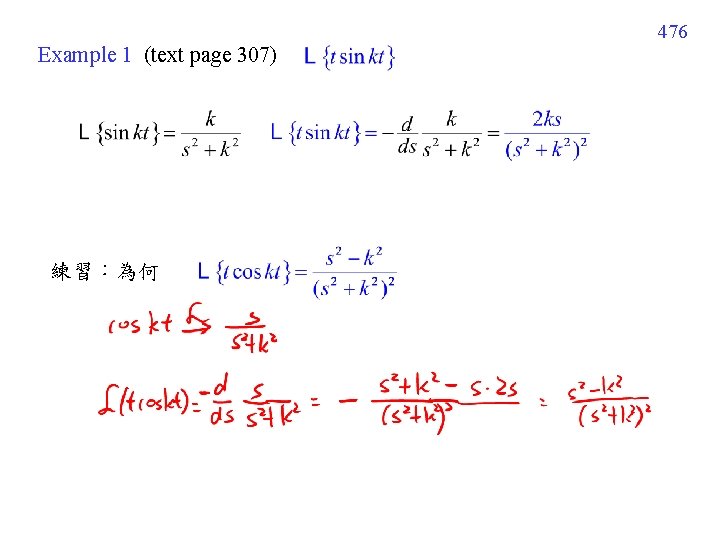
476 Example 1 (text page 307) 練習:為何

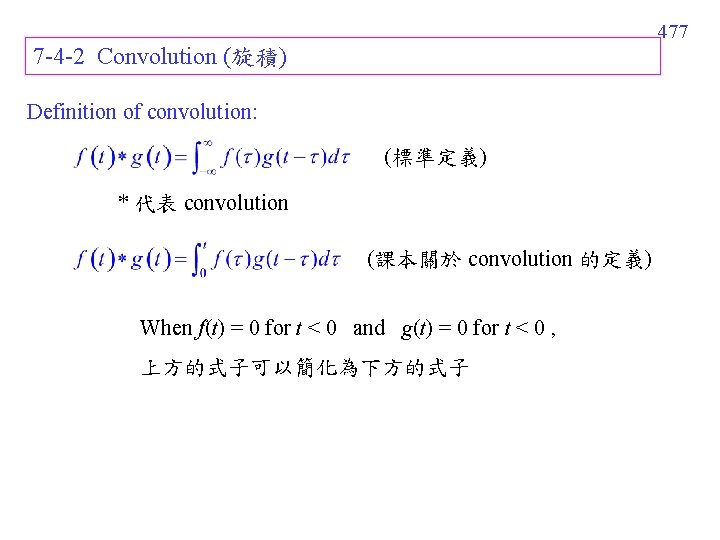
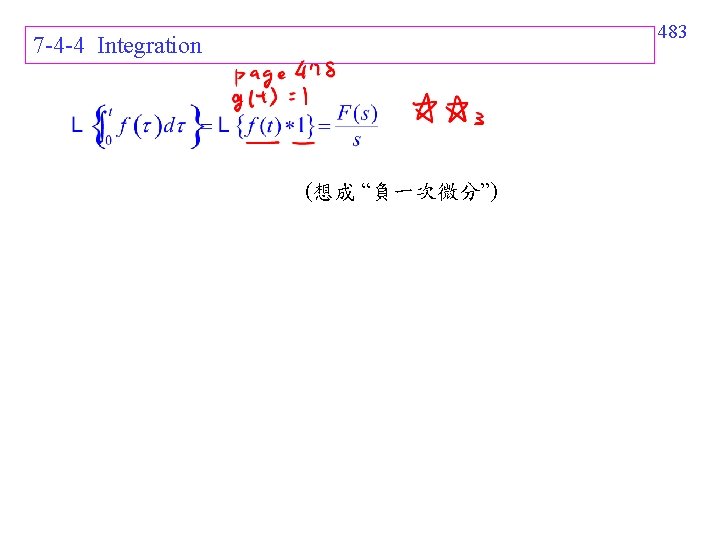
477 7 -4 -2 Convolution (旋積) Definition of convolution: (標準定義) * 代表 convolution (課本關於 convolution 的定義) When f(t) = 0 for t < 0 and g(t) = 0 for t < 0 , 上方的式子可以簡化為下方的式子



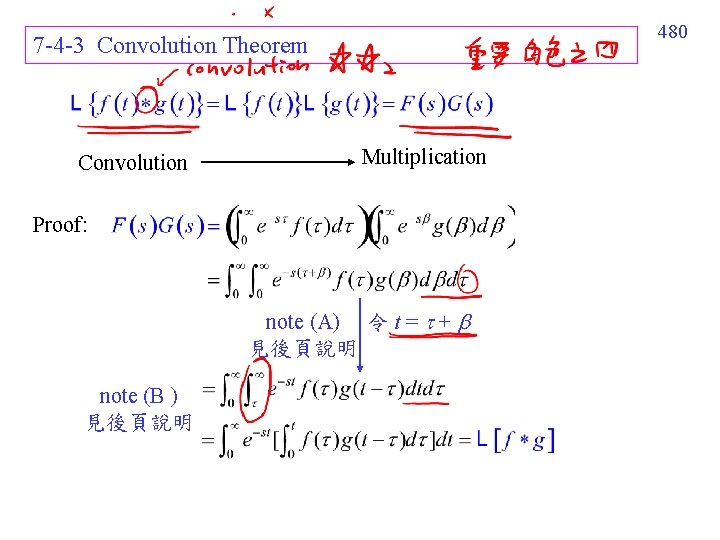
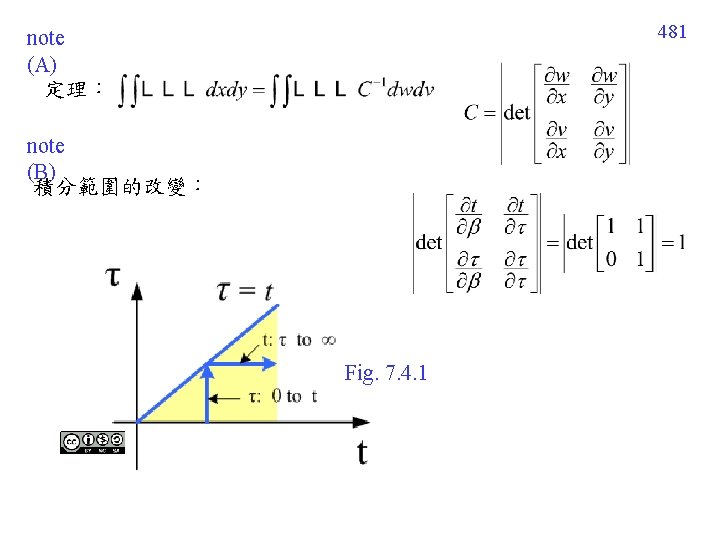
480 7 -4 -3 Convolution Theorem Convolution Multiplication Proof: note (A) 令 t = + 見後頁說明 note (B ) 見後頁說明


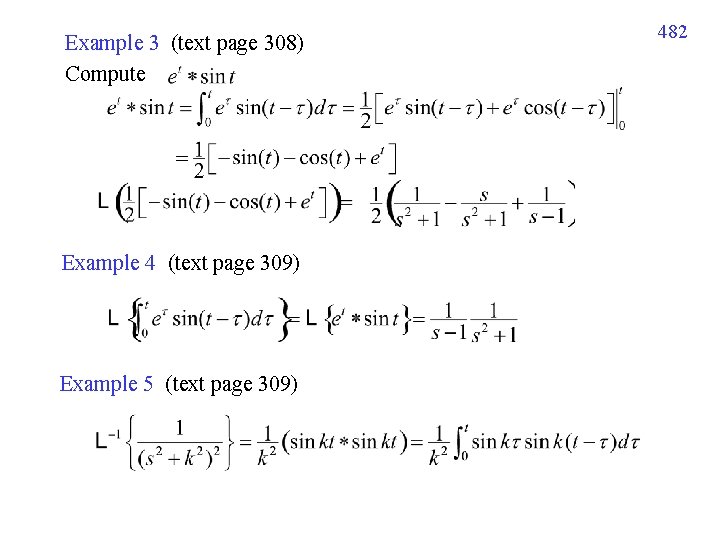
Example 3 (text page 308) Compute Example 4 (text page 309) Example 5 (text page 309) 482

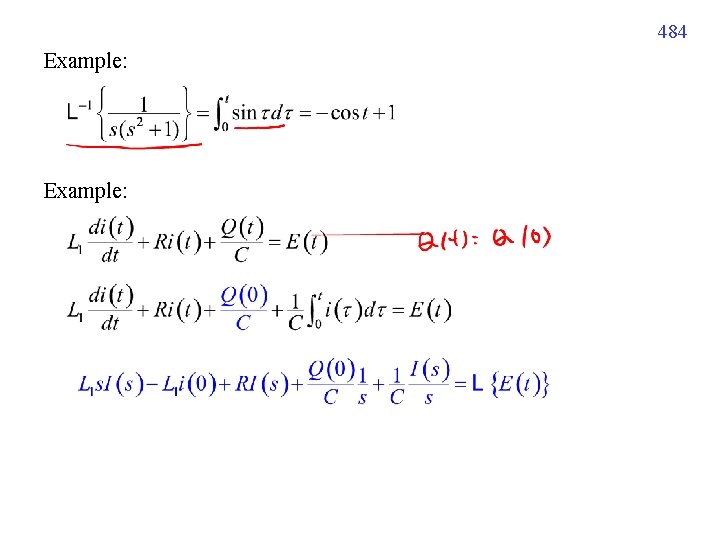
484 Example:

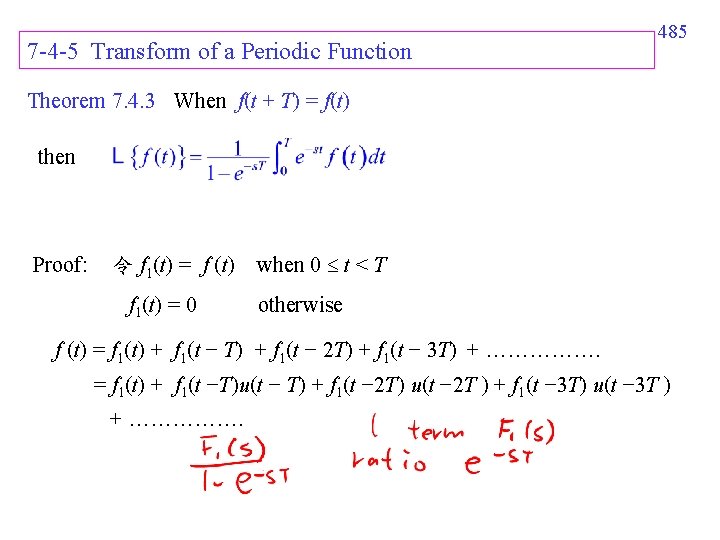
7 -4 -5 Transform of a Periodic Function 485 Theorem 7. 4. 3 When f(t + T) = f(t) then Proof: 令 f 1(t) = f (t) f 1(t) = 0 when 0 t < T otherwise f (t) = f 1(t) + f 1(t − T) + f 1(t − 2 T) + f 1(t − 3 T) + ……………. = f 1(t) + f 1(t −T)u(t − T) + f 1(t − 2 T) u(t − 2 T ) + f 1(t − 3 T) u(t − 3 T ) + …………….
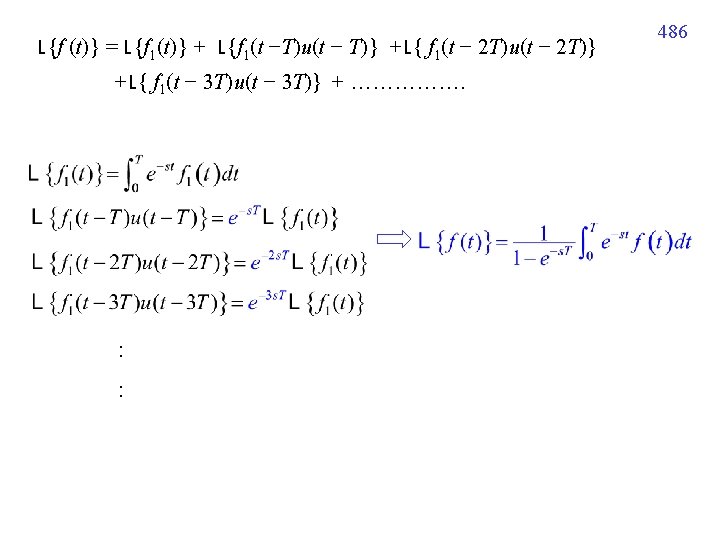

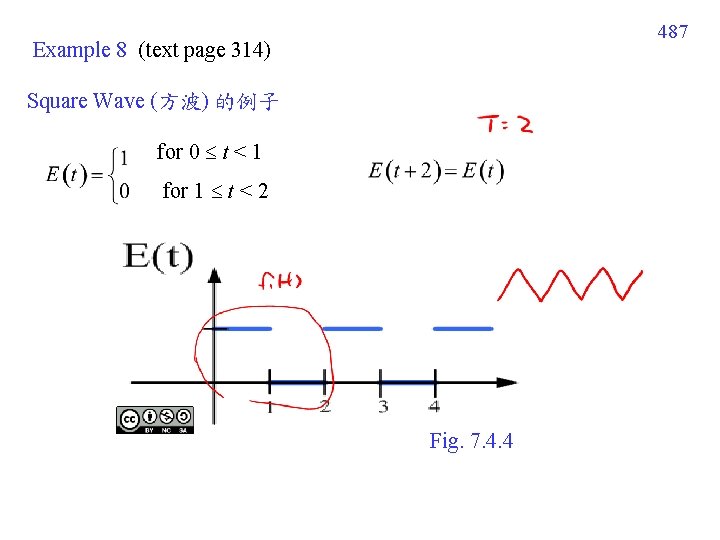
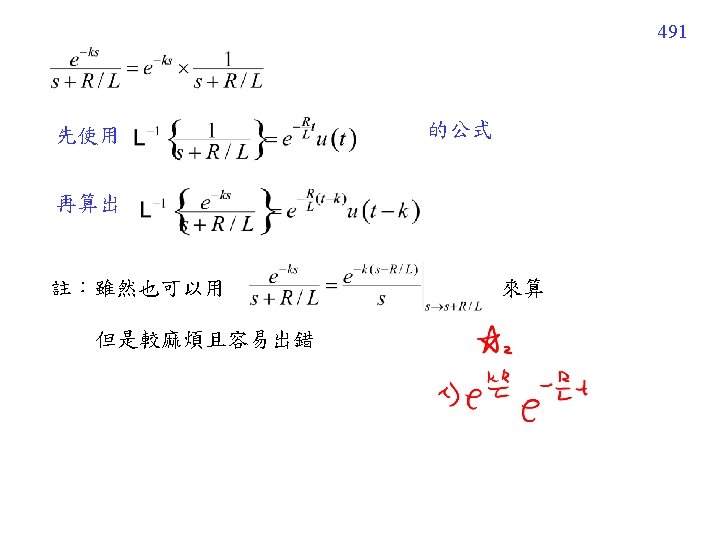
487 Example 8 (text page 314) Square Wave (方波) 的例子 for 0 t < 1 for 1 t < 2 Fig. 7. 4. 4
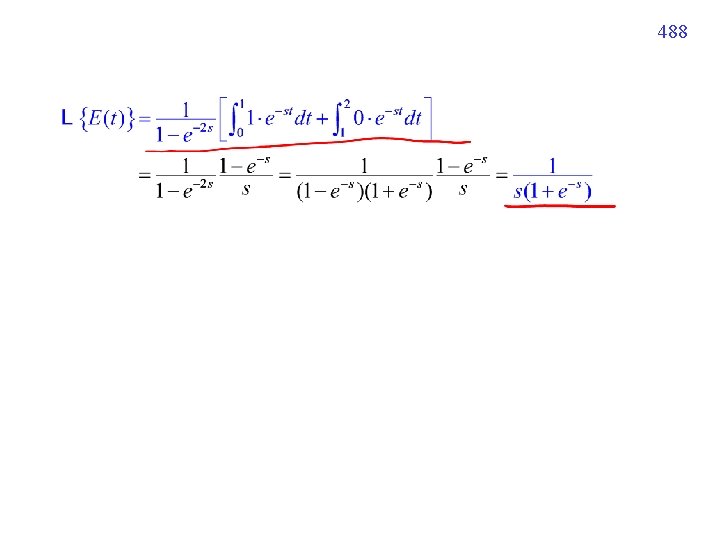
488

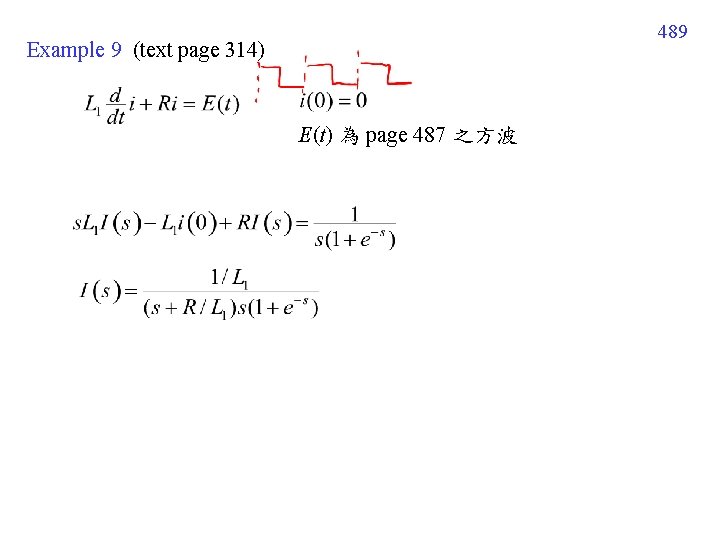
489 Example 9 (text page 314) E(t) 為 page 487 之方波




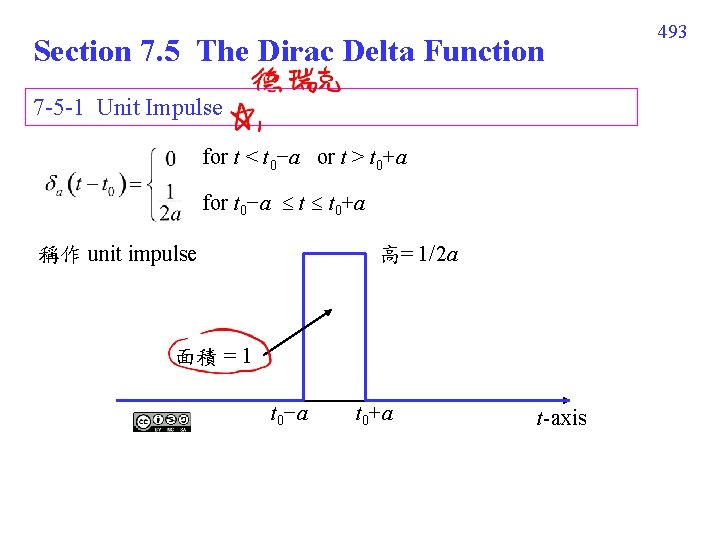
Section 7. 5 The Dirac Delta Function 7 -5 -1 Unit Impulse for t < t 0−a or t > t 0+a for t 0−a t t 0+a 稱作 unit impulse 高= 1/2 a 面積 = 1 t 0−a t 0+a t-axis 493

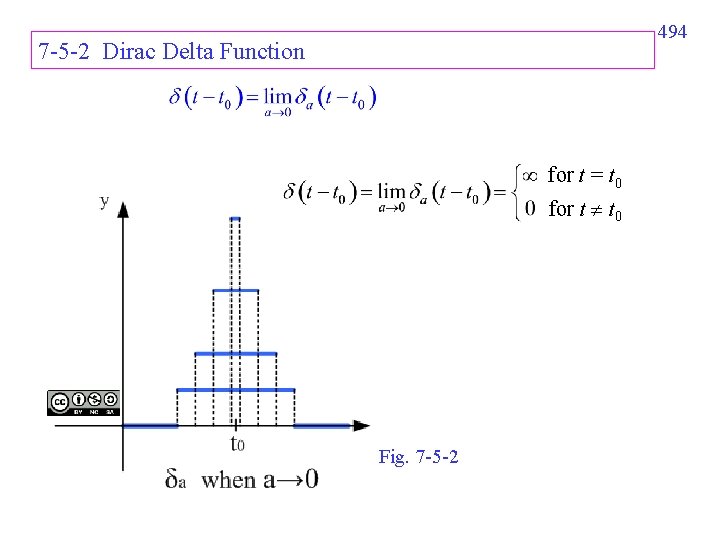
494 7 -5 -2 Dirac Delta Function for t = t 0 for t t 0 Fig. 7 -5 -2

7 -5 -3 Properties of the Dirac Delta Function (1) Integration (2) Sifting when t 0 [p, q] Proof: 當 a 很小的時候,f(t) f(t 0) for t 0−a t t 0+a 495

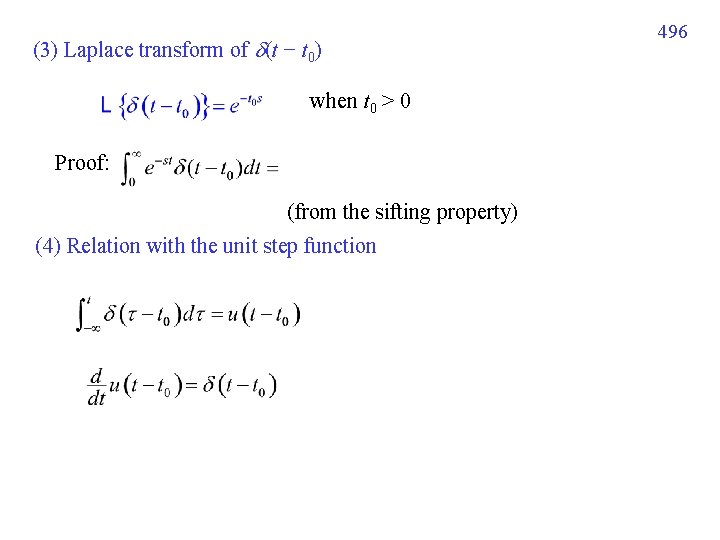
(3) Laplace transform of (t − t 0) when t 0 > 0 Proof: (from the sifting property) (4) Relation with the unit step function 496

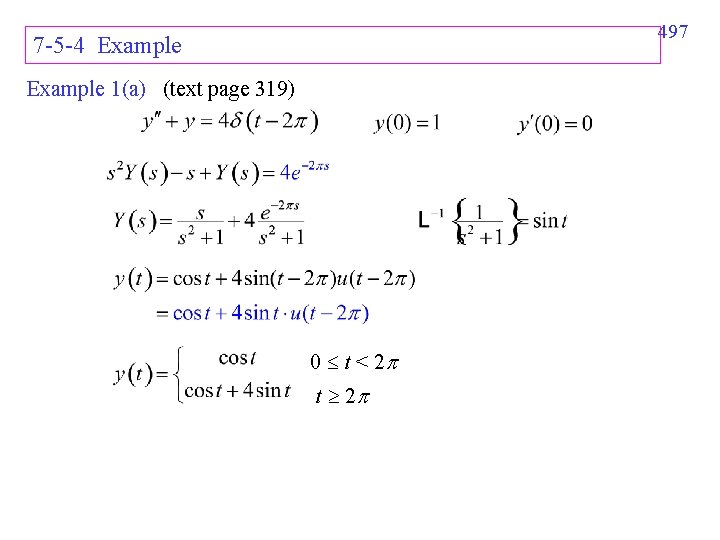
497 7 -5 -4 Example 1(a) (text page 319) 0 t < 2 t 2

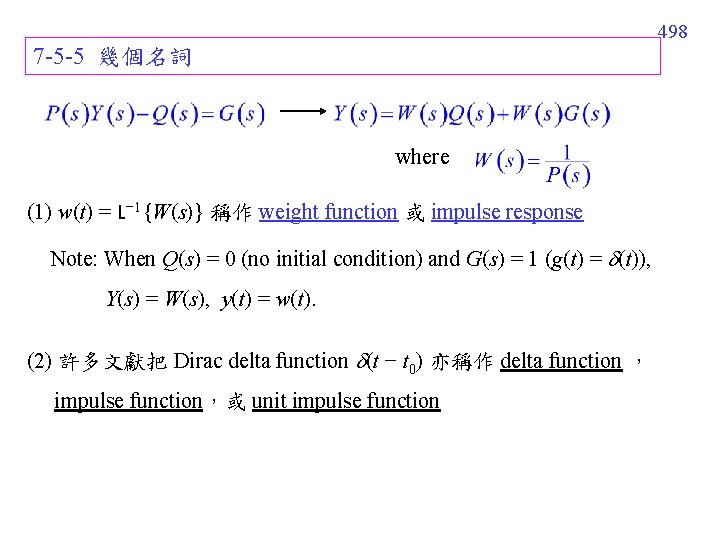
498 7 -5 -5 幾個名詞 where (1) w(t) = L− 1{W(s)} 稱作 weight function 或 impulse response Note: When Q(s) = 0 (no initial condition) and G(s) = 1 (g(t) = (t)), Y(s) = W(s), y(t) = w(t). (2) 許多文獻把 Dirac delta function (t − t 0) 亦稱作 delta function , impulse function,或 unit impulse function


Section 7 -6 Systems of Linear Differential Equations Chapter 7 的應用題 比較:類似的問題,也曾經在 Section 4 -9 出現過 7 -6 -1 雙彈簧的例子 500


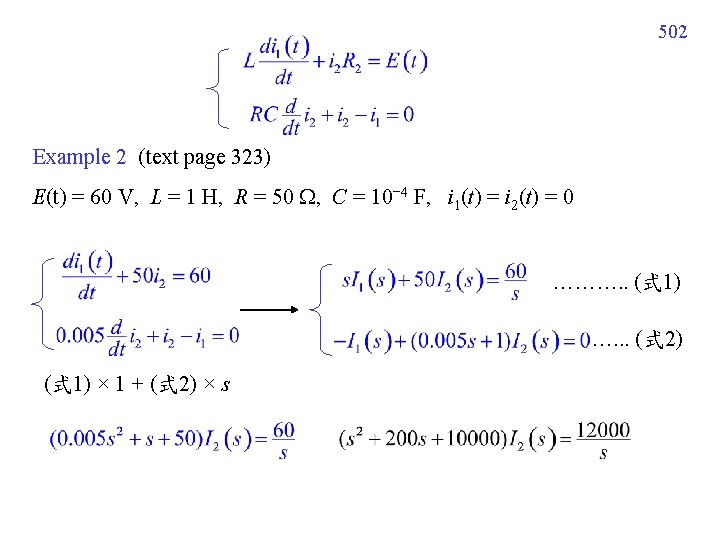
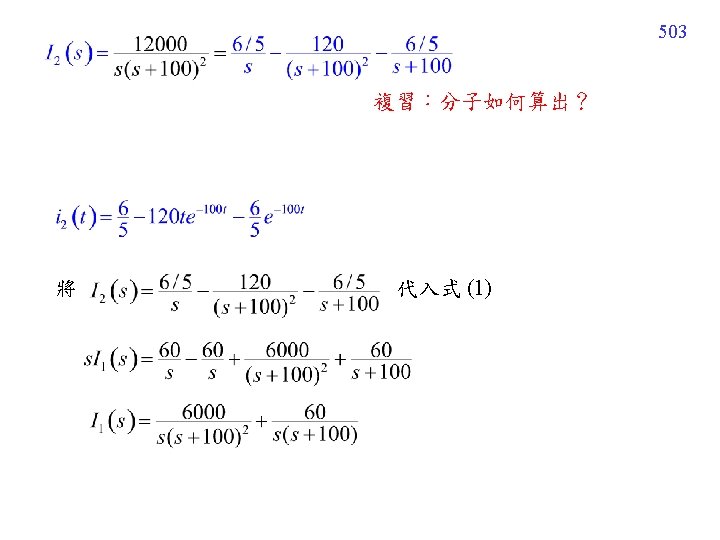
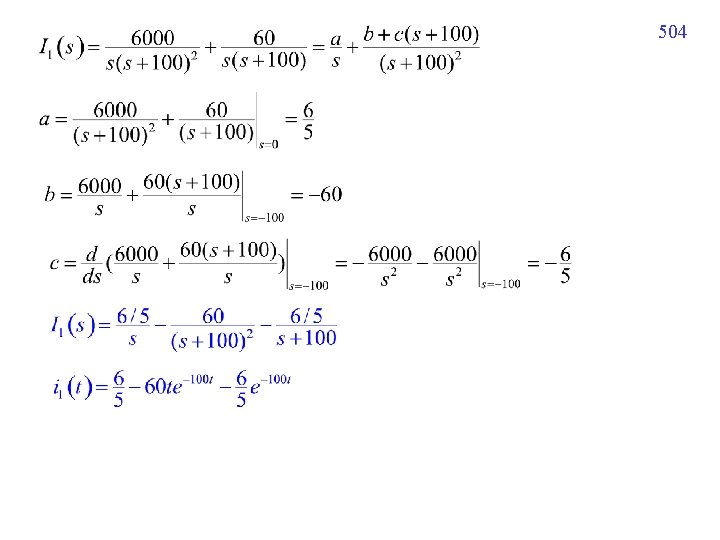
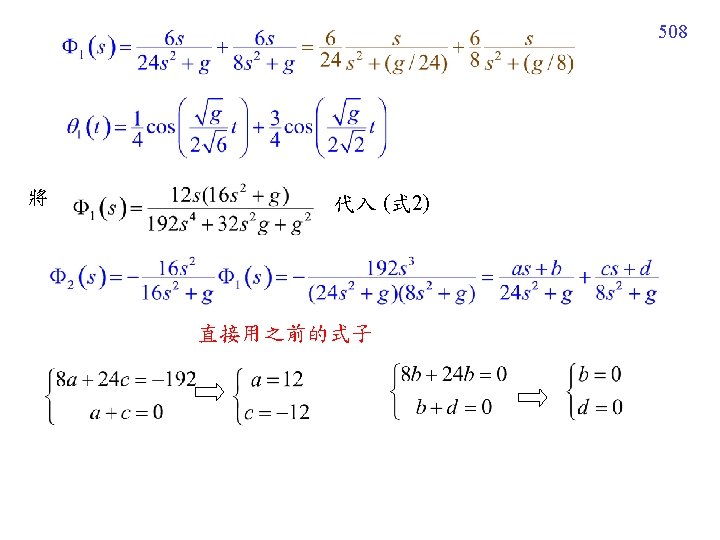
502 Example 2 (text page 323) E(t) = 60 V, L = 1 H, R = 50 , C = 10− 4 F, i 1(t) = i 2(t) = 0 ………. . (式 1) …. . . (式 2) (式 1) × 1 + (式 2) × s


504

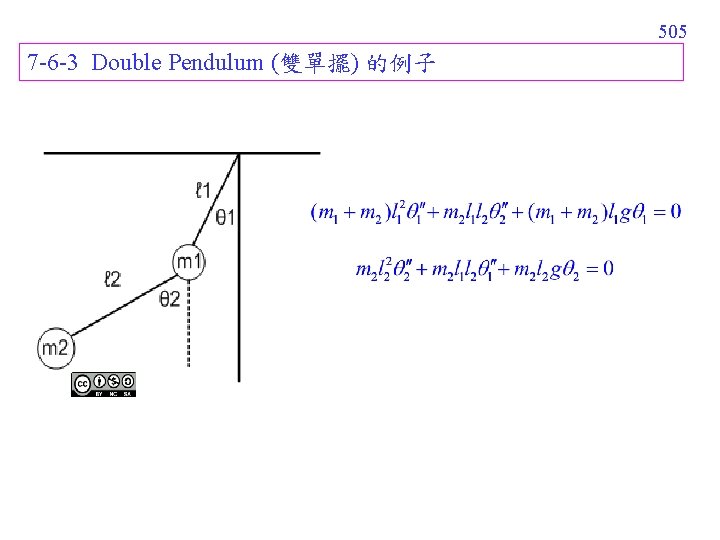
505 7 -6 -3 Double Pendulum (雙單擺) 的例子

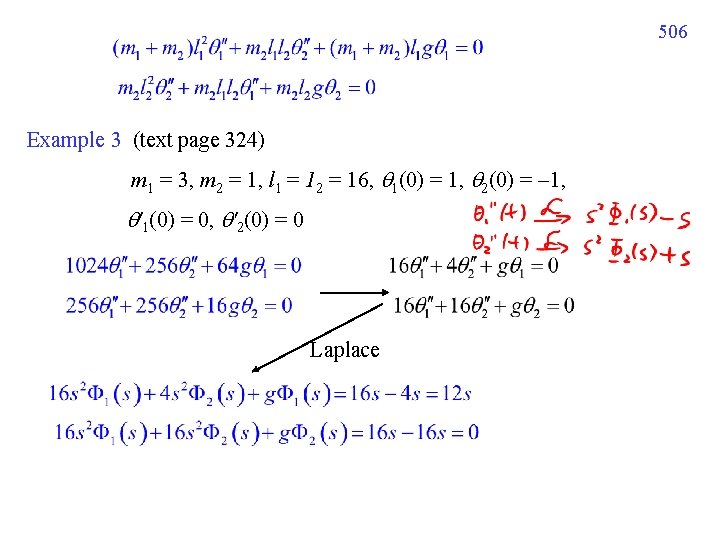
506 Example 3 (text page 324) m 1 = 3, m 2 = 1, l 1 = 12 = 16, 1(0) = 1, 2(0) = 1, '1(0) = 0, '2(0) = 0 Laplace
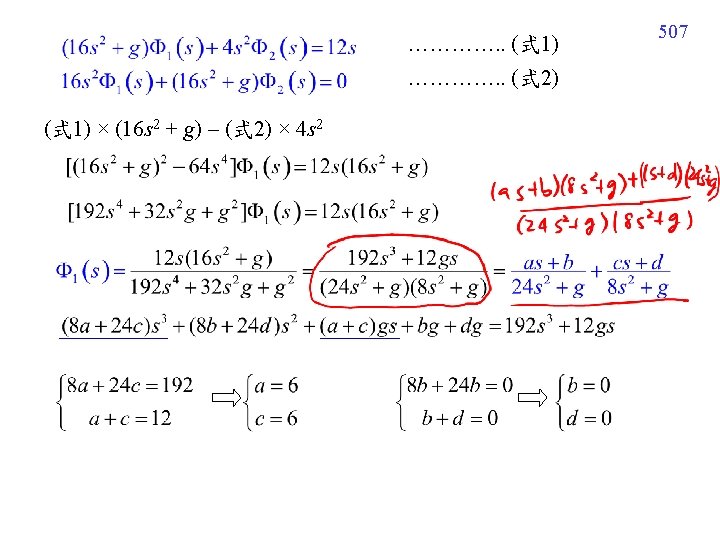

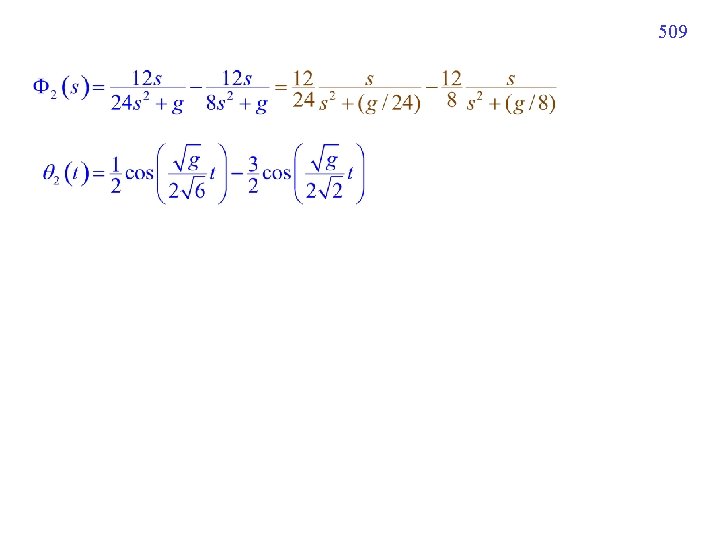
509



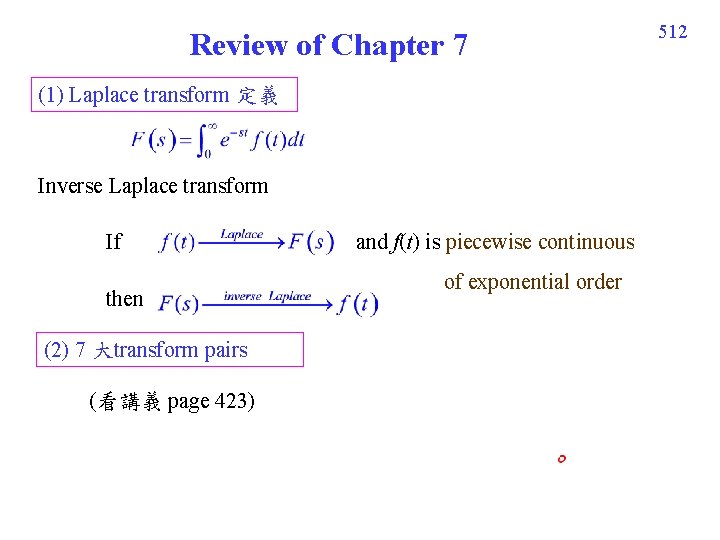
Review of Chapter 7 (1) Laplace transform 定義 Inverse Laplace transform If then (2) 7 大transform pairs (看講義 page 423) and f(t) is piecewise continuous of exponential order 512

513 transform pairs 補充 f(t) F(s) tsin(kt) tcos(kt) tsinh(kt) tcosh(kt) u(t a) 0 1 a b 0 f (t) = f (t+2 a)

514 (3) 7 大 properties input (1) Differentiation (Sec 7 -2) (2) Multiplication by t (Sec 74) (3) Integration (Sec 7 -4) (續) Laplace transform

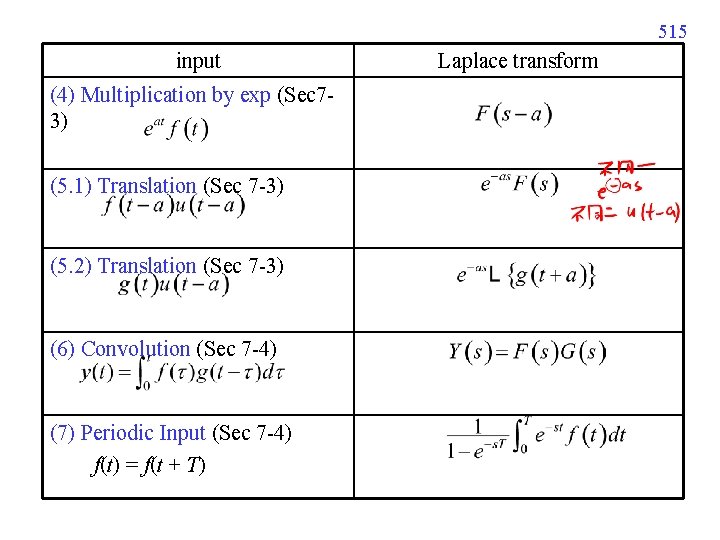
515 input (4) Multiplication by exp (Sec 73) (5. 1) Translation (Sec 7 -3) (5. 2) Translation (Sec 7 -3) (6) Convolution (Sec 7 -4) (7) Periodic Input (Sec 7 -4) f(t) = f(t + T) Laplace transform

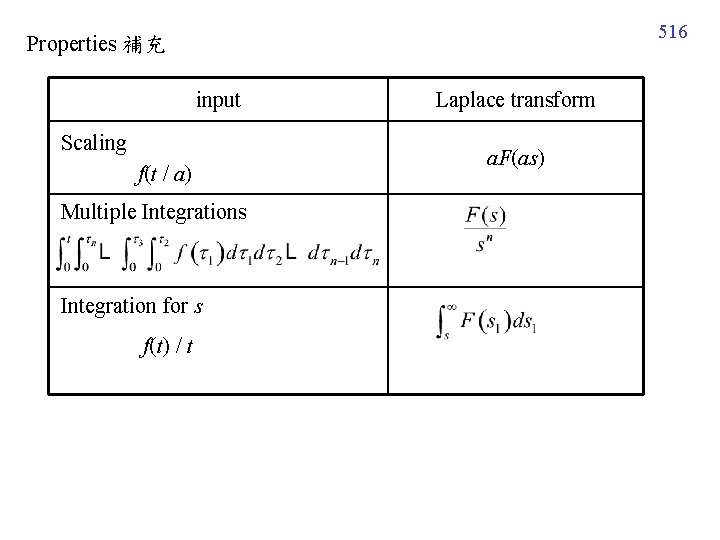
516 Properties 補充 input Scaling f(t / a) Multiple Integrations Integration for s f(t) / t Laplace transform a. F(as)

(4) 簡化運算的方法 分式分解 (see pages 442 -447) Initial conditions (see pages 455, 456) (5) Delta function 的四大性質 Pages 495, 496 517

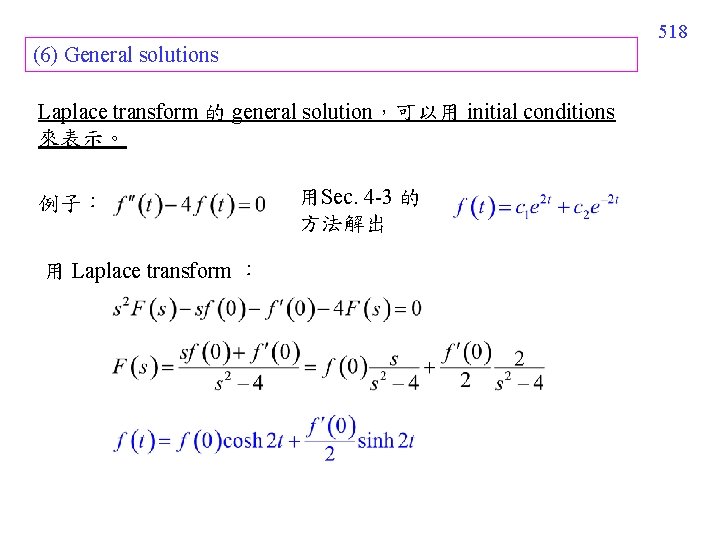
518 (6) General solutions Laplace transform 的 general solution,可以用 initial conditions 來表示。 例子: 用 Laplace transform : 用Sec. 4 -3 的 方法解出


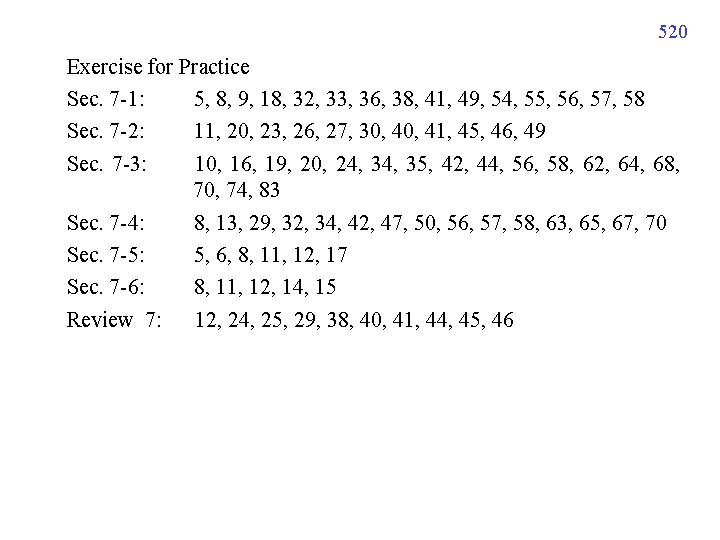
520 Exercise for Practice Sec. 7 -1: 5, 8, 9, 18, 32, 33, 36, 38, 41, 49, 54, 55, 56, 57, 58 Sec. 7 -2: 11, 20, 23, 26, 27, 30, 41, 45, 46, 49 Sec. 7 -3: 10, 16, 19, 20, 24, 35, 42, 44, 56, 58, 62, 64, 68, 70, 74, 83 Sec. 7 -4: 8, 13, 29, 32, 34, 42, 47, 50, 56, 57, 58, 63, 65, 67, 70 Sec. 7 -5: 5, 6, 8, 11, 12, 17 Sec. 7 -6: 8, 11, 12, 14, 15 Review 7: 12, 24, 25, 29, 38, 40, 41, 44, 45, 46
 Inverse z transforms
Inverse z transforms Uu
Uu How does eurylochus justify killing the cattle
How does eurylochus justify killing the cattle Laplace transform.table
Laplace transform.table Transform data into information
Transform data into information Transforms of discontinuous functions
Transforms of discontinuous functions This transforms a bare stage into the world of the play
This transforms a bare stage into the world of the play What is the drama
What is the drama Transforms eroded parts of earth's surface into lakes
Transforms eroded parts of earth's surface into lakes Pole vault energy transformation
Pole vault energy transformation Which phase transforms srs document
Which phase transforms srs document Piere simon laplace
Piere simon laplace Friction transforms mechanical energy to
Friction transforms mechanical energy to Z transform of ramp function
Z transform of ramp function Image transforms
Image transforms Image transforms in digital image processing
Image transforms in digital image processing Photosynthesis transforms light energy into chemical energy
Photosynthesis transforms light energy into chemical energy Section line symbol
Section line symbol Section 2 describing energy worksheet answers
Section 2 describing energy worksheet answers Dimensioning section views
Dimensioning section views Revolve section
Revolve section Chapter 10 section 1 meiosis worksheet answer key
Chapter 10 section 1 meiosis worksheet answer key Range of inverse sine function
Range of inverse sine function Domain and range of an inverse function
Domain and range of an inverse function Forward and inverse fourier transform
Forward and inverse fourier transform Sin^-1(-1)
Sin^-1(-1) How to use inverse trig functions to find angles
How to use inverse trig functions to find angles Inverse relations and functions
Inverse relations and functions Dr frost interior and exterior angles
Dr frost interior and exterior angles Inverse variation
Inverse variation Direct variation vs inverse variation
Direct variation vs inverse variation Direct inverse graph
Direct inverse graph Derivatives of inverse functions and logarithms
Derivatives of inverse functions and logarithms Converse geo
Converse geo What is the difference between direct and inverse variation
What is the difference between direct and inverse variation Inverse circular functions
Inverse circular functions Inverse variation graph calculator
Inverse variation graph calculator Inverse function domain and range
Inverse function domain and range What is multiplicative inverse
What is multiplicative inverse Inverse proportion worksheet
Inverse proportion worksheet Composition of functions inverse
Composition of functions inverse Lesson 6-2 graphs of inverse functions
Lesson 6-2 graphs of inverse functions Reverse exponential graph
Reverse exponential graph Parameter estimation and inverse problems
Parameter estimation and inverse problems 6-7 inverse relations and functions
6-7 inverse relations and functions 1-7 inverse relations and functions
1-7 inverse relations and functions Properties of addition and multiplication
Properties of addition and multiplication Identity and inverse matrices
Identity and inverse matrices Direct and inverse proportion activity
Direct and inverse proportion activity Inverse functions and relations
Inverse functions and relations Slogan p
Slogan p Inverse variation and the reciprocal function
Inverse variation and the reciprocal function Arcsin 1
Arcsin 1 Multiplicative inverse and reciprocal
Multiplicative inverse and reciprocal Inverse cariation
Inverse cariation Differentiation of cot theta
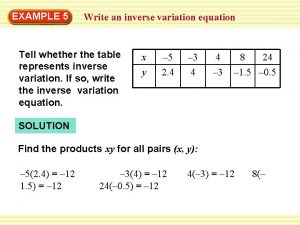
Differentiation of cot theta Write an inverse variation equation that relates x and y
Write an inverse variation equation that relates x and y Boyle's law inverse relationship
Boyle's law inverse relationship Inverse proportion graph
Inverse proportion graph Inverse of log function
Inverse of log function Transformée de fourier dirac
Transformée de fourier dirac Inverse functions in real life
Inverse functions in real life Inverse of log function
Inverse of log function Additive inverse of 2
Additive inverse of 2 Soh cah toa inverse
Soh cah toa inverse Inverse laplace of complex roots
Inverse laplace of complex roots Inverse error discrete math
Inverse error discrete math Sinc to rect
Sinc to rect Dirac delta function fourier transform
Dirac delta function fourier transform Multiplicative inverse of matrix
Multiplicative inverse of matrix Inverse stretch reflex
Inverse stretch reflex Stretch reflex
Stretch reflex Pythagorean theorem inverse
Pythagorean theorem inverse Example of inverse operations
Example of inverse operations Domain requirements
Domain requirements Inverse requirements in software engineering
Inverse requirements in software engineering Reciprocal vs inverse
Reciprocal vs inverse Trigonometric function
Trigonometric function Esercizi sopra e sotto cento con risultati
Esercizi sopra e sotto cento con risultati Y is inversely proportional to the cube of x
Y is inversely proportional to the cube of x Commutative associative and identity property of addition
Commutative associative and identity property of addition Alpha and gamma motor neurons
Alpha and gamma motor neurons Horizontal line
Horizontal line Importance of peak inverse voltage
Importance of peak inverse voltage Cos parent function table
Cos parent function table