There are two things to aim at in


























![Miller Indices 4 Miller indices of a family of symmetry related directions = [uvw] Miller Indices 4 Miller indices of a family of symmetry related directions = [uvw]](https://slidetodoc.com/presentation_image_h2/d4332574de8c28379e5cc1b71d6c56ed/image-57.jpg)



![CUBIC CRYSTALS [111] [hkl] (hkl) (111) Angle between two directions [h 1 k 1 CUBIC CRYSTALS [111] [hkl] (hkl) (111) Angle between two directions [h 1 k 1](https://slidetodoc.com/presentation_image_h2/d4332574de8c28379e5cc1b71d6c56ed/image-67.jpg)

![Summary of Notation convention for Indices [uvw] Miller indices of a direction (i. e. Summary of Notation convention for Indices [uvw] Miller indices of a direction (i. e.](https://slidetodoc.com/presentation_image_h2/d4332574de8c28379e5cc1b71d6c56ed/image-69.jpg)

























- Slides: 112

“There are two things to aim at in life: first, to get what you want; and, after that, to enjoy it. Only the wisest of mankind achieve the second. ” Logan Pearsall Smith, Afterthought (1931), “Life and Human Nature”

Chapter 3 Crystal Geometry and Structure Determination

Contents Crystal, Lattice and Motif Symmetry Crystal systems Bravais lattices Miller Indices Structure Determination

Crystal ? A 3 D translationaly periodic arrangement of atoms in space is called a crystal.

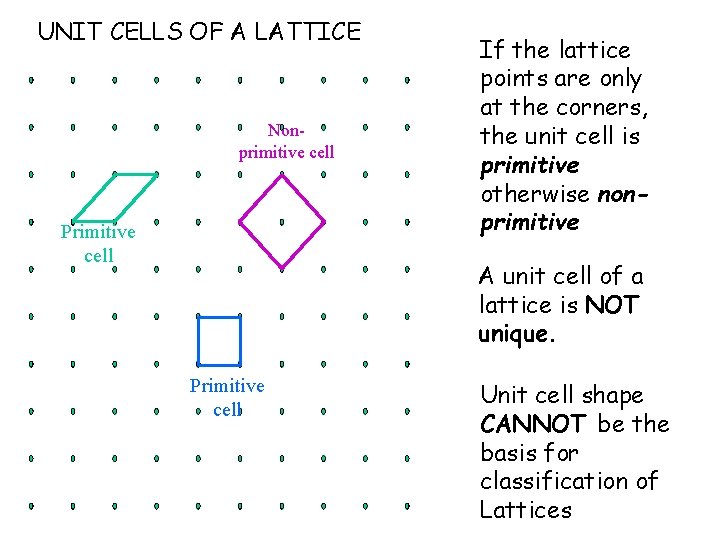
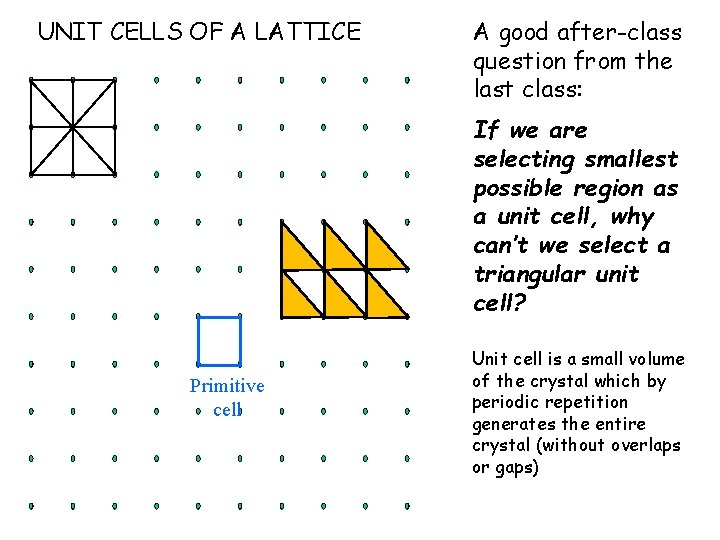
Unit cell description : 1 Translational Periodicity One can select a small volume of the crystal which by periodic repetition generates the entire crystal (without overlaps or gaps) Unit Cell

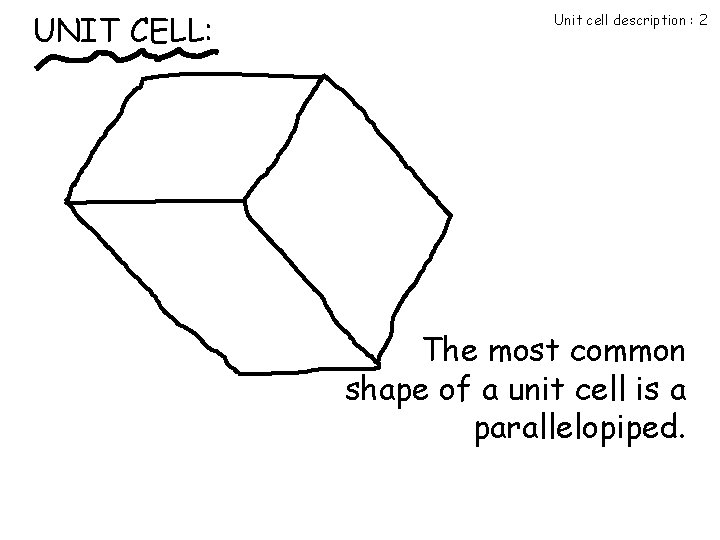
UNIT CELL: Unit cell description : 2 The most common shape of a unit cell is a parallelopiped.

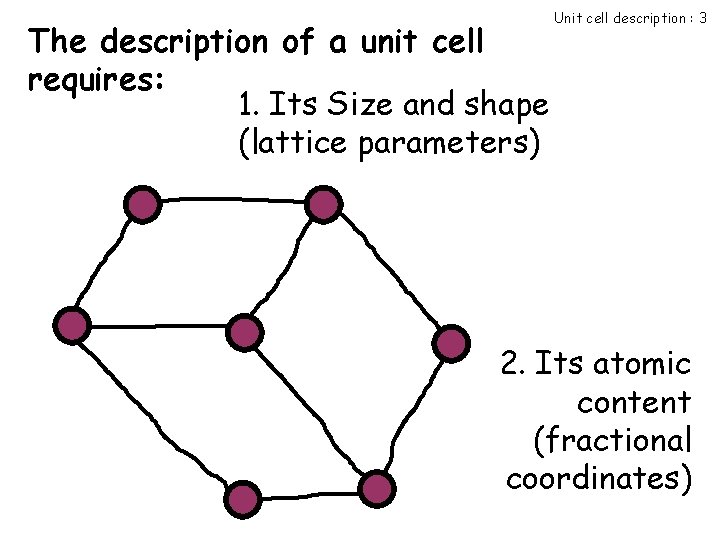
The description of a unit cell requires: 1. Its Size and shape (lattice parameters) Unit cell description : 3 2. Its atomic content (fractional coordinates)

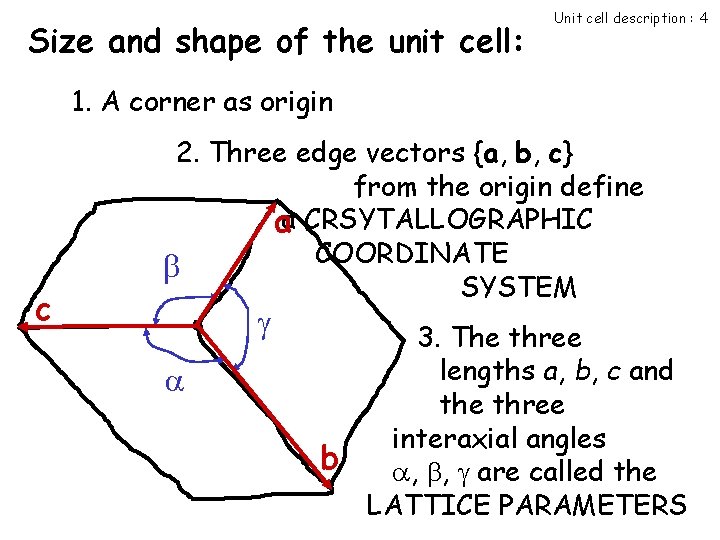
Size and shape of the unit cell: Unit cell description : 4 1. A corner as origin c 2. Three edge vectors {a, b, c} from the origin define aa CRSYTALLOGRAPHIC COORDINATE SYSTEM b 3. The three lengths a, b, c and the three interaxial angles , , are called the LATTICE PARAMETERS

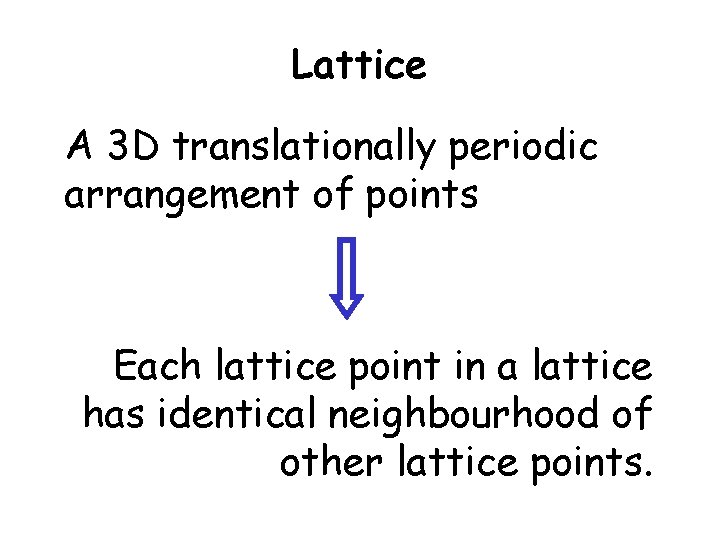
Lattice? A 3 D translationally periodic arrangement of points in space is called a lattice.

Lattice A 3 D translationally periodic arrangement of points Each lattice point in a lattice has identical neighbourhood of other lattice points.

Classification of lattice The Seven Crystal System And The Fourteen Bravais Lattices

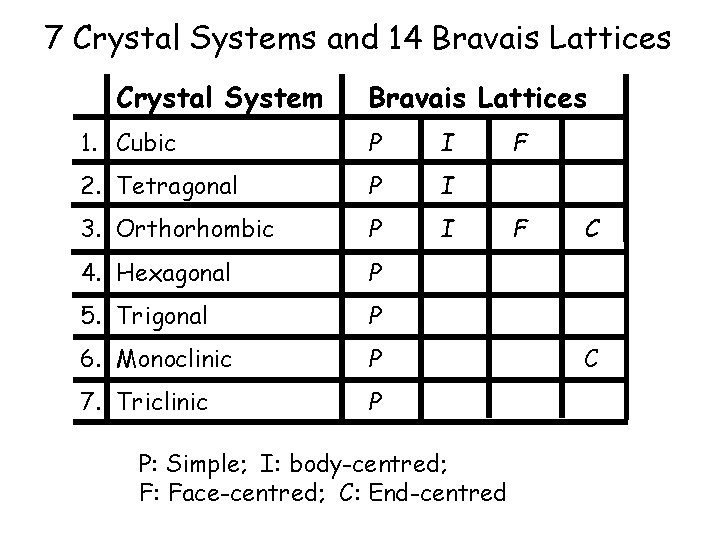
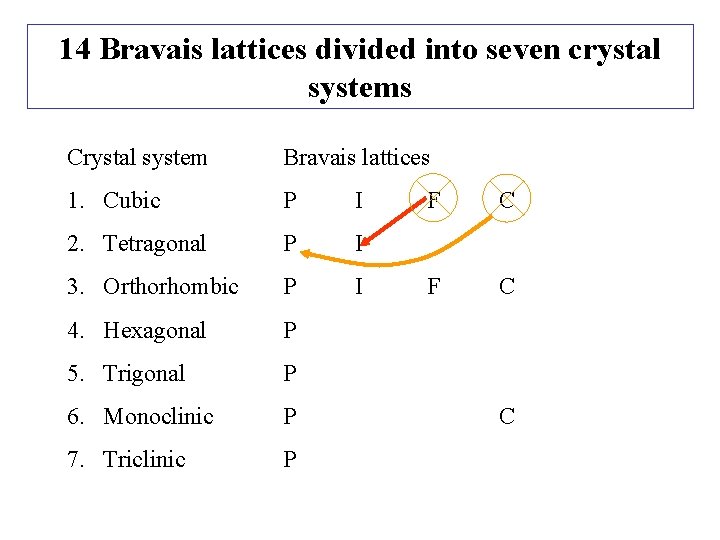
7 Crystal Systems and 14 Bravais Lattices Crystal System Bravais Lattices 1. Cubic P I 2. Tetragonal P I 3. Orthorhombic P I 4. Hexagonal P 5. Trigonal P 6. Monoclinic P 7. Triclinic P P: Simple; I: body-centred; F: Face-centred; C: End-centred F F C C

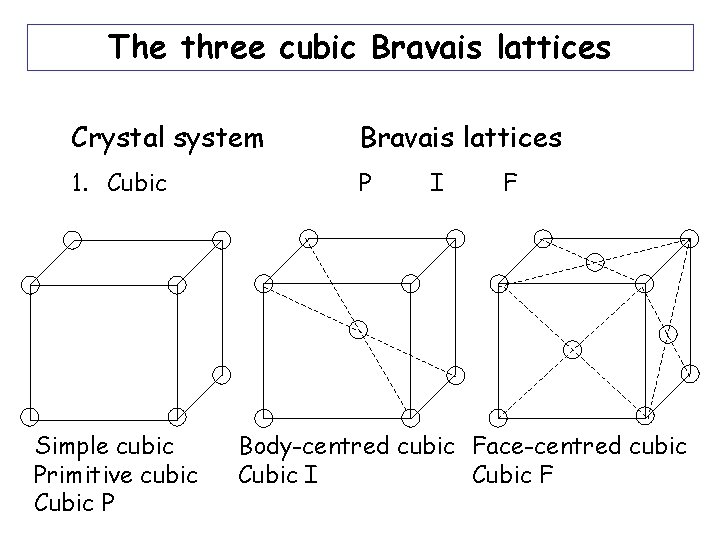
The three cubic Bravais lattices Crystal system Bravais lattices 1. Cubic P Simple cubic Primitive cubic Cubic P I F Body-centred cubic Face-centred cubic Cubic I Cubic F

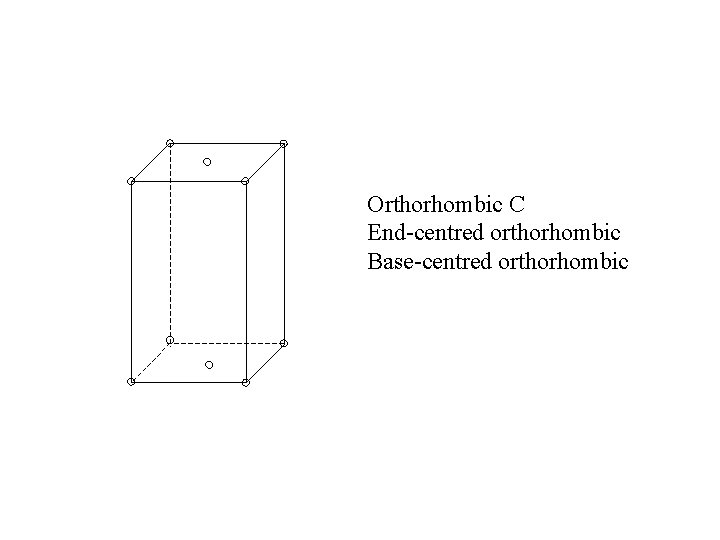
Orthorhombic C End-centred orthorhombic Base-centred orthorhombic

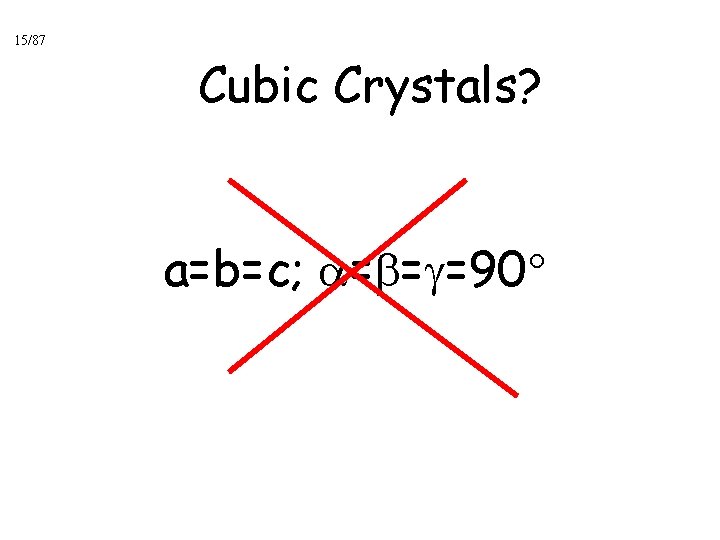
15/87 Cubic Crystals? a=b=c; = = =90

7 crystal Systems Unit Cell Shape Crystal System 1. a=b=c, = = =90 Cubic 2. a=b c, = = =90 Tetragonal 3. a b c, = = =90 Orthorhombic 4. a=b c, = = 90 , =120 Hexagonal 5. a=b=c, = = 90 Rhombohedral OR Trigonal 6. a b c, = =90 Monoclinic 7. a b c, Triclinic

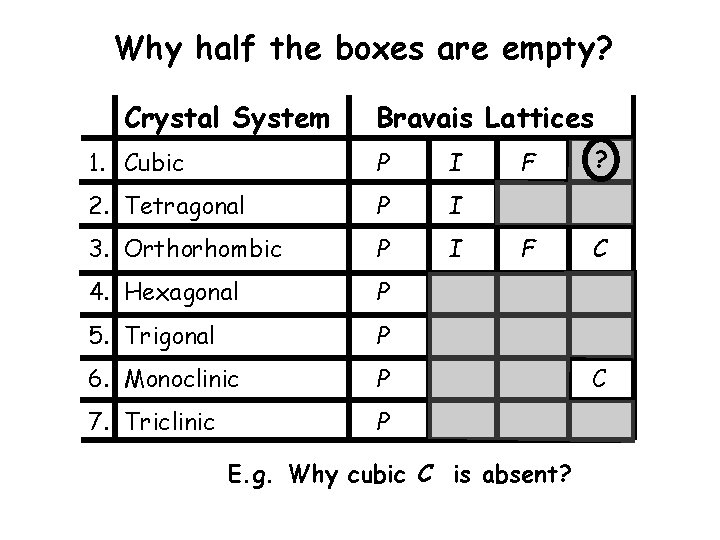
Why half the boxes are empty? Crystal System Bravais Lattices 1. Cubic P I 2. Tetragonal P I 3. Orthorhombic P I 4. Hexagonal P 5. Trigonal P 6. Monoclinic P 7. Triclinic P F ? F C E. g. Why cubic C is absent? C

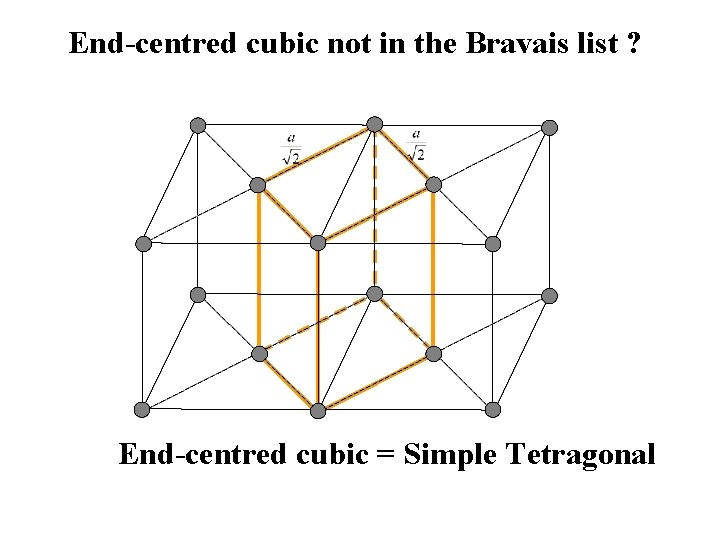
End-centred cubic not in the Bravais list ? End-centred cubic = Simple Tetragonal

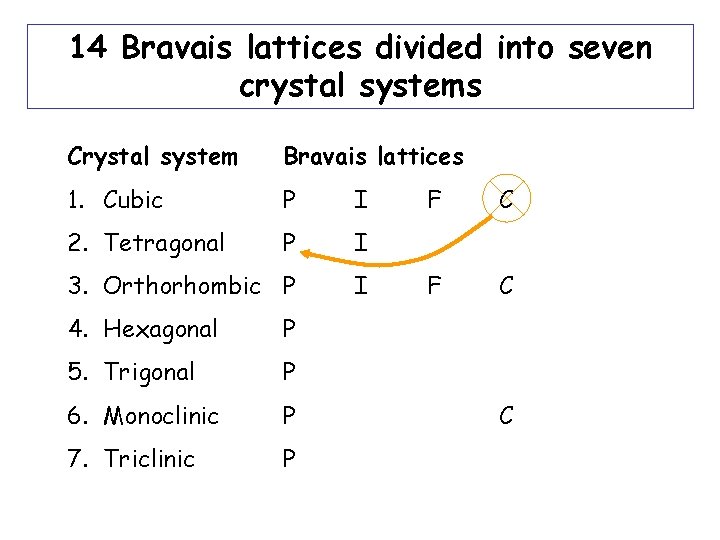
14 Bravais lattices divided into seven crystal systems Crystal system Bravais lattices 1. Cubic P I 2. Tetragonal P I 3. Orthorhombic P I 4. Hexagonal P 5. Trigonal P 6. Monoclinic P 7. Triclinic P F C C

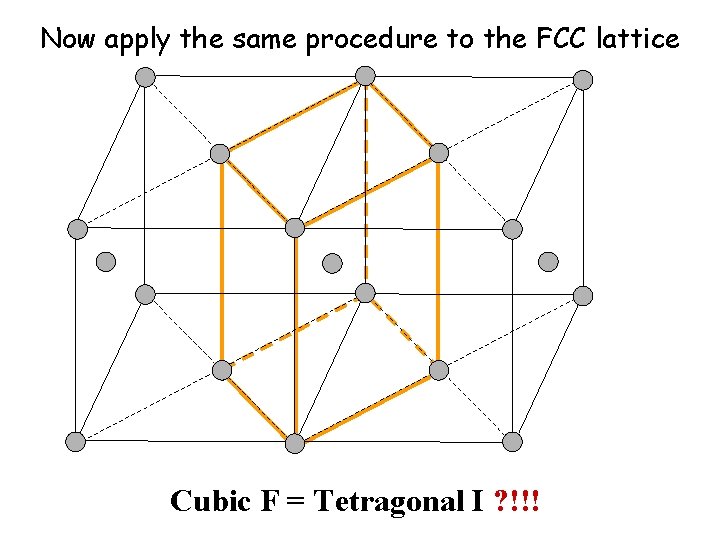
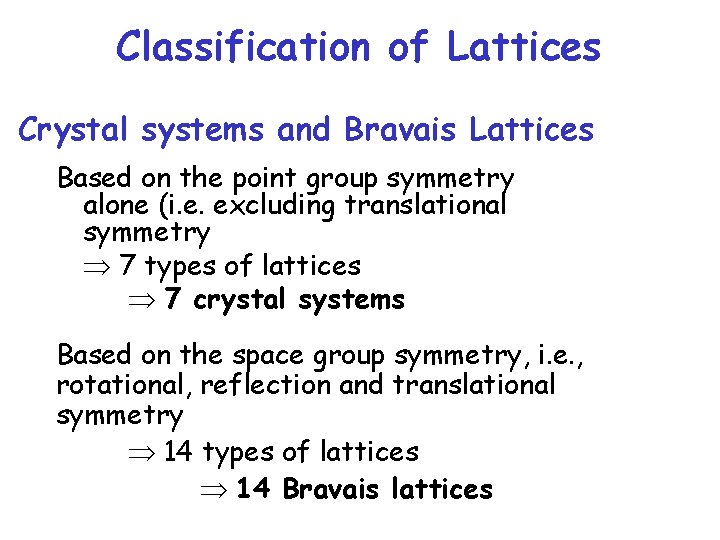
Now apply the same procedure to the FCC lattice Cubic F = Tetragonal I ? !!!

14 Bravais lattices divided into seven crystal systems Crystal system Bravais lattices 1. Cubic P I 2. Tetragonal P I 3. Orthorhombic P I 4. Hexagonal P 5. Trigonal P 6. Monoclinic P 7. Triclinic P F C C

History: ML Frankenheim 1801 -1869 1835: 1856: 15 lattices X 14 lattices Couldn’t find his photo on the net IIT-Delhi Auguste Bravais 1811 -1863 1850: 14 lattices 26 th July 2013: AML 120 Class Sem I 2013 -2014 13 lattices !!!

UNIT CELLS OF A LATTICE Nonprimitive cell Primitive cell If the lattice points are only at the corners, the unit cell is primitive otherwise nonprimitive A unit cell of a lattice is NOT unique. Primitive cell Unit cell shape CANNOT be the basis for classification of Lattices

End of Lec 3 (Lec 1 on crystallography)

UNIT CELLS OF A LATTICE A good after-class question from the last class: If we are selecting smallest possible region as a unit cell, why can’t we select a triangular unit cell? Primitive cell Unit cell is a small volume of the crystal which by periodic repetition generates the entire crystal (without overlaps or gaps)

Why can’t the Face. Centred Cubic lattice (Cubic F) be considered as a Body-Centred Tetragonal lattice (Tetragonal I) ?

What is the basis for classification of lattices into 7 crystal systems and 14 Bravais lattices?

Lattices are classified on the basis of their symmetry

Symmetry? If an object is brought into selfcoincidence after some operation it said to possess symmetry with respect to that operation.

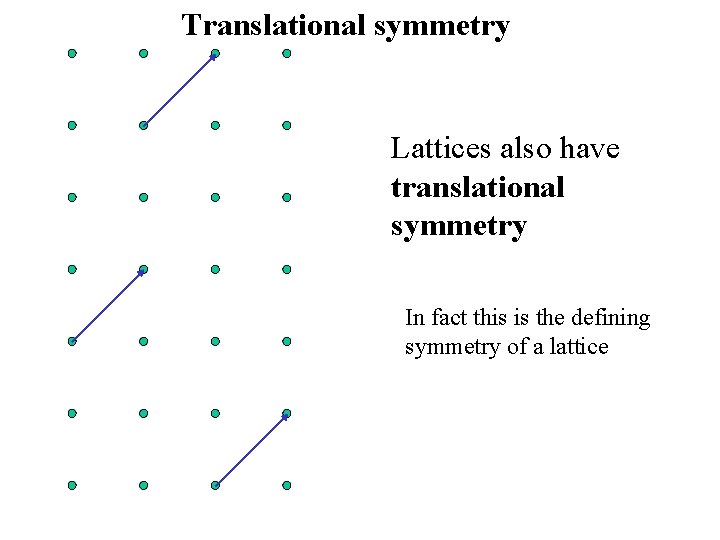
Translational symmetry Lattices also have translational symmetry In fact this is the defining symmetry of a lattice

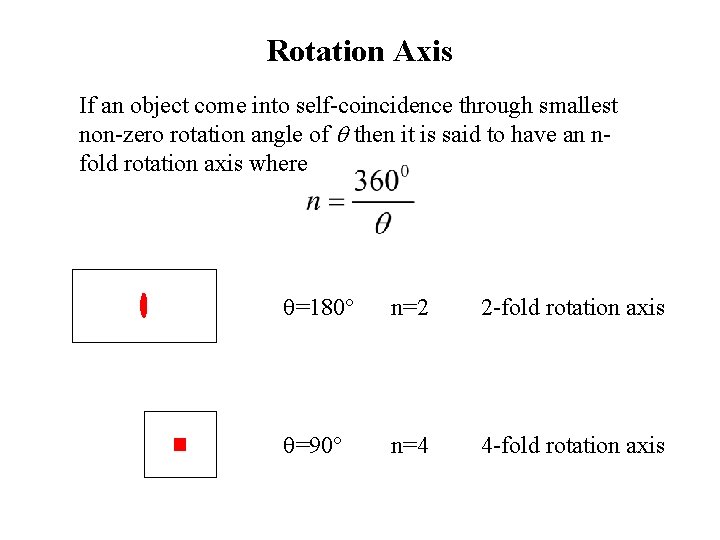
Rotation Axis If an object come into self-coincidence through smallest non-zero rotation angle of then it is said to have an nfold rotation axis where =180 n=2 2 -fold rotation axis =90 n=4 4 -fold rotation axis

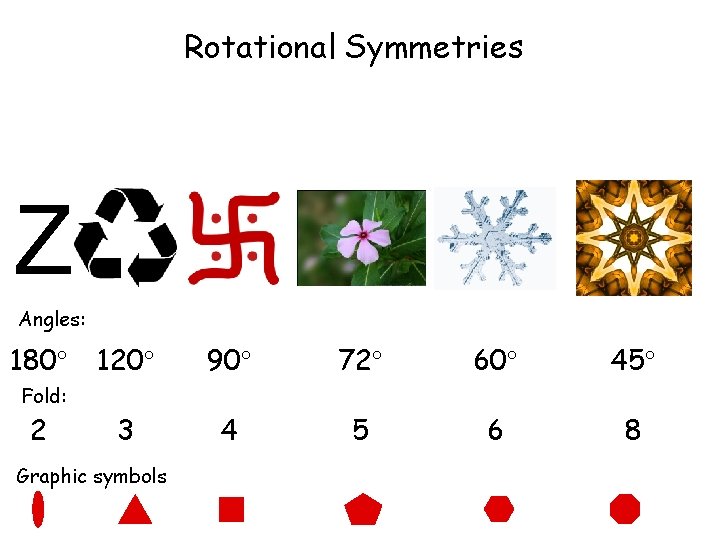
Rotational Symmetries Z Angles: 180 120 90 72 60 45 3 4 5 6 8 Fold: 2 Graphic symbols

Crsytallographic Restriction 5 -fold symmetry or Pentagonal symmetry is not possible for Periodic Tilings Symmetries higher than 6 -fold also not possible Only possible rotational symmetries for lattices 2 3 4 5 6 7 8 9…

Symmetry of lattices Lattices have Translational symmetry Rotational symmetry Reflection symmetry

Point Group and Space Group The group of all symmetry elements of a crystal except translations (e. g. rotation, reflection etc. ) is called its POINT GROUP. The complete group of all symmetry elements including translations of a crystal is called its SPACE GROUP

Classification of Lattices Crystal systems and Bravais Lattices Classification of lattices Based on the point group symmetry alone (i. e. excluding translational symmetry 7 types of lattices 7 crystal systems Based on the space group symmetry, i. e. , rotational, reflection and translational symmetry 14 types of lattices 14 Bravais lattices

7 crystal Systems 37/87 Defining symmetry 4 A single 3 1 Crystal system Conventional unit cell Cubic a=b=c, = = =90 Tetragonal a=b c, = = =90 Orthorhombic a b c, = = =90 Hexagonal a=b c, = = 90 , =120 A single Rhombohedral a=b=c, = = 90 A single Monoclinic a b c, = =90 Triclinic a b c, none

38/87 Tetragonal symmetry Cubic C = Tetragonal P Cubic symmetry Cubic F Tetragonal I

End of Lec 4 on 30. 07. 2013 Lec 2 on crystallography

Crystal Lattice A 3 D translationally periodic arrangement of atoms A 3 D translationally periodic arrangement of points

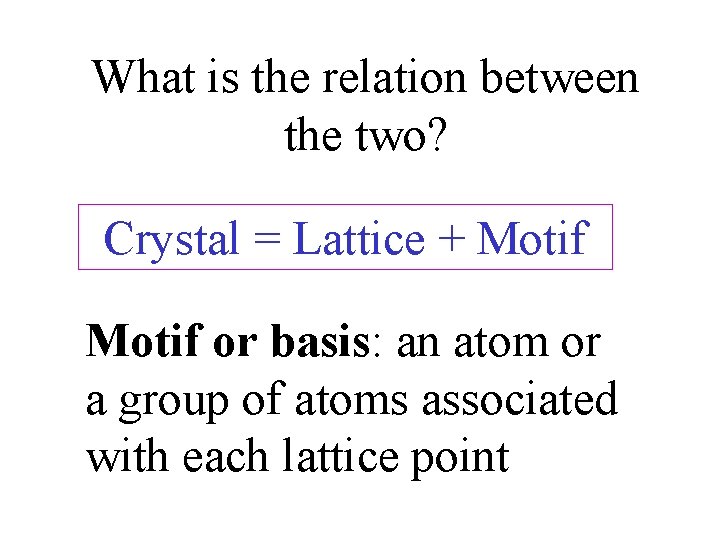
What is the relation between the two? Crystal = Lattice + Motif or basis: an atom or a group of atoms associated with each lattice point

Crystal=lattice+basis Lattice: the underlying periodicity of the crystal, Basis: atom or group of atoms associated with each lattice points Lattice: how to repeat Motif: what to repeat

Lattice + Motif = Crystal + Love Lattice + Heart = = Love Pattern

Air, Water and Earth by M. C. Esher

Every periodic pattern (and hence a crystal) has a unique lattice associated with it


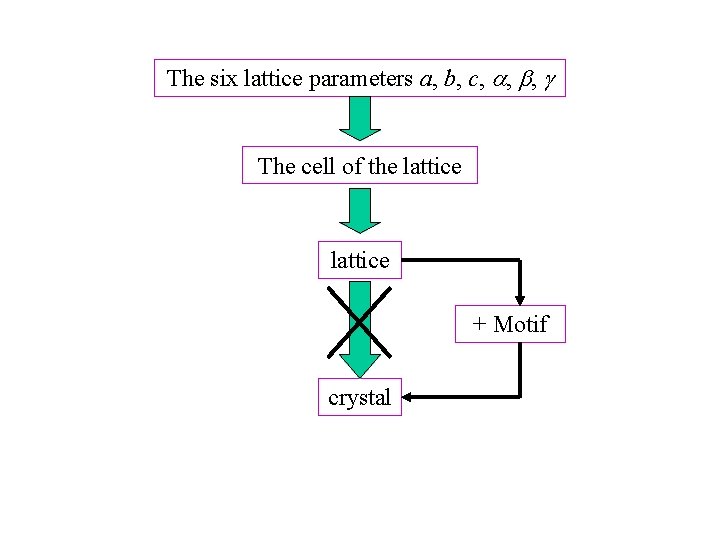
The six lattice parameters a, b, c, , , The cell of the lattice + Motif crystal

Richard P. Feynman Nobel Prize in Physics, 1965

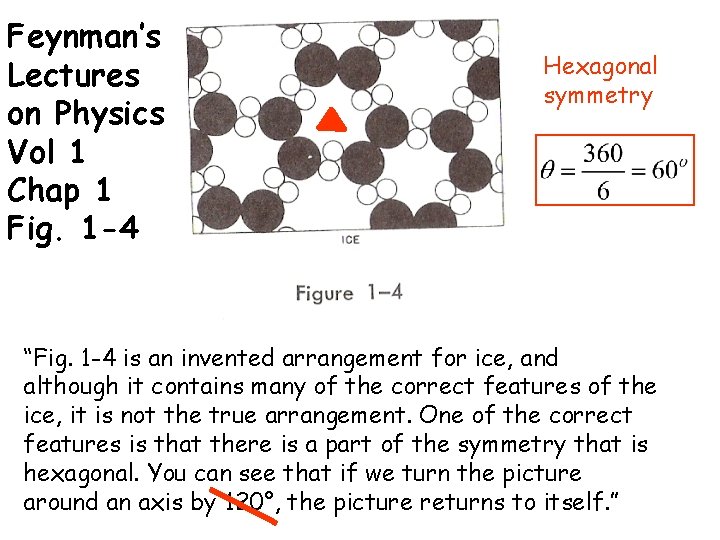
Feynman’s Lectures on Physics Vol 1 Chap 1 Fig. 1 -4 Hexagonal symmetry “Fig. 1 -4 is an invented arrangement for ice, and although it contains many of the correct features of the ice, it is not the true arrangement. One of the correct features is that there is a part of the symmetry that is hexagonal. You can see that if we turn the picture around an axis by 120°, the picture returns to itself. ”

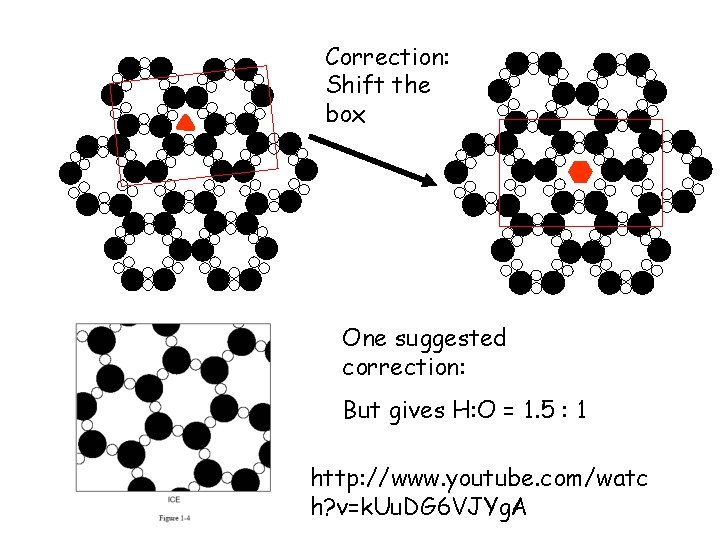
Correction: Shift the box One suggested correction: But gives H: O = 1. 5 : 1 http: //www. youtube. com/watc h? v=k. Uu. DG 6 VJYg. A

The errata has been accepted by Michael Gottlieb of Caltech and the corrections will appear in future editions Website www. feynmanlectures. info

QUESTIONS?

Miller Indices 1 Miller Indices of directions and planes William Hallowes Miller (1801 – 1880) University of Cambridge

Miller Indices 2 Miller Indices of Directions z 1. Choose a point on the direction as the origin. 2. Choose a coordinate system with axes parallel to the unit cell edges. y 3. Find the coordinates of another point on the direction in terms of a, b and c x 1 a+0 b+0 c 1, 0, 0 4. Reduce the coordinates to smallest integers. 1, 0, 0 5. Put in square brackets [100]

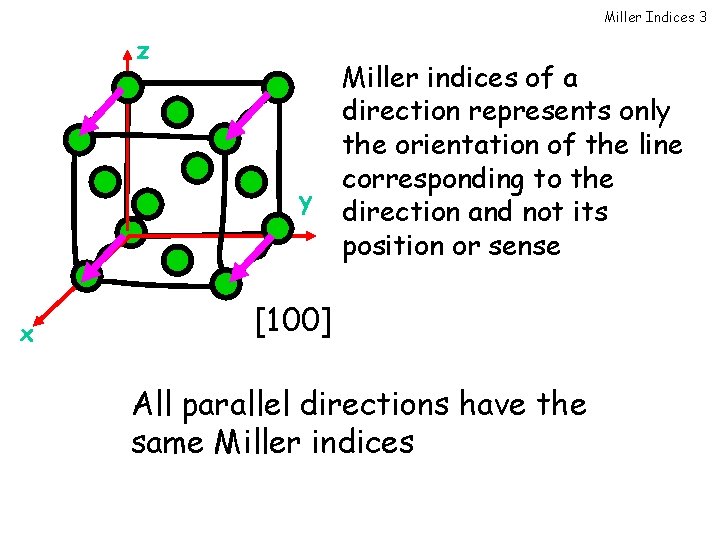
Miller Indices 3 z y x Miller indices of a direction represents only the orientation of the line corresponding to the direction and not its position or sense [100] All parallel directions have the same Miller indices

Miller Indices of Directions (contd. ) z OA=1/2 a + 1/2 b + 1 c Q A z 1/2, 1 [1 1 2] y y O P x PQ = -1 a -1 b + 1 c -1, 1 __ [111] x -ve steps are shown as bar over the number
![Miller Indices 4 Miller indices of a family of symmetry related directions uvw Miller Indices 4 Miller indices of a family of symmetry related directions = [uvw]](https://slidetodoc.com/presentation_image_h2/d4332574de8c28379e5cc1b71d6c56ed/image-57.jpg)
Miller Indices 4 Miller indices of a family of symmetry related directions = [uvw] and all other directions related to [uvw] by the symmetry of the crystal [001] Tetragonal Cubic [010] [100] = [100], [010], [001] [100] = [100], [010]

End of Lec 05 31/07/2013 Lec 3 on crystallography

Lec 6 Lec 4 on crystallography Miller Indices Continues

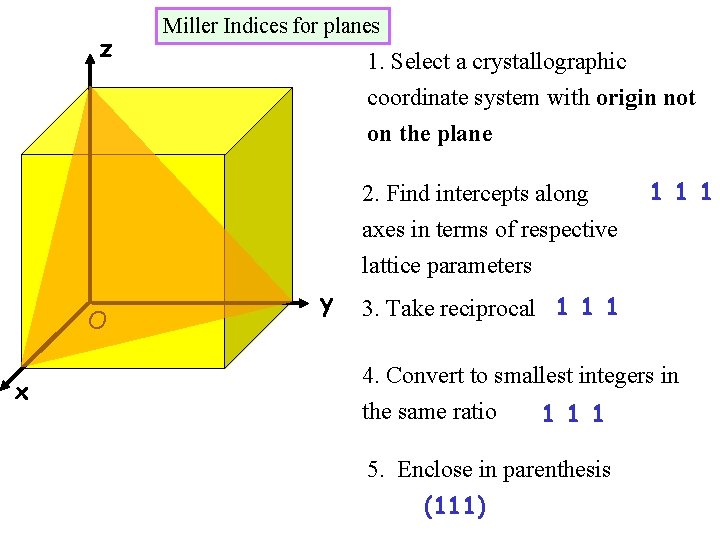
z Miller Indices for planes 1. Select a crystallographic coordinate system with origin not on the plane 2. Find intercepts along 1 1 1 axes in terms of respective lattice parameters O x y 3. Take reciprocal 1 1 1 4. Convert to smallest integers in the same ratio 1 1 1 5. Enclose in parenthesis (111)

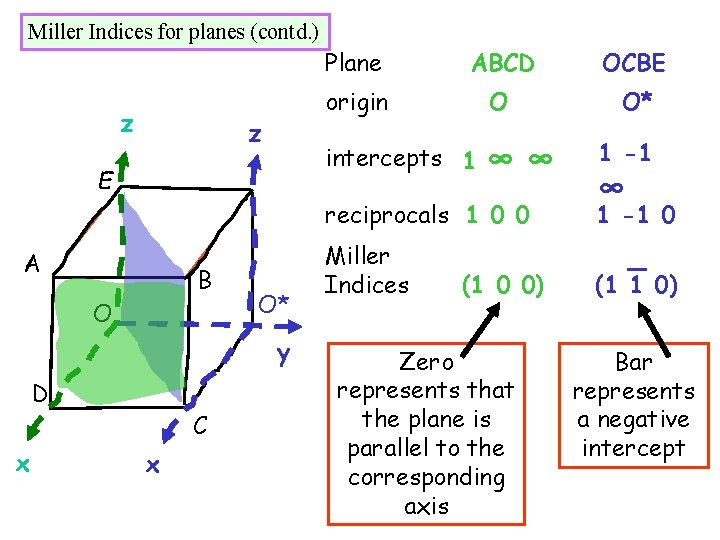
Miller Indices for planes (contd. ) z z E Plane ABCD OCBE origin O O* intercepts 1 ∞ ∞ reciprocals 1 0 0 A B O O* y D x C x Miller Indices (1 0 0) Zero represents that the plane is parallel to the corresponding axis 1 -1 ∞ 1 -1 0 _ (1 1 0) Bar represents a negative intercept

Miller indices of a plane specifies only its orientation in space not its position z E All parallel planes have the same Miller Indices _ _ _ (h k l ) A B O y D C x (100) _ (100)

Z Y X

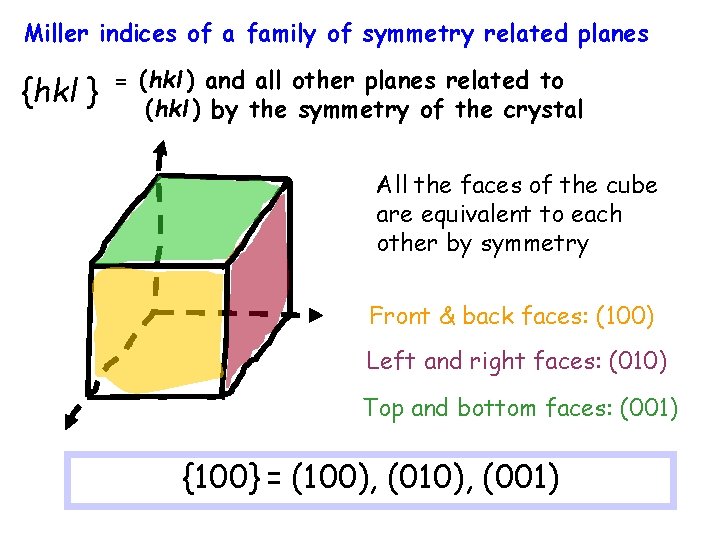
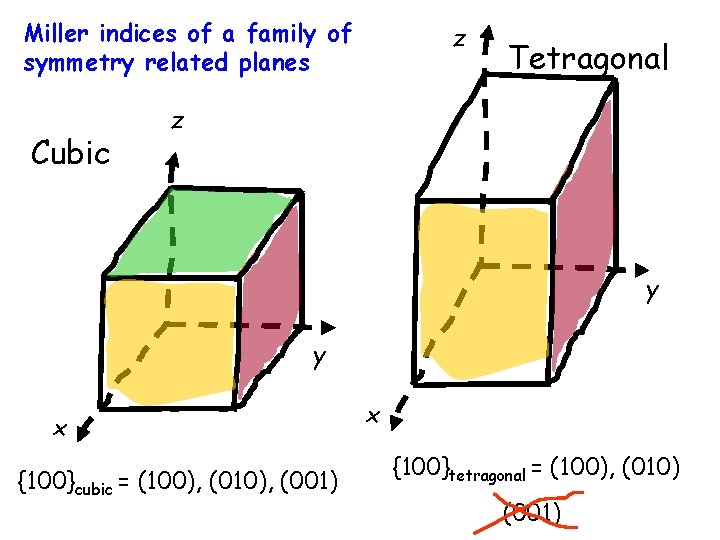
Miller indices of a family of symmetry related planes {hkl } = (hkl ) and all other planes related to (hkl ) by the symmetry of the crystal All the faces of the cube are equivalent to each other by symmetry Front & back faces: (100) Left and right faces: (010) Top and bottom faces: (001) {100} = (100), (010), (001)

Miller indices of a family of symmetry related planes Cubic z Tetragonal z y y x {100}cubic = (100), (010), (001) x {100}tetragonal = (100), (010) (001)

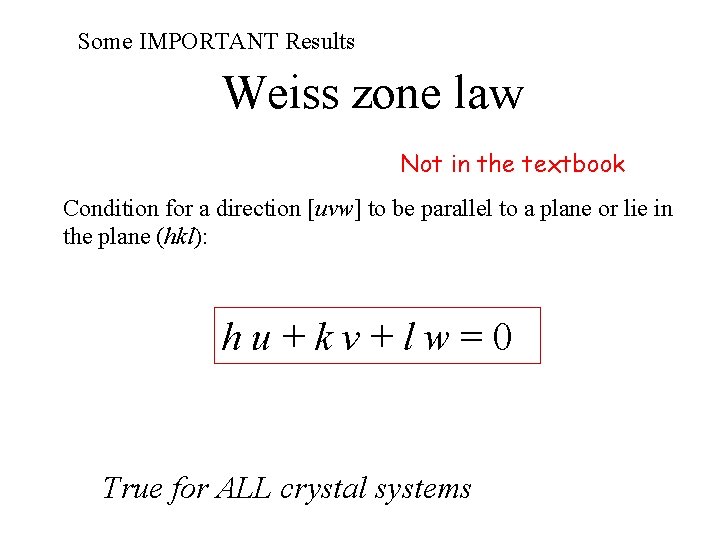
Some IMPORTANT Results Weiss zone law Not in the textbook Condition for a direction [uvw] to be parallel to a plane or lie in the plane (hkl): hu+kv+lw=0 True for ALL crystal systems
![CUBIC CRYSTALS 111 hkl hkl 111 Angle between two directions h 1 k 1 CUBIC CRYSTALS [111] [hkl] (hkl) (111) Angle between two directions [h 1 k 1](https://slidetodoc.com/presentation_image_h2/d4332574de8c28379e5cc1b71d6c56ed/image-67.jpg)
CUBIC CRYSTALS [111] [hkl] (hkl) (111) Angle between two directions [h 1 k 1 l 1] and [h 2 k 2 l 2]: C

dhkl Interplanar spacing between ‘successive’ (hkl) planes passing through the corners of the unit cell z E B O x (100) O x
![Summary of Notation convention for Indices uvw Miller indices of a direction i e Summary of Notation convention for Indices [uvw] Miller indices of a direction (i. e.](https://slidetodoc.com/presentation_image_h2/d4332574de8c28379e5cc1b71d6c56ed/image-69.jpg)
Summary of Notation convention for Indices [uvw] Miller indices of a direction (i. e. a set of parallel directions) (hkl) Miller Indices of a plane (i. e. a set of parallel planes) <uvw> Miller indices of a family of symmetry related directions {hkl} Miller indices of a family of symmetry related planes

In the fell clutch of circumstance I have not winced nor cried aloud. Under the bludgeonings of chance My head is bloody, but unbowed. From "Invictus" by William Ernest Henley (1849– 1903).

End of Lecture 6 Lec 4 on crystallography

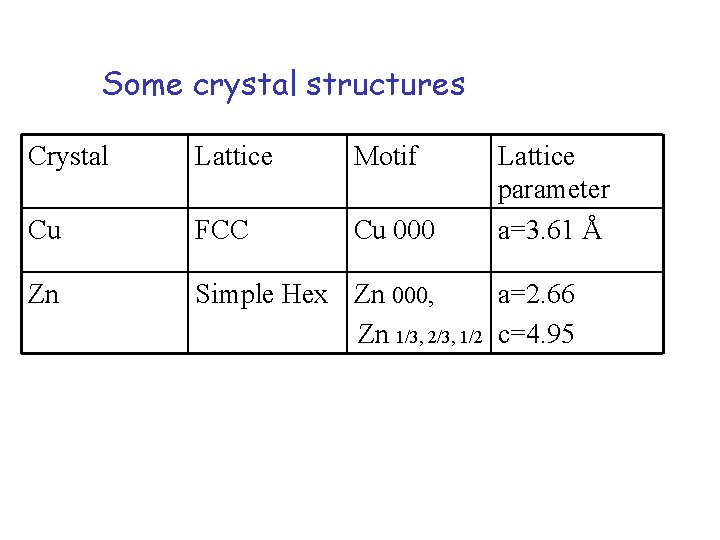
Some crystal structures Crystal Lattice Motif Lattice parameter a=3. 61 Å Cu FCC Cu 000 Zn Simple Hex Zn 000, a=2. 66 Zn 1/3, 2/3, 1/2 c=4. 95

Q 1: How do we determine the crystal structure?

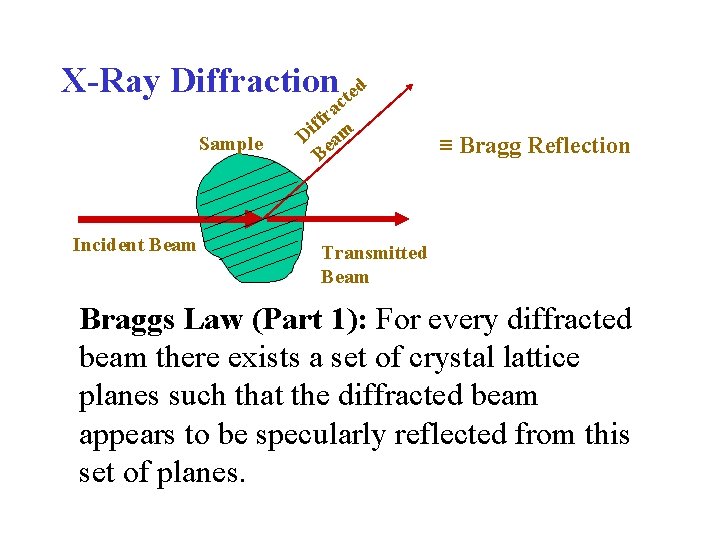
X-Ray Diffraction d e t c am e B a r f if D Sample Incident Beam Transmitted Beam Diffracted Beam

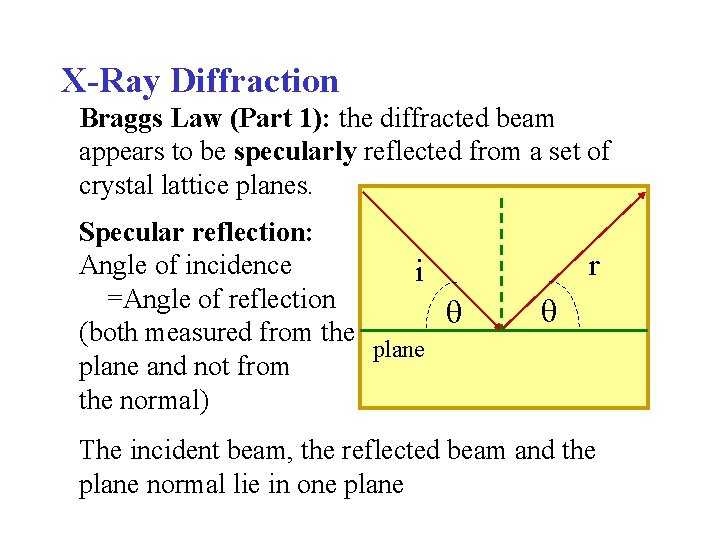
X-Ray Diffraction cted Sample Incident Beam a r f f m i D ea B ≡ Bragg Reflection Transmitted Beam Braggs Law (Part 1): For every diffracted beam there exists a set of crystal lattice planes such that the diffracted beam appears to be specularly reflected from this set of planes.

X-Ray Diffraction Braggs’ recipe for Nobel prize? Call the diffraction a reflection!!! “The important thing in science is not so much to obtain new facts as to discover new ways of thinking about them”. W. L. Bragg


X-Ray Diffraction Braggs Law (Part 1): the diffracted beam appears to be specularly reflected from a set of crystal lattice planes. Specular reflection: Angle of incidence i =Angle of reflection (both measured from the plane and not from the normal) r The incident beam, the reflected beam and the plane normal lie in one plane

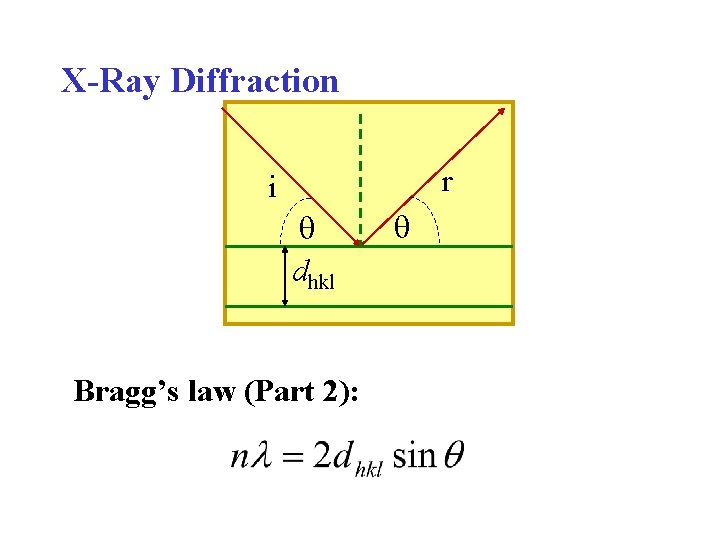
X-Ray Diffraction r i dhkl Bragg’s law (Part 2):

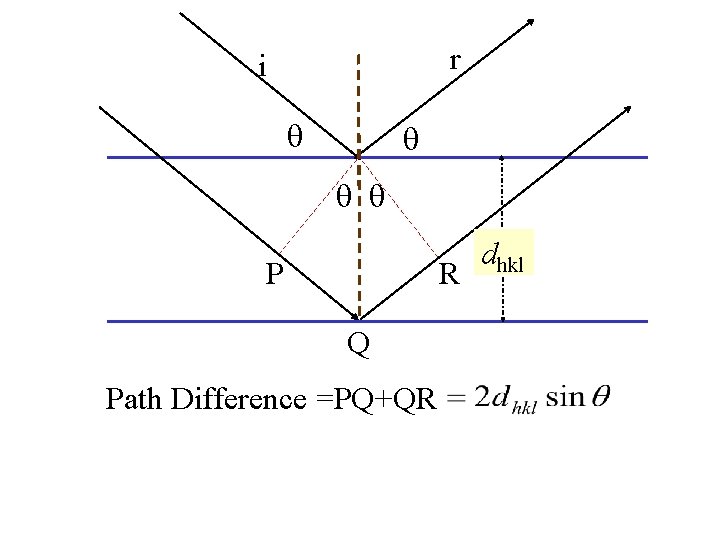
r i P R Q Path Difference =PQ+QR dhkl

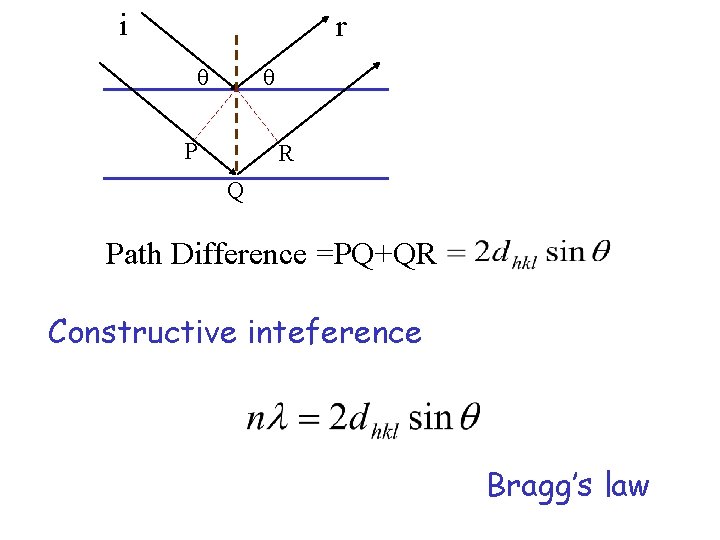
i r P R Q Path Difference =PQ+QR Constructive inteference Bragg’s law

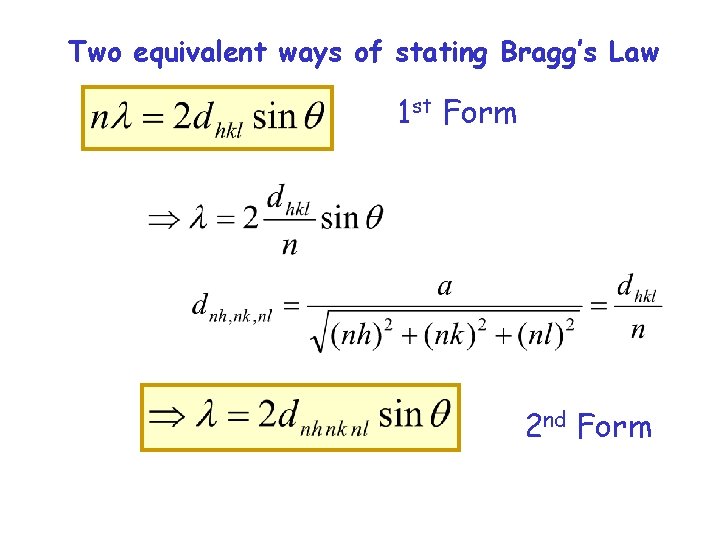
Two equivalent ways of stating Bragg’s Law 1 st Form 2 nd Form

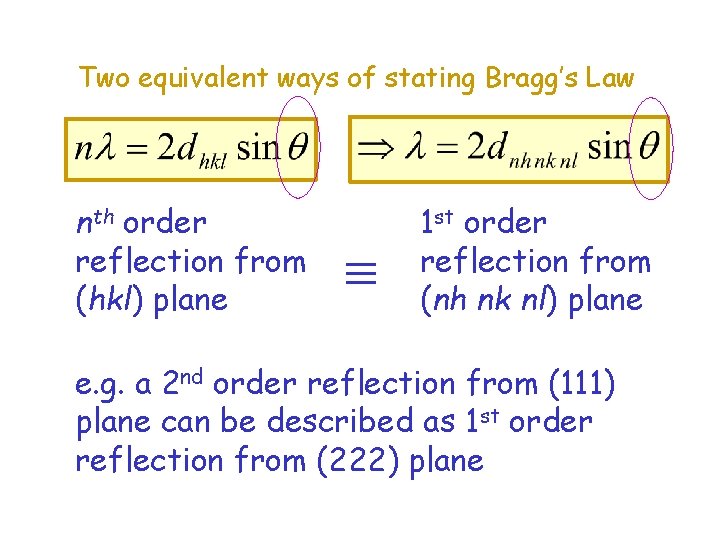
Two equivalent ways of stating Bragg’s Law nth order reflection from (hkl) plane 1 st order reflection from (nh nk nl) plane e. g. a 2 nd order reflection from (111) plane can be described as 1 st order reflection from (222) plane

X-rays Characteristic Radiation, K Target Mo Cu Co Fe Cr Wavelength, Å 0. 71 1. 54 1. 79 1. 94 2. 29

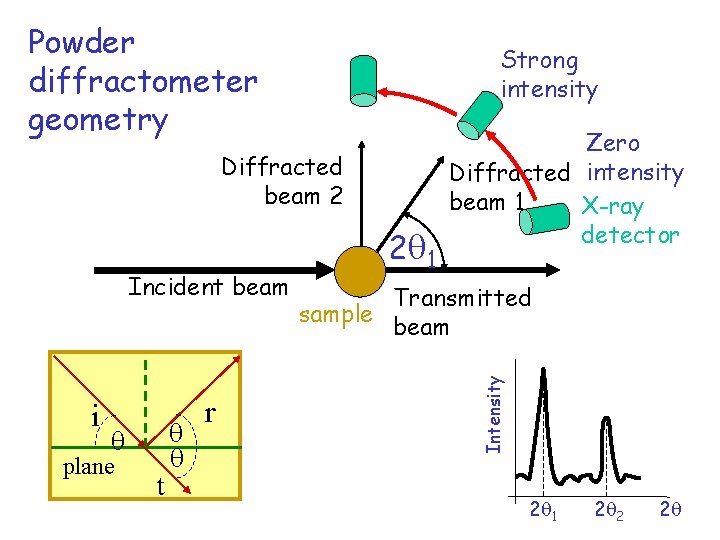
Powder Method is fixed (K radiation) is variable – specimen consists of millions of powder particles – each being a crystallite and these are randomly oriented in space – amounting to the rotation of a crystal about all possible axes

Powder diffractometer geometry Strong intensity Diffracted beam 2 i plane t r Transmitted sample beam Intensity Incident beam 2 1 Zero Diffracted intensity beam 1 X-ray detector 2 1 2 2 2

Crystal monochromator detector X-ray tube X-ray powder diffractometer

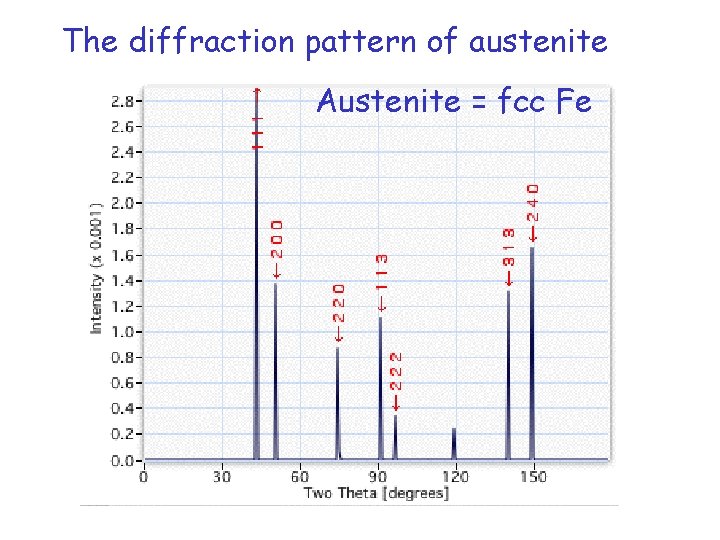
The diffraction pattern of austenite Austenite = fcc Fe

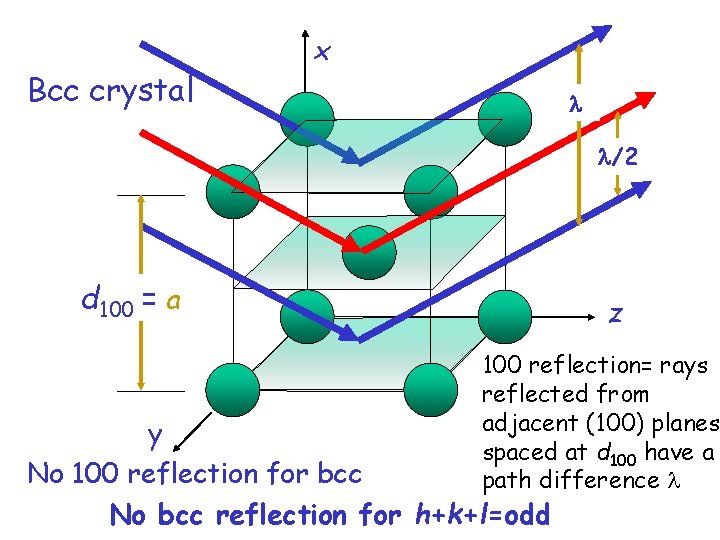
Bcc crystal x /2 d 100 = a z 100 reflection= rays reflected from adjacent (100) planes spaced at d 100 have a path difference y No 100 reflection for bcc No bcc reflection for h+k+l=odd

Extinction Rules: Table 3. 3 Bravais Lattice Allowed Reflections SC All BCC (h + k + l) even FCC h, k and l unmixed DC h, k and l are all odd Or if all are even then (h + k + l) divisible by 4

End of Lec 7 06/08/13 Lec 5 on crystallography (Last lecture)

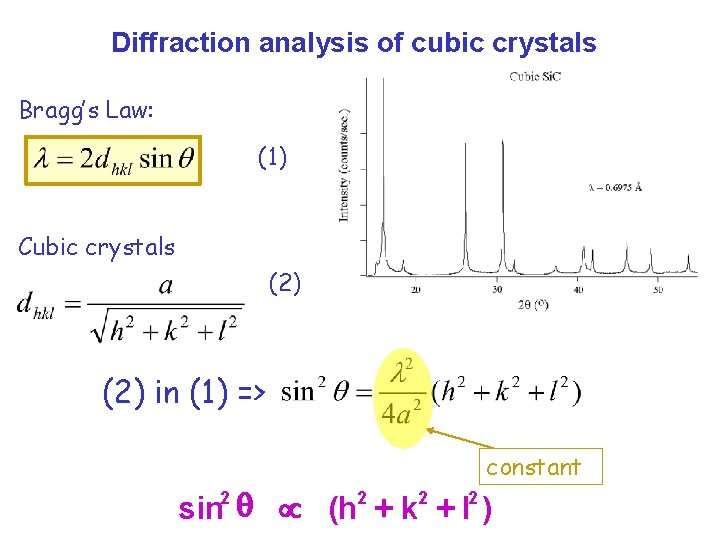
Diffraction analysis of cubic crystals Bragg’s Law: (1) Cubic crystals (2) in (1) => constant sin q µ (h + k + l ) 2 2

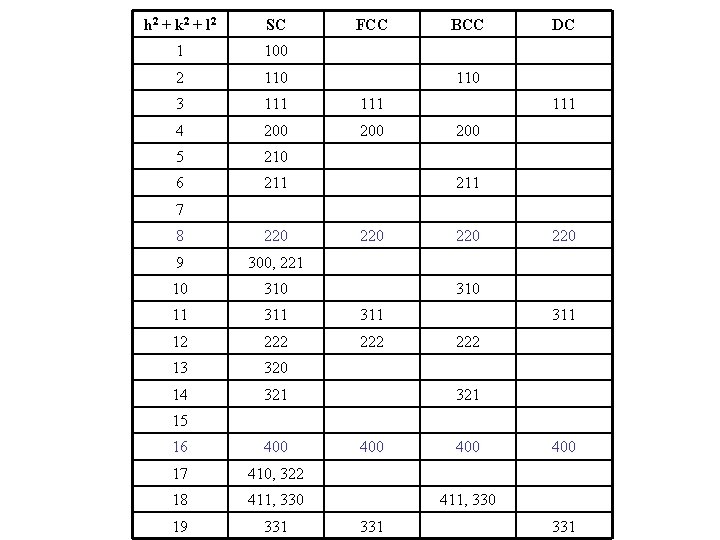
h 2 + k 2 + l 2 SC FCC 1 100 2 110 3 111 4 200 5 210 6 211 BCC DC 110 111 200 211 7 8 220 9 300, 221 10 310 11 311 12 222 13 320 14 321 220 310 311 222 321 15 16 400 17 410, 322 18 411, 330 19 331 400 400 411, 330 331

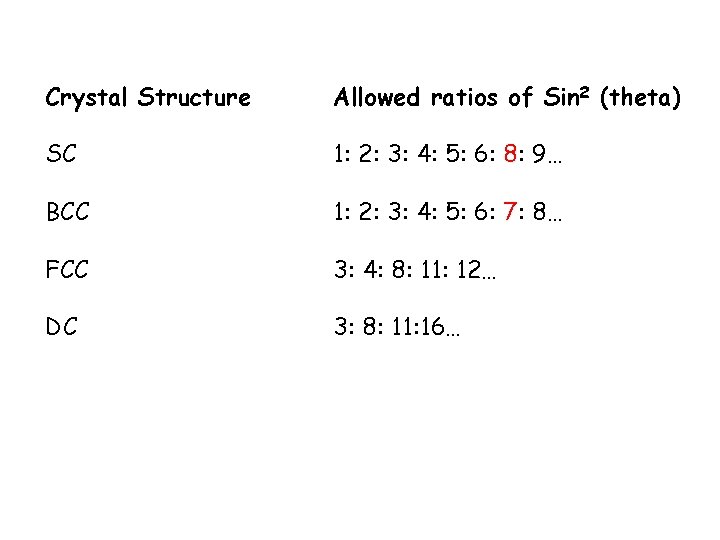
Crystal Structure Allowed ratios of Sin 2 (theta) SC 1: 2: 3: 4: 5: 6: 8: 9… BCC 1: 2: 3: 4: 5: 6: 7: 8… FCC 3: 4: 8: 11: 12… DC 3: 8: 11: 16…

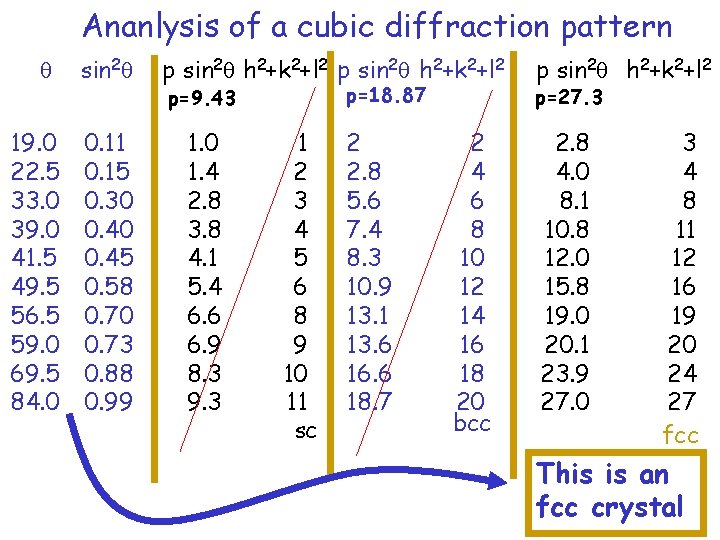
Ananlysis of a cubic diffraction pattern 19. 0 22. 5 33. 0 39. 0 41. 5 49. 5 56. 5 59. 0 69. 5 84. 0 sin 2 0. 11 0. 15 0. 30 0. 45 0. 58 0. 70 0. 73 0. 88 0. 99 p sin 2 h 2+k 2+l 2 p=18. 87 p=9. 43 1. 0 1. 4 2. 8 3. 8 4. 1 5. 4 6. 6 6. 9 8. 3 9. 3 1 2 3 4 5 6 8 9 10 11 sc 2 2. 8 5. 6 7. 4 8. 3 10. 9 13. 1 13. 6 16. 6 18. 7 2 4 6 8 10 12 14 16 18 20 bcc p sin 2 h 2+k 2+l 2 p=27. 3 2. 8 4. 0 8. 1 10. 8 12. 0 15. 8 19. 0 20. 1 23. 9 27. 0 3 4 8 11 12 16 19 20 24 27 fcc This is an fcc crystal

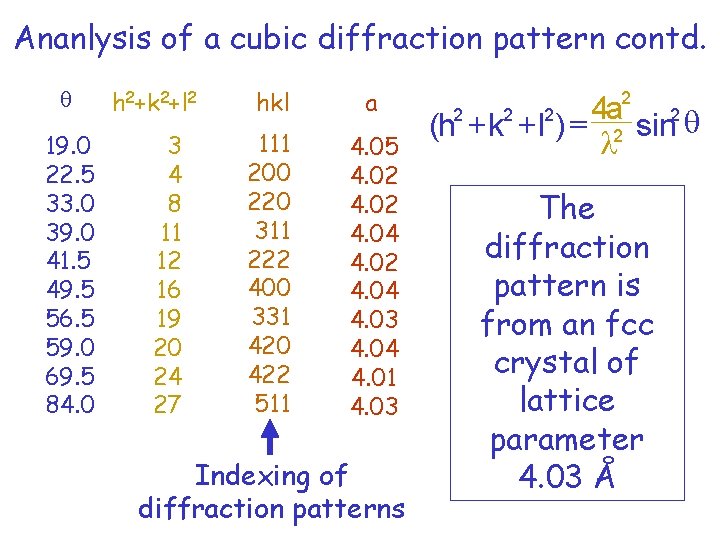
Ananlysis of a cubic diffraction pattern contd. 19. 0 22. 5 33. 0 39. 0 41. 5 49. 5 56. 5 59. 0 69. 5 84. 0 h 2+k 2+l 2 3 4 8 11 12 16 19 20 24 27 hkl a 111 200 220 311 222 400 331 420 422 511 4. 05 4. 02 4. 04 4. 03 4. 04 4. 01 4. 03 Indexing of diffraction patterns 2 4 a (h + k + l ) = 2 sin 2 2 The diffraction pattern is from an fcc crystal of lattice parameter 4. 03 Å

Education is an admirable thing, but it is well to remember from time to time that nothing that is worth knowing can be taught. -Oscar Wilde

A father-son team that shared a Nobel Prize William Henry Bragg (1862– 1942), William Lawrence Bragg (1890– 1971) Nobel Prize (1915)

One of the greatest scientific discoveries of twentieth century

Max von Laue, 1879 -1960 Nobel 1914

Two Questions Q 1: X-rays waves or particles? Father Bragg: Particles Son Bragg: Waves “Even after they shared a Nobel Prize in 1915, … this tension persisted…” – Ioan James in Remarkable Physicists Q 2: Crystals: Perodic arrangement of atoms? X-RAY DIFFRACTION: X-rays are waves and crystals are periodic arrangement of atoms

If it is permissible to evaluate a human discovery according to the fruits which it bears then there are not many discoveries ranking on par with that made by von Laue. -from Nobel Presentation Talk




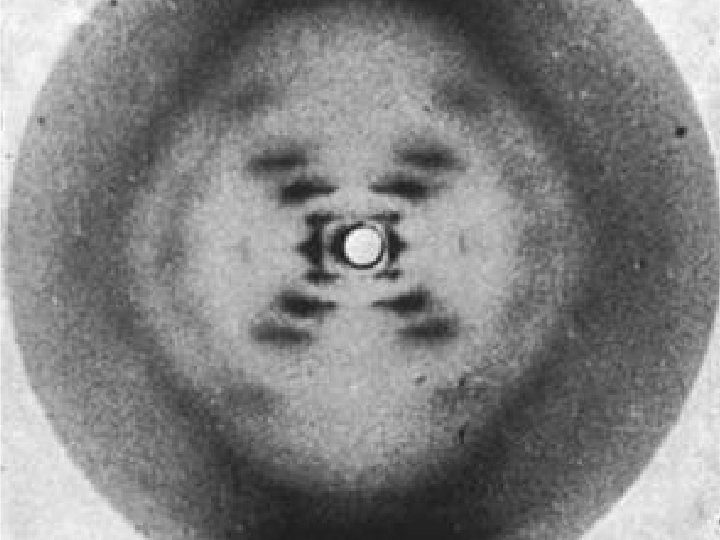
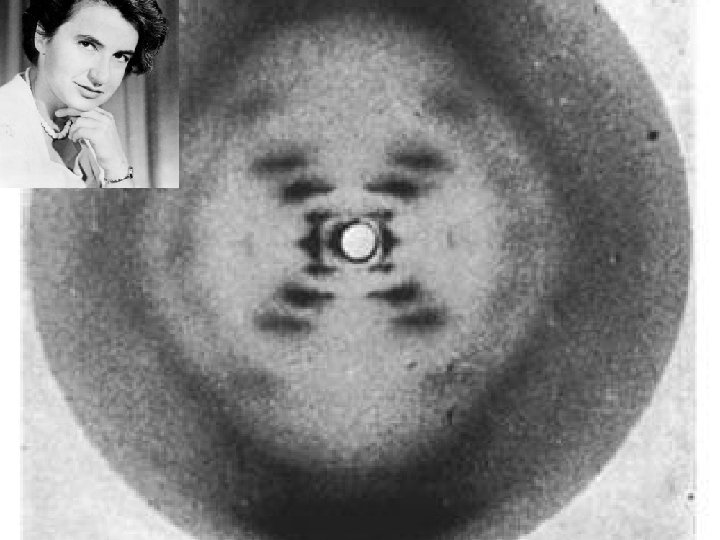
Watson, Crick and Wilkins Nobel Prize, 1962

Rosalind Elsie Franklin


Rosy, of course, did not directly give us her data. For that matter, no one at King's realized they were in our hands. J. D. Watson "However, the data which really helped us to obtain the structure was mainly obtained by Rosalind Franklin". -Francis Crick


Google Doodle on 25 th July, 2013 Franklins birth centenary 25 July 2020

Rosalind Elsie Franklin 25 July 1920 – 16 April 1958
 Antigentest åre
Antigentest åre There are only two things that are infinite
There are only two things that are infinite Ecosystem living and nonliving things
Ecosystem living and nonliving things The seven life processes of living things
The seven life processes of living things All things denote there is a god
All things denote there is a god There is there are ejemplos
There is there are ejemplos There is there are part of speech
There is there are part of speech There is there are negative form
There is there are negative form There are there is
There are there is There aren't any carrots
There aren't any carrots There is there are
There is there are Eight dollars the price of a movie these days
Eight dollars the price of a movie these days Here there over there
Here there over there A an any some правило
A an any some правило Cheese contable o incontable
Cheese contable o incontable There is and there
There is and there There was there were ile ilgili cümleler
There was there were ile ilgili cümleler There is there are exercises
There is there are exercises There is there are
There is there are There
There Sightseeing countable or uncountable
Sightseeing countable or uncountable If there is no struggle there is no progress examples
If there is no struggle there is no progress examples Two pillars of web of things
Two pillars of web of things Contrastive structure is used to compare two things.
Contrastive structure is used to compare two things. What two things do tree rings indicate
What two things do tree rings indicate A simile compares two things
A simile compares two things Whats the definition of simile
Whats the definition of simile A comparison of two dissimilar things using the word like
A comparison of two dissimilar things using the word like Figurative language comparing two things
Figurative language comparing two things Metaphor in the poem the road not taken
Metaphor in the poem the road not taken Quickly comparative
Quickly comparative Literary devices comparison
Literary devices comparison Let's compare two things
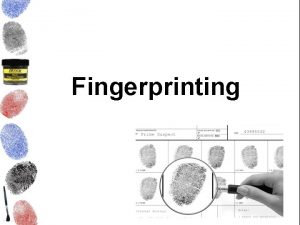
Let's compare two things Ninhydrin fingerprints
Ninhydrin fingerprints What two things determine the strength of gravity
What two things determine the strength of gravity Comparing things without using like or as
Comparing things without using like or as Two characteristics of living things
Two characteristics of living things In your notebook write the correct word for each definition
In your notebook write the correct word for each definition Homeric simile definition
Homeric simile definition Poem repetition examples
Poem repetition examples Rotation causes seasons true or false
Rotation causes seasons true or false Objective of first aider
Objective of first aider School system england
School system england He had two sons
He had two sons Yes there are two paths
Yes there are two paths Singular and plural pronouns chart
Singular and plural pronouns chart Likability as a brand elements include
Likability as a brand elements include There are two special forms of verbs
There are two special forms of verbs Two basic types
Two basic types There are two equal and opposite errors
There are two equal and opposite errors Once upon a time there lived a little girl
Once upon a time there lived a little girl The little one said roll over
The little one said roll over Some factors that personalize our footwear include:
Some factors that personalize our footwear include: Dollz aim
Dollz aim Slds aim osu
Slds aim osu Auto transformer construction
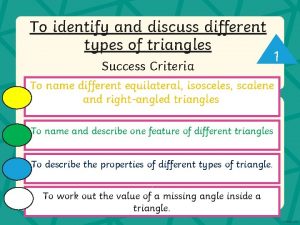
Auto transformer construction Different types of triangles
Different types of triangles The aims of scouting
The aims of scouting Aim suffix
Aim suffix Aimradc
Aimradc Resistance welding
Resistance welding Aim isen
Aim isen Applications of fractional distillation
Applications of fractional distillation Defects of existing curriculum
Defects of existing curriculum Aim project management
Aim project management Pans aim doc 10066
Pans aim doc 10066 What is the purpose of narrative
What is the purpose of narrative Aim sample
Aim sample Role of supervisor in microteaching
Role of supervisor in microteaching The aim chemistry
The aim chemistry Conclusion of indian education commission (1964-66)
Conclusion of indian education commission (1964-66) Kothari commission agencies india
Kothari commission agencies india Ifr flight planning
Ifr flight planning Ansp swim
Ansp swim The legal aid board objectives
The legal aid board objectives Filtration objective
Filtration objective Health and social care unit 5 coursework example p2
Health and social care unit 5 coursework example p2 Learning aim c btec business
Learning aim c btec business Unit 14 learning aim d
Unit 14 learning aim d Unit 14 learning aim d
Unit 14 learning aim d Aims of pollution
Aims of pollution Aims and objectives of establishment of pakistan in urdu
Aims and objectives of establishment of pakistan in urdu Spartan aim
Spartan aim What is previewing
What is previewing Open door policy aim
Open door policy aim Observation vs inference worksheet doc
Observation vs inference worksheet doc Suffix -acy
Suffix -acy G3nrw
G3nrw Aim to flourish
Aim to flourish Aim words list
Aim words list Seminar objectives
Seminar objectives Aim of health psychology
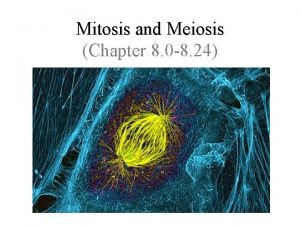
Aim of health psychology Purpose of mitosis
Purpose of mitosis When to use did or done
When to use did or done Health education ki definition
Health education ki definition Health and social care component 2 learning aim b example
Health and social care component 2 learning aim b example Quadruple aim
Quadruple aim Laney et al
Laney et al Goals of clinical supervision
Goals of clinical supervision Aim of argumentative essay
Aim of argumentative essay Scientific method aim
Scientific method aim Aim what
Aim what Aim 12 forces
Aim 12 forces Aim 13
Aim 13 Aim for success
Aim for success Advantages and disadvantages essay structure
Advantages and disadvantages essay structure Classification of rapid tooling
Classification of rapid tooling Ann's bad aim
Ann's bad aim Aim in psychology
Aim in psychology What is the main aim of commercial farming
What is the main aim of commercial farming Augustan poetry aim
Augustan poetry aim The great aim of education is not knowledge
The great aim of education is not knowledge Aim of classification
Aim of classification