Chapter 9 Properties of Transformations Translate Figures and






















- Slides: 90

Chapter 9 Properties of Transformations

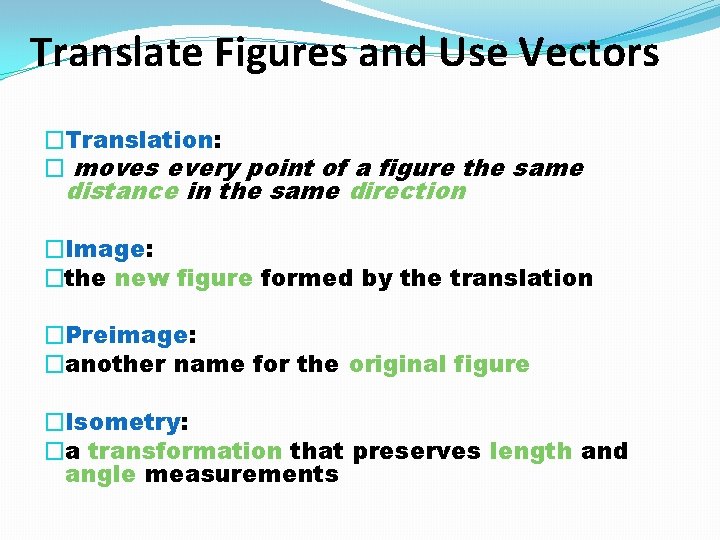
Translate Figures and Use Vectors �Translation: � moves every point of a figure the same distance in the same direction �Image: �the new figure formed by the translation �Preimage: �another name for the original figure �Isometry: �a transformation that preserves length and angle measurements

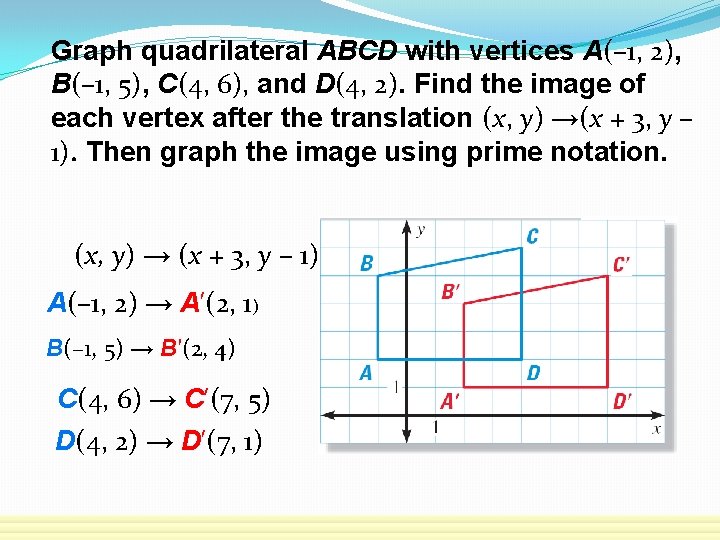
Graph quadrilateral ABCD with vertices A(– 1, 2), B(– 1, 5), C(4, 6), and D(4, 2). Find the image of each vertex after the translation (x, y) →(x + 3, y – 1). Then graph the image using prime notation. SOLUTION First, draw ABCD. Find the translation of each vertex by adding 3 to its x-coordinate and subtracting 1 from its y-coordinate. Then graph the image.

Graph quadrilateral ABCD with vertices A(– 1, 2), B(– 1, 5), C(4, 6), and D(4, 2). Find the image of each vertex after the translation (x, y) →(x + 3, y – 1). Then graph the image using prime notation. (x, y) → (x + 3, y – 1) A(– 1, 2) → A′(2, 1) B(– 1, 5) → B′(2, 4) C(4, 6) → C′(7, 5) D(4, 2) → D′(7, 1)

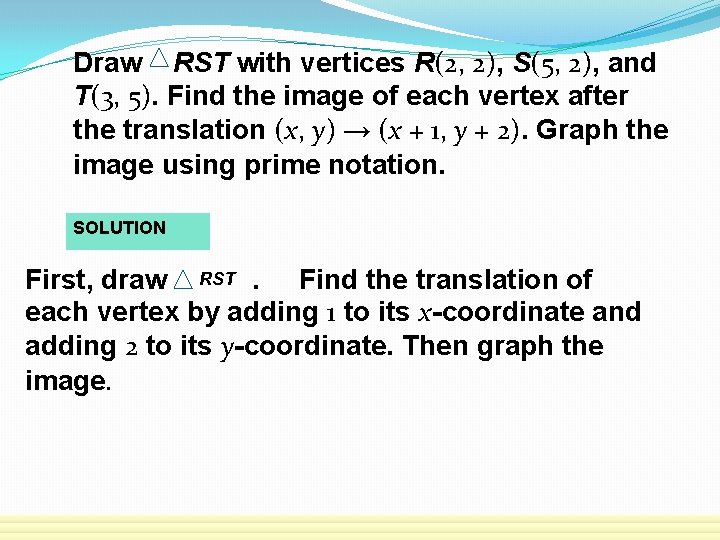
Draw RST with vertices R(2, 2), S(5, 2), and T(3, 5). Find the image of each vertex after the translation (x, y) → (x + 1, y + 2). Graph the image using prime notation. SOLUTION First, draw RST. Find the translation of each vertex by adding 1 to its x-coordinate and adding 2 to its y-coordinate. Then graph the image.

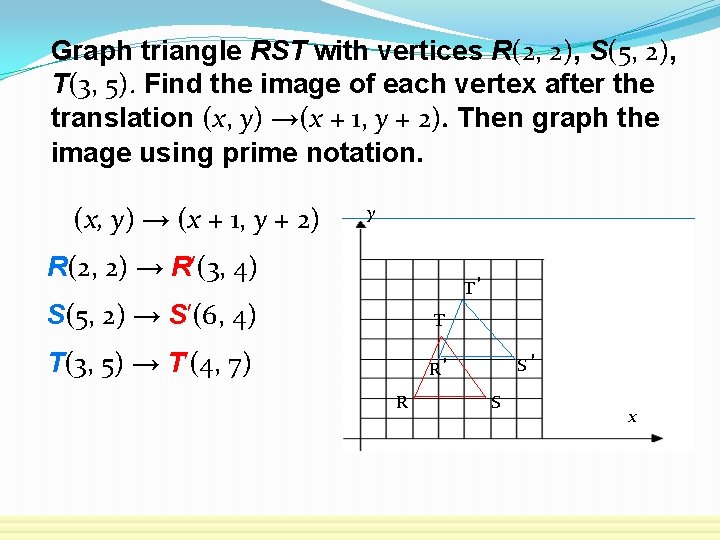
Graph triangle RST with vertices R(2, 2), S(5, 2), T(3, 5). Find the image of each vertex after the translation (x, y) →(x + 1, y + 2). Then graph the image using prime notation. (x, y) → (x + 1, y + 2) y R(2, 2) → R′(3, 4) T′ S(5, 2) → S′(6, 4) T T(3, 5) → T′(4, 7) R′ R S′ S x

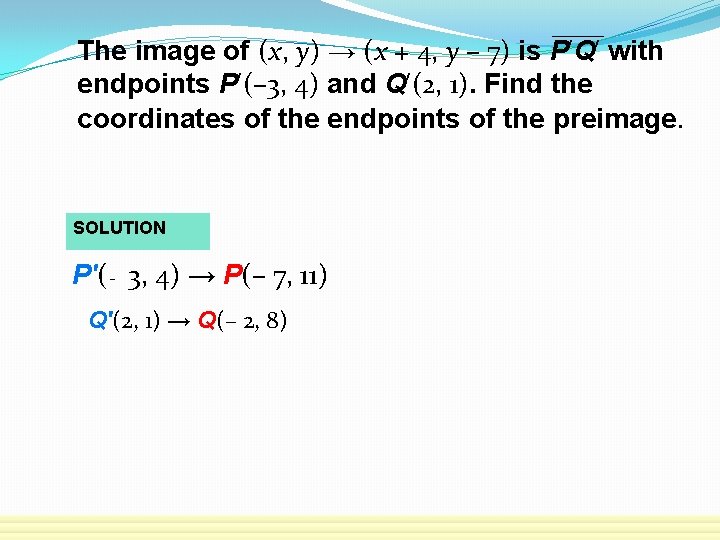
The image of (x, y) → (x + 4, y – 7) is P′Q′ with endpoints P′(– 3, 4) and Q′(2, 1). Find the coordinates of the endpoints of the preimage. SOLUTION P'( - 3, 4) → P(– 7, 11) Q'(2, 1) → Q(– 2, 8)

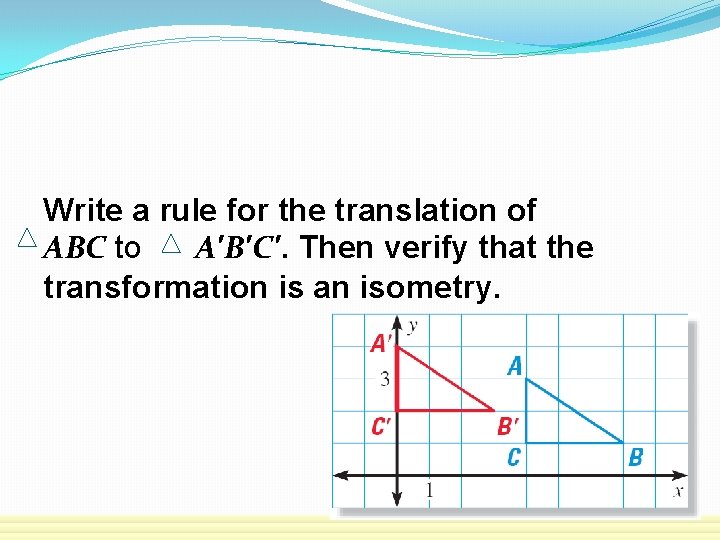
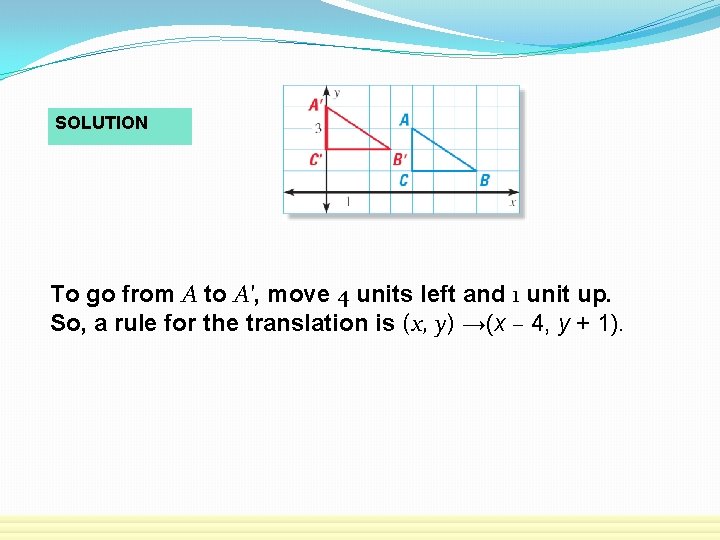
Write a rule for the translation of ABC to A′B′C′. Then verify that the transformation is an isometry.

SOLUTION To go from A to A′, move 4 units left and 1 unit up. So, a rule for the translation is (x, y) →(x – 4, y + 1).

�Vectors: �a quantity that has both direction and magnitude and is represented in the coordinate plane by an arrow drawn from one point to another. �Initial point: �the starting point of the vector �Terminal point: �the ending point of the vector

�Component form: � combines the horizontal and vertical components �Horizontal component goes left to right. �Vertical component goes up and down.

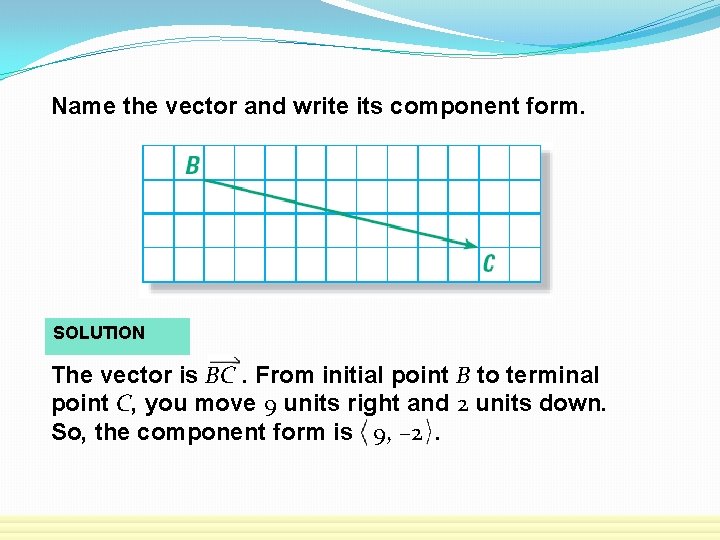
Name the vector and write its component form. SOLUTION The vector is BC. From initial point B to terminal point C, you move 9 units right and 2 units down. So, the component form is 9, – 2.

Name the vector and write its component form. SOLUTION The vector is ST. From initial point S to terminal point T, you move 8 units left and 0 units vertically. The component form is – 8, 0.

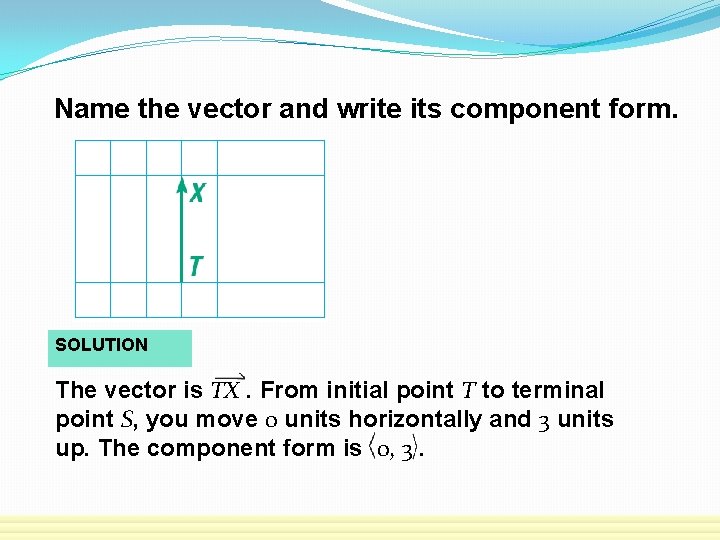
Name the vector and write its component form. SOLUTION The vector is TX. From initial point T to terminal point S, you move 0 units horizontally and 3 units up. The component form is 0, 3.

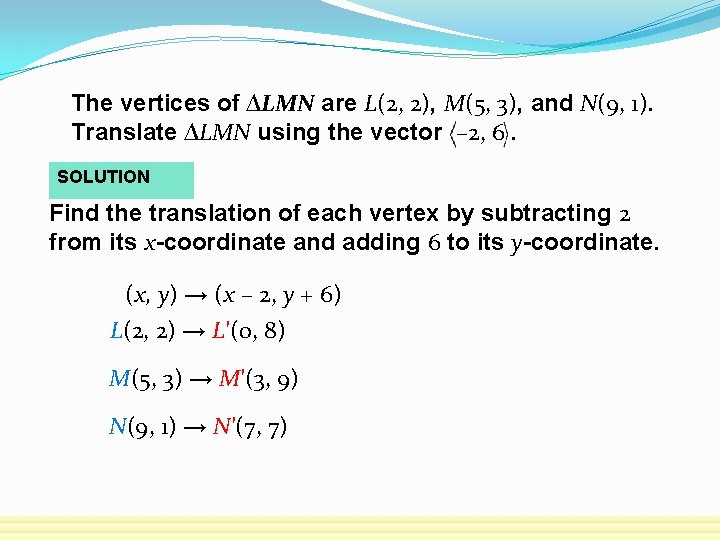
The vertices of ∆LMN are L(2, 2), M(5, 3), and N(9, 1). Translate ∆LMN using the vector – 2, 6. SOLUTION Find the translation of each vertex by subtracting 2 from its x-coordinate and adding 6 to its y-coordinate. (x, y) → (x – 2, y + 6) L(2, 2) → L′(0, 8) M(5, 3) → M′(3, 9) N(9, 1) → N′(7, 7)

Lesson – 9. 3 Perform Reflections Reflection: a transformation that uses a line like a mirror to reflect an image Line of reflection: a line that acts like a mirror to reflect an image.

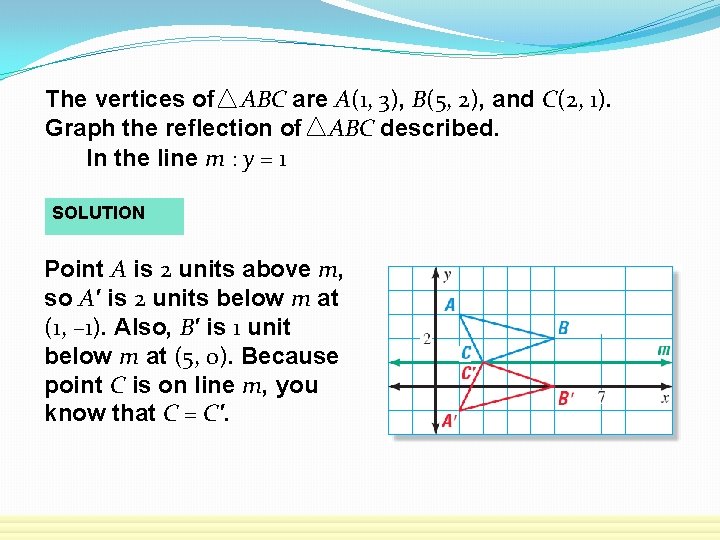
The vertices of ABC are A(1, 3), B(5, 2), and C(2, 1). Graph the reflection of ABC described. In the line m : y = 1 SOLUTION Point A is 2 units above m, so A′ is 2 units below m at (1, – 1). Also, B′ is 1 unit below m at (5, 0). Because point C is on line m, you know that C = C′.

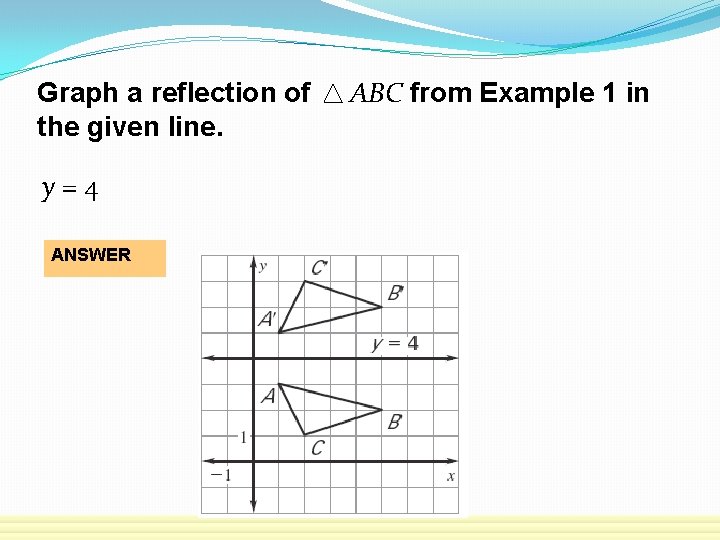
Graph a reflection of the given line. y=4 ANSWER ABC from Example 1 in

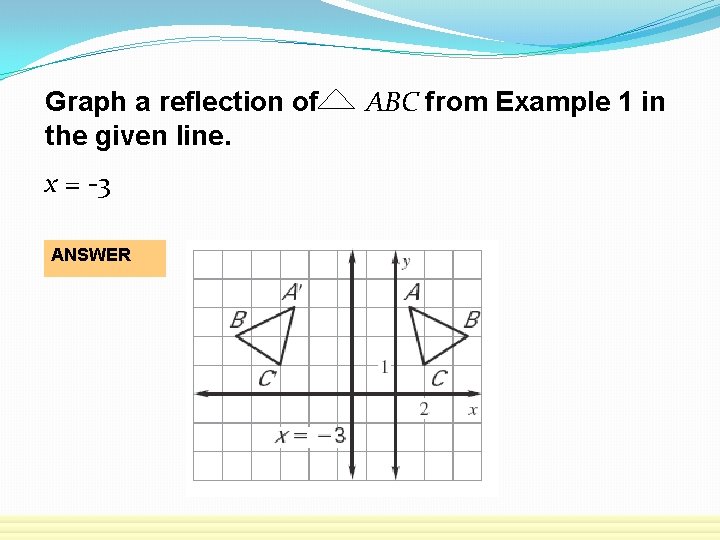
Graph a reflection of the given line. x = -3 ANSWER ABC from Example 1 in

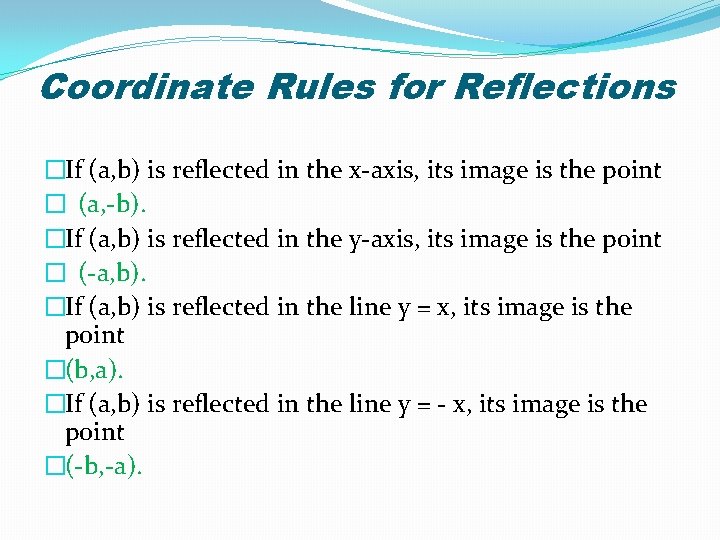
Coordinate Rules for Reflections �If (a, b) is reflected in the x-axis, its image is the point � (a, -b). �If (a, b) is reflected in the y-axis, its image is the point � (-a, b). �If (a, b) is reflected in the line y = x, its image is the point �(b, a). �If (a, b) is reflected in the line y = - x, its image is the point �(-b, -a).

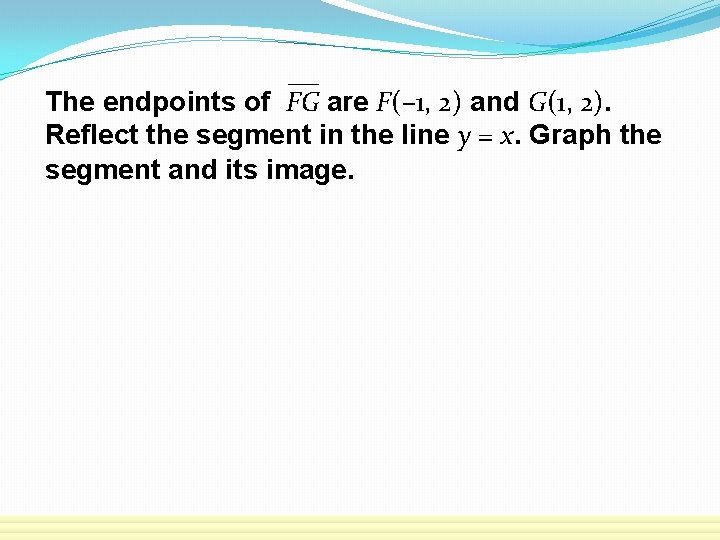
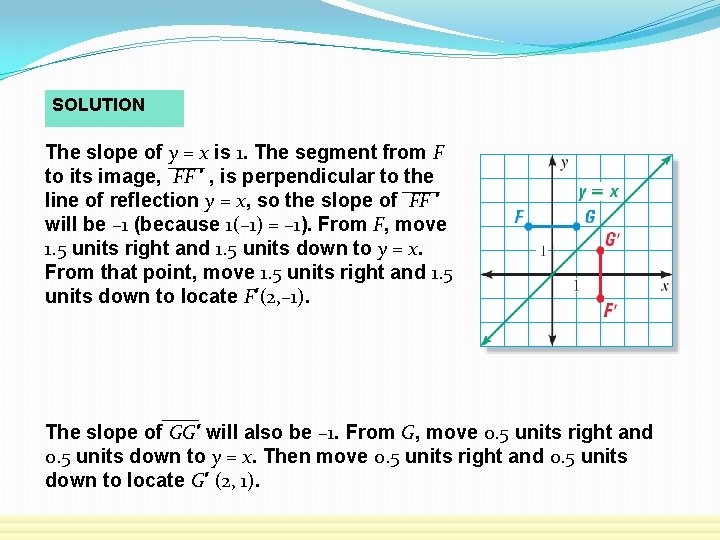
The endpoints of FG are F(– 1, 2) and G(1, 2). Reflect the segment in the line y = x. Graph the segment and its image.

SOLUTION The slope of y = x is 1. The segment from F to its image, FF ′ , is perpendicular to the line of reflection y = x, so the slope of FF ′ will be – 1 (because 1(– 1) = – 1). From F, move 1. 5 units right and 1. 5 units down to y = x. From that point, move 1. 5 units right and 1. 5 units down to locate F′(2, – 1). The slope of GG′ will also be – 1. From G, move 0. 5 units right and 0. 5 units down to y = x. Then move 0. 5 units right and 0. 5 units down to locate G′ (2, 1).

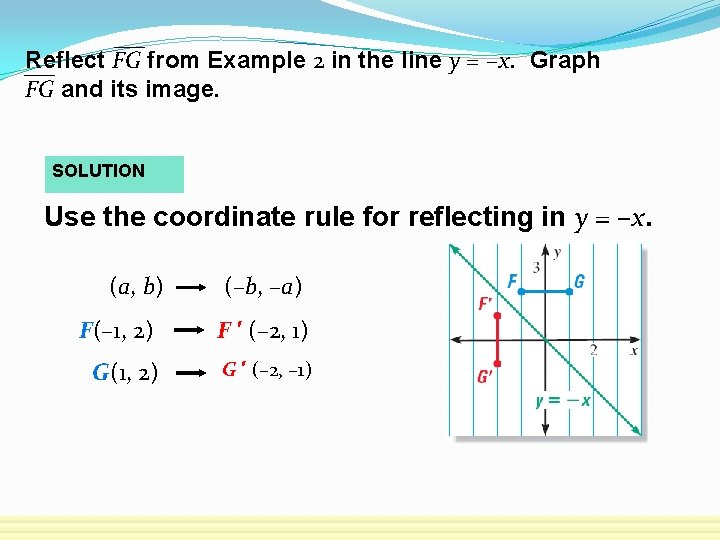
Reflect FG from Example 2 in the line y = –x. Graph FG and its image. SOLUTION Use the coordinate rule for reflecting in y = –x. (a, b) (–b, –a) F(– 1, 2) F ′ (– 2, 1) G(1, 2) G ′ (– 2, – 1)

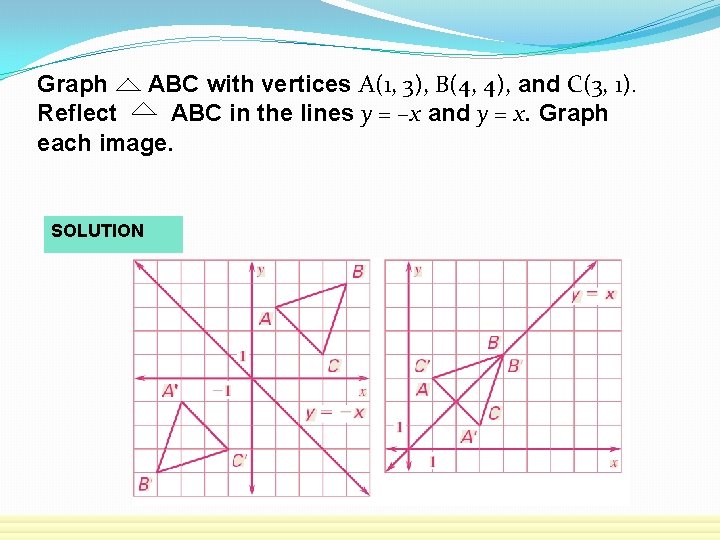
Graph ABC with vertices A(1, 3), B(4, 4), and C(3, 1). Reflect ABC in the lines y = –x and y = x. Graph each image. SOLUTION

� Homework: �p. 593 -595 {1 -12}

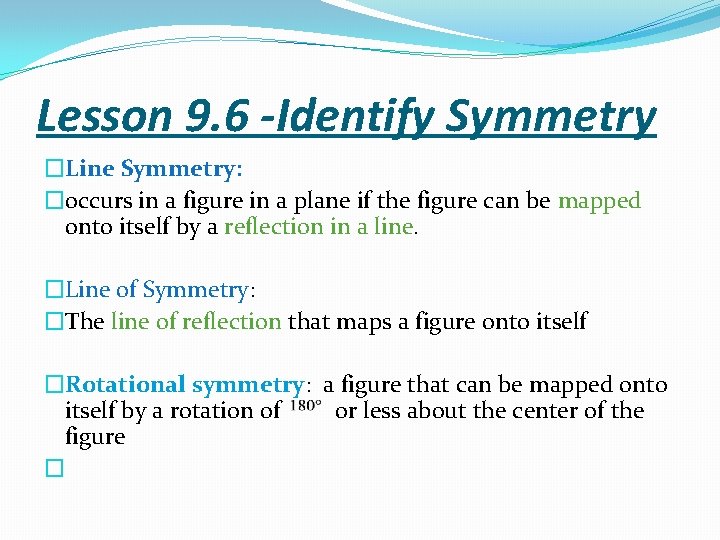
Lesson 9. 6 -Identify Symmetry �Line Symmetry: �occurs in a figure in a plane if the figure can be mapped onto itself by a reflection in a line. �Line of Symmetry: �The line of reflection that maps a figure onto itself �Rotational symmetry: a figure that can be mapped onto itself by a rotation of or less about the center of the figure �

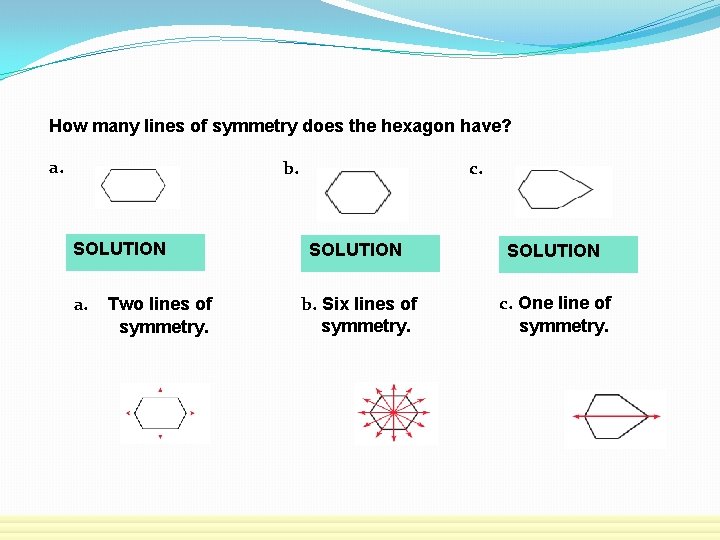
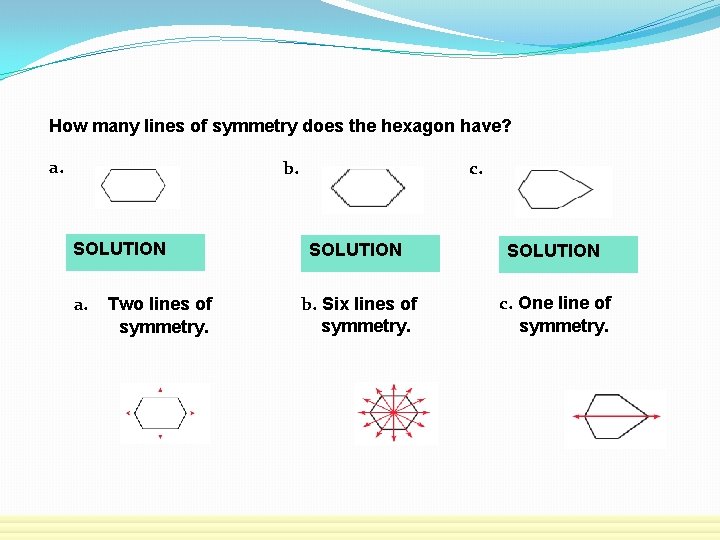
How many lines of symmetry does the hexagon have? a. c. b. SOLUTION a. Two lines of symmetry. SOLUTION b. Six lines of symmetry. c. One line of symmetry.

How many lines of symmetry does the object appear to have? 1. 2. ANSWER 8 3. ANSWER 5 ANSWER 1

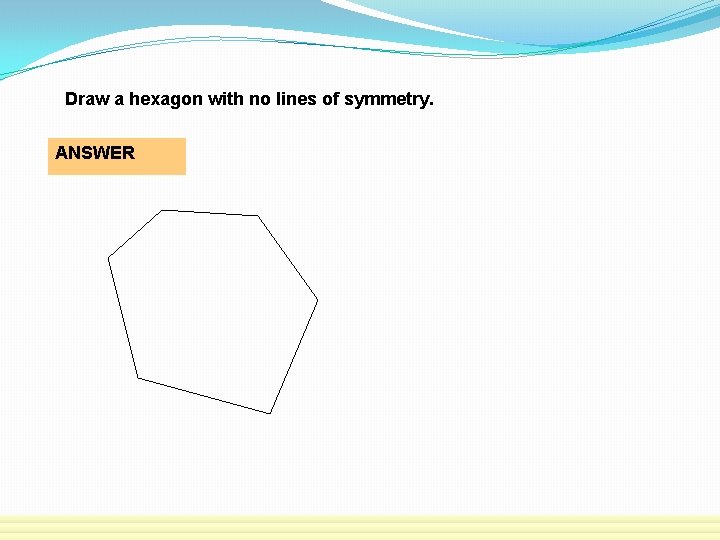
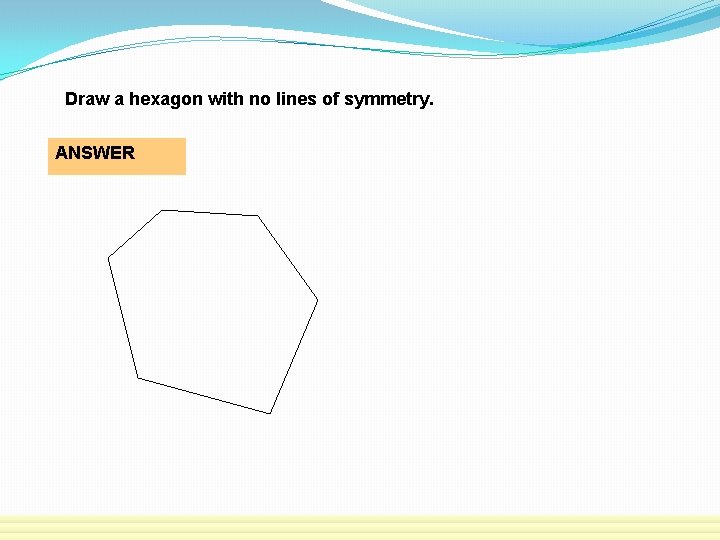
Draw a hexagon with no lines of symmetry. ANSWER

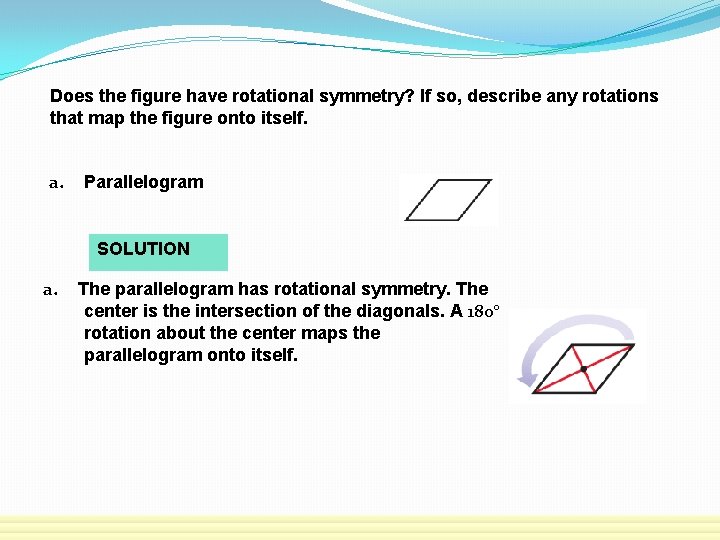
Does the figure have rotational symmetry? If so, describe any rotations that map the figure onto itself. a. Parallelogram SOLUTION a. The parallelogram has rotational symmetry. The center is the intersection of the diagonals. A 180° rotation about the center maps the parallelogram onto itself.

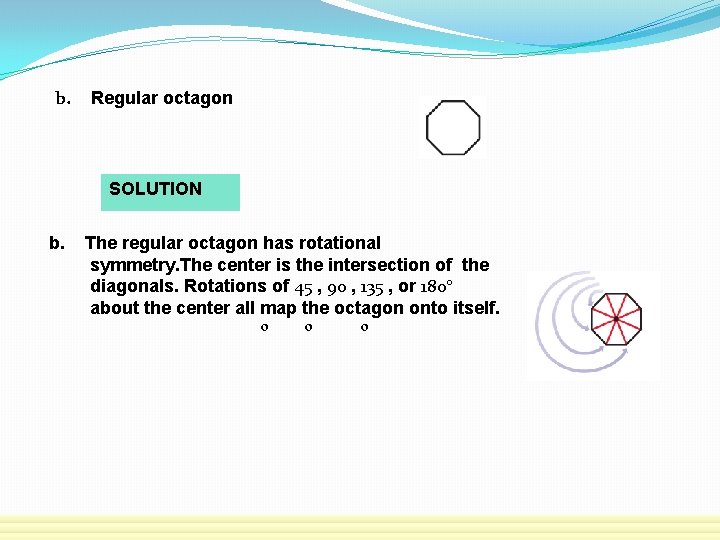
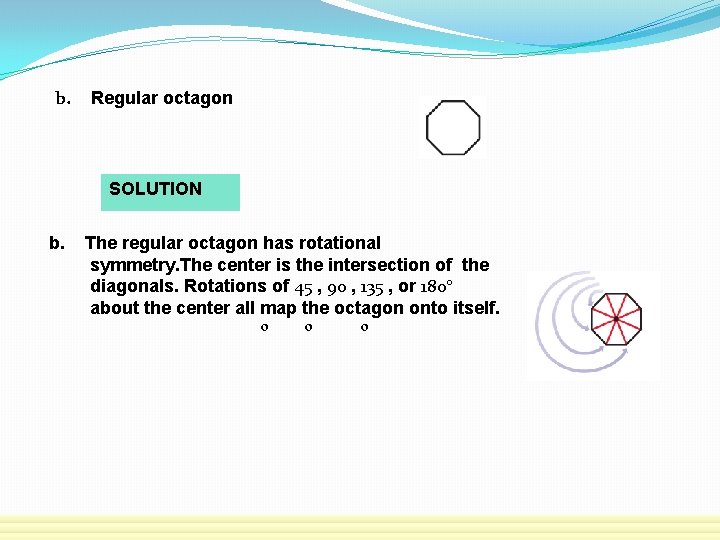
b. Regular octagon SOLUTION b. The regular octagon has rotational symmetry. The center is the intersection of the diagonals. Rotations of 45 , 90 , 135 , or 180° about the center all map the octagon onto itself. o o o

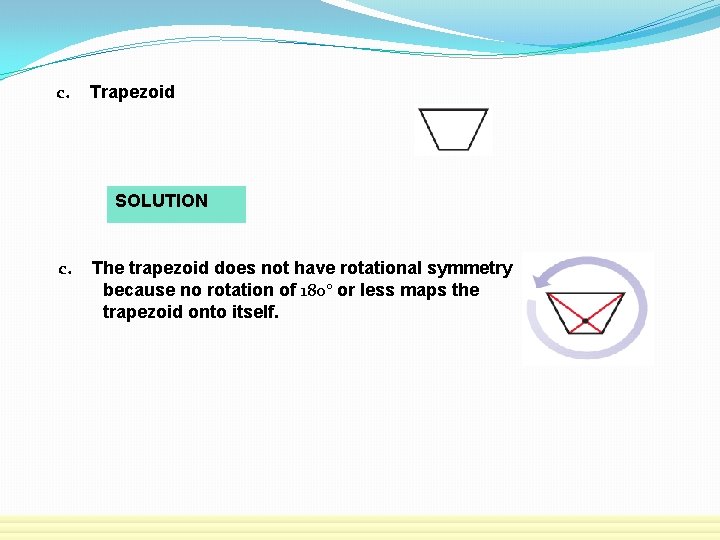
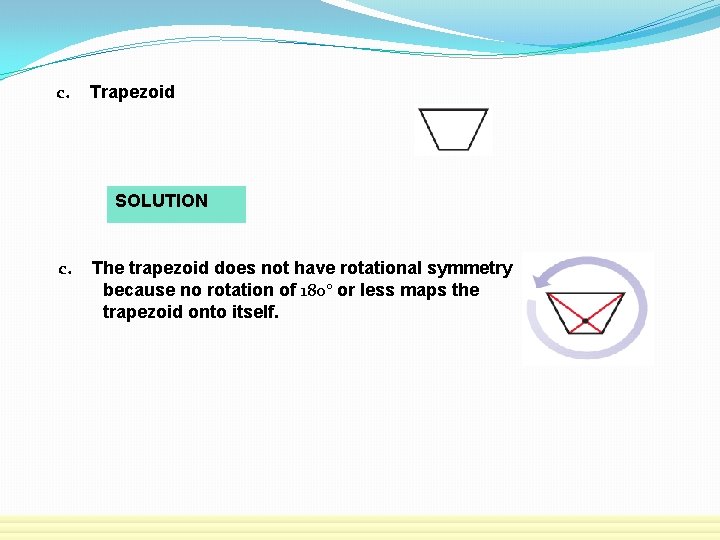
c. Trapezoid SOLUTION c. The trapezoid does not have rotational symmetry because no rotation of 180° or less maps the trapezoid onto itself.

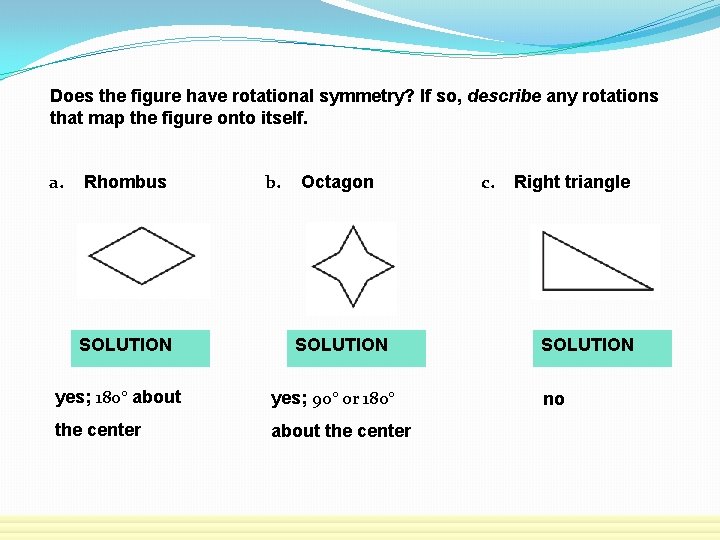
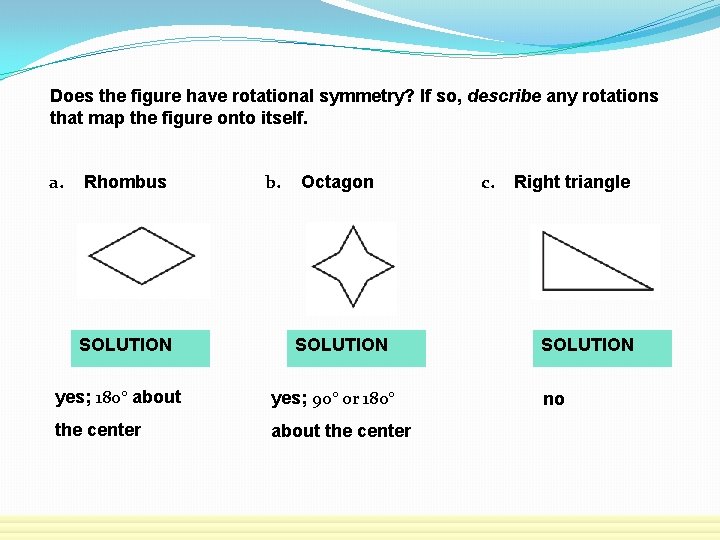
Does the figure have rotational symmetry? If so, describe any rotations that map the figure onto itself. a. Rhombus SOLUTION b. Octagon SOLUTION yes; 180° about yes; 90° or 180° the center about the center c. Right triangle SOLUTION no

Homework: p 621: {3 -5, 6, 13, 14, 17, 18}

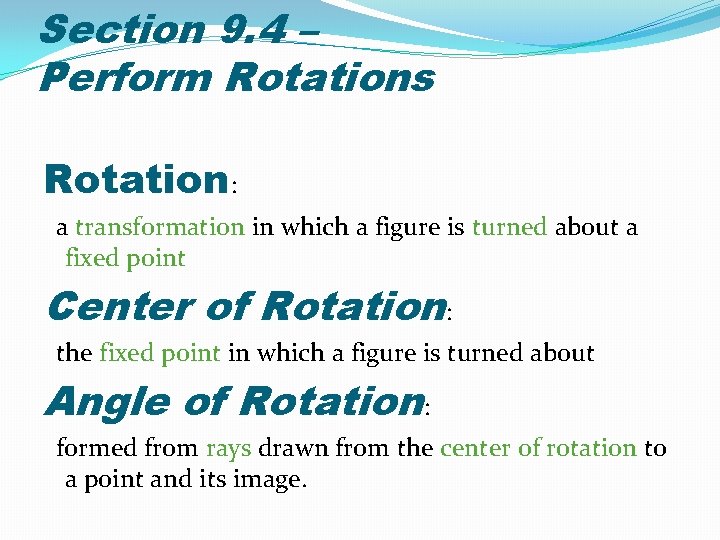
Section 9. 4 – Perform Rotations Rotation: a transformation in which a figure is turned about a fixed point Center of Rotation: the fixed point in which a figure is turned about Angle of Rotation: formed from rays drawn from the center of rotation to a point and its image.

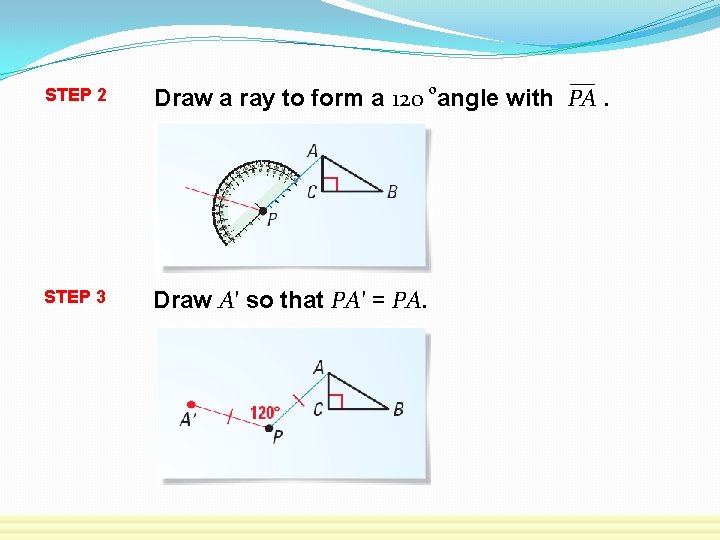
Draw a 120 o rotation of ABC about P. SOLUTION STEP 1 Draw a segment from A to P.

o STEP 2 Draw a ray to form a 120 angle with PA. STEP 3 Draw A′ so that PA′ = PA.

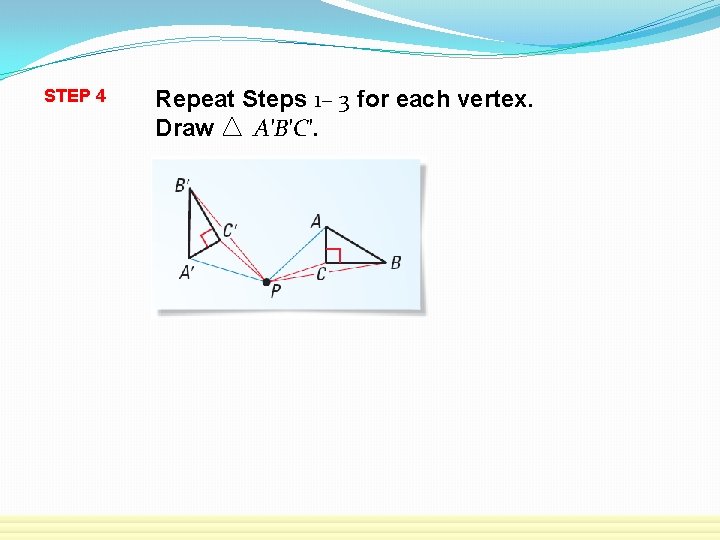
STEP 4 Repeat Steps 1– 3 for each vertex. Draw A′B′C′.

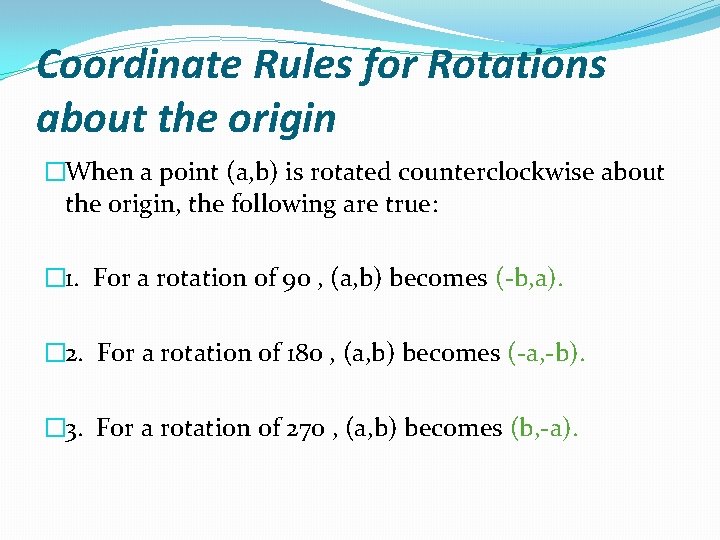
Coordinate Rules for Rotations about the origin �When a point (a, b) is rotated counterclockwise about the origin, the following are true: � 1. For a rotation of 90 , (a, b) becomes (-b, a). � 2. For a rotation of 180 , (a, b) becomes (-a, -b). � 3. For a rotation of 270 , (a, b) becomes (b, -a).

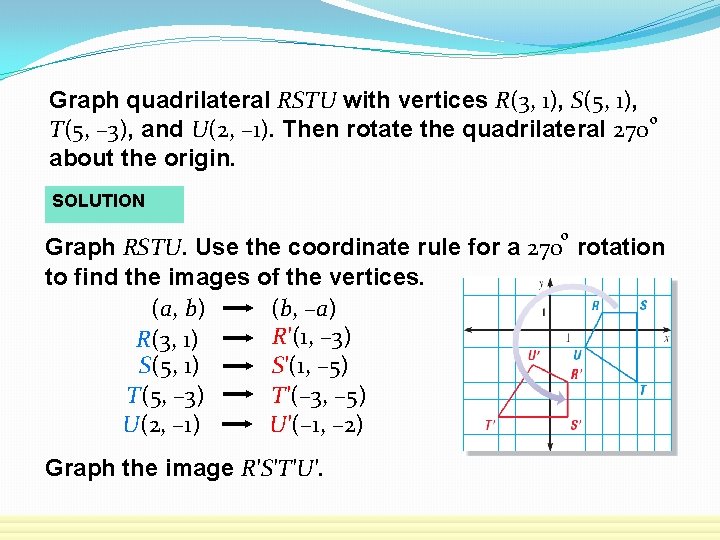
Graph quadrilateral RSTU with vertices R(3, 1), S(5, 1), o T(5, – 3), and U(2, – 1). Then rotate the quadrilateral 270 about the origin. SOLUTION o Graph RSTU. Use the coordinate rule for a 270 rotation to find the images of the vertices. (a, b) (b, –a) R′(1, – 3) R(3, 1) S(5, 1) S′(1, – 5) T(5, – 3) T′(– 3, – 5) U(2, – 1) U′(– 1, – 2) Graph the image R′S′T′U′.

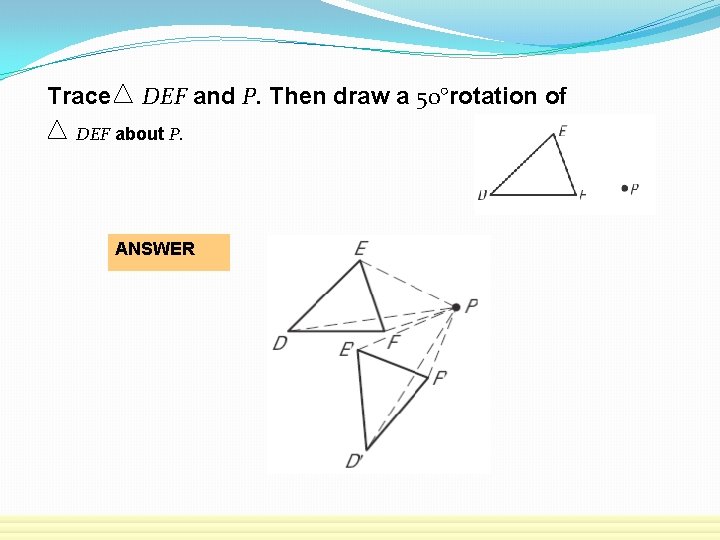
Trace DEF and P. Then draw a 50°rotation of DEF about P. ANSWER

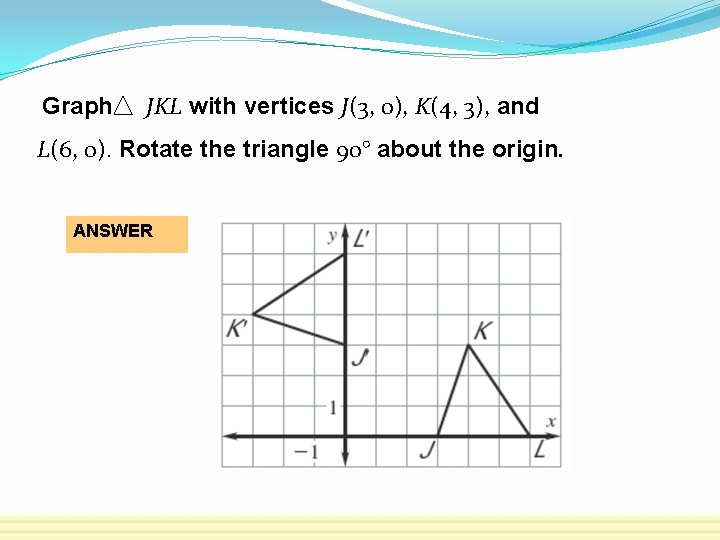
Graph JKL with vertices J(3, 0), K(4, 3), and L(6, 0). Rotate the triangle 90° about the origin. ANSWER

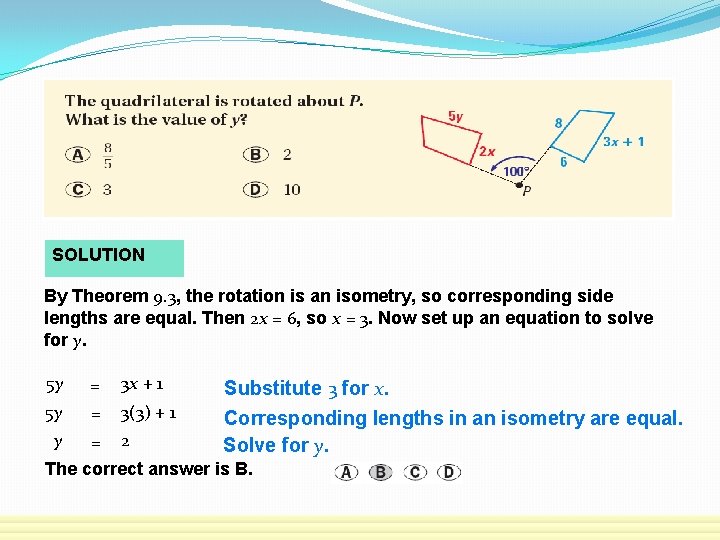
SOLUTION By Theorem 9. 3, the rotation is an isometry, so corresponding side lengths are equal. Then 2 x = 6, so x = 3. Now set up an equation to solve for y. 5 y = 3 x + 1 5 y = 3(3) + 1 y Substitute 3 for x. Corresponding lengths in an isometry are equal. Solve for y. = 2 The correct answer is B.

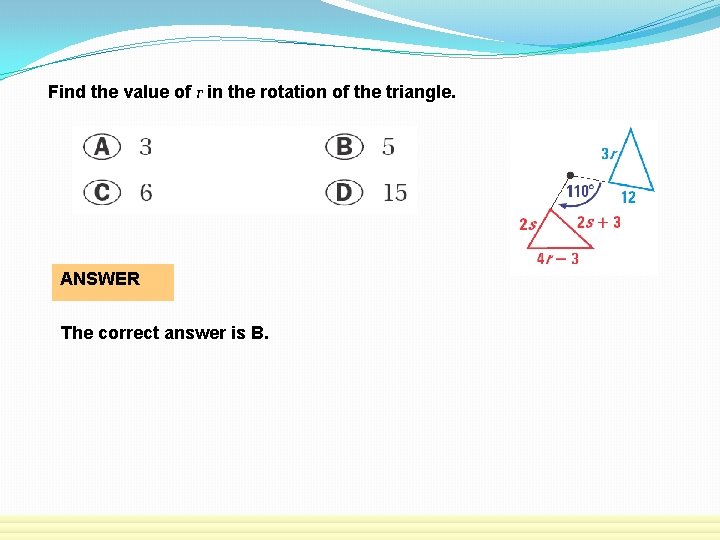
Find the value of r in the rotation of the triangle. ANSWER The correct answer is B.

HOMEWORK: p. 602 -603 {1, 3 -14, 20, 21, 23

TESSELLATIO NS HOW TO DO IT YOURSELF! SLICE METHOD

�Slice Method - Example 1 �First a very simple one to show the principle. 1. Start with a shape that is known to tessellate - in this case, a square. . .

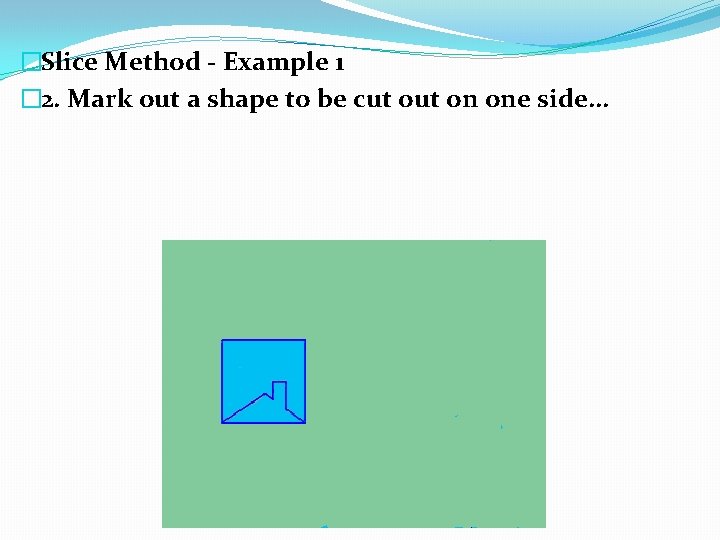
�Slice Method - Example 1 � 2. Mark out a shape to be cut on one side. . .

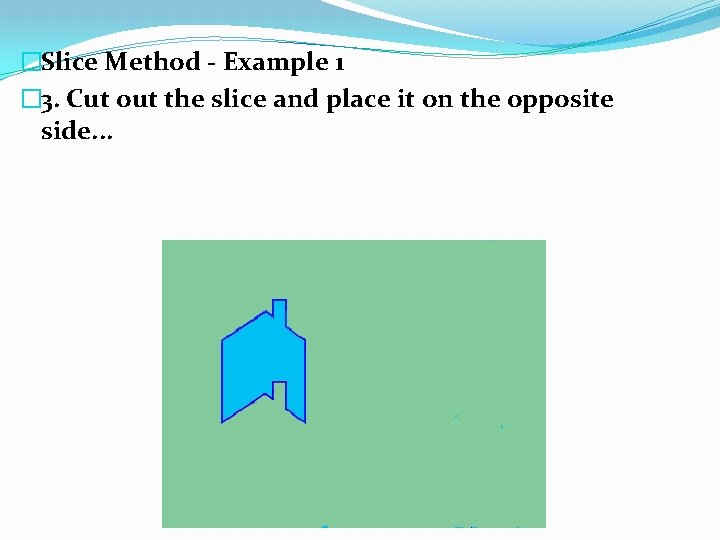
�Slice Method - Example 1 � 3. Cut out the slice and place it on the opposite side. . .

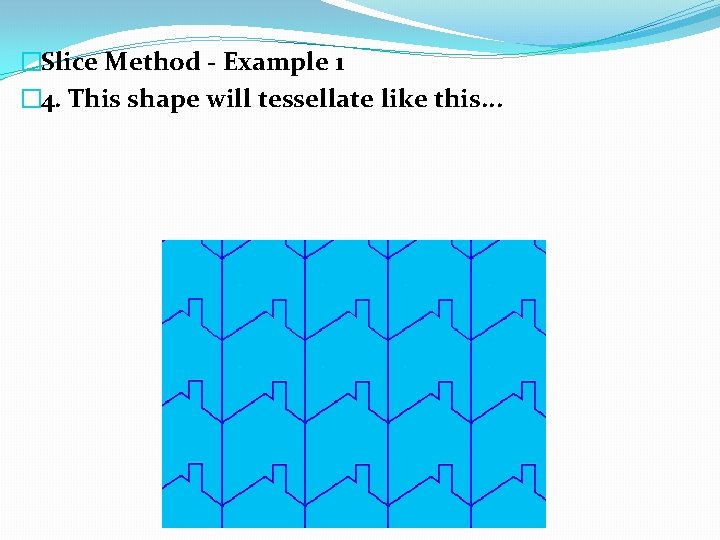
�Slice Method - Example 1 � 4. This shape will tessellate like this. . .

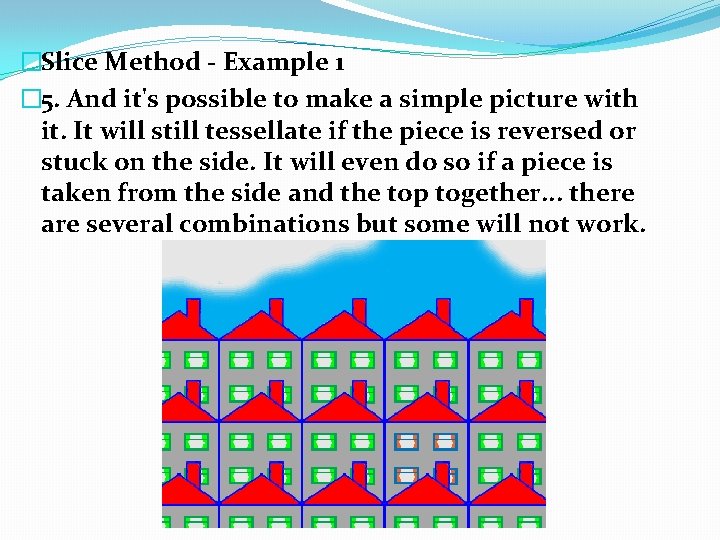
�Slice Method - Example 1 � 5. And it's possible to make a simple picture with it. It will still tessellate if the piece is reversed or stuck on the side. It will even do so if a piece is taken from the side and the top together. . . there are several combinations but some will not work.

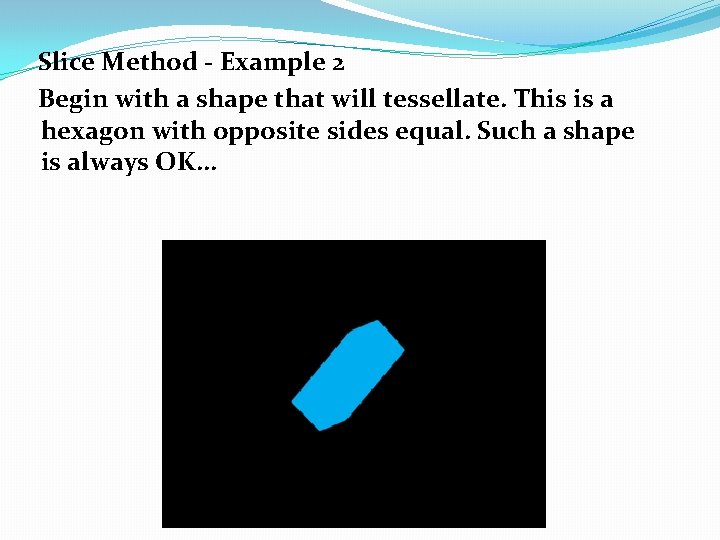
Slice Method - Example 2 Begin with a shape that will tessellate. This is a hexagon with opposite sides equal. Such a shape is always OK. . .

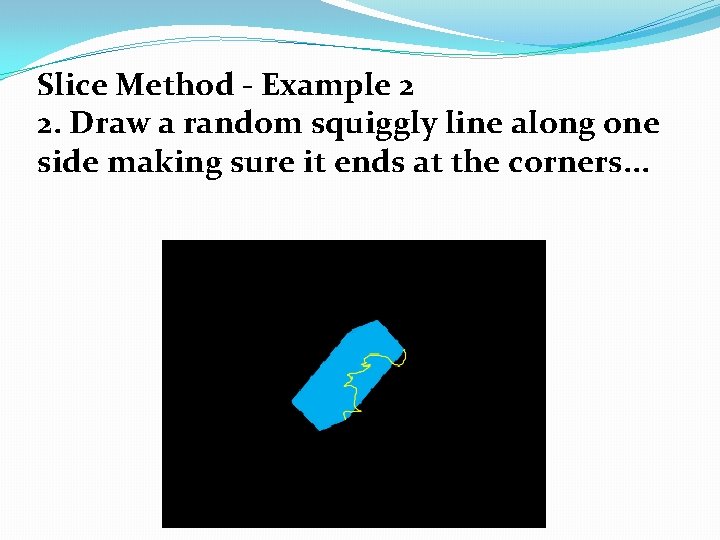
Slice Method - Example 2 2. Draw a random squiggly line along one side making sure it ends at the corners. . .

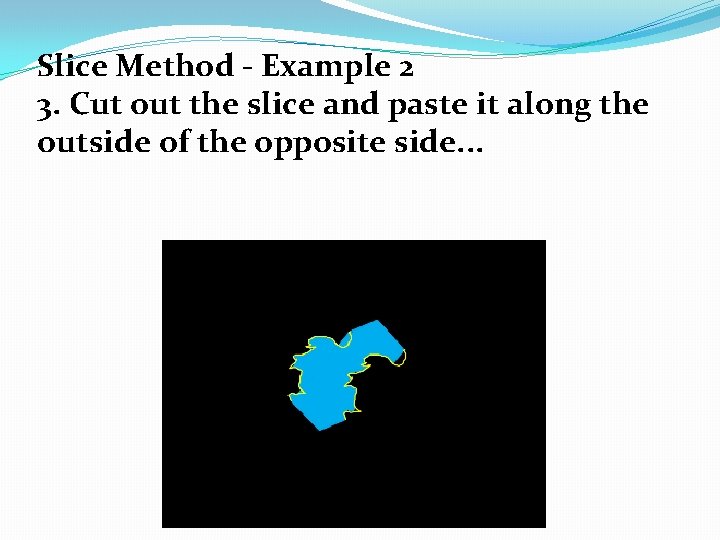
Slice Method - Example 2 3. Cut out the slice and paste it along the outside of the opposite side. . .

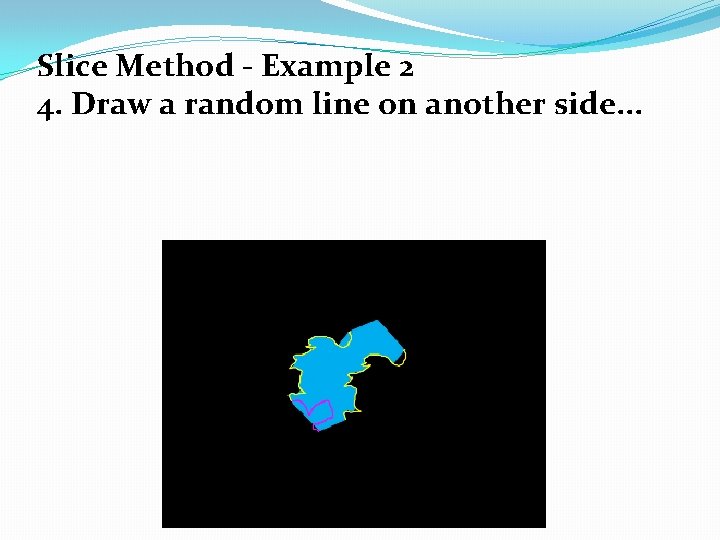
Slice Method - Example 2 4. Draw a random line on another side. . .

Slice Method - Example 2 5. Cut the slice out and paste it on the opposite side as before. . .

Slice Method - Example 2 6. Repeat for the remaining side. . .

Slice Method - Example 2 7. Look at the resulting shape - any ideas yet?

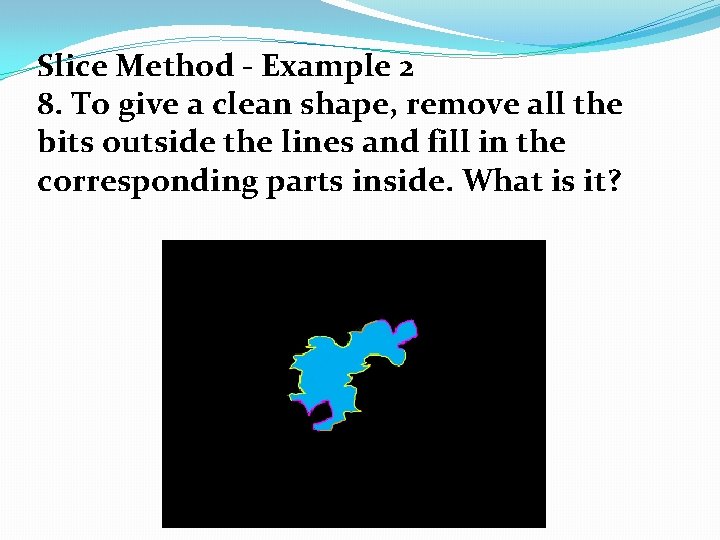
Slice Method - Example 2 8. To give a clean shape, remove all the bits outside the lines and fill in the corresponding parts inside. What is it?

Slice Method - Example 2 9. This is what I saw! As you see, the shape was arrived at quite by accident but I acknowledge the Disney copyright of my artistic filling in. Will it tessellate?

Slice Method - Example 2 10. Yes, of course! Can we improve it?

Slice Method - Example 2 11. Add a road, wall and pavement and we have a parade!

YOUR TURN! GOOD LUCK!!!

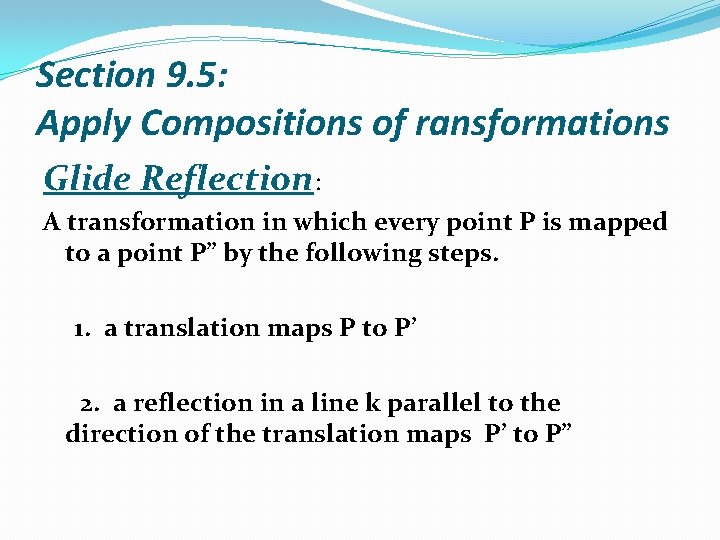
Section 9. 5: Apply Compositions of ransformations Glide Reflection: A transformation in which every point P is mapped to a point P” by the following steps. 1. a translation maps P to P’ 2. a reflection in a line k parallel to the direction of the translation maps P’ to P”

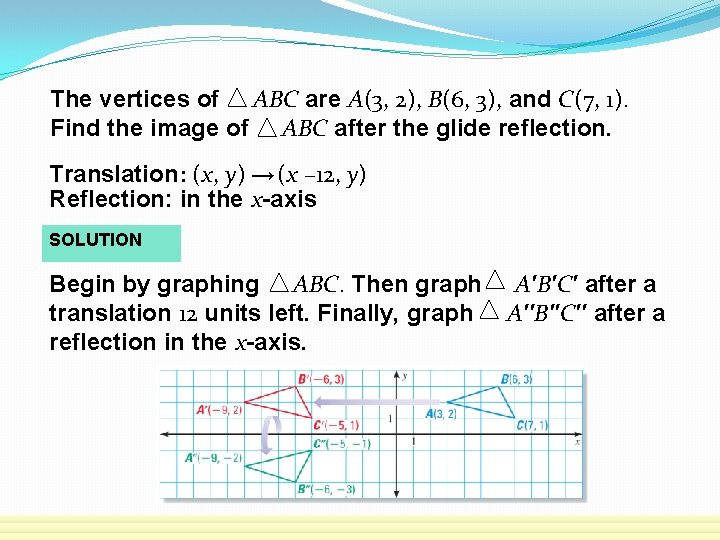
The vertices of ABC are A(3, 2), B(6, 3), and C(7, 1). Find the image of ABC after the glide reflection. Translation: (x, y) → (x – 12, y) Reflection: in the x-axis SOLUTION Begin by graphing ABC. Then graph translation 12 units left. Finally, graph reflection in the x-axis. A′B′C′ after a A′′B′′C′′ after a

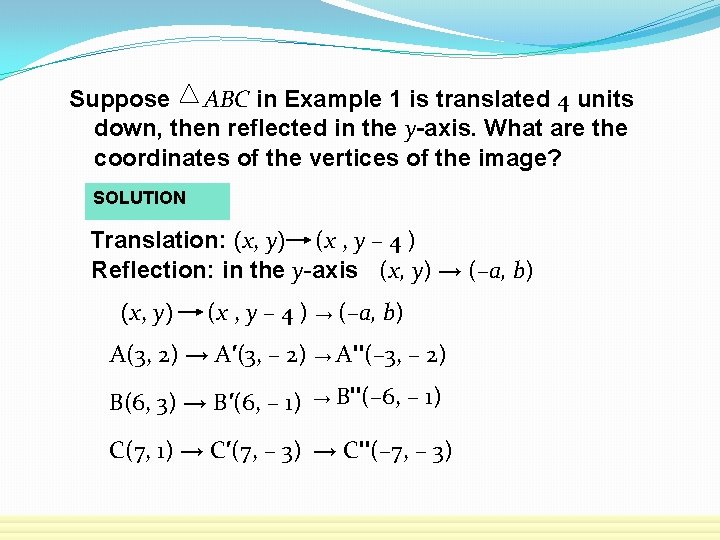
Suppose ABC in Example 1 is translated 4 units down, then reflected in the y-axis. What are the coordinates of the vertices of the image? SOLUTION Translation: (x, y) (x , y – 4 ) Reflection: in the y-axis (x, y) → (–a, b) (x, y) (x , y – 4 ) → (–a, b) A(3, 2) → A′(3, – 2) → A"(– 3, – 2) B(6, 3) → B′(6, – 1) → B"(– 6, – 1) C(7, 1) → C′(7, – 3) → C"(– 7, – 3)

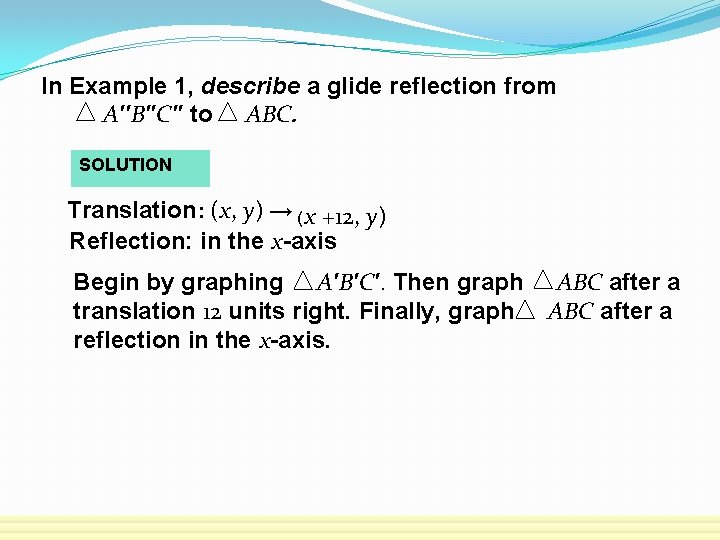
In Example 1, describe a glide reflection from A′′B′′C′′ to ABC. SOLUTION Translation: (x, y) → (x +12, y) Reflection: in the x-axis Begin by graphing A′B′C′. Then graph translation 12 units right. Finally, graph reflection in the x-axis. ABC after a

�Composition of Transformations: �the result of two or more transformations that are combined to form a single transformation

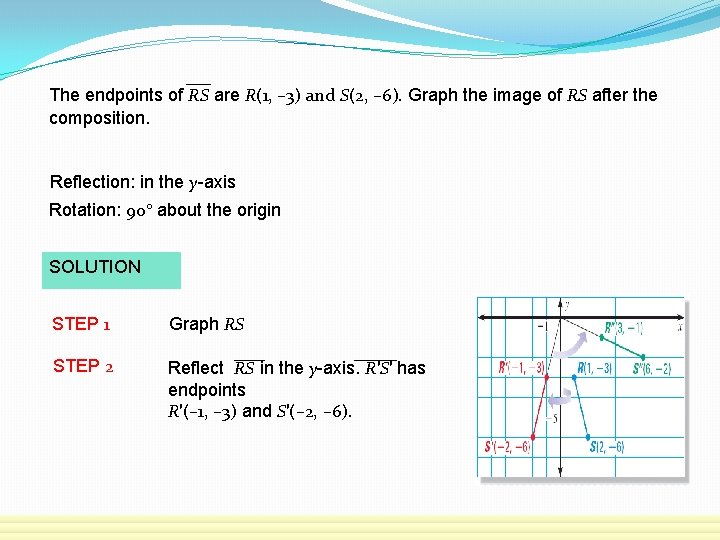
The endpoints of RS are R(1, – 3) and S(2, – 6). Graph the image of RS after the composition. Reflection: in the y-axis Rotation: 90° about the origin SOLUTION STEP 1 Graph RS STEP 2 Reflect RS in the y-axis. R′S′ has endpoints R′(– 1, – 3) and S′(– 2, – 6).

STEP 3 o Rotate R′S′ 90 about the origin. R′′S′′ has endpoints R′′(3, – 1) and S′′(6, – 2).

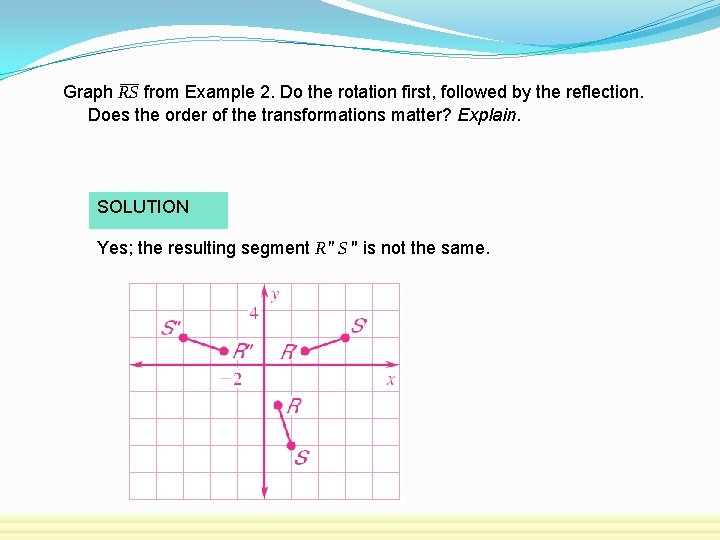
Graph RS from Example 2. Do the rotation first, followed by the reflection. Does the order of the transformations matter? Explain. SOLUTION Yes; the resulting segment R′′ S ′′ is not the same.

HOMEWORK �p. 602 -603 {1 , 3 -14, 20, 21, 23} �p. 611 -612 {1 -4, 7, 9, 11, 13, 14}

Lesson 9. 6 -Identify Symmetry �Line Symmetry occurs in a figure in a plane if the figure can be mapped onto itself by a reflection in a line. �Line of Symmetry: �The line of reflection that maps a figure onto itself �Rotational symmetry: a figure that can be mapped onto itself by a rotation of or less about the center of the figure �

How many lines of symmetry does the hexagon have? a. b. SOLUTION a. Two lines of symmetry. c. SOLUTION b. Six lines of symmetry. c. One line of symmetry.

How many lines of symmetry does the object appear to have? 1. 2. ANSWER 8 3. ANSWER 5 ANSWER 1

Draw a hexagon with no lines of symmetry. ANSWER

Does the figure have rotational symmetry? If so, describe any rotations that map the figure onto itself. a. Parallelogram SOLUTION a. The parallelogram has rotational symmetry. The center is the intersection of the diagonals. A 180° rotation about the center maps the parallelogram onto itself.

b. Regular octagon SOLUTION b. The regular octagon has rotational symmetry. The center is the intersection of the diagonals. Rotations of 45 , 90 , 135 , or 180° about the center all map the octagon onto itself. o o o

c. Trapezoid SOLUTION c. The trapezoid does not have rotational symmetry because no rotation of 180° or less maps the trapezoid onto itself.

Does the figure have rotational symmetry? If so, describe any rotations that map the figure onto itself. a. Rhombus SOLUTION b. Octagon SOLUTION yes; 180° about yes; 90° or 180° the center about the center c. Right triangle SOLUTION no

Homework: p 621: {3 -5, 6, 13, 14, 17, 18}

Lesson 9. 7 Identify and Perform Dilations �Dilation: a transformation that stretches or shrinks a figure to create a similar figure. �Center of Dilation: the fixed point �Scale Factor of a Dilation: the ratio of a side length of the image to the corresponding side length of the original figure.

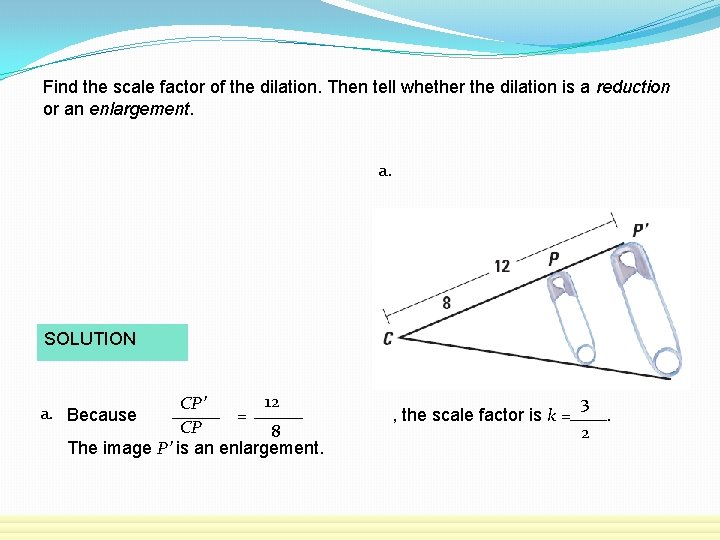
Find the scale factor of the dilation. Then tell whether the dilation is a reduction or an enlargement. a. SOLUTION 12 CP’ = CP 8 The image P’ is an enlargement. a. Because , the scale factor is k = 3 2 .

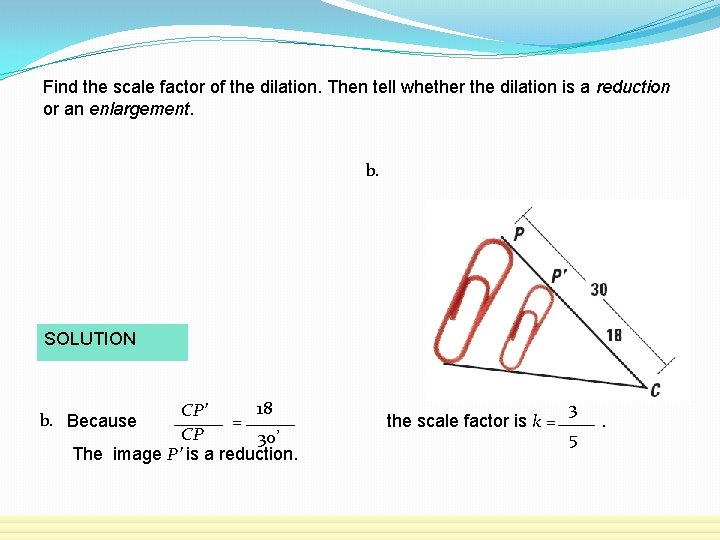
Find the scale factor of the dilation. Then tell whether the dilation is a reduction or an enlargement. b. SOLUTION 18 CP’ = CP 30’ The image P’ is a reduction. b. Because the scale factor is k = 3 5 .

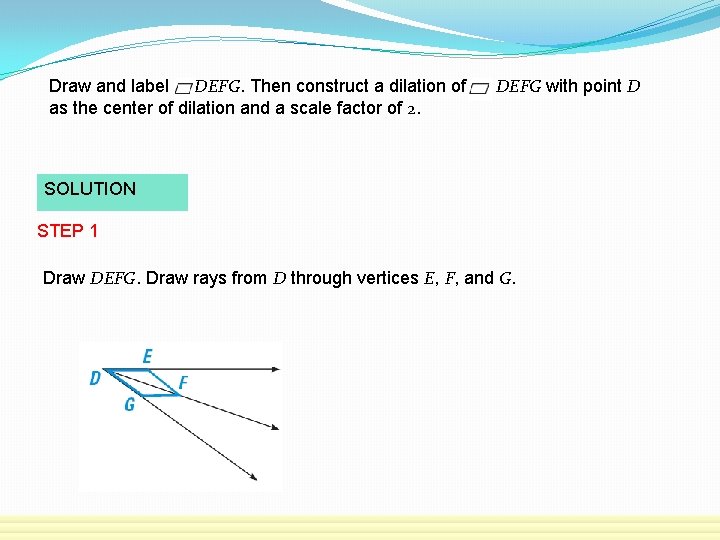
Draw and label DEFG. Then construct a dilation of as the center of dilation and a scale factor of 2. DEFG with point D SOLUTION STEP 1 Draw DEFG. Draw rays from D through vertices E, F, and G.

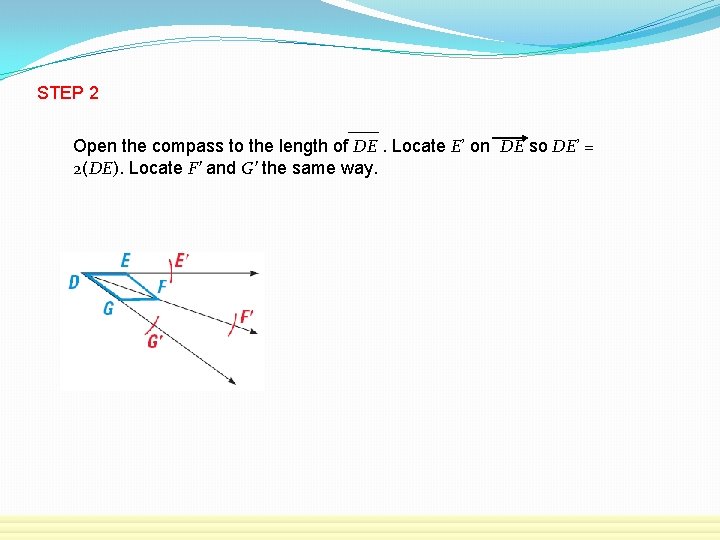
STEP 2 Open the compass to the length of DE. Locate E’ on DE so DE’ = 2(DE). Locate F’ and G’ the same way.

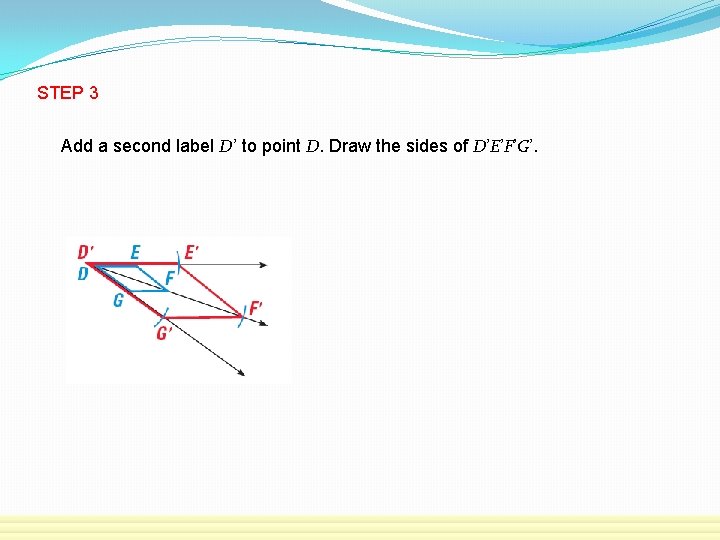
STEP 3 Add a second label D’ to point D. Draw the sides of D’E’F’G’.

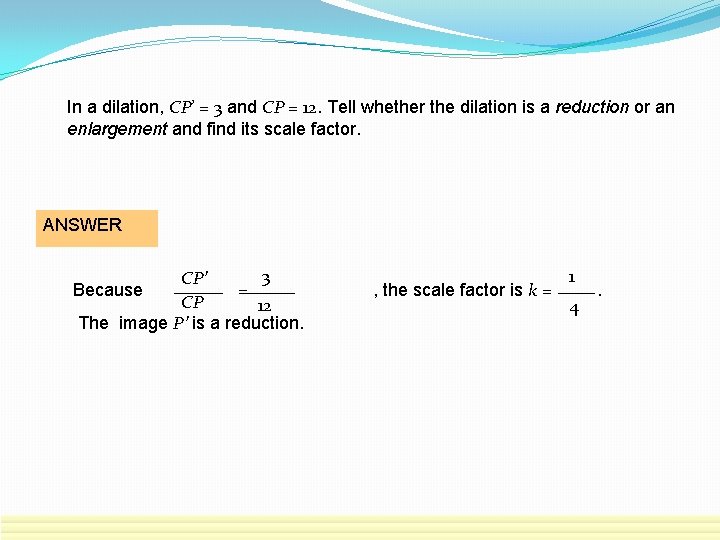
In a dilation, CP’ = 3 and CP = 12. Tell whether the dilation is a reduction or an enlargement and find its scale factor. ANSWER 3 CP’ Because = CP 12 The image P’ is a reduction. , the scale factor is k = 1 4 .

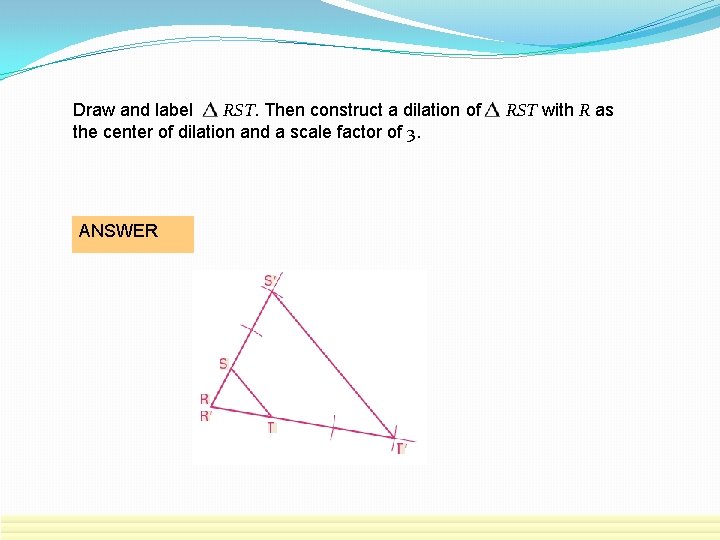
Draw and label RST. Then construct a dilation of the center of dilation and a scale factor of 3. ANSWER RST with R as

Homework: �p. 629 - 630 {3 -5, 7, 11, 13, 21, 22, 23}
 Imagep unscramble
Imagep unscramble Proving figures are similar using transformations
Proving figures are similar using transformations Lesson 2 session 3
Lesson 2 session 3 Congruent figures have the same
Congruent figures have the same A polygon with eight sides and eight angles
A polygon with eight sides and eight angles Is a trapezoid a plane figure
Is a trapezoid a plane figure Properties of transformations
Properties of transformations Plane figures and their properties
Plane figures and their properties Applying properties of similar figures
Applying properties of similar figures 4 sided polygons
4 sided polygons Properties of solid figures
Properties of solid figures Chapter 15 cultural transformations religion and science
Chapter 15 cultural transformations religion and science Chapter 5 political transformations empires and encounters
Chapter 5 political transformations empires and encounters Intensive property and extensive properties
Intensive property and extensive properties Chemical and physical properties
Chemical and physical properties Chapter 28 transformations around the globe
Chapter 28 transformations around the globe Rigid vs non rigid transformations
Rigid vs non rigid transformations Personality and its transformation
Personality and its transformation Function family graphs
Function family graphs Types of lexical correspondences
Types of lexical correspondences 2-7 parent functions and transformations answer key
2-7 parent functions and transformations answer key Absolute value functions and transformations
Absolute value functions and transformations Trig functions transformations
Trig functions transformations How to find amplitude of sine function
How to find amplitude of sine function 7-2 similarity transformations
7-2 similarity transformations Parent functions and transformations
Parent functions and transformations Geometry topic 1 review
Geometry topic 1 review Multiple transformations occur when
Multiple transformations occur when Energy transformation and conservation
Energy transformation and conservation 100 examples of energy transformation
100 examples of energy transformation Economic transformations commerce and consequence
Economic transformations commerce and consequence 4-1 congruence
4-1 congruence The study of energy and its transformations.
The study of energy and its transformations. Introduction to personality and its transformations
Introduction to personality and its transformations Political transformations empires and encounters
Political transformations empires and encounters Doterra taiwan
Doterra taiwan Intensity transformations and spatial filtering
Intensity transformations and spatial filtering Intensity transformations and spatial filtering
Intensity transformations and spatial filtering Intensity transformation functions in image processing
Intensity transformation functions in image processing Graphing tangent and cotangent
Graphing tangent and cotangent Congruent segments
Congruent segments Cultural transformations religion and science
Cultural transformations religion and science Intensity transformations and spatial filtering
Intensity transformations and spatial filtering 4-1 quadratic functions and transformations
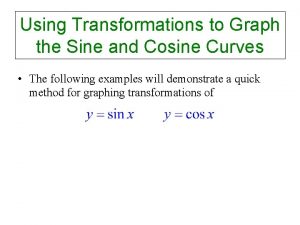
4-1 quadratic functions and transformations Transformations of sine and cosine functions
Transformations of sine and cosine functions Hadamard basis
Hadamard basis Square root function transformations
Square root function transformations Study jams transformations
Study jams transformations Study of energy and its transformations
Study of energy and its transformations Appolinian
Appolinian What are the attributes of a triangle?
What are the attributes of a triangle? Chapter 11 properties of the hair and scalp answers
Chapter 11 properties of the hair and scalp answers Elements and their properties chapter 17
Elements and their properties chapter 17 Limits and their properties
Limits and their properties Ringed hair milady
Ringed hair milady Chapter 1 limits and their properties
Chapter 1 limits and their properties Special trig limits
Special trig limits Elements and their properties section 1 metals
Elements and their properties section 1 metals Summary of romeo and juliet prologue
Summary of romeo and juliet prologue Translating equations
Translating equations Translate words into equations
Translate words into equations Food and beverage translate
Food and beverage translate Bleu
Bleu Translate
Translate Sin graph points
Sin graph points Using transformations to graph quadratic functions
Using transformations to graph quadratic functions Quadratic transformations quiz
Quadratic transformations quiz Rational function parent
Rational function parent Logarithmic functions
Logarithmic functions Rotation rules
Rotation rules Trig transformations
Trig transformations Vertically stretched or compressed
Vertically stretched or compressed 270 rotation
270 rotation Translation slide
Translation slide Translation rule geometry
Translation rule geometry Energy transformation in digestion
Energy transformation in digestion Mathisfun tetris
Mathisfun tetris Abcye
Abcye Graph transformations
Graph transformations Reported speech transformations
Reported speech transformations Standard transformation form
Standard transformation form Lorentz transformation equation derivation
Lorentz transformation equation derivation Types of lexical transformations
Types of lexical transformations Four types of transformations
Four types of transformations Dilation of quadratic function
Dilation of quadratic function Ac source transformation
Ac source transformation Multiple transformations geometry
Multiple transformations geometry Intensity transformation
Intensity transformation Identify energy transformations
Identify energy transformations Graph transformations worksheet
Graph transformations worksheet L
L