Chapter 1 Limits and Their Properties 1 2
































- Slides: 81

Chapter 1 Limits and Their Properties 1 -2 Finding Limits Graphically and Numerically • 1 -3 Evaluating Limits Analytically • 1 -4 Continuity and One-Sided Limits • 1 -5 infinite Limits • This will test the “Limits” of your brain!

1. 3 Evaluating Limits Analytically • Properties of Limits • Techniques for Evaluating Limits – Direct Substitution – Factoring and Cancelling – Rationalizing – Algebraic Manipulation • Trigonometric Rules • The Sandwich Theorem

Properties of Limits

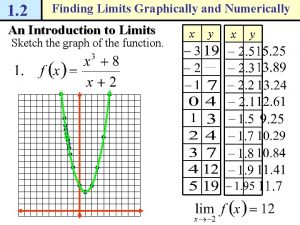
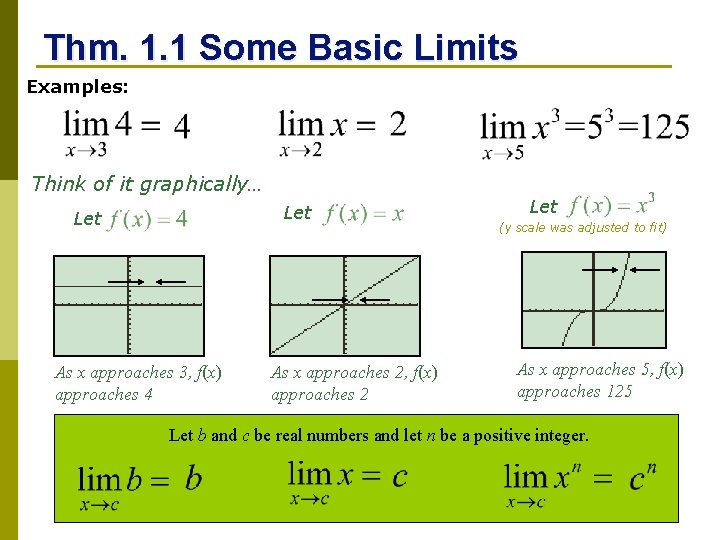
Thm. 1. 1 Some Basic Limits Examples: Think of it graphically… Let As x approaches 3, f(x) approaches 4 As x approaches 2, f(x) approaches 2 Let (y scale was adjusted to fit) As x approaches 5, f(x) approaches 125 Let b and c be real numbers and let n be a positive integer.

Thm. 1. 2 Properties of Limits: Let b and c be real numbers, let n be a positive integer, and let f and g be functions whose limits exist: üScalar Multiplication – limx c b f(x) = b limx cf(x) Ex: limx 2 3 x = 3 limx 2 x = 3 2 = 6 üSum/Difference - limx c f(x) + g(x) = limx c f(x) + limx c g(x) Ex: limx 4 x 2 + x = limx 4 x 2 + limx 4 x = 16 + 4 = 20 üProduct - limx c f(x) g(x) = limx c f(x) limx c g(x) Ex: limx πx 2 cos x =(limx π x 2)(limx π cos x)= π2 -1= -π2

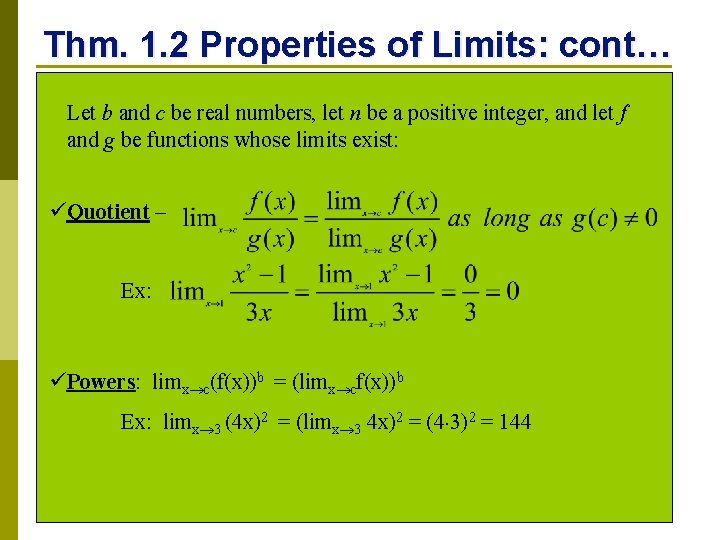
Thm. 1. 2 Properties of Limits: cont… Let b and c be real numbers, let n be a positive integer, and let f and g be functions whose limits exist: üQuotient – Ex: üPowers: limx c(f(x))b = (limx cf(x))b Ex: limx 3 (4 x)2 = (limx 3 4 x)2 = (4 3)2 = 144

You Try… Given: limx c f(x) = 3 and limx c g(x) = -5 evaluate the following. 1. limx c 4 f(x) = 3. limx c [g(x)]3 = 2. limx c f(x)+g(x) = 12 4. limx c f(x) g(x) = -125 5. limx c f(x)/g(x) = -2 -15 -3/5 Note: You can only drop limx c after you substitute in the value of c.

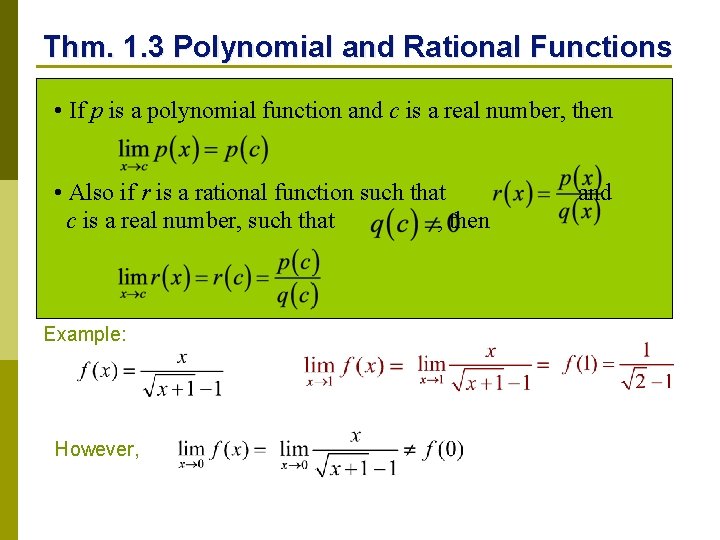
Thm. 1. 3 Polynomial and Rational Functions • If p is a polynomial function and c is a real number, then • Also if r is a rational function such that c is a real number, such that , then Example: However, and

You Try… Evaluate each limit. You may use a calculator to verify your answer. = 30 = 7/6 If the denominator had been 0, we would NOT have been able to use direct substitution. =0 =5

Theorem 1. 4 Limits Involving a Radical Ø When evaluating limits involving radicals, remember we CANNOT take a square root, fourth root, or any other even root of negative numbers. Ø However, we CAN take a cube root, fifth root, or any other odd root of negative numbers. Ex: Evaluate each limit. DNE

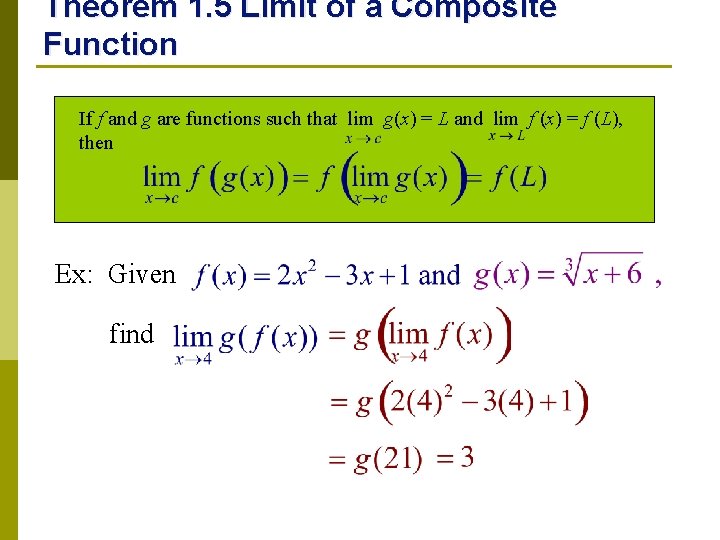
Theorem 1. 5 Limit of a Composite Function If f and g are functions such that lim g(x) = L and lim f (x) = f (L), then Ex: Given find

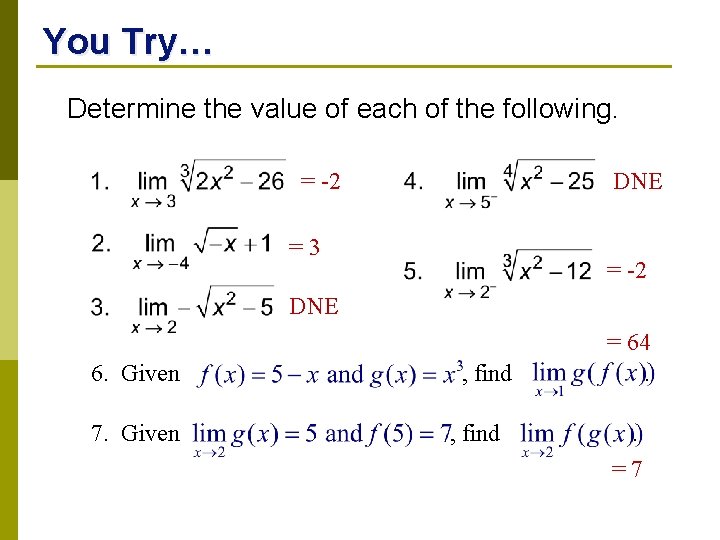
You Try… Determine the value of each of the following. = -2 DNE =3 = -2 DNE 6. Given 7. Given , find = 64. . =7

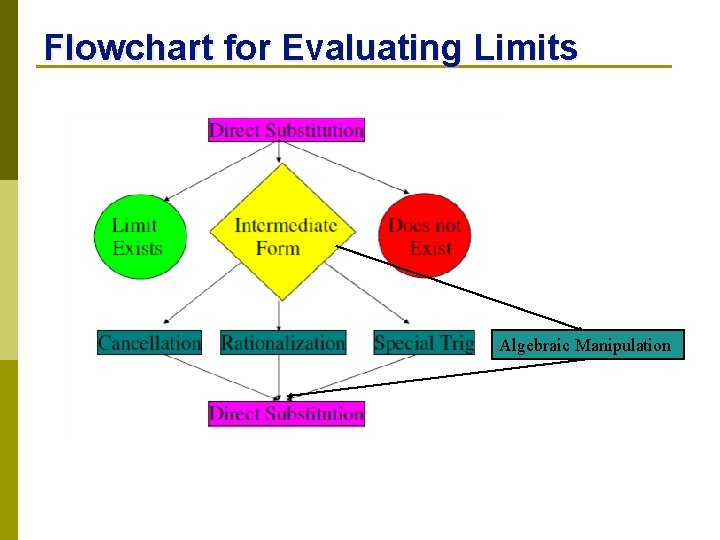
Flowchart for Evaluating Limits Algebraic Manipulation

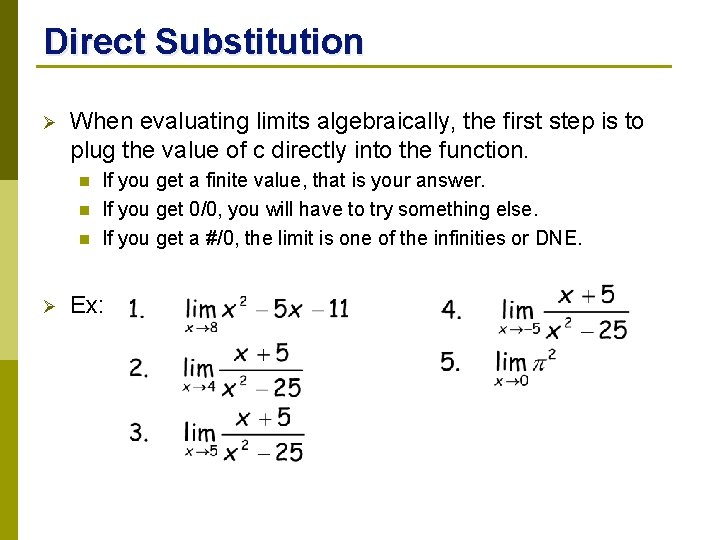
Direct Substitution Ø When evaluating limits algebraically, the first step is to plug the value of c directly into the function. n n n Ø If you get a finite value, that is your answer. If you get 0/0, you will have to try something else. If you get a #/0, the limit is one of the infinities or DNE. Ex:

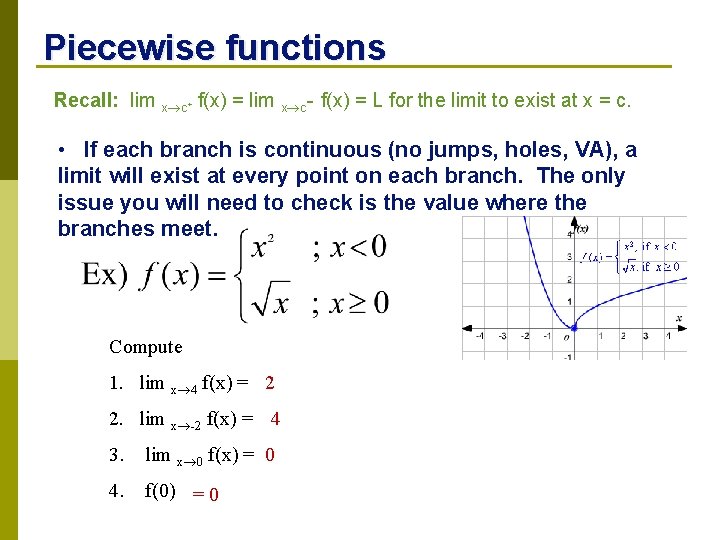
Piecewise functions Recall: lim x c f(x) = lim x c- f(x) = L for the limit to exist at x = c. + • If each branch is continuous (no jumps, holes, VA), a limit will exist at every point on each branch. The only issue you will need to check is the value where the branches meet. Compute 1. lim x 4 f(x) = 2 2. lim x -2 f(x) = 4 3. lim x 0 f(x) = 0 4. f(0) = 0

One more piecewise: Compute 1. lim x -6 g(x) = 0 2. lim x -3 g(x) = 3 3. lim x 3 g(x) DNE 4. lim x 5 g(x) = 5 5. f(3) = 5

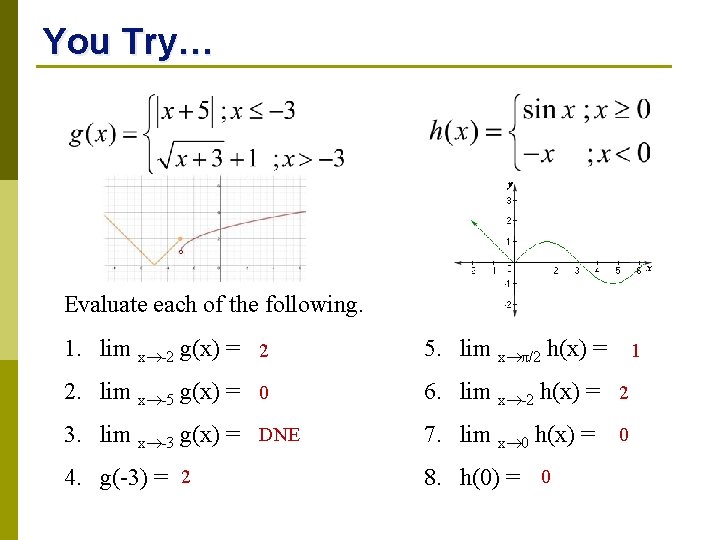
You Try… Evaluate each of the following. 1. lim x -2 g(x) = 2 5. lim x π/2 h(x) = 1 2. lim x -5 g(x) = 0 6. lim x -2 h(x) = 2 3. lim x -3 g(x) = DNE 7. lim x 0 h(x) = 4. g(-3) = 2 8. h(0) = 0 0

You Try… Determine the value of c so that the limit exists.

Closure Explain what to do first when evaluating limits and explain the three cases that could occur.

1. 3 Evaluating Limits Analytically • Properties of Limits • Techniques for Evaluating Limits – Direct Substitution – Factoring and Cancelling – Rationalizing – Algebraic Manipulation • Trigonometric Rules • The Sandwich Theorem

Review First… A rational function is a function that can be expressed in the form where both f(x) and g(x) are polynomial functions and q(x) 0. Examples of rational functions would be:

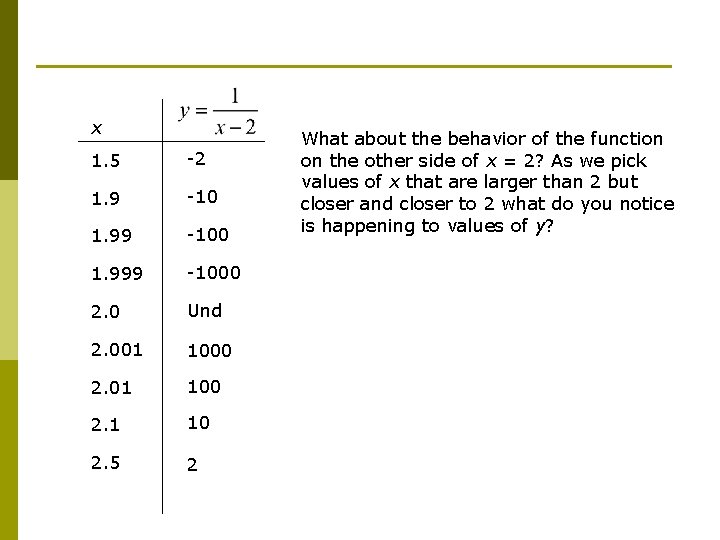
First let’s look at This function has one value of x that is banned from the domain. Why? x 1. 5 -2 1. 9 -10 1. 99 -100 1. 999 -1000 2. 0 undefined Fill in the values in the table to see what is happening to the y-values as the x-values get closer and closer to 2 from the left side.

x 1. 5 -2 1. 9 -10 1. 99 -100 1. 999 -1000 2. 0 Und 2. 001 1000 2. 01 100 2. 1 10 2. 5 2 What about the behavior of the function on the other side of x = 2? As we pick values of x that are larger than 2 but closer and closer to 2 what do you notice is happening to values of y?

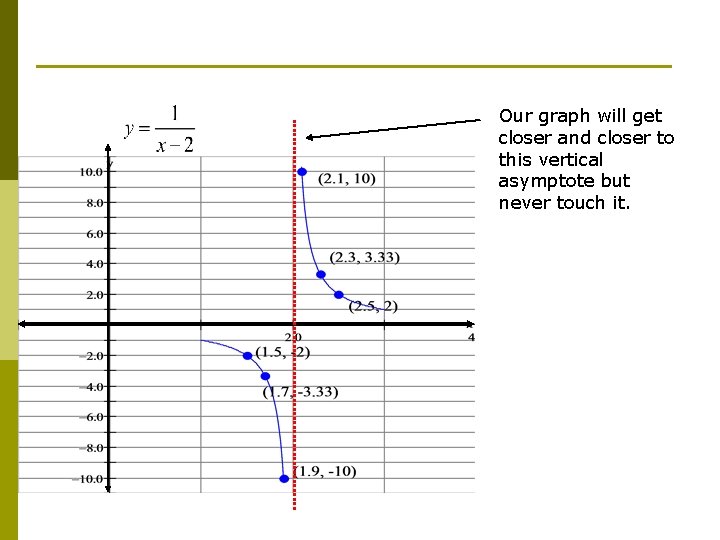
Our graph will get closer and closer to this vertical asymptote but never touch it.

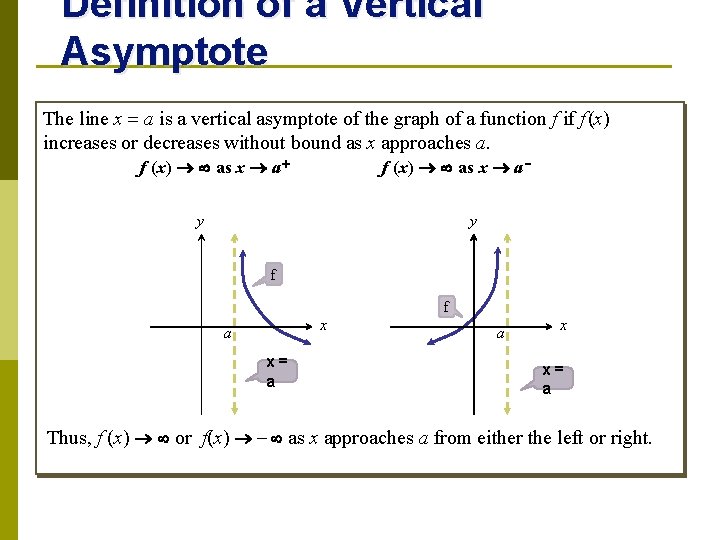
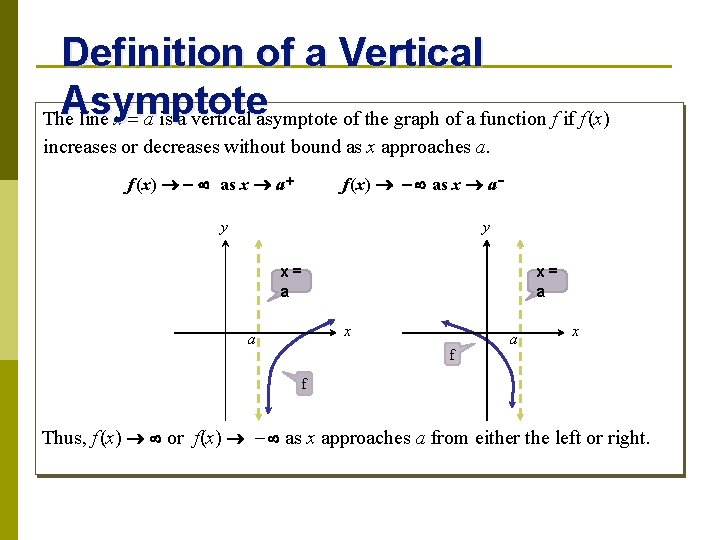
Definition of a Vertical Asymptote The line x = a is a vertical asymptote of the graph of a function f if f (x) increases or decreases without bound as x approaches a. f (x) as x a + f (x) as x a y y f x a x= a f a x x= a Thus, f (x) or f(x) - as x approaches a from either the left or right.

Definition of a Vertical Asymptote The line x = a is a vertical asymptote of the graph of a function f if f (x) increases or decreases without bound as x approaches a. f (x) as x a + f (x) as x a y y x= a x a f a x f Thus, f (x) or f(x) - as x approaches a from either the left or right.

Finding VA’s VERTICAL ASYMPTOTES There will be a vertical asymptote at any “restricted” x value, so anywhere that would make the denominator = 0 (numerator 0). Plug both in numerator… So there are VA’s at x = 4 and x = -1. Let’s set the bottom = 0 and factor and solve to find where the vertical asymptote(s) should be.

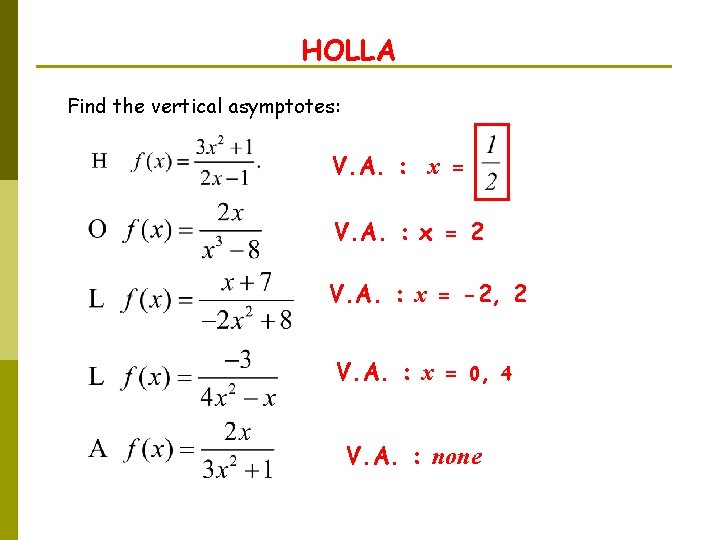
HOLLA Find the vertical asymptotes: V. A. : x = 2 V. A. : x = -2, 2 V. A. : x = 0, V. A. : none 4

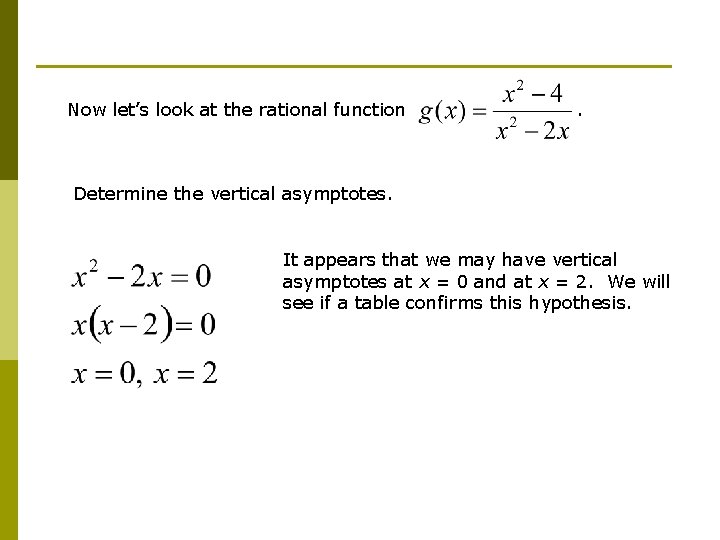
Now let’s look at the rational function . Determine the vertical asymptotes. It appears that we may have vertical asymptotes at x = 0 and at x = 2. We will see if a table confirms this hypothesis.

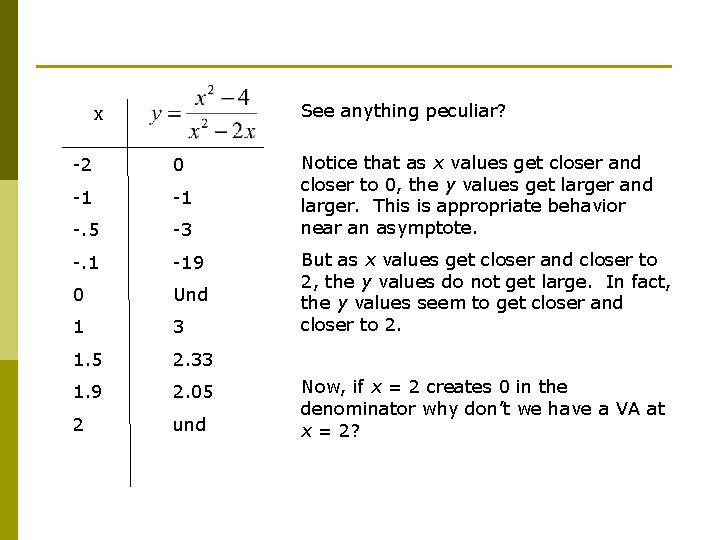
See anything peculiar? x -2 0 -1 -1 -. 5 -3 -. 1 -19 0 Und 1 3 1. 5 2. 33 1. 9 2. 05 2 und Notice that as x values get closer and closer to 0, the y values get larger and larger. This is appropriate behavior near an asymptote. But as x values get closer and closer to 2, the y values do not get large. In fact, the y values seem to get closer and closer to 2. Now, if x = 2 creates 0 in the denominator why don’t we have a VA at x = 2?

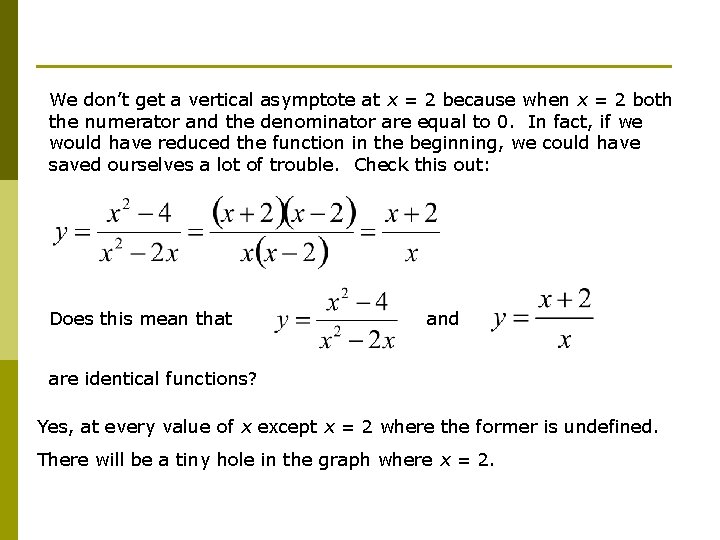
We don’t get a vertical asymptote at x = 2 because when x = 2 both the numerator and the denominator are equal to 0. In fact, if we would have reduced the function in the beginning, we could have saved ourselves a lot of trouble. Check this out: Does this mean that and are identical functions? Yes, at every value of x except x = 2 where the former is undefined. There will be a tiny hole in the graph where x = 2.

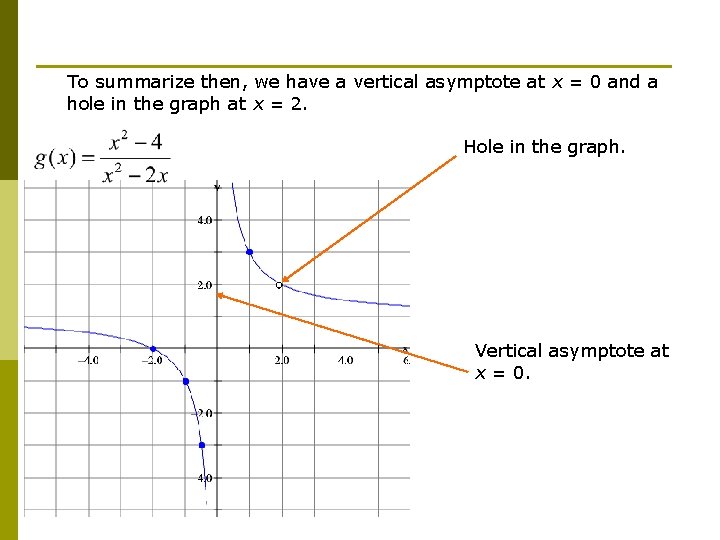
To summarize then, we have a vertical asymptote at x = 0 and a hole in the graph at x = 2. Hole in the graph. Vertical asymptote at x = 0.

Holes (Removable Discontinuities) Ø Ø If x – b is a factor of both the numerator and denominator of a rational function, then there is a hole in the graph of the function where x = b. The exact point of the hole can be found by plugging b into the function after it has been simplified. The hole is known as a removable singularity or a removable discontinuity. When you graph the function on your calculator you won’t be able to see the hole but the function is still discontinuous (has a break or jump).

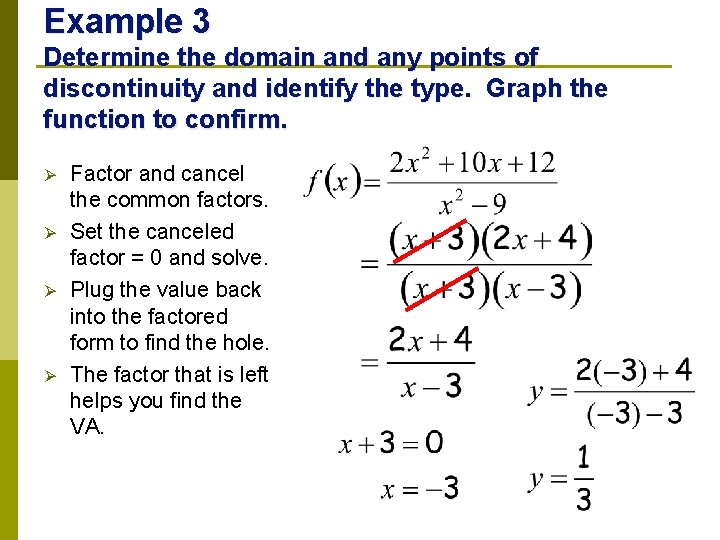
Example 3 Determine the domain and any points of discontinuity and identify the type. Graph the function to confirm. Ø Ø Factor and cancel the common factors. Set the canceled factor = 0 and solve. Plug the value back into the factored form to find the hole. The factor that is left helps you find the VA.

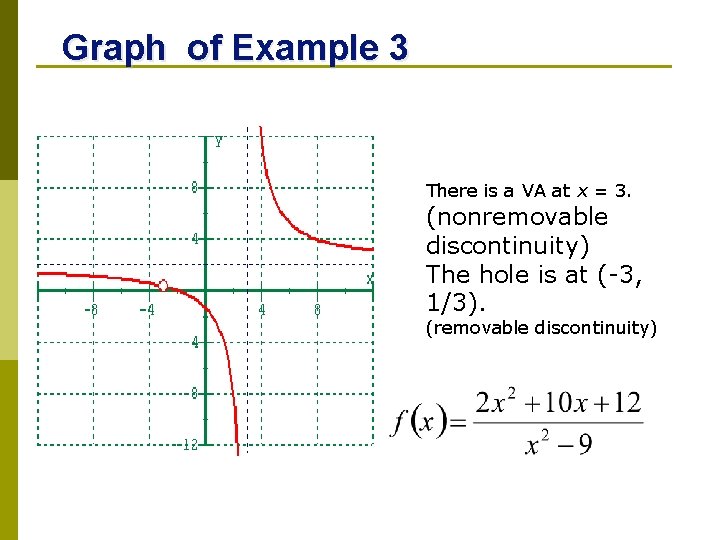
Graph of Example 3 There is a VA at x = 3. (nonremovable discontinuity) The hole is at (-3, 1/3). (removable discontinuity)

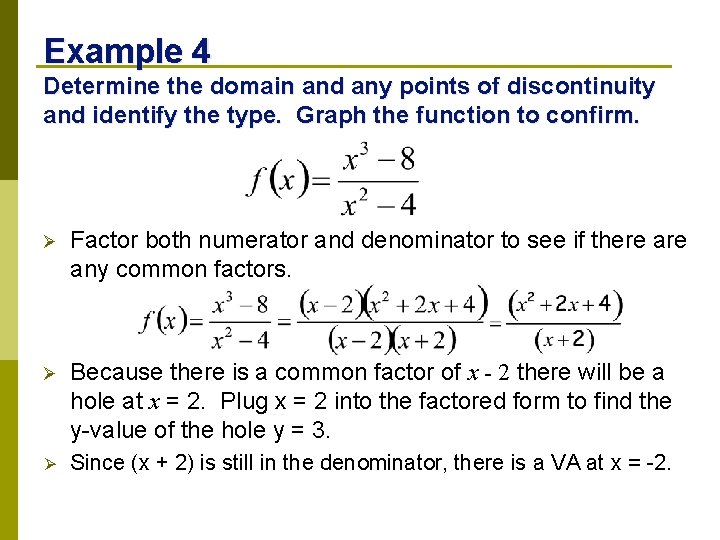
Example 4 Determine the domain and any points of discontinuity and identify the type. Graph the function to confirm. Ø Factor both numerator and denominator to see if there any common factors. Ø Because there is a common factor of x - 2 there will be a hole at x = 2. Plug x = 2 into the factored form to find the y-value of the hole y = 3. Ø Since (x + 2) is still in the denominator, there is a VA at x = -2.

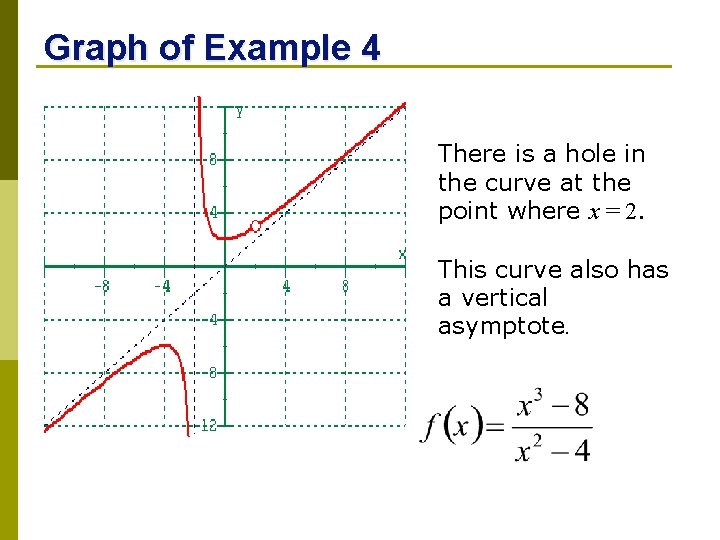
Graph of Example 4 There is a hole in the curve at the point where x = 2. This curve also has a vertical asymptote.

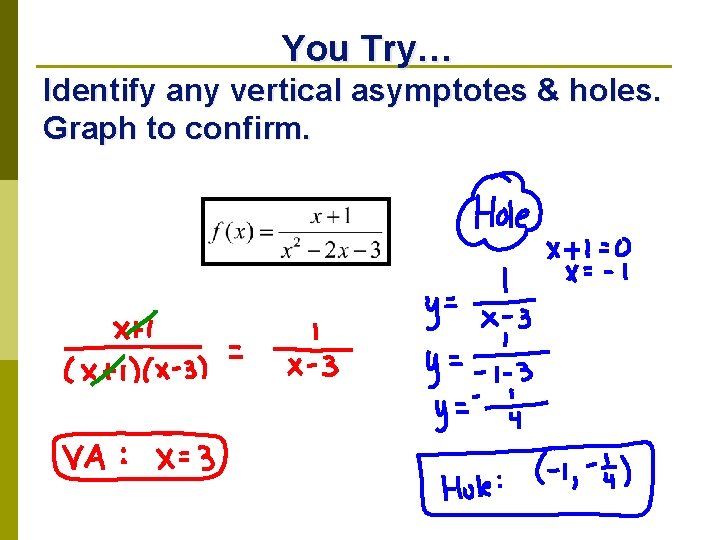
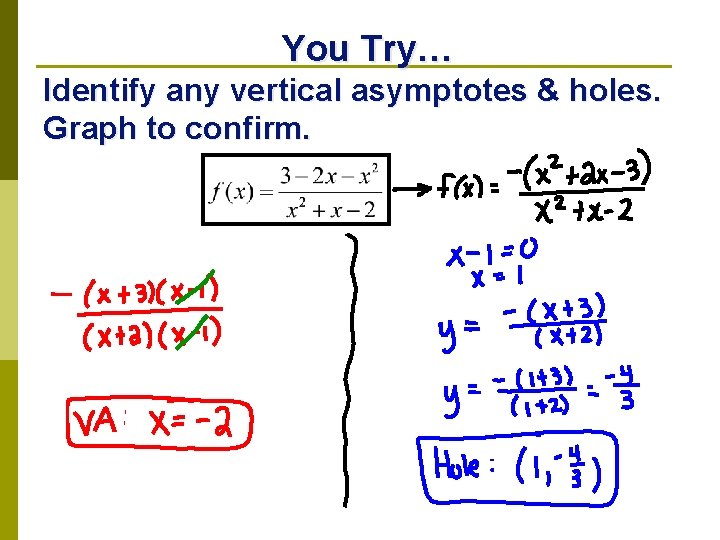
You Try… Identify any vertical asymptotes & holes. Graph to confirm.

You Try… Identify any vertical asymptotes & holes. Graph to confirm.

You Try… Identify any vertical asymptotes & holes. Graph to confirm.

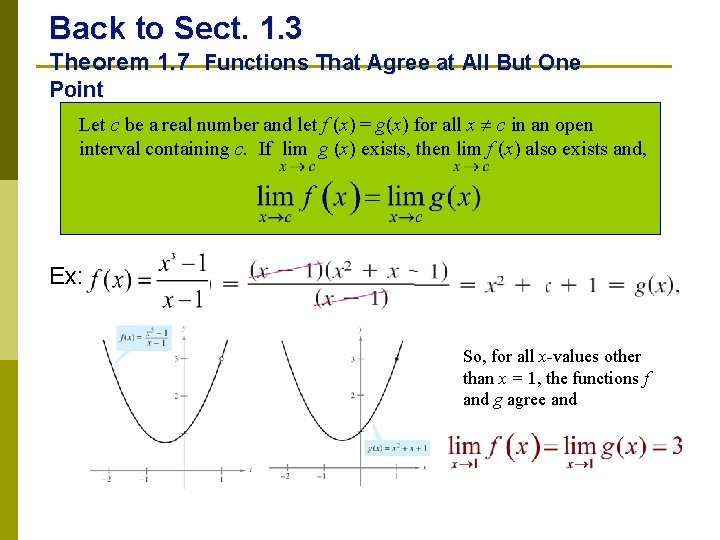
Back to Sect. 1. 3 Theorem 1. 7 Functions That Agree at All But One Point Let c be a real number and let f (x) = g(x) for all x c in an open interval containing c. If lim g (x) exists, then lim f (x) also exists and, Ex: So, for all x-values other than x = 1, the functions f and g agree and

Flowchart for Evaluating Limits Algebraic Manipulation

Factoring and Cancelling Note: Expressions such as since are called indeterminate form the limit cannot be determined. Technique - factor, cancel, and then try direct substitution one more time.

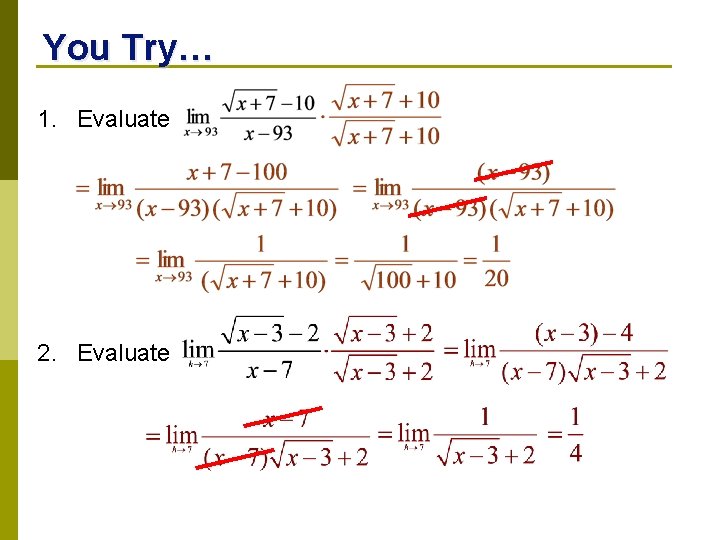
You Try… 1. Evaluate 2. Evaluate

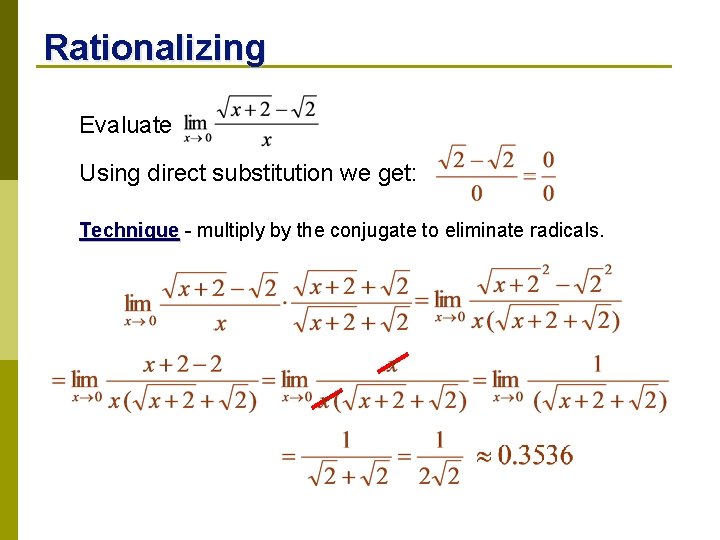
Rationalizing Evaluate Using direct substitution we get: Technique - multiply by the conjugate to eliminate radicals.

A table or a graph can reinforce your conclusion that the limit is.

You Try… 1. Evaluate 2. Evaluate

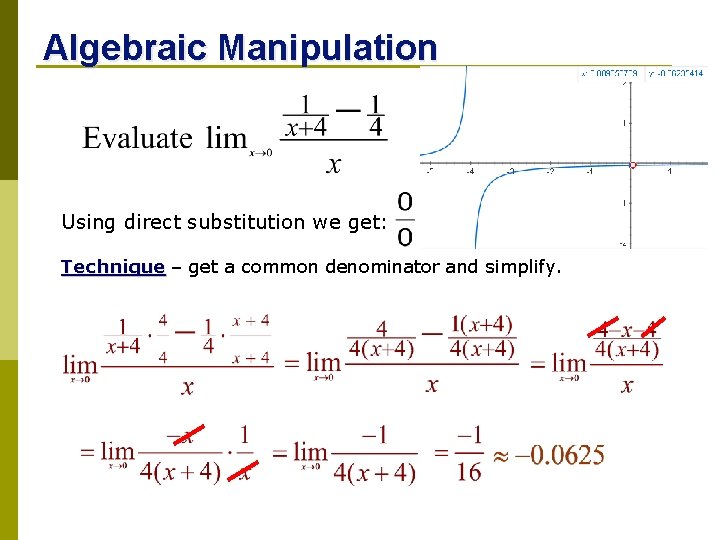
Algebraic Manipulation Using direct substitution we get: Technique – get a common denominator and simplify.

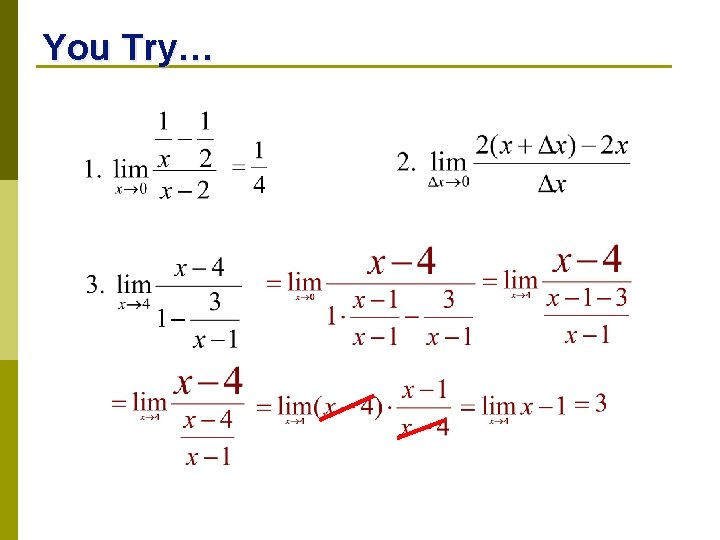
You Try…

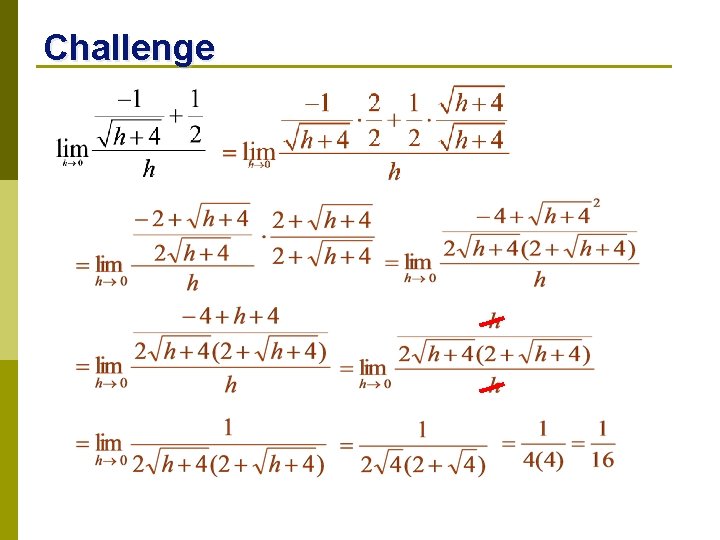
Challenge

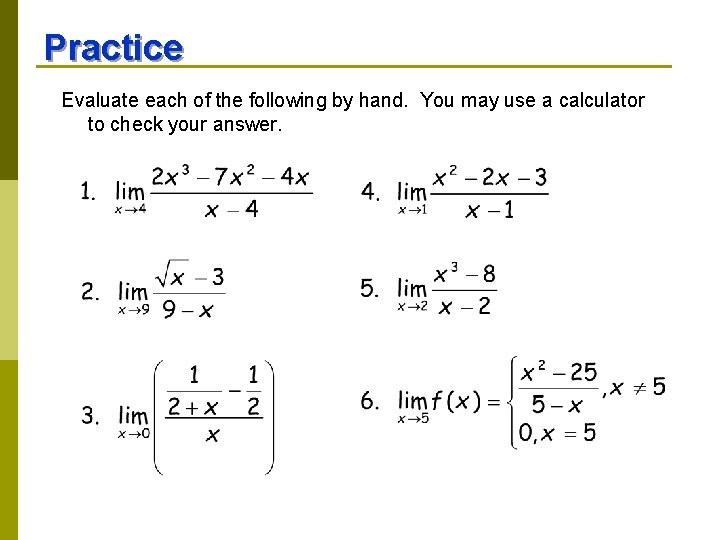
Practice Evaluate each of the following by hand. You may use a calculator to check your answer.

Closure What is meant by an indeterminate form? Give an example where an indeterminate form gives different answers.

1. 3 Evaluating Limits Analytically • Properties of Limits • Techniques for Evaluating Limits – Direct Substitution – Factoring and Cancelling – Rationalizing – Algebraic Manipulation • Trigonometric Rules • The Sandwich Theorem

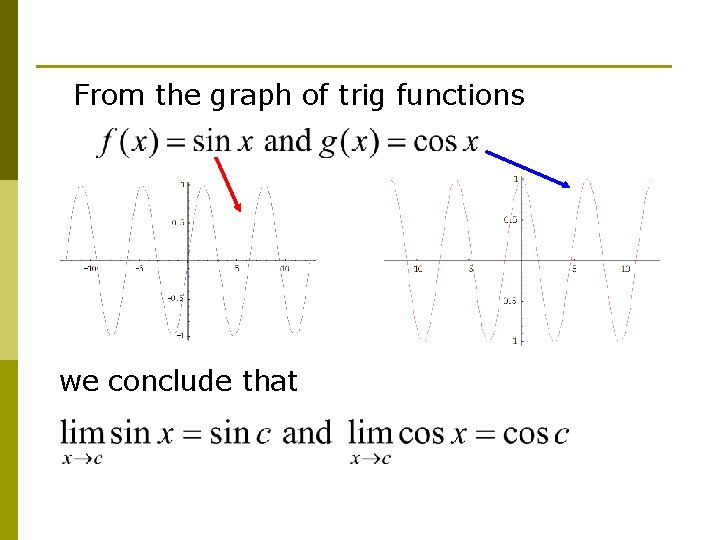
From the graph of trig functions we conclude that

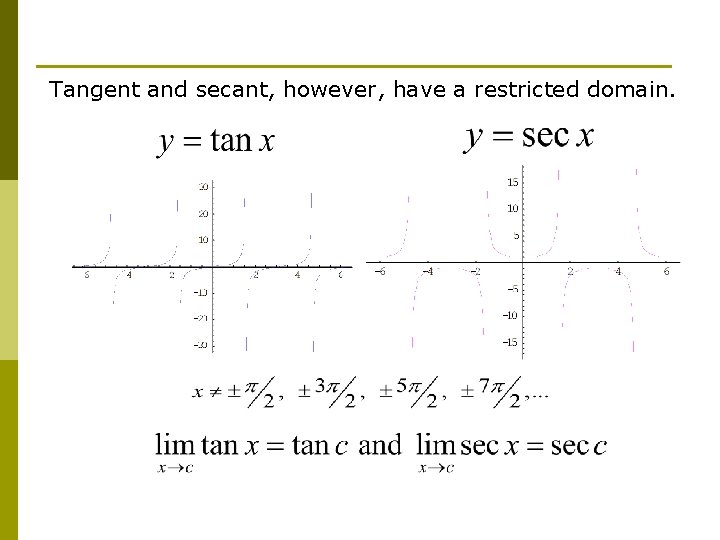
Tangent and secant, however, have a restricted domain.

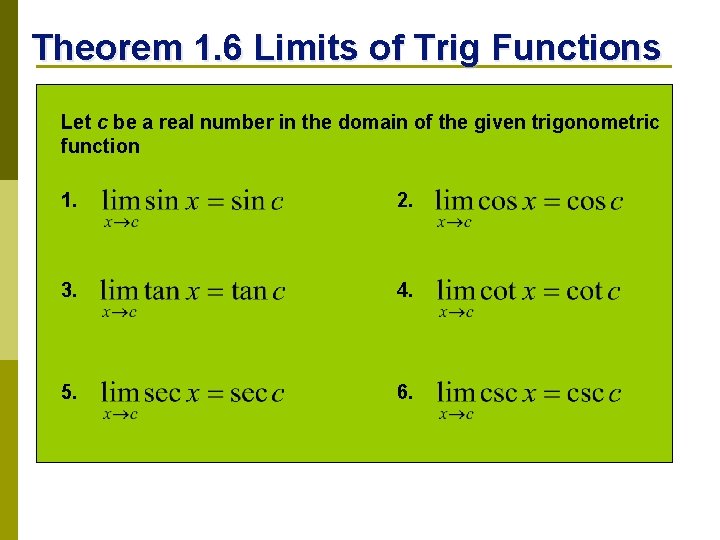
Theorem 1. 6 Limits of Trig Functions Let c be a real number in the domain of the given trigonometric function 1. 2. 3. 4. 5. 6.

Limits of Trig Functions Examples: 1. 2. 3.

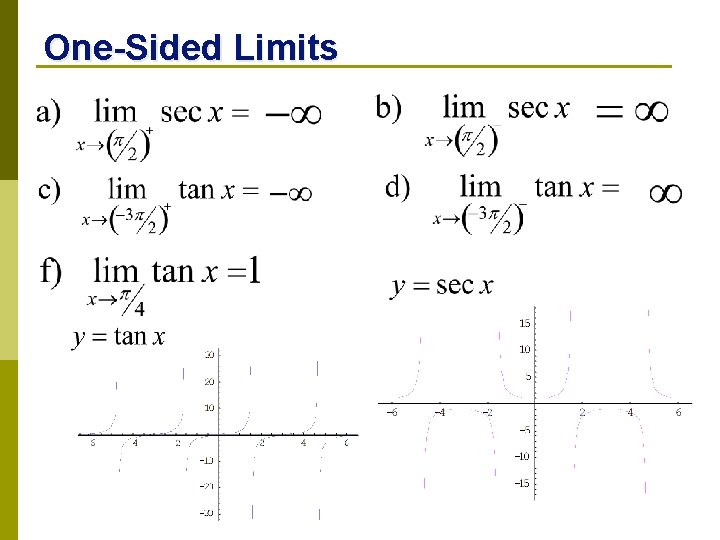
One-Sided Limits

Flowchart for Evaluating Limits Algebraic Manipulation

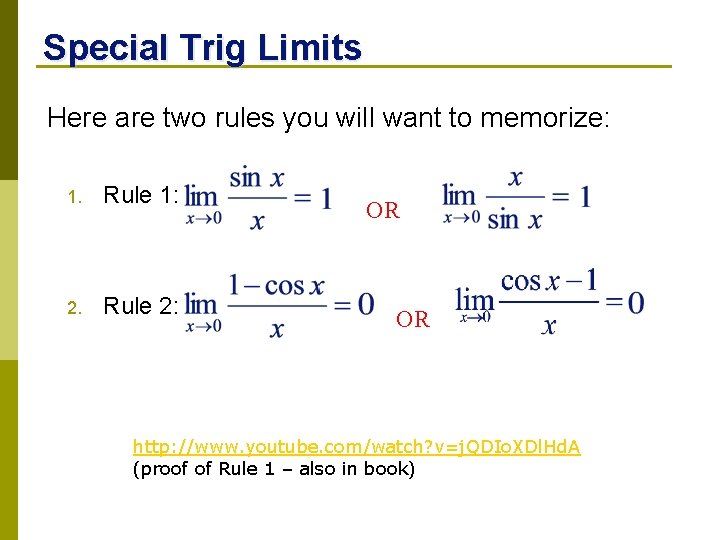
Special Trig Limits Here are two rules you will want to memorize: 1. Rule 1: 2. Rule 2: OR OR http: //www. youtube. com/watch? v=j. QDIo. XDl. Hd. A (proof of Rule 1 – also in book)

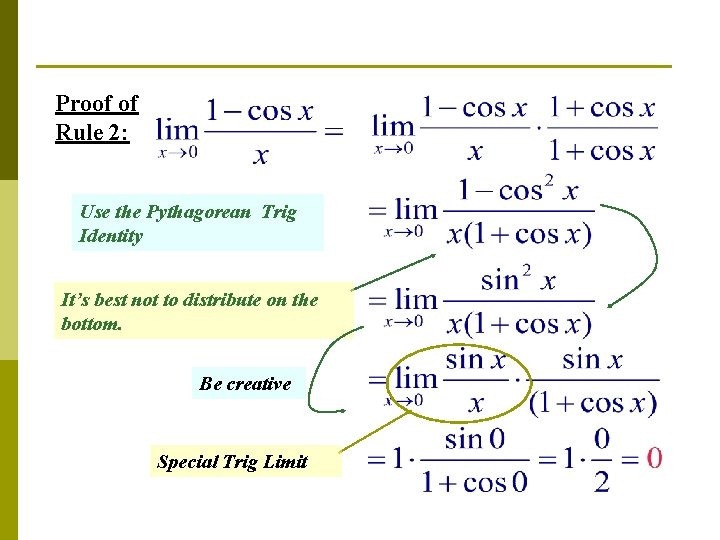
Proof of Rule 2: Use the Pythagorean Trig Identity It’s best not to distribute on the bottom. Be creative Special Trig Limit

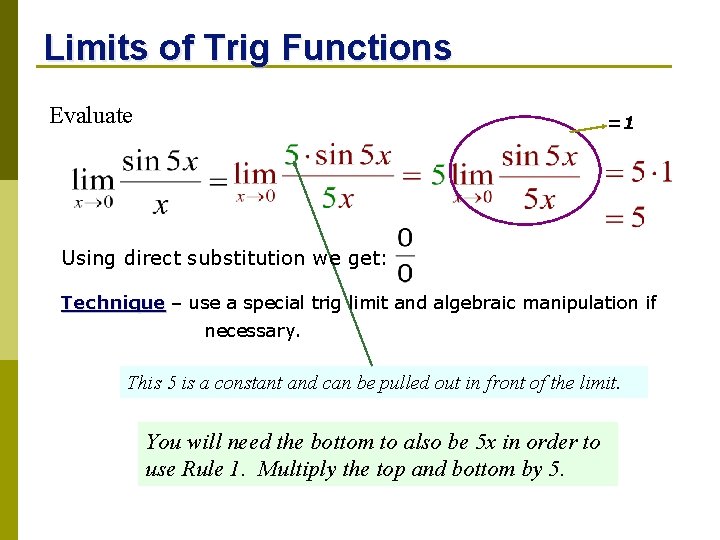
Limits of Trig Functions Evaluate =1 Using direct substitution we get: Technique – use a special trig limit and algebraic manipulation if necessary. This 5 is a constant and can be pulled out in front of the limit. You will need the bottom to also be 5 x in order to use Rule 1. Multiply the top and bottom by 5.

Evaluate =1 Direct substitution won’t work. We can use the sine trig limit, but first we’ll have to use some algebra since we need the bottom to be a 3 x. Evaluate Direct substitution works at this point since the bottom of the fraction will not be 0 when you use π/2.

You Try… Calculate each of the following limits. 1. 2. 3.

Evaluate 3 7

You Try… Calculate each of the following limits. 1. 2. 3.

Trigonometric Rules of Limits Here a few more (but are not necessary to memorize): Rule 3: OR Rule 4: OR Rule 5: OR

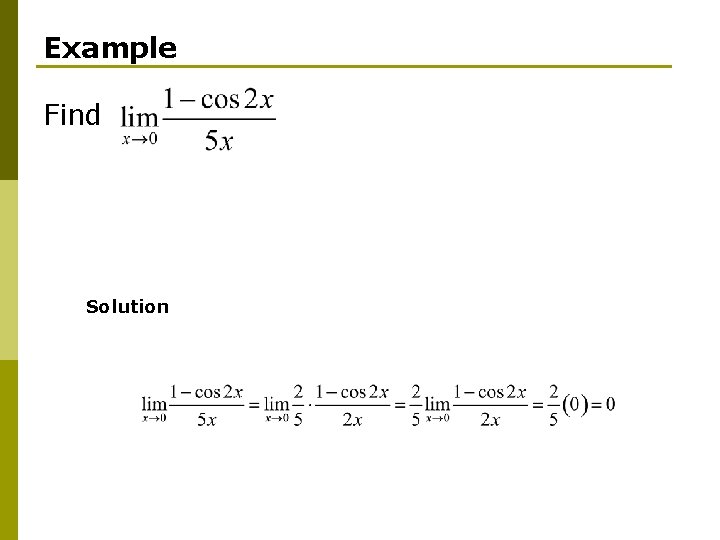
Example Find Solution

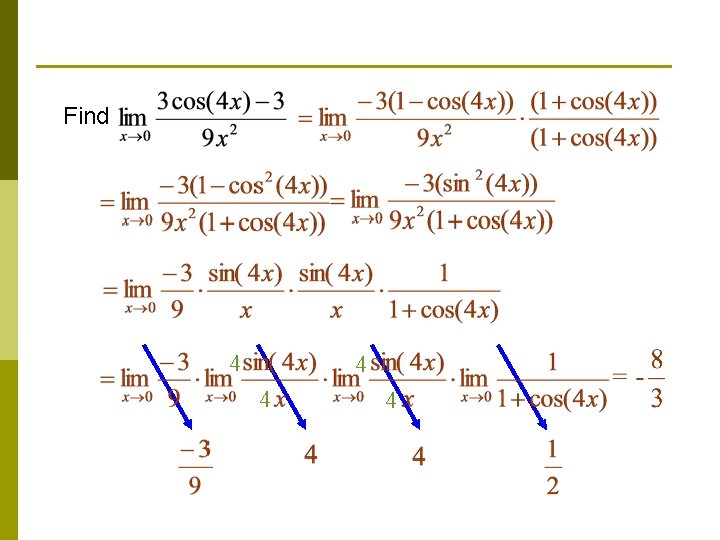
Find 4 4

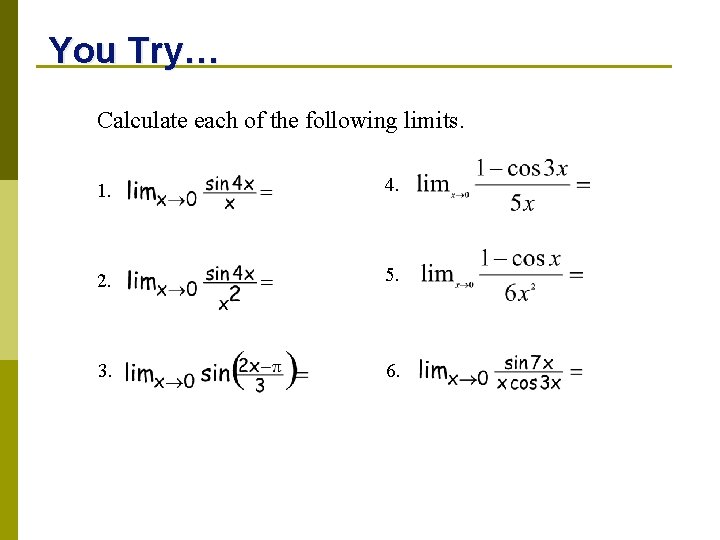
You Try… Calculate each of the following limits. 1. 4. 2. 5. 3. 6.

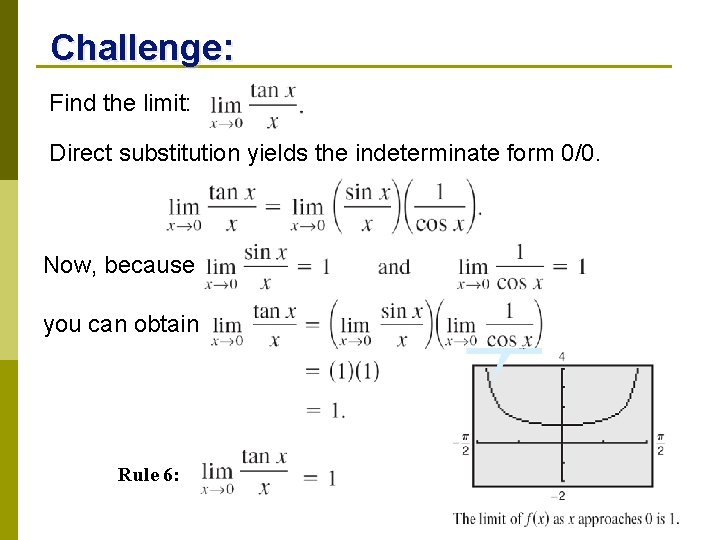
Challenge: Find the limit: Direct substitution yields the indeterminate form 0/0. Now, because you can obtain Rule 6:

You Try… Calculate each of the following limits. 1. 2. 3.

Flowchart for Evaluating Limits Algebraic Manipulation

Evaluating Limits Summary 1 st substitution get answer? ? Then you are done get ? ? Then limit is 0. get ? ? Then limit DNE (∞ or -∞). get ? ? indeterminate form Þ polynomial ? ? Try factoring and canceling. Þ radical? ? Try rationalizing (multiply by the conjugate). Þ fraction in a fraction? ? Try a common denominator and simplify. Þ trig function? ? Try a special trig limit

1. 3 Evaluating Limits Analytically • Properties of Limits • Techniques for Evaluating Limits – Direct Substitution – Factoring and Cancelling – Rationalizing – Algebraic Manipulation • Trigonometric Rules • The Sandwich Theorem

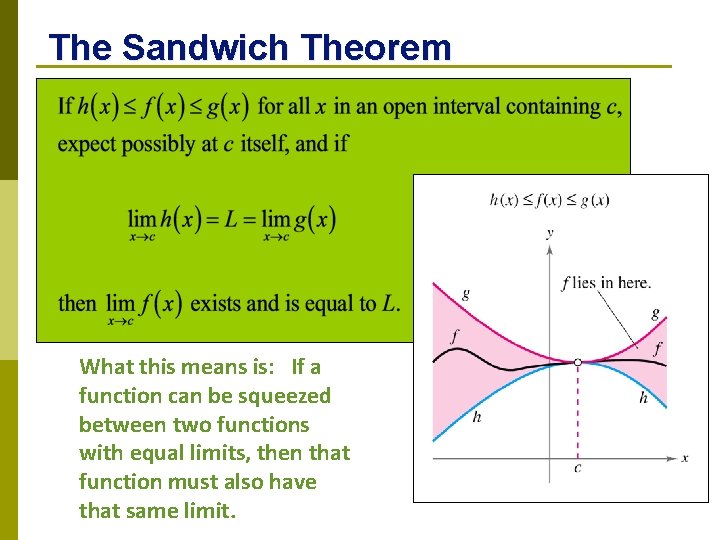
The Sandwich Theorem What this means is: If a function can be squeezed between two functions with equal limits, then that function must also have that same limit.

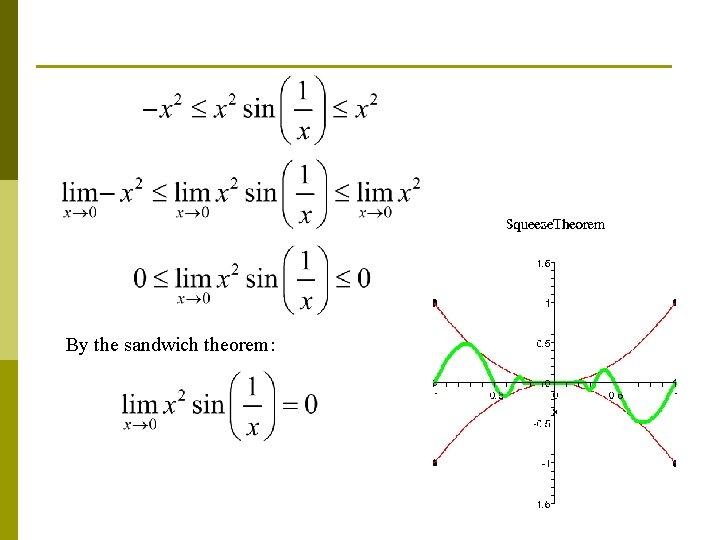
Example Show that: The maximum value of sine is 1, so The minimum value of sine is -1, so So:

By the sandwich theorem:

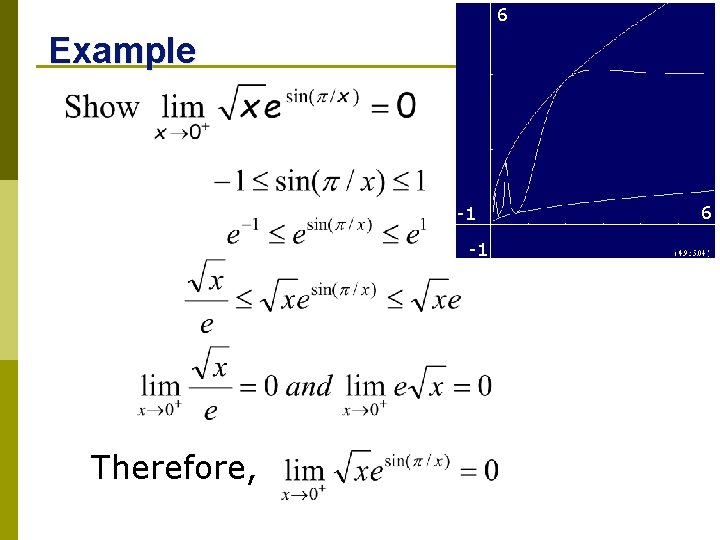
6 Example -1 -1 Therefore, 6

You Try… Ø Calculate the following limits. 1. =0 2. = 29 200 5 -5 -200

Techniques for Evaluating Limits Ø It can be a little trickier to determine limits without the benefit of looking at a graph; but with the help of a few techniques, it’s very manageable: 1. Direct substitution Ø 2. Factor and Cancel Ø 3. Get a common denominator and simplify. Trigonometric Rules Ø 6. Multiply by the conjugate to eliminate radicals Algebraic Manipulation Ø 5. Factor, cancel, then use direct substitution Rationalization Ø 4. Plug in c into the function Apply special trigonometric limits The Sandwich Theorem Ø Try to squeeze the function between two functions whose limits can be easily calculated and are equal.
 Limits and their properties
Limits and their properties Chapter 1 limits and their properties
Chapter 1 limits and their properties Limits of composite functions
Limits of composite functions Limits and their properties
Limits and their properties Infinite limits and limits at infinity
Infinite limits and limits at infinity Shape 65
Shape 65 Elements and their properties chapter 17
Elements and their properties chapter 17 Elements and their properties section 1 metals
Elements and their properties section 1 metals Calculus chapter 2 limits and continuity answers
Calculus chapter 2 limits and continuity answers Chapter 1 limits alternatives and choices
Chapter 1 limits alternatives and choices Extensive examples
Extensive examples Physical properties and chemical properties
Physical properties and chemical properties 9-1 quadratic graphs and their properties
9-1 quadratic graphs and their properties Plane figures and their properties
Plane figures and their properties Quadrilaterals and their properties activity 15
Quadrilaterals and their properties activity 15 Site:slidetodoc.com
Site:slidetodoc.com Properties of cartridge paper
Properties of cartridge paper Lesson 2 session 3
Lesson 2 session 3 Relations and their properties
Relations and their properties Relations and their properties
Relations and their properties Relations and their properties
Relations and their properties Chapter 17 section 3 mixed groups
Chapter 17 section 3 mixed groups Classify polygons
Classify polygons Inner transition metals
Inner transition metals China limits european contacts
China limits european contacts Chapter 5 section 5 parliament limits the english monarchy
Chapter 5 section 5 parliament limits the english monarchy How did hongwu bring stability to china
How did hongwu bring stability to china Chapter 5 section 5 parliament limits the english monarchy
Chapter 5 section 5 parliament limits the english monarchy Investigating graphs of polynomial functions
Investigating graphs of polynomial functions Investigating graphs of functions for their properties
Investigating graphs of functions for their properties Property of solids
Property of solids Classification of waste according to their properties
Classification of waste according to their properties Doth with their death
Doth with their death Limit and continuity solved problems
Limit and continuity solved problems Ternology
Ternology Ionizing radiation examples
Ionizing radiation examples Difference between allowance and tolerance
Difference between allowance and tolerance 50f7 tolerance
50f7 tolerance Limits and derivatives class 11 pdf
Limits and derivatives class 11 pdf Thomas calculus limits and continuity
Thomas calculus limits and continuity Liquid limit of soil formula
Liquid limit of soil formula Limits and derivatives
Limits and derivatives Lesson 2: understanding limits graphically and numerically
Lesson 2: understanding limits graphically and numerically Estimating limits numerically
Estimating limits numerically Finding limits graphically
Finding limits graphically Limits at infinity horizontal asymptotes
Limits at infinity horizontal asymptotes Estimating limits graphically
Estimating limits graphically Limits and continuity
Limits and continuity Finding limits graphically and numerically
Finding limits graphically and numerically Limits and continuity
Limits and continuity Lesson 2 understanding limits graphically and numerically
Lesson 2 understanding limits graphically and numerically Application of limits and continuity
Application of limits and continuity Limits and derivatives
Limits and derivatives Limits at infinity (horizontal asymptotes)
Limits at infinity (horizontal asymptotes) Find the measure of mn
Find the measure of mn Chapter 11 properties of the hair and scalp answers
Chapter 11 properties of the hair and scalp answers Hair shaft diagram milady
Hair shaft diagram milady Chapter 26 section 1 arthropod characteristics answer key
Chapter 26 section 1 arthropod characteristics answer key Section 1 organisms and their relationships
Section 1 organisms and their relationships Principles of ecology section 2 flow of energy
Principles of ecology section 2 flow of energy Principles of ecology chapter 2 section 3 answer key
Principles of ecology chapter 2 section 3 answer key Chapter 2 principles of ecology answer key
Chapter 2 principles of ecology answer key Chapter 18 genomes and their evolution
Chapter 18 genomes and their evolution Chapter 1 functions and their graphs
Chapter 1 functions and their graphs Chapter 11 school policies and their functions
Chapter 11 school policies and their functions Chapter 1 laws and their ethical foundation
Chapter 1 laws and their ethical foundation Chapter 2 functions and their graphs answers
Chapter 2 functions and their graphs answers Chapter 2 section 1 organisms and their relationships
Chapter 2 section 1 organisms and their relationships Chapter 17 atlantic revolutions and their echoes
Chapter 17 atlantic revolutions and their echoes Chapter 1 laws and their ethical foundation
Chapter 1 laws and their ethical foundation Dts-500
Dts-500 Limit rules
Limit rules 117 table b
117 table b The limits of quantum computers
The limits of quantum computers Sec asymptotes
Sec asymptotes Royal london medical underwriting limits
Royal london medical underwriting limits How to calculate control limits
How to calculate control limits Content uniformity test definition
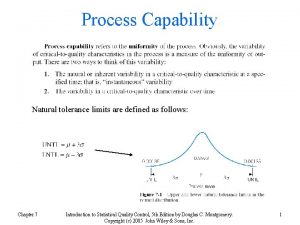
Content uniformity test definition Natural tolerance
Natural tolerance Gonad shielding
Gonad shielding Examples of limits of authority
Examples of limits of authority Trigonometric limit identities
Trigonometric limit identities