Reflection and Transmission Asst Prof Dr zgr Tamer

Reflection and Transmission Asst. Prof. Dr. Özgür Tamer

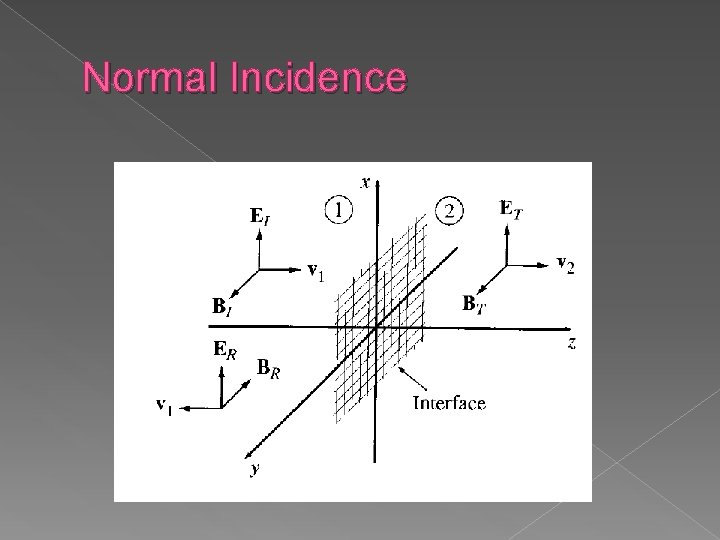
Normal Incidence

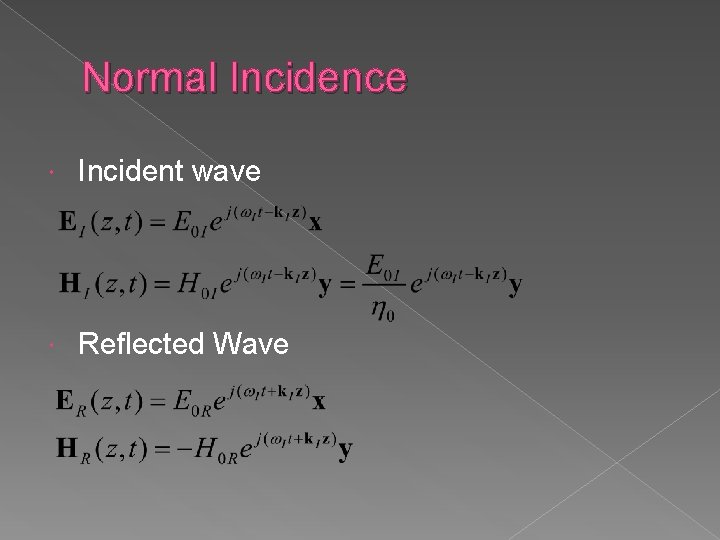
Normal Incidence Incident wave Reflected Wave

Normal Incidence Transmitted wave

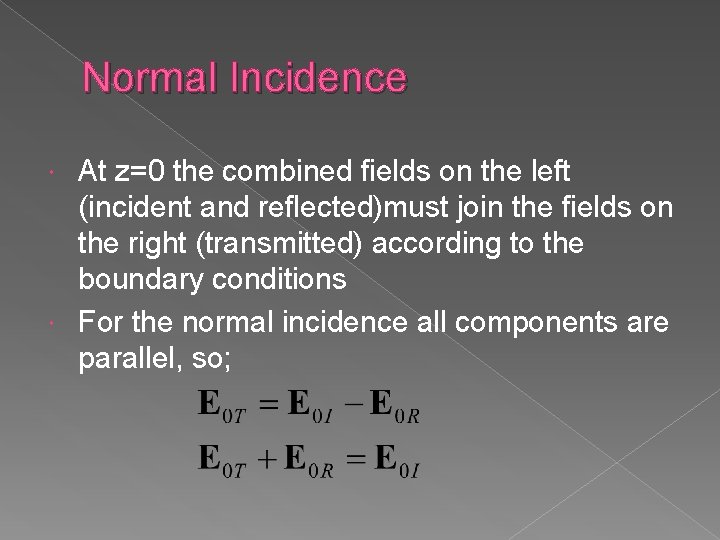
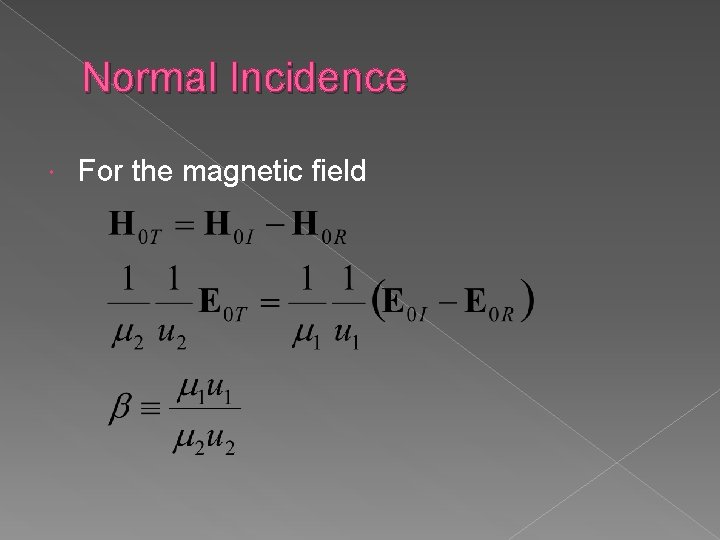
Normal Incidence At z=0 the combined fields on the left (incident and reflected)must join the fields on the right (transmitted) according to the boundary conditions For the normal incidence all components are parallel, so;

Normal Incidence For the magnetic field

Normal Incidence Reflected and Transmitted waves can be found as

Normal Incidence If the permeabilities are close to μ 0, then;

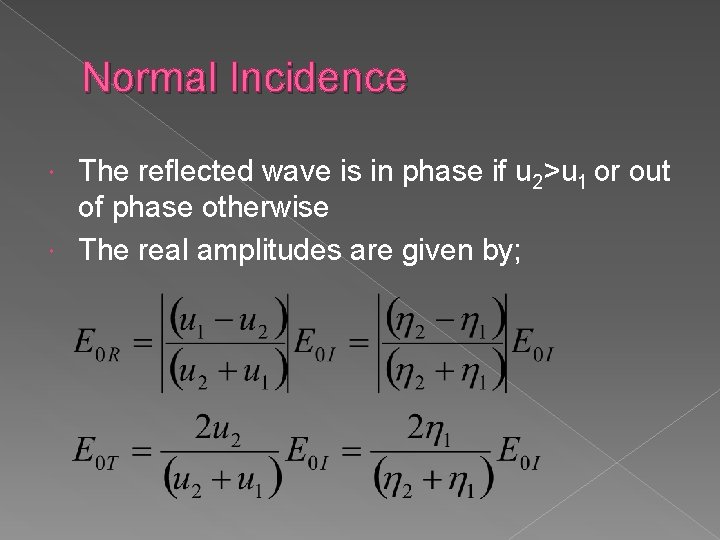
Normal Incidence The reflected wave is in phase if u 2>u 1 or out of phase otherwise The real amplitudes are given by;

Normal Incidence Energy: The Intensity (average power per unit area) is given by

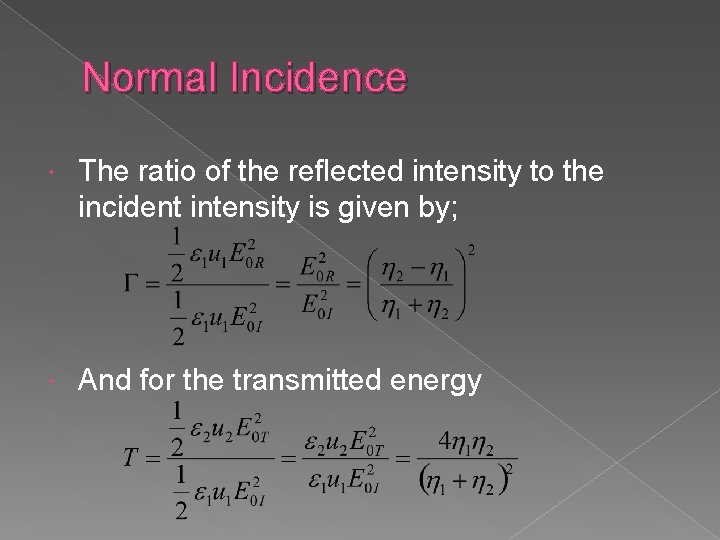
Normal Incidence The ratio of the reflected intensity to the incident intensity is given by; And for the transmitted energy

Normal Incidence Г is called the reflection coefficient and T is called the transmission coefficient

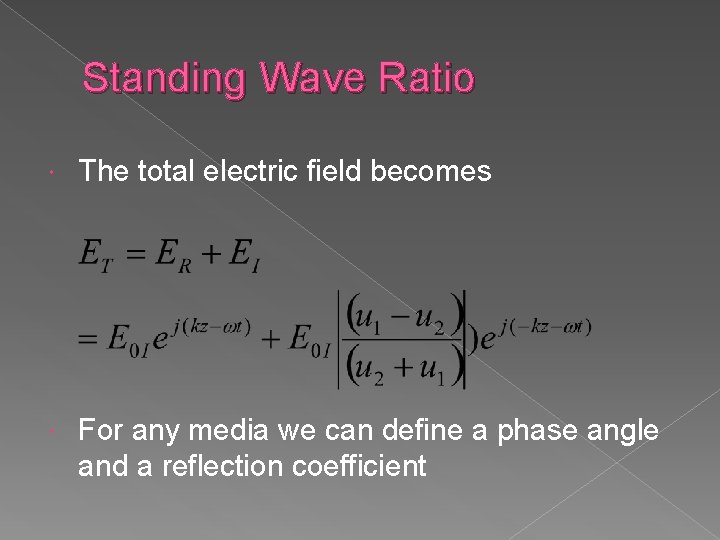
Standing Wave Ratio The total electric field becomes For any media we can define a phase angle and a reflection coefficient

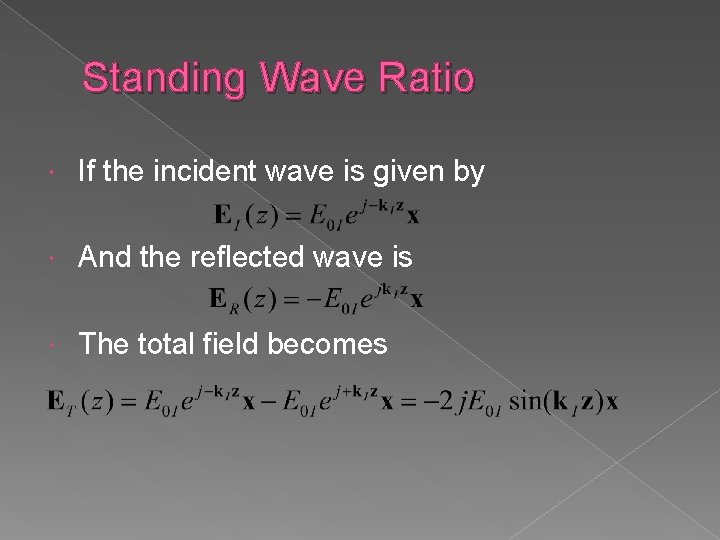
Standing Wave Ratio If the incident wave is given by And the reflected wave is The total field becomes

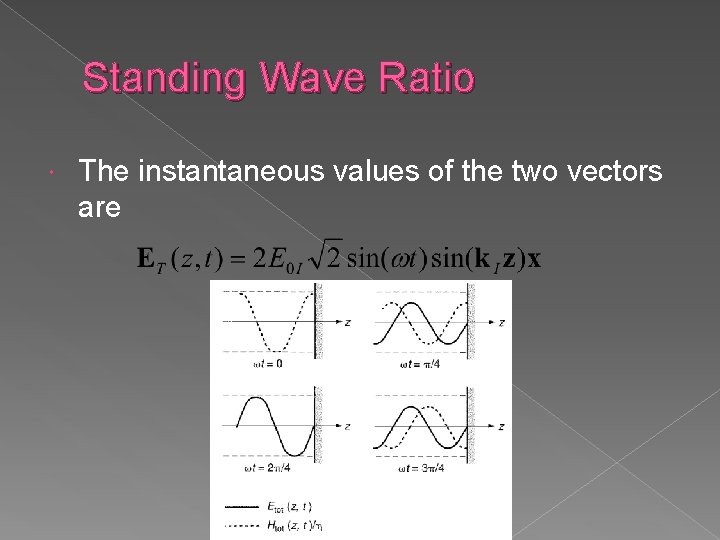
Standing Wave Ratio Since The total wave does not contain the factor it is a standing wave instead of a travelling wave. Etot(Z, t)=EI(Z, t)+ER(Z, t) There are planes in which Etot(Z, t) is minimum and maximum at all times

Standing Wave Ratio The instantaneous values of the two vectors are

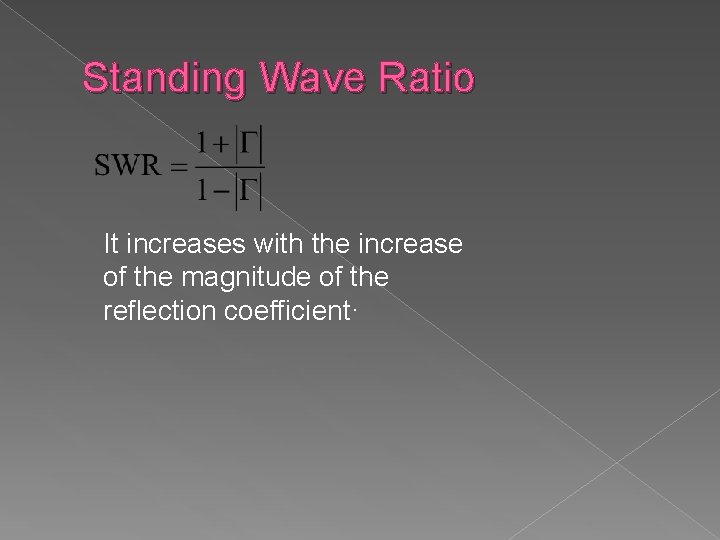
Standing Wave Ratio

Standing Wave Ratio It increases with the increase of the magnitude of the reflection coefficient·

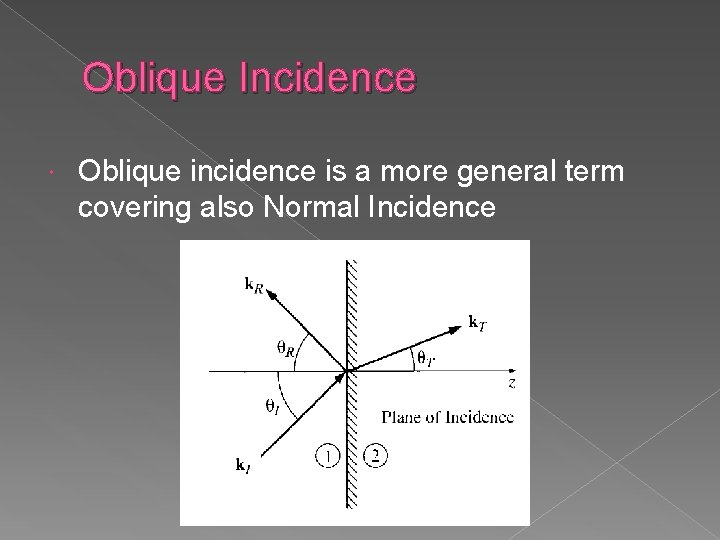
Oblique Incidence Oblique incidence is a more general term covering also Normal Incidence

Oblique Incidence Again we have an incident wave and the reflected wave becomes

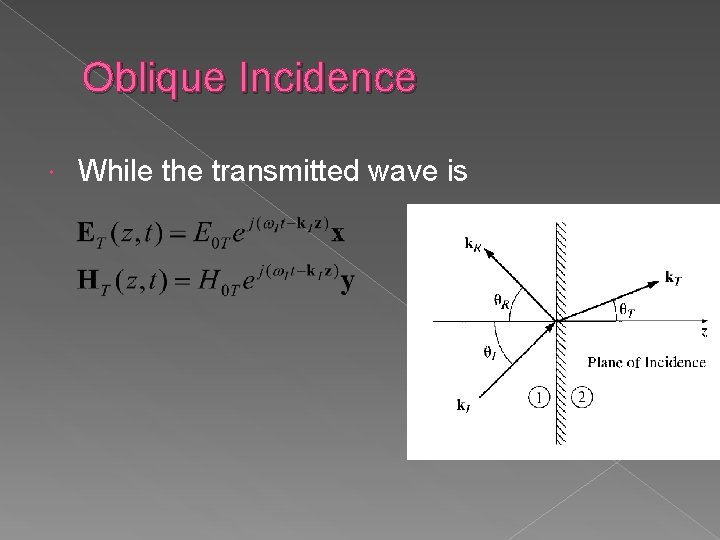
Oblique Incidence While the transmitted wave is

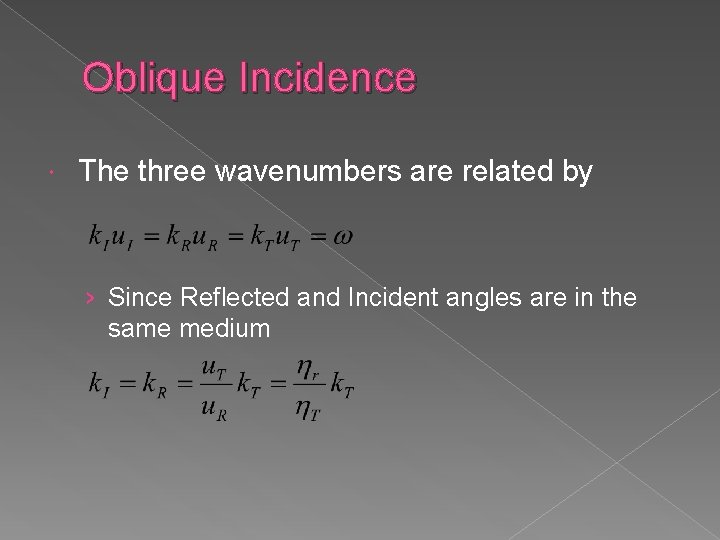
Oblique Incidence The three wavenumbers are related by › Since Reflected and Incident angles are in the same medium

Oblique Incidence The combined fields in medium 1 Must be joined with the fields in medium 2 Boundary conditions must be satisfied all points on the plane

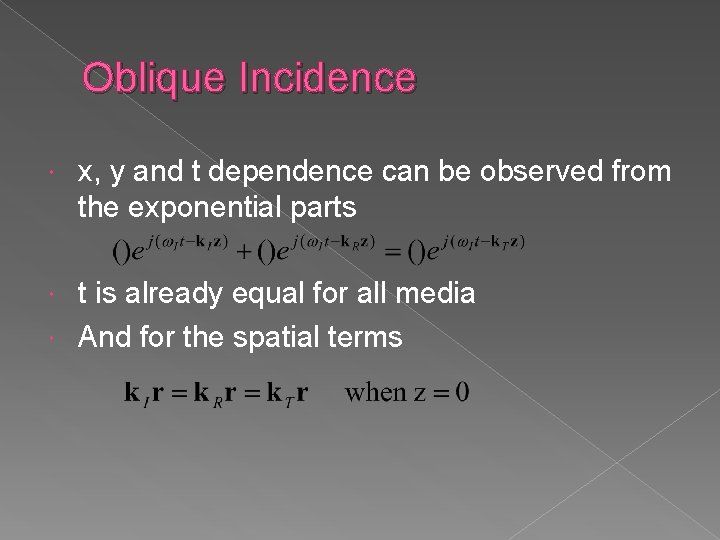
Oblique Incidence x, y and t dependence can be observed from the exponential parts t is already equal for all media And for the spatial terms

Oblique Incidence More explicitly

Oblique Incidence Lets be more specific and For this case kı lies in the x z plane as well as k. R and k. T Concluding: › The incident, reflected and transmitted wave vectors form a plane which also includes the normal to the surface

Oblique Incidence where θI is the angle of incidence, θR is the angle of reflection, and θT is the angle of transmission (more commonly known as the angle of refraction) all of them measured with respect to the normal

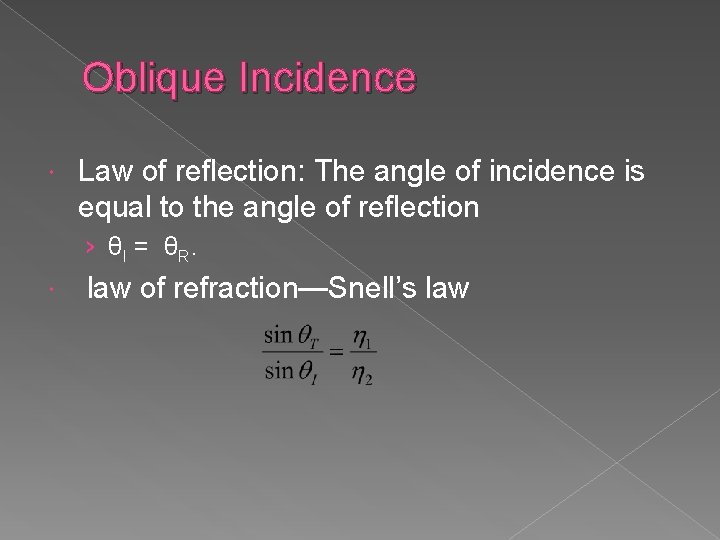
Oblique Incidence Law of reflection: The angle of incidence is equal to the angle of reflection › θ I = θ R. law of refraction—Snell’s law

Oblique Incidence Since we got rid of the exponential parts the boundary conditions become

Oblique Incidence If the polarization of the incident wave is parallel to the plane of incidence, reflected and transmitted waves are also polarized in the same plane Then we have using the first boundary condition

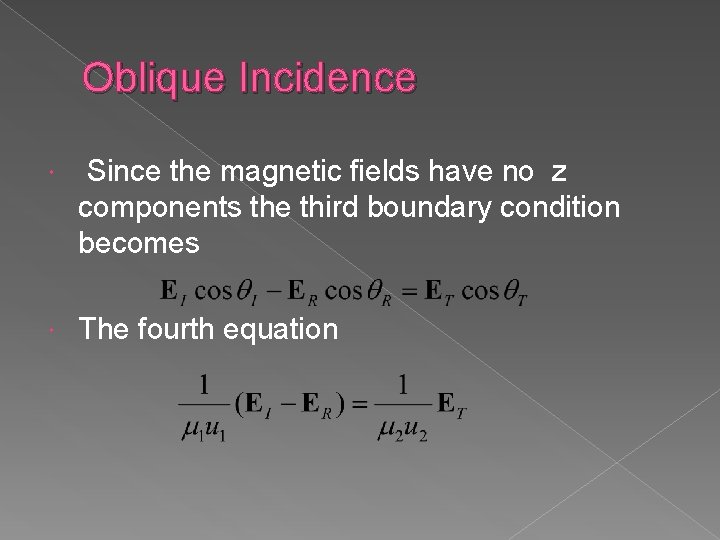
Oblique Incidence Since the magnetic fields have no z components the third boundary condition becomes The fourth equation

Oblique Incidence

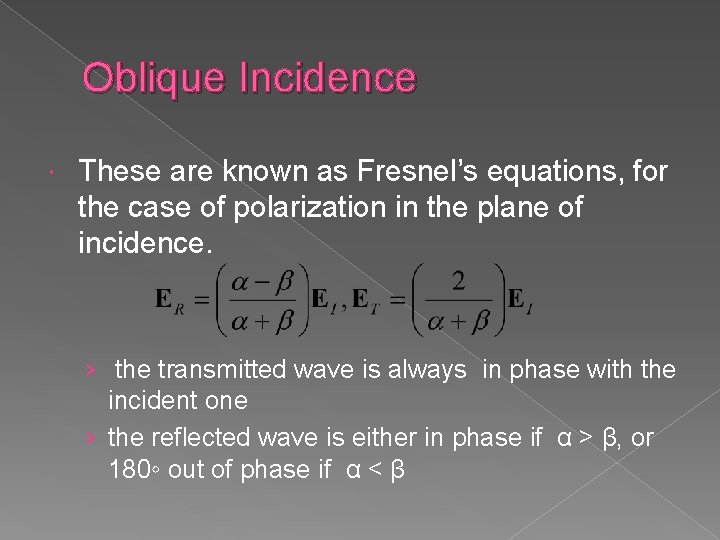
Oblique Incidence These are known as Fresnel’s equations, for the case of polarization in the plane of incidence. › the transmitted wave is always in phase with the incident one › the reflected wave is either in phase if α > β, or 180◦ out of phase if α < β

Oblique Incidence The amplitudes of the transmitted and reflected waves depend on the angle of incidence, because α is a function of θI › In the case of normal incidence (θI=0), α=1 › At grazing incidence (θI= 90◦), α diverges, and the wave is totally reflected

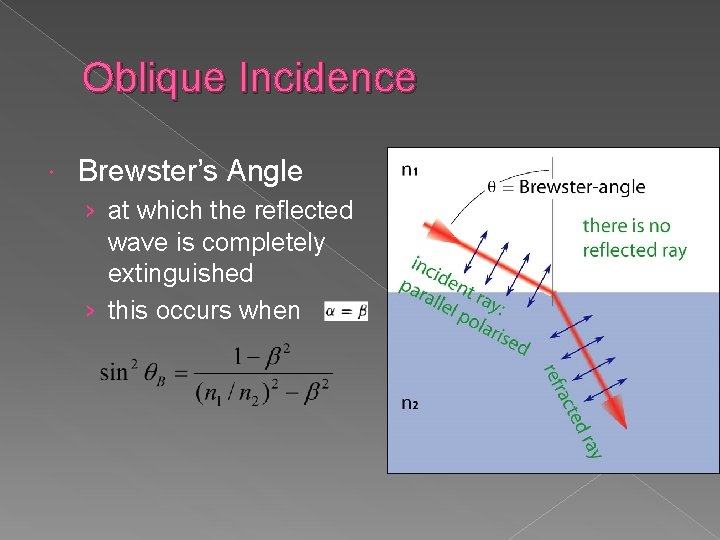
Oblique Incidence Brewster’s Angle › at which the reflected wave is completely extinguished › this occurs when

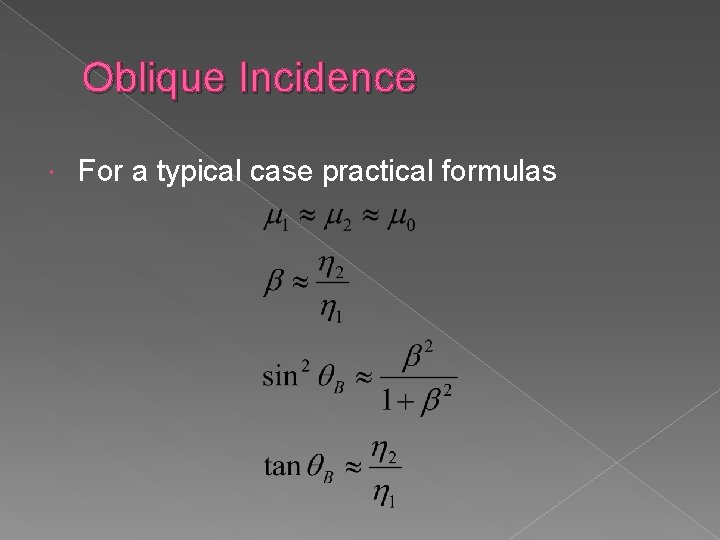
Oblique Incidence For a typical case practical formulas

Oblique Incidence › light incident on glass (n 2=1. 5) from air (n 1=1)

Oblique Incidence The incident intensity is while the reflected and transmitted intensities are

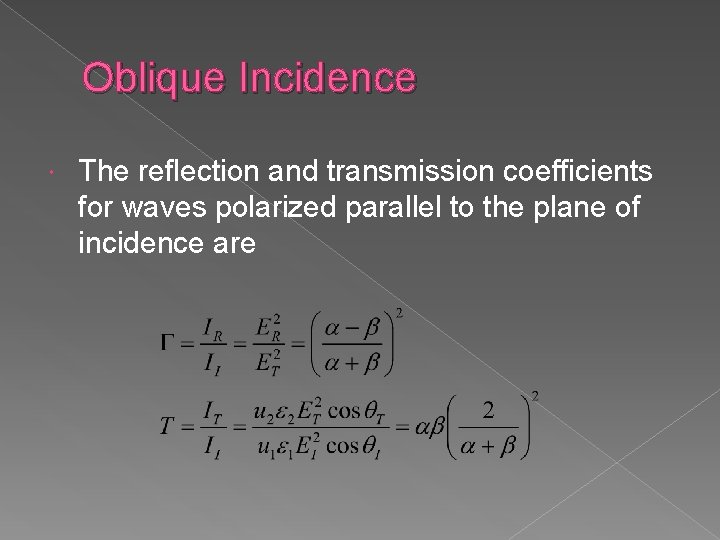
Oblique Incidence The reflection and transmission coefficients for waves polarized parallel to the plane of incidence are

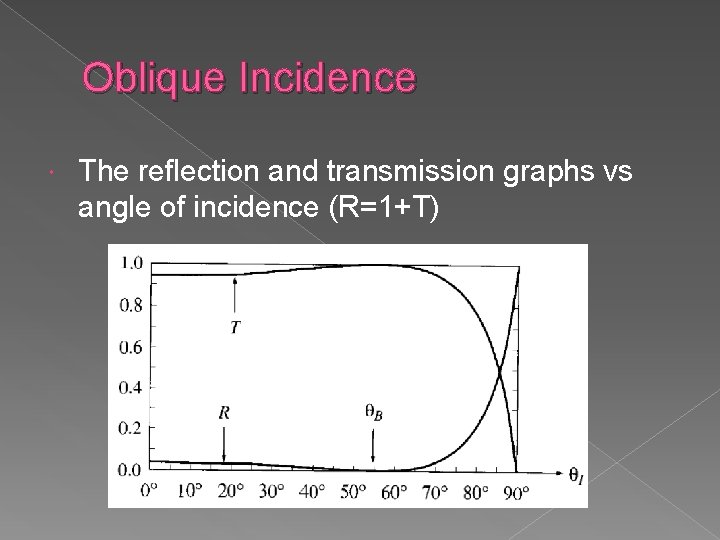
Oblique Incidence The reflection and transmission graphs vs angle of incidence (R=1+T)

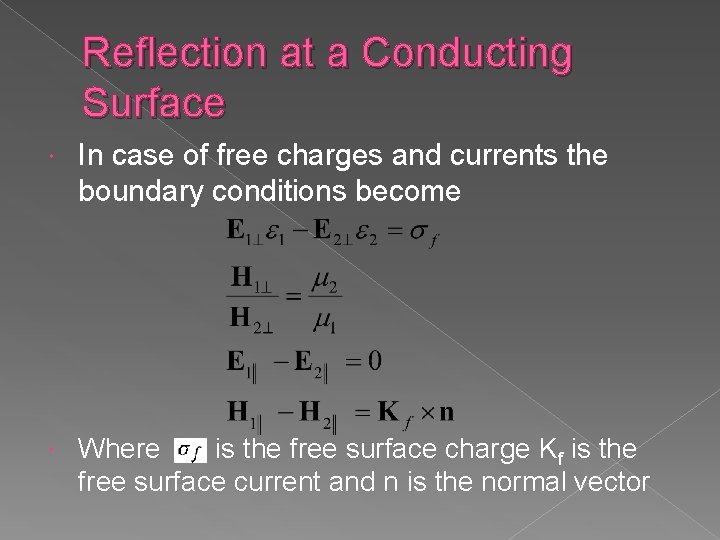
Reflection at a Conducting Surface In case of free charges and currents the boundary conditions become Where is the free surface charge Kf is the free surface current and n is the normal vector

Reflection at a Conducting Surface For ohmic conductors there can be no free surface current since J is a volume current density which is a current per unit area perpendicular to the flow direction A surface current density is amps per unit length perpendicular to the flow. This 'length' is just a 1 -D line. . . it has no area. We set K=0 in the boundary conditions

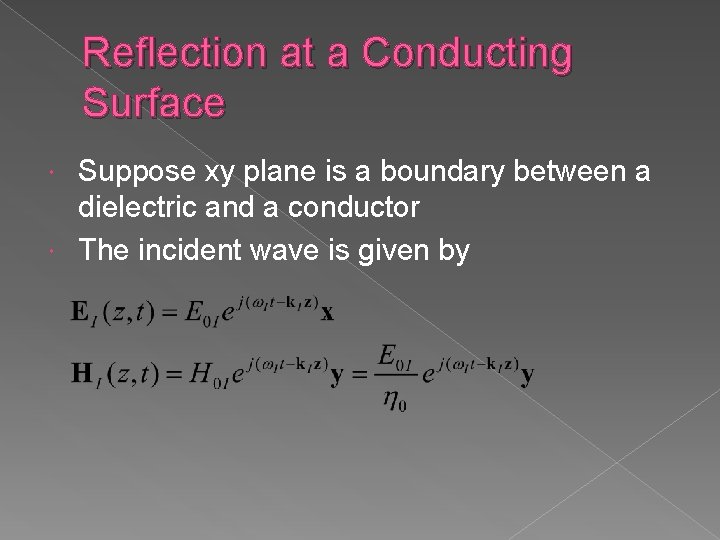
Reflection at a Conducting Surface Suppose xy plane is a boundary between a dielectric and a conductor The incident wave is given by

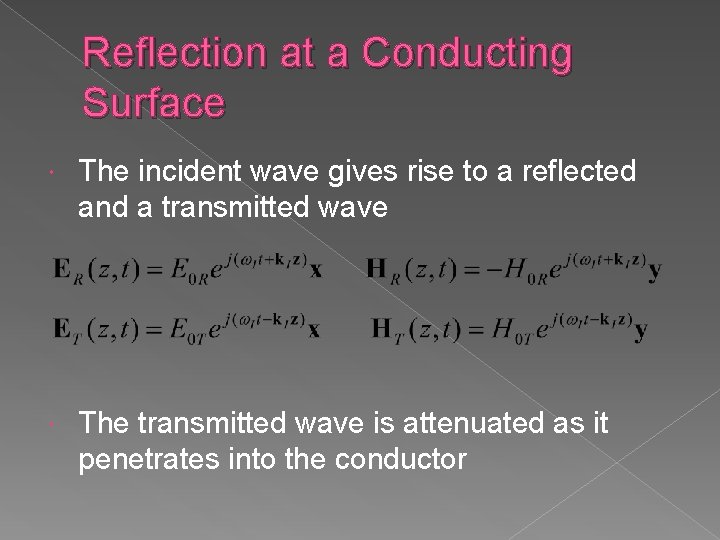
Reflection at a Conducting Surface The incident wave gives rise to a reflected and a transmitted wave The transmitted wave is attenuated as it penetrates into the conductor

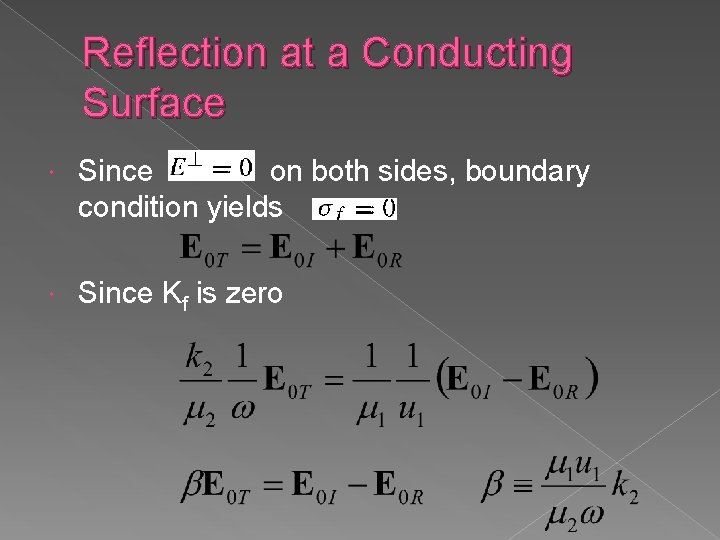
Reflection at a Conducting Surface Since on both sides, boundary condition yields Since Kf is zero

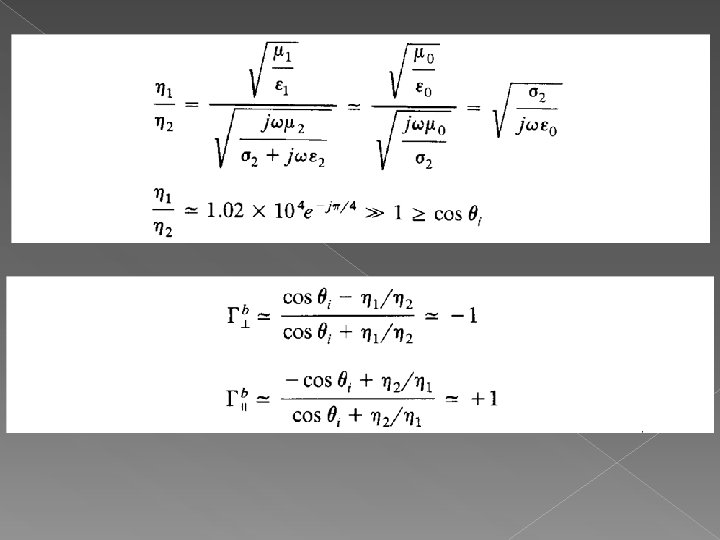
Reflection at a Conducting Surface And similarly we have For a perfect conductor The wave is totally reflected with a 180 degrees phase shift

Problem 1 Find the reflection and transmission coefficients for the interface between air and fresh water (E = 81ε 0, σ = 0), in the case of perpendicular incidence.

Problem 2 A plane wave is normally incident on the interface between air and a dielectric having a permeability μ= μo, and an unknown permittivity. The measured standing-wave ratio in air is 1. 8. Determine ε.

Problem 3 A plane wave with parallel polarization is incident at an angle of π/4 from air on a perfect dielectric with εr = 4 and μ = μo. Find the Fresnel coefficients. What fraction of the incident power is reflected, and what is transmitted into the dielectric?

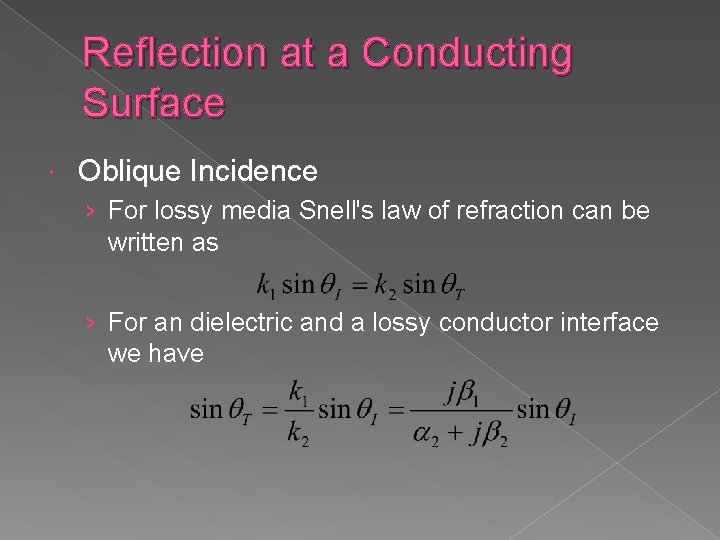
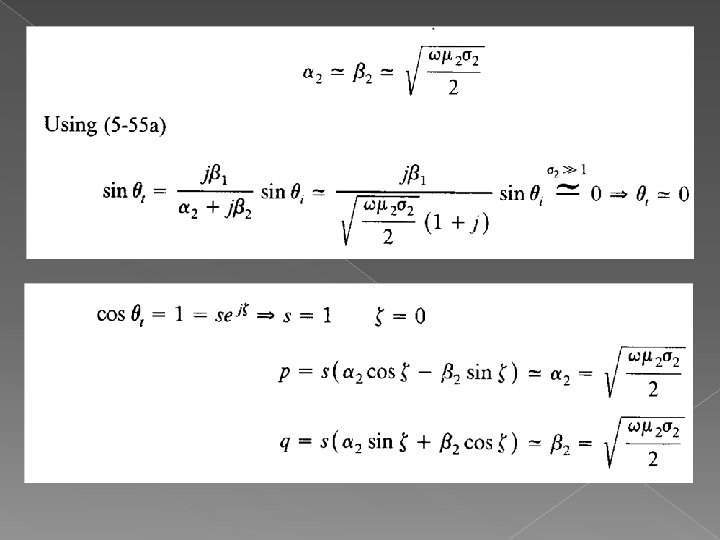
Reflection at a Conducting Surface Oblique Incidence › For lossy media Snell's law of refraction can be written as › For an dielectric and a lossy conductor interface we have

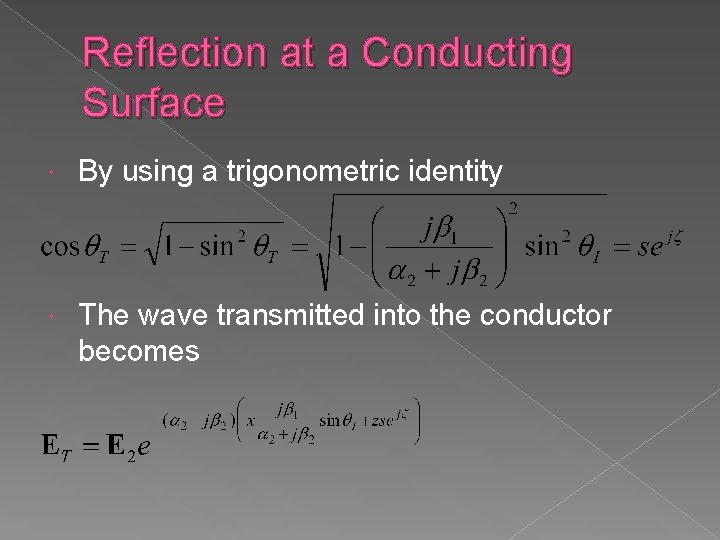
Reflection at a Conducting Surface By using a trigonometric identity The wave transmitted into the conductor becomes

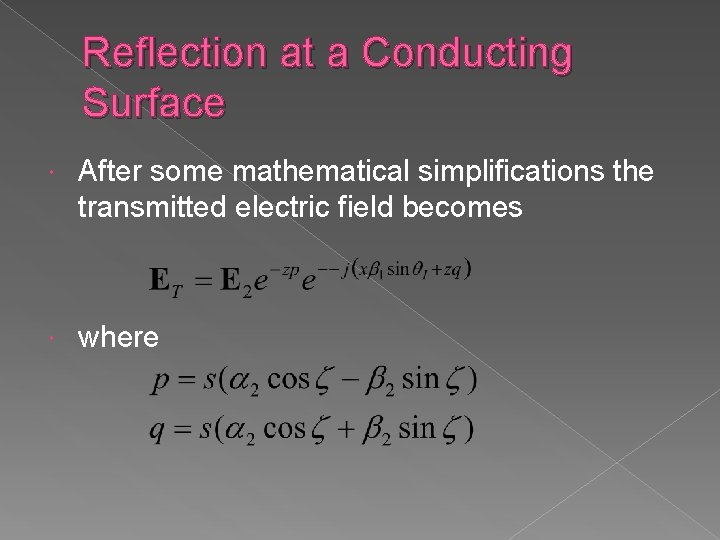
Reflection at a Conducting Surface After some mathematical simplifications the transmitted electric field becomes where

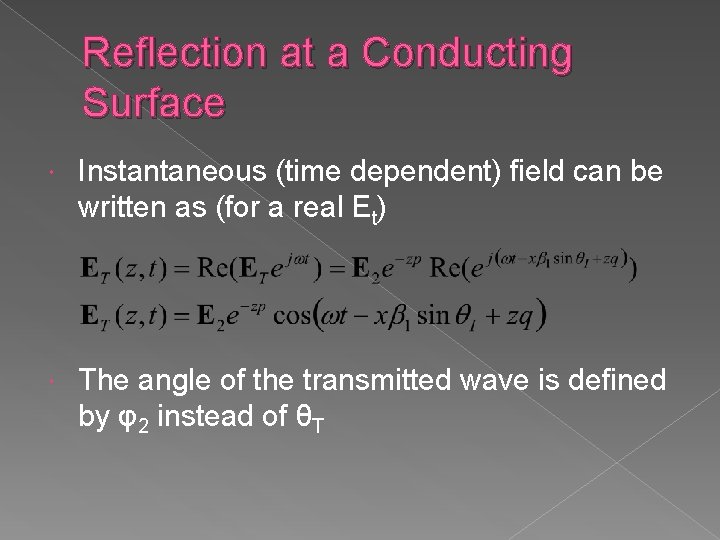
Reflection at a Conducting Surface Instantaneous (time dependent) field can be written as (for a real Et) The angle of the transmitted wave is defined by φ2 instead of θT

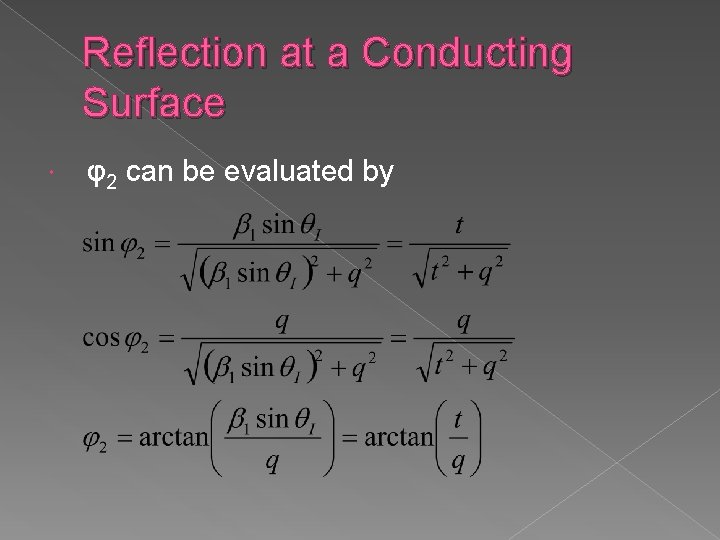
Reflection at a Conducting Surface φ2 can be evaluated by

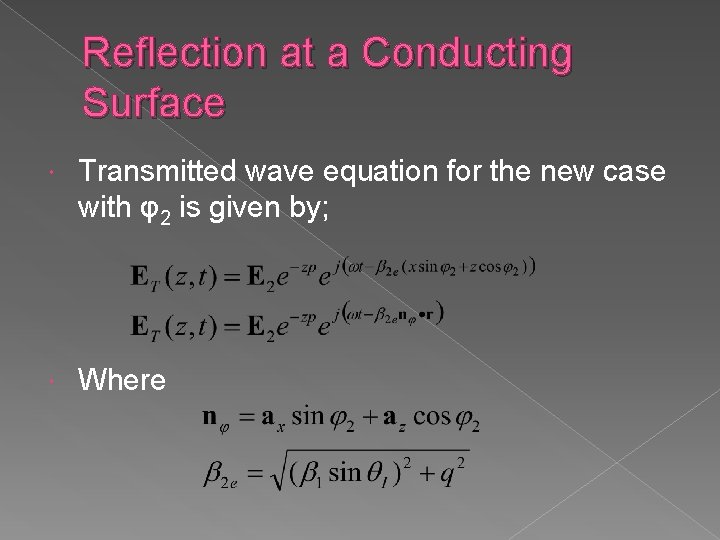
Reflection at a Conducting Surface Transmitted wave equation for the new case with φ2 is given by; Where

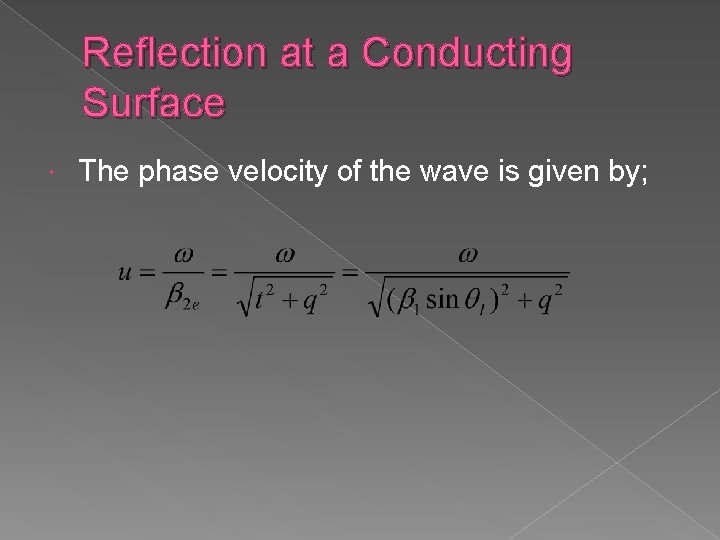
Reflection at a Conducting Surface The phase velocity of the wave is given by;

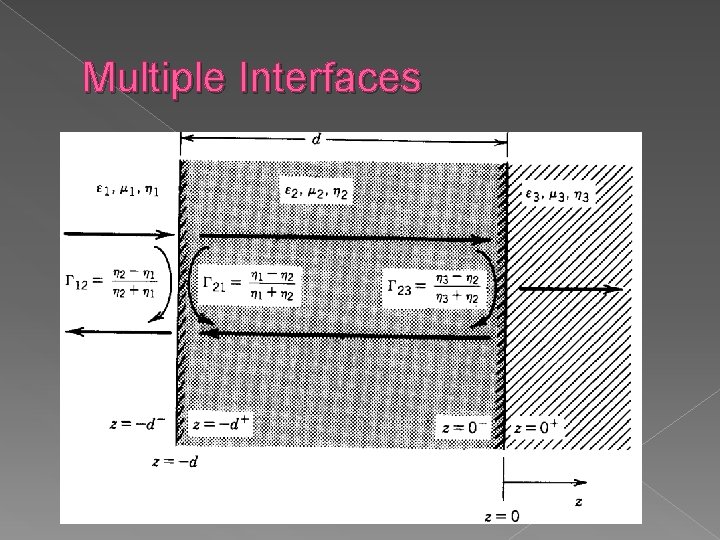
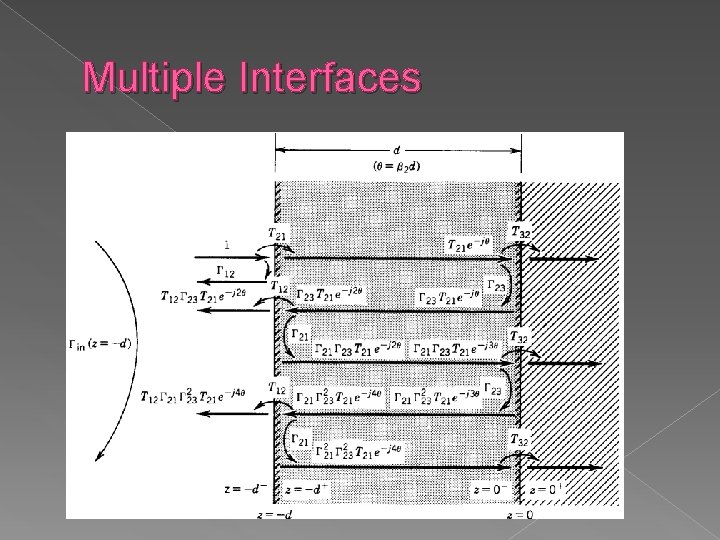
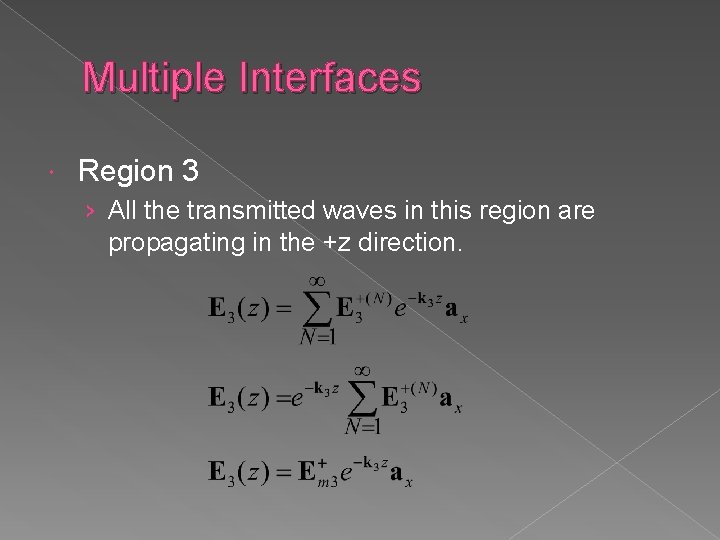
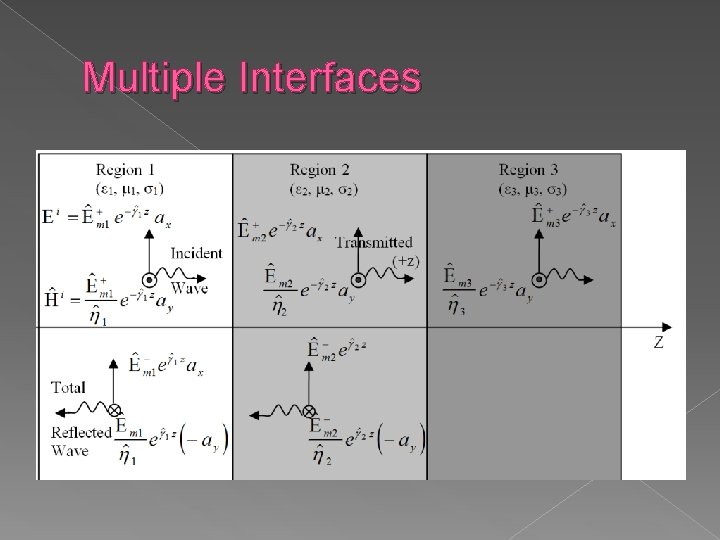
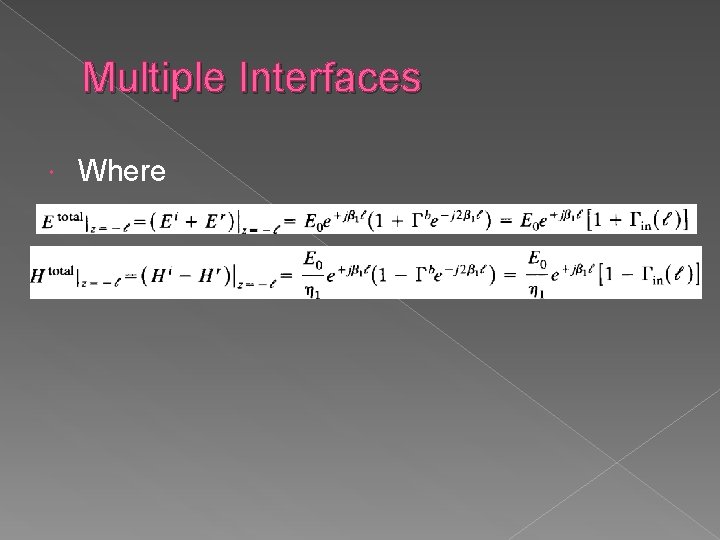
Multiple Interfaces Normal Incidence › A uniform plane wave propagating in the z direction is normally incident onto the interface between regions 1 and 2 a second interface exists between regions 2 and 3

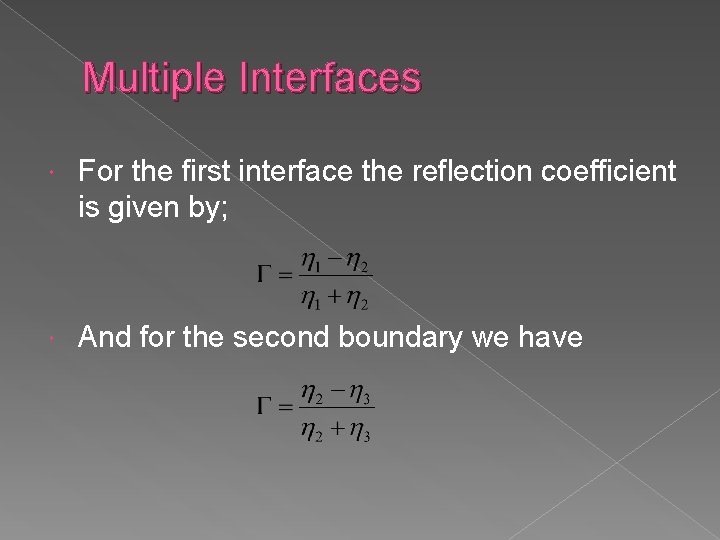
Multiple Interfaces For the first interface the reflection coefficient is given by; And for the second boundary we have

Multiple Interfaces

Multiple Interfaces

Multiple Interfaces By summing all the fields that resulted from the multiple reflection process the steadystate expressions can be obtained

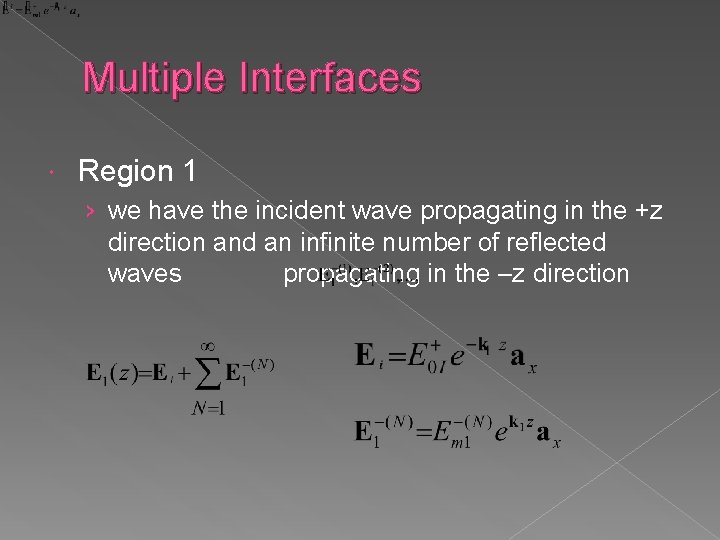
Multiple Interfaces Region 1 › we have the incident wave propagating in the +z direction and an infinite number of reflected waves propagating in the –z direction

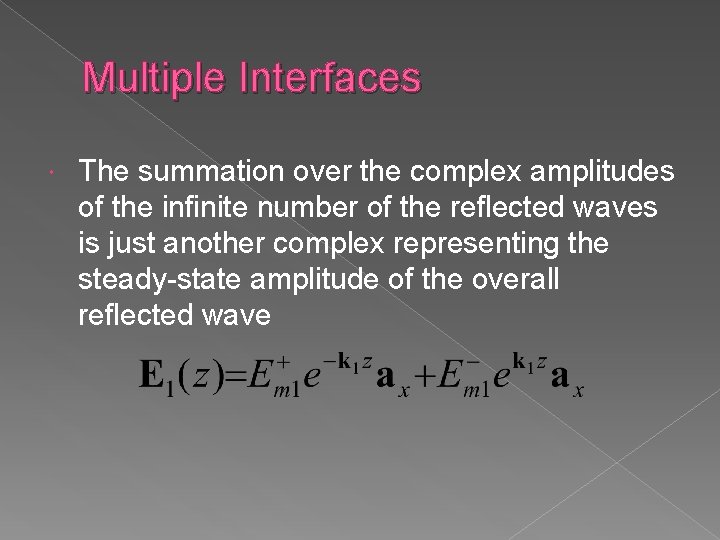
Multiple Interfaces The summation over the complex amplitudes of the infinite number of the reflected waves is just another complex representing the steady-state amplitude of the overall reflected wave

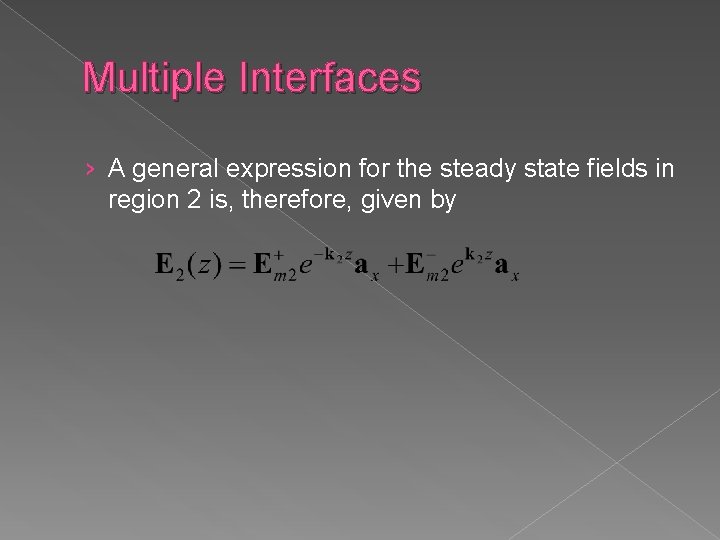
Multiple Interfaces Region 2 › In this region at steady state there will be an infinite number of waves propagating in the +z direction and an infinite number of other waves propagating in the –z direction.

Multiple Interfaces › A general expression for the steady state fields in region 2 is, therefore, given by

Multiple Interfaces Region 3 › All the transmitted waves in this region are propagating in the +z direction.

Multiple Interfaces

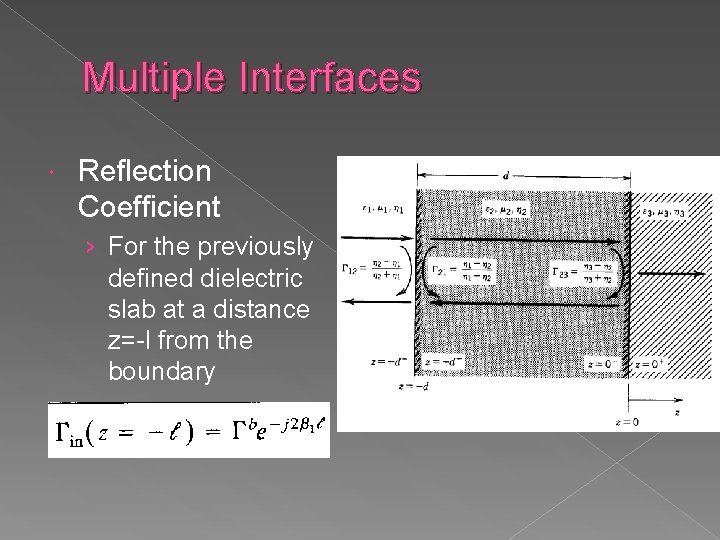
Multiple Interfaces Reflection Coefficient › For the previously defined dielectric slab at a distance z=-l from the boundary

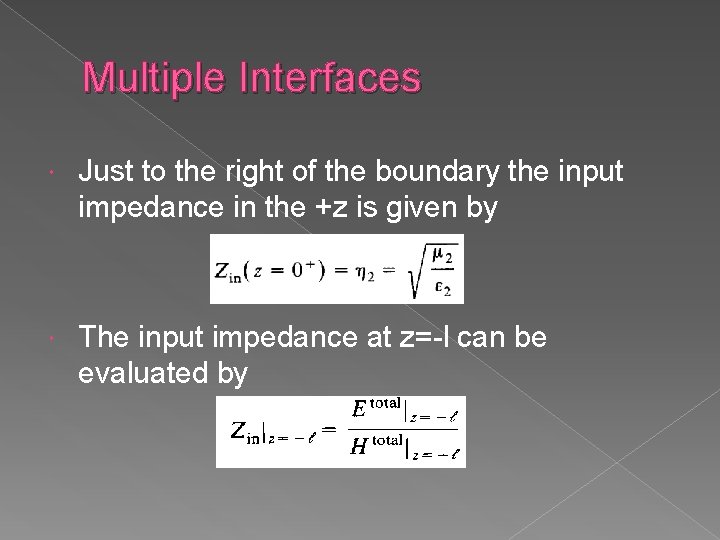
Multiple Interfaces Just to the right of the boundary the input impedance in the +z is given by The input impedance at z=-l can be evaluated by

Multiple Interfaces Where

Multiple Interfaces Therefore This is also known as the impedance transfer equation

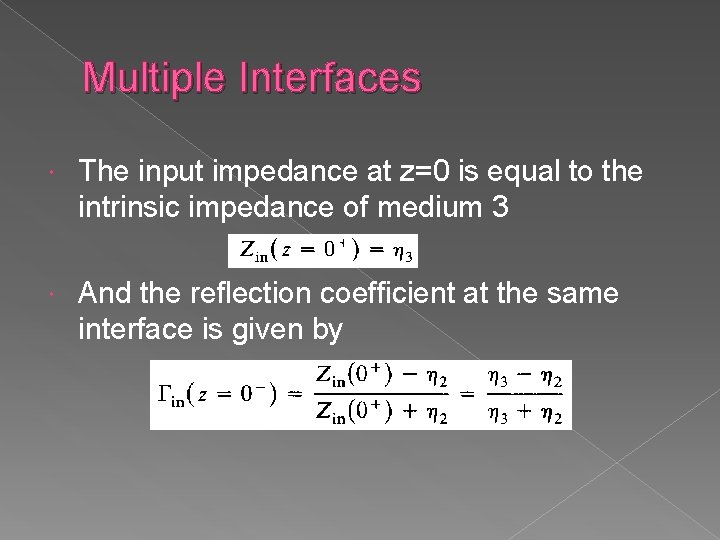
Multiple Interfaces The input impedance at z=0 is equal to the intrinsic impedance of medium 3 And the reflection coefficient at the same interface is given by

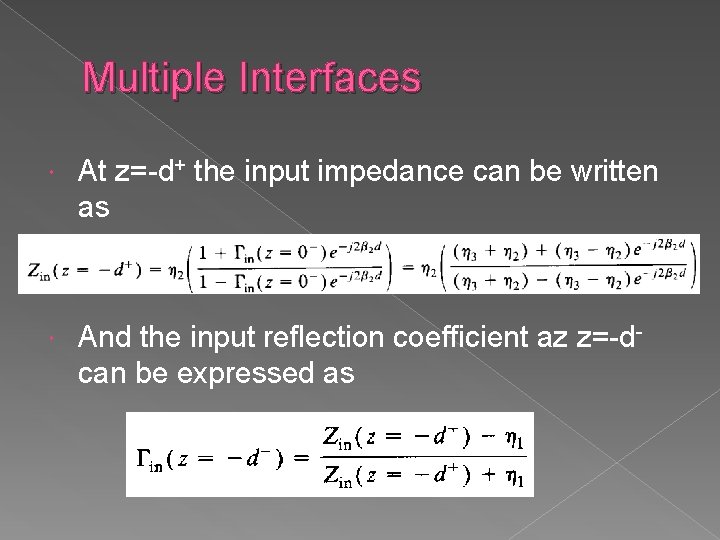
Multiple Interfaces At z=-d+ the input impedance can be written as And the input reflection coefficient az z=-dcan be expressed as

Multiple Interfaces

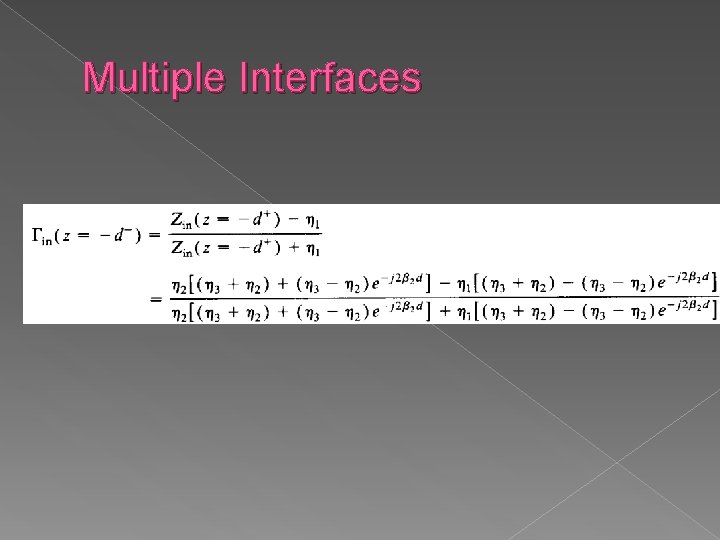
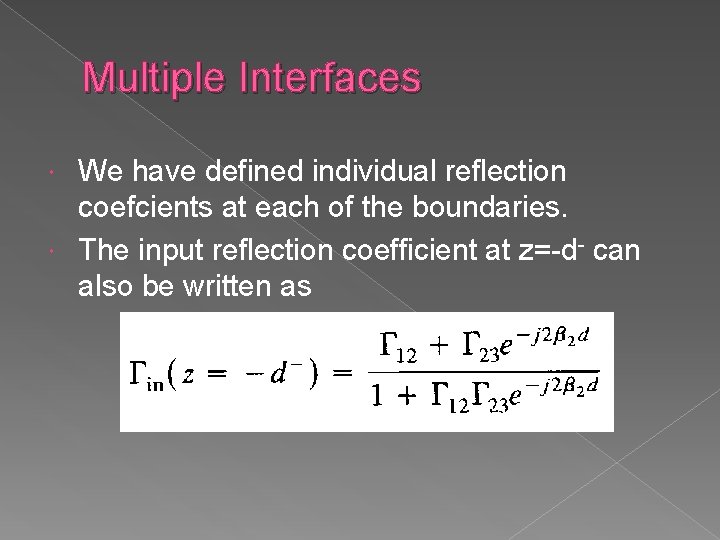
Multiple Interfaces We have defined individual reflection coefcients at each of the boundaries. The input reflection coefficient at z=-d- can also be written as

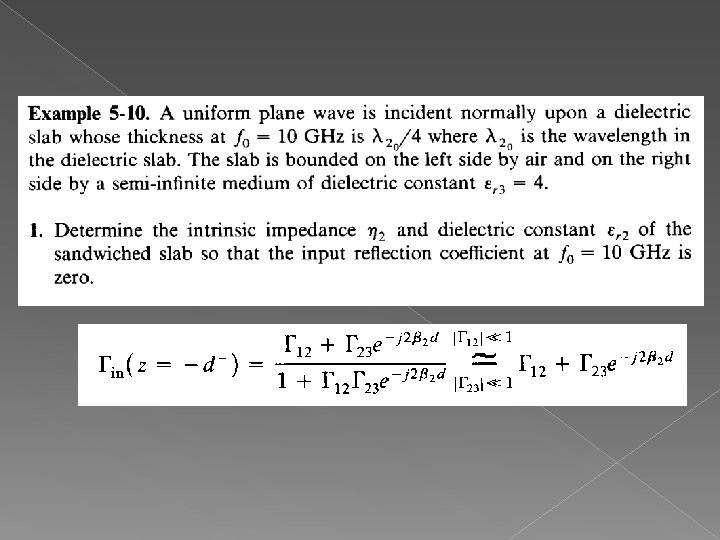
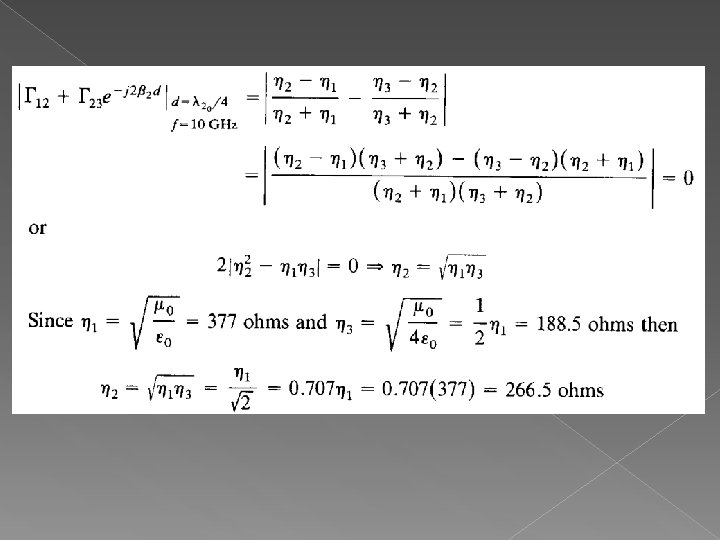
Multiple Interfaces Example: › A uniform plane wave at a frequency of 10 GHz is incident normally on a dielectric slab of thickness d and bounded on both sides of air. Assume that the relative dielectric constant of the slab is 2. 56. › Determine thickness of the slab so that the input reflection coefficient at 10 GHz is zero.

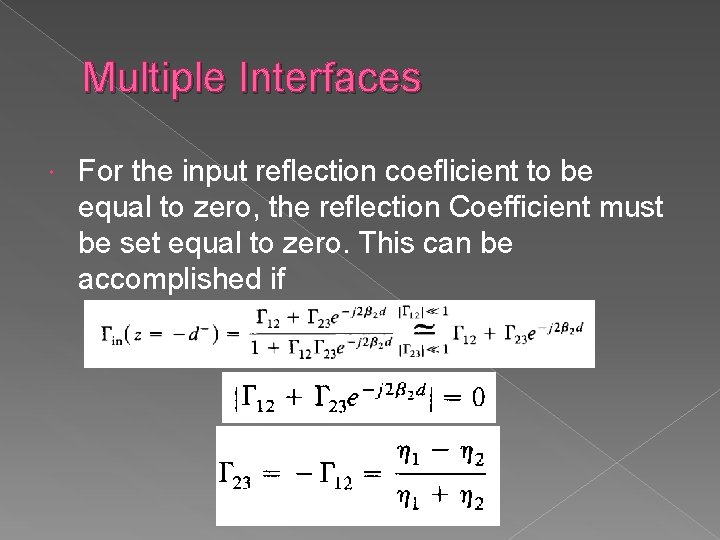
Multiple Interfaces For the input reflection coeflicient to be equal to zero, the reflection Coefficient must be set equal to zero. This can be accomplished if

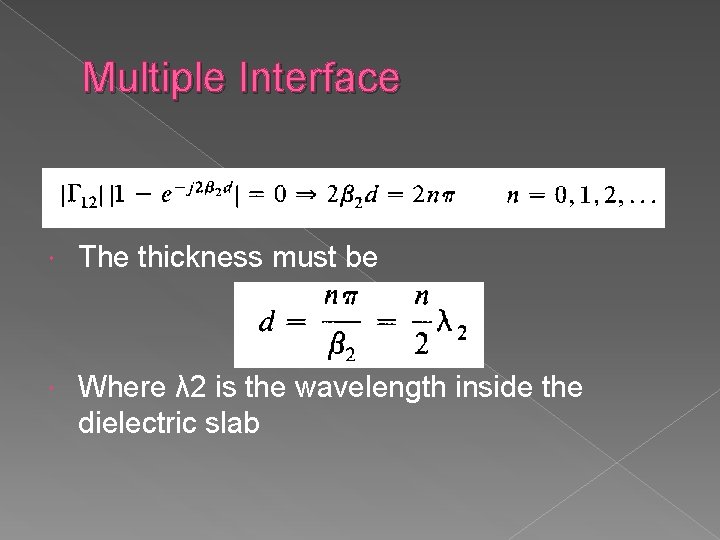
Multiple Interface The thickness must be Where λ 2 is the wavelength inside the dielectric slab

Multiple Interface The thickness of the slab must be an integral number of half wavelengths inside the dielectric; at 10 GHz the wavelength is

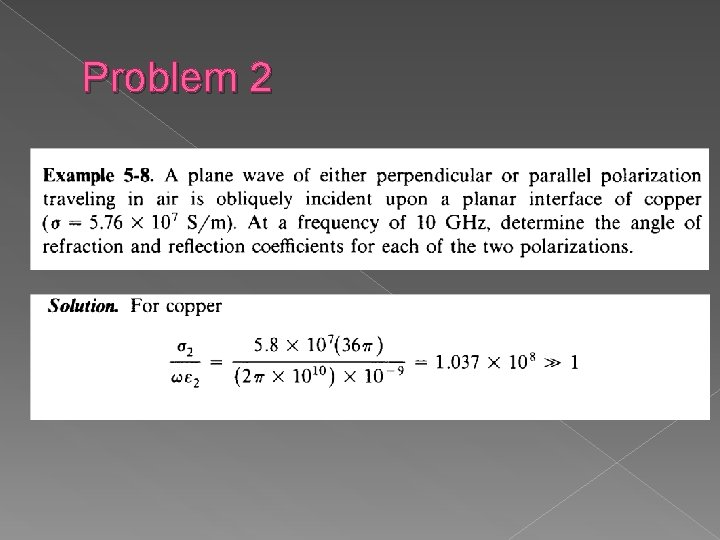
Problem 2