ASLevel Maths Core 1 for Edexcel C 1

AS-Level Maths: Core 1 for Edexcel C 1. 1 Algebra and functions 1 This icon indicates the slide contains activities created in Flash. These activities are not editable. For more detailed instructions, see the Getting Started presentation. 1 of 38 © Boardworks Ltd 2005

Using and manipulating surds Contents Rationalizing the denominator The index laws Zero and negative indices Fractional indices Solving equations involving indices Examination-style questions 2 of 38 © Boardworks Ltd 2005

Types of number We can classify numbers into the following sets: The set of natural numbers, : Positive whole numbers {0, 1, 2, 3, 4 …} The set of integers, : Positive and negative whole numbers {0, ± 1, ± 2, ± 3 …} The set of rational numbers, : Numbers that can be expressed in the form , where n and m are integers. All fractions and all terminating and recurring decimals are rational numbers; for example, ¾, – 0. 63, 0. 2. The set of real numbers, : All numbers including irrational numbers; that is, numbers that cannot be expressed in the form , where n and m are integers. For example, and. 3 of 38 © Boardworks Ltd 2005

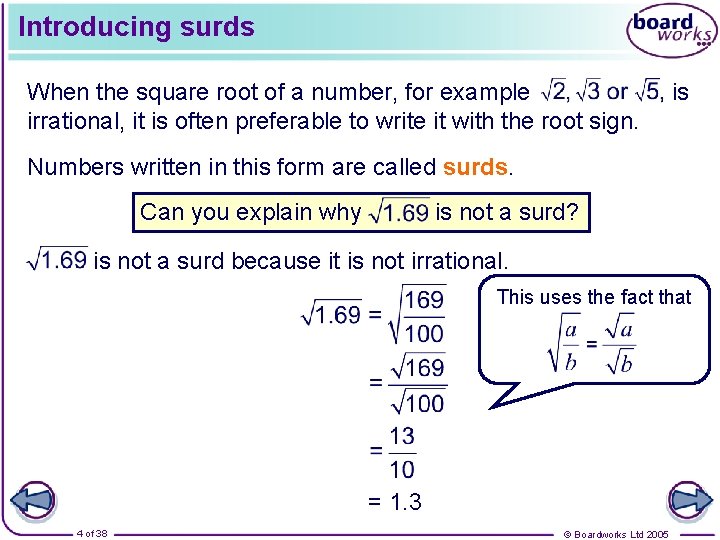
Introducing surds When the square root of a number, for example √ 2, √ 3 or √ 5, is irrational, it is often preferable to write it with the root sign. Numbers written in this form are called surds. Can you explain why √ 1. 69 is not a surd? √ 1. 69 is not a surd because it is not irrational. This uses the fact that = 1. 3 4 of 38 © Boardworks Ltd 2005

Manipulating surds When working with surds it is important to remember the following two rules: and Also: You should also remember that, by definition, √a means the positive square root of a. 5 of 38 © Boardworks Ltd 2005

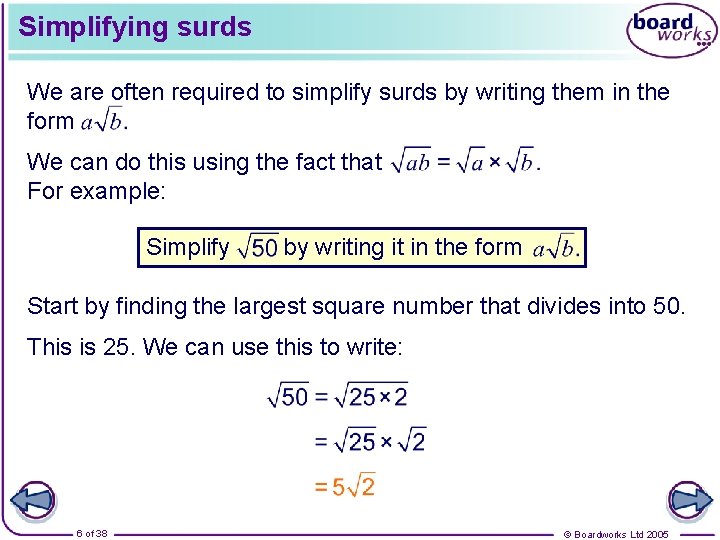
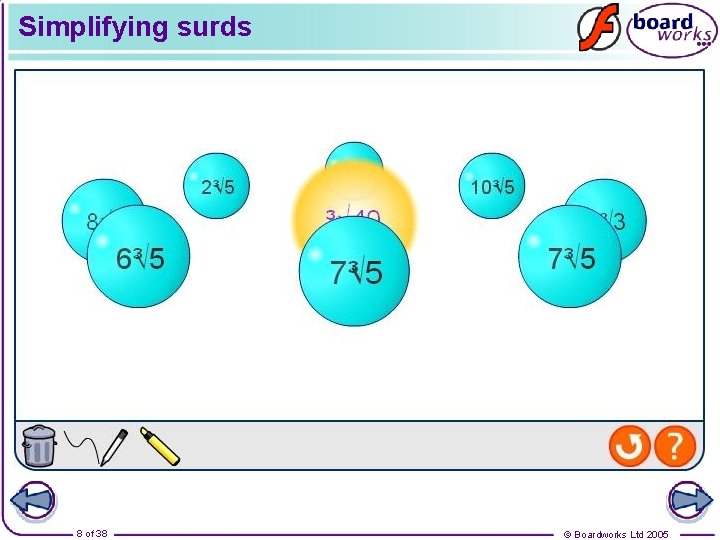
Simplifying surds We are often required to simplify surds by writing them in the form We can do this using the fact that For example: Simplify by writing it in the form Start by finding the largest square number that divides into 50. This is 25. We can use this to write: 6 of 38 © Boardworks Ltd 2005

Simplifying surds Simplify the following surds by writing them in the form a√b. 7 of 38 © Boardworks Ltd 2005

Simplifying surds 8 of 38 © Boardworks Ltd 2005

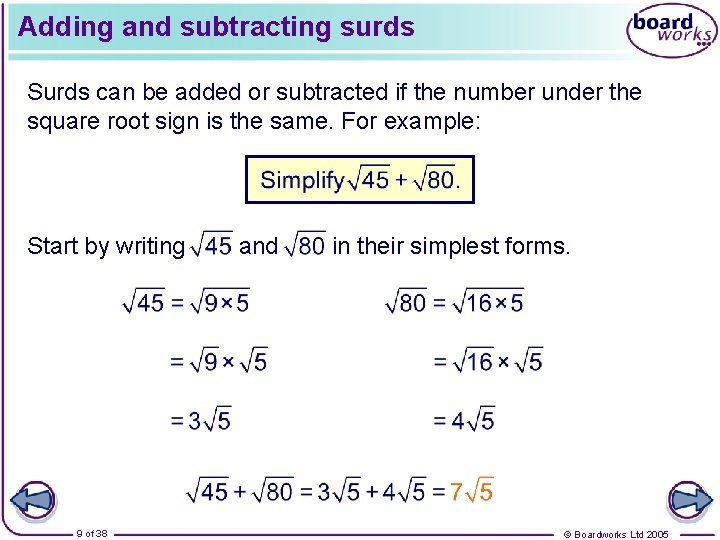
Adding and subtracting surds Surds can be added or subtracted if the number under the square root sign is the same. For example: Start by writing 9 of 38 and in their simplest forms. © Boardworks Ltd 2005

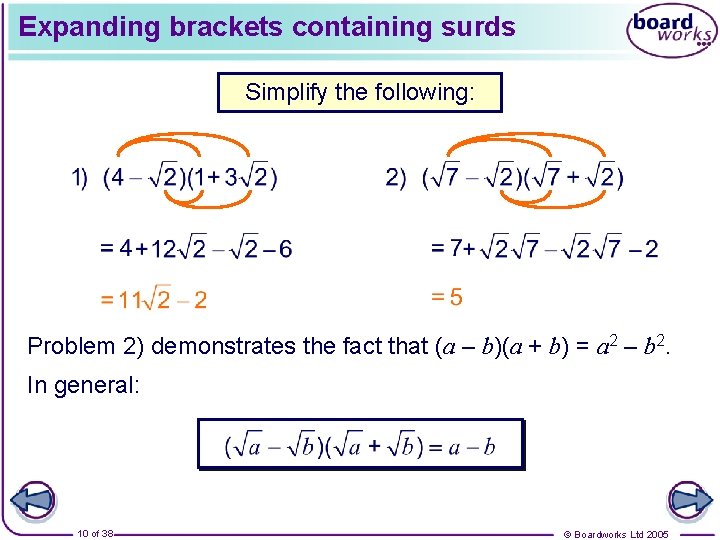
Expanding brackets containing surds Simplify the following: Problem 2) demonstrates the fact that (a – b)(a + b) = a 2 – b 2. In general: 10 of 38 © Boardworks Ltd 2005

Rationalizing the denominator Contents Using and manipulating surds Rationalizing the denominator The index laws Zero and negative indices Fractional indices Solving equations involving indices Examination-style questions 11 of 38 © Boardworks Ltd 2005

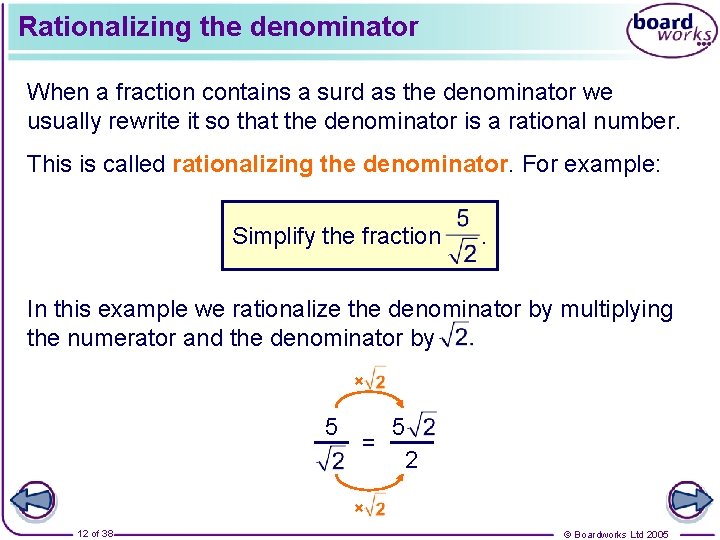
Rationalizing the denominator When a fraction contains a surd as the denominator we usually rewrite it so that the denominator is a rational number. This is called rationalizing the denominator. For example: Simplify the fraction . In this example we rationalize the denominator by multiplying the numerator and the denominator by × 5 5 = 2 × 12 of 38 © Boardworks Ltd 2005

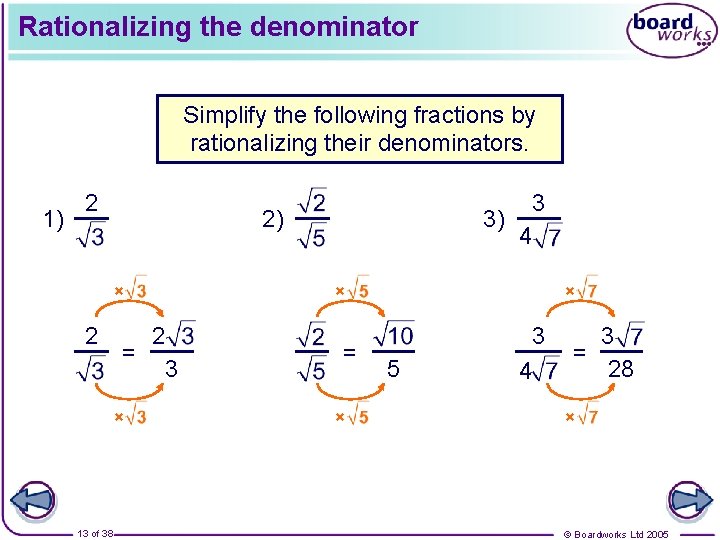
Rationalizing the denominator Simplify the following fractions by rationalizing their denominators. 1) 2 2) × 2 2 = 3 × 13 of 38 3 3) 4 × = × × 5 3 4 3 = 28 × © Boardworks Ltd 2005

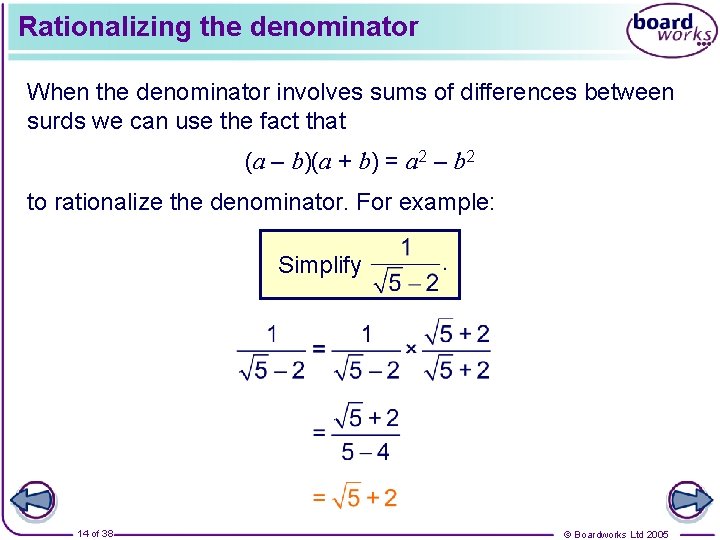
Rationalizing the denominator When the denominator involves sums of differences between surds we can use the fact that (a – b)(a + b) = a 2 – b 2 to rationalize the denominator. For example: Simplify 14 of 38 © Boardworks Ltd 2005

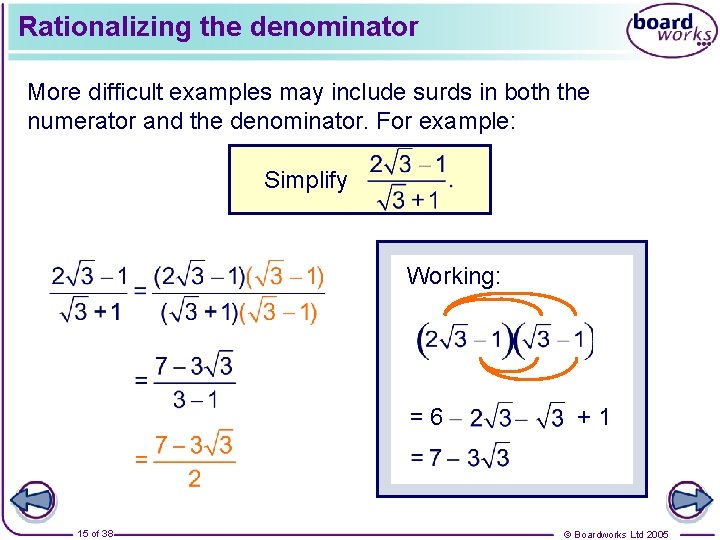
Rationalizing the denominator More difficult examples may include surds in both the numerator and the denominator. For example: Simplify Working: =6 15 of 38 +1 © Boardworks Ltd 2005

The index laws Contents Using and manipulating surds Rationalizing the denominator The index laws Zero and negative indices Fractional indices Solving equations involving indices Examination-style questions 16 of 38 © Boardworks Ltd 2005

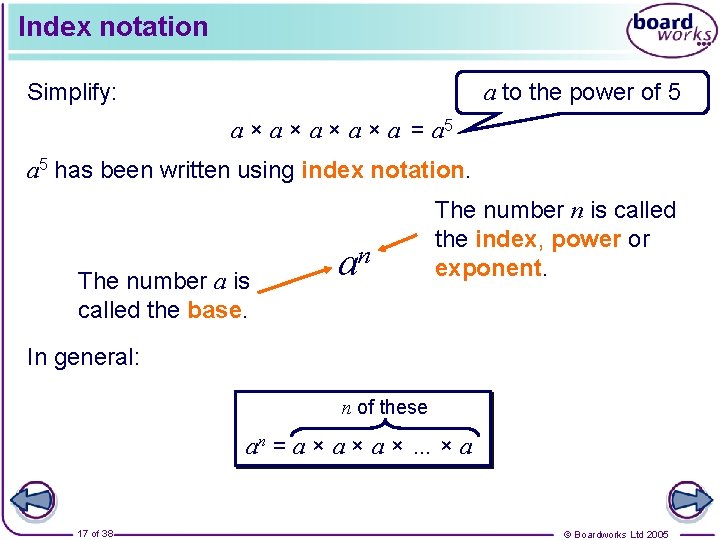
Index notation Simplify: a to the power of 5 a × a × a = a 5 has been written using index notation. The number a is called the base. an The number n is called the index, power or exponent. In general: n of these an = a × a × … × a 17 of 38 © Boardworks Ltd 2005

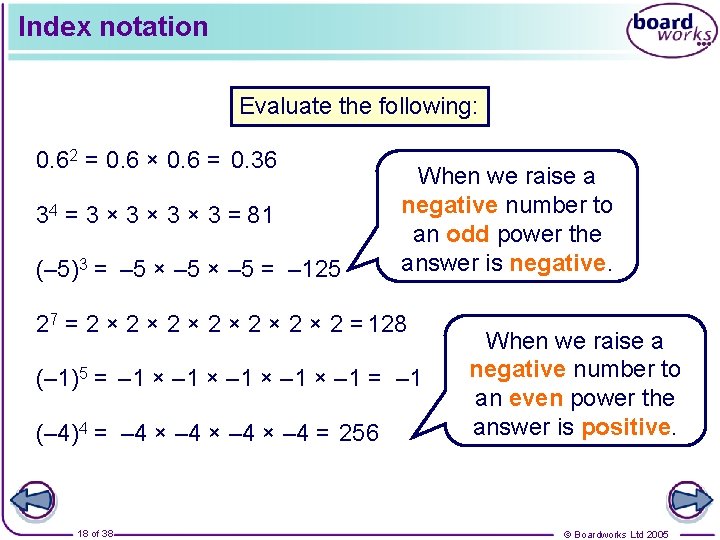
Index notation Evaluate the following: 0. 62 = 0. 6 × 0. 6 = 0. 36 34 = 3 × 3 × 3 = 81 (– 5)3 = – 5 × – 5 = – 125 When we raise a negative number to an odd power the answer is negative. 27 = 2 × 2 × 2 × 2 = 128 (– 1)5 = – 1 × – 1 = – 1 (– 4)4 = – 4 × – 4 = 256 18 of 38 When we raise a negative number to an even power the answer is positive. © Boardworks Ltd 2005

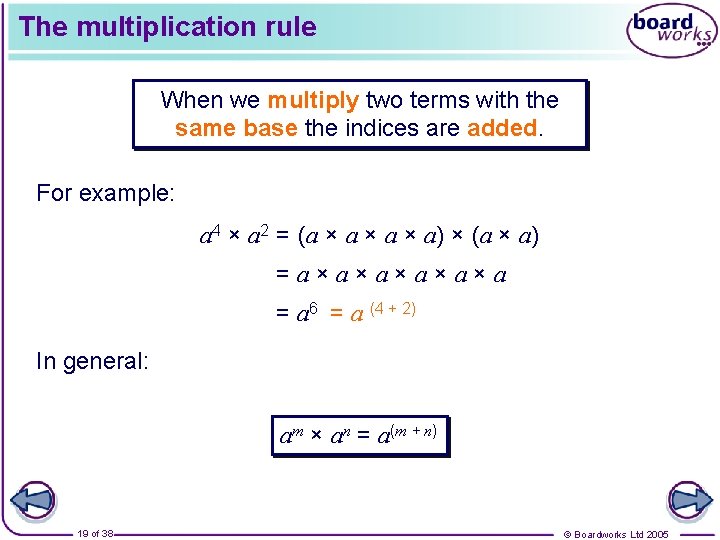
The multiplication rule When we multiply two terms with the same base the indices are added. For example: a 4 × a 2 = (a × a × a) × (a × a) =a×a×a×a = a 6 = a (4 + 2) In general: am × an = a(m + n) 19 of 38 © Boardworks Ltd 2005

The division rule When we divide two terms with the same base the indices are subtracted. For example: a 5 ÷ a 2 a×a×a = a 3 = a (5 – 2) 2 4 p 6 ÷ 2 p 4 4×p×p×p = = 2 p 2 = 2 p(6 – 4) 2×p×p In general: am ÷ an = a(m – n) 20 of 38 © Boardworks Ltd 2005

The power rule When a term is raised to a power and the result raised to another power, the powers are multiplied. For example: (y 3)2 = y 3 × y 3 (pq 2)4 = pq 2 × pq 2 = (y × y) × (y × y) = p 4 × q (2 + 2 + 2) = y 6 = y 3× 2 = p 4 × q 8 = p 4 q 8 = p 1× 4 q 2× 4 In general: (am)n = amn 21 of 38 © Boardworks Ltd 2005

Using index laws 22 of 38 © Boardworks Ltd 2005

Zero and negative indices Contents Using and manipulating surds Rationalizing the denominator The index laws Zero and negative indices Fractional indices Solving equations involving indices Examination-style questions 23 of 38 © Boardworks Ltd 2005

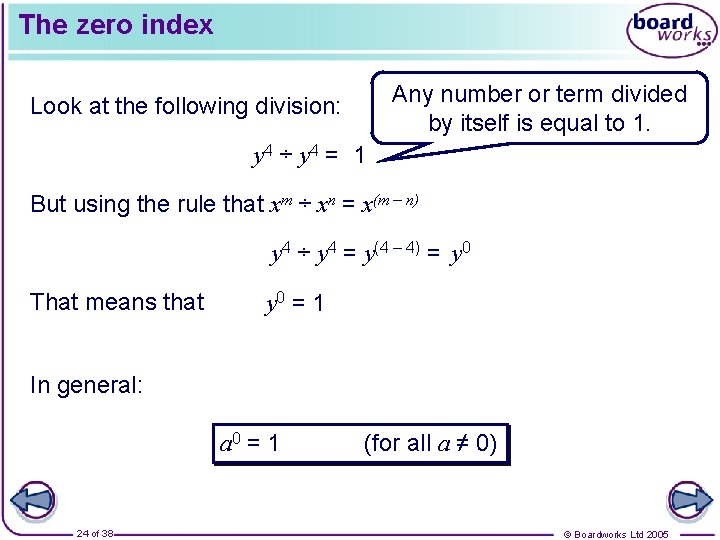
The zero index Any number or term divided by itself is equal to 1. Look at the following division: y 4 ÷ y 4 = 1 But using the rule that xm ÷ xn = x(m – n) y 4 ÷ y 4 = y(4 – 4) = y 0 That means that y 0 = 1 In general: a 0 = 1 24 of 38 (for all a ≠ 0) © Boardworks Ltd 2005

Negative indices Look at the following division: b 2 ÷ b 4 = b×b×b×b 1 1 = = 2 b×b b But using the rule that am ÷ an = a(m – n) b 2 ÷ b 4 = b(2 – 4) = b– 2 That means that b– 2 = 1 b 2 In general: a–n = 25 of 38 1 an © Boardworks Ltd 2005

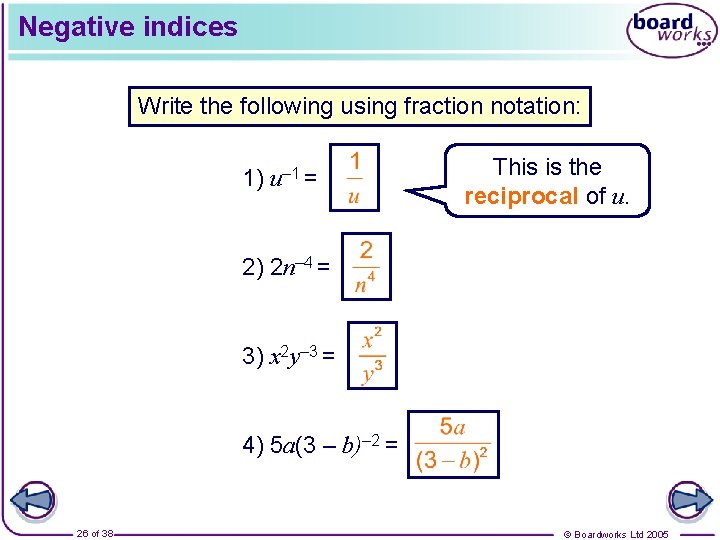
Negative indices Write the following using fraction notation: 1) u– 1 = This is the reciprocal of u. 2) 2 n– 4 = 3) x 2 y– 3 = 4) 5 a(3 – b)– 2 = 26 of 38 © Boardworks Ltd 2005

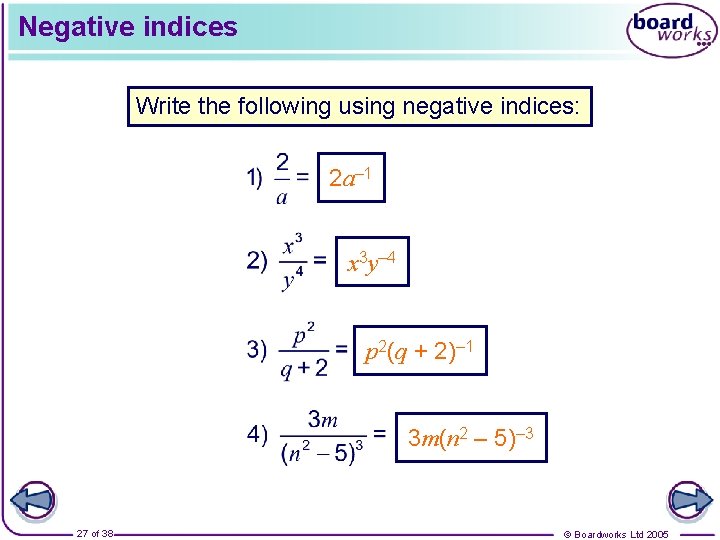
Negative indices Write the following using negative indices: 2 a– 1 x 3 y– 4 p 2(q + 2)– 1 3 m(n 2 – 5)– 3 27 of 38 © Boardworks Ltd 2005

Fractional indices Contents Using and manipulating surds Rationalizing the denominator The index laws Zero and negative indices Fractional indices Solving equations involving indices Examination-style questions 28 of 38 © Boardworks Ltd 2005

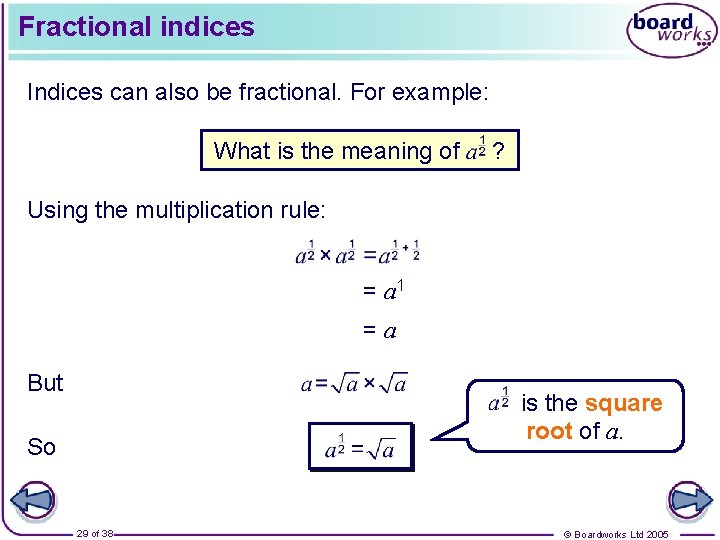
Fractional indices Indices can also be fractional. For example: What is the meaning of ? Using the multiplication rule: = a 1 =a But is the square root of a. So 29 of 38 © Boardworks Ltd 2005

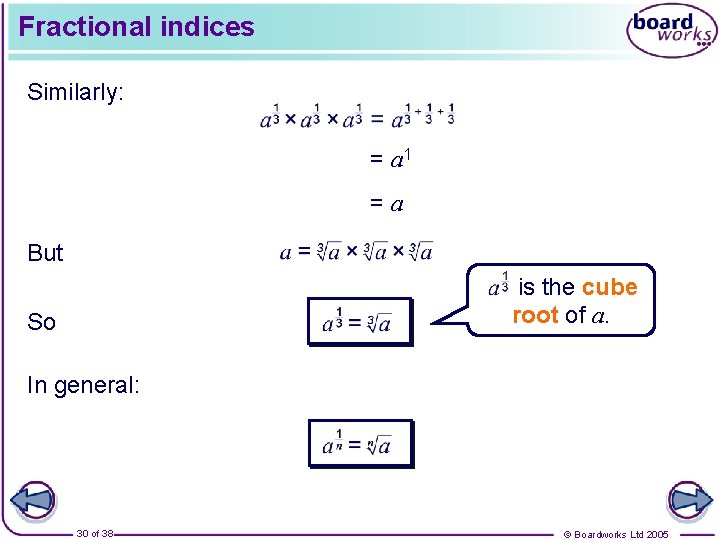
Fractional indices Similarly: = a 1 =a But is the cube root of a. So In general: 30 of 38 © Boardworks Ltd 2005

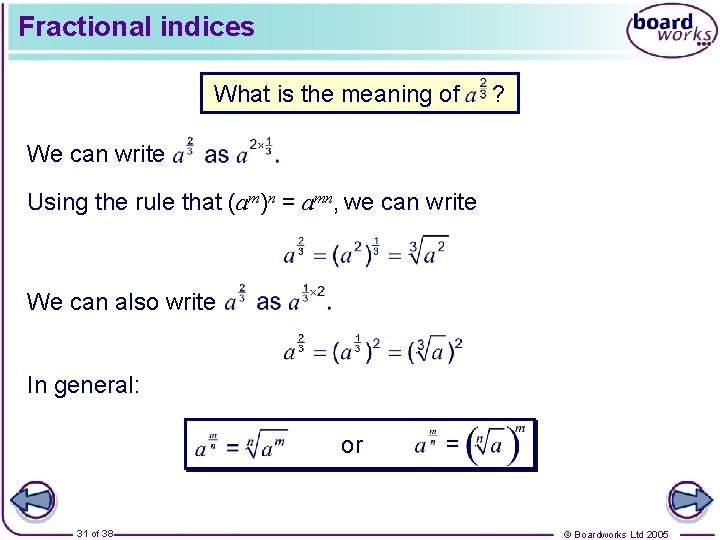
Fractional indices What is the meaning of ? We can write Using the rule that (am)n = amn, we can write We can also write In general: or 31 of 38 © Boardworks Ltd 2005

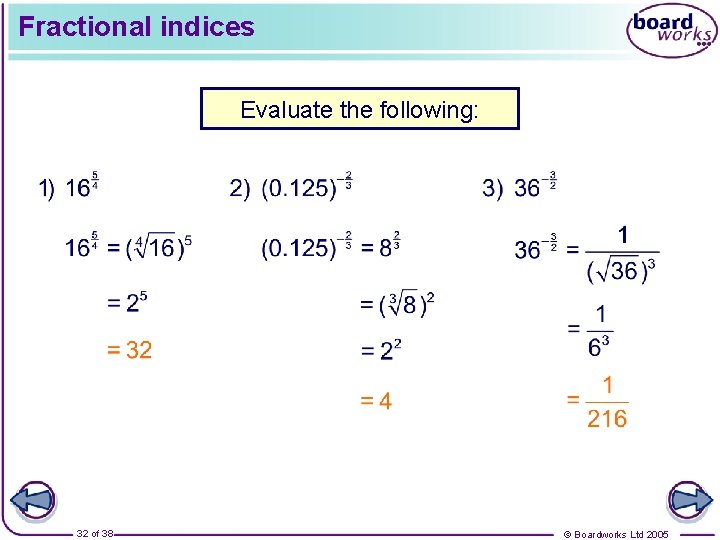
Fractional indices Evaluate the following: 32 of 38 © Boardworks Ltd 2005

Summary of the index laws Here is a summary of the index laws for all rational exponents: 33 of 38 © Boardworks Ltd 2005

Solving equations involving indices Contents Using and manipulating surds Rationalizing the denominator The index laws Zero and negative indices Fractional indices Solving equations involving indices Examination-style questions 34 of 38 © Boardworks Ltd 2005

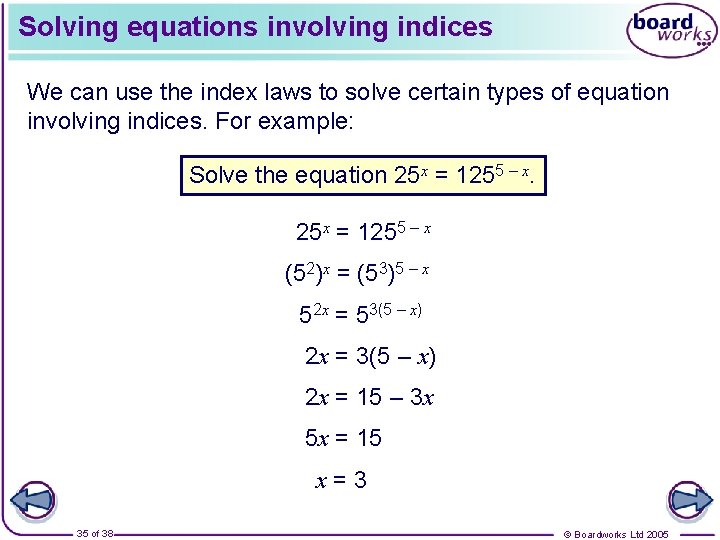
Solving equations involving indices We can use the index laws to solve certain types of equation involving indices. For example: Solve the equation 25 x = 1255 – x (52)x = (53)5 – x 52 x = 53(5 – x) 2 x = 15 – 3 x 5 x = 15 x=3 35 of 38 © Boardworks Ltd 2005

Examination-style questions Contents Using and manipulating surds Rationalizing the denominator The index laws Zero and negative indices Fractional indices Solving equations involving indices Examination-style questions 36 of 38 © Boardworks Ltd 2005

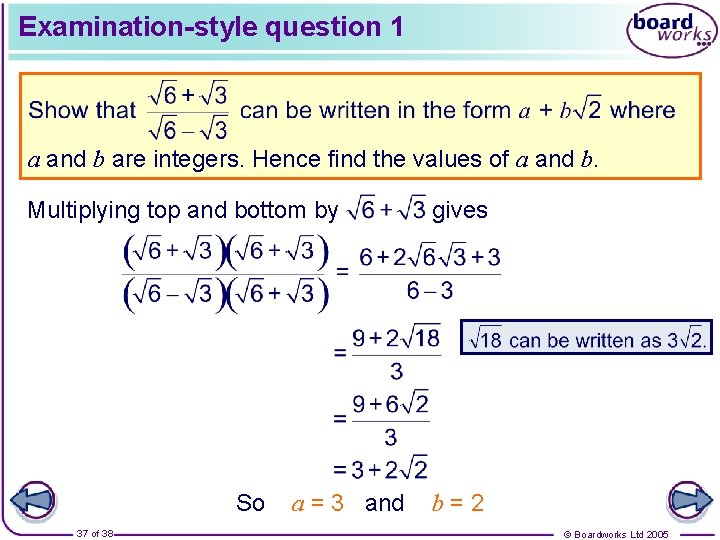
Examination-style question 1 a and b are integers. Hence find the values of a and b. Multiplying top and bottom by So 37 of 38 a = 3 and gives b=2 © Boardworks Ltd 2005

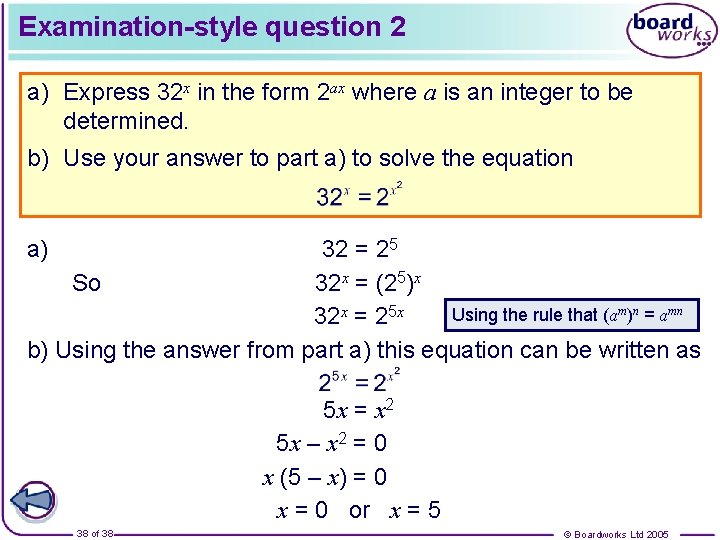
Examination-style question 2 a) Express 32 x in the form 2 ax where a is an integer to be determined. b) Use your answer to part a) to solve the equation a) 32 = 25 So 32 x = (25)x Using the rule that (am)n = amn 32 x = 25 x b) Using the answer from part a) this equation can be written as 5 x = x 2 5 x – x 2 = 0 x (5 – x) = 0 x = 0 or x = 5 38 of 38 © Boardworks Ltd 2005