Integral Calculus MEANING In calculus the reverse or

Integral Calculus MEANING. In calculus, the reverse or inverse process of “Differentiation” is called “Indefinite Integration” or “Integral Calculus” or “Anti differentiation”. The process of Integration is finding the function whose Derivative or Differential is given

Differentiation: If Total Utility Function is q 2, then the Marginal utility function is 2 q. Integration (Indefinite Integration): If the Marginal Utility Function is 2 q, then the Total Utility Function is q 2. That is, if derivative is given, our problem is to find out the function.

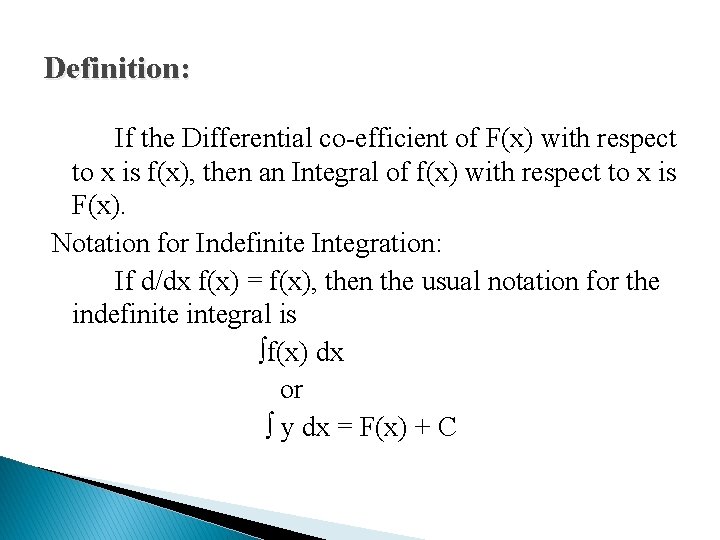
Definition: If the Differential co-efficient of F(x) with respect to x is f(x), then an Integral of f(x) with respect to x is F(x). Notation for Indefinite Integration: If d/dx f(x) = f(x), then the usual notation for the indefinite integral is ∫f(x) dx or ∫ y dx = F(x) + C

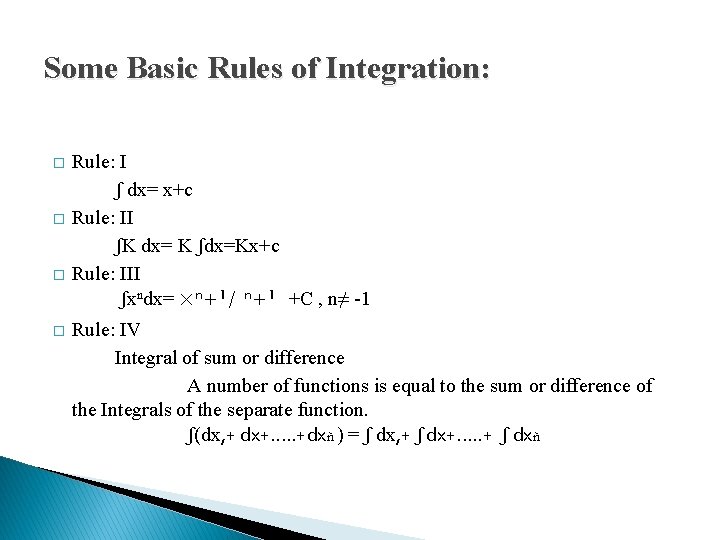
Some Basic Rules of Integration: � � Rule: I ʃ dx= x+c Rule: II ʃK dx= K ʃdx=Kx+c Rule: III ʃxⁿdx= ×ⁿ+¹/ ⁿ+¹ +C , n≠ -1 Rule: IV Integral of sum or difference A number of functions is equal to the sum or difference of the Integrals of the separate function. ʃ(dx, + dx+. . . +dxñ ) = ʃ dx, + ʃ dx+. . . + ʃ dxñ

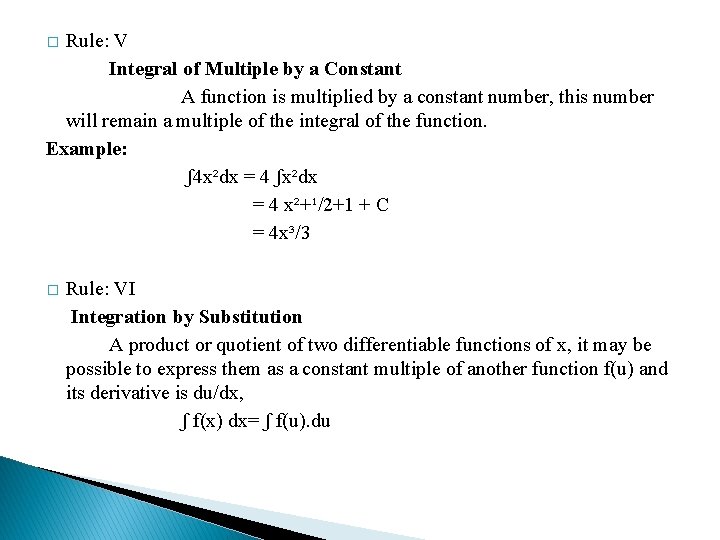
Rule: V Integral of Multiple by a Constant A function is multiplied by a constant number, this number will remain a multiple of the integral of the function. Example: ʃ4 x²dx = 4 ʃx²dx = 4 x²+¹/2+1 + C = 4 x³/3 � � Rule: VI Integration by Substitution A product or quotient of two differentiable functions of x, it may be possible to express them as a constant multiple of another function f(u) and its derivative is du/dx, ʃ f(x) dx= ʃ f(u). du

Rule: VII Logarithmic Function Rule: y= log X ʃ 1/x dx= logx + C. where C is constant. � Rule: VIII Exponential Function Rule y= ₑx y= ʃ ₑx dx= ₑx +c. �

Definite Integration In definite integration a definite numerical solution to the problem is possible. In definite integration a limit is added in the process of integration. There should be the lower and upper limits. This is represented as. This integral has upper limit as b and lower limit as a. If f(x) is a continuous function on closed interval (a, b) and F(x) is an integral function of f(x) then -is called definite integration

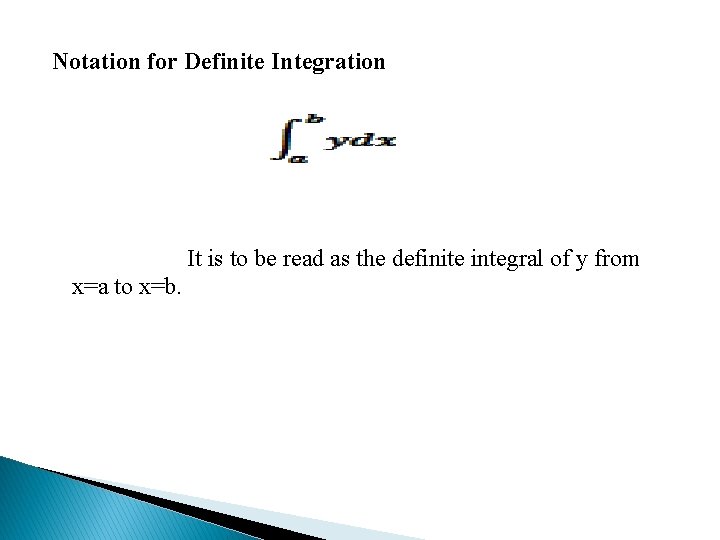
Notation for Definite Integration It is to be read as the definite integral of y from x=a to x=b.

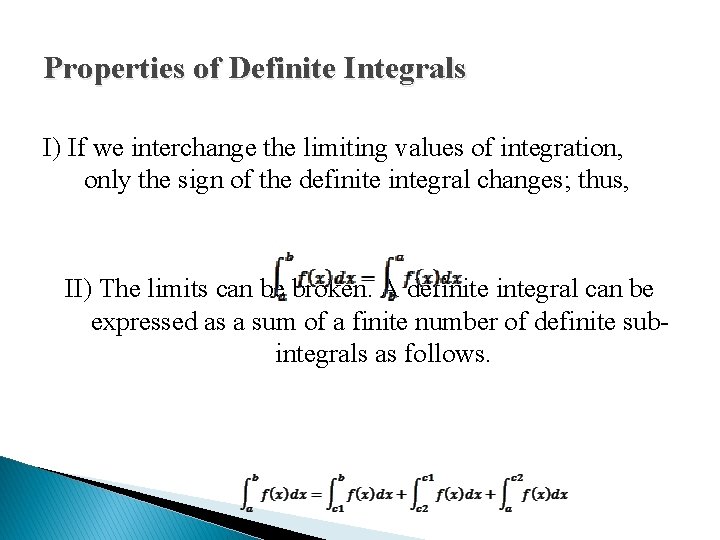
Properties of Definite Integrals I) If we interchange the limiting values of integration, only the sign of the definite integral changes; thus, II) The limits can be broken. A definite integral can be expressed as a sum of a finite number of definite subintegrals as follows.

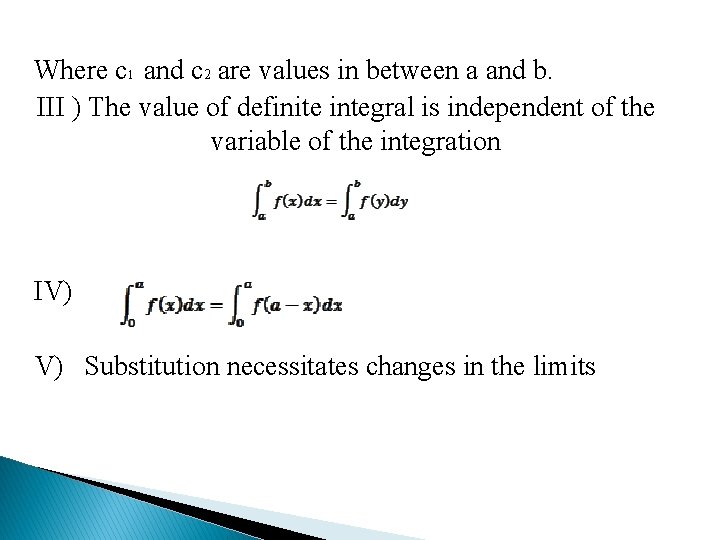
Where c 1 and c 2 are values in between a and b. III ) The value of definite integral is independent of the variable of the integration IV) V) Substitution necessitates changes in the limits

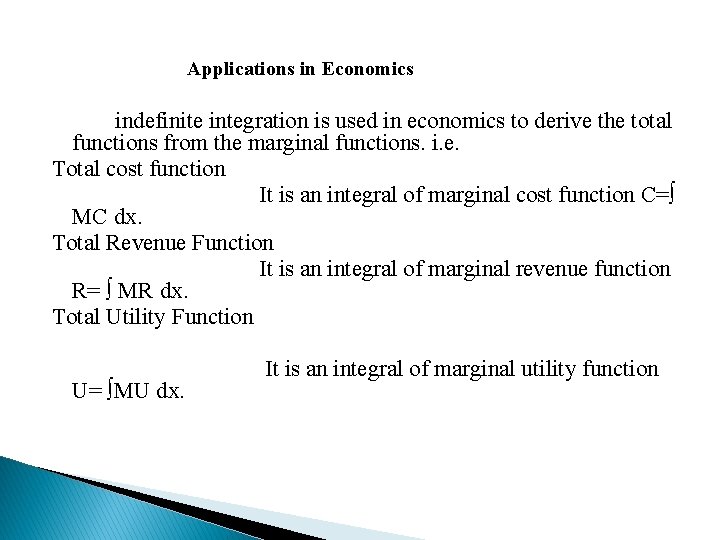
Applications in Economics indefinite integration is used in economics to derive the total functions from the marginal functions. i. e. Total cost function It is an integral of marginal cost function C=∫ MC dx. Total Revenue Function It is an integral of marginal revenue function R= ∫ MR dx. Total Utility Function U= ∫MU dx. It is an integral of marginal utility function

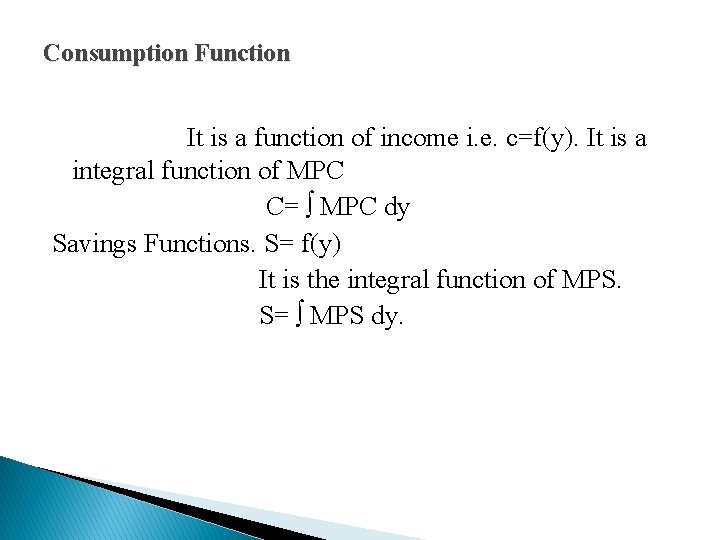
Consumption Function It is a function of income i. e. c=f(y). It is a integral function of MPC C= ∫ MPC dy Savings Functions. S= f(y) It is the integral function of MPS. S= ∫ MPS dy.

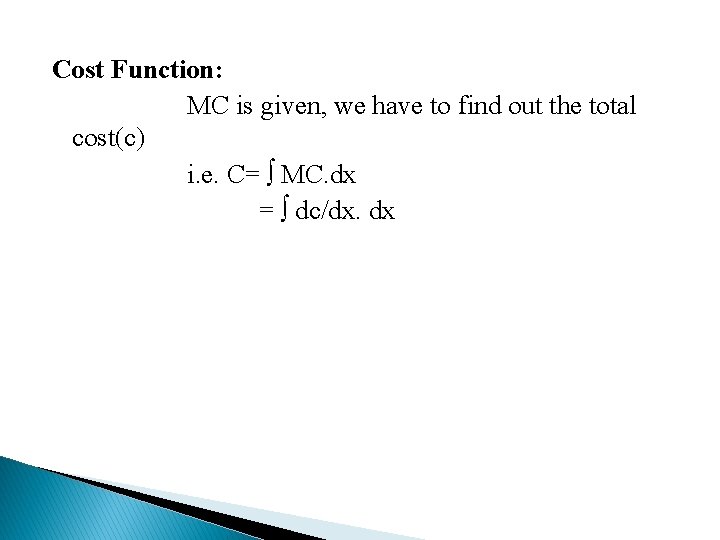
Cost Function: MC is given, we have to find out the total cost(c) i. e. C= ∫ MC. dx = ∫ dc/dx. dx

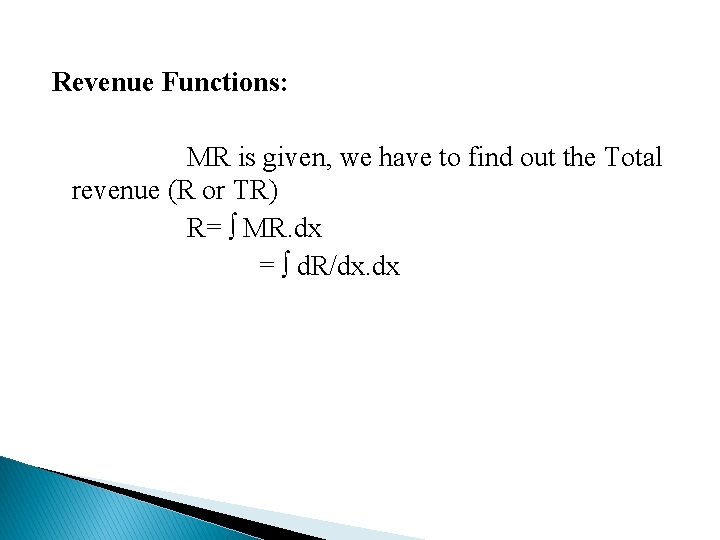
Revenue Functions: MR is given, we have to find out the Total revenue (R or TR) R= ∫ MR. dx = ∫ d. R/dx. dx

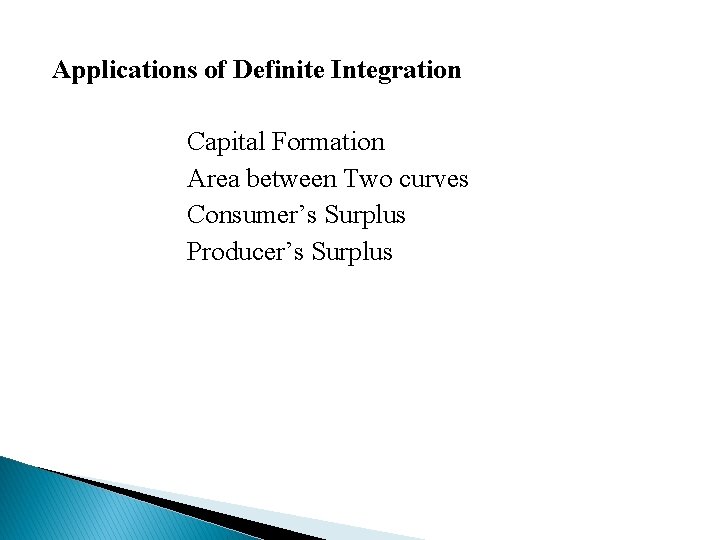
Applications of Definite Integration Capital Formation Area between Two curves Consumer’s Surplus Producer’s Surplus

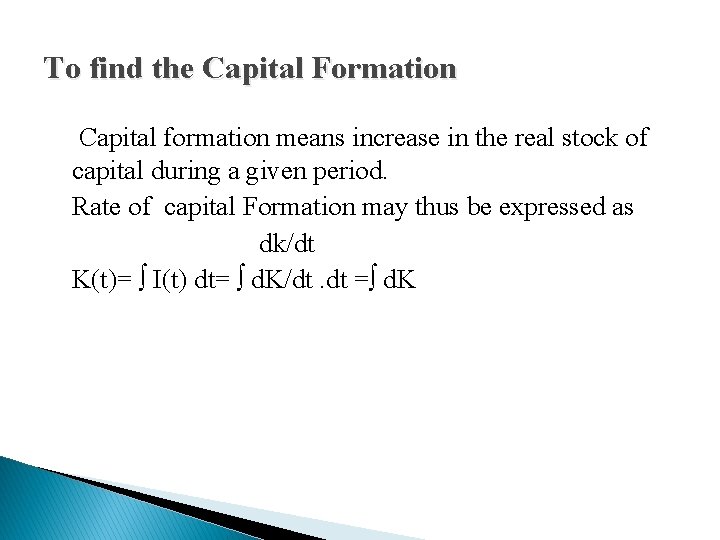
To find the Capital Formation Capital formation means increase in the real stock of capital during a given period. Rate of capital Formation may thus be expressed as dk/dt K(t)= ∫ I(t) dt= ∫ d. K/dt. dt =∫ d. K

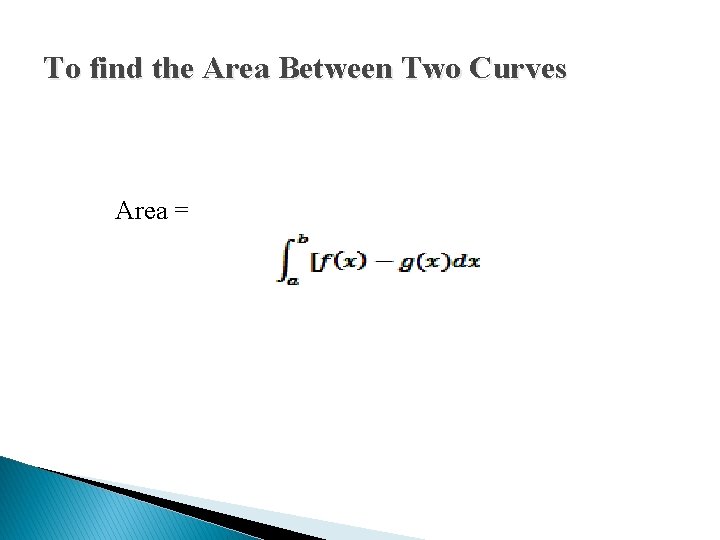
To find the Area Between Two Curves Area =

To find the Consumer’s Surplus is the difference between the price that a consumer is willing to pay for a commodity rather than go without it and the actual price he pays for the commodity Consumer’s surplus= Potential price- Actual price

Consumer’s Surplus=

1. 2. 3. Given the demand function p= 35 -2 x-x², find consumer’s surplus at x=3. If the demand function at p= 85 -4 x-x², find consumer’s surplus at po= 64 The demand function for a commodity p=20 -3 x. The supply function p=2 x. Find consumer’s surplus.

To find the Producer’s Surplus Profit = Revenue - Cost. Producer’s Surplus= Demand Price- Supply Price P. S= Poxo -

1. 2. 3. The supply function for a commodity p=2+D². Find the Producer’s surplus when p=18. Find the producer’s surplus for the supply function p=2 x²+x+5. when x 0=3. The demand function for a commodity p=25 D-20. the supply function p=5 D+60

Consumer’s surplus and Producer’s surplus: 1. Given the demand function p=8 -2 x and the supply function p=2+x, find the consumer’s surplus and the producer’s surplus.