Differential Analysis of Fluid Flow Part II Potential




















- Slides: 78

Differential Analysis of Fluid Flow Part II Potential Flows

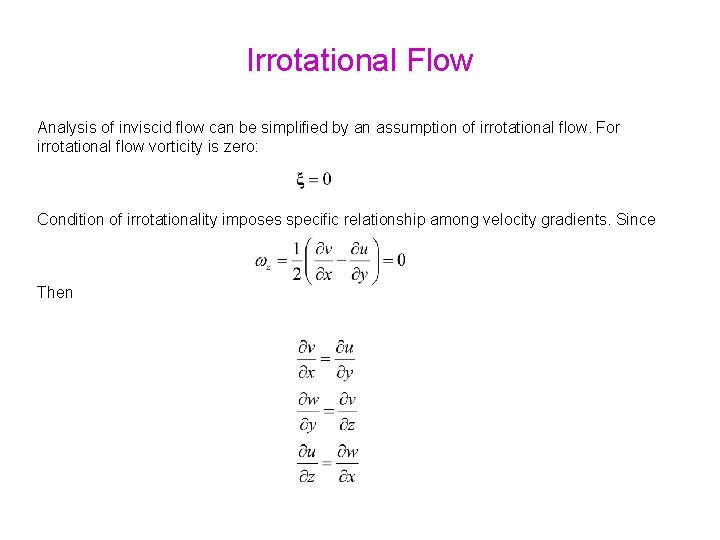
Irrotational Flow Analysis of inviscid flow can be simplified by an assumption of irrotational flow. For irrotational flow vorticity is zero: Condition of irrotationality imposes specific relationship among velocity gradients. Since Then

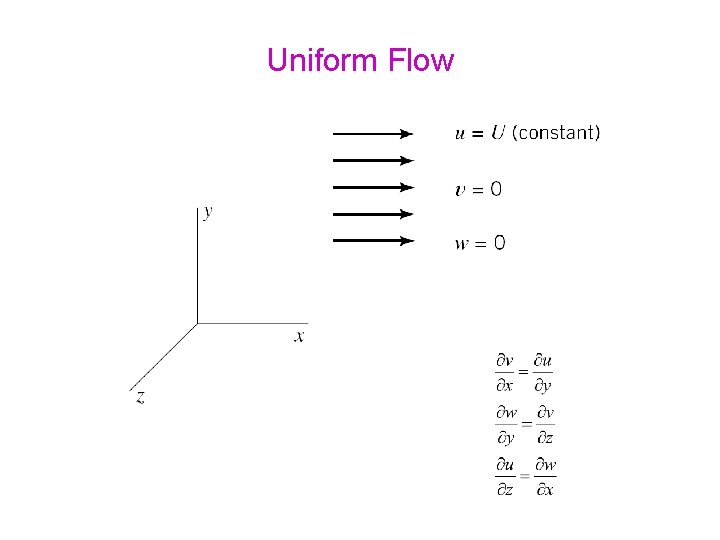
Uniform Flow

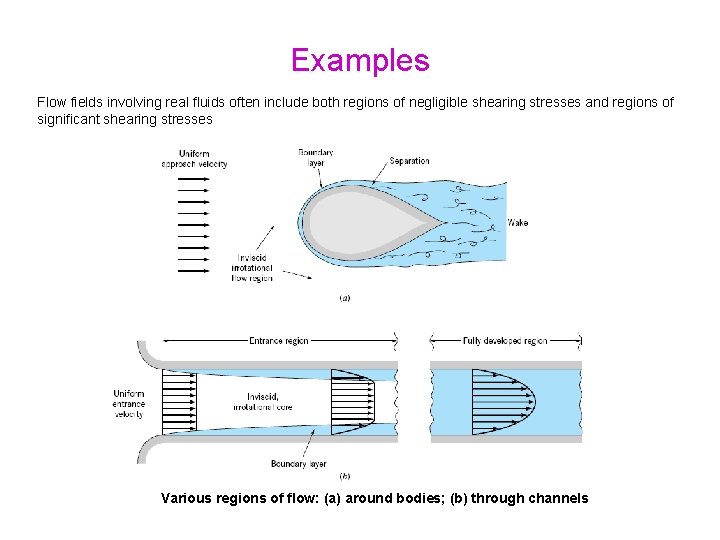
Examples Flow fields involving real fluids often include both regions of negligible shearing stresses and regions of significant shearing stresses Uniform flow in x direction Various regions of flow: (a) around bodies; (b) through channels

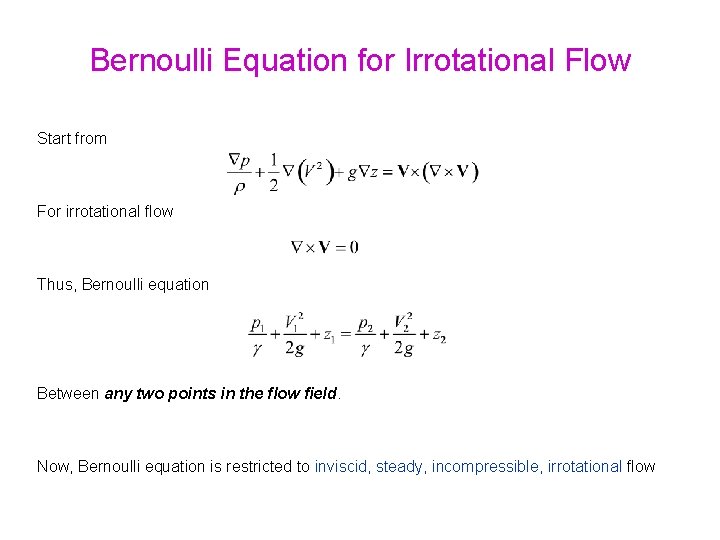
Bernoulli Equation for Irrotational Flow Start from For irrotational flow Thus, Bernoulli equation Between any two points in the flow field. Now, Bernoulli equation is restricted to inviscid, steady, incompressible, irrotational flow

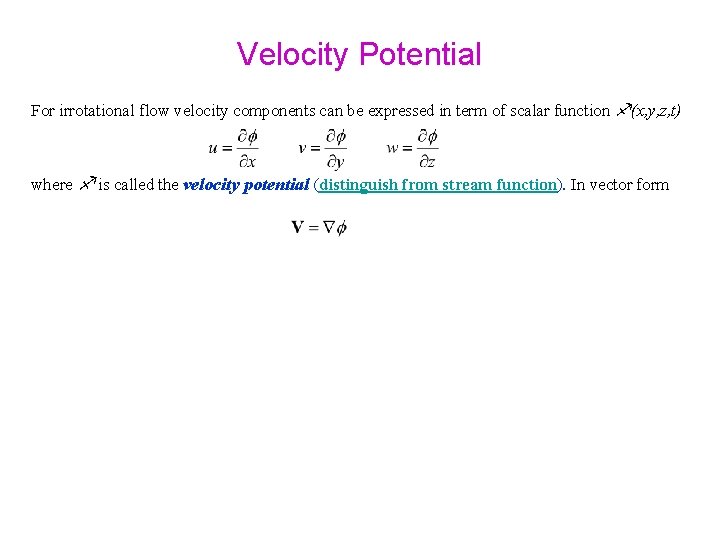
Velocity Potential For irrotational flow velocity components can be expressed in term of scalar function (x, y, z, t)

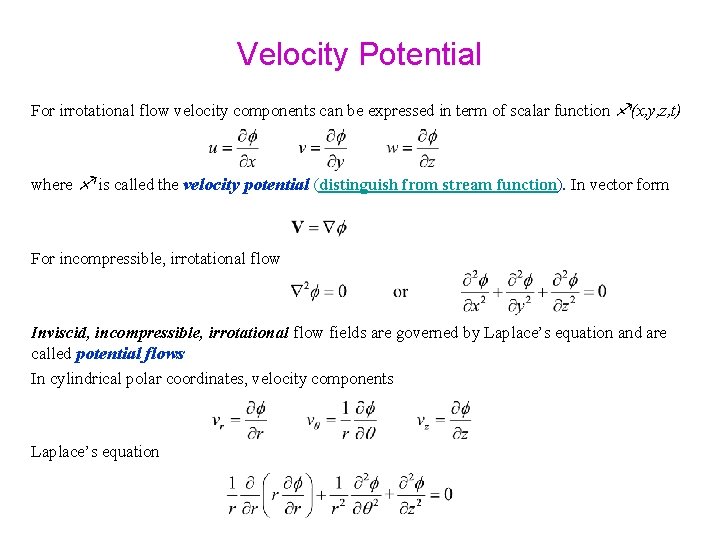
Velocity Potential For irrotational flow velocity components can be expressed in term of scalar function (x, y, z, t) where is called the velocity potential (distinguish from stream function). In vector form

Velocity Potential For irrotational flow velocity components can be expressed in term of scalar function (x, y, z, t) where is called the velocity potential (distinguish from stream function). In vector form For incompressible, irrotational flow Inviscid, incompressible, irrotational flow fields are governed by Laplace’s equation and are called potential flows In cylindrical polar coordinates, velocity components Laplace’s equation

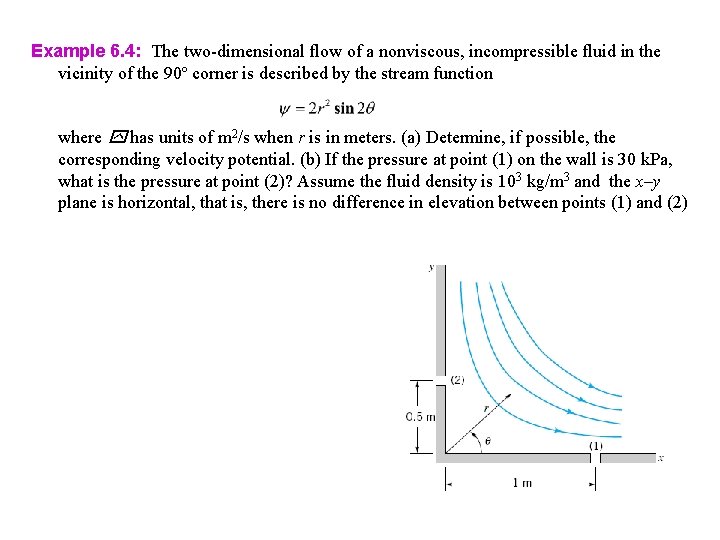
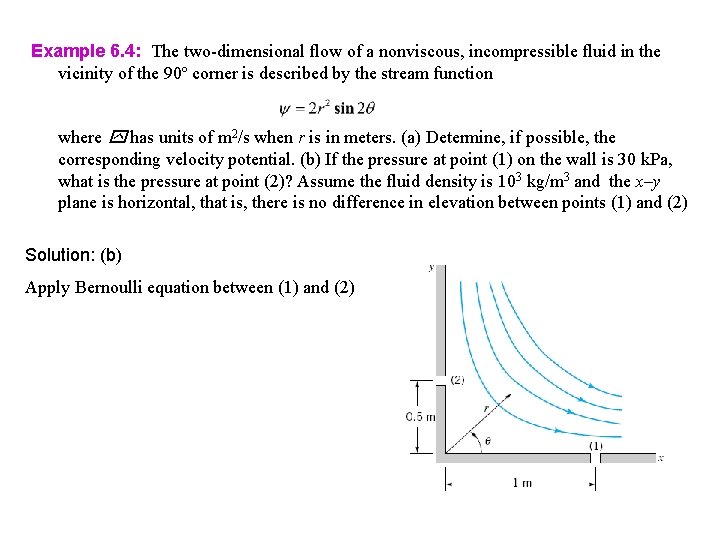
Example 6. 4: The two-dimensional flow of a nonviscous, incompressible fluid in the vicinity of the 90º corner is described by the stream function where has units of m 2/s when r is in meters. (a) Determine, if possible, the corresponding velocity potential. (b) If the pressure at point (1) on the wall is 30 k. Pa, what is the pressure at point (2)? Assume the fluid density is 103 kg/m 3 and the x–y plane is horizontal, that is, there is no difference in elevation between points (1) and (2)

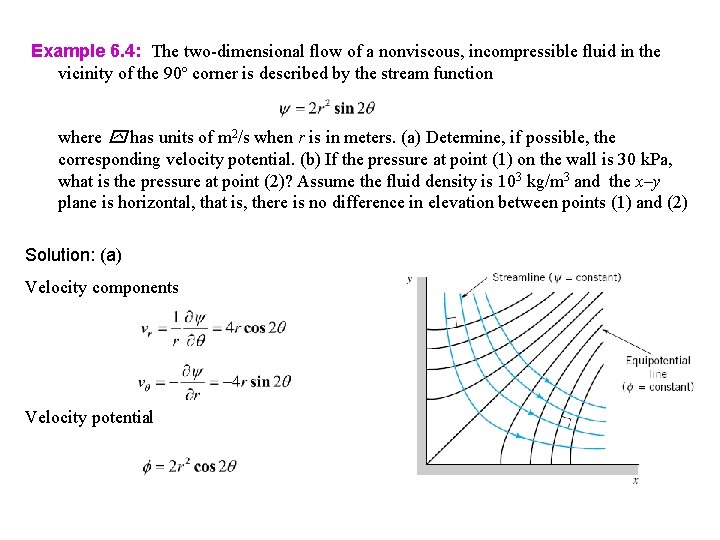
Example 6. 4: The two-dimensional flow of a nonviscous, incompressible fluid in the vicinity of the 90º corner is described by the stream function where has units of m 2/s when r is in meters. (a) Determine, if possible, the corresponding velocity potential. (b) If the pressure at point (1) on the wall is 30 k. Pa, what is the pressure at point (2)? Assume the fluid density is 103 kg/m 3 and the x–y plane is horizontal, that is, there is no difference in elevation between points (1) and (2) Solution: (a) Velocity components Velocity potential

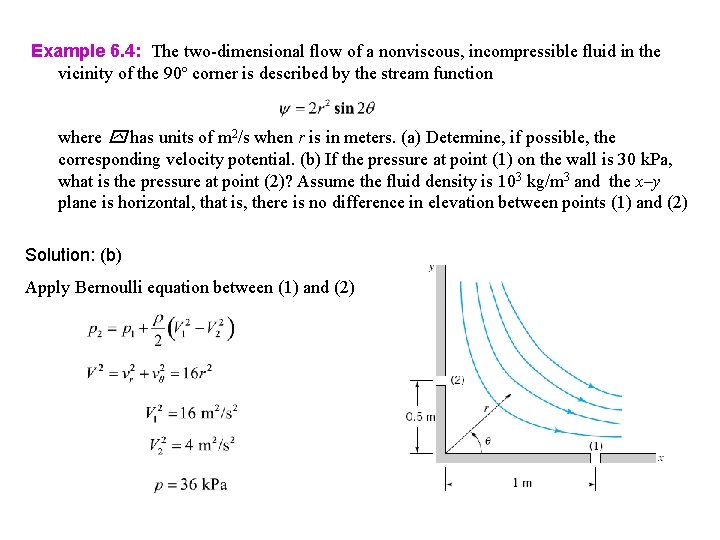
Example 6. 4: The two-dimensional flow of a nonviscous, incompressible fluid in the vicinity of the 90º corner is described by the stream function where has units of m 2/s when r is in meters. (a) Determine, if possible, the corresponding velocity potential. (b) If the pressure at point (1) on the wall is 30 k. Pa, what is the pressure at point (2)? Assume the fluid density is 103 kg/m 3 and the x–y plane is horizontal, that is, there is no difference in elevation between points (1) and (2) Solution: (b) Apply Bernoulli equation between (1) and (2)

Example 6. 4: The two-dimensional flow of a nonviscous, incompressible fluid in the vicinity of the 90º corner is described by the stream function where has units of m 2/s when r is in meters. (a) Determine, if possible, the corresponding velocity potential. (b) If the pressure at point (1) on the wall is 30 k. Pa, what is the pressure at point (2)? Assume the fluid density is 103 kg/m 3 and the x–y plane is horizontal, that is, there is no difference in elevation between points (1) and (2) Solution: (b) Apply Bernoulli equation between (1) and (2)

Basic, Plane Potential Flows

Basic, Plane Potential Flows • Laplace’s equation is a linear partial differential equation • For potential flow, basic solutions can be added to obtain more complicated solutions • Only plane flows will be considered • For plane irrotational flow one can use either velocity potential or the stream function – both must satisfy Laplace’s equation • Flow net consists of a family of streamlines and equipotential lines

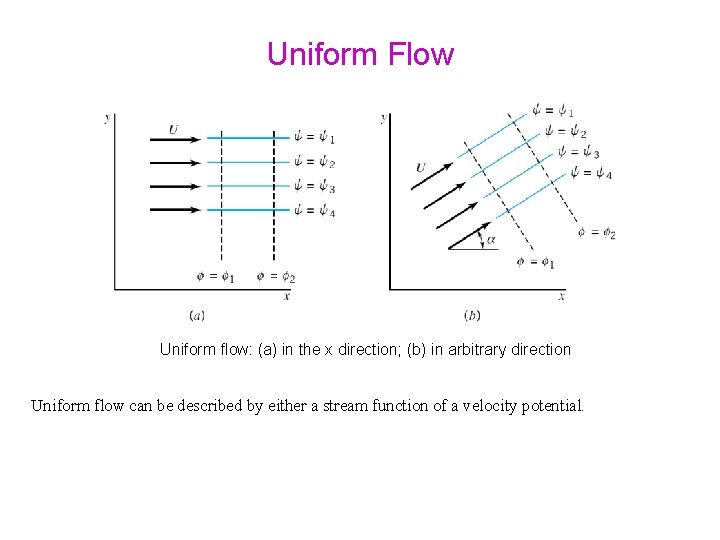
Uniform Flow Uniform flow: (a) in the x direction; (b) in arbitrary direction Uniform flow can be described by either a stream function of a velocity potential.

Uniform Flow Uniform flow: (a) in the x direction; (b) in arbitrary direction Uniform flow can be described by either a stream function of a velocity potential. For case (a) for case (b)

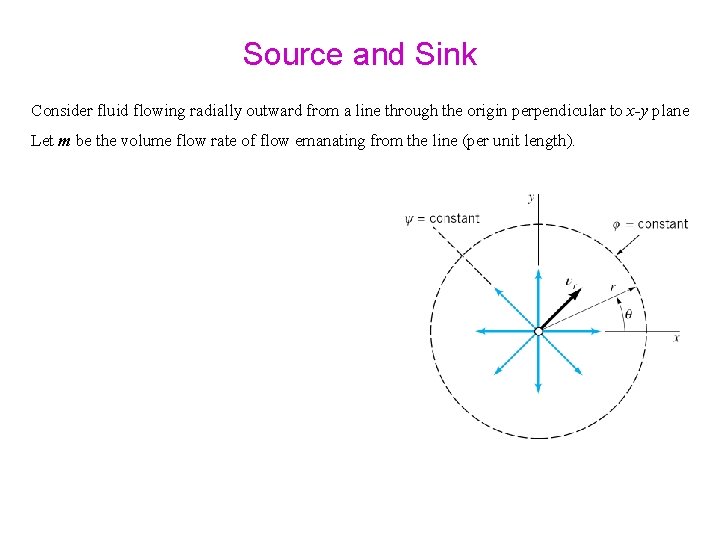
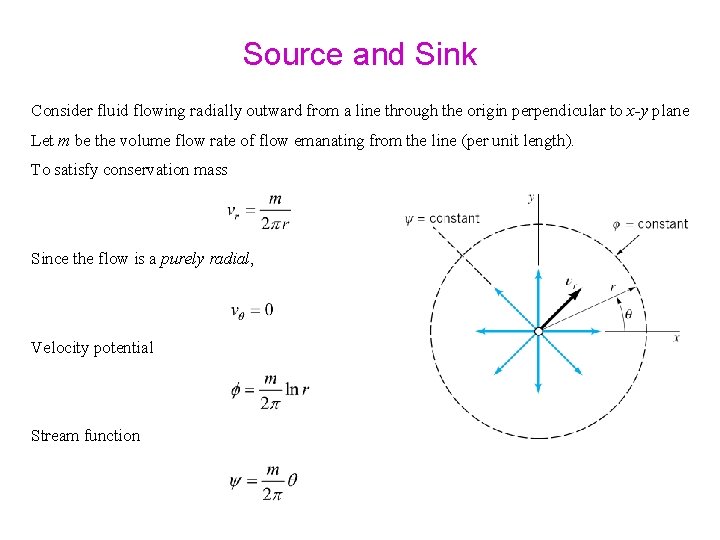
Source and Sink Consider fluid flowing radially outward from a line through the origin perpendicular to x-y plane Let m be the volume flow rate of flow emanating from the line (per unit length).

Source and Sink Consider fluid flowing radially outward from a line through the origin perpendicular to x-y plane Let m be the volume flow rate of flow emanating from the line (per unit length). To satisfy conservation mass Since the flow is a purely radial, Velocity potential Stream function

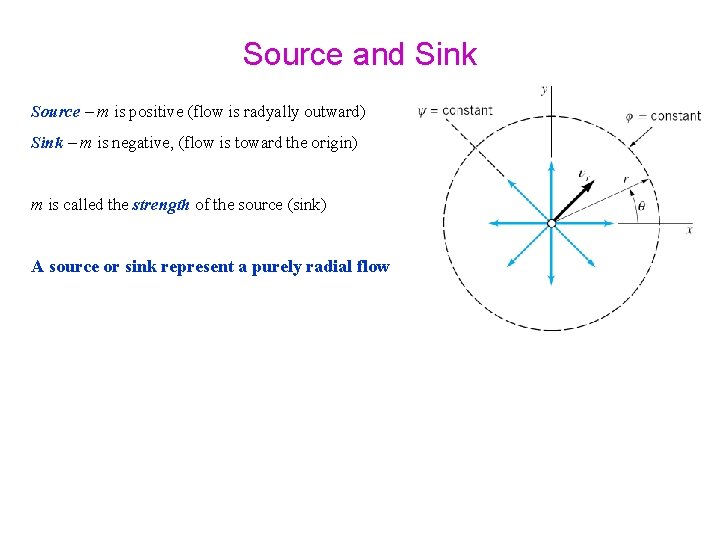
Source and Sink Source – m is positive (flow is radyally outward) Sink – m is negative, (flow is toward the origin) m is called the strength of the source (sink) A source or sink represent a purely radial flow

Source and Sink Source – m is positive (flow is radyally outward) Sink – m is negative, (flow is toward the origin) m is called the strength of the source (sink) A source or sink represent a purely radial flow At the origin r = 0 and which is impossible Thus, sources and sinks do not really exist in real flow fields, and the line representing the source or sink is a mathematical singularity in the flow field. However, some real flows can be approximated at points away from the origin by using sources of sinks

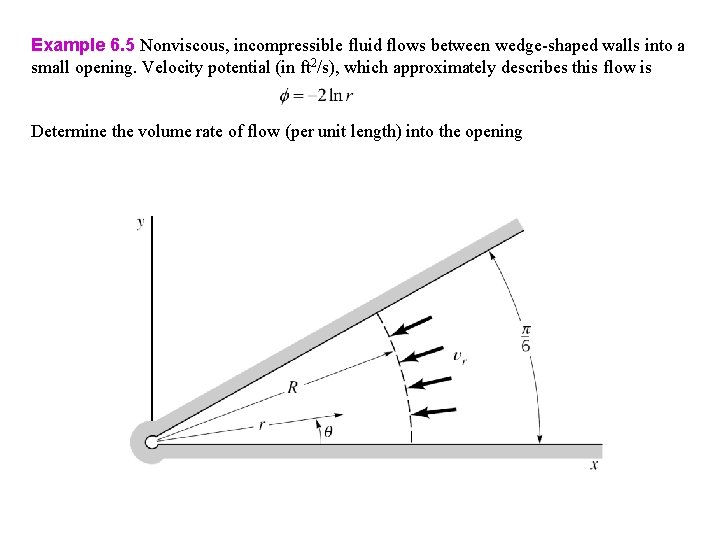
Example 6. 5 Nonviscous, incompressible fluid flows between wedge-shaped walls into a small opening. Velocity potential (in ft 2/s), which approximately describes this flow is Determine the volume rate of flow (per unit length) into the opening

Example 6. 5 Nonviscous, incompressible fluid flows between wedge-shaped walls into a small opening. Velocity potential (in ft 2/s), which approximately describes this flow is Determine the volume rate of flow (per unit length) into the opening Solution Velocity components Flowrate Note that the radius R is arbitrary since the flowrate crossing any curve between the two walls must be the same. The negative sign indicate that the flow is toward the opening

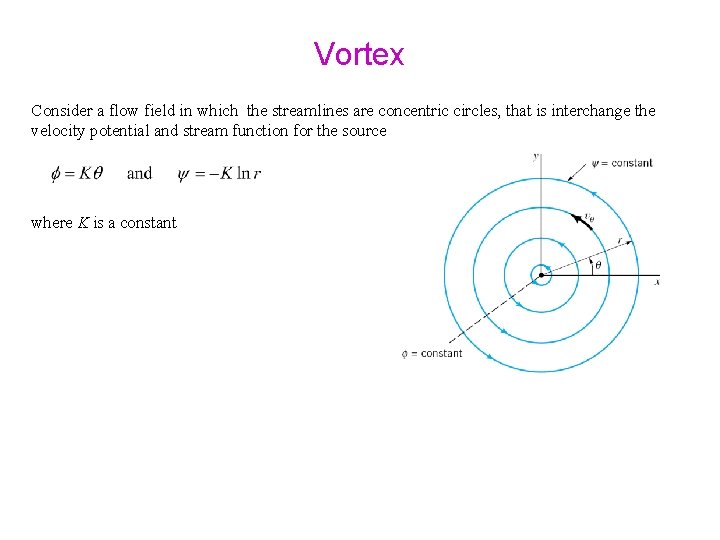
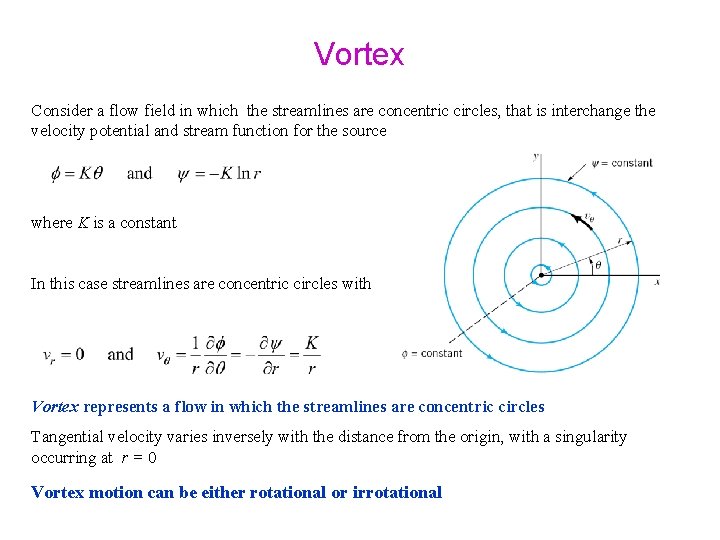
Vortex Consider a flow field in which the streamlines are concentric circles, that is interchange the velocity potential and stream function for the source where K is a constant

Vortex Consider a flow field in which the streamlines are concentric circles, that is interchange the velocity potential and stream function for the source where K is a constant In this case streamlines are concentric circles with Vortex represents a flow in which the streamlines are concentric circles Tangential velocity varies inversely with the distance from the origin, with a singularity occurring at r = 0 Vortex motion can be either rotational or irrotational

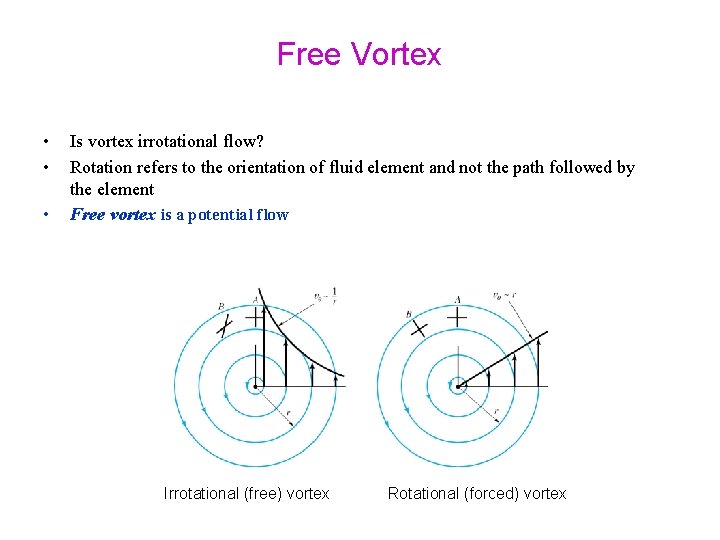
Free Vortex • • • Is vortex irrotational flow? Rotation refers to the orientation of fluid element and not the path followed by the element Free vortex is a potential flow Irrotational (free) vortex Rotational (forced) vortex

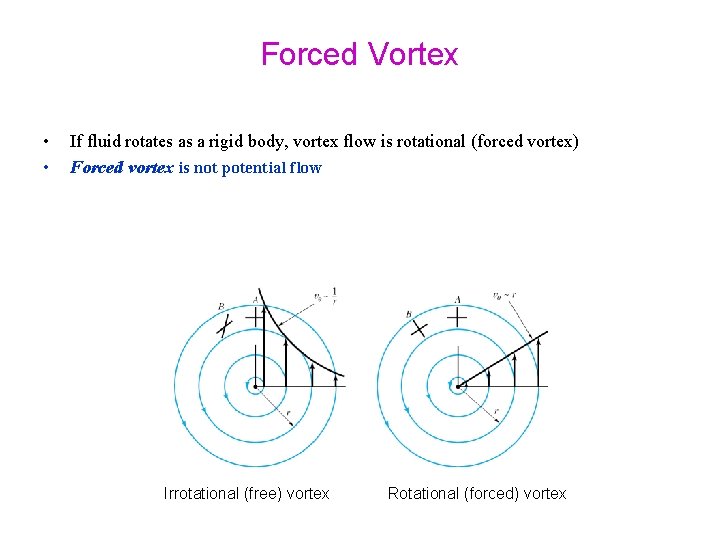
Forced Vortex • • If fluid rotates as a rigid body, vortex flow is rotational (forced vortex) Forced vortex is not potential flow Irrotational (free) vortex Rotational (forced) vortex

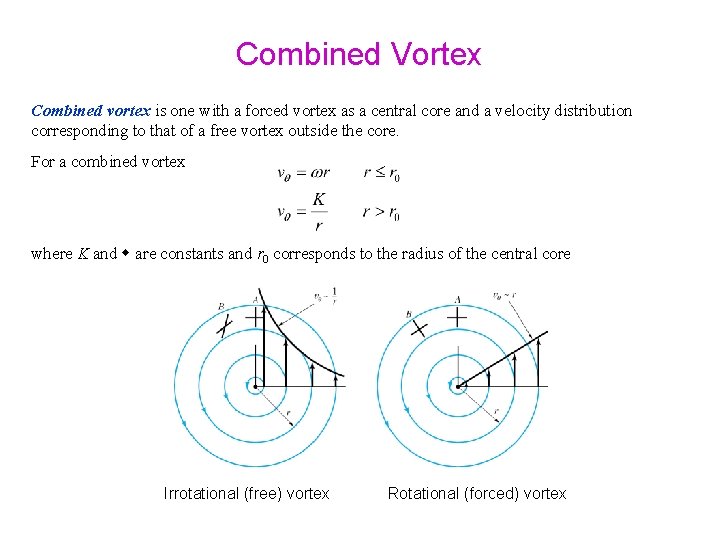
Combined Vortex Combined vortex is one with a forced vortex as a central core and a velocity distribution corresponding to that of a free vortex outside the core. For a combined vortex where K and are constants and r 0 corresponds to the radius of the central core Irrotational (free) vortex Rotational (forced) vortex

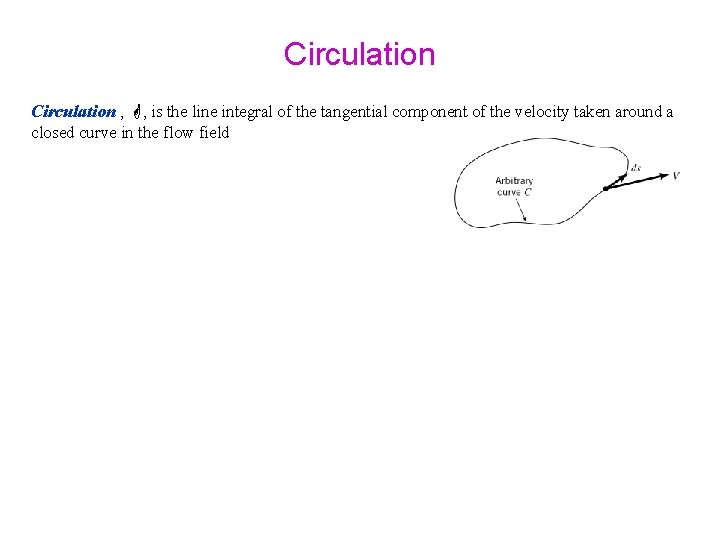
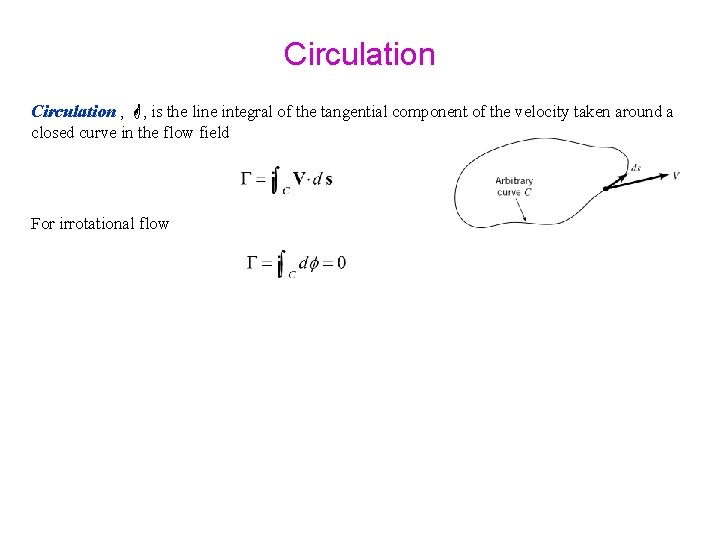
Circulation , , is the line integral of the tangential component of the velocity taken around a closed curve in the flow field

Circulation , , is the line integral of the tangential component of the velocity taken around a closed curve in the flow field For irrotational flow

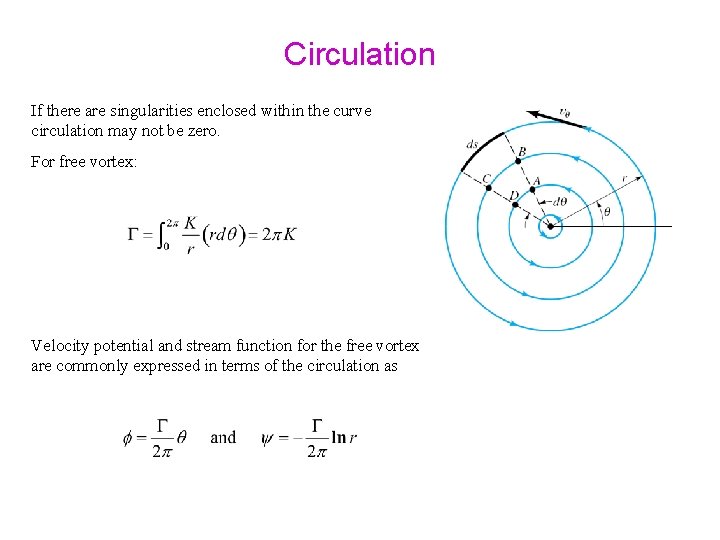
Circulation If there are singularities enclosed within the curve circulation may not be zero. For free vortex: Velocity potential and stream function for the free vortex are commonly expressed in terms of the circulation as

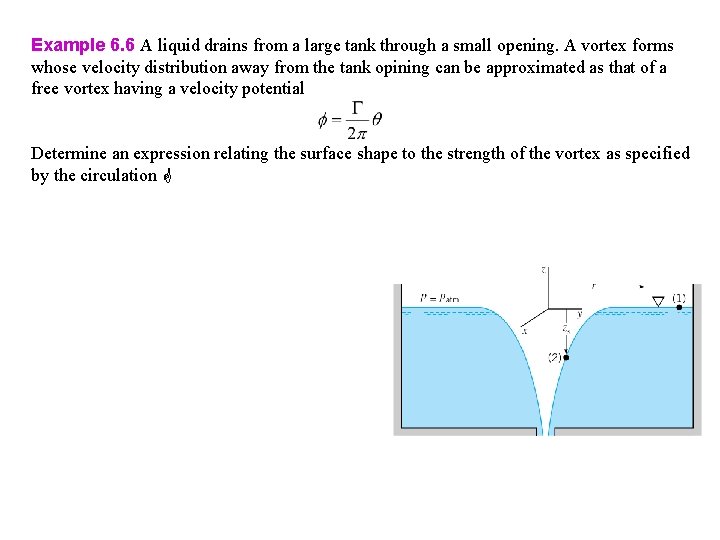
Example 6. 6 A liquid drains from a large tank through a small opening. A vortex forms whose velocity distribution away from the tank opining can be approximated as that of a free vortex having a velocity potential Determine an expression relating the surface shape to the strength of the vortex as specified by the circulation

Example 6. 6 A liquid drains from a large tank through a small opening. A vortex forms whose velocity distribution away from the tank opining can be approximated as that of a free vortex having a velocity potential Determine an expression relating the surface shape to the strength of the vortex as specified by the circulation Solution Apply Bernoulli equation between (1) and (2) Velocity Expression for surface shape

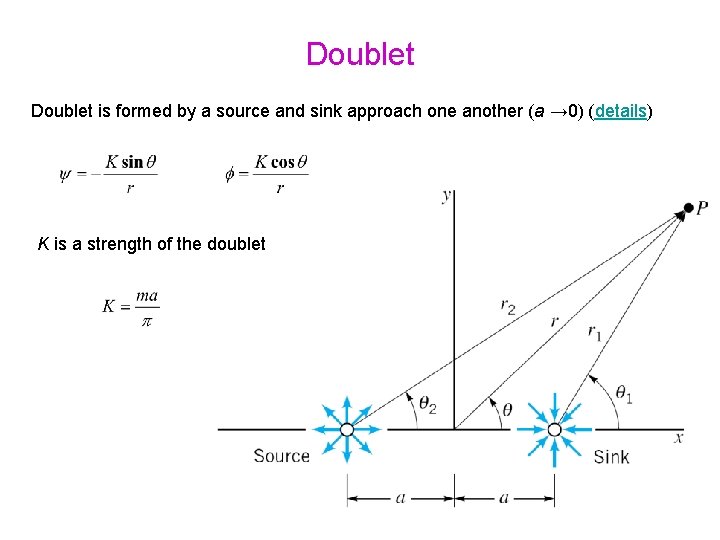
Doublet is formed by a source and sink approach one another (a → 0) (details) K is a strength of the doublet

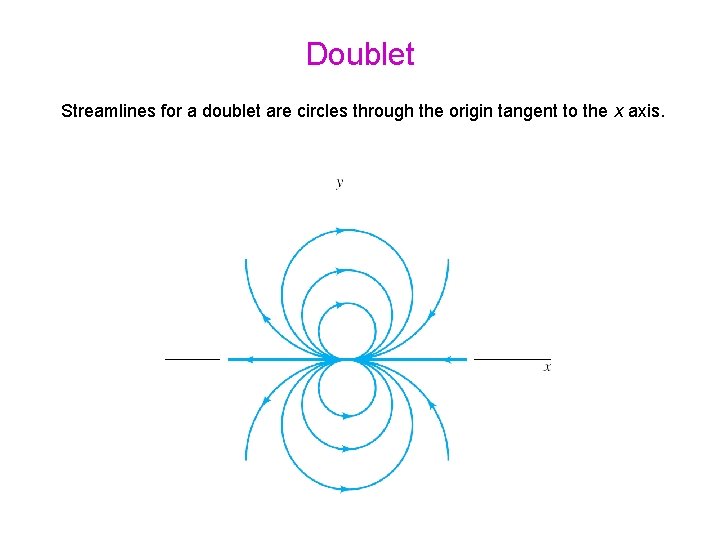
Doublet Streamlines for a doublet are circles through the origin tangent to the x axis.

Summary of Basic Potential Flows

Superposition of Basic, Plane Potential Flows

Superposition of Basic, Plane Potential Flows • Potential flows are governed by Laplace’s equation, which is linear partial differential equation. • Various basic velocity potentials and stream functions can be combined to form new potentials and stream functions. • Any streamline in an inviscid flow field can be considered as a solid boundary (no flow through the boundary or the streamline) • If we combine some basic velocity potentials or stream functions to yield a streamline that corresponds to a particular body shape, that combination can be used to describe the flow around that body. • This method is called the method of superposition

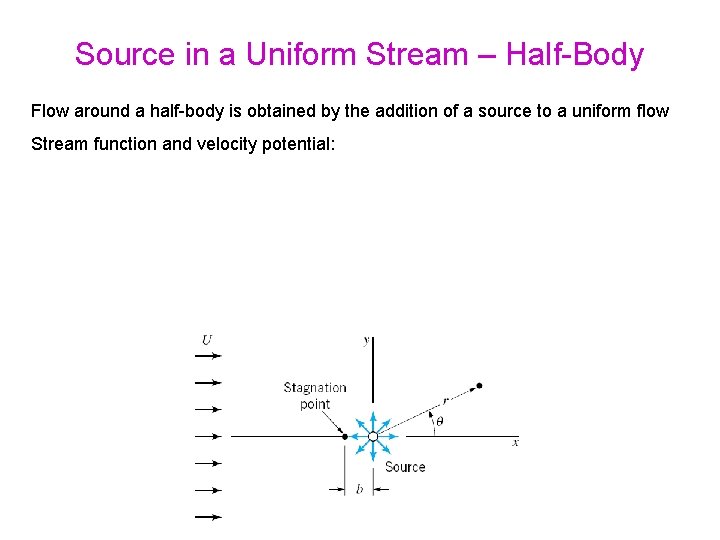
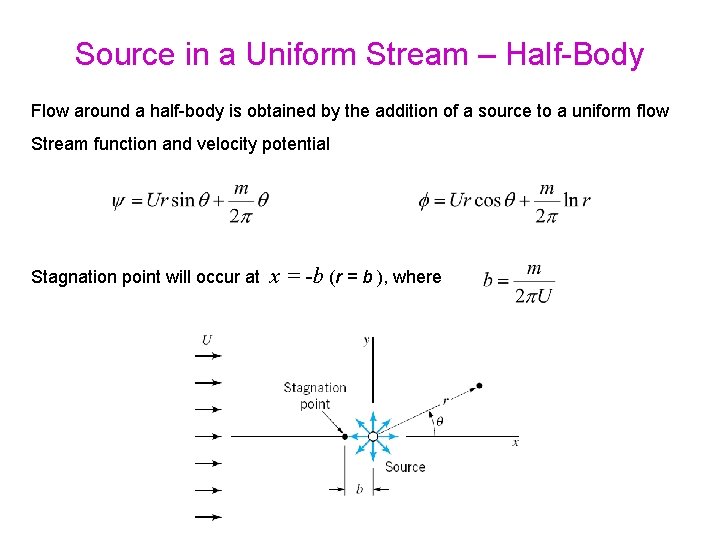
Source in a Uniform Stream – Half-Body Flow around a half-body is obtained by the addition of a source to a uniform flow Stream function and velocity potential:

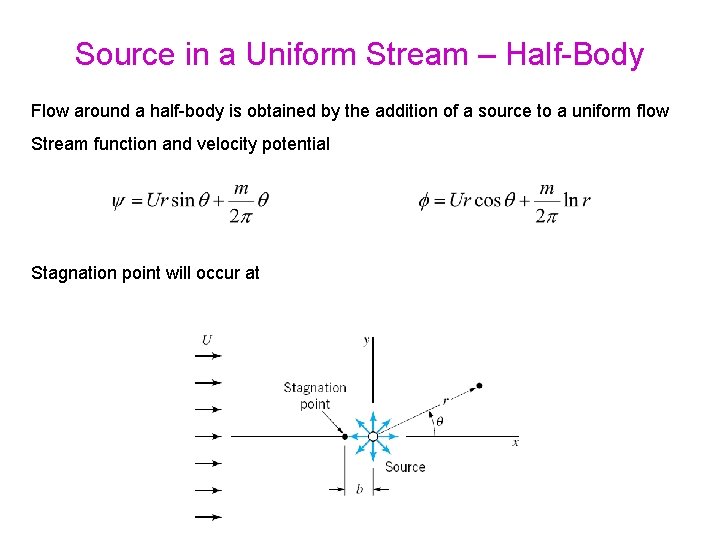
Source in a Uniform Stream – Half-Body Flow around a half-body is obtained by the addition of a source to a uniform flow Stream function and velocity potential Stagnation point will occur at

Source in a Uniform Stream – Half-Body Flow around a half-body is obtained by the addition of a source to a uniform flow Stream function and velocity potential Stagnation point will occur at x = -b (r = b ), where

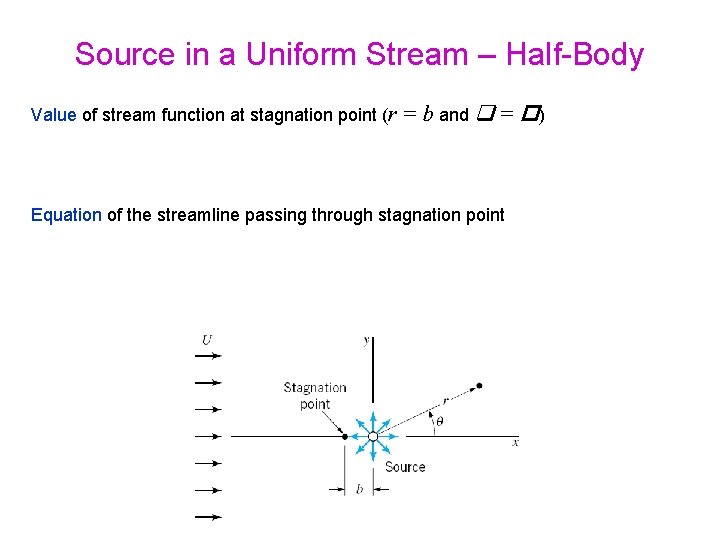
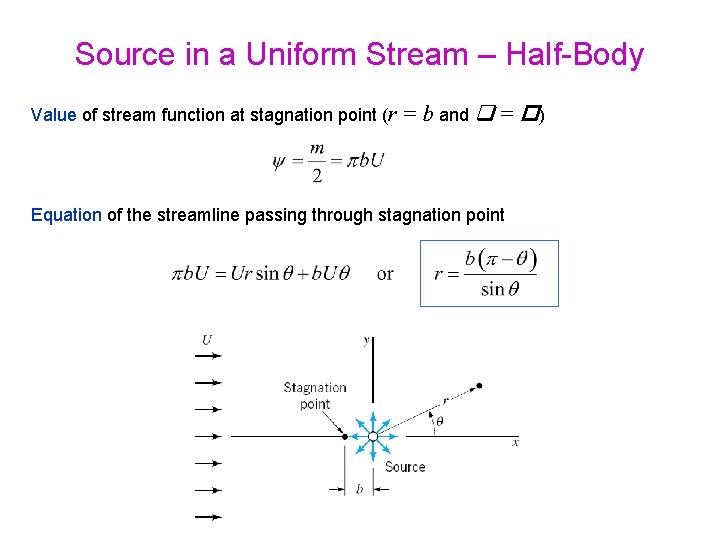
Source in a Uniform Stream – Half-Body Value of stream function at stagnation point (r = b and = ) Equation of the streamline passing through stagnation point

Source in a Uniform Stream – Half-Body Value of stream function at stagnation point (r = b and = ) Equation of the streamline passing through stagnation point

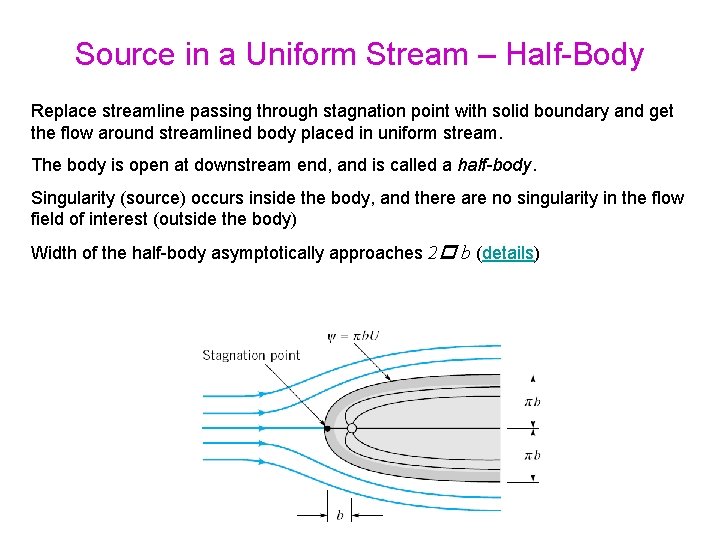
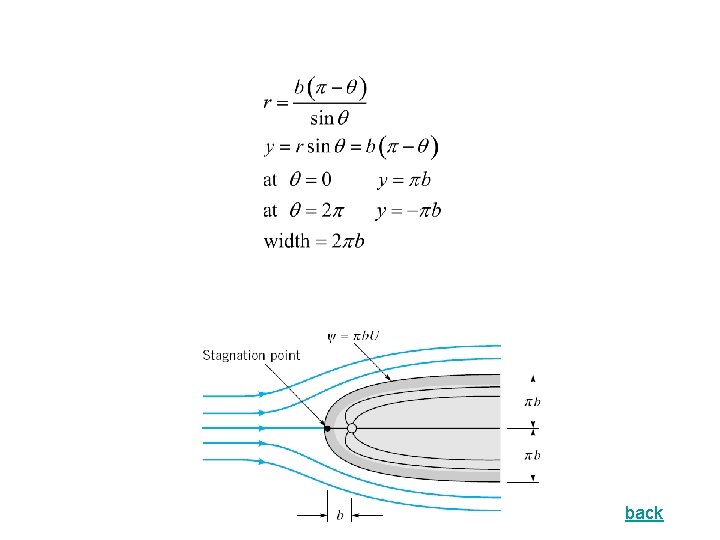
Source in a Uniform Stream – Half-Body Replace streamline passing through stagnation point with solid boundary and get the flow around streamlined body placed in uniform stream. The body is open at downstream end, and is called a half-body. Singularity (source) occurs inside the body, and there are no singularity in the flow field of interest (outside the body) Width of the half-body asymptotically approaches 2 b (details)

Source in a Uniform Stream – Half-Body Velocity for half-body

Source in a Uniform Stream – Half-Body For half-body Square of magnitude of velocity, V, at any point and since With velocity known, the pressure at any point can be determined from Bernoulli equation

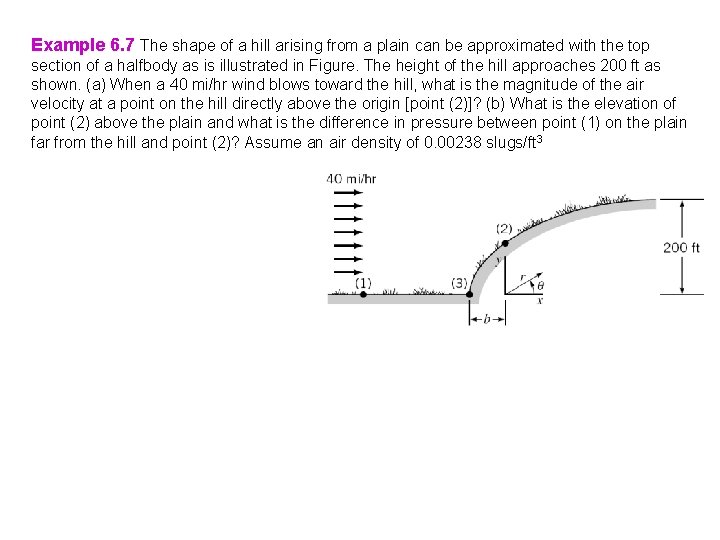
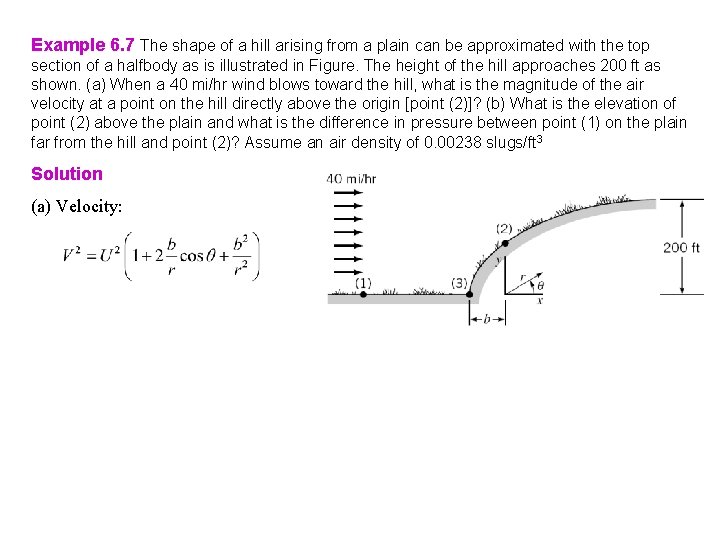
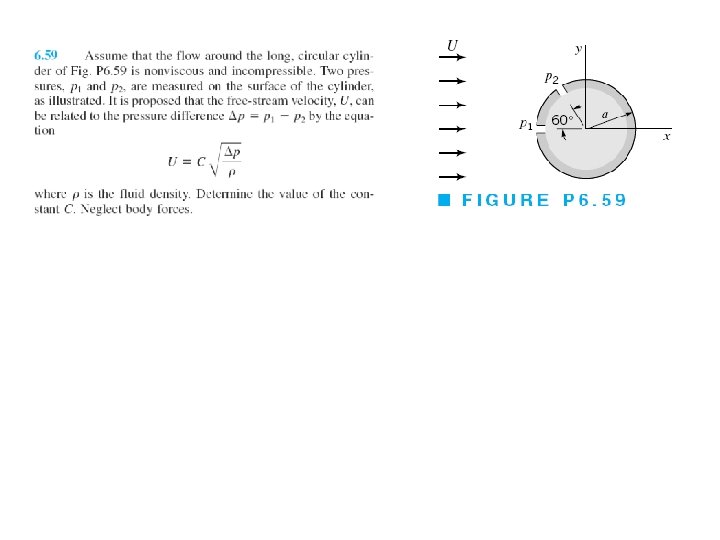
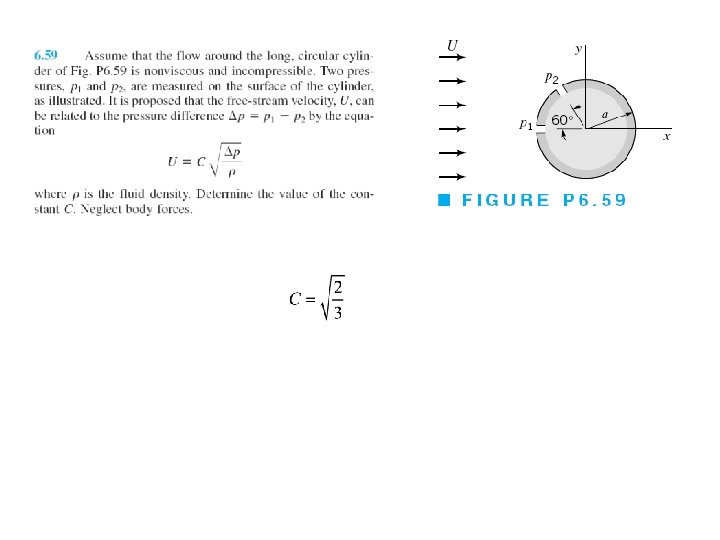
Example 6. 7 The shape of a hill arising from a plain can be approximated with the top section of a halfbody as is illustrated in Figure. The height of the hill approaches 200 ft as shown. (a) When a 40 mi/hr wind blows toward the hill, what is the magnitude of the air velocity at a point on the hill directly above the origin [point (2)]? (b) What is the elevation of point (2) above the plain and what is the difference in pressure between point (1) on the plain far from the hill and point (2)? Assume an air density of 0. 00238 slugs/ft 3

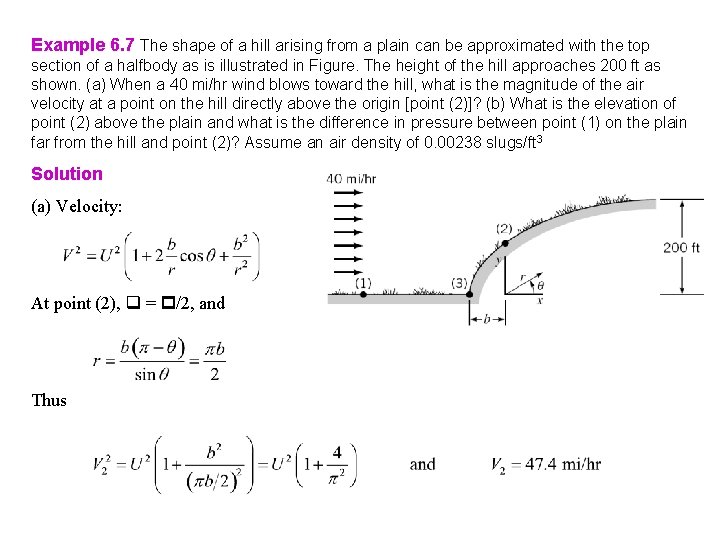
Example 6. 7 The shape of a hill arising from a plain can be approximated with the top section of a halfbody as is illustrated in Figure. The height of the hill approaches 200 ft as shown. (a) When a 40 mi/hr wind blows toward the hill, what is the magnitude of the air velocity at a point on the hill directly above the origin [point (2)]? (b) What is the elevation of point (2) above the plain and what is the difference in pressure between point (1) on the plain far from the hill and point (2)? Assume an air density of 0. 00238 slugs/ft 3 Solution (a) Velocity:

Example 6. 7 The shape of a hill arising from a plain can be approximated with the top section of a halfbody as is illustrated in Figure. The height of the hill approaches 200 ft as shown. (a) When a 40 mi/hr wind blows toward the hill, what is the magnitude of the air velocity at a point on the hill directly above the origin [point (2)]? (b) What is the elevation of point (2) above the plain and what is the difference in pressure between point (1) on the plain far from the hill and point (2)? Assume an air density of 0. 00238 slugs/ft 3 Solution (a) Velocity: At point (2), = /2, and Thus

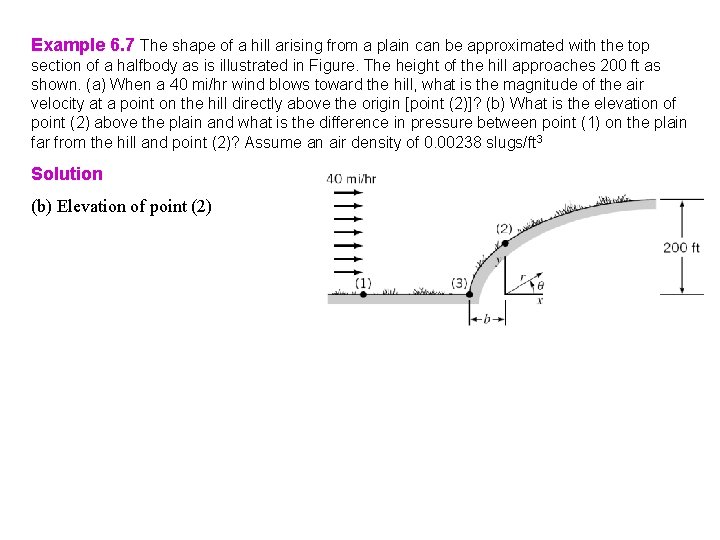
Example 6. 7 The shape of a hill arising from a plain can be approximated with the top section of a halfbody as is illustrated in Figure. The height of the hill approaches 200 ft as shown. (a) When a 40 mi/hr wind blows toward the hill, what is the magnitude of the air velocity at a point on the hill directly above the origin [point (2)]? (b) What is the elevation of point (2) above the plain and what is the difference in pressure between point (1) on the plain far from the hill and point (2)? Assume an air density of 0. 00238 slugs/ft 3 Solution (b) Elevation of point (2)

Example 6. 7 The shape of a hill arising from a plain can be approximated with the top section of a halfbody as is illustrated in Figure. The height of the hill approaches 200 ft as shown. (a) When a 40 mi/hr wind blows toward the hill, what is the magnitude of the air velocity at a point on the hill directly above the origin [point (2)]? (b) What is the elevation of point (2) above the plain and what is the difference in pressure between point (1) on the plain far from the hill and point (2)? Assume an air density of 0. 00238 slugs/ft 3 Solution (b) Elevation of point (2) From Bernoulli equation

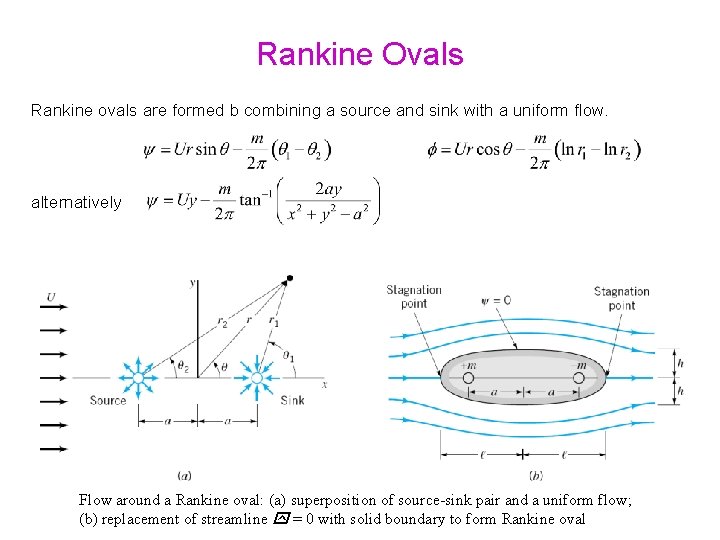
Rankine Ovals Rankine ovals are formed b combining a source and sink with a uniform flow. alternatively Flow around a Rankine oval: (a) superposition of source-sink pair and a uniform flow; (b) replacement of streamline = 0 with solid boundary to form Rankine oval

Rankine Ovals Streamline with = 0 forms closed body which is called Rankine oval Flow around a Rankine oval: (a) superposition of source-sink pair and a uniform flow; (b) replacement of streamline = 0 with solid boundary to form Rankine oval

Rankine Ovals Stagnation points occur at the upstream and downstream ends of the body Location of stagnation points depend on a, m and U Flow around a Rankine oval: (a) superposition of source-sink pair and a uniform flow; (b) replacement of streamline = 0 with solid boundary to form Rankine oval

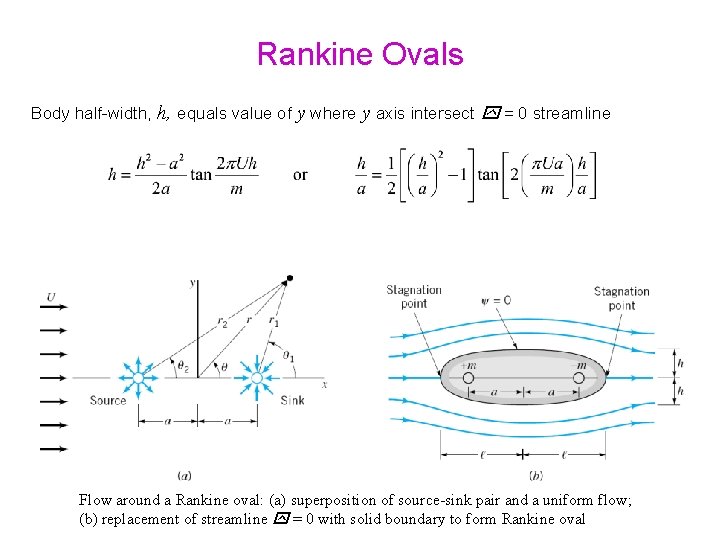
Rankine Ovals Body half-width, h, equals value of y where y axis intersect = 0 streamline Flow around a Rankine oval: (a) superposition of source-sink pair and a uniform flow; (b) replacement of streamline = 0 with solid boundary to form Rankine oval

Rankine Ovals Parameter Ua/m determines body shape Large value of Ua/m – flow around long slender body Small value of Ua/m – flow around blunt body Flow around a Rankine oval: (a) superposition of source-sink pair and a uniform flow; (b) replacement of streamline = 0 with solid boundary to form Rankine oval

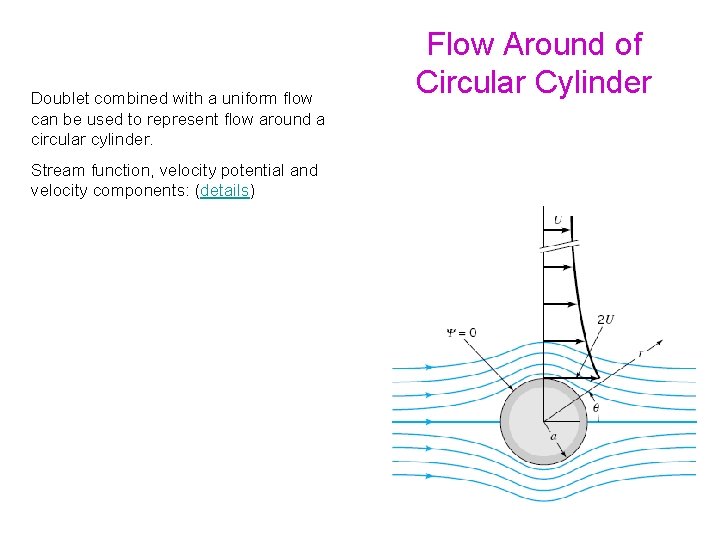
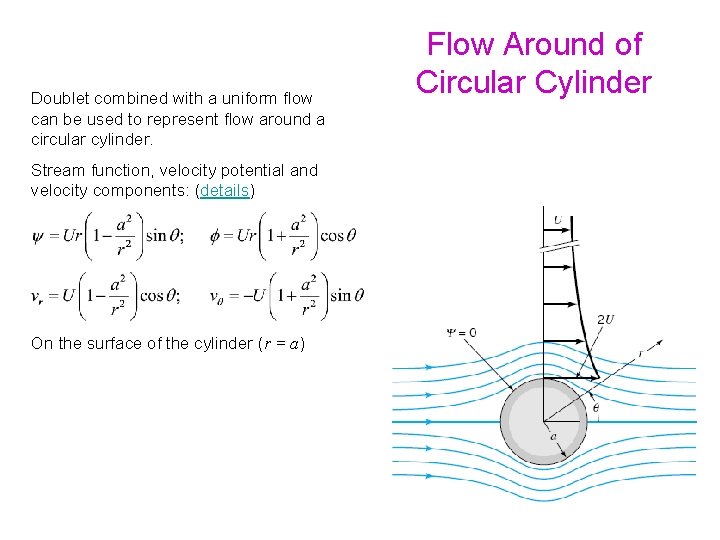
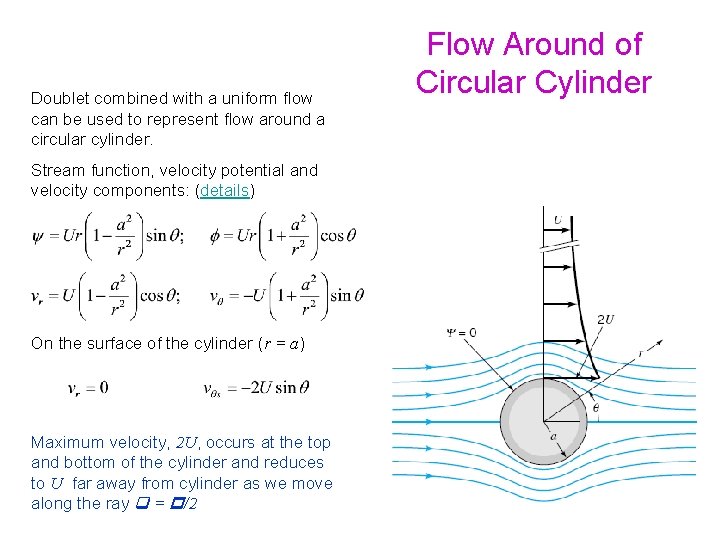
Doublet combined with a uniform flow can be used to represent flow around a circular cylinder. Stream function, velocity potential and velocity components: (details) Flow Around of Circular Cylinder

Doublet combined with a uniform flow can be used to represent flow around a circular cylinder. Stream function, velocity potential and velocity components: (details) On the surface of the cylinder (r = a) Flow Around of Circular Cylinder

Doublet combined with a uniform flow can be used to represent flow around a circular cylinder. Stream function, velocity potential and velocity components: (details) On the surface of the cylinder (r = a) Maximum velocity, 2 U, occurs at the top and bottom of the cylinder and reduces to U far away from cylinder as we move along the ray = /2 Flow Around of Circular Cylinder

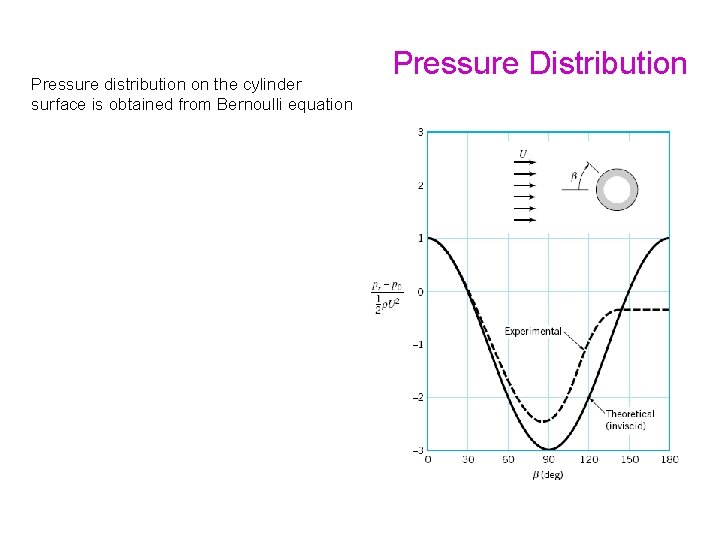
Pressure distribution on the cylinder surface is obtained from Bernoulli equation Pressure Distribution

Pressure distribution on the cylinder surface is obtained from Bernoulli equation Only on the upstream part of the cylinder is there approximate agreement between the potential flow and the experimental results Because to the viscous boundary layer that develops on the cylinder, the main flow separates from the surface of the cylinder, leading to the large difference between theoretical, frictionless fluid solution ant the experimental results on the downstream side of the cylinder Pressure Distribution

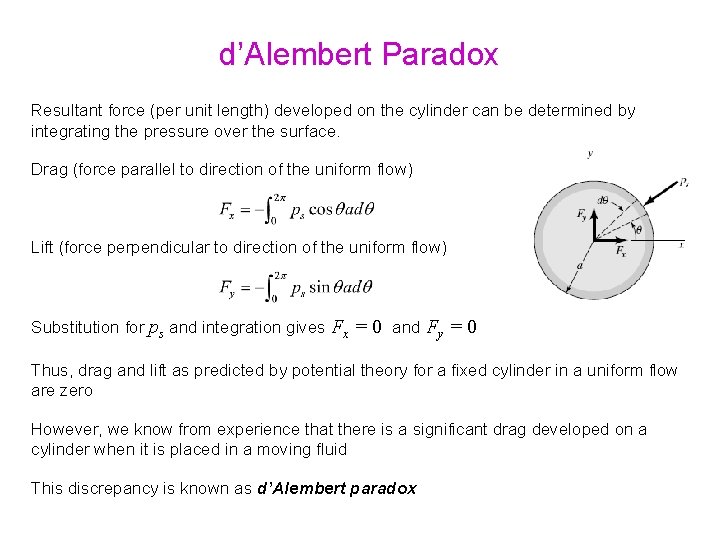
d’Alembert Paradox Resultant force (per unit length) developed on the cylinder can be determined by integrating the pressure over the surface. Drag (force parallel to direction of the uniform flow)

d’Alembert Paradox Resultant force (per unit length) developed on the cylinder can be determined by integrating the pressure over the surface. Drag (force parallel to direction of the uniform flow) Lift (force perpendicular to direction of the uniform flow) Substitution for ps and integration gives Fx = 0 and Fy = 0 Thus, drag and lift as predicted by potential theory for a fixed cylinder in a uniform flow are zero However, we know from experience that there is a significant drag developed on a cylinder when it is placed in a moving fluid This discrepancy is known as d’Alembert paradox

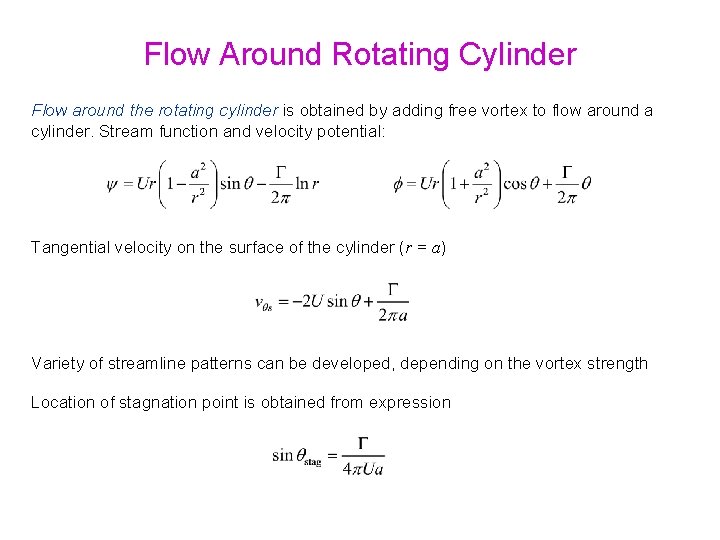
Flow Around Rotating Cylinder Flow around the rotating cylinder is obtained by adding free vortex to flow around a cylinder. Stream function and velocity potential: Tangential velocity on the surface of the cylinder (r = a) Variety of streamline patterns can be developed, depending on the vortex strength Location of stagnation point is obtained from expression

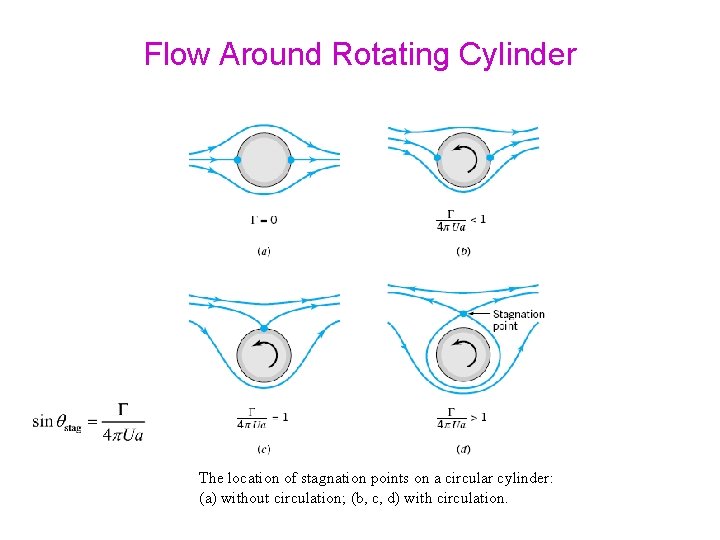
Flow Around Rotating Cylinder The location of stagnation points on a circular cylinder: (a) without circulation; (b, c, d) with circulation.

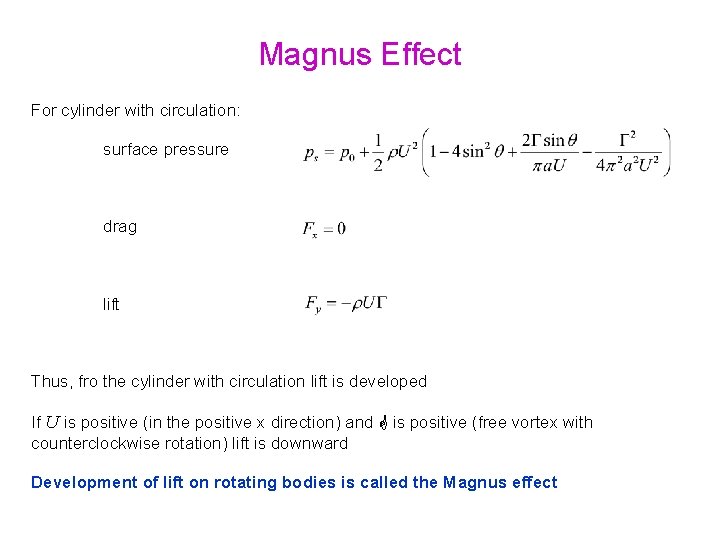
Magnus Effect For cylinder with circulation: surface pressure drag lift Thus, fro the cylinder with circulation lift is developed If U is positive (in the positive x direction) and is positive (free vortex with counterclockwise rotation) lift is downward Development of lift on rotating bodies is called the Magnus effect

End of lecture

Supplementary Slides

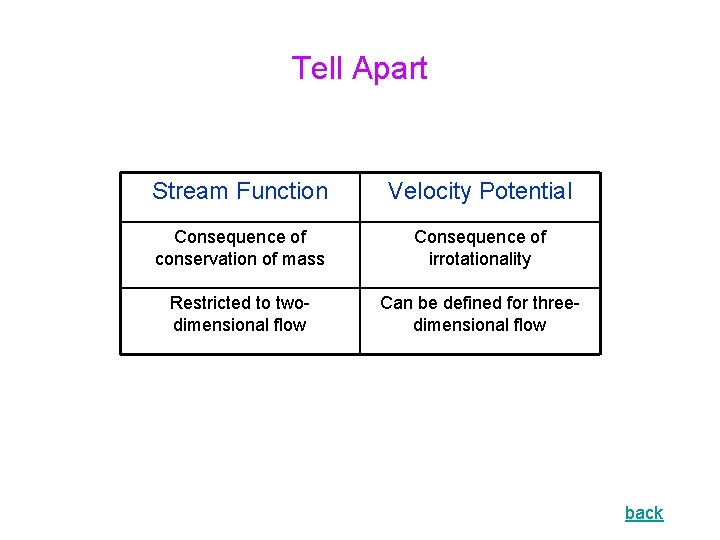
Tell Apart Stream Function Velocity Potential Consequence of conservation of mass Consequence of irrotationality Restricted to twodimensional flow Can be defined for threedimensional flow back

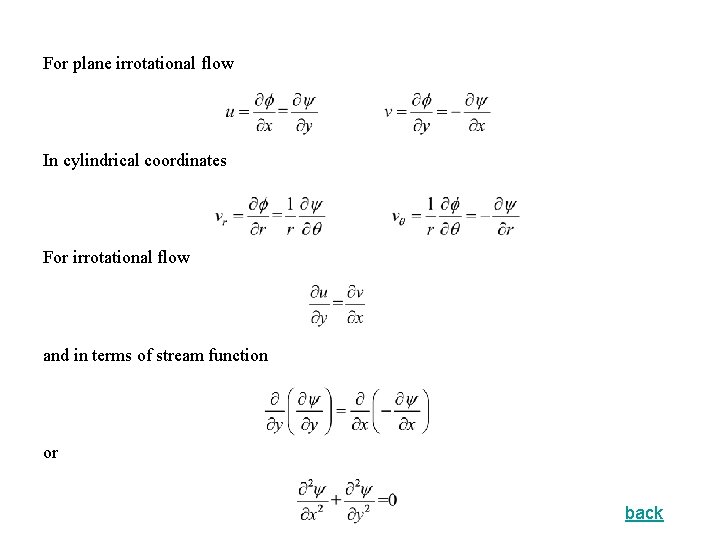
For plane irrotational flow In cylindrical coordinates For irrotational flow and in terms of stream function or back

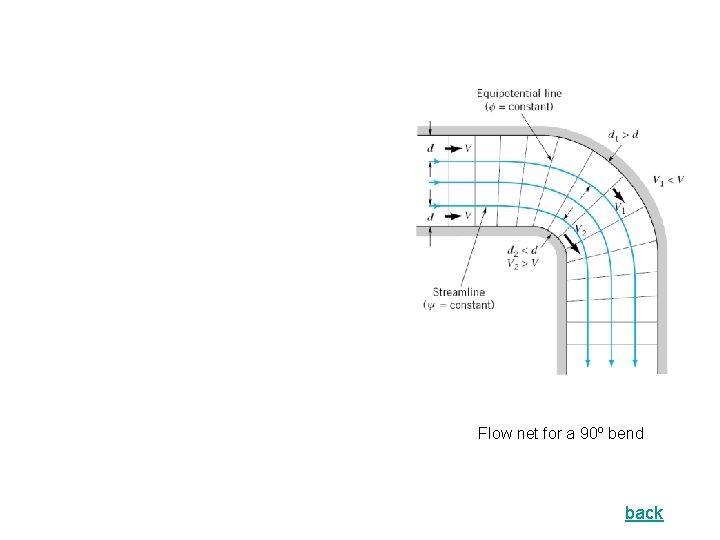
Flow net for a 90º bend back

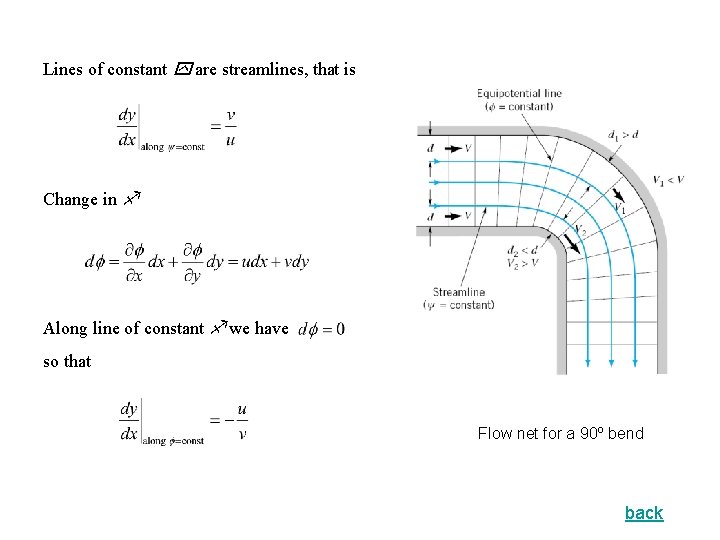
Lines of constant are streamlines, that is Change in Along line of constant we have so that Flow net for a 90º bend back

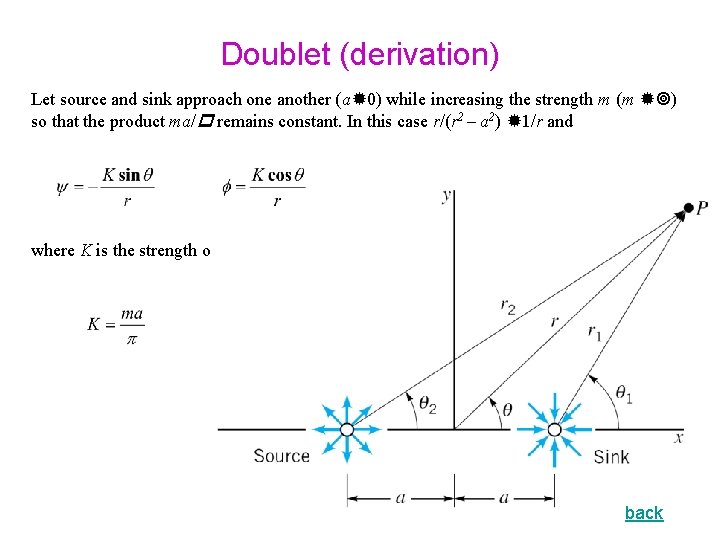
Doublet (derivation) Consider equal strength, source-sink pair. combined stream function for the pair is

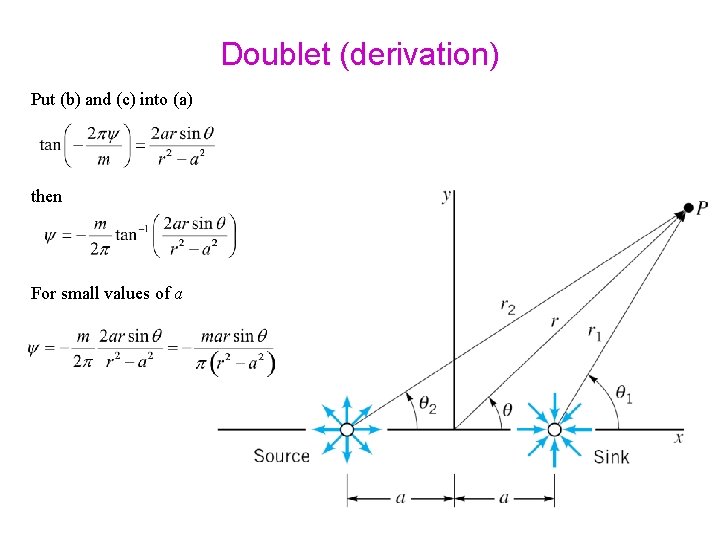
Doublet (derivation) Put (b) and (c) into (a) then For small values of a

Doublet (derivation) Let source and sink approach one another (a 0) while increasing the strength m (m ) so that the product ma/ remains constant. In this case r/(r 2 – a 2) 1/r and where K is the strength of the doublet back

back

Doublet combined with a uniform flow can be used to represent flow around a circular cylinder Flow Around of Circular Cylinder To represent flow around circular cylinder stream function must be constant for r = a, where a is the radius of the cylinder. From it follows that = 0 for r = a if Thus stream function and velocity potential for flow around circular cylinder are back


 Viscous fluid example
Viscous fluid example Timeline fluid mechanics
Timeline fluid mechanics Fluid sf
Fluid sf P1-p2
P1-p2 Fluid statics deals with fluid at rest
Fluid statics deals with fluid at rest Total body water
Total body water Intracellular extracellular fluid
Intracellular extracellular fluid Interstitial vs intracellular
Interstitial vs intracellular Movement of body fluids
Movement of body fluids Morison pouch
Morison pouch Potential differential field in repertory
Potential differential field in repertory Osmotic potential vs water potential
Osmotic potential vs water potential Flaccid turgid and plasmolysis
Flaccid turgid and plasmolysis Calculating solute potential
Calculating solute potential Neuronal pool
Neuronal pool Nerve action potential
Nerve action potential Graded vs action potential
Graded vs action potential Graded potential vs action potential
Graded potential vs action potential Saltatory conduction
Saltatory conduction Osmotic potential vs water potential
Osmotic potential vs water potential Sources of biopotential
Sources of biopotential End-plate potential vs action potential
End-plate potential vs action potential Intubating dose succinylcholine
Intubating dose succinylcholine Action potential resting potential
Action potential resting potential Action potential resting potential
Action potential resting potential Market potential and forecasting
Market potential and forecasting Equipotential lines
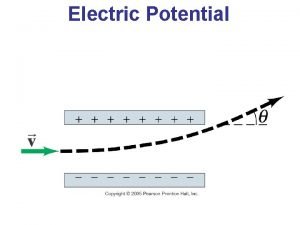
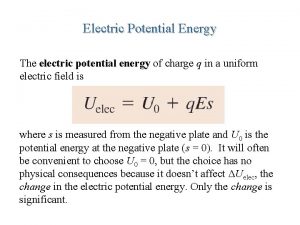
Equipotential lines Electric potential energy
Electric potential energy Electric potential
Electric potential Potential due to electric dipole
Potential due to electric dipole Electrostatic potential energy definition
Electrostatic potential energy definition V=pe/q
V=pe/q What is electrical potential
What is electrical potential Traffic flow differential equations
Traffic flow differential equations Differential pressure flow meters
Differential pressure flow meters Fluid portion of blood
Fluid portion of blood Cerebrospinal fluid breathing
Cerebrospinal fluid breathing Benoulli equation
Benoulli equation Momentum thickness turbulent boundary layer
Momentum thickness turbulent boundary layer Fluid statics
Fluid statics Eulerian description of fluid flow
Eulerian description of fluid flow Bernoulli fluid flow equation
Bernoulli fluid flow equation Flow divider valve symbol
Flow divider valve symbol Fluid flow products
Fluid flow products Specific force in open channel flow
Specific force in open channel flow Pitot tube bernoulli equation
Pitot tube bernoulli equation Complex potential of a doublet
Complex potential of a doublet Potential flow theory
Potential flow theory Circuit solution
Circuit solution Tga analysis
Tga analysis Differential thermal analysis
Differential thermal analysis Single ended ac voltage gain
Single ended ac voltage gain Differential cost analysis
Differential cost analysis Differential analysis: the key to decision making
Differential analysis: the key to decision making Power differential analysis
Power differential analysis Signal amplifier
Signal amplifier Dsc
Dsc Digital differential analysis
Digital differential analysis Differential cost analysis
Differential cost analysis Differential analysis the key to decision making
Differential analysis the key to decision making Addition symbol
Addition symbol Unit ratio definition
Unit ratio definition Brainpop ratios
Brainpop ratios What is a technical description?
What is a technical description? What are the 3 parts of the bar
What are the 3 parts of the bar The part of a shadow surrounding the darkest part
The part of a shadow surrounding the darkest part Minitab adalah
Minitab adalah Synovial fluid color analysis
Synovial fluid color analysis Csf and blood
Csf and blood Csf analysis
Csf analysis Mild ascites
Mild ascites Dimensional analysis fluid mechanics
Dimensional analysis fluid mechanics Ascitic fluid
Ascitic fluid Repeating variables are present in
Repeating variables are present in Magic box respiratory therapy
Magic box respiratory therapy Venturi mask 50 percent
Venturi mask 50 percent 3 liters fio2
3 liters fio2 Internal vs external flow
Internal vs external flow Flow energy review
Flow energy review