Recapitulate We need to redefine momentum Introduced the

Recapitulate • We need to redefine momentum. • Introduced the concept of four vector, by extending the concept of traditional vector.

Dot Product of Four Vectors Consider two four vectors, with components written in the frame S.

The dot product of these two four vectors is defined as follows.

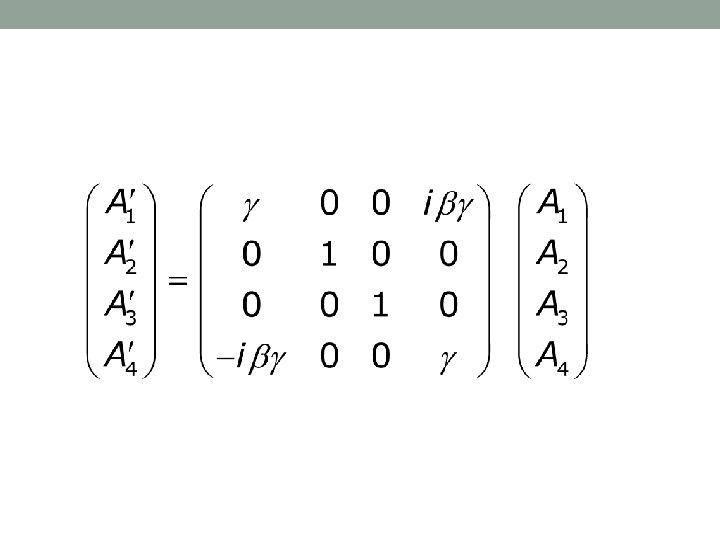
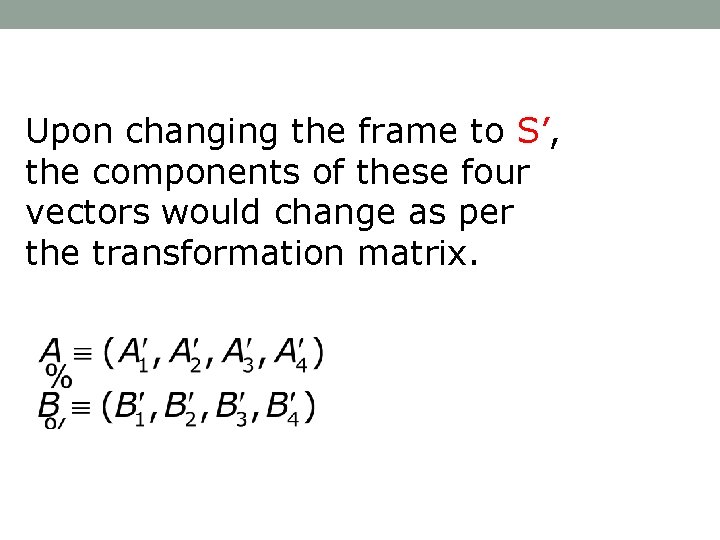
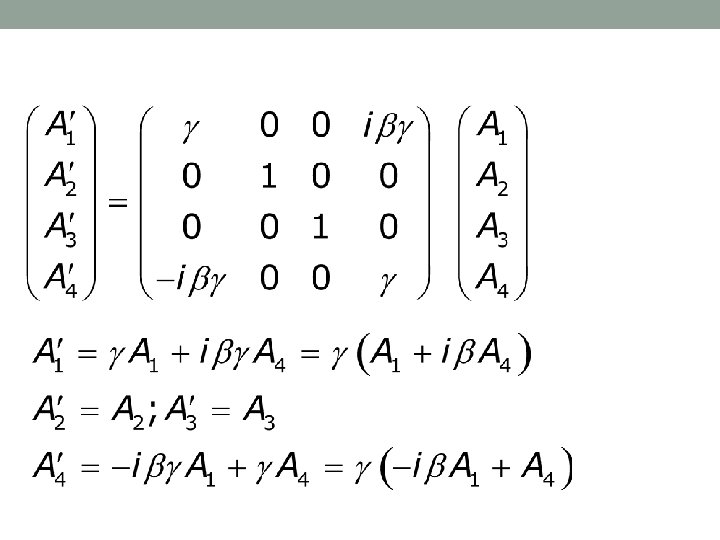
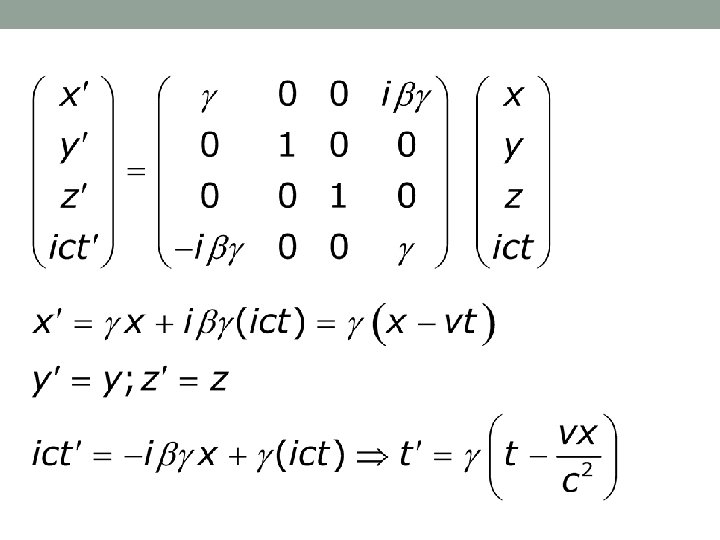
Upon changing the frame to S’, the components of these four vectors would change as per the transformation matrix.

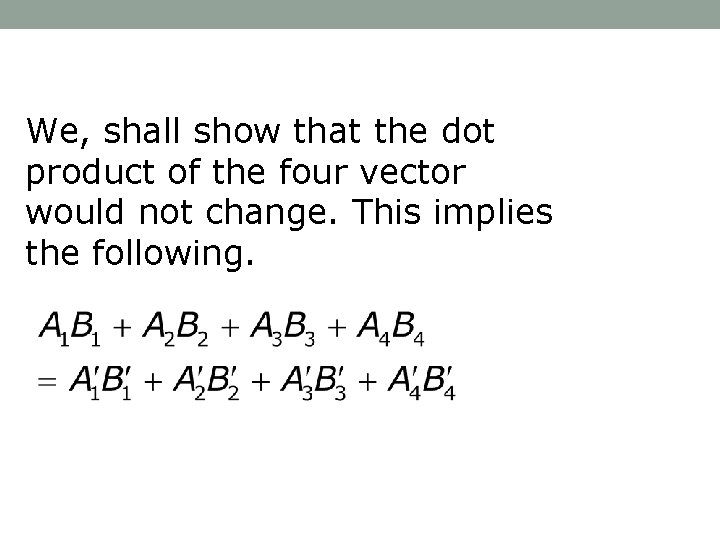
We, shall show that the dot product of the four vector would not change. This implies the following.

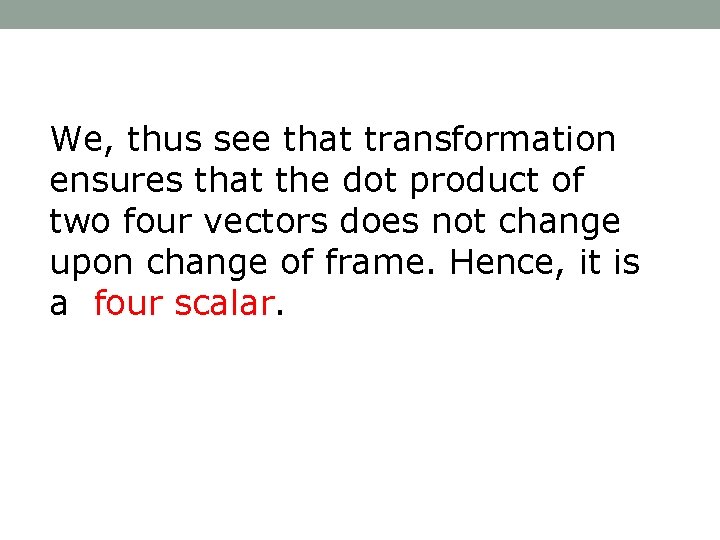
We, thus see that transformation ensures that the dot product of two four vectors does not change upon change of frame. Hence, it is a four scalar.

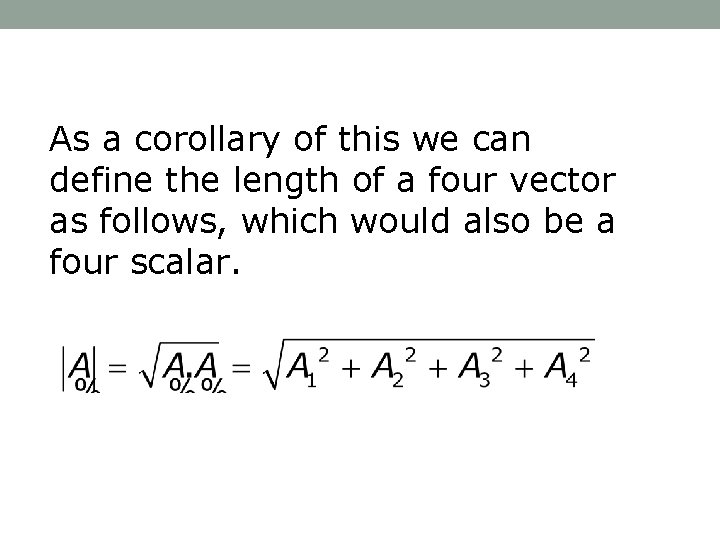
As a corollary of this we can define the length of a four vector as follows, which would also be a four scalar.

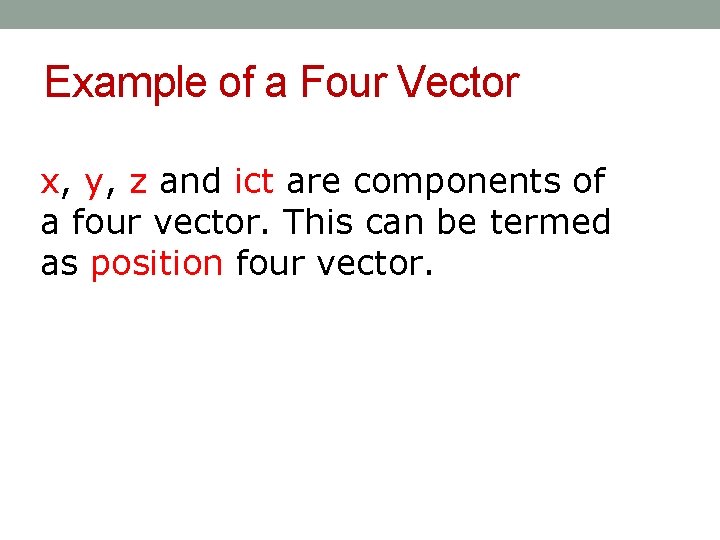
Example of a Four Vector x, y, z and ict are components of a four vector. This can be termed as position four vector.

Alternate Ways 1. Avoid imaginary number in transformation equation of four vector and correspondingly changes the definition of dot produce.

2. Put the fourth component as the first component and write transformation equation as follows.

We see that the transformation resembles rotation in Minkowski Space.

Displacement Four Vector Imagine an event occurs at a position x 1, y 1, z 1 at a time t 1. Let at time t 2, another event occurs, the co-ordinates of which are x 2, y 2, z 2 in a frame S.

Δx=x 2 -x 1, Δy=y 2 -y 1, Δz=z 2 -z 1 and icΔt=ic(t 2 -t 1) shall also be components of four vector. This can be termed as displacement four vector.

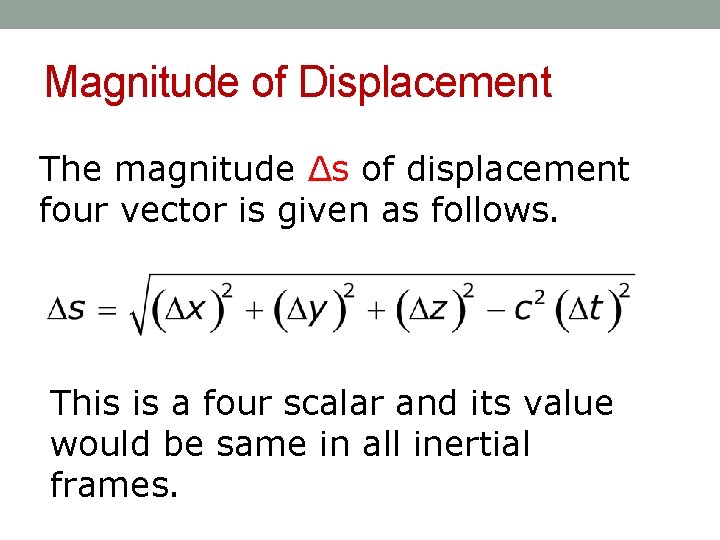
Magnitude of Displacement The magnitude Δs of displacement four vector is given as follows. This is a four scalar and its value would be same in all inertial frames.

Proper Time Interval The proper time interval Δ , another four scalar, between these two events is given as follows.

1. This definition is more general definition of proper time interval and is consistent with the older definition. 2. For space like separated events the proper time interval is imaginary. It is real for time like separated events.

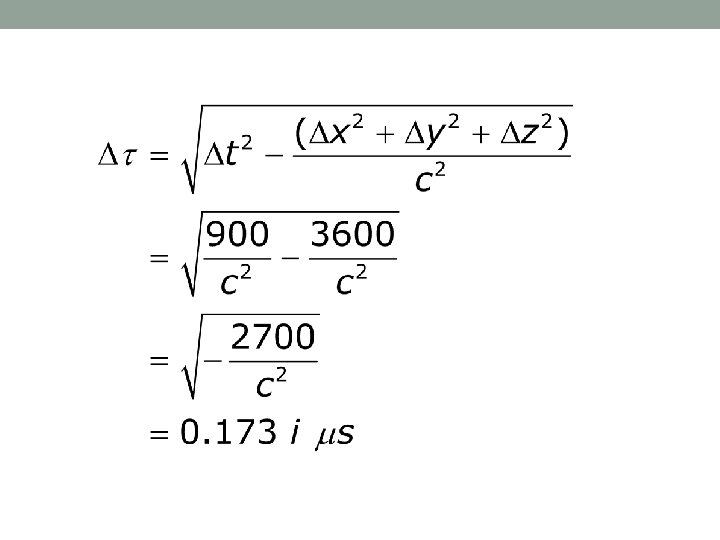
Example 1 Consider Two events in a frame S at the following co-ordinates. E 1: x 1=150 m, t 1=0. 3 s E 2: x 2=210 m, t 2=0. 4 s

Another Frame S’ Consider a frame S’ moving relative to S, with a velocity 0. 6 c.

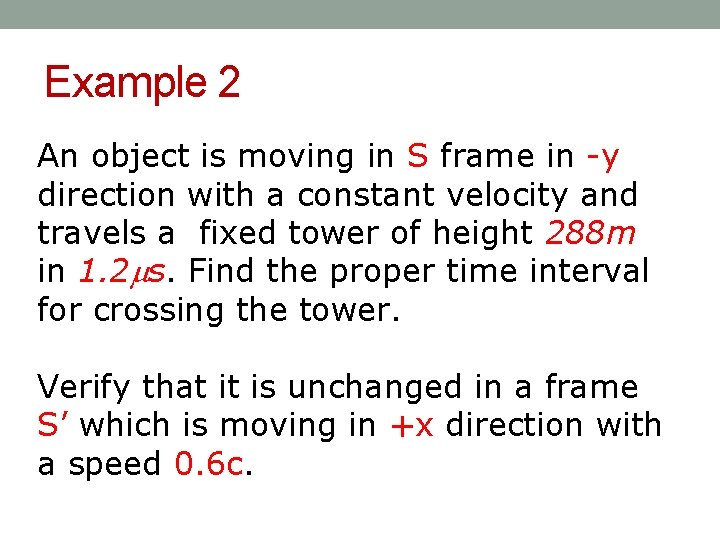
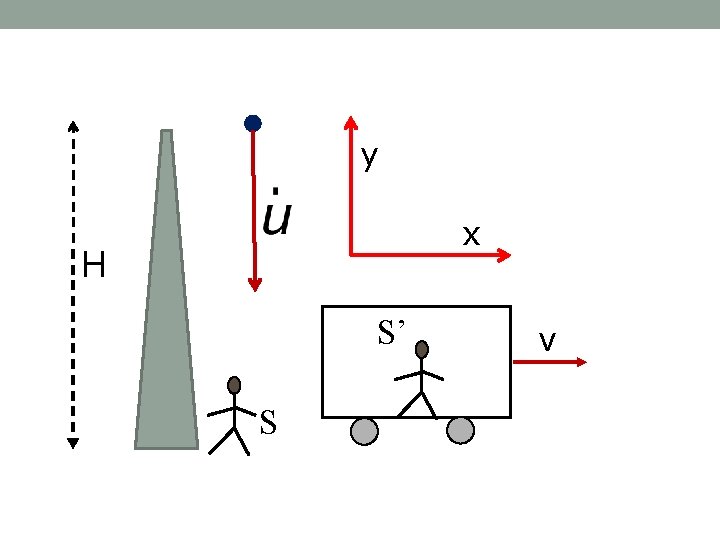
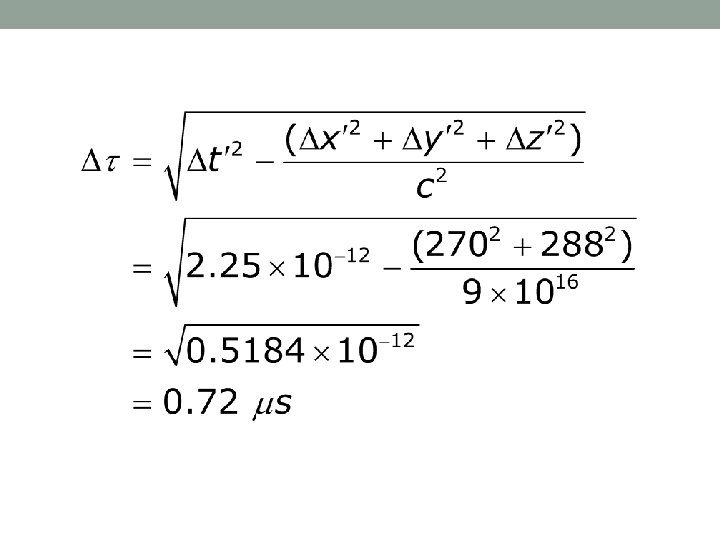
Example 2 An object is moving in S frame in -y direction with a constant velocity and travels a fixed tower of height 288 m in 1. 2 s. Find the proper time interval for crossing the tower. Verify that it is unchanged in a frame S’ which is moving in +x direction with a speed 0. 6 c.

y x H S’ S v

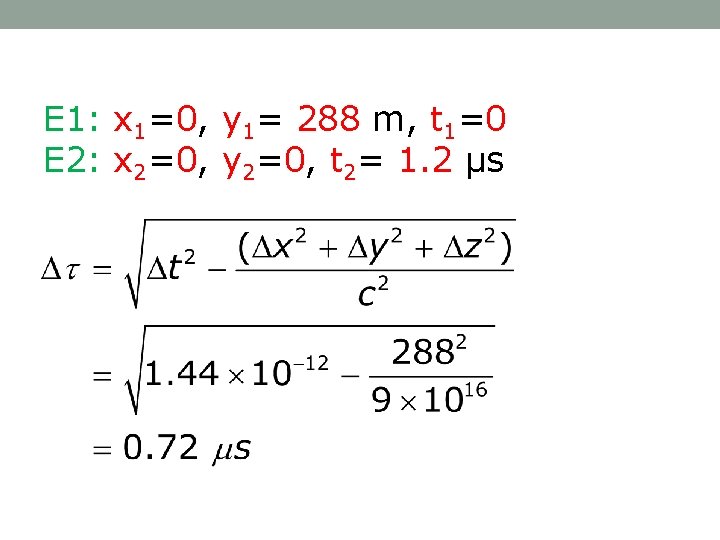
E 1: x 1=0, y 1= 288 m, t 1=0 E 2: x 2=0, y 2=0, t 2= 1. 2 μs

In S’

Note We note that, even though Δt is not a proper time interval The use of time dilation formula requires only x co-ordinate to be same.

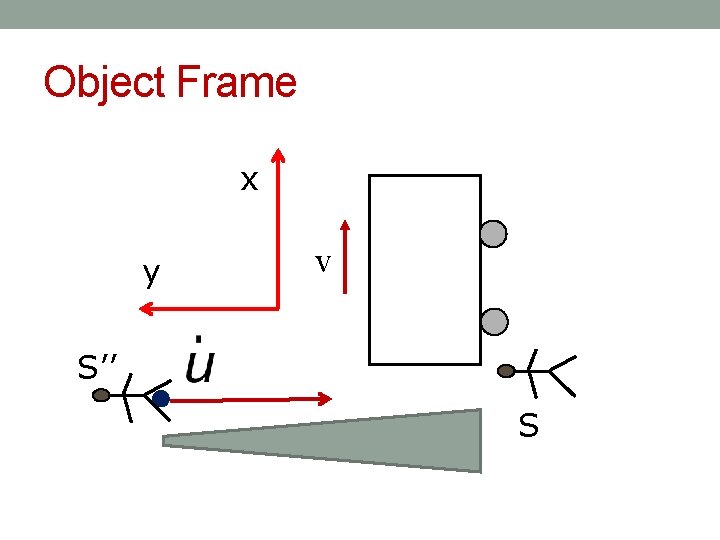
Object Frame x y v S’’ S

We see that the relative velocity of frame S” is in –y direction. The Lorentz transformation for this case would, therefore, be written as follows.

Time Dilation S” to S

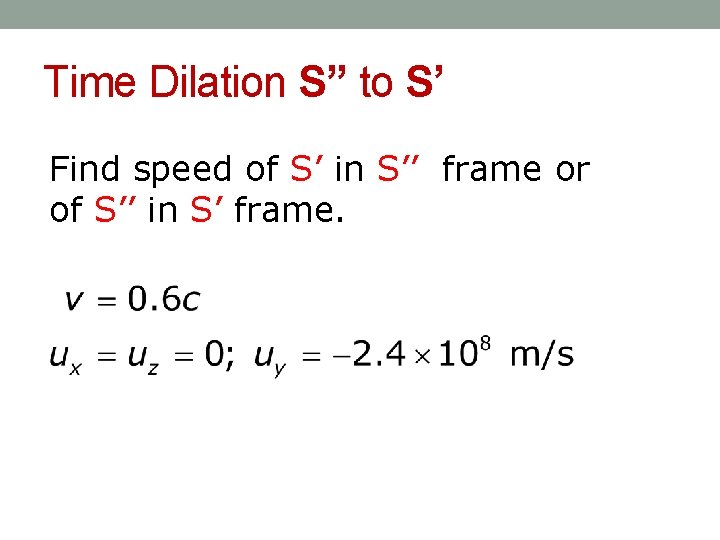
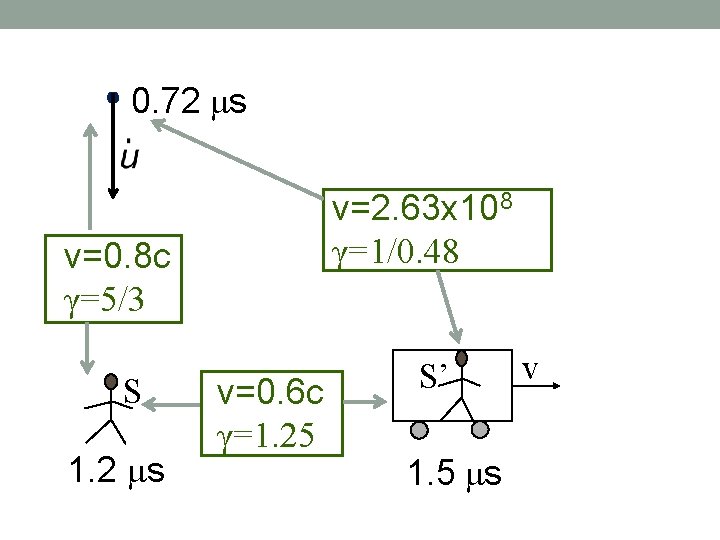
Time Dilation S” to S’ Find speed of S’ in S’’ frame or of S’’ in S’ frame.

0. 72 μs v=2. 63 x 108 γ=1/0. 48 v=0. 8 c γ=5/3 S 1. 2 μs v=0. 6 c γ=1. 25 S’ 1. 5 μs v

Velocity Four Vector Imagine that the two events are related to motion of a particle. Imagine a particle is located at a position x 1, y 1, z 1 at a time t 1. Later at time t 2, it is found at co-ordinates x 2, y 2, z 2. Both observations are made in a frame S.

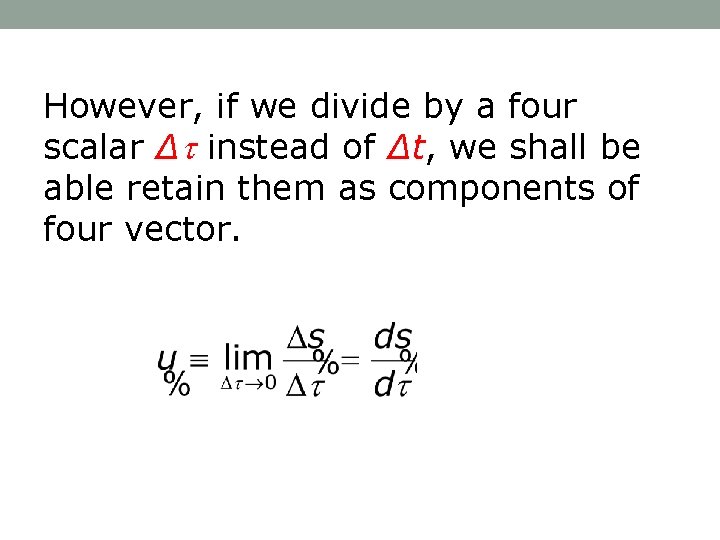
We realize that Δx, Δy, Δz and icΔt are the components of a four vector. However, once they are divided byΔt, they cease to be so.

However, if we divide by a four scalar Δ instead of Δt, we shall be able retain them as components of four vector.

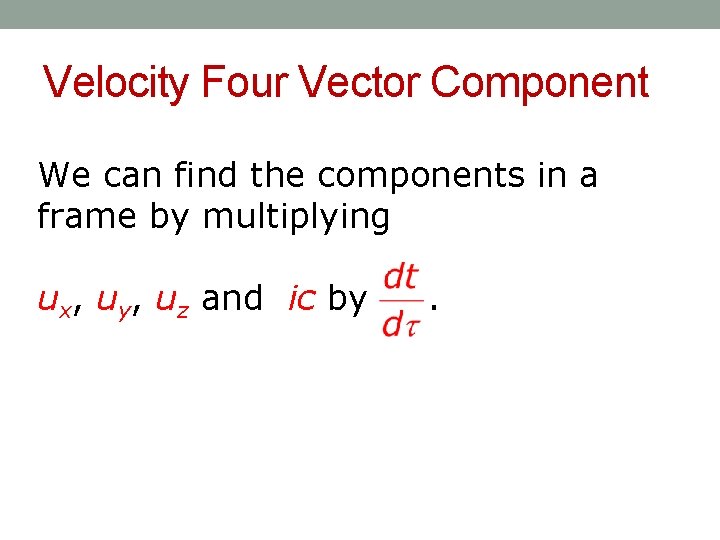
Velocity Four Vector Component We can find the components in a frame by multiplying ux, uy, uz and ic by .

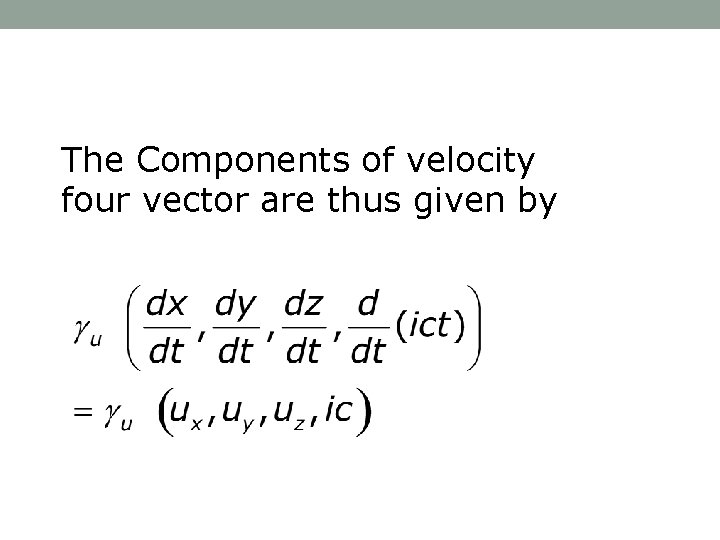
The Components of velocity four vector are thus given by

Transformation