The principle of inclusion and exclusion inversion formulae




![• Theorem 2 [Gauss] Let with. Then Proof: Let Since every integer from • Theorem 2 [Gauss] Let with. Then Proof: Let Since every integer from](https://slidetodoc.com/presentation_image_h/b96c59e946c79e255bff8d1933bc067b/image-24.jpg)



- Slides: 37

The principle of inclusion and exclusion; inversion formulae Speaker: 藍國元 Date: 2005. 11. 28

Outline • • • An overview of principle of inclusion-exclusion Principle of inclusion-exclusion Applications of PIE Möbius Inversion formula Applications of MIF

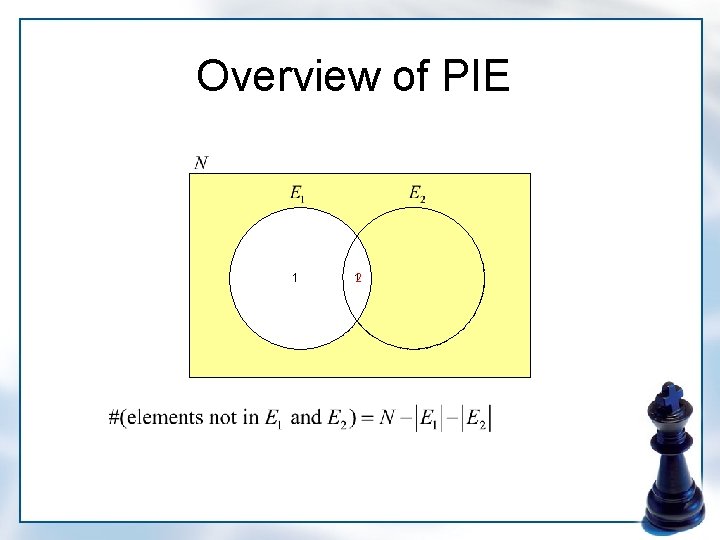
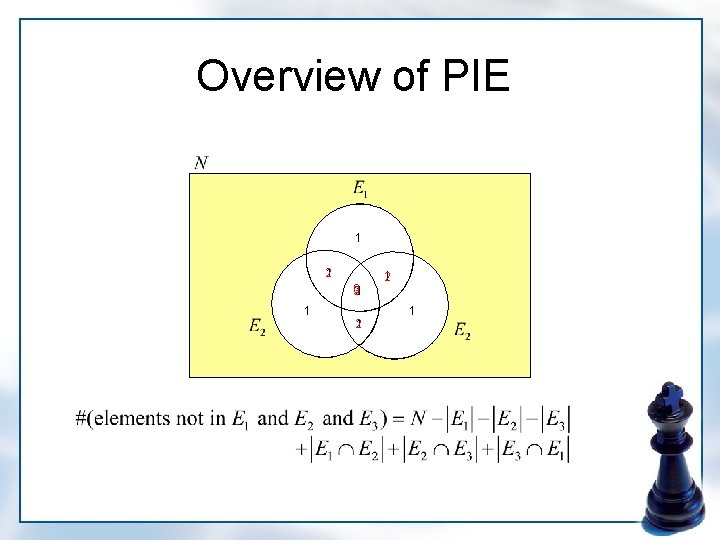
Overview of PIE

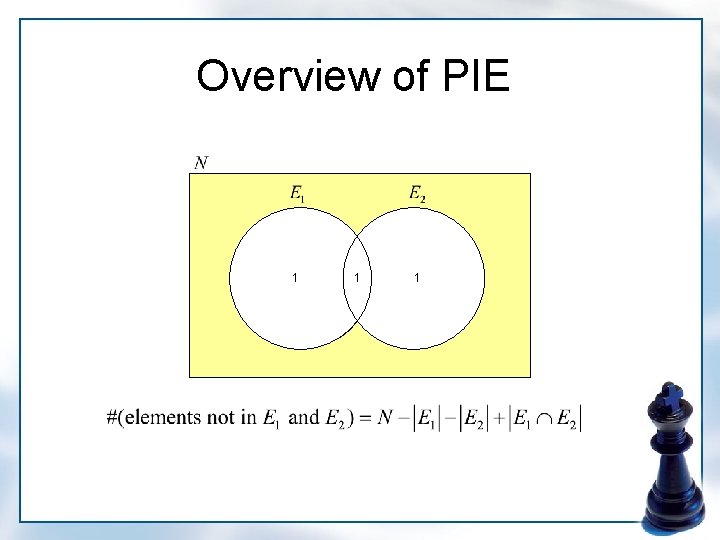
Overview of PIE 1 1

Overview of PIE 1 2 1 1

Overview of PIE 1 1 1

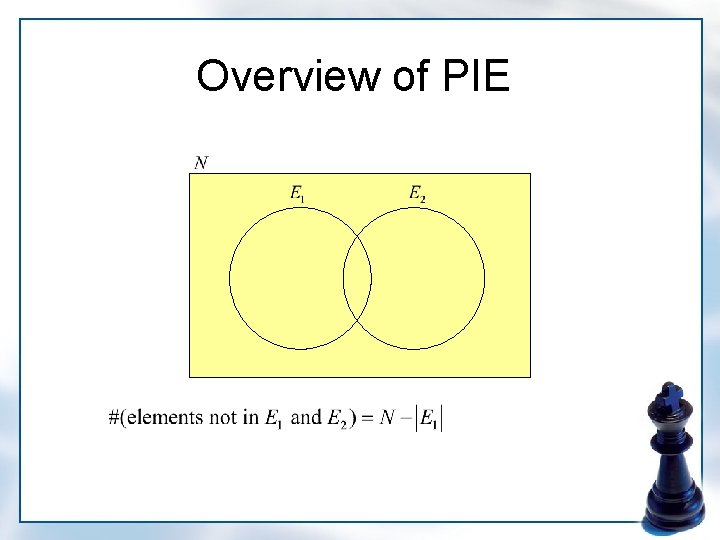
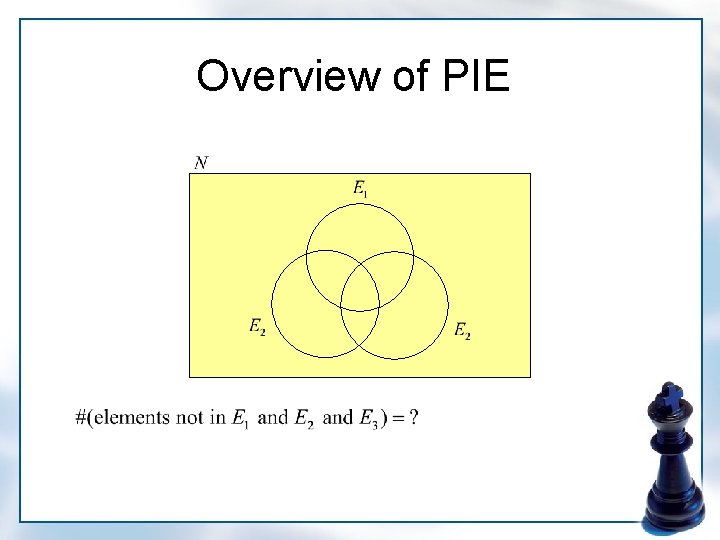
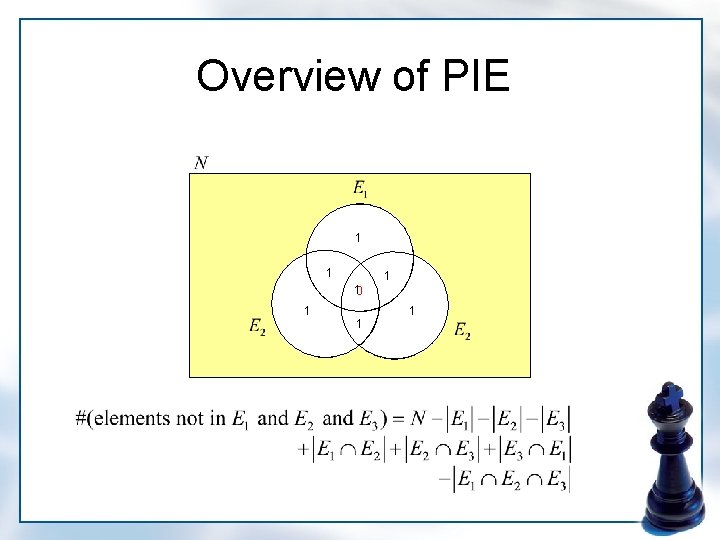
Overview of PIE

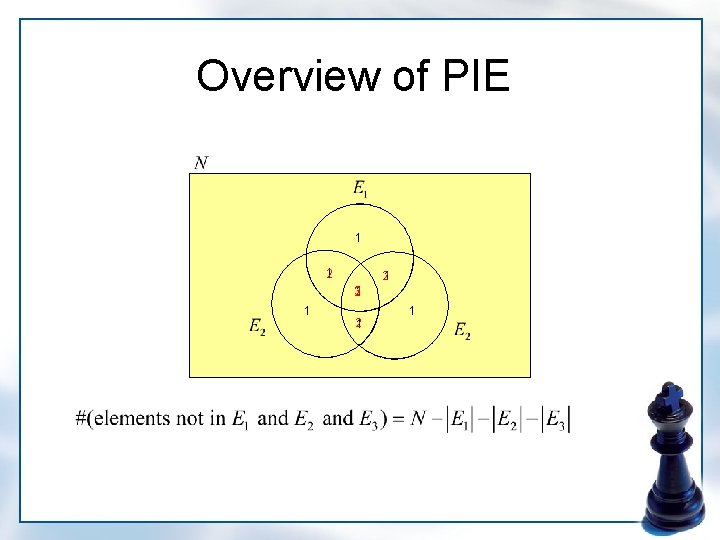
Overview of PIE 1 1 2 21 31 2 1 21 1

Overview of PIE 1 2 1 0 21 3 1 1 21

Overview of PIE 1 10 1 1 1

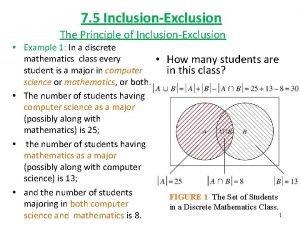
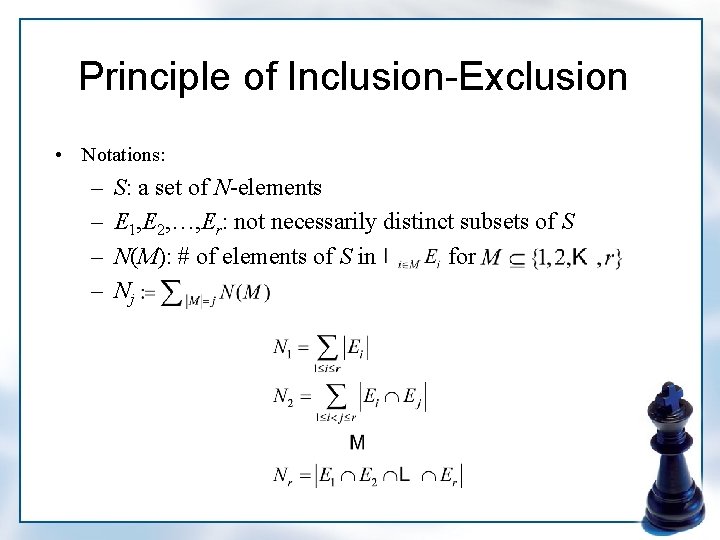
Principle of Inclusion-Exclusion • Notations: – – S: a set of N-elements E 1, E 2, …, Er: not necessarily distinct subsets of S N(M): # of elements of S in for Nj

Principle of Inclusion-Exclusion • Theorem 1 (PIE) Proof: Case 1. and is in none of contributes 1 to left-hand side and right-hand side. Case 2. and is in exactly k of the sets contributes 0 to left-hand. The contribution of to right-hand side equals

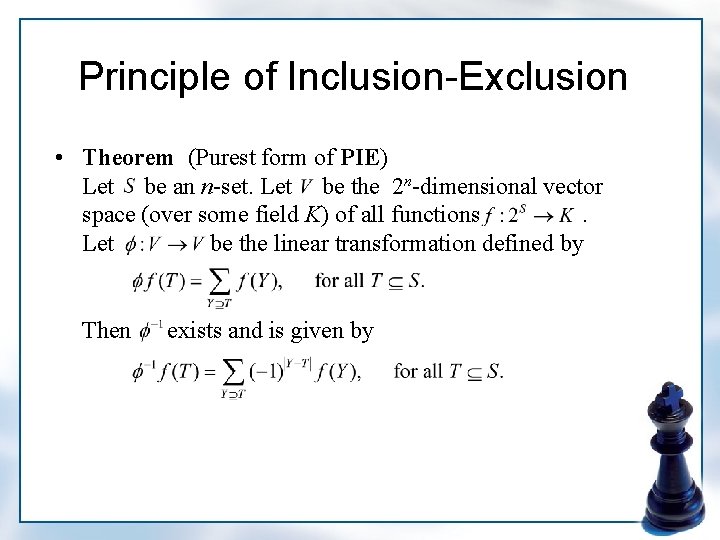
Principle of Inclusion-Exclusion • Theorem (Purest form of PIE) Let be an n-set. Let be the 2 n-dimensional vector space (over some field K) of all functions. Let be the linear transformation defined by Then exists and is given by

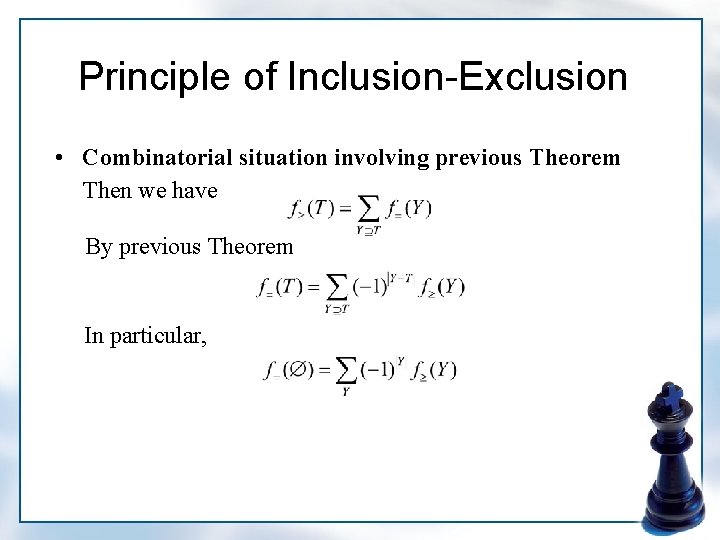
Principle of Inclusion-Exclusion • Combinatorial situation involving previous Theorem : given set : properties set : subset of : # of objects in that have exactly the properties in : # of objects in that have at least the properties in

Principle of Inclusion-Exclusion • Combinatorial situation involving previous Theorem Then we have By previous Theorem In particular,

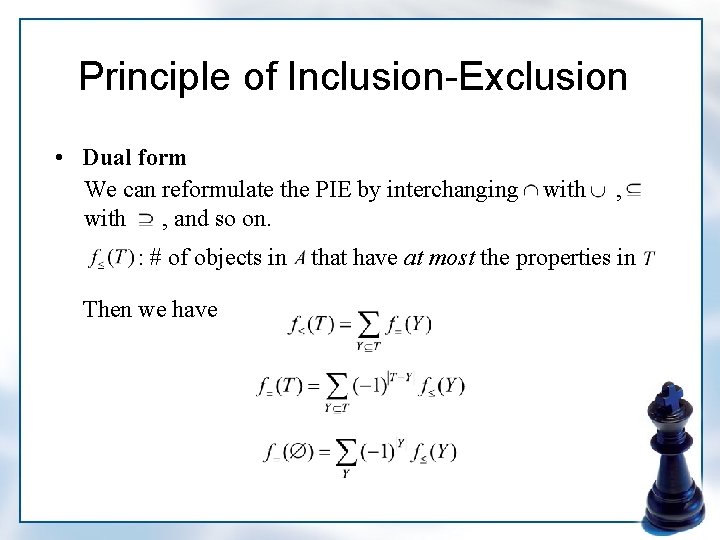
Principle of Inclusion-Exclusion • Dual form We can reformulate the PIE by interchanging with , and so on. : # of objects in Then we have , that have at most the properties in

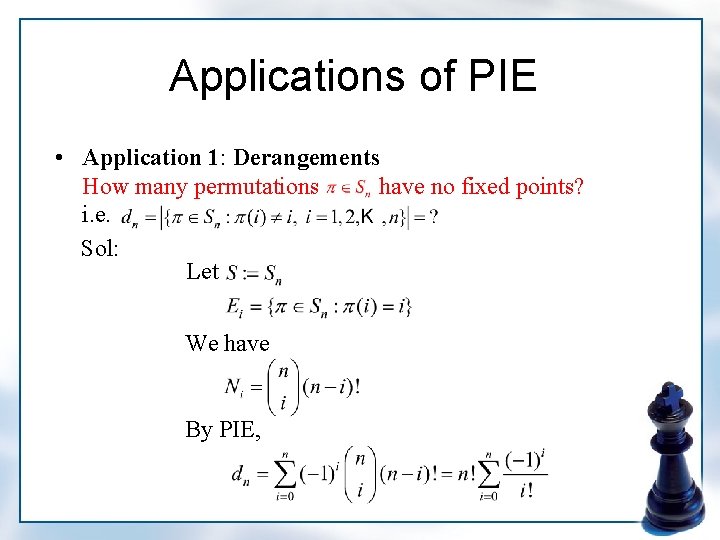
Applications of PIE • Application 1: Derangements How many permutations have no fixed points? i. e. Sol: Let We have By PIE,

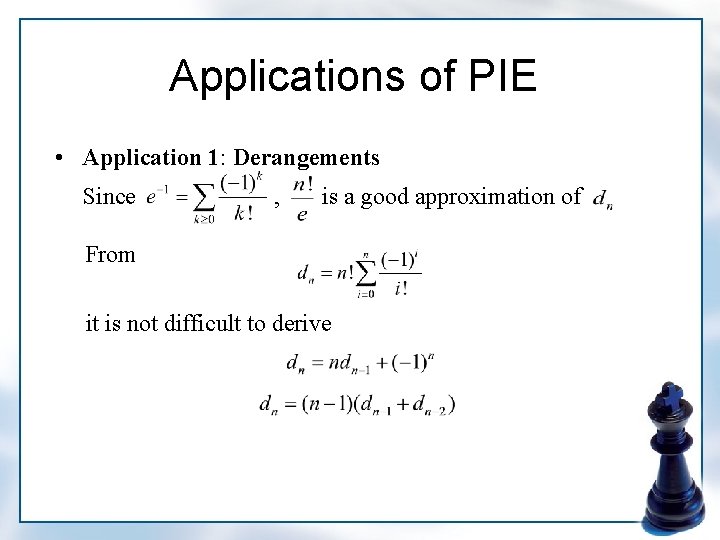
Applications of PIE • Application 1: Derangements Since , is a good approximation of From it is not difficult to derive

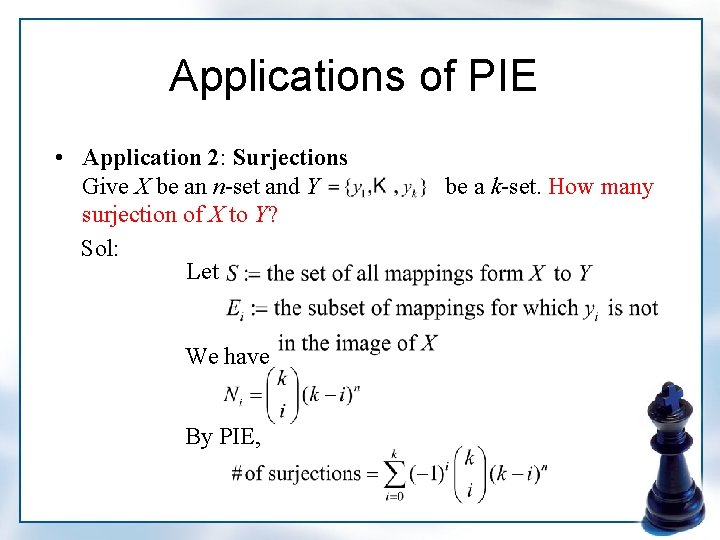
Applications of PIE • Application 2: Surjections Give X be an n-set and Y surjection of X to Y? Sol: Let We have By PIE, be a k-set. How many

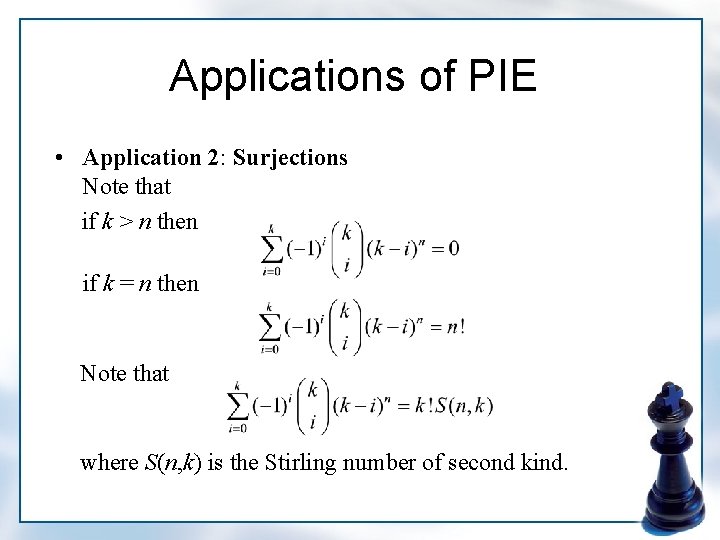
Applications of PIE • Application 2: Surjections Note that if k > n then if k = n then Note that where S(n, k) is the Stirling number of second kind.

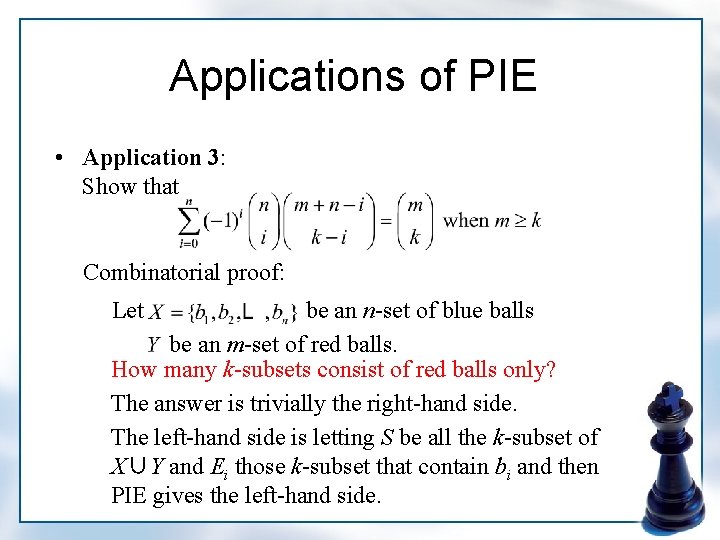
Applications of PIE • Application 3: Show that Combinatorial proof: Let be an n-set of blue balls be an m-set of red balls. How many k-subsets consist of red balls only? The answer is trivially the right-hand side. The left-hand side is letting S be all the k-subset of X∪Y and Ei those k-subset that contain bi and then PIE gives the left-hand side.

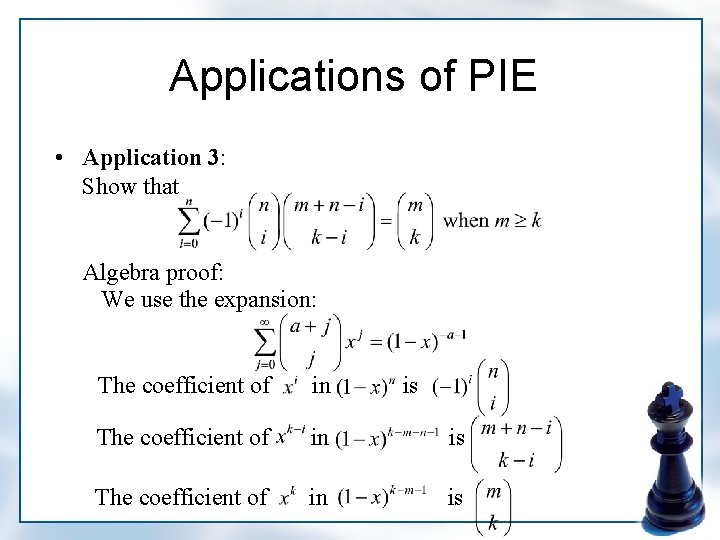
Applications of PIE • Application 3: Show that Algebra proof: We use the expansion: The coefficient of in is

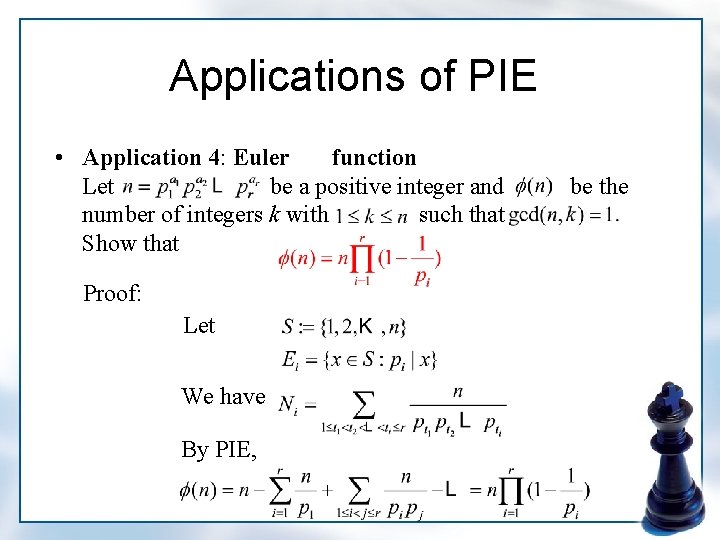
Applications of PIE • Application 4: Euler function Let be a positive integer and number of integers k with such that Show that Proof: Let We have By PIE, be the
![Theorem 2 Gauss Let with Then Proof Let Since every integer from • Theorem 2 [Gauss] Let with. Then Proof: Let Since every integer from](https://slidetodoc.com/presentation_image_h/b96c59e946c79e255bff8d1933bc067b/image-24.jpg)
• Theorem 2 [Gauss] Let with. Then Proof: Let Since every integer from 1 to n belongs only one , we have Since d runs all positive divisor of n, we have

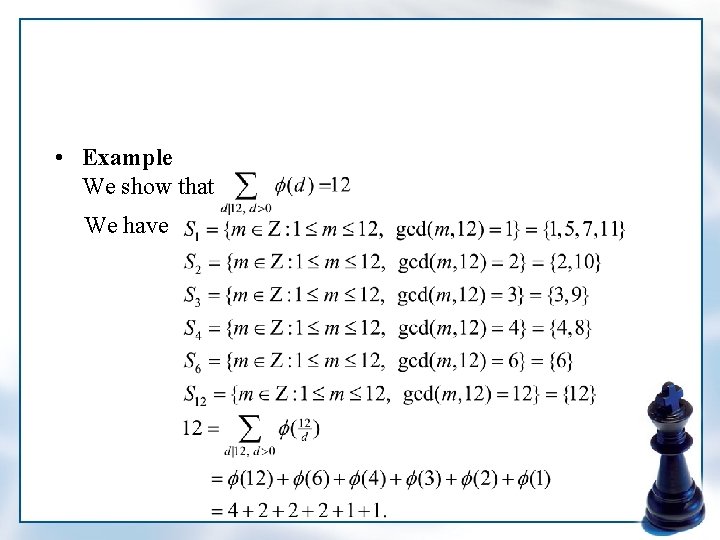
• Example We show that We have

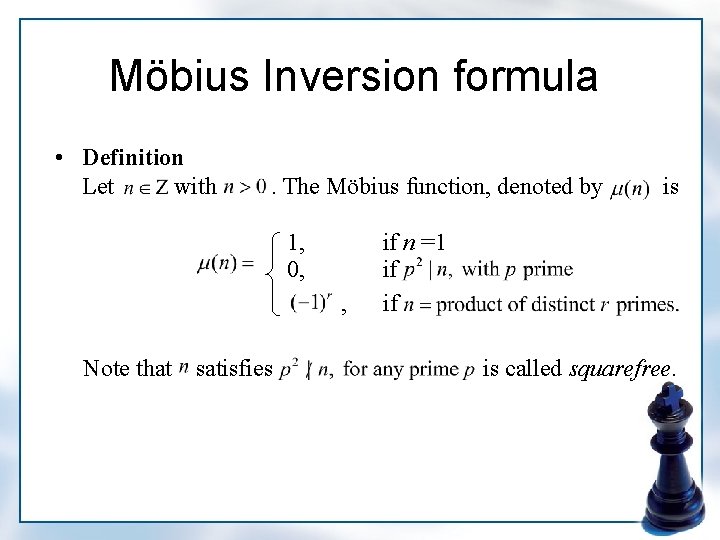
Möbius Inversion formula • Definition Let with . The Möbius function, denoted by 1, 0, , Note that satisfies is if n =1 if if is called squarefree.

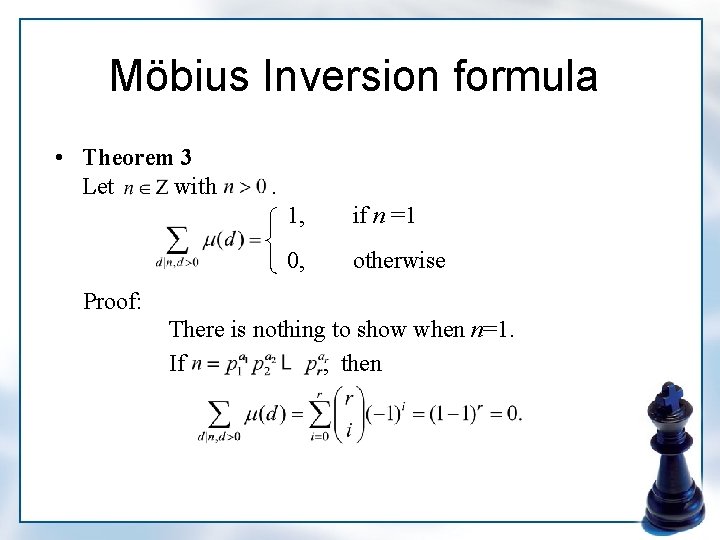
Möbius Inversion formula • Theorem 3 Let with . 1, if n =1 0, otherwise Proof: There is nothing to show when n=1. If , then

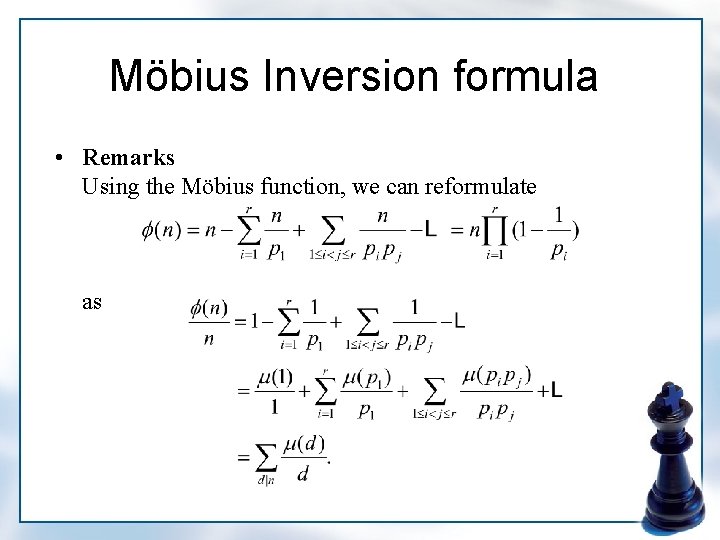
Möbius Inversion formula • Remarks Using the Möbius function, we can reformulate as

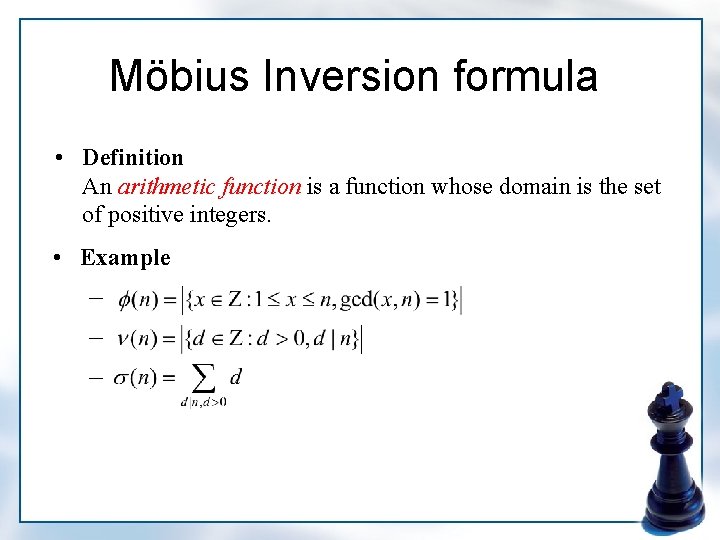
Möbius Inversion formula • Definition An arithmetic function is a function whose domain is the set of positive integers. • Example – – –

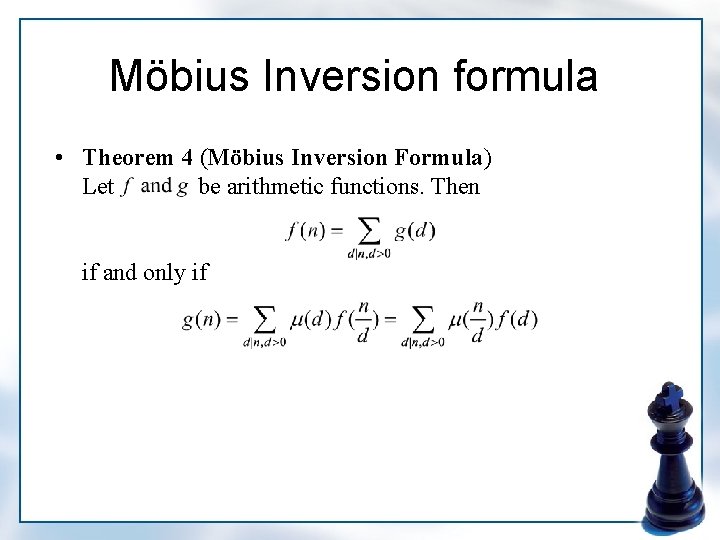
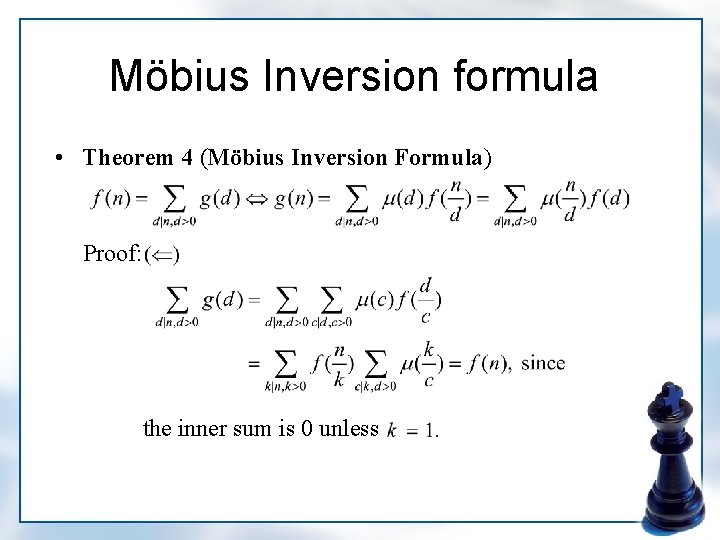
Möbius Inversion formula • Theorem 4 (Möbius Inversion Formula) Let be arithmetic functions. Then if and only if

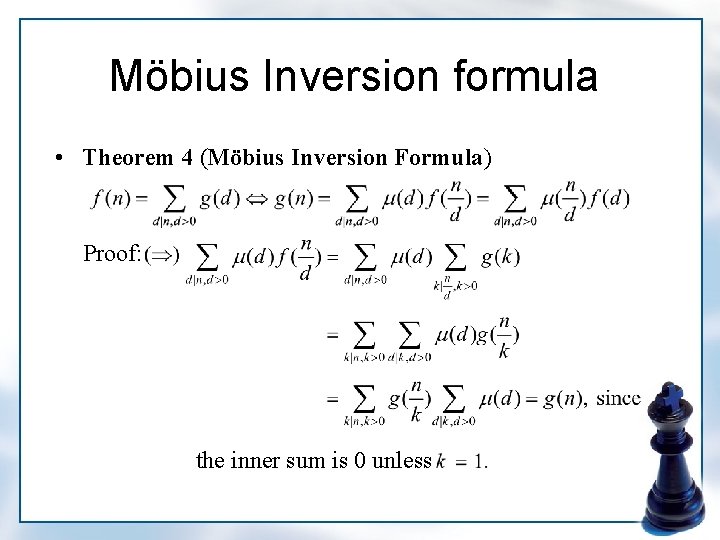
Möbius Inversion formula • Theorem 4 (Möbius Inversion Formula) Proof: the inner sum is 0 unless

Möbius Inversion formula • Theorem 4 (Möbius Inversion Formula) Proof: the inner sum is 0 unless

Applications of MIF • The Möbius Inversion Formula can be used to obtain nontrivial identities among arithmetic functions from trivial identities among arithmetic functions.

Applications of MIF • Example 1 Let with and let for all such n. We have By MIF, we obtain the nontrivial identity or , equivalently

Applications of MIF • Example 2 Let with and let . We have By MIF, we obtain the nontrivial identity

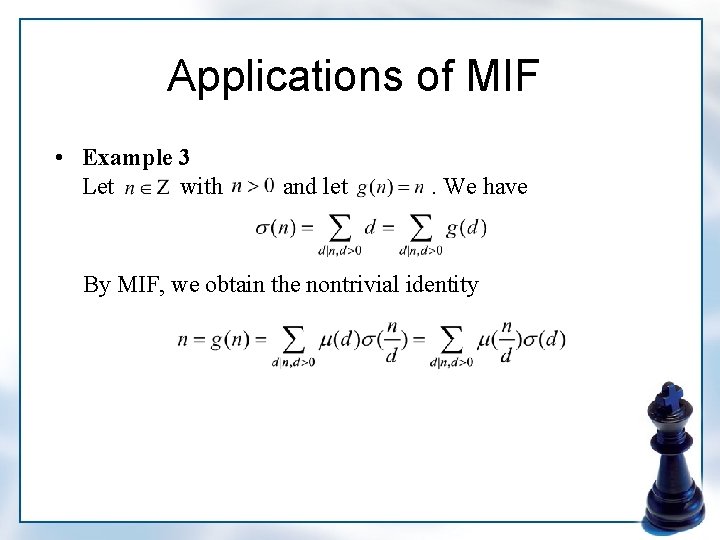
Applications of MIF • Example 3 Let with and let . We have By MIF, we obtain the nontrivial identity

-END-
 Inclusion exclusion principle proof
Inclusion exclusion principle proof Inclusion-exclusion principle
Inclusion-exclusion principle Inclusion-exclusion principle
Inclusion-exclusion principle Inclusion exclusion principle
Inclusion exclusion principle Inclusion-exclusion principle exercises
Inclusion-exclusion principle exercises Inclusion-exclusion principle exercises
Inclusion-exclusion principle exercises Example of statement of the problem
Example of statement of the problem What is inclusion and exclusion criteria
What is inclusion and exclusion criteria What is the inclusion and exclusion criteria in research
What is the inclusion and exclusion criteria in research Inclusion y exclusion
Inclusion y exclusion Criterios de inclusión y exclusión sampieri
Criterios de inclusión y exclusión sampieri Criterios de inclusión y exclusión sampieri
Criterios de inclusión y exclusión sampieri Inclusion y exclusion
Inclusion y exclusion State pauli's exclusion principle class 11
State pauli's exclusion principle class 11 Gel filtration calibration kit
Gel filtration calibration kit Pauli exclusion principal
Pauli exclusion principal Pauli exclusion principle electron configuration
Pauli exclusion principle electron configuration Electron configuration
Electron configuration Orbital notation for oxygen
Orbital notation for oxygen Ricart agrawala second algorithm
Ricart agrawala second algorithm Pauli exclusion principle examples
Pauli exclusion principle examples Pauli exclusion principle class 11
Pauli exclusion principle class 11 Pauli exclusion principle
Pauli exclusion principle Pauli exclusion principle violation
Pauli exclusion principle violation Sequence and series all formula
Sequence and series all formula La quantité de matière
La quantité de matière Mole forumla
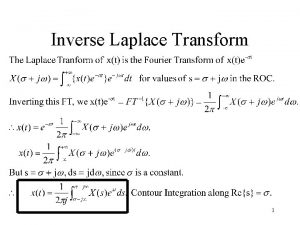
Mole forumla Inverse laplace formula
Inverse laplace formula Transposition of equations
Transposition of equations Suvat formulas
Suvat formulas Dr frost substitution
Dr frost substitution Bcdefgab
Bcdefgab Dr frost maths completing the square
Dr frost maths completing the square Sequences dr frost
Sequences dr frost Global consumer culture positioning example
Global consumer culture positioning example Formula of interest
Formula of interest Suvat formulae
Suvat formulae Hc roi
Hc roi