MAT 360 Lecture 8 Neutral Geometry Remarks n

MAT 360 Lecture 8 Neutral Geometry

Remarks n n n Midterm Grades Next Tuesday: Sketchpad homework 11/13: Chapter 4: 1, 3, 4, 5, 6 15 (estimated) 11/20: Sketchpad project 11/27: Proof project: q q q n Choose one of theorems we studied and write a detailed proof. The proof should have at least 10 steps. Each step must be numbered and justified. 12/13: Chapter 5: 8 Chapter 6: : 2, 3, 5, 14 (estimated)

Euclid’s postulates (modern formulation) I. II. III. IV. V. For every point P and every point Q not equal to P there exists a unique line l that passes for P and Q. For every segment AB and for every segment CD there exists a unique point E such that B is between A and E and the segment CD is congruent to the segment BE. For every point O and every point A not equal to O there exists a circle with center O and radius OA All right angles are congruent to each other For every line l and for every point P that does not lie on l there exists a unique line m through P that is parallel to l.

In Chapter 3, n n n we will study results that can be proved using only the axioms of incidence, betweenness, congruence and continuity. That is, all Hilbert’s axioms with the exception of the axiom of parallelism. Neutral geometry consists in all the results that can be proved in this way.

Exercise: n Let t be a line intersecting the lines l and l’. Define alternate interior angles.

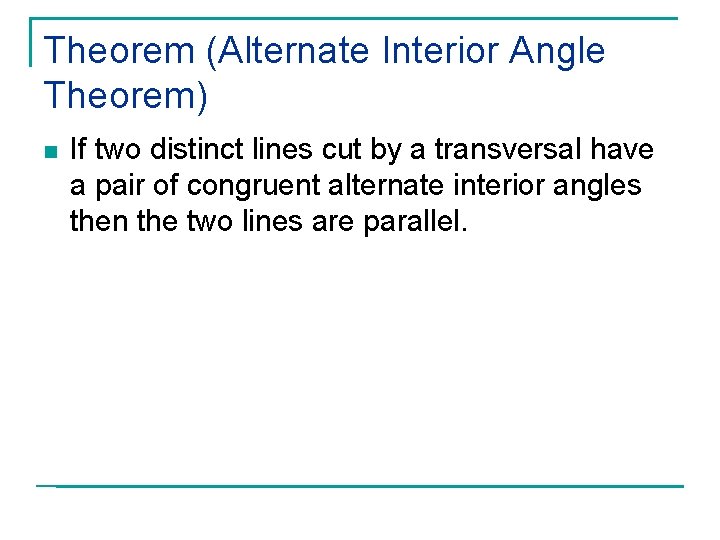
Theorem (Alternate Interior Angle Theorem) n If two distinct lines cut by a transversal have a pair of congruent alternate interior angles then the two lines are parallel.

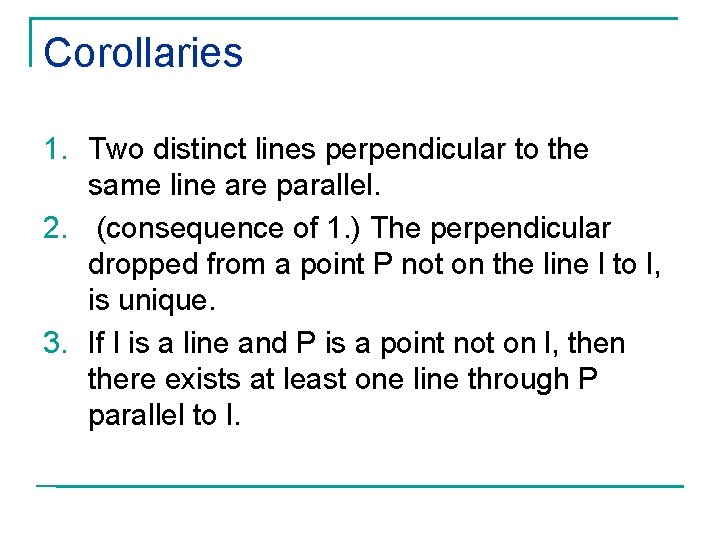
Corollaries 1. Two distinct lines perpendicular to the same line are parallel. 2. (consequence of 1. ) The perpendicular dropped from a point P not on the line l to l, is unique. 3. If l is a line and P is a point not on l, then there exists at least one line through P parallel to l.

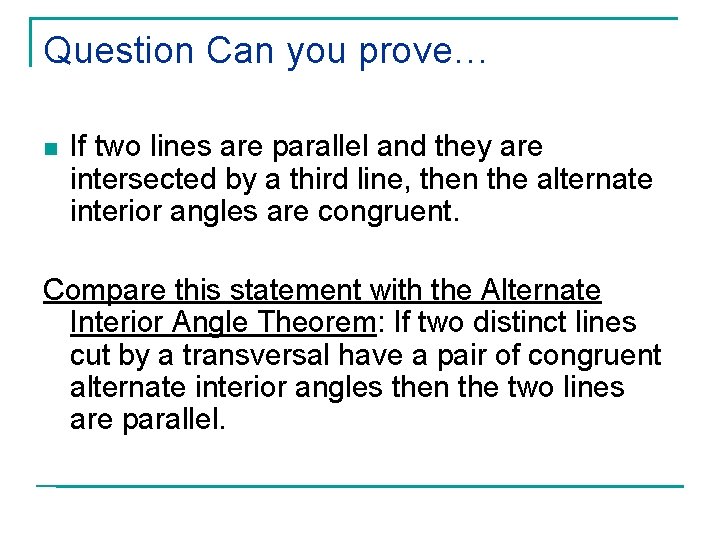
Question Can you prove… n If two lines are parallel and they are intersected by a third line, then the alternate interior angles are congruent. Compare this statement with the Alternate Interior Angle Theorem: If two distinct lines cut by a transversal have a pair of congruent alternate interior angles then the two lines are parallel.

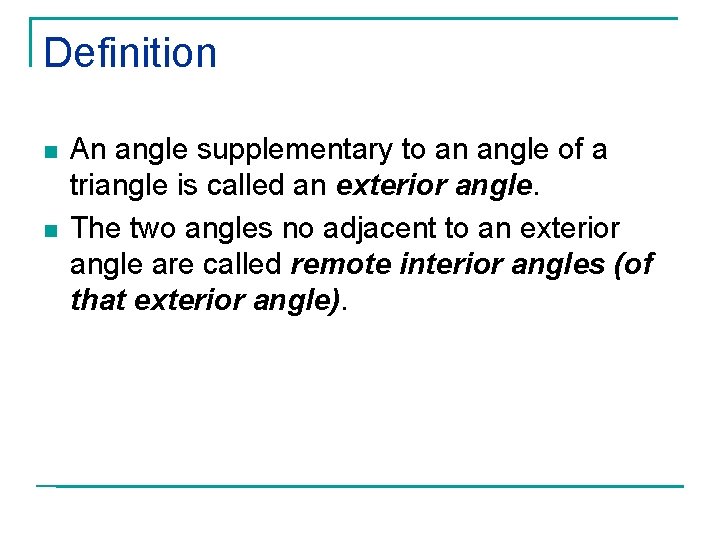
Definition n n An angle supplementary to an angle of a triangle is called an exterior angle. The two angles no adjacent to an exterior angle are called remote interior angles (of that exterior angle).

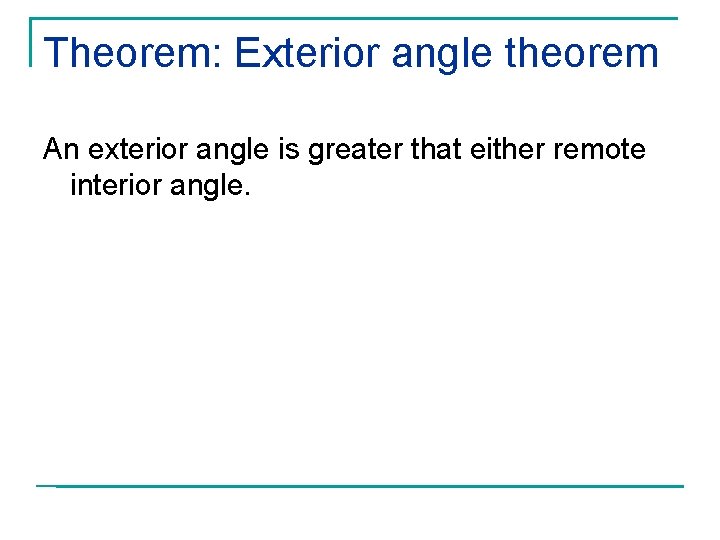
Theorem: Exterior angle theorem An exterior angle is greater that either remote interior angle.

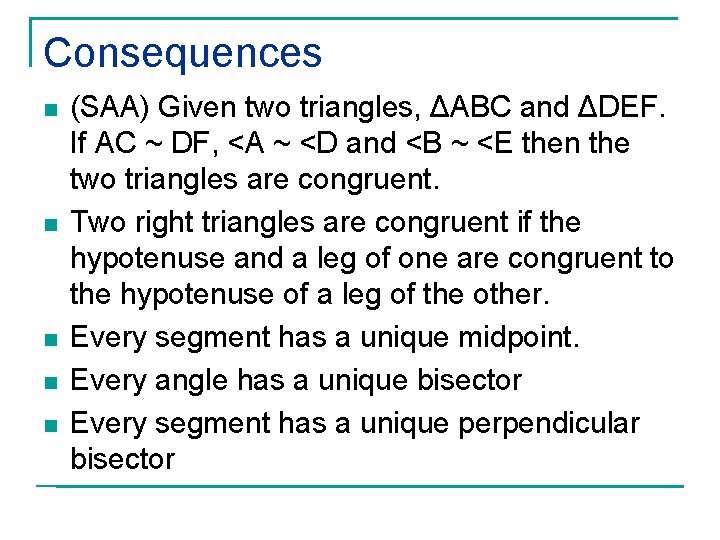
Consequences n n n (SAA) Given two triangles, ΔABC and ΔDEF. If AC ~ DF, <A ~ <D and <B ~ <E then the two triangles are congruent. Two right triangles are congruent if the hypotenuse and a leg of one are congruent to the hypotenuse of a leg of the other. Every segment has a unique midpoint. Every angle has a unique bisector Every segment has a unique perpendicular bisector

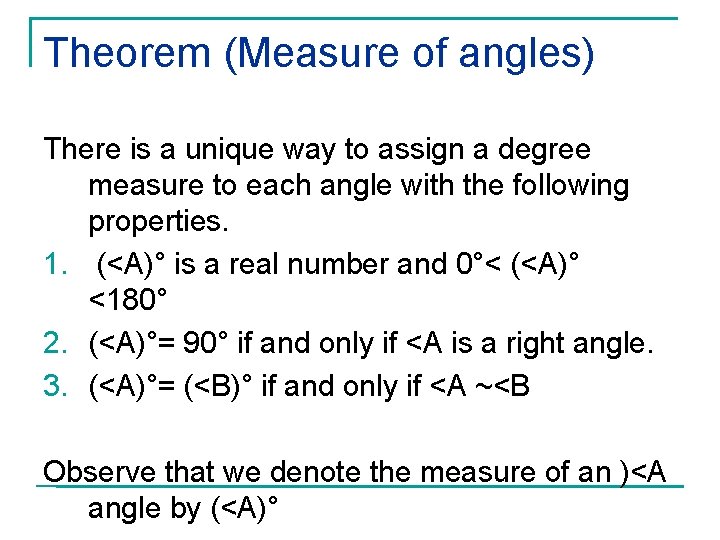
Theorem (Measure of angles) There is a unique way to assign a degree measure to each angle with the following properties. 1. (<A)° is a real number and 0°< (<A)° <180° 2. (<A)°= 90° if and only if <A is a right angle. 3. (<A)°= (<B)° if and only if <A ~<B Observe that we denote the measure of an )<A angle by (<A)°

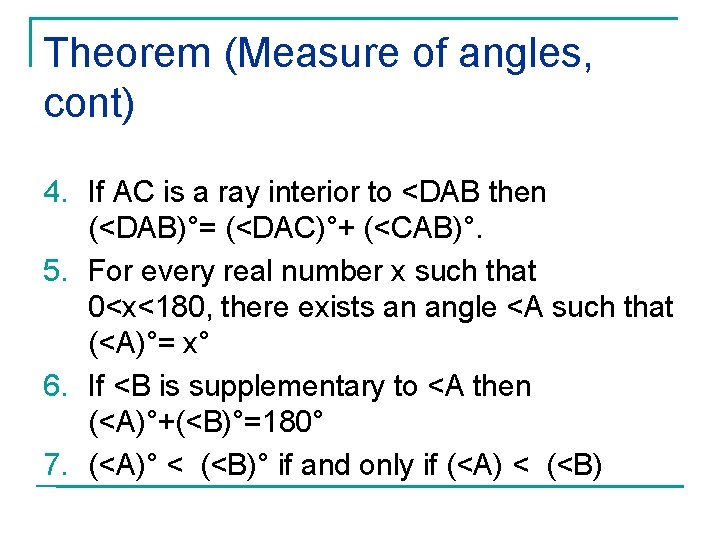
Theorem (Measure of angles, cont) 4. If AC is a ray interior to <DAB then (<DAB)°= (<DAC)°+ (<CAB)°. 5. For every real number x such that 0<x<180, there exists an angle <A such that (<A)°= x° 6. If <B is supplementary to <A then (<A)°+(<B)°=180° 7. (<A)° < (<B)° if and only if (<A) < (<B)

Idea of the proof 1. We “know” which angles measure 90° 2. We can bisect angles, so we find angles that measure 45° and 90 °+45° 3. By bisecting again and “adding” we find angles of 22. 5°, 45°+22. 5°, 90°+22. 5°, 135°+22. 5°, 4. And so on… 5. Using continuity one can show that it is possible to “squeeze” all the other angles between two of the ones above

Theorem (Measure of segments) Given a segment OI (called a unit segment) there is a unique way of assigning a length AB to each segment such that the following property holds 1. OI =1 2. AB is a positive real number 3. AB=CD if and only if AB ~ CD 4. A*B*C if and only if AC=AB+BC 5. AB<CD if and only if AB<CD 6. For every positive real number x, there exist a segment AB such that AB=x.

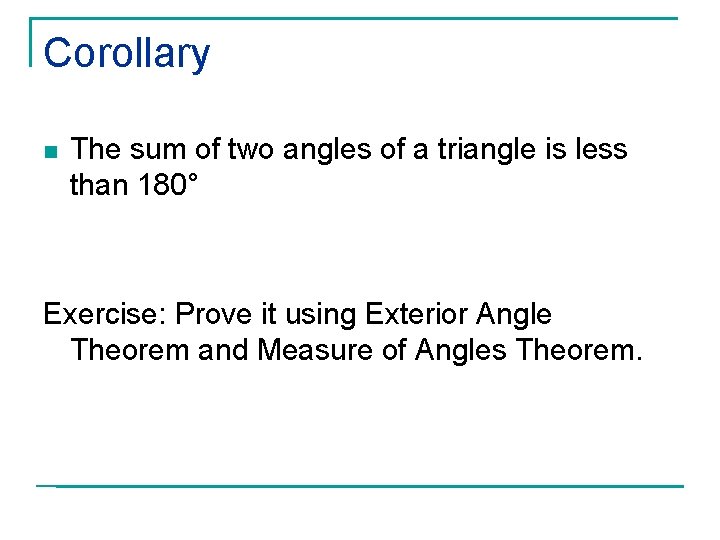
Corollary n The sum of two angles of a triangle is less than 180° Exercise: Prove it using Exterior Angle Theorem and Measure of Angles Theorem.

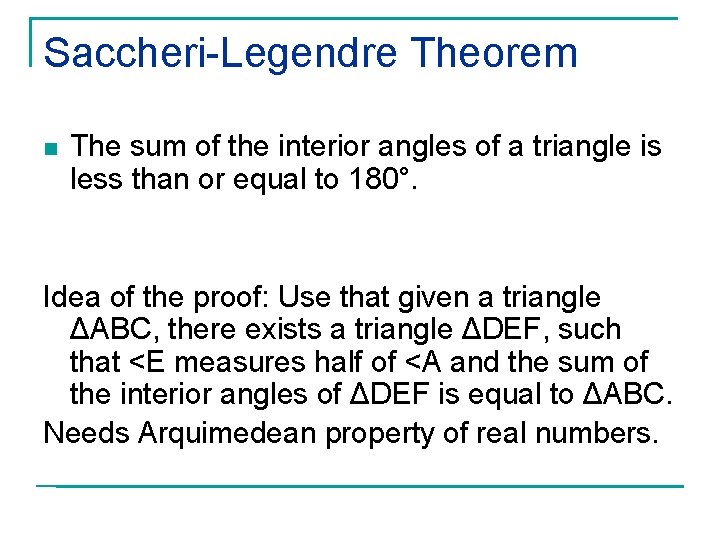
Saccheri-Legendre Theorem n The sum of the interior angles of a triangle is less than or equal to 180°. Idea of the proof: Use that given a triangle ΔABC, there exists a triangle ΔDEF, such that <E measures half of <A and the sum of the interior angles of ΔDEF is equal to ΔABC. Needs Arquimedean property of real numbers.

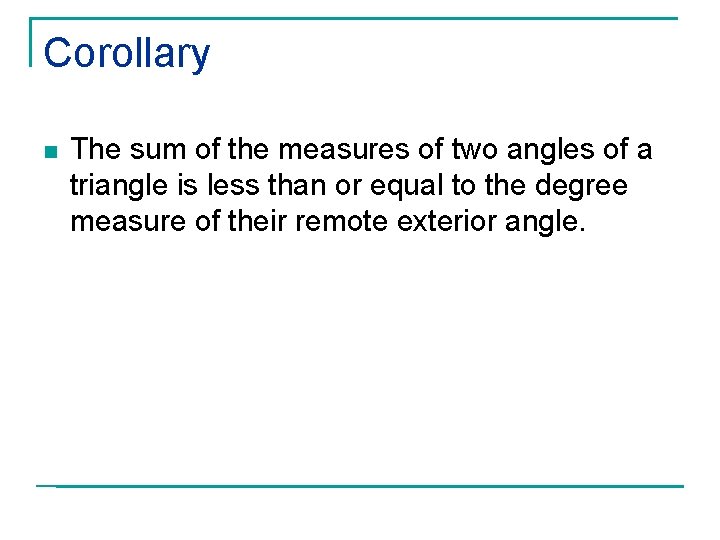
Corollary n The sum of the measures of two angles of a triangle is less than or equal to the degree measure of their remote exterior angle.

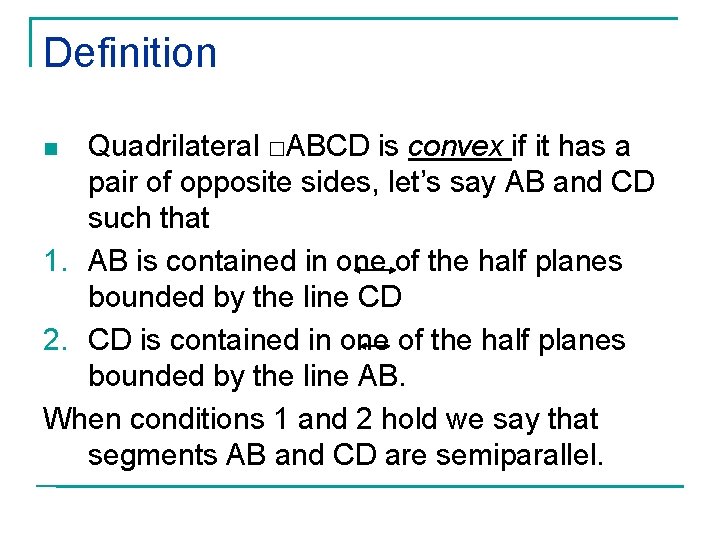
Definition Quadrilateral □ABCD is convex if it has a pair of opposite sides, let’s say AB and CD such that 1. AB is contained in one of the half planes bounded by the line CD 2. CD is contained in one of the half planes bounded by the line AB. When conditions 1 and 2 hold we say that segments AB and CD are semiparallel. n

Corollary of Saccheri-Legendre Theorem n The sum of the interior angles in any convex quadrilateral is less than or equal to 360° Proof: Step 1: Show that if □ABCD is a convex quadrilateral then both pairs of opposite sides are semiparallel. In other words, AB and CD are semiparallel and BC and AD are semiparallel. Step 2: Find triangles to apply Saccheri. Legendre.

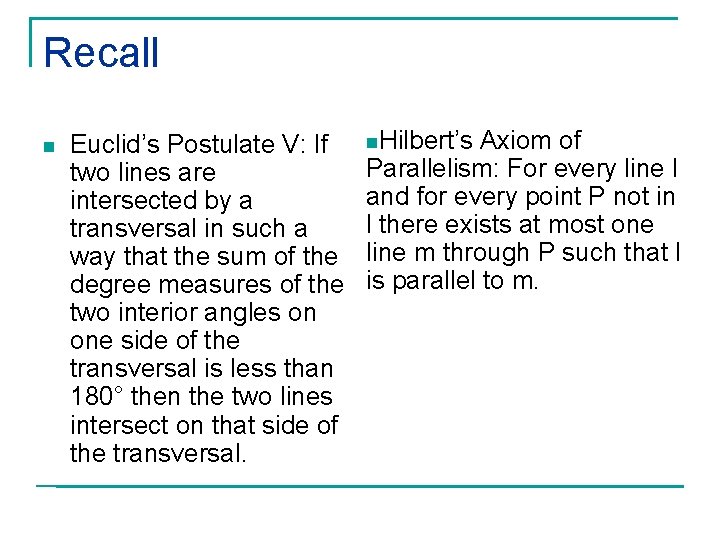
Recall n Euclid’s Postulate V: If two lines are intersected by a transversal in such a way that the sum of the degree measures of the two interior angles on one side of the transversal is less than 180° then the two lines intersect on that side of the transversal. n. Hilbert’s Axiom of Parallelism: For every line l and for every point P not in l there exists at most one line m through P such that l is parallel to m.

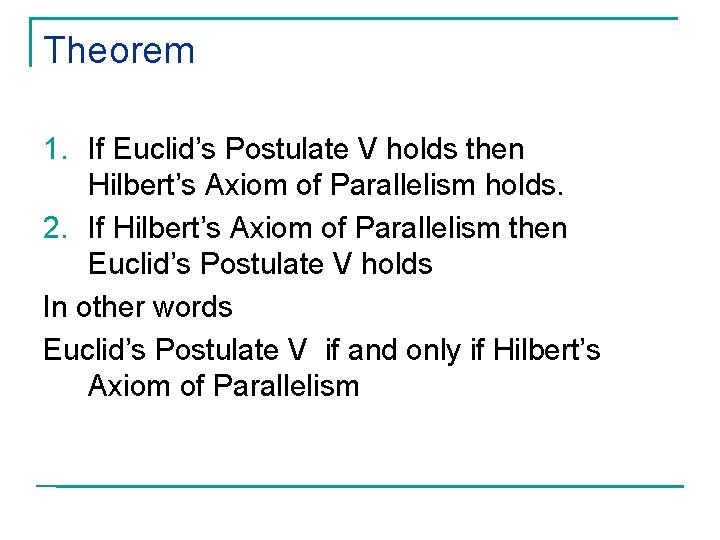
Theorem 1. If Euclid’s Postulate V holds then Hilbert’s Axiom of Parallelism holds. 2. If Hilbert’s Axiom of Parallelism then Euclid’s Postulate V holds In other words Euclid’s Postulate V if and only if Hilbert’s Axiom of Parallelism

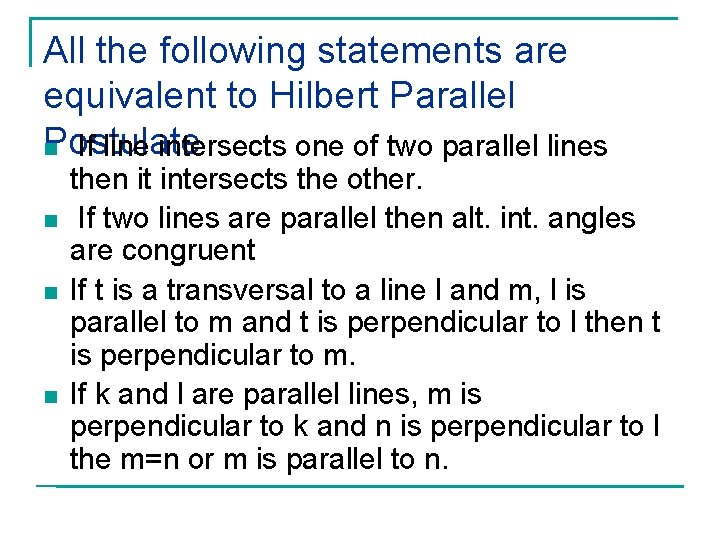
All the following statements are equivalent to Hilbert Parallel Postulate n If line intersects one of two parallel lines n n n then it intersects the other. If two lines are parallel then alt. int. angles are congruent If t is a transversal to a line l and m, l is parallel to m and t is perpendicular to l then t is perpendicular to m. If k and l are parallel lines, m is perpendicular to k and n is perpendicular to l the m=n or m is parallel to n.

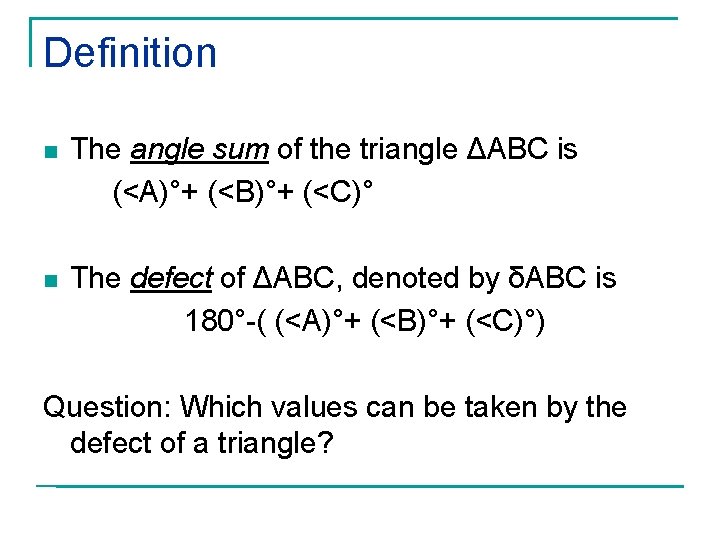
Definition n The angle sum of the triangle ΔABC is (<A)°+ (<B)°+ (<C)° n The defect of ΔABC, denoted by δABC is 180°-( (<A)°+ (<B)°+ (<C)°) Question: Which values can be taken by the defect of a triangle?

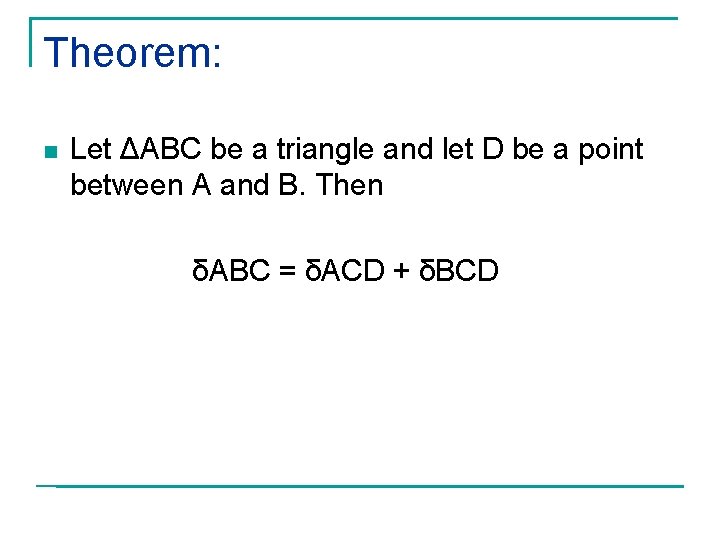
Theorem: n Let ΔABC be a triangle and let D be a point between A and B. Then δABC = δACD + δBCD

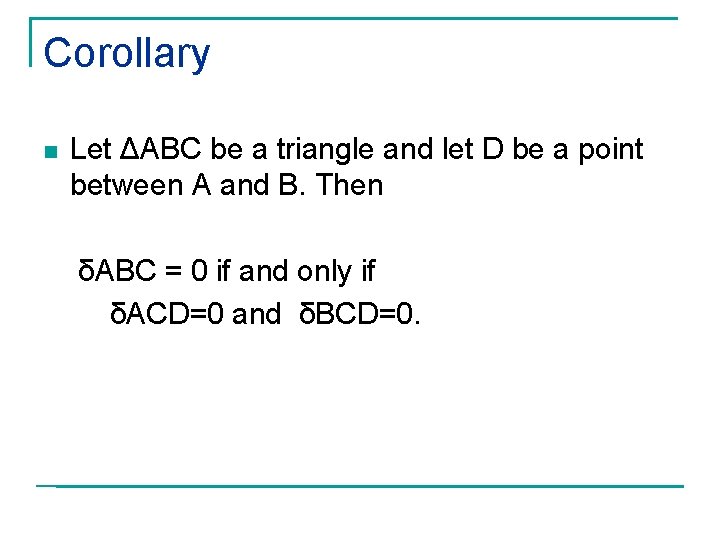
Corollary n Let ΔABC be a triangle and let D be a point between A and B. Then δABC = 0 if and only if δACD=0 and δBCD=0.

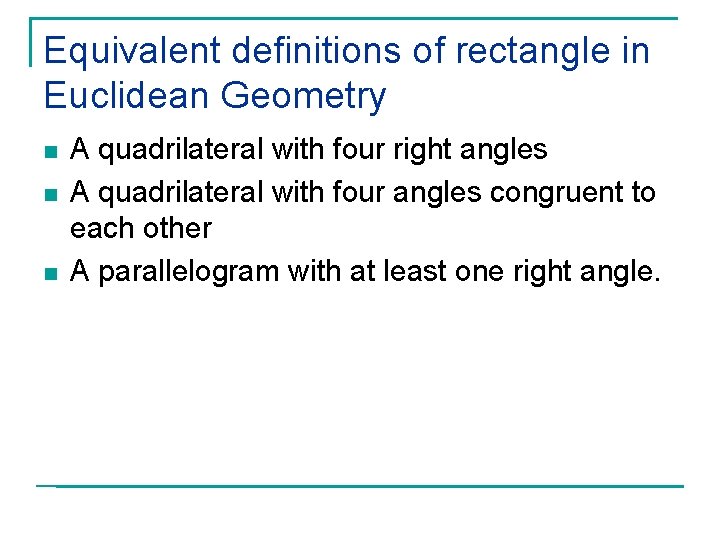
Equivalent definitions of rectangle in Euclidean Geometry n n n A quadrilateral with four right angles A quadrilateral with four angles congruent to each other A parallelogram with at least one right angle.

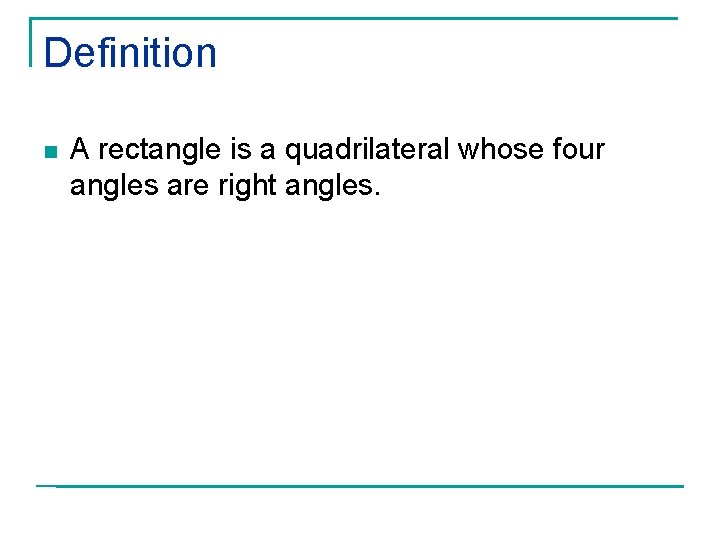
Definition n A rectangle is a quadrilateral whose four angles are right angles.

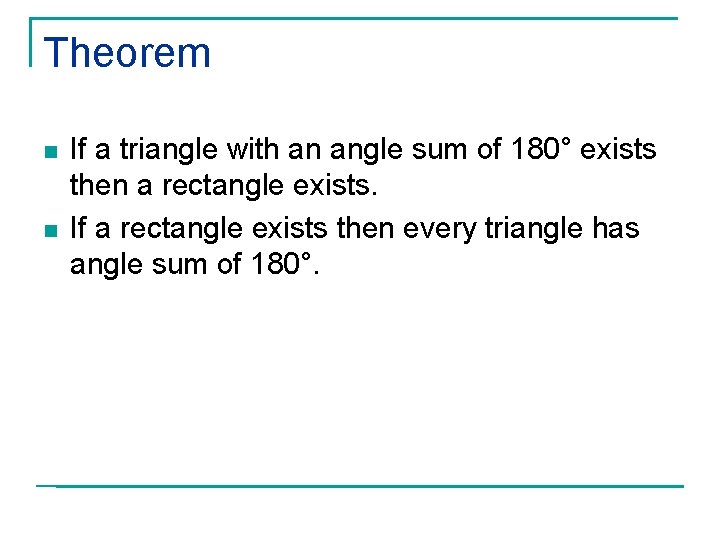
Theorem n n If a triangle with an angle sum of 180° exists then a rectangle exists. If a rectangle exists then every triangle has angle sum of 180°.

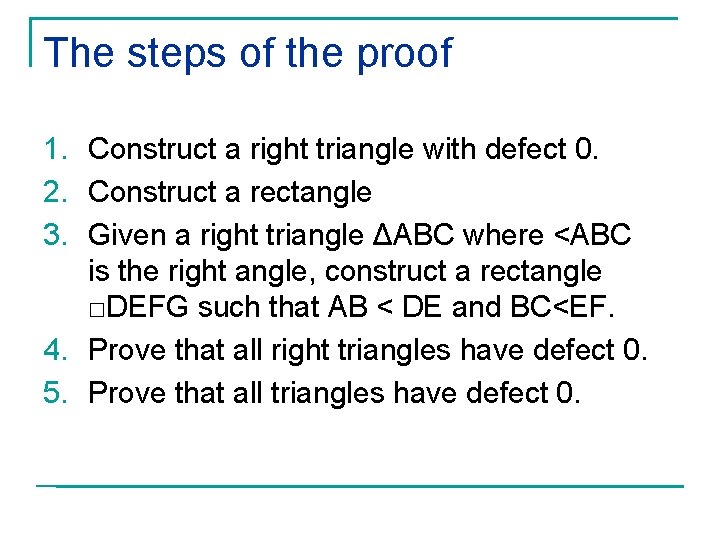
The steps of the proof 1. Construct a right triangle with defect 0. 2. Construct a rectangle 3. Given a right triangle ΔABC where <ABC is the right angle, construct a rectangle □DEFG such that AB < DE and BC<EF. 4. Prove that all right triangles have defect 0. 5. Prove that all triangles have defect 0.