Disorder and chaos in quantum systems II Lecture























- Slides: 55

Disorder and chaos in quantum systems II. Lecture 3. Boris Altshuler Physics Department, Columbia University

Lecture 3. 1. Introduction

Previous Lectures: 1. Anderson Localization as Metal-Insulator Transition Anderson model. Localized and extended states. Mobility edges. 2. Spectral Statistics and Localization. Poisson versus Wigner-Dyson. Anderson transition as a transition between different types of spectra. Thouless conductance 3 Quantum Chaos and Integrability and Localization. Integrable Poisson; Chaotic Wigner-Dyson 4. Anderson transition beyond real space

Previous Lectures: 4. Anderson Localization and Many-Body Spectrum in finite systems. Q: Why nuclear spectra are statistically the same as RM spectra – Wigner-Dyson? A: Delocalization in the Fock space. Q: What is relation of exact Many Body states and quasiparticles? A: Quasiparticles are “wave packets” 5. Anderson Model and Localization on the Cayley tree Ergodic and Nonergodic extended states Wigner – Dyson statistics requires ergodicity!

Definition: We will call a quantum state ergodic if it occupies the number of sites on the Anderson lattice, which is proportional to the total number of sites : nonergodic

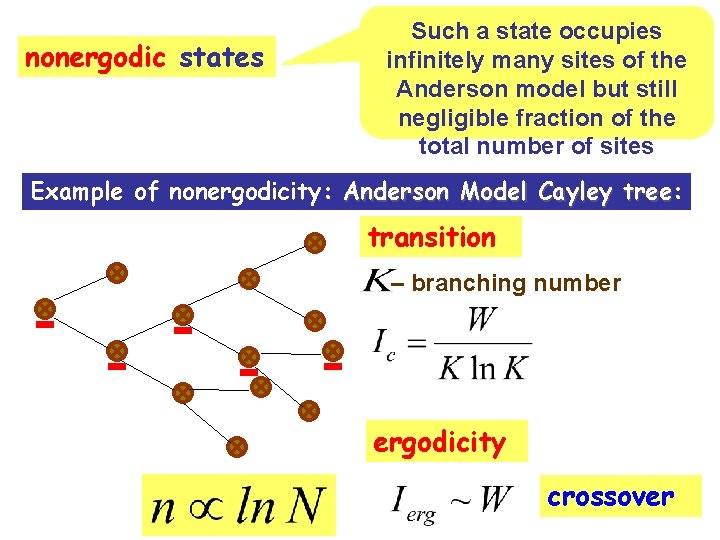
nonergodic states Such a state occupies infinitely many sites of the Anderson model but still negligible fraction of the total number of sites Example of nonergodicity: Anderson Model Cayley tree: tree transition – branching number ergodicity crossover

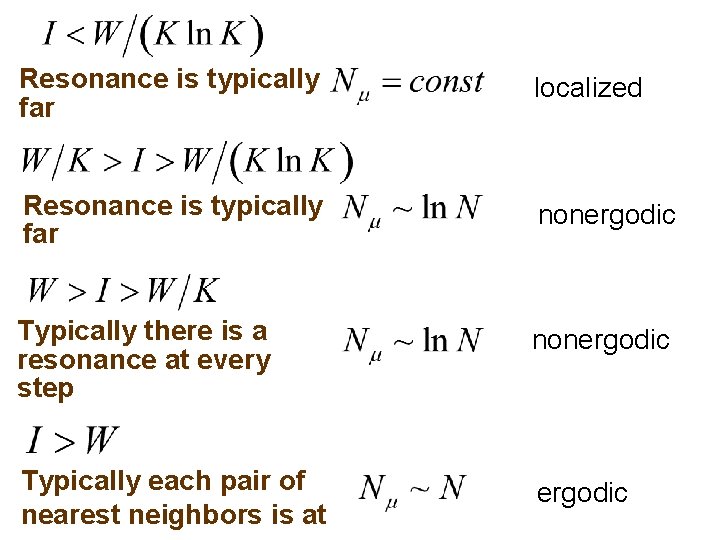
Resonance is typically far localized Resonance is typically far nonergodic Typically there is a resonance at every step nonergodic Typically each pair of nearest neighbors is at ergodic

Lecture 3. 2. Many-Body localization

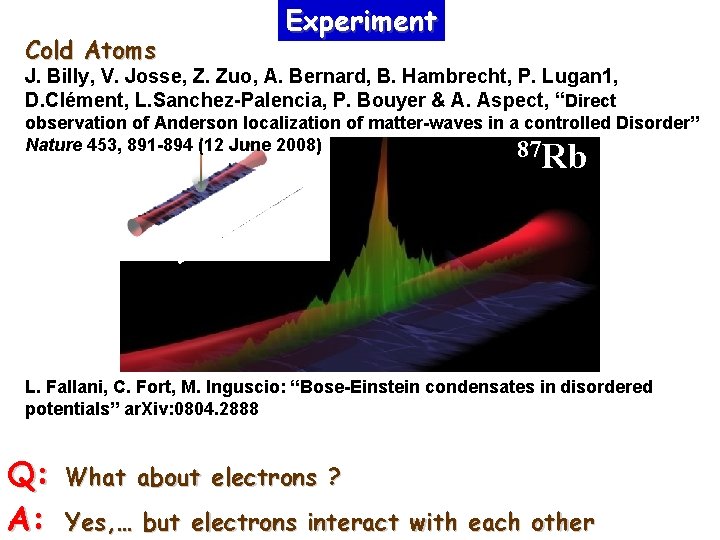
Cold Atoms Experiment J. Billy, V. Josse, Z. Zuo, A. Bernard, B. Hambrecht, P. Lugan 1, D. Clément, L. Sanchez-Palencia, P. Bouyer & A. Aspect, “Direct observation of Anderson localization of matter-waves in a controlled Disorder” Nature 453, 891 -894 (12 June 2008) 87 Rb L. Fallani, C. Fort, M. Inguscio: “Bose-Einstein condensates in disordered potentials” ar. Xiv: 0804. 2888 Q: A: What about electrons ? Yes, … but electrons interact with each other

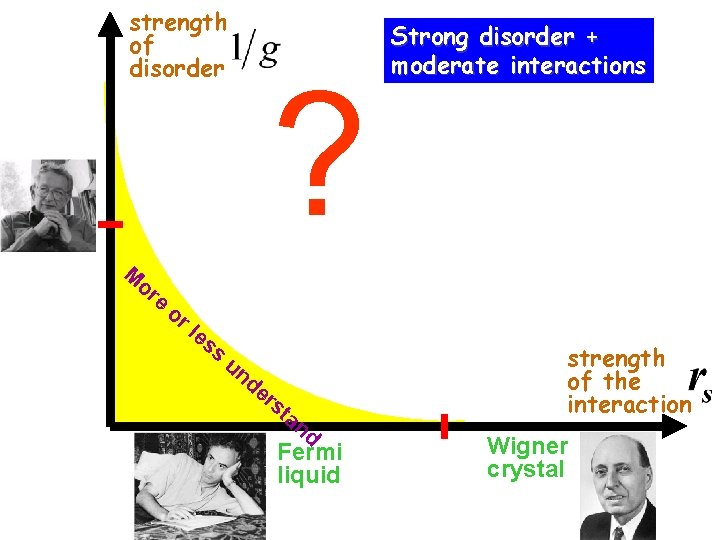
strength of disorder ? Strong disorder + moderate interactions M or e or le ss un de rs t strength of the interaction an d Fermi liquid Wigner crystal

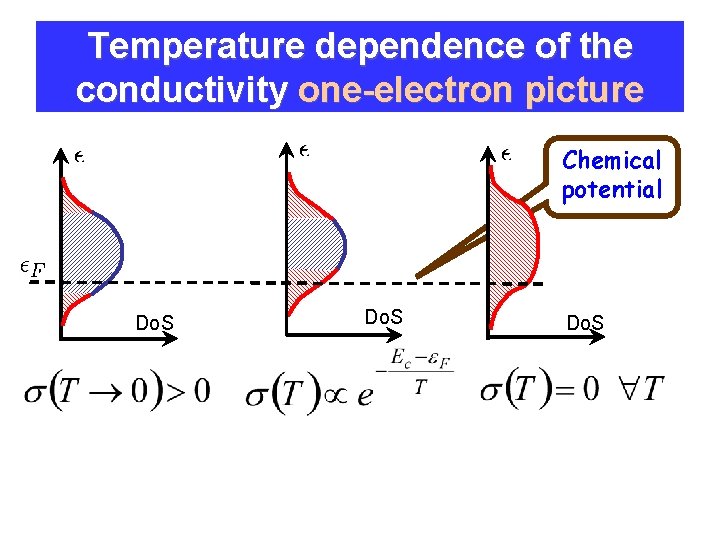
Temperature dependence of the conductivity one-electron picture Chemical potential Do. S

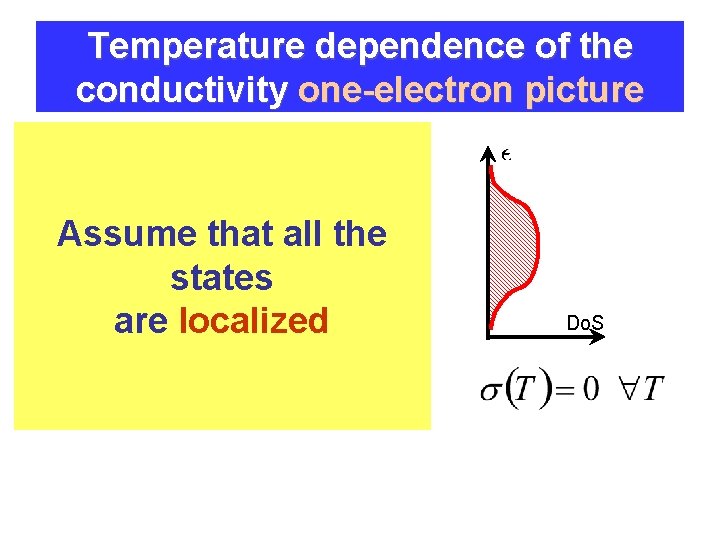
Temperature dependence of the conductivity one-electron picture Assume that all the states are localized Do. S

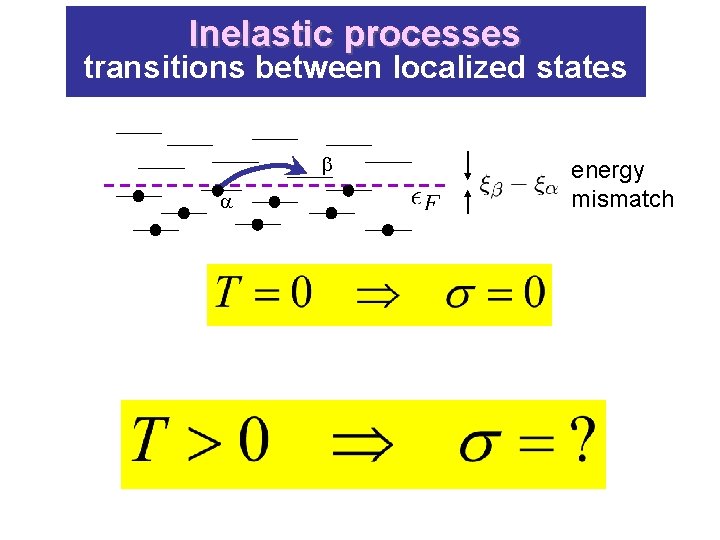
Inelastic processes transitions between localized states energy mismatch

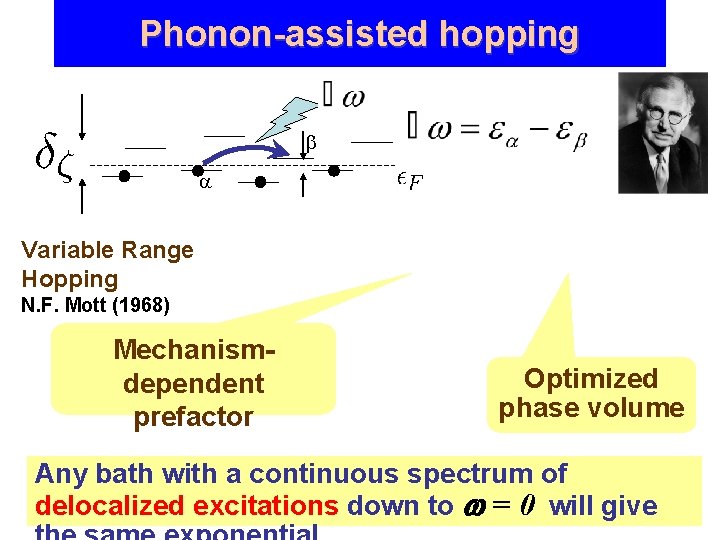
Phonon-assisted hopping Variable Range Hopping N. F. Mott (1968) Mechanismdependent prefactor Optimized phase volume Any bath with a continuous spectrum of delocalized excitations down to = 0 will give

Phonon-assisted hopping Variable Range Hopping N. F. Mott (1968) is mean localization energy spacing – typical energy separation between two localized states, which strongly overlap Any bath with a continuous spectrum of delocalized excitations down to = 0 will give

In disordered metals phonons limit the conductivity, but at low temperatures one can evaluate ohmic conductivity without phonons, i. e. without appealing to any bath (Drude formula)! A bath is needed only to stabilize the temperature of electrons. Q 1: Q 2: Is the existence of a bath crucial even for ohmic conductivity? Can a system of electrons left alone relax to thermal equilibrium without any bath? ? ?

Main postulate of the Gibbs Statistical Mechanics – equipartition (microcanonical distribution): In the equilibrium all states with the same energy are realized with the same probability. Without interaction between particles the equilibrium would never be reached – each one-particle energy is conserved. Common believe: Even weak interaction should drive the system to the equilibrium. Is it always true? No external bath!

Common belief: Anderson Insulator weak e-e interactions Phonon assisted hopping transport Can hopping conductivity exist without phonons ? Given: 1. All one-electron states are localized 2. Electrons interact with each other 3. The system is closed (no Find: DC phonons) conductivity (T, =0) (zero or finite? )

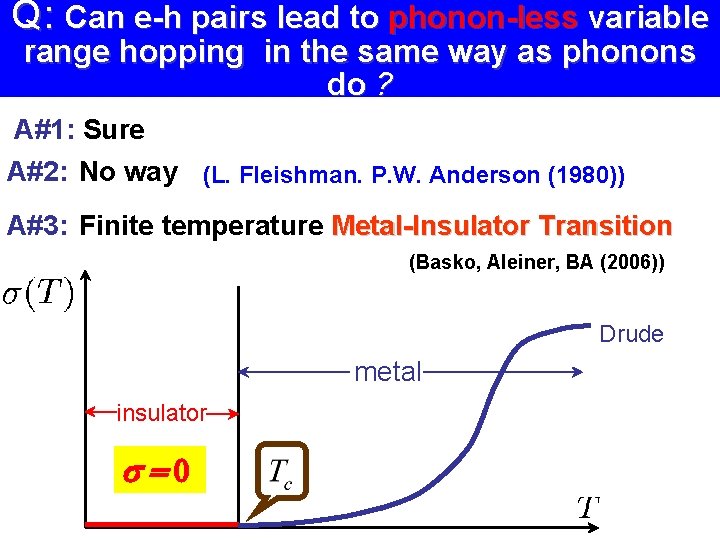
Q: Can e-h pairs lead to phonon-less variable range hopping in the same way as phonons do ? A#1: Sure 1. Recall phonon-less AC conductivity: N. F. Mott (1970) 2. FDT: there should be Nyquist noise 3. Use this noise as a bath instead of phonons 4. Self-consistency (whatever it means)

Q: Can e-h pairs lead to phonon-less variable range hopping in the same way as phonons do ? A#1: Sure A#2: No way (L. Fleishman. P. W. Anderson (1980)) Except maybe Coulomb interaction in 3 D is contributed by rare resonances R d g matrix element vanishes 0

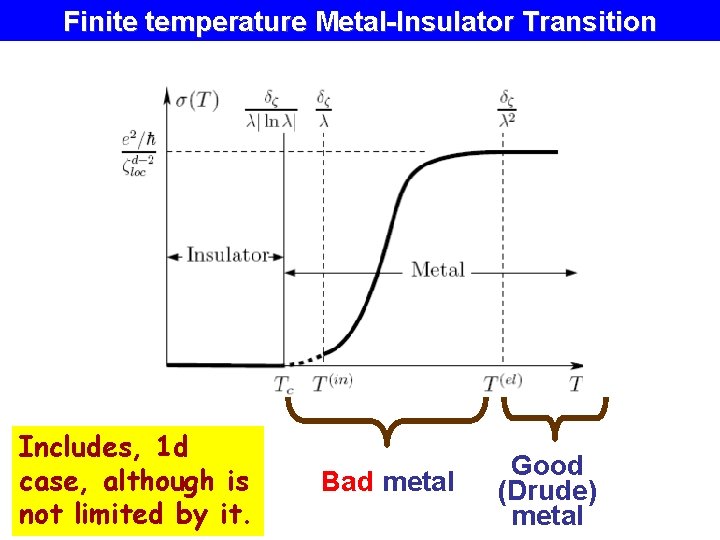
Q: Can e-h pairs lead to phonon-less variable range hopping in the same way as phonons do ? A#1: Sure A#2: No way (L. Fleishman. P. W. Anderson (1980)) A#3: Finite temperature Metal-Insulator Transition (Basko, Aleiner, BA (2006)) Drude metal insulator =0

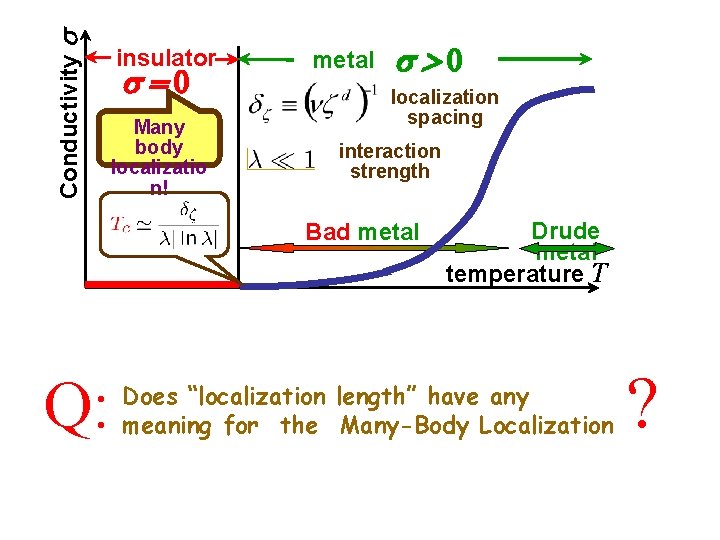
Finite temperature Metal-Insulator Transition Many body wave functions Manyare localized in functional space body metal localizatio insulator n! =0 Drude Interaction strength Localization spacing

`Main postulate of the Gibbs Statistical Mechanics – equipartition (microcanonical distribution): In the equilibrium all states with the same energy are realized with the same probability. Without interaction between particles the equilibrium would never be reached – each one-particle energy is conserved. Common believe: Even weak interaction should drive the system to the equilibrium. Is it always true? Many-Body Localization: 1. It is not localization in a real space! 2. There is no relaxation in the localized state in the same way as wave packets of localized wave functions do not spread.

Finite temperature Metal-Insulator Transition Includes, 1 d case, although is not limited by it. Bad metal Good (Drude) metal

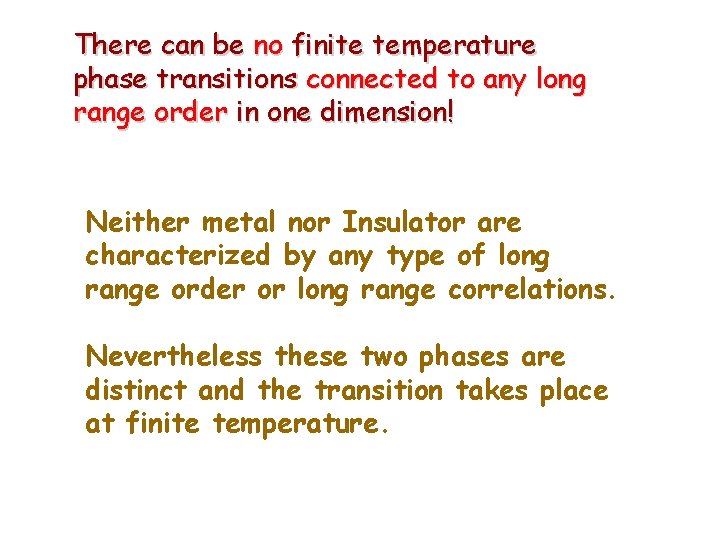
There can be no finite temperature phase transitions in one dimension! This is a dogma. Justification: 1. Another dogma: every phase transition is connected with the appearance (disappearance) of a long range order 2. Thermal fluctuations in 1 d systems destroy any long range order, lead to exponential decay of all spatial correlation functions and thus make phase transitions impossible

There can be no finite temperature phase transitions connected to any long range order in one dimension! Neither metal nor Insulator are characterized by any type of long range order or long range correlations. Nevertheless these two phases are distinct and the transition takes place at finite temperature.

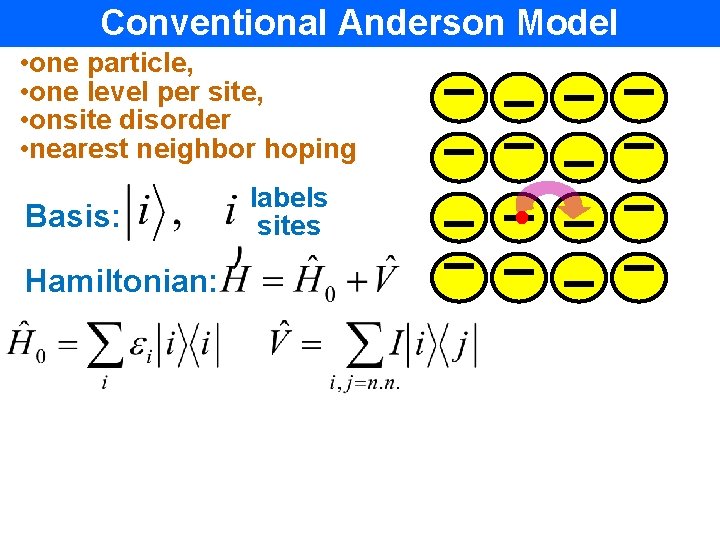
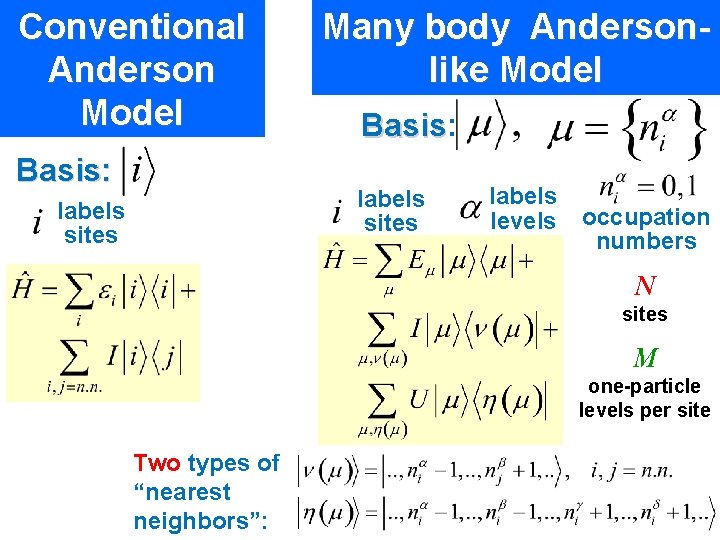
Conventional Anderson Model • one particle, • one level per site, • onsite disorder • nearest neighbor hoping Basis: Hamiltonian: labels sites

Many body Anderson-like Model

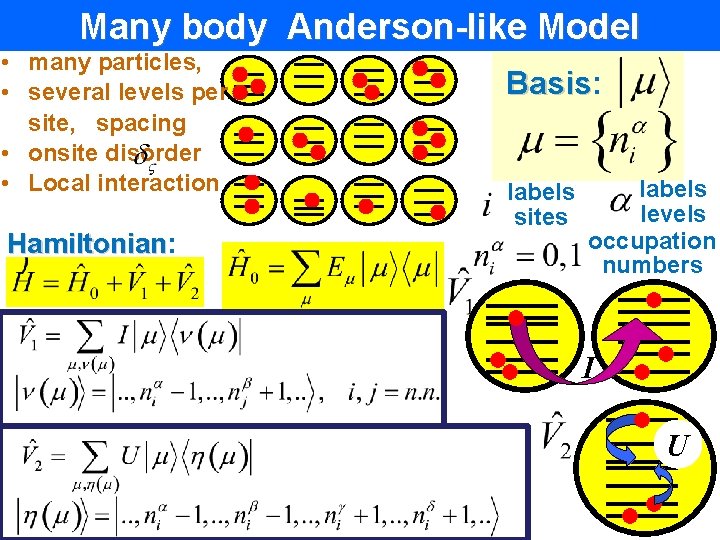
Many body Anderson-like Model • many particles, • several levels per site, spacing • onsite disorder • Local interaction Hamiltonian: Hamiltonian Basis: Basis labels sites labels levels occupation numbers I U

Conventional Anderson Model Basis: Many body Andersonlike Model Basis: Basis labels sites labels levels occupation numbers N sites M one-particle levels per site Two types of “nearest neighbors”:

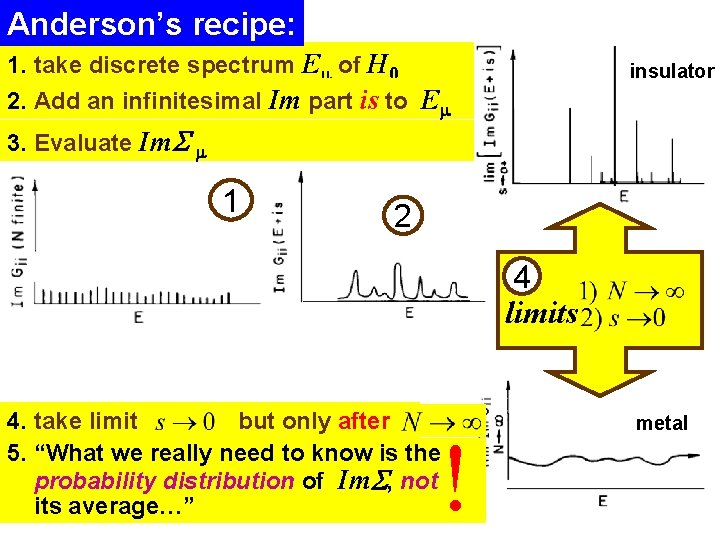
Anderson’s recipe: 1. take discrete spectrum Em of H 0 2. Add an infinitesimal Im part is to Em insulator 3. Evaluate Im. S m 1 2 4 limits 4. take limit but only after 5. “What we really need to know is the probability distribution of Im. S, not its average…” ! metal

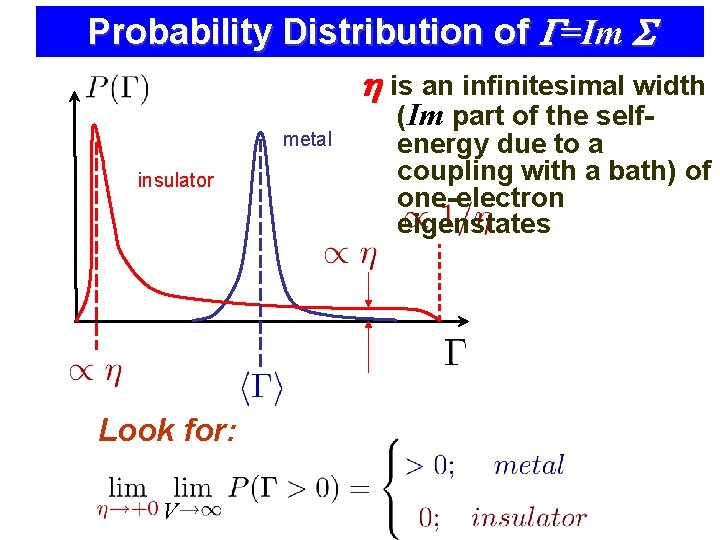
Probability Distribution of G=Im S h is an infinitesimal width metal insulator Look for: V (Im part of the selfenergy due to a coupling with a bath) of one-electron eigenstates

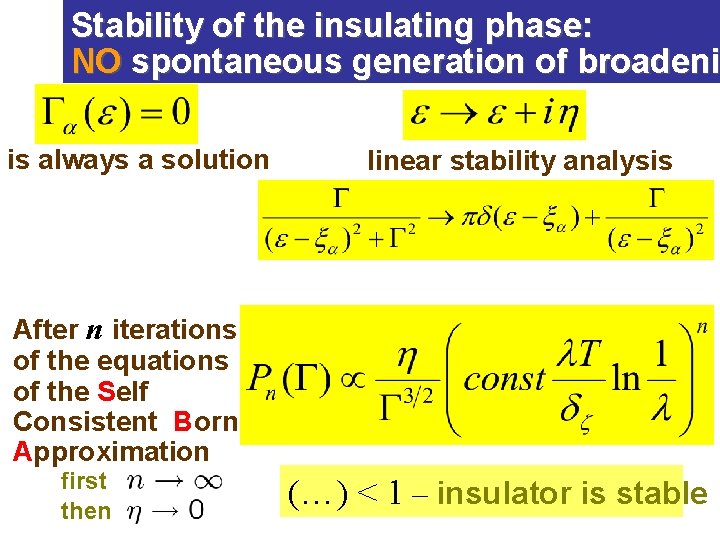
Stability of the insulating phase: NO spontaneous generation of broadenin broadeni is always a solution linear stability analysis After n iterations of the equations of the Self Consistent Born Approximation first then (…) < 1 – insulator is stable !

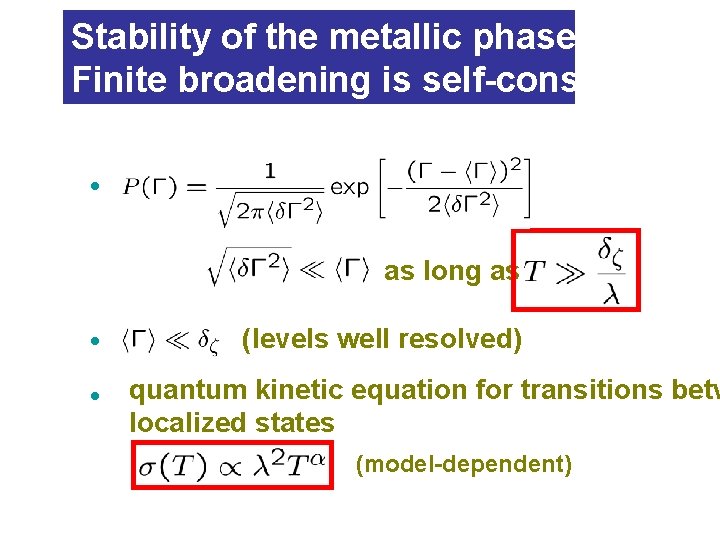
Stability of the metallic phase: Finite broadening is self-consistent • as long as • (levels well resolved) • quantum kinetic equation for transitions betw localized states (model-dependent)

Conductivity insulator =0 Many body localizatio n! metal >0 localization spacing interaction strength Bad metal Q: Drude metal temperature T Does “localization length” have any meaning for the Many-Body Localization ?

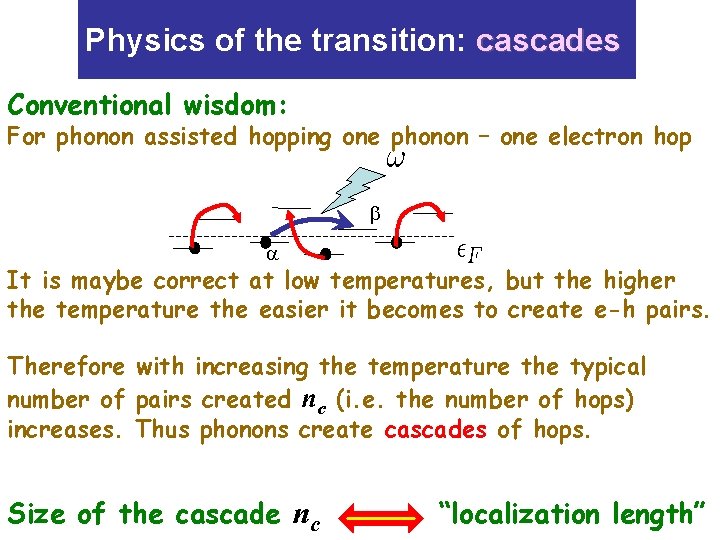
Physics of the transition: cascades Conventional wisdom: For phonon assisted hopping one phonon – one electron hop It is maybe correct at low temperatures, but the higher the temperature the easier it becomes to create e-h pairs. Therefore with increasing the temperature the typical number of pairs created nc (i. e. the number of hops) increases. Thus phonons create cascades of hops. Size of the cascade nc “localization length”

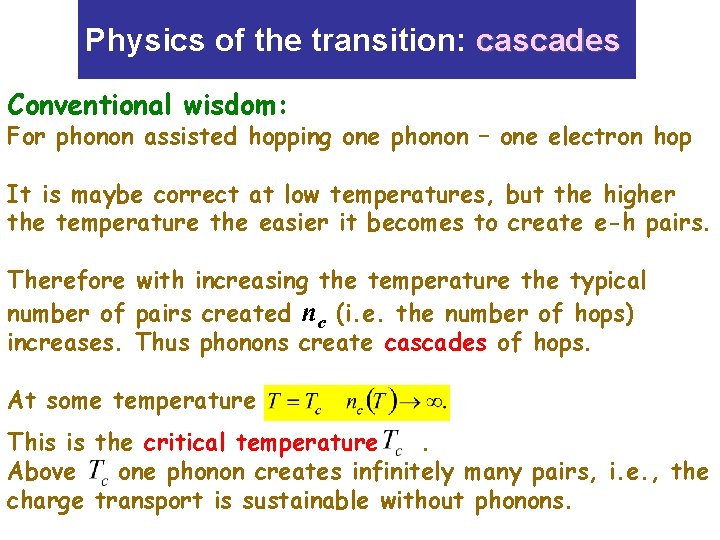
Physics of the transition: cascades Conventional wisdom: For phonon assisted hopping one phonon – one electron hop It is maybe correct at low temperatures, but the higher the temperature the easier it becomes to create e-h pairs. Therefore with increasing the temperature the typical number of pairs created nc (i. e. the number of hops) increases. Thus phonons create cascades of hops. At some temperature This is the critical temperature. Above one phonon creates infinitely many pairs, i. e. , the charge transport is sustainable without phonons.

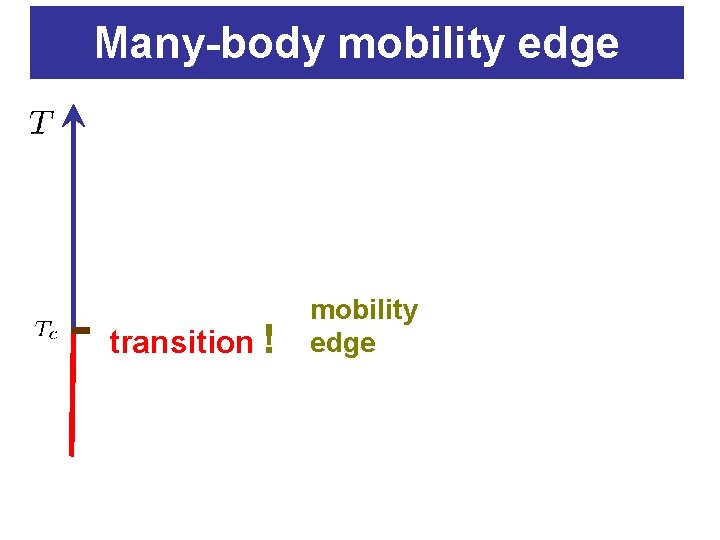
Many-body mobility edge transition ! mobility edge

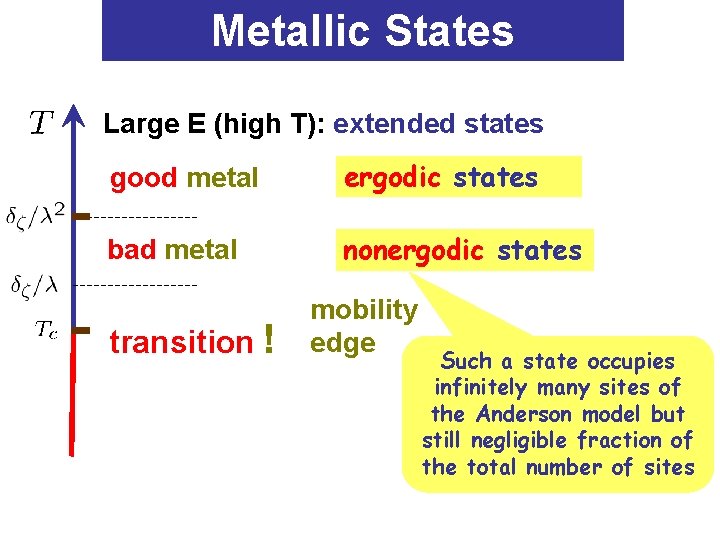
Metallic States Large E (high T): extended states good metal ergodic states bad metal nonergodic states transition ! mobility edge Such a state occupies infinitely many sites of the Anderson model but still negligible fraction of the total number of sites

Large E (high T): extended states good metal ergodic states bad metal nonergodic states transition ! mobility edge No relaxation to microcanonical distribution – no equipartition crossover ?

Large E (high T): extended states good metal ergodic states bad metal nonergodic states mobility edge transition ! ? Why no activation

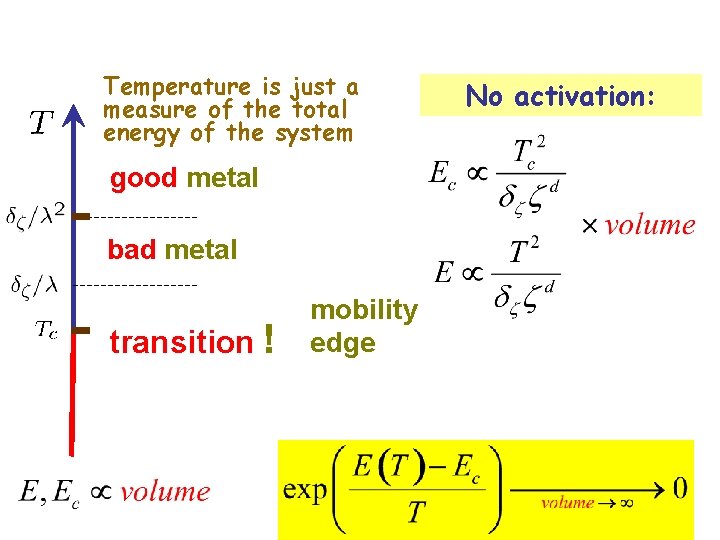
Temperature is just a measure of the total energy of the system good metal bad metal transition ! mobility edge No activation:

Lecture 3. 3. Experiment

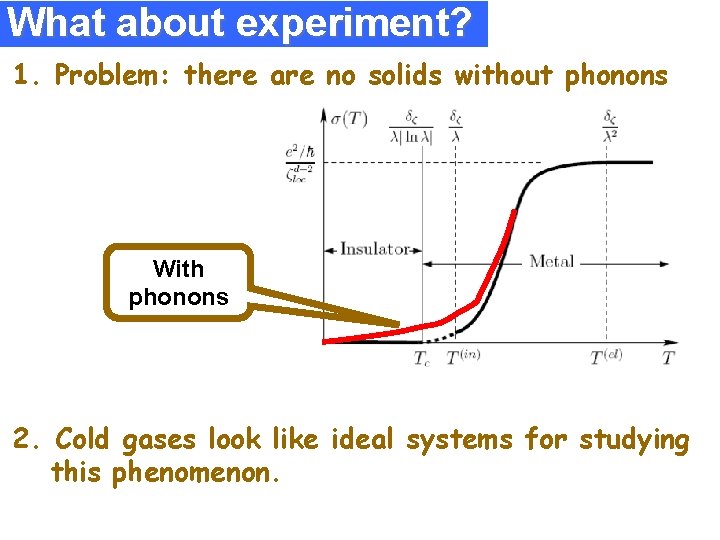
What about experiment? 1. Problem: there are no solids without phonons With phonons 2. Cold gases look like ideal systems for studying this phenomenon.

G. Sambandamurthy, L. Engel, A. Johansson, E. Peled & D. Shahar, Phys. Rev. Lett. 94, 017003 (2005). M. Ovadia, B. Sacepe, and D. Shahar, PRL (2009). YSi In. O } V. M. Vinokur, T. I. Baturina, M. V. Fistul, A. Y. Mironov, M. R. Baklanov, & C. Strunk, Nature 452, 613 (2008) Ti. N S. Lee, A. Fursina, J. T. Mayo, C. T. Yavuz, V. L. Colvin, R. G. S. Sofin, I. V. Shvetz and D. Natelson, Nature Materials v 7 (2008) Fe. O 4 Superconductor – Insulator transition F. Ladieu, M. Sanquer, and J. P. Bouchaud, Phys. Rev. B 53, 973 (1996) magnetite

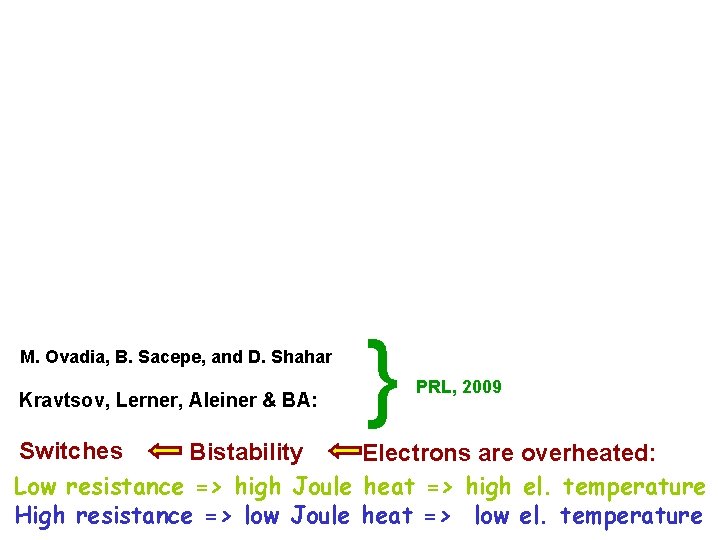
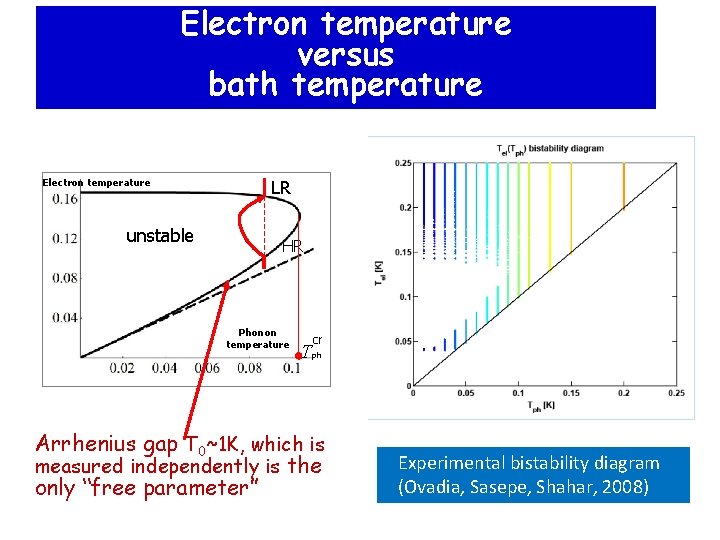
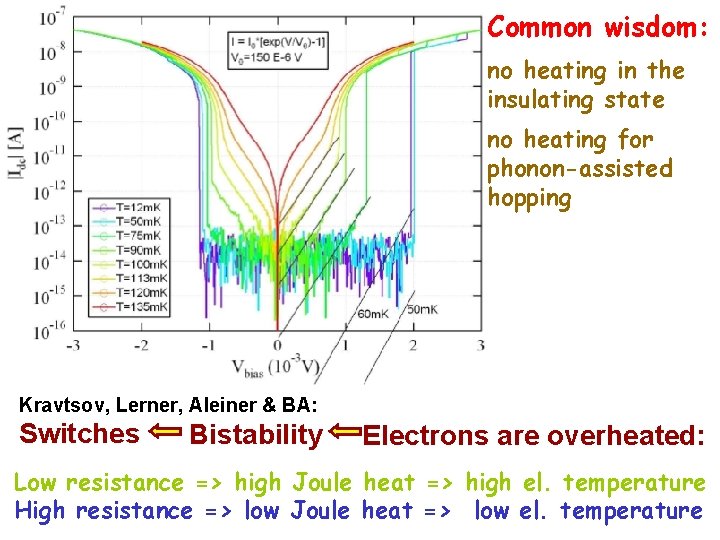
M. Ovadia, B. Sacepe, and D. Shahar Kravtsov, Lerner, Aleiner & BA: } PRL, 2009 Switches Bistability Electrons are overheated: Low resistance => high Joule heat => high el. temperature High resistance => low Joule heat => low el. temperature

Electron temperature versus bath temperature Electron temperature unstable LR HR Phonon temperature T cr ph Arrhenius gap T 0~1 K, which is measured independently is the only “free parameter” Experimental bistability diagram (Ovadia, Sasepe, Shahar, 2008)

Common wisdom: no heating in the insulating state no heating for phonon-assisted hopping Heating appears only together with cascades Kravtsov, Lerner, Aleiner & BA: Switches Bistability Electrons are overheated: Low resistance => high Joule heat => high el. temperature High resistance => low Joule heat => low el. temperature

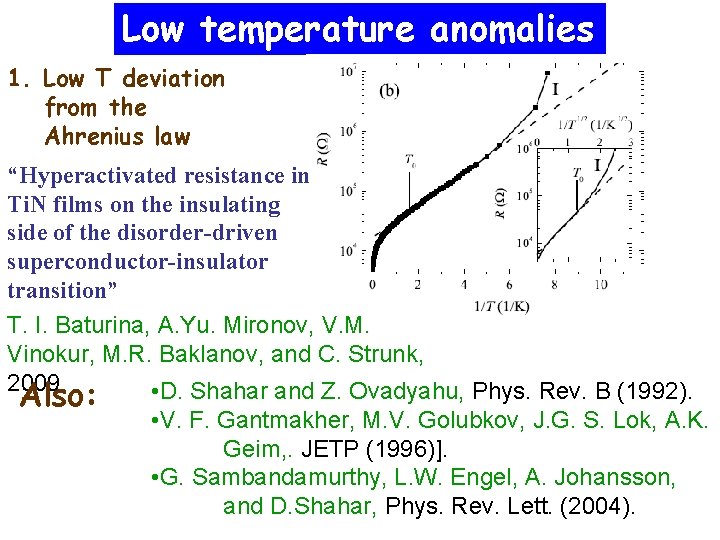
Low temperature anomalies 1. Low T deviation from the Ahrenius law “Hyperactivated resistance in Ti. N films on the insulating side of the disorder-driven superconductor-insulator transition” T. I. Baturina, A. Yu. Mironov, V. M. Vinokur, M. R. Baklanov, and C. Strunk, 2009 • D. Shahar and Z. Ovadyahu, Phys. Rev. B (1992). Also: • V. F. Gantmakher, M. V. Golubkov, J. G. S. Lok, A. K. Geim, . JETP (1996)]. • G. Sambandamurthy, L. W. Engel, A. Johansson, and D. Shahar, Phys. Rev. Lett. (2004).

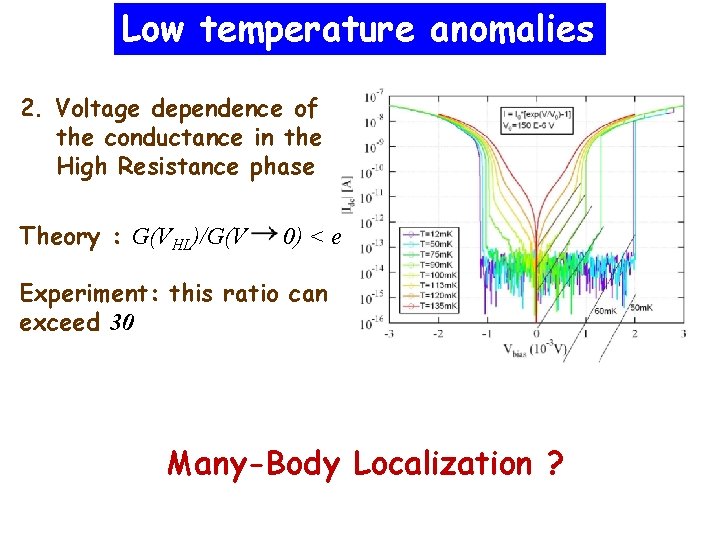
Low temperature anomalies 2. Voltage dependence of the conductance in the High Resistance phase Theory : G(VHL)/G(V 0) < e Experiment: this ratio can exceed 30 Many-Body Localization ?

Lecture 3. 4. Speculations

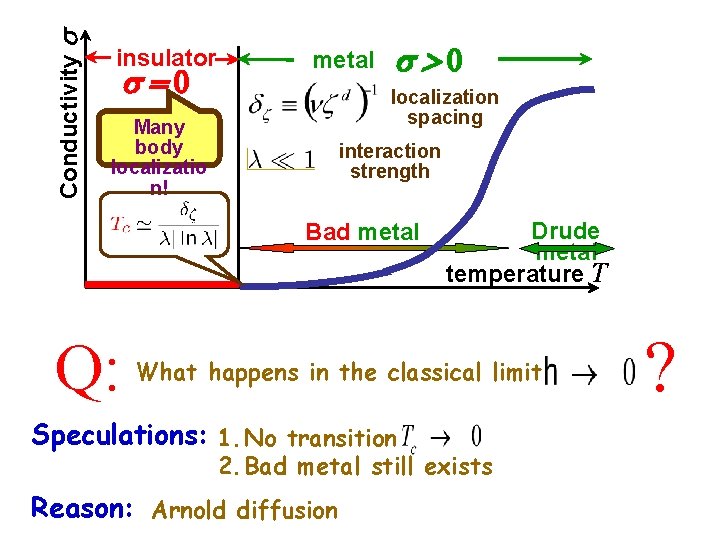
Conductivity insulator =0 metal >0 localization spacing Many body localizatio n! interaction strength Bad metal Q: Drude metal temperature T What happens in the classical limit Speculations: 1. No transition 2. Bad metal still exists Reason: Arnold diffusion ?

Conclusions Anderson Localization provides a relevant language for description of a wide class of physical phenomena – far beyond conventional Metal to Insulator transitions. Transition between integrability and chaos in quantum systems Interacting quantum particles + strong disorder. Three types of behavior: ordinary ergodic metal “bad” nonergodic metal “true” insulator A closed system without a bath can relaxation to a microcanonical distribution only if it is an ergodic metal

Open Questions Both “bad” metal and insulator resemble glasses? ? ? What about strong electron-electron interactions? Melting of a pined Wigner crystal – delocalization of vibration modes? Coulomb interaction in 3 D. Is it a bad metal till T=0 or there is a transition? Role of Re. S ? Effects of quantum condensation? Nonergodic states and nonergodic systems

Thank you
 Somatization disorder dsm 5
Somatization disorder dsm 5 Origin of quantum mechanics
Origin of quantum mechanics Quantum physics vs mechanics
Quantum physics vs mechanics 01:640:244 lecture notes - lecture 15: plat, idah, farad
01:640:244 lecture notes - lecture 15: plat, idah, farad Big crunch
Big crunch Chaos theory and cryptology
Chaos theory and cryptology Fractals and chaos
Fractals and chaos Operating systems lecture notes
Operating systems lecture notes Lecture sound systems
Lecture sound systems Lecture sound systems
Lecture sound systems Na początku był chaos biblia
Na początku był chaos biblia Bitterrezeptoren
Bitterrezeptoren God's firmament
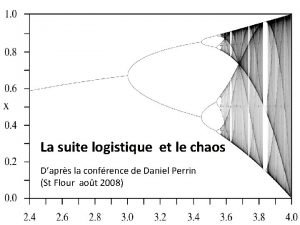
God's firmament Suite logistique
Suite logistique Griekse goden stamboom
Griekse goden stamboom Greek god and goddesses family tree
Greek god and goddesses family tree What animal symbolizes chaos
What animal symbolizes chaos Copyright chaos
Copyright chaos Third iteration jurassic park
Third iteration jurassic park Chaos sound test
Chaos sound test Chaos theory management
Chaos theory management Six degrees of separation synopsis
Six degrees of separation synopsis Nietzsche draw
Nietzsche draw Chaos ruled ok in the classroom
Chaos ruled ok in the classroom Mitologia grecka drzewo genealogiczne
Mitologia grecka drzewo genealogiczne Chaos in greek mythology
Chaos in greek mythology Callbacks
Callbacks Chaos in de klas
Chaos in de klas Applied physics letters
Applied physics letters Affluence synonym
Affluence synonym Facts about hera
Facts about hera Gaia and uranus family tree
Gaia and uranus family tree Cauliflower chaos fractals every
Cauliflower chaos fractals every Generally restful,like the horizon,where the sky meets land
Generally restful,like the horizon,where the sky meets land Poema por un clavo se perdio una herradura
Poema por un clavo se perdio una herradura After feeding several quarters into the gumball machine
After feeding several quarters into the gumball machine In the beginning there was chaos
In the beginning there was chaos Report chaos
Report chaos What did the molten sea represent
What did the molten sea represent Standish group chaos report 2020
Standish group chaos report 2020 Chaos report
Chaos report Aip chaos
Aip chaos Decision support systems and intelligent systems
Decision support systems and intelligent systems Dicapine
Dicapine Embedded systems vs cyber physical systems
Embedded systems vs cyber physical systems Engineering elegant systems: theory of systems engineering
Engineering elegant systems: theory of systems engineering Bpd comorbidity
Bpd comorbidity Adhd or borderline personality disorder
Adhd or borderline personality disorder Kode icd 10 alopecia areata
Kode icd 10 alopecia areata Thoracogenic scoliosis of thoracolumbar region
Thoracogenic scoliosis of thoracolumbar region Disorder of synovium and tendon
Disorder of synovium and tendon Disorder of synovium and tendon
Disorder of synovium and tendon Quantum and nuclear physics
Quantum and nuclear physics Quantum mechanical model atom
Quantum mechanical model atom Quantum numbers and electron configuration
Quantum numbers and electron configuration Schrodingers cay
Schrodingers cay