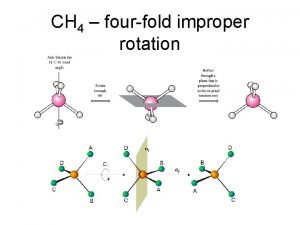
Lecture 3 Principal rotation axis Any C 2






- Slides: 32

Lecture 3


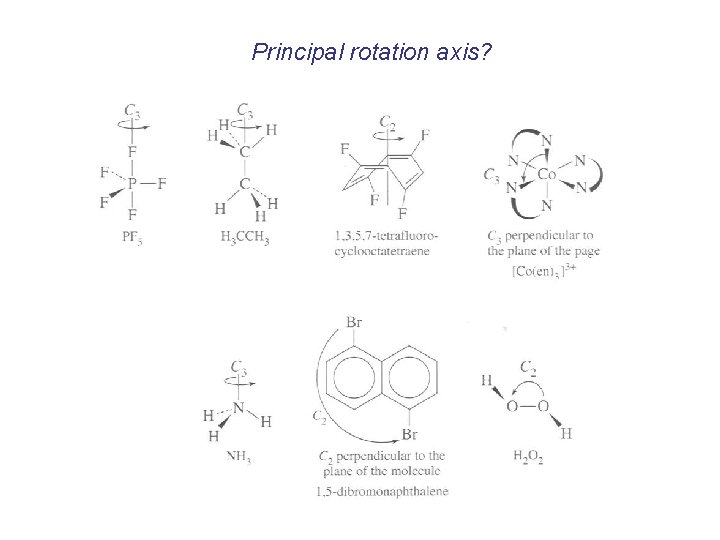
Principal rotation axis?

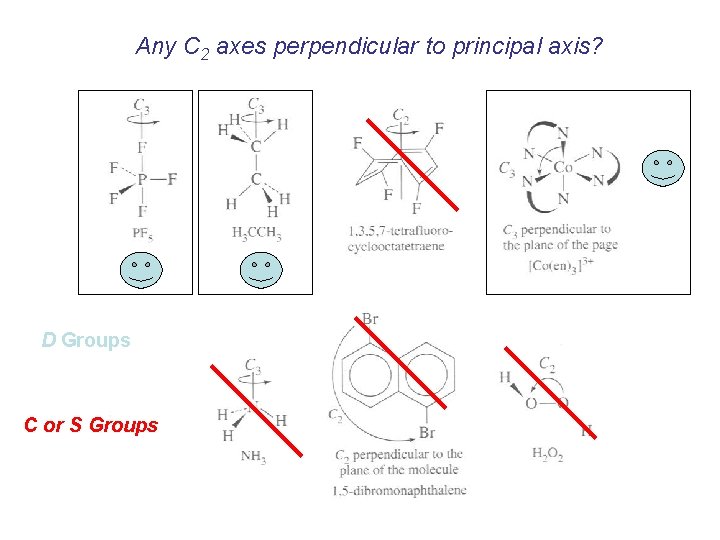
Any C 2 axes perpendicular to principal axis? D Groups C or S Groups

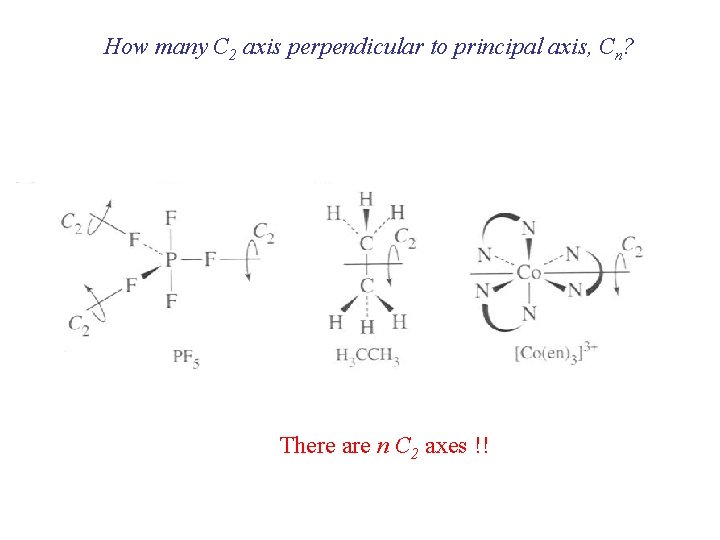
How many C 2 axis perpendicular to principal axis, Cn? There are n C 2 axes !!

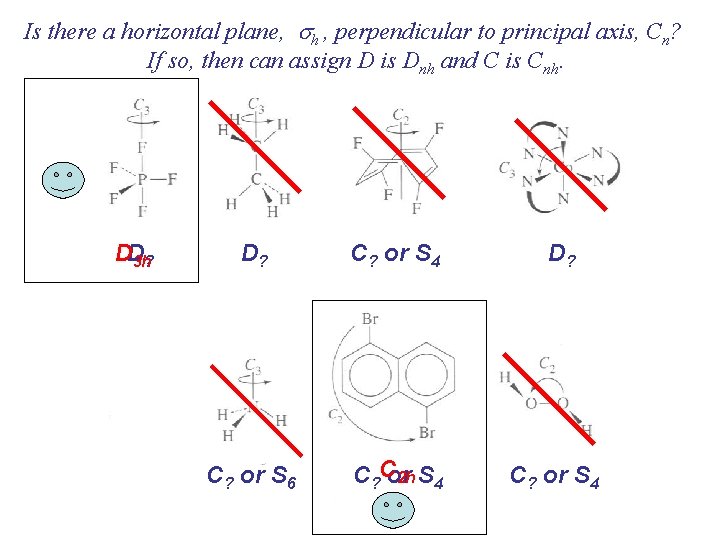
Is there a horizontal plane, sh , perpendicular to principal axis, Cn? If so, then can assign D is Dnh and C is Cnh. DD 3 h? D? C? or S 4 C? or S 6 C? Cor 2 h S 4 D? C? or S 4

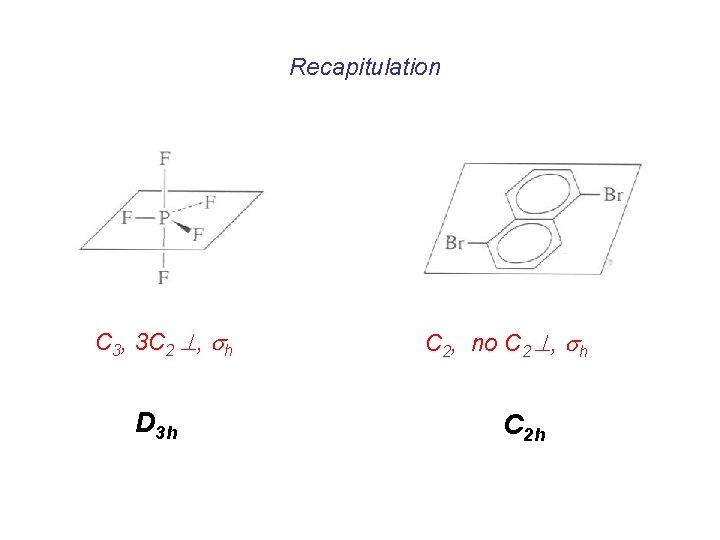
Recapitulation C 3, 3 C 2 , sh D 3 h C 2, no C 2 , sh C 2 h

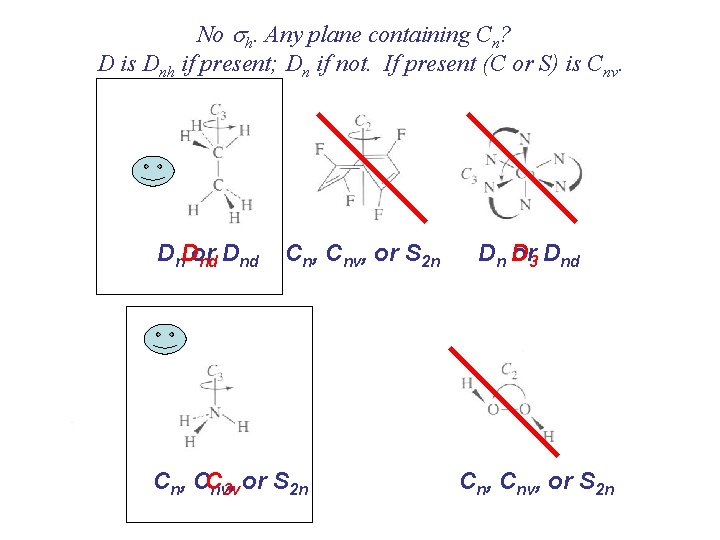
No sh. Any plane containing Cn? D is Dnh if present; Dn if not. If present (C or S) is Cnv. Dn. Dor nd Dnd Cn, Cnv, or S 2 n Cn, CC , or S 2 n nv 3 v Dn D or 3 Dnd Cn, Cnv, or S 2 n

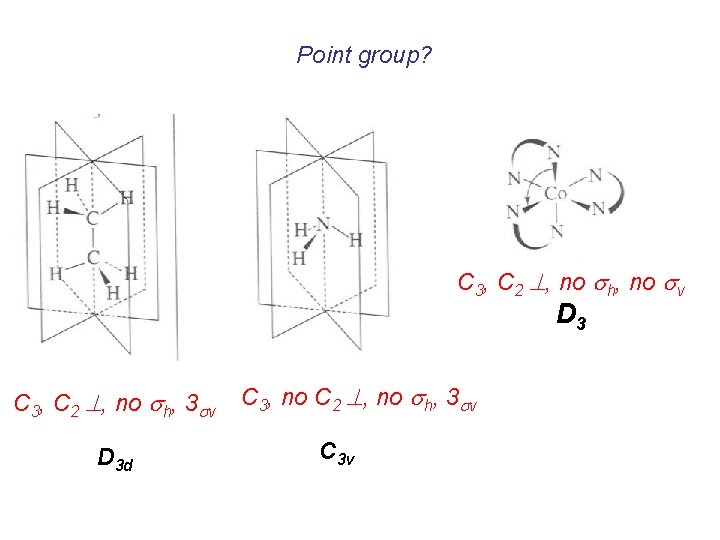
Point group? C 3, C 2 , no sh, no sv D 3 C 3, C 2 , no sh, 3 sv D 3 d C 3, no C 2 , no sh, 3 sv C 3 v

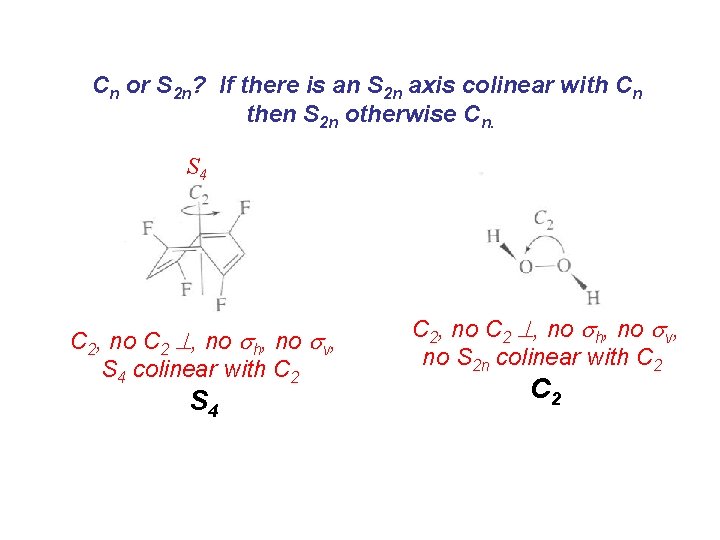
Cn or S 2 n? If there is an S 2 n axis colinear with Cn then S 2 n otherwise Cn. S 4 C 2, no C 2 , no sh, no sv, S 4 colinear with C 2 S 4 C 2, no C 2 , no sh, no sv, no S 2 n colinear with C 2

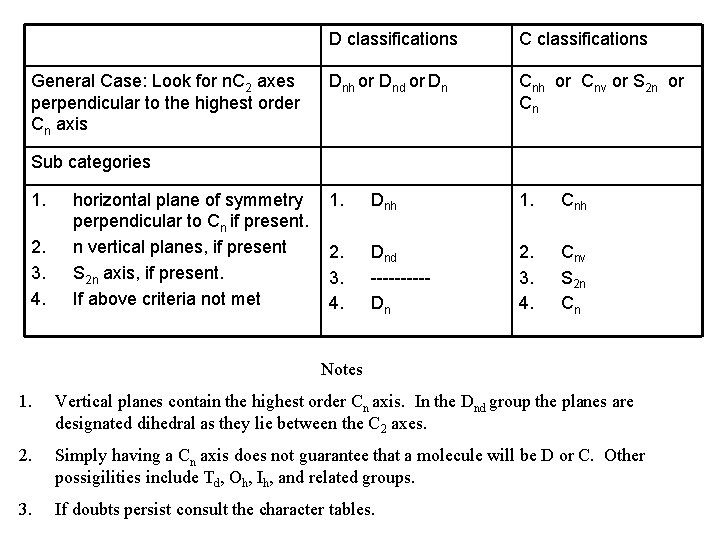
General Case: Look for n. C 2 axes perpendicular to the highest order Cn axis D classifications C classifications Dnh or Dnd or Dn Cnh or Cnv or S 2 n or Cn 1. Dnh 1. Cnh 2. 3. 4. Dnd -----Dn 2. 3. 4. Cnv S 2 n Cn Sub categories 1. 2. 3. 4. horizontal plane of symmetry perpendicular to Cn if present. n vertical planes, if present S 2 n axis, if present. If above criteria not met Notes 1. Vertical planes contain the highest order Cn axis. In the Dnd group the planes are designated dihedral as they lie between the C 2 axes. 2. Simply having a Cn axis does not guarantee that a molecule will be D or C. Other possigilities include Td, Oh, Ih, and related groups. 3. If doubts persist consult the character tables.

The point groups of common molecular shapes D∞h D 3 h C 2 v C 3 v C 2 v

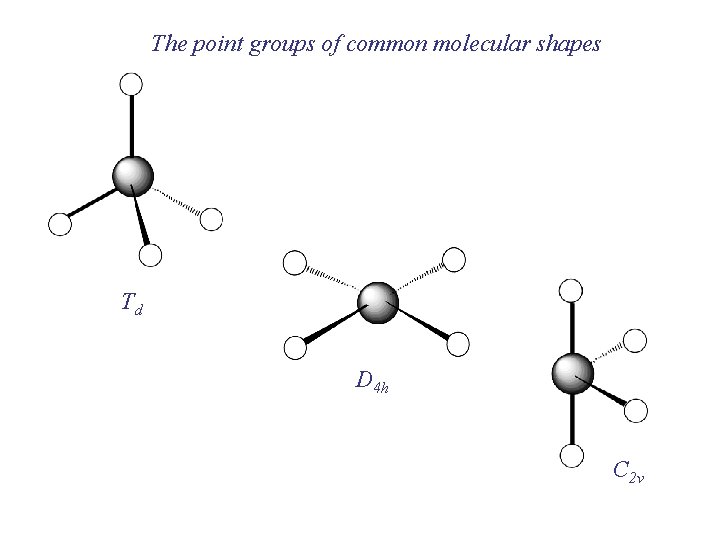
The point groups of common molecular shapes Td D 4 h C 2 v

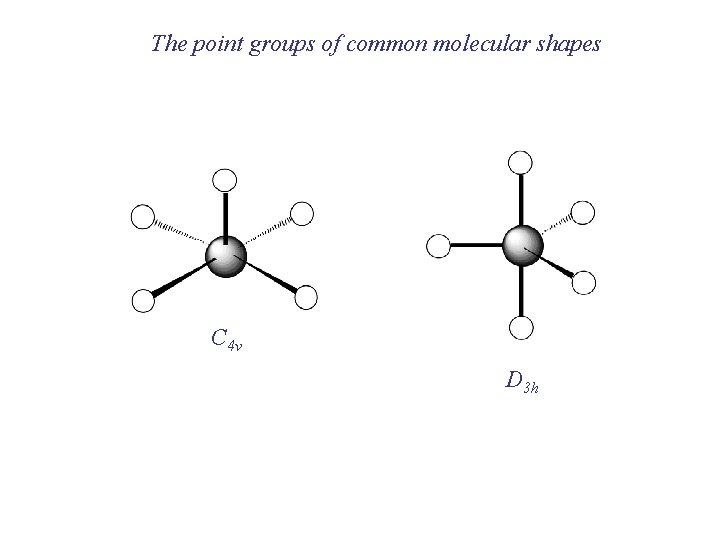
The point groups of common molecular shapes C 4 v D 3 h

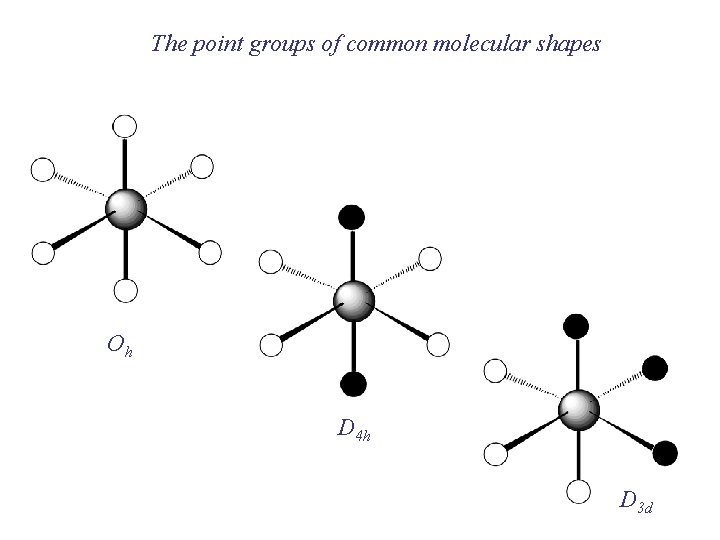
The point groups of common molecular shapes Oh D 4 h D 3 d

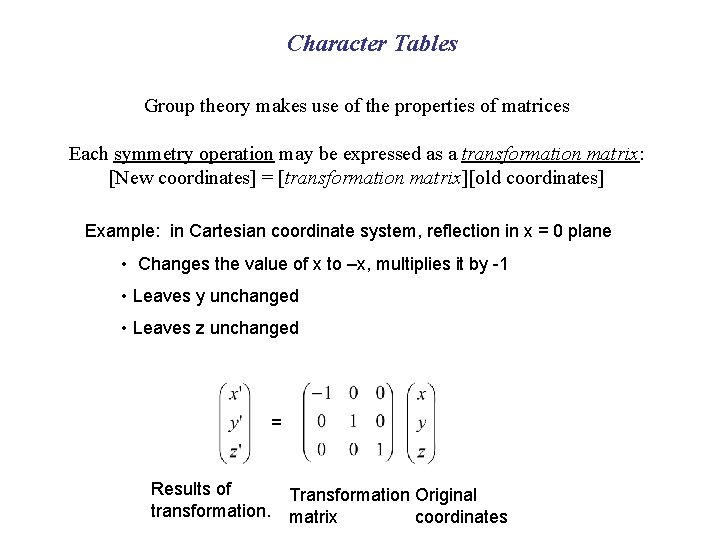
Character Tables Group theory makes use of the properties of matrices Each symmetry operation may be expressed as a transformation matrix: [New coordinates] = [transformation matrix][old coordinates] Example: in Cartesian coordinate system, reflection in x = 0 plane • Changes the value of x to –x, multiplies it by -1 • Leaves y unchanged • Leaves z unchanged = Results of transformation. Transformation Original matrix coordinates

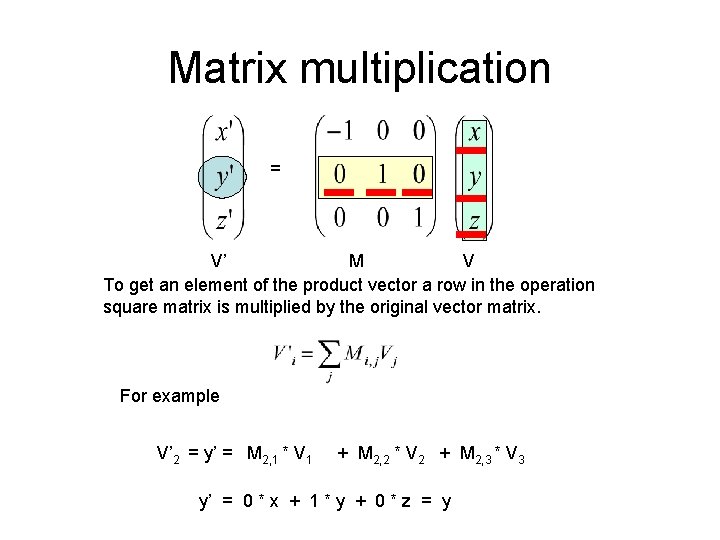
Matrix multiplication = V’ M V To get an element of the product vector a row in the operation square matrix is multiplied by the original vector matrix. For example V’ 2 = y’ = M 2, 1 * V 1 + M 2, 2 * V 2 + M 2, 3 * V 3 y’ = 0 * x + 1 * y + 0 * z = y

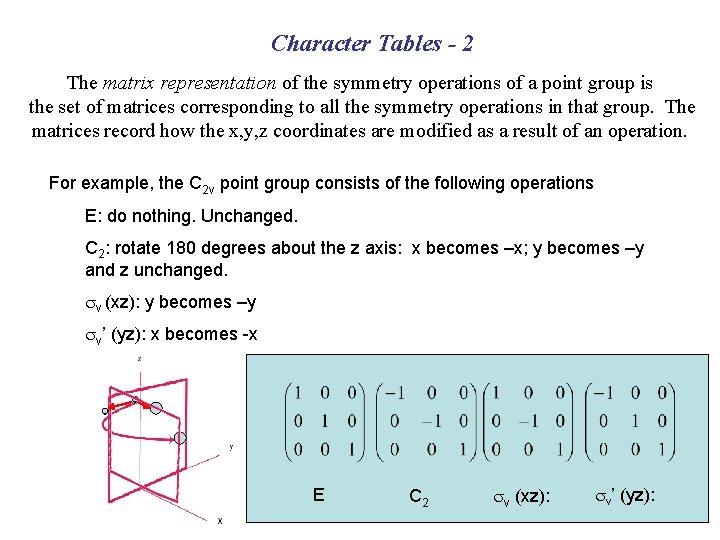
Character Tables - 2 The matrix representation of the symmetry operations of a point group is the set of matrices corresponding to all the symmetry operations in that group. The matrices record how the x, y, z coordinates are modified as a result of an operation. For example, the C 2 v point group consists of the following operations E: do nothing. Unchanged. C 2: rotate 180 degrees about the z axis: x becomes –x; y becomes –y and z unchanged. sv (xz): y becomes –y sv’ (yz): x becomes -x E C 2 sv (xz): sv’ (yz):

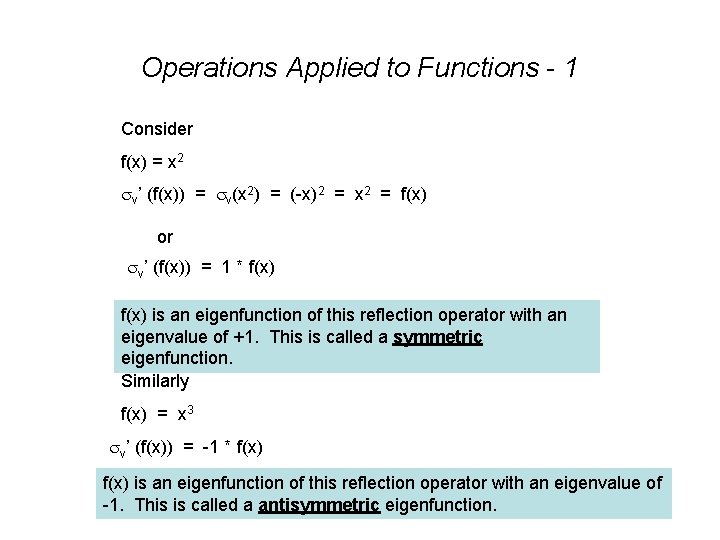
Operations Applied to Functions - 1 Consider f(x) = x 2 sv’ (f(x)) = sv(x 2) = (-x)2 = x 2 = f(x) or sv’ (f(x)) = 1 * f(x) is an eigenfunction of this reflection operator with an eigenvalue of +1. This is called a symmetric eigenfunction. Similarly f(x) = x 3 sv’ (f(x)) = -1 * f(x) is an eigenfunction of this reflection operator with an eigenvalue of -1. This is called a antisymmetric eigenfunction.

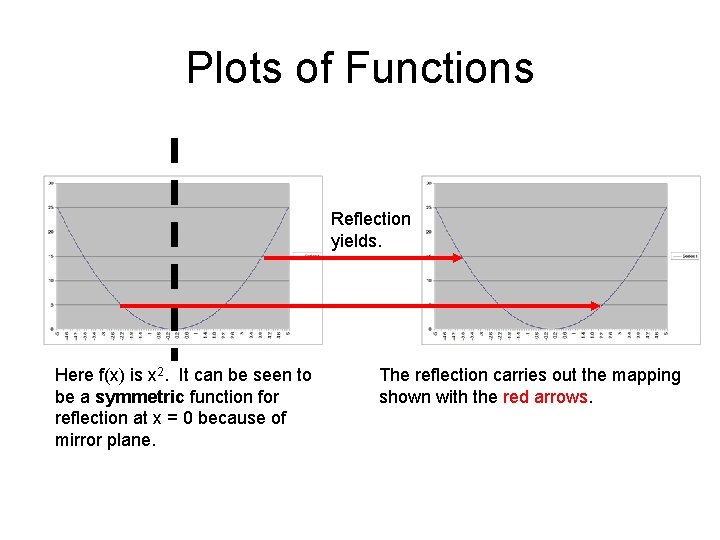
Plots of Functions Reflection yields. Here f(x) is x 2. It can be seen to be a symmetric function for reflection at x = 0 because of mirror plane. The reflection carries out the mapping shown with the red arrows.

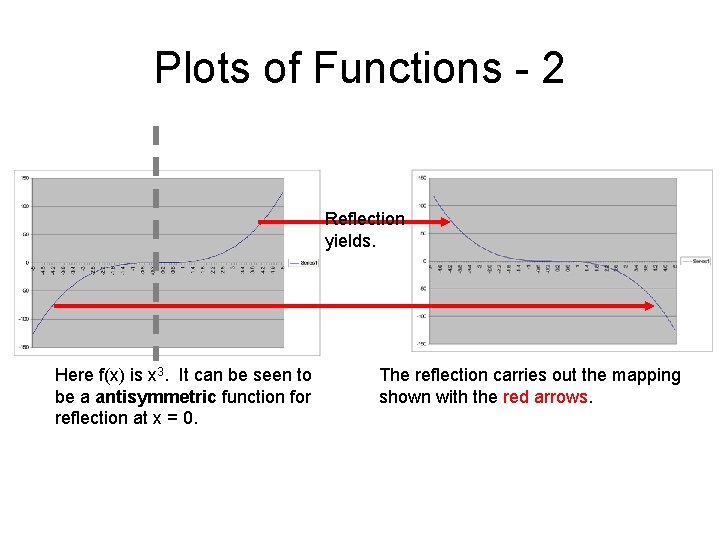
Plots of Functions - 2 Reflection yields. Here f(x) is x 3. It can be seen to be a antisymmetric function for reflection at x = 0. The reflection carries out the mapping shown with the red arrows.

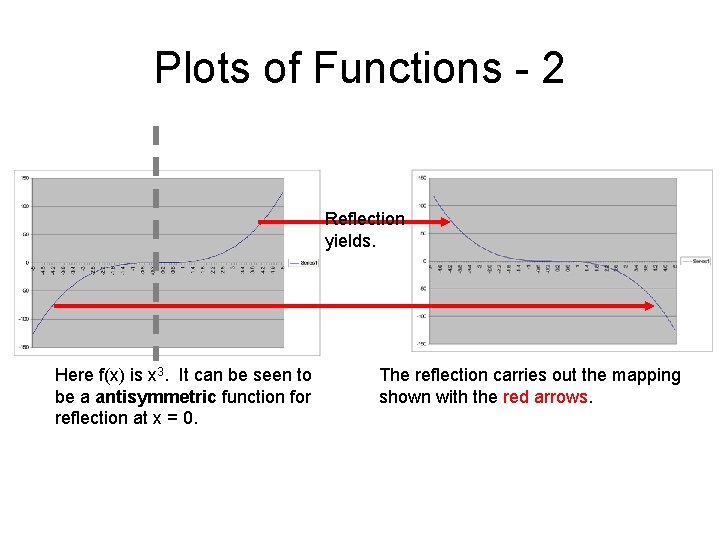
Plots of Functions - 2 Reflection yields. Here f(x) is x 3. It can be seen to be a antisymmetric function for reflection at x = 0. The reflection carries out the mapping shown with the red arrows.

Operations Applied to Functions - 2 Now consider f(x) = (x-2)2 = x 2 – 4 x + 4 sv’ (f(x)) = sv(x-2)2 = (-x-2)2 = x 2 + 4 x + 4 f(x) = (x-2)2 is not an eigenfunction of this reflection operator because it does not return a constant times f(x). Reflection yields this function, not an eigenfunc tion. Neither symmetric nor antisymmetric for reflection thru x = 0.

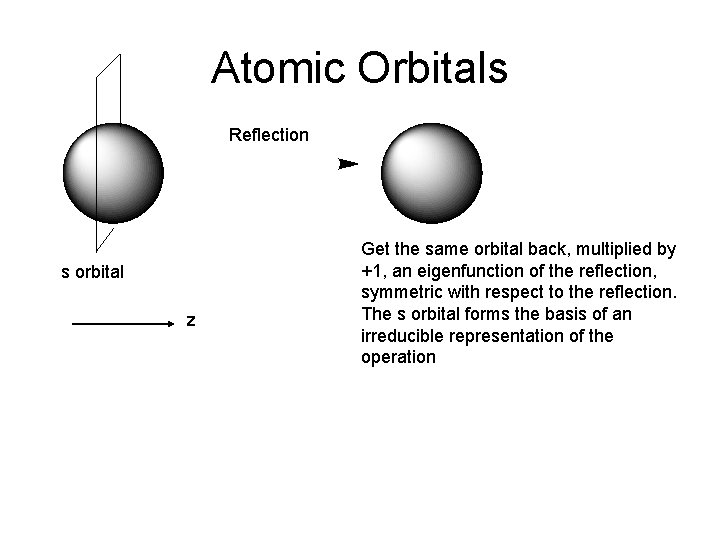
Atomic Orbitals Reflection s orbital z Get the same orbital back, multiplied by +1, an eigenfunction of the reflection, symmetric with respect to the reflection. The s orbital forms the basis of an irreducible representation of the operation

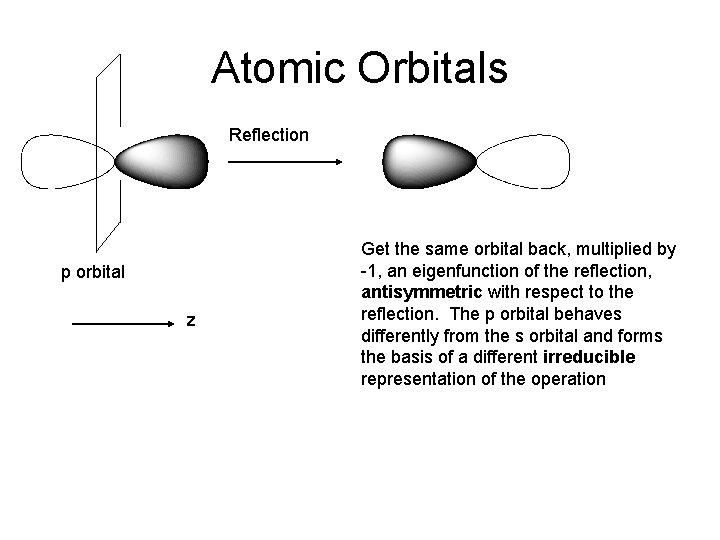
Atomic Orbitals Reflection p orbital z Get the same orbital back, multiplied by -1, an eigenfunction of the reflection, antisymmetric with respect to the reflection. The p orbital behaves differently from the s orbital and forms the basis of a different irreducible representation of the operation

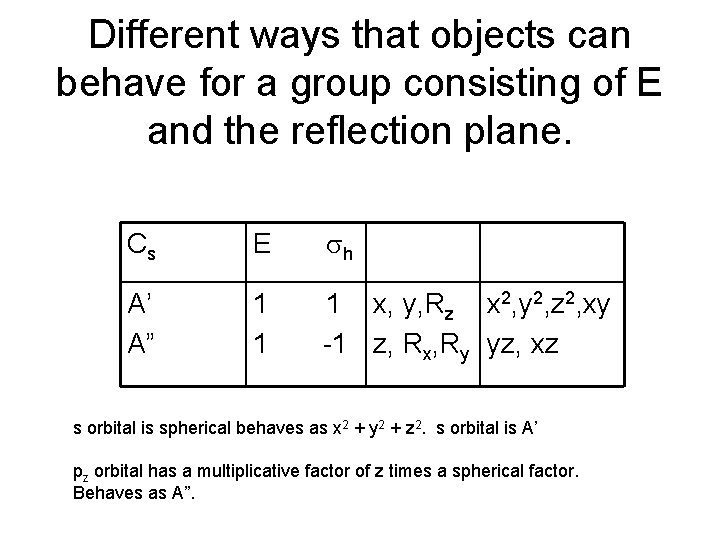
Different ways that objects can behave for a group consisting of E and the reflection plane. Cs E sh A’ A” 1 1 1 x, y, Rz x 2, y 2, z 2, xy -1 z, Rx, Ry yz, xz s orbital is spherical behaves as x 2 + y 2 + z 2. s orbital is A’ pz orbital has a multiplicative factor of z times a spherical factor. Behaves as A”.

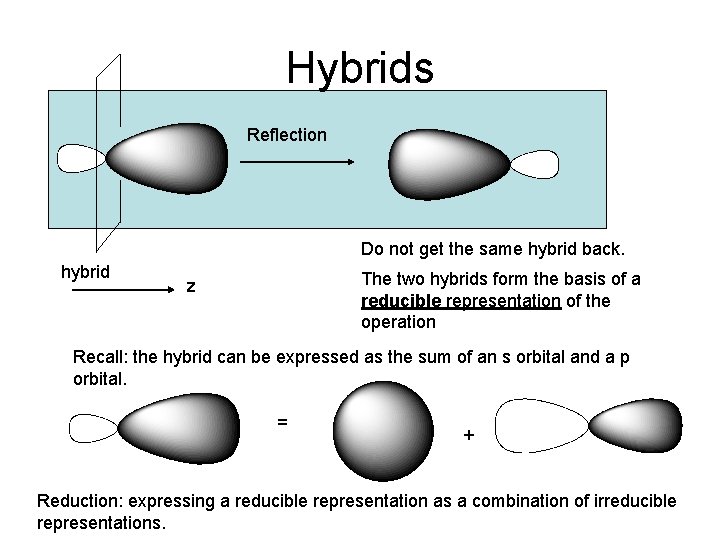
Hybrids Reflection Do not get the same hybrid back. hybrid The two hybrids form the basis of a reducible representation of the operation z Recall: the hybrid can be expressed as the sum of an s orbital and a p orbital. = + Reduction: expressing a reducible representation as a combination of irreducible representations.

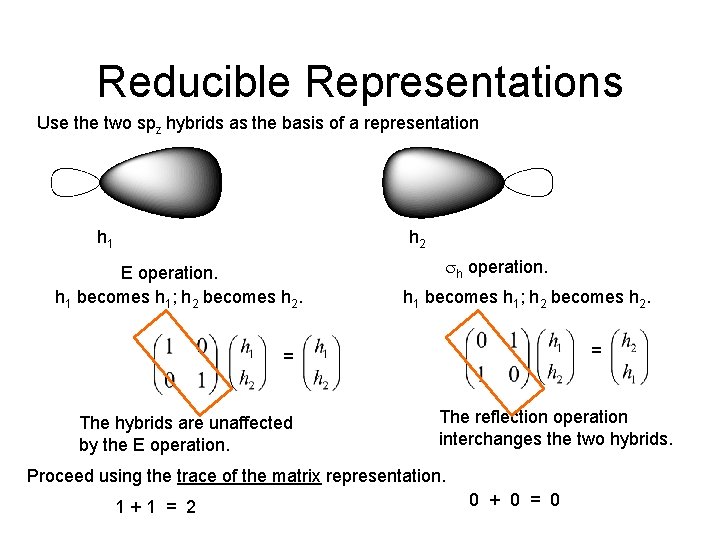
Reducible Representations Use the two spz hybrids as the basis of a representation h 1 h 2 E operation. h 1 becomes h 1; h 2 becomes h 2. sh operation. h 1 becomes h 1; h 2 becomes h 2. = = The hybrids are unaffected by the E operation. The reflection operation interchanges the two hybrids. Proceed using the trace of the matrix representation. 1+1 = 2 0 + 0 = 0

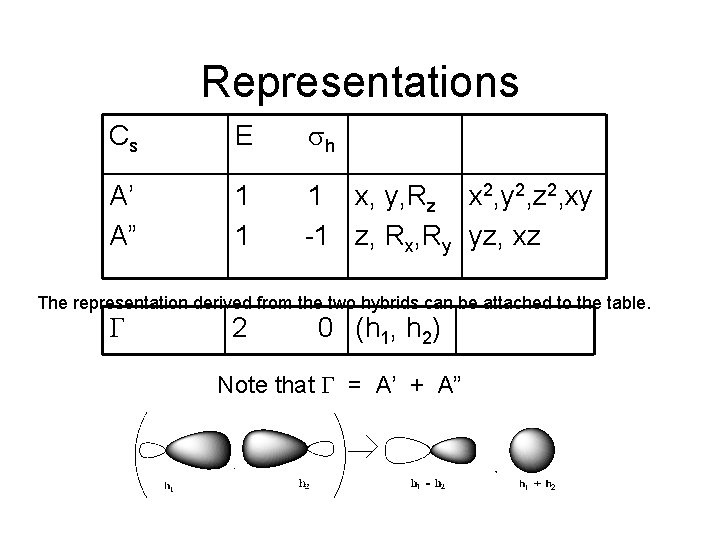
Representations Cs E sh A’ A” 1 1 1 x, y, Rz x 2, y 2, z 2, xy -1 z, Rx, Ry yz, xz The representation derived from the two hybrids can be attached to the table. G 2 0 (h 1, h 2) Note that G = A’ + A”

Character Tables - 3 Irreducible representations are not linear combinations of other representation (Reducible representations are) # of irreducible representations = # of classes of symmetry operations Instead of the matrices, the characters are used (traces of matrices) A character Table is the complete set of irreducible representations of a point group

Character Table Symmetry operations Point group Mülliken symbols Characters +1 symmetric behavior -1 antisymmetric Each row is an irreducible representation

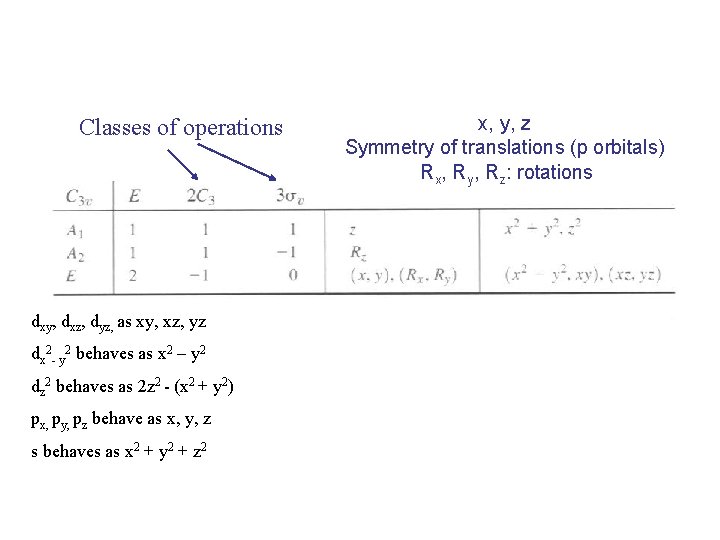
Classes of operations dxy, dxz, dyz, as xy, xz, yz dx 2 - y 2 behaves as x 2 – y 2 dz 2 behaves as 2 z 2 - (x 2 + y 2) px, py, pz behave as x, y, z s behaves as x 2 + y 2 + z 2 x, y, z Symmetry of translations (p orbitals) Rx, Ry, Rz: rotations
 Matrix representation of c3v point group
Matrix representation of c3v point group Specific rotation of sugar solution
Specific rotation of sugar solution Improper rotation axis
Improper rotation axis Ih point group example
Ih point group example Nature of roots of quadratic equation
Nature of roots of quadratic equation Rotation axis
Rotation axis Rotation of a rigid body about a fixed axis
Rotation of a rigid body about a fixed axis Equations of motion rotation about a fixed axis
Equations of motion rotation about a fixed axis Tom suk
Tom suk Rotate about y axis
Rotate about y axis Dark lamp method
Dark lamp method Axis 1 and axis 2 disorders
Axis 1 and axis 2 disorders Four conic sections
Four conic sections A six pole ,60 hz synchoronous machine
A six pole ,60 hz synchoronous machine Axis 1 and axis 2 disorders
Axis 1 and axis 2 disorders What is conjugate axis in hyperbola
What is conjugate axis in hyperbola Principal axis transformation
Principal axis transformation Principal axis graph
Principal axis graph 01:640:244 lecture notes - lecture 15: plat, idah, farad
01:640:244 lecture notes - lecture 15: plat, idah, farad There are some cake
There are some cake Any to any connectivity
Any to any connectivity Seknder
Seknder Right rotation
Right rotation Work done by torque
Work done by torque Translations reflections and rotations
Translations reflections and rotations Hip rom
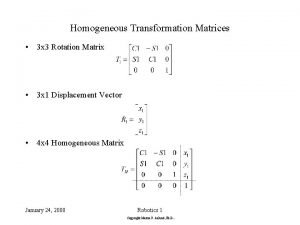
Hip rom Homogeneous transformation matrices
Homogeneous transformation matrices Rotation x y rules
Rotation x y rules Why do we have seaosns
Why do we have seaosns Wind belts
Wind belts Z rotation
Z rotation Kinematik der translationsbewegungen
Kinematik der translationsbewegungen Trunk flexor
Trunk flexor