QUADRATIC EQUATIONS QUADRATIC EQUATIONS CONCEPT OF COMMON ROOTS










- Slides: 43

QUADRATIC EQUATIONS

QUADRATIC EQUATIONS CONCEPT OF COMMON ROOTS

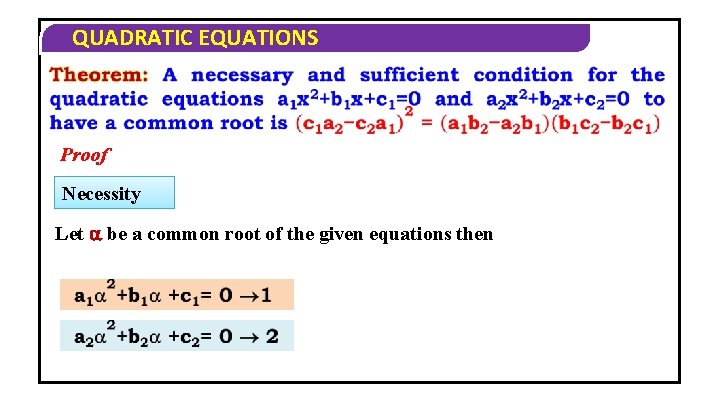
QUADRATIC EQUATIONS Proof Necessity Let be a common root of the given equations then

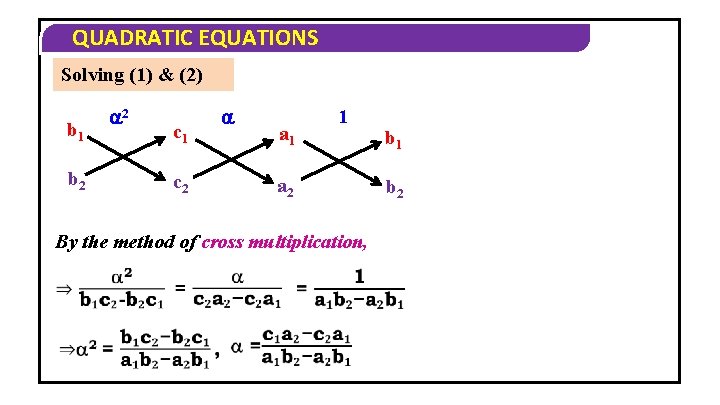
QUADRATIC EQUATIONS Solving (1) & (2) b 1 b 2 2 c 1 c 2 a 1 1 a 2 By the method of cross multiplication, b 1 b 2

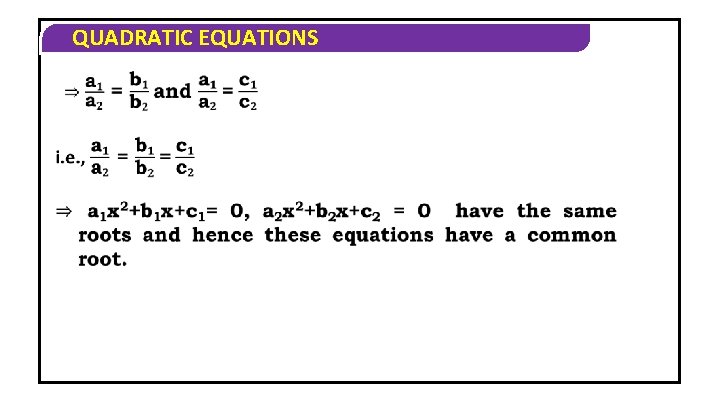
QUADRATIC EQUATIONS Sufficiency Suppose that Case (i) a 1 b 2 – a 2 b 1=0 then c 1 a 2 -c 2 a 1=0 (from 5)

QUADRATIC EQUATIONS

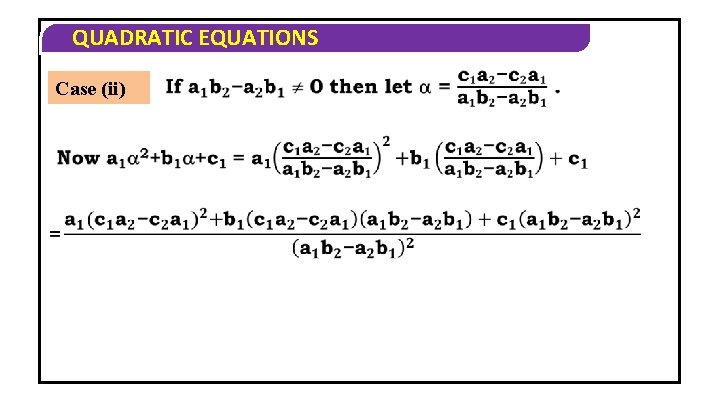
QUADRATIC EQUATIONS Case (ii)

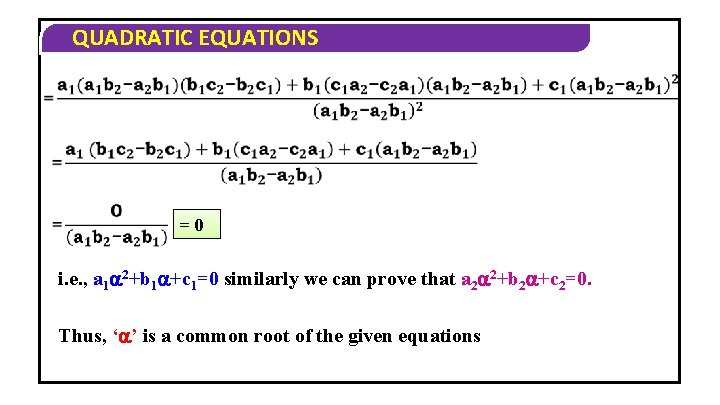
QUADRATIC EQUATIONS =0 i. e. , a 1 2+b 1 +c 1=0 similarly we can prove that a 2 2+b 2 +c 2=0. Thus, ‘ ’ is a common root of the given equations

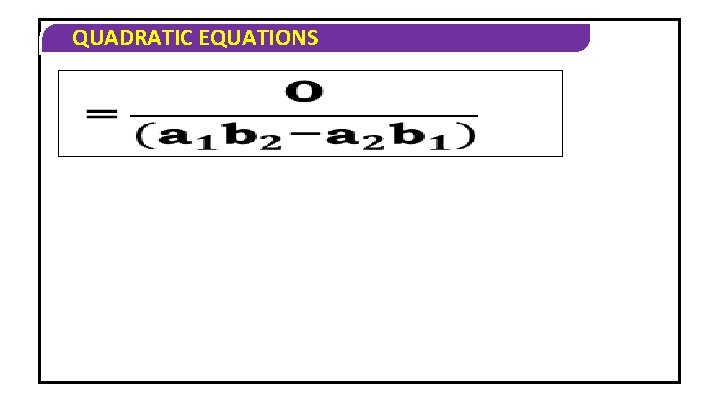
QUADRATIC EQUATIONS 1) If x 2+4 ax+3=0 and 2 x 2+3 ax-9=0 have a common root, then find the values of ‘a’ and the common roots. Solution a =1, b 1=4 a, c 1=3, Here 1 a 2=2, b 2=3 a, c 2=-9 are substitute in the condition

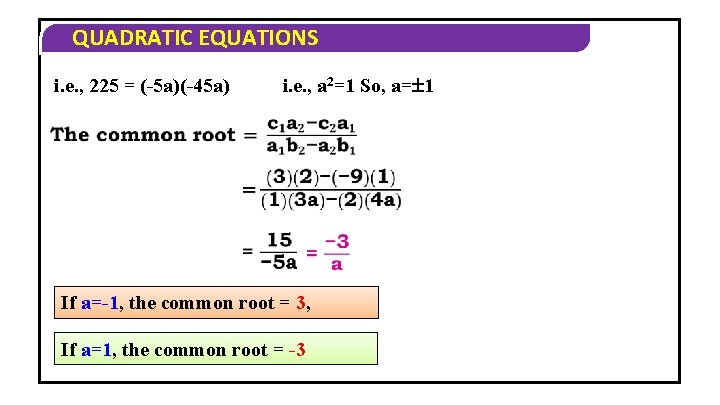
QUADRATIC EQUATIONS i. e. , 225 = (-5 a)(-45 a) i. e. , a 2=1 So, a= 1 If a=-1, the common root = 3, If a=1, the common root = -3

QUADRATIC EQUATIONS APPLICATIONS RELATED TO Q. E.

QUADRATIC EQUATIONS REDUCIBLE TO QUADRATIC EQUATIONS Here is an explanation with some illustrations how to solve some equations which are reducible to quadratic equations by suitable substitutions.

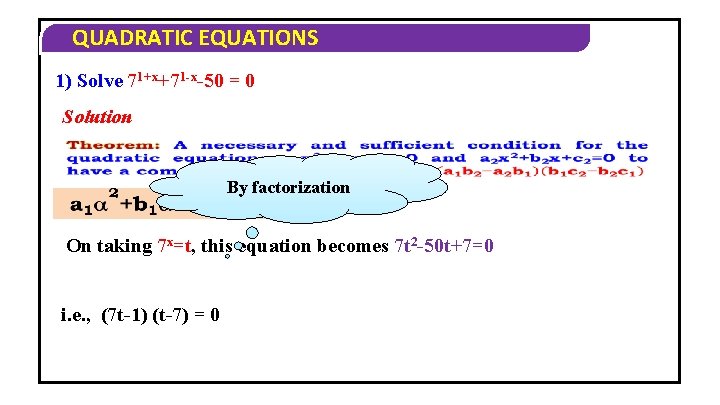
QUADRATIC EQUATIONS 1) Solve 71+x+71 -x-50 = 0 Solution By factorization On taking 7 x=t, this equation becomes 7 t 2 -50 t+7=0 i. e. , (7 t-1) (t-7) = 0

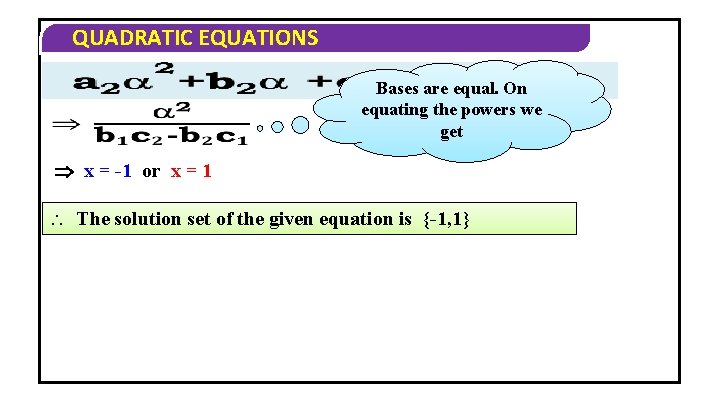
QUADRATIC EQUATIONS Bases are equal. On equating the powers we get x = -1 or x = 1 The solution set of the given equation is {-1, 1}

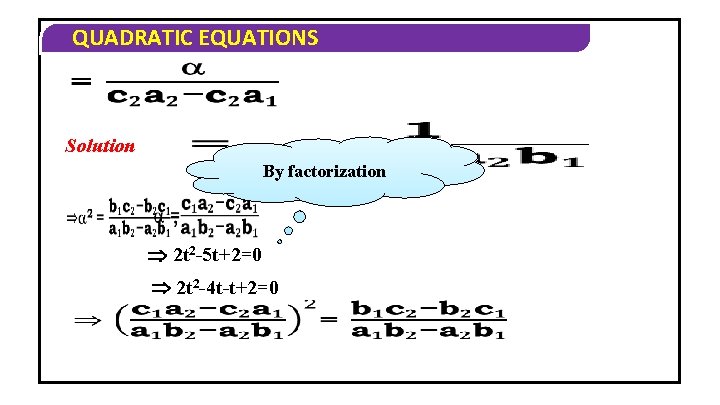
QUADRATIC EQUATIONS Solution By factorization 2 t 2 -5 t+2=0 2 t 2 -4 t-t+2=0

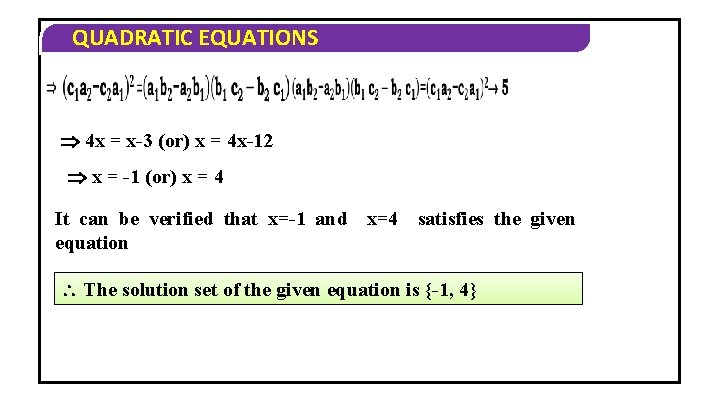
QUADRATIC EQUATIONS 4 x = x-3 (or) x = 4 x-12 x = -1 (or) x = 4 It can be verified that x=-1 and x=4 satisfies the given equation The solution set of the given equation is {-1, 4}

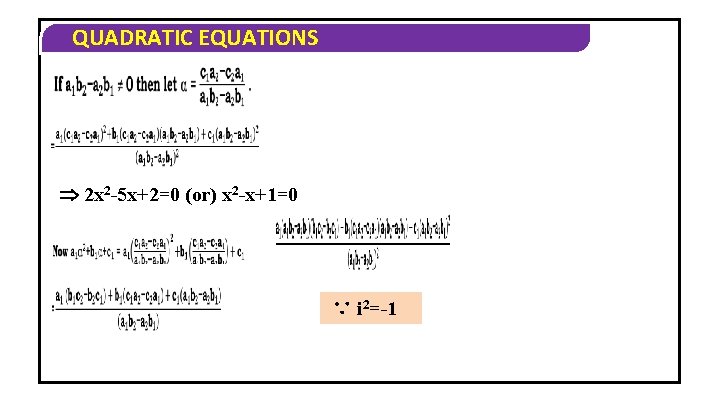
QUADRATIC EQUATIONS Solution 2 t 2 -7 t+5=0 (2 t-5)(t-1)=0 By factorization

QUADRATIC EQUATIONS 2 x 2 -5 x+2=0 (or) x 2 -x+1=0 ∵ i 2=-1

QUADRATIC EQUATIONS

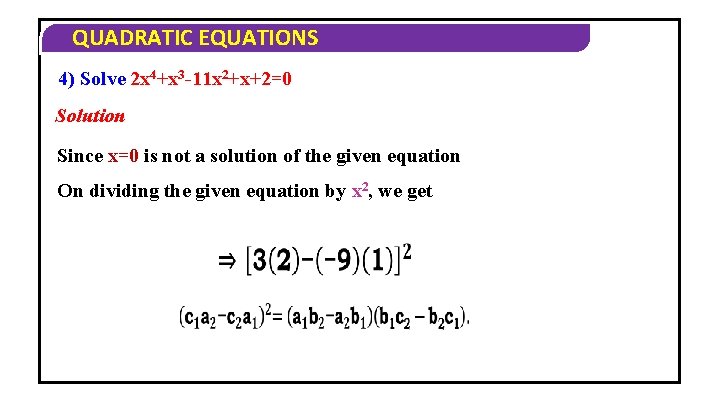
QUADRATIC EQUATIONS 4) Solve 2 x 4+x 3 -11 x 2+x+2=0 Solution Since x=0 is not a solution of the given equation On dividing the given equation by x 2, we get

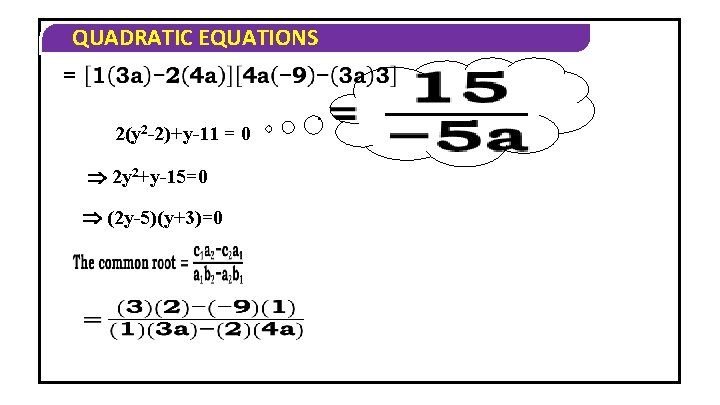
QUADRATIC EQUATIONS 2(y 2 -2)+y-11 = 0 2 y 2+y-15=0 (2 y-5)(y+3)=0

QUADRATIC EQUATIONS 2 x 2 -5 x+2=0 (or) x 2+3 x+1=0

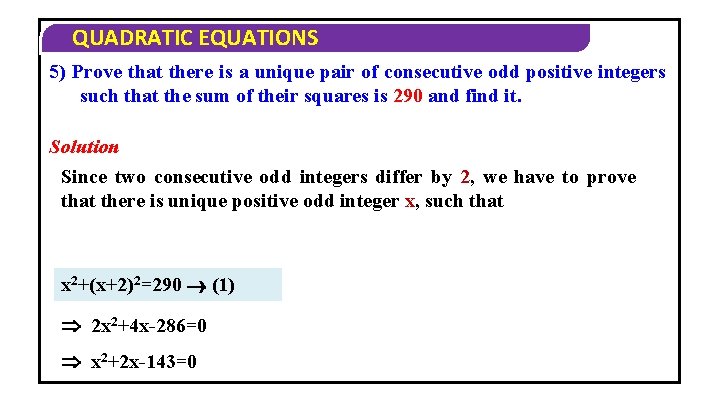
QUADRATIC EQUATIONS 5) Prove that there is a unique pair of consecutive odd positive integers such that the sum of their squares is 290 and find it. Solution Since two consecutive odd integers differ by 2, we have to prove that there is unique positive odd integer x, such that x 2+(x+2)2=290 (1) 2 x 2+4 x-286=0 x 2+2 x-143=0

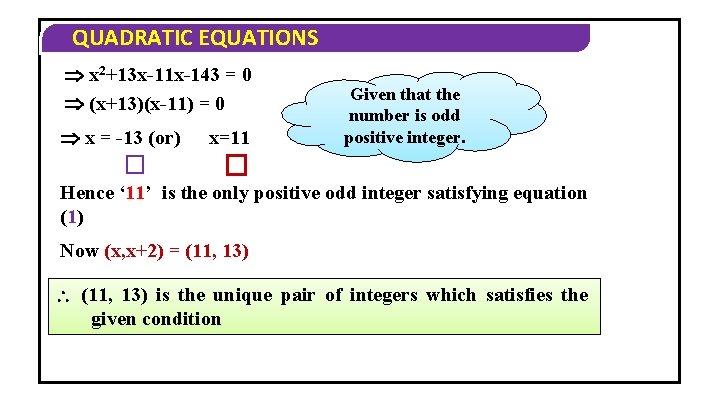
QUADRATIC EQUATIONS x 2+13 x-11 x-143 = 0 (x+13)(x-11) = 0 x = -13 (or) � x=11 Given that the number is odd positive integer. � Hence ‘ 11’ is the only positive odd integer satisfying equation (1) Now (x, x+2) = (11, 13) is the unique pair of integers which satisfies the given condition

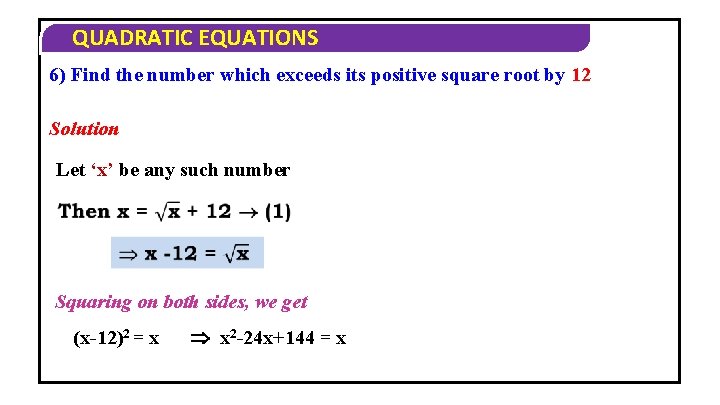
QUADRATIC EQUATIONS 6) Find the number which exceeds its positive square root by 12 Solution Let ‘x’ be any such number Squaring on both sides, we get (x-12)2 = x x 2 -24 x+144 = x

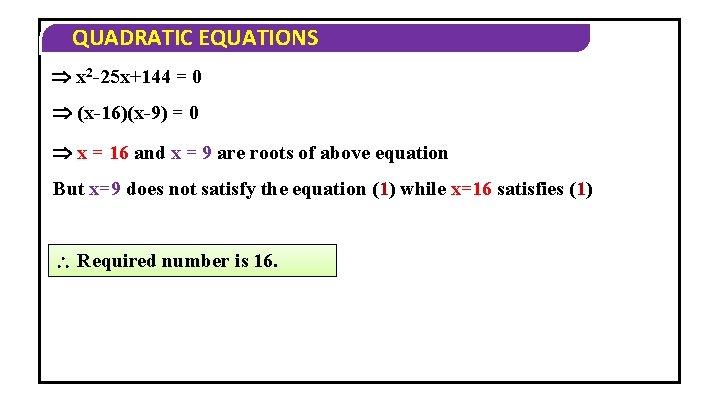
QUADRATIC EQUATIONS x 2 -25 x+144 = 0 (x-16)(x-9) = 0 x = 16 and x = 9 are roots of above equation But x=9 does not satisfy the equation (1) while x=16 satisfies (1) Required number is 16.

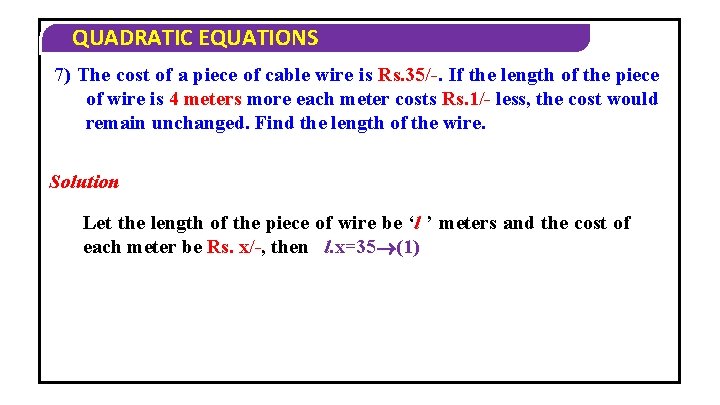
QUADRATIC EQUATIONS 7) The cost of a piece of cable wire is Rs. 35/-. If the length of the piece of wire is 4 meters more each meter costs Rs. 1/- less, the cost would remain unchanged. Find the length of the wire. Solution Let the length of the piece of wire be ‘l ’ meters and the cost of each meter be Rs. x/-, then l. x=35 (1)

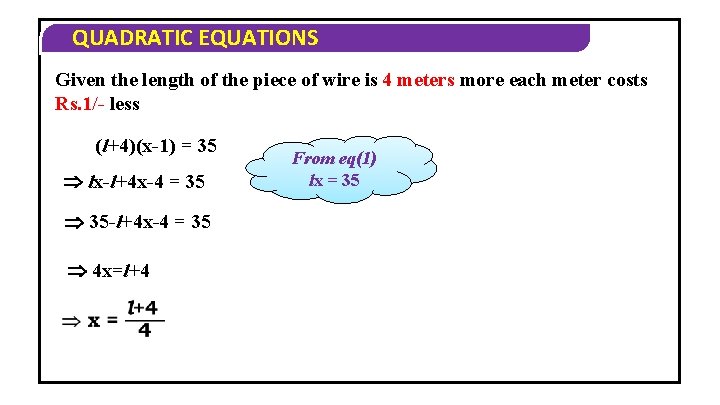
QUADRATIC EQUATIONS Given the length of the piece of wire is 4 meters more each meter costs Rs. 1/- less (l+4)(x-1) = 35 lx-l+4 x-4 = 35 35 -l+4 x-4 = 35 4 x=l+4 From eq(1) lx = 35

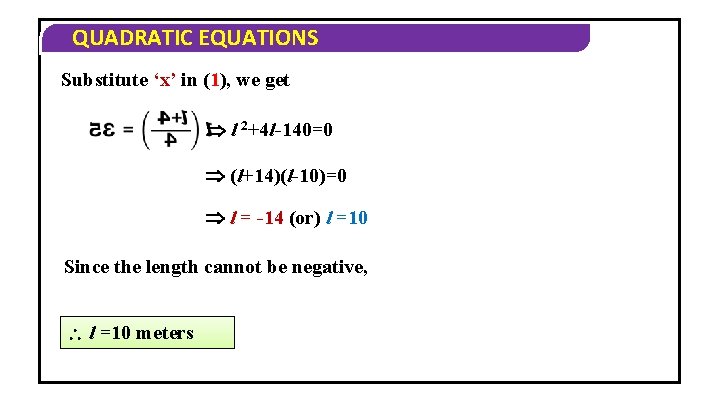
QUADRATIC EQUATIONS Substitute ‘x’ in (1), we get l 2+4 l-140=0 (l+14)(l-10)=0 l = -14 (or) l =10 Since the length cannot be negative, l =10 meters

QUADRATIC EQUATIONS 8) If x 1, x 2 are the roots of ax 2+bx+c=0 then find the value of (ax 1+b)2+(ax +b)-2 2 Solution x 1 is the root of ax 2+bx+c=0 x 1(ax 1+b) = -c ax 12+bx 1+c=0

QUADRATIC EQUATIONS

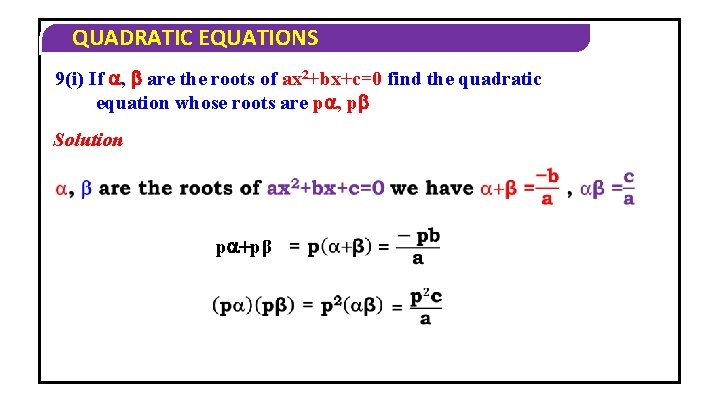
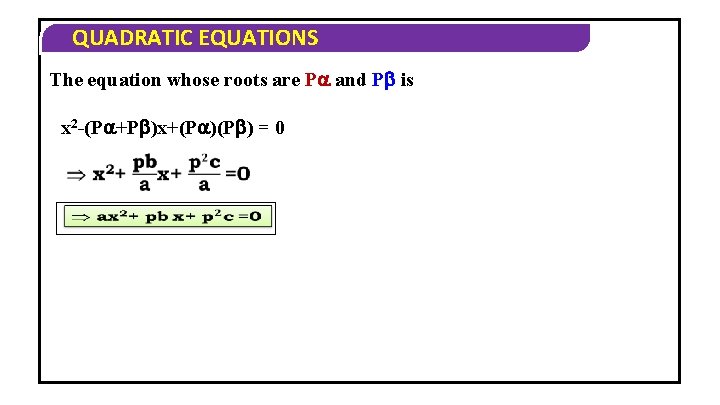
QUADRATIC EQUATIONS 9(i) If , are the roots of ax 2+bx+c=0 find the quadratic equation whose roots are p , p Solution p pβ

QUADRATIC EQUATIONS The equation whose roots are P and P is x 2 -(P +P )x+(P ) = 0

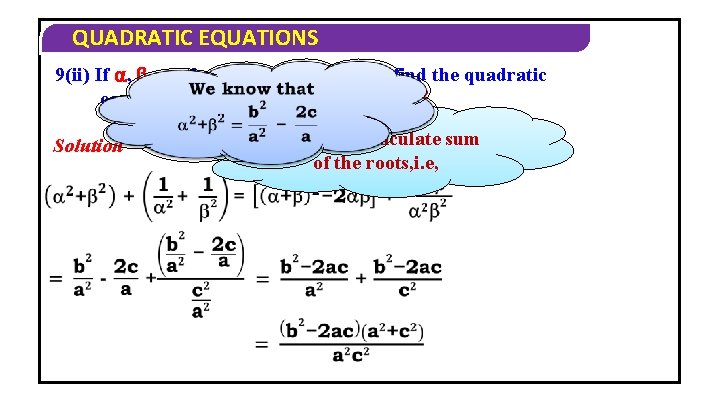
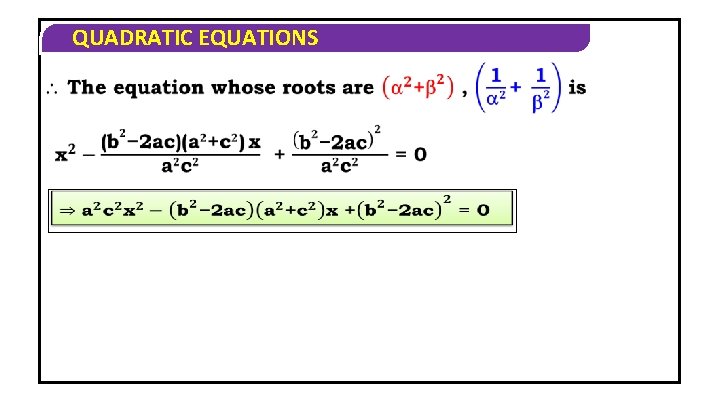
QUADRATIC EQUATIONS 9(ii) If , are the roots of ax 2+bx+c=0 find the quadratic equation whose roots are 2+ 2, -2+ -2 Solution Firstly we calculate sum of the roots, i. e,

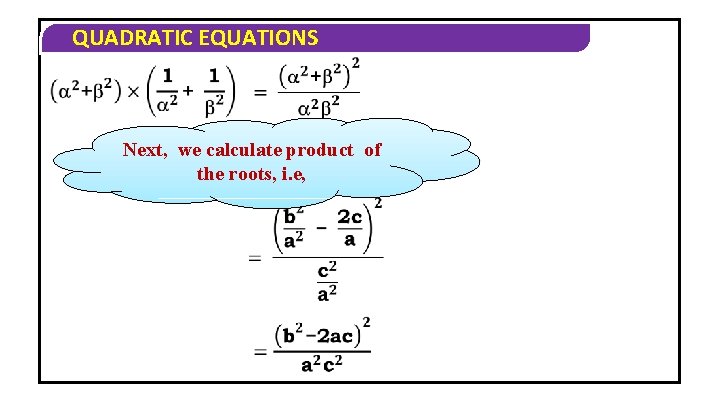
QUADRATIC EQUATIONS Next, we calculate product of the roots, i. e,

QUADRATIC EQUATIONS

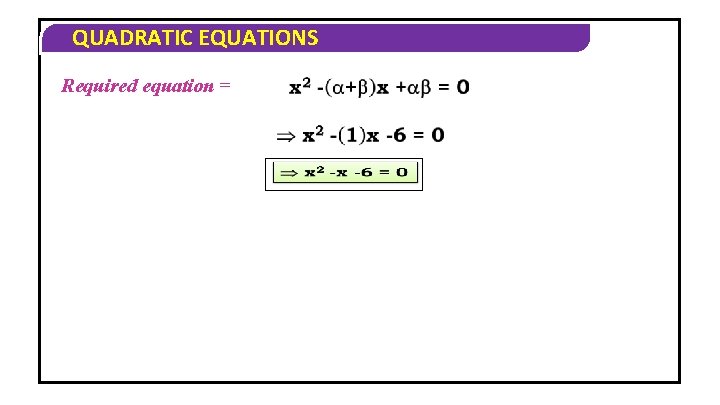
QUADRATIC EQUATIONS 10) Find the quadratic equation for which the sum of the roots is 1 and the sum of the squares of the roots is 13. Solution Let and be the roots of the required equation, then + =1 2+ 2 =13 = -6

QUADRATIC EQUATIONS Required equation =

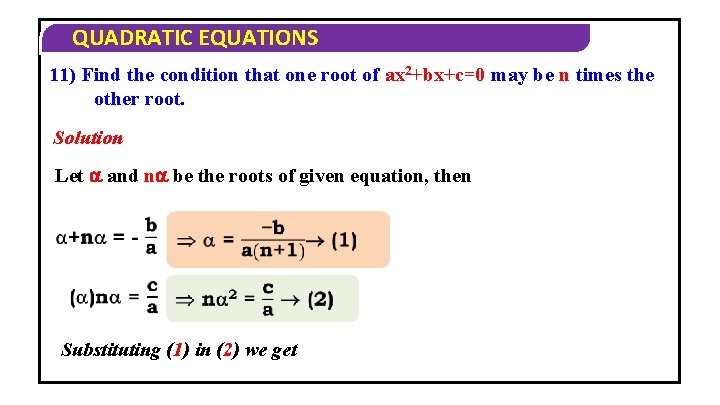
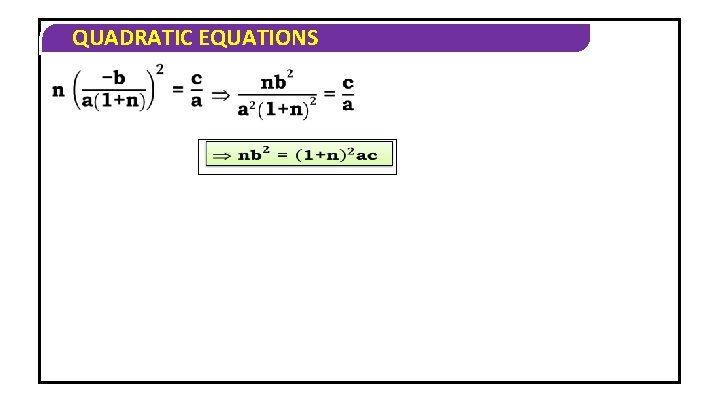
QUADRATIC EQUATIONS 11) Find the condition that one root of ax 2+bx+c=0 may be n times the other root. Solution Let and n be the roots of given equation, then Substituting (1) in (2) we get

QUADRATIC EQUATIONS

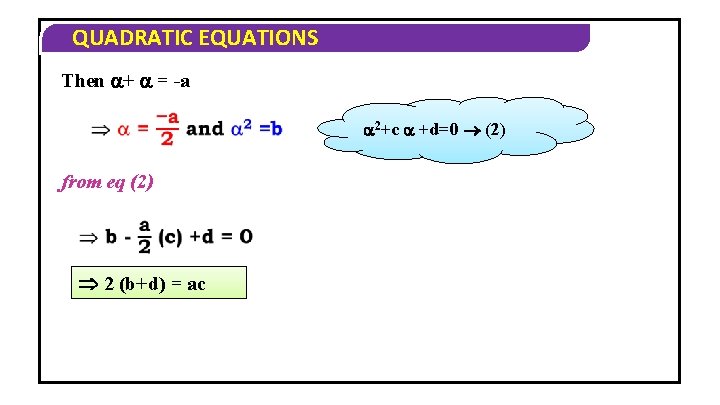
QUADRATIC EQUATIONS 12) If the equation x 2+ax+b=0 and x 2+cx+d=0 have a common root and the first equation has equal roots then prove that 2(b+d)=ac Solution Let be a common root of x 2+ax+b=0 and x 2+cx+d=0 then 2+a +b=0 (1) 2+c +d=0 (2) But first equation has equal roots

QUADRATIC EQUATIONS Then + = -a 2+c +d=0 (2) from eq (2) 2 (b+d) = ac

QUADRATIC EQUATIONS Thank you…
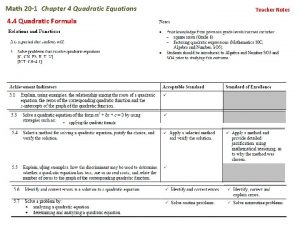
 Solving quadratic equations with square roots
Solving quadratic equations with square roots 9-7 solving quadratic equations by using square roots
9-7 solving quadratic equations by using square roots Completing the square (continued) quiz
Completing the square (continued) quiz 9-7 solving quadratic equations by using square roots
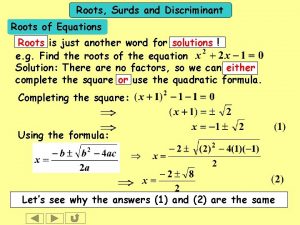
9-7 solving quadratic equations by using square roots Quadratic equation roots
Quadratic equation roots Chonp
Chonp Estimating square roots guided notes
Estimating square roots guided notes Existence and uniqueness of square roots and cube roots
Existence and uniqueness of square roots and cube roots The roots of american imperialism 1. economic roots
The roots of american imperialism 1. economic roots All the perfect squares
All the perfect squares Factoring method quadratic equations
Factoring method quadratic equations Quadratic graphs roots and turning points
Quadratic graphs roots and turning points Graph of y=f(x)
Graph of y=f(x) Factorise quadratic equations
Factorise quadratic equations Real roots
Real roots حل معادله درجه 2
حل معادله درجه 2 Roots and zeros
Roots and zeros Solving equations using nth roots
Solving equations using nth roots What are the roots of a polynomial function
What are the roots of a polynomial function 5-5 theorems about roots of polynomial equations
5-5 theorems about roots of polynomial equations Real roots
Real roots Finding the real roots of polynomial equations
Finding the real roots of polynomial equations 6-5 finding real roots of polynomial equations
6-5 finding real roots of polynomial equations Finding real roots of polynomial equations
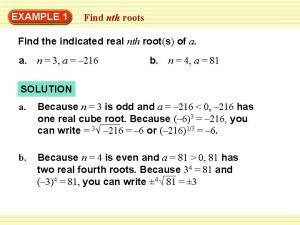
Finding real roots of polynomial equations How to find the indicated real nth roots of a
How to find the indicated real nth roots of a Solving square root and other radical equations
Solving square root and other radical equations Lcm for 4 and 12
Lcm for 4 and 12 Common anode and common cathode
Common anode and common cathode Factors of 60 and 72
Factors of 60 and 72 What are the factors for 54
What are the factors for 54 The gcf of 12 and 18
The gcf of 12 and 18 Highest common factors and lowest common multiples
Highest common factors and lowest common multiples Ideal self description
Ideal self description Contoh selling concept
Contoh selling concept Concept map of some applications of trigonometry class 10
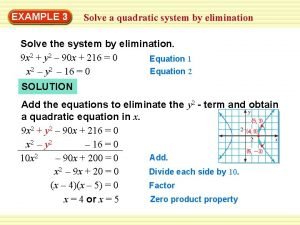
Concept map of some applications of trigonometry class 10 Solving quadratic systems by elimination
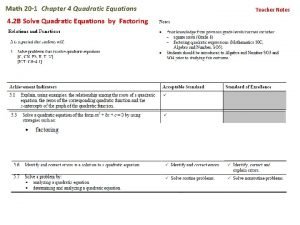
Solving quadratic systems by elimination Unit 4 lesson 4 factoring to solve quadratic equations
Unit 4 lesson 4 factoring to solve quadratic equations Minus b formula
Minus b formula Solving quadratic equations algebraically
Solving quadratic equations algebraically My hrw
My hrw Factoring zero product property
Factoring zero product property Factoring steps
Factoring steps 5-3 solving quadratic equations by factoring answer key
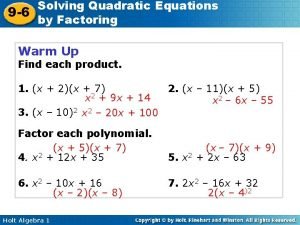
5-3 solving quadratic equations by factoring answer key 9-6 solving quadratic equations by factoring
9-6 solving quadratic equations by factoring