9 7 Completing the Square Preview Warm Up



















- Slides: 45

9 -7 Completing the Square Preview Warm Up California Standards Lesson Presentation

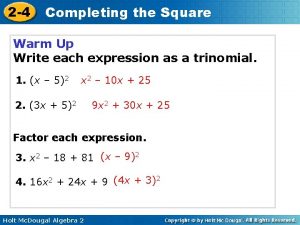
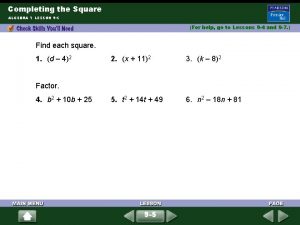
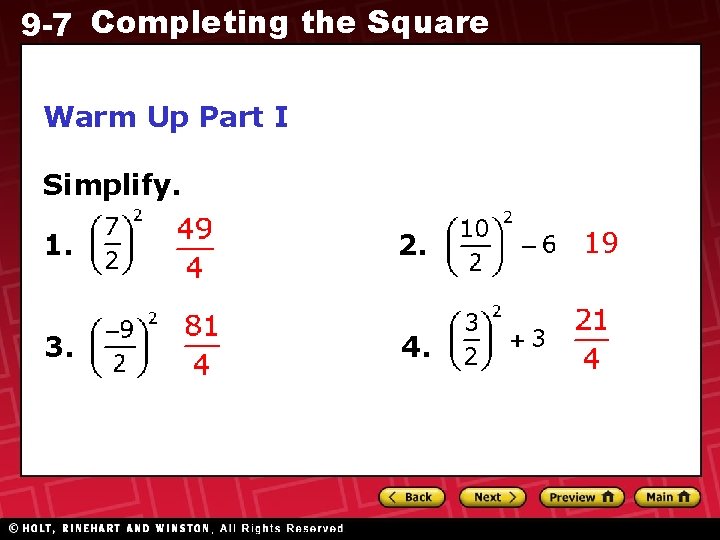
9 -7 Completing the Square Warm Up Part I Simplify. 1. 2. 3. 4. 19

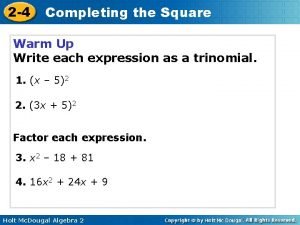
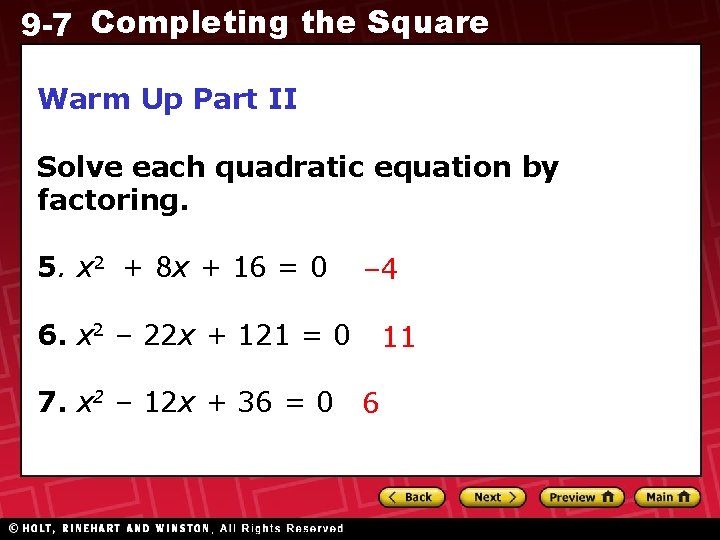
9 -7 Completing the Square Warm Up Part II Solve each quadratic equation by factoring. 5. x 2 + 8 x + 16 = 0 – 4 6. x 2 – 22 x + 121 = 0 7. x 2 – 12 x + 36 = 0 11 6

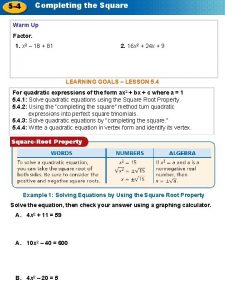
9 -7 Completing the Square California Standards 14. 0 Students solve a quadratic equation by factoring or completing the square. 23. 0 Students apply quadratic equations to physical problems, such as the motion of an object under the force of gravity. Also covered: 2. 0

9 -7 Completing the Square Vocabulary completing the square

9 -7 Completing the Square In the previous lesson, you solved quadratic equations by isolating x 2 and then using square roots. This method also works if the quadratic equation, when written in standard form, is a perfect square.

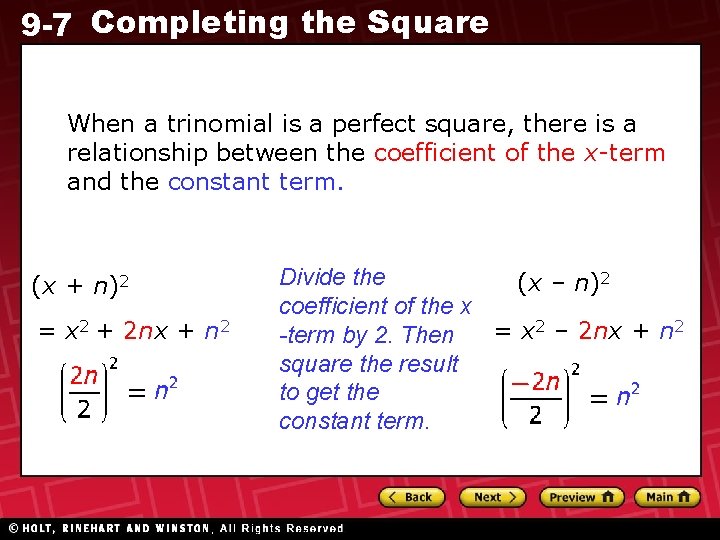
9 -7 Completing the Square When a trinomial is a perfect square, there is a relationship between the coefficient of the x-term and the constant term. (x + n)2 = x 2 + 2 nx + n 2 Divide the (x – n)2 coefficient of the x = x 2 – 2 nx + n 2 -term by 2. Then square the result to get the constant term.

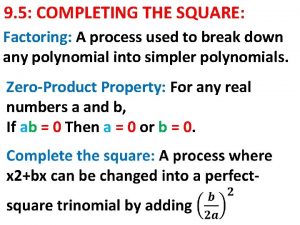
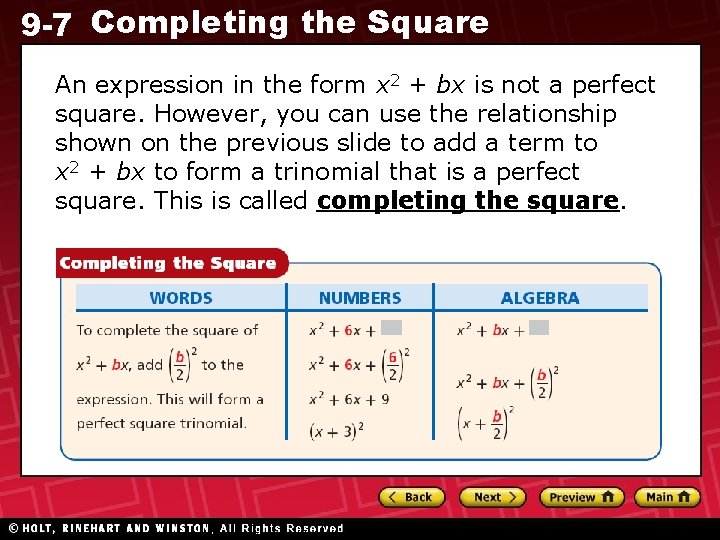
9 -7 Completing the Square An expression in the form x 2 + bx is not a perfect square. However, you can use the relationship shown on the previous slide to add a term to x 2 + bx to form a trinomial that is a perfect square. This is called completing the square.

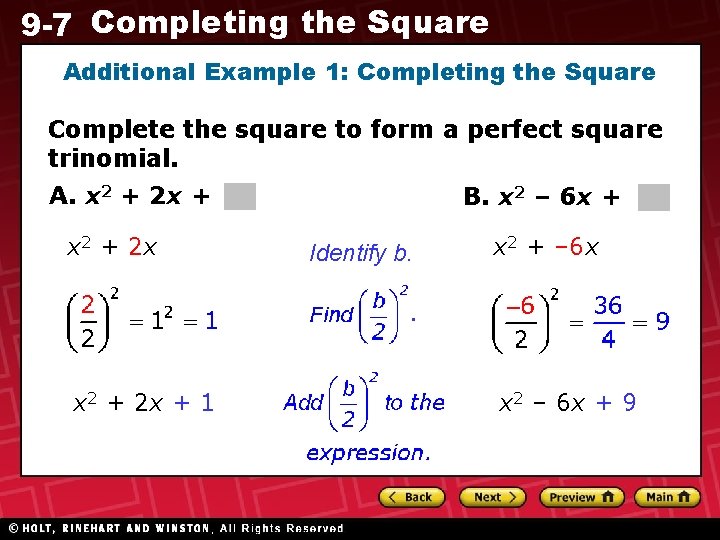
9 -7 Completing the Square Additional Example 1: Completing the Square Complete the square to form a perfect square trinomial. A. x 2 + 2 x + x 2 + 2 x B. x 2 – 6 x + Identify b. x 2 + – 6 x . x 2 + 2 x + 1 x 2 – 6 x + 9

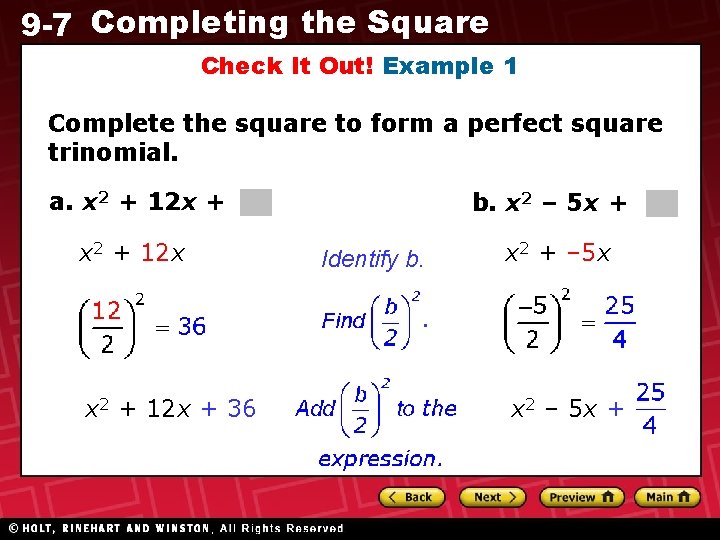
9 -7 Completing the Square Check It Out! Example 1 Complete the square to form a perfect square trinomial. a. x 2 + 12 x + x 2 + 12 x b. x 2 – 5 x + Identify b. x 2 + – 5 x . x 2 + 12 x + 36 x 2 – 5 x +

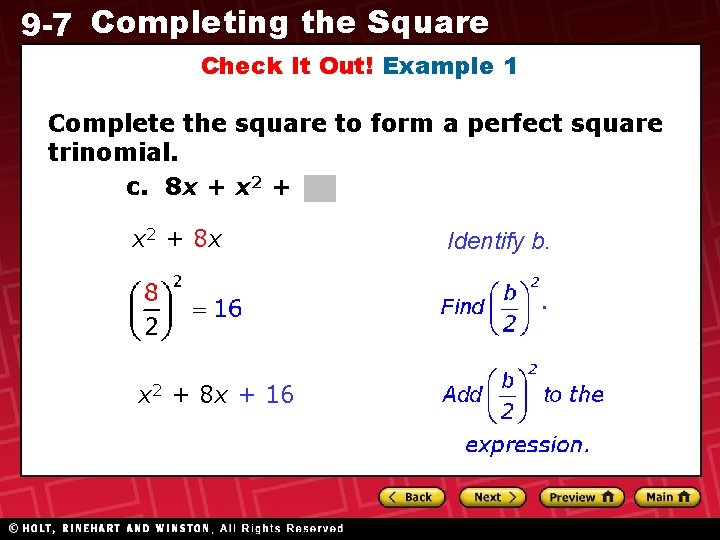
9 -7 Completing the Square Check It Out! Example 1 Complete the square to form a perfect square trinomial. c. 8 x + x 2 + 8 x Identify b. . x 2 + 8 x + 16

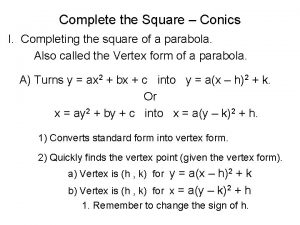
9 -7 Completing the Square To solve a quadratic equation in the form x 2 + bx = c, first complete the square of x 2 + bx. Then you can solve using square roots.

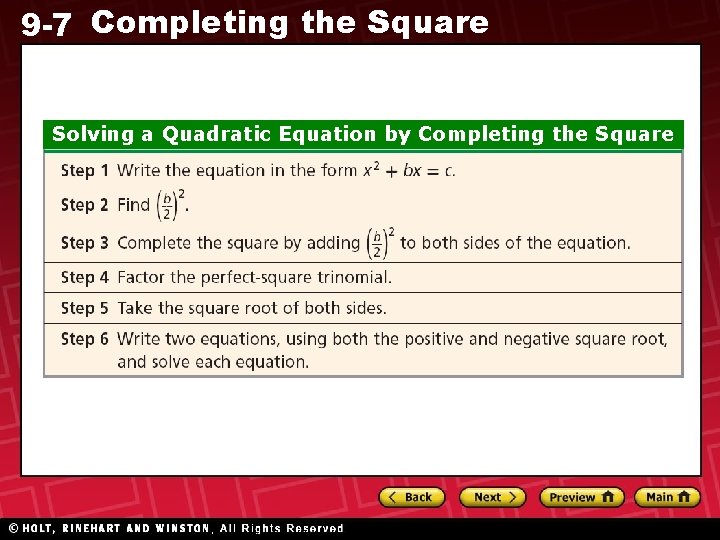
9 -7 Completing the Square Solving a Quadratic Equation by Completing the Square

9 -7 Completing the Square Additional Example 2 A: Solving x 2 +bx = c by Completing the Square Solve by completing the square. Check your answer. x 2 + 16 x = – 15 Step 1 x 2 + 16 x = – 15 The equation is in the form x 2 + bx = c. Step 2 . Step 3 x 2 + 16 x + 64 = – 15 + 64 Complete the square. Step 4 (x + 8)2 = 49 Step 5 x + 8 = ± 7 Step 6 x + 8 = 7 or x + 8 = – 7 x = – 1 or x = – 15 Factor and simplify. Take the square root of both sides. Write and solve two equations.

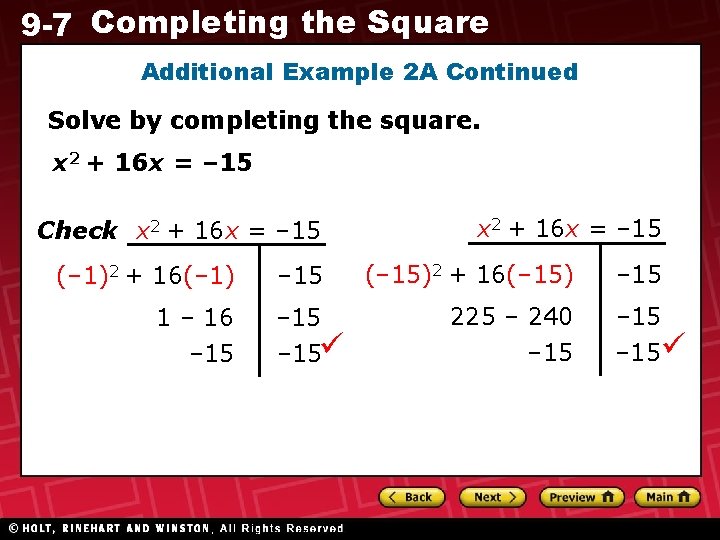
9 -7 Completing the Square Additional Example 2 A Continued Solve by completing the square. x 2 + 16 x = – 15 Check x 2 + 16 x = – 15 (– 1)2 + 16(– 1) 1 – 16 – 15 x 2 + 16 x = – 15 (– 15)2 + 16(– 15) 225 – 240 – 15

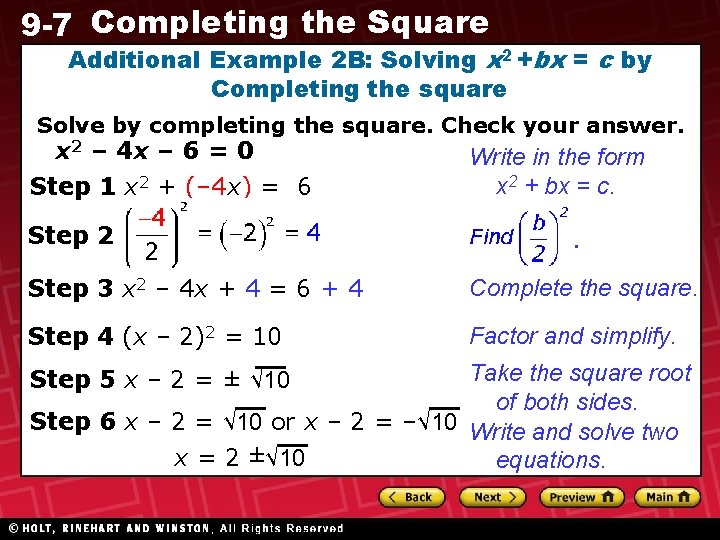
9 -7 Completing the Square Additional Example 2 B: Solving x 2 +bx = c by Completing the square Solve by completing the square. Check your answer. x 2 – 4 x – 6 = 0 Step 1 x 2 + (– 4 x) = 6 Step 2 Write in the form x 2 + bx = c. . Step 3 x 2 – 4 x + 4 = 6 + 4 Complete the square. Step 4 (x – 2)2 = 10 Factor and simplify. Take the square root of both sides. Step 6 x – 2 = √ 10 or x – 2 = –√ 10 Write and solve two x = 2 ±√ 10 equations. Step 5 x – 2 = ± √ 10

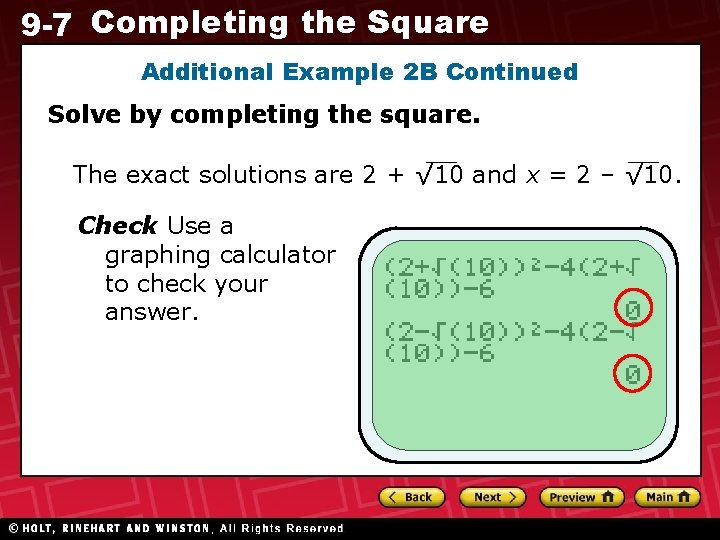
9 -7 Completing the Square Additional Example 2 B Continued Solve by completing the square. The exact solutions are 2 + √ 10 and x = 2 – √ 10. Check Use a graphing calculator to check your answer.

9 -7 Completing the Square Writing Math The expressions and can be written as one expression: , which is read as “ 1 plus or minus the square root of 3. ”

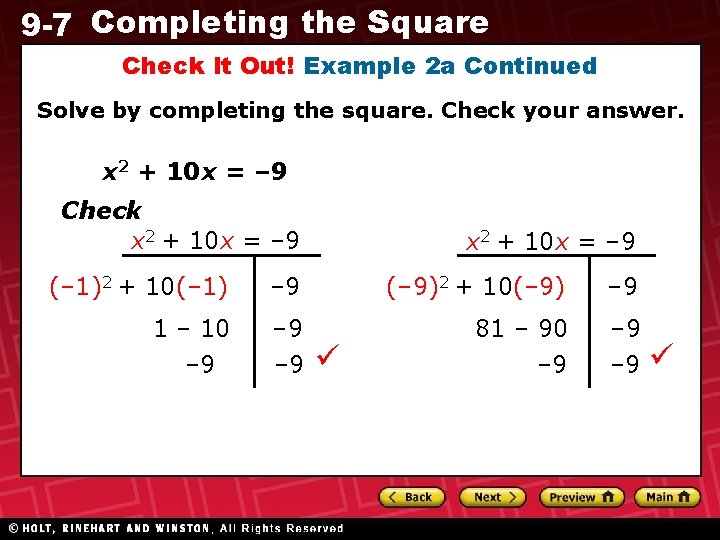
9 -7 Completing the Square Check It Out! Example 2 a Solve by completing the square. Check your answer. x 2 + 10 x = – 9 Step 1 x 2 + 10 x = – 9 Step 2 Step 3 x 2 + 10 x + 25 = – 9 + 25 Step 4 (x + 5)2 = 16 Step 5 x + 5 = ± 4 Step 6 x + 5 = 4 or x + 5 = – 4 x = – 1 or x = – 9 The equation is in the form x 2 + bx = c. . Complete the square. Factor and simplify. Take the square root of both sides. Write and solve two equations.

9 -7 Completing the Square Check It Out! Example 2 a Continued Solve by completing the square. Check your answer. x 2 + 10 x = – 9 Check x 2 + 10 x = – 9 (– 1)2 + 10(– 1) – 9 (– 9)2 + 10(– 9) – 9 1 – 10 – 9 – 9 81 – 90 – 9 – 9

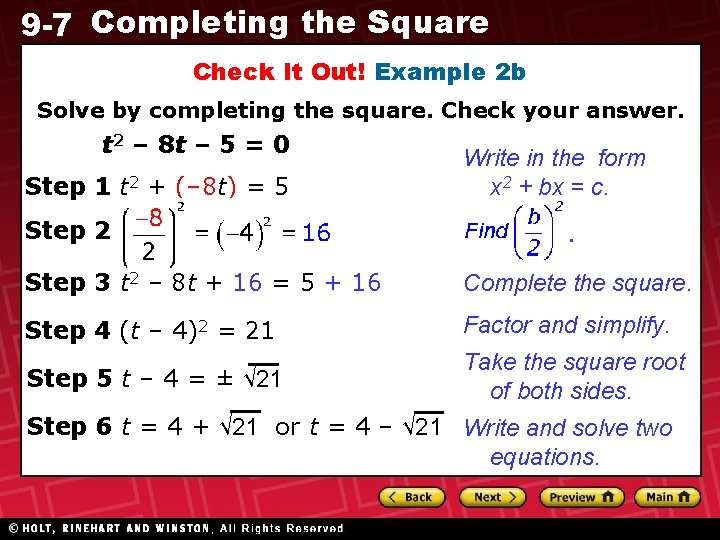
9 -7 Completing the Square Check It Out! Example 2 b Solve by completing the square. Check your answer. t 2 – 8 t – 5 = 0 Step 1 t 2 + (– 8 t) = 5 Step 2 Write in the form x 2 + bx = c. . Step 3 t 2 – 8 t + 16 = 5 + 16 Complete the square. Step 4 (t – 4)2 = 21 Factor and simplify. Step 5 t – 4 = ± √ 21 Take the square root of both sides. Step 6 t = 4 + √ 21 or t = 4 – √ 21 Write and solve two equations.

9 -7 Completing the Square Check It Out! Example 2 b Continued Solve by completing the square. Check your answer. The exact solutions are 4 – √ 21 and 4 + √ 21. Check Use a graphing calculator to check your answer.

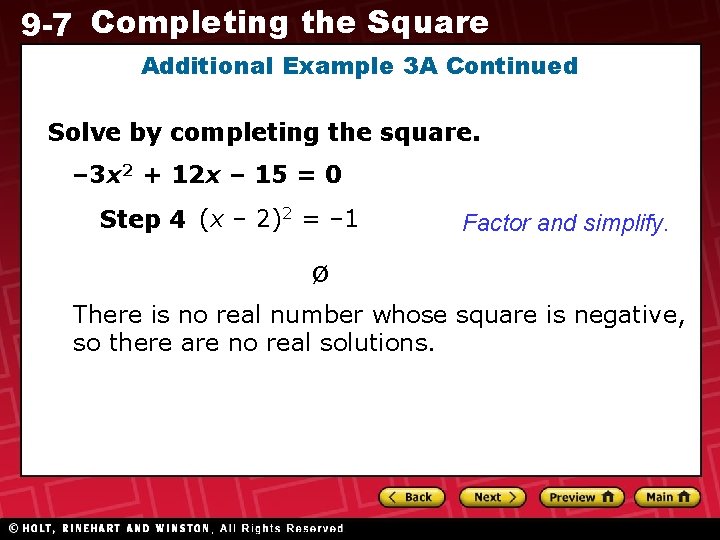
9 -7 Completing the Square Additional Example 3 A: Solving ax 2 + bx = c by Completing the Square Solve by completing the square. – 3 x 2 + 12 x – 15 = 0 Step 1 x 2 – 4 x + 5 = 0 x 2 – 4 x = – 5 x 2 + (– 4 x) = – 5 Step 2 Step 3 Divide both sides of the equation by – 3 so that a = 1. Write in the form x 2 + bx = c. . x 2 – 4 x + 4 = – 5 + 4 Complete the square by adding 4 to both sides.

9 -7 Completing the Square Additional Example 3 A Continued Solve by completing the square. – 3 x 2 + 12 x – 15 = 0 Step 4 (x – 2)2 = – 1 Factor and simplify. ø There is no real number whose square is negative, so there are no real solutions.

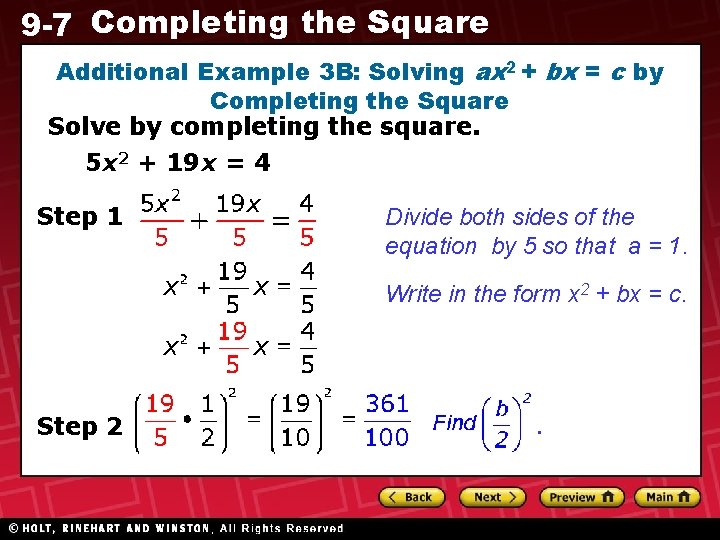
9 -7 Completing the Square Additional Example 3 B: Solving ax 2 + bx = c by Completing the Square Solve by completing the square. 5 x 2 + 19 x = 4 Step 1 Divide both sides of the equation by 5 so that a = 1. Write in the form x 2 + bx = c. Step 2 .

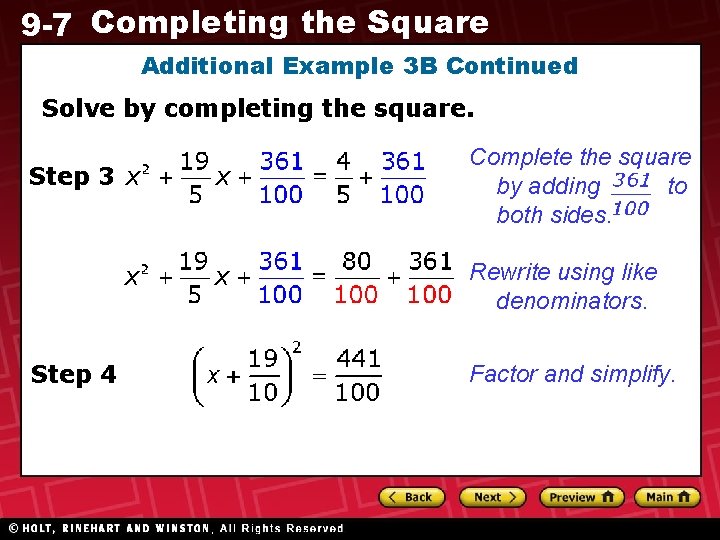
9 -7 Completing the Square Additional Example 3 B Continued Solve by completing the square. Step 3 Complete the square by adding to both sides. Rewrite using like denominators. Step 4 Factor and simplify.

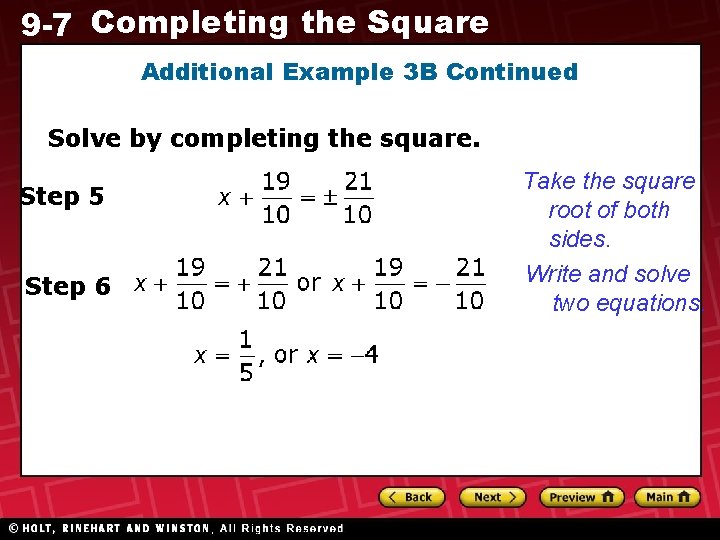
9 -7 Completing the Square Additional Example 3 B Continued Solve by completing the square. Step 5 Step 6 Take the square root of both sides. Write and solve two equations.

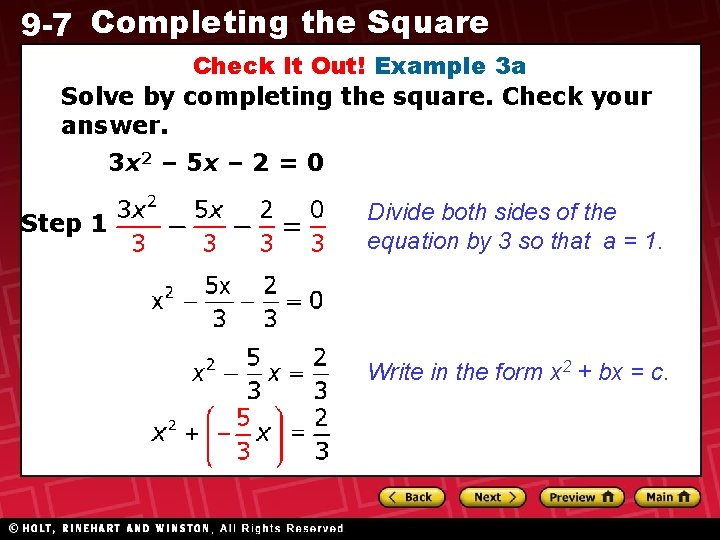
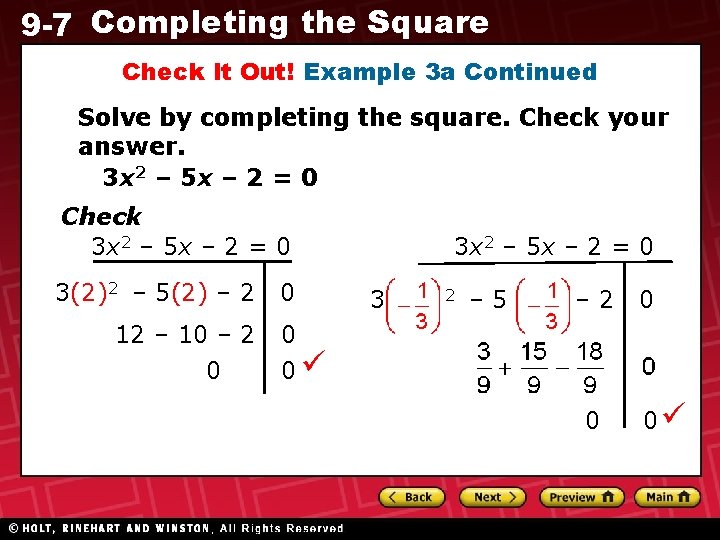
9 -7 Completing the Square Check It Out! Example 3 a Solve by completing the square. Check your answer. 3 x 2 – 5 x – 2 = 0 Step 1 Divide both sides of the equation by 3 so that a = 1. Write in the form x 2 + bx = c.

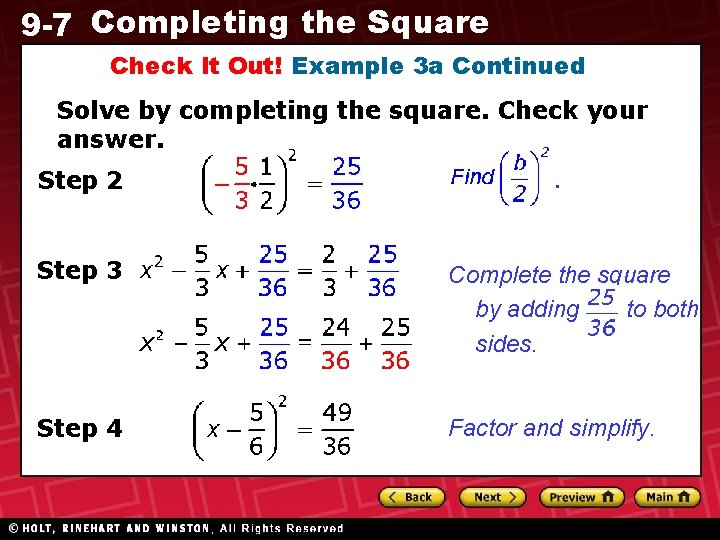
9 -7 Completing the Square Check It Out! Example 3 a Continued Solve by completing the square. Check your answer. Step 2 . Step 3 Complete the square by adding to both sides. Step 4 Factor and simplify.

9 -7 Completing the Square Check It Out! Example 3 a Continued Solve by completing the square. Check your answer. Step 5 Take the square root of both sides. Step 6 Write and solve two equations.

9 -7 Completing the Square Check It Out! Example 3 a Continued Solve by completing the square. Check your answer. 3 x 2 – 5 x – 2 = 0 Check 3 x 2 – 5 x – 2 = 0 3(2)2 – 5(2) – 2 12 – 10 – 2 0 0 3 x 2 – 5 x – 2 = 0 3 2 – 5 – 2 0 0 0

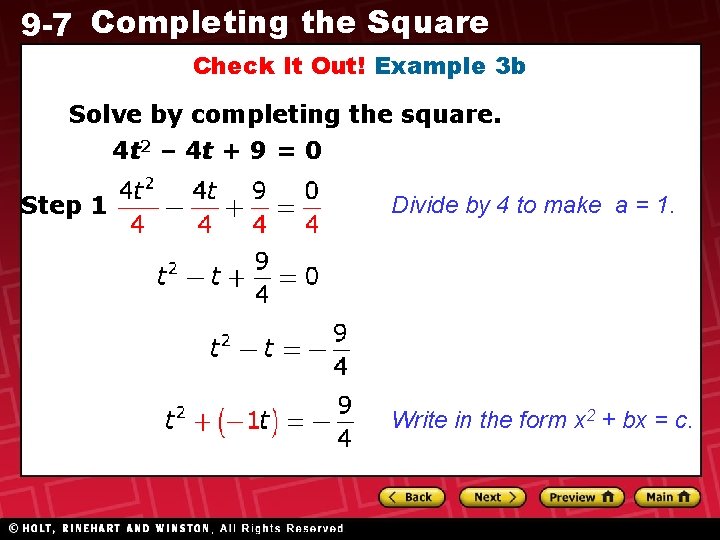
9 -7 Completing the Square Check It Out! Example 3 b Solve by completing the square. 4 t 2 – 4 t + 9 = 0 Step 1 Divide by 4 to make a = 1. Write in the form x 2 + bx = c.

9 -7 Completing the Square Check It Out! Example 3 b Continued Solve by completing the square. 4 t 2 – 4 t + 9 = 0 Step 2 . Step 3 Complete the square. Step 4 Factor and simplify. There is no real number whose square is negative, so there are no real solutions.

9 -7 Completing the Square Additional Example 4: Problem-Solving Application A rectangular room has an area of 195 square feet. Its width is 2 feet shorter than its length. Find the dimensions of the room. Round to the nearest hundredth of a foot, if necessary. 1 Understand the Problem The answer will be the length and width of the room. List the important information: • The room area is 195 square feet. • The width is 2 feet less than the length.

9 -7 Completing the Square Additional Example 4 Continued 2 Make a Plan Set the formula for the area of a rectangle equal to 195, the area of the room. Solve the equation.

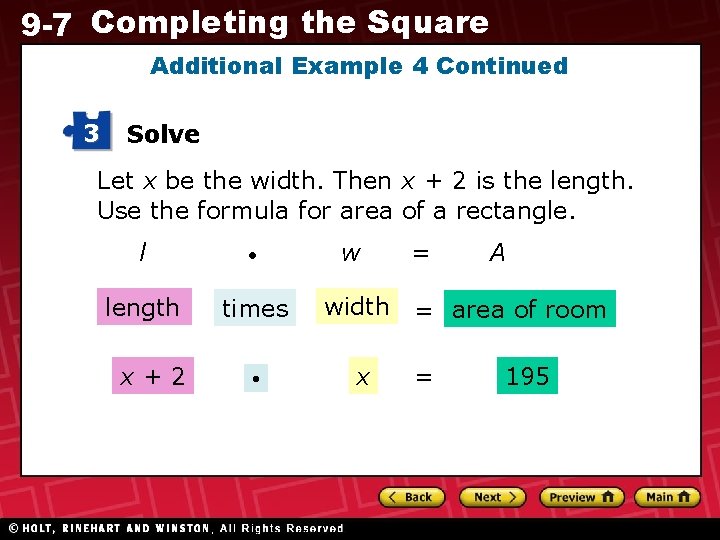
9 -7 Completing the Square Additional Example 4 Continued 3 Solve Let x be the width. Then x + 2 is the length. Use the formula for area of a rectangle. l • length times x+2 • w width x = A = area of room = 195

9 -7 Completing the Square Additional Example 4 Continued Step 1 x 2 + 2 x = 195 Step 2 Simplify. . Step 3 x 2 + 2 x + 1 = 195 + 1 Complete the square by adding 1 to both sides. Step 4 (x + 1)2 = 196 Factor the perfect-square trinomial. Step 5 x + 1 = ± 14 Take the square root of both sides.

9 -7 Completing the Square Additional Example 4 Continued Step 6 x + 1 = 14 or x + 1 = – 14 Write and solve two equations. x = 13 or x = – 15 Negative numbers are not reasonable for length, so x = 13 is the only solution that makes sense. The width is 13 feet, and the length is 13 + 2, or 15 feet. 4 Look Back The length of the room is 2 feet greater than the width. Also 13(15) = 195.

9 -7 Completing the Square Check It Out! Example 4 A rectangular room has an area of 400 ft 2. The length is 8 ft longer than the width. Find the dimensions of the room. Round to the nearest tenth of a foot. 1 Understand the Problem The answer will be the length and width of the room. List the important information: • The room area is 400 square feet. • The length is 8 feet more than the width.

9 -7 Completing the Square Check It Out! Example 4 Continued 2 Make a Plan Set the formula for the area of a rectangle equal to 400, the area of the room. Solve the equation.

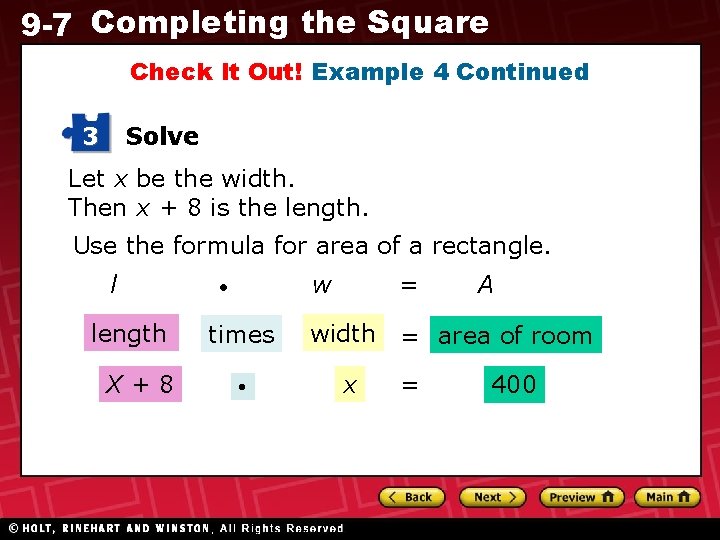
9 -7 Completing the Square Check It Out! Example 4 Continued 3 Solve Let x be the width. Then x + 8 is the length. Use the formula for area of a rectangle. l length X+8 • times • w = width = area of room x = A 400

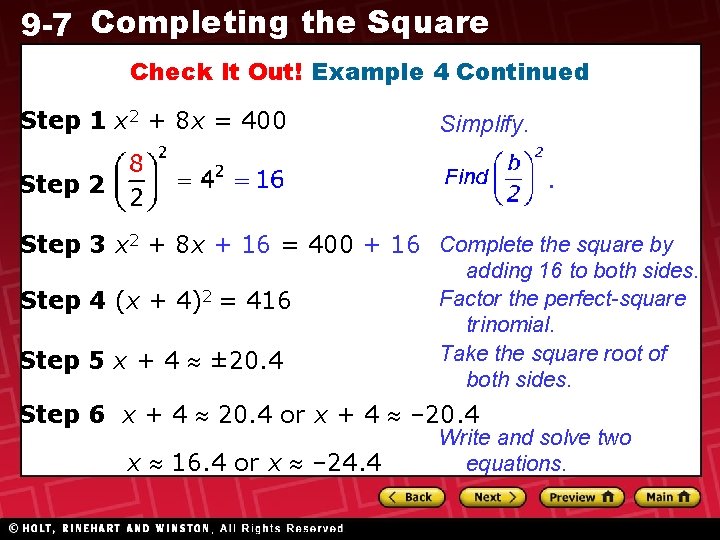
9 -7 Completing the Square Check It Out! Example 4 Continued Step 1 x 2 + 8 x = 400 Simplify. . Step 2 Step 3 x 2 + 8 x + 16 = 400 + 16 Complete the square by Step 4 (x + 4)2 = 416 Step 5 x + 4 ± 20. 4 adding 16 to both sides. Factor the perfect-square trinomial. Take the square root of both sides. Step 6 x + 4 20. 4 or x + 4 – 20. 4 x 16. 4 or x – 24. 4 Write and solve two equations.

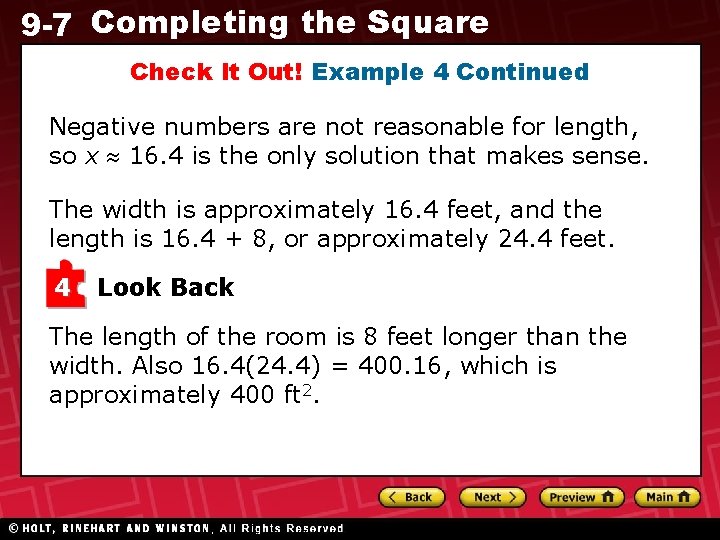
9 -7 Completing the Square Check It Out! Example 4 Continued Negative numbers are not reasonable for length, so x 16. 4 is the only solution that makes sense. The width is approximately 16. 4 feet, and the length is 16. 4 + 8, or approximately 24. 4 feet. 4 Look Back The length of the room is 8 feet longer than the width. Also 16. 4(24. 4) = 400. 16, which is approximately 400 ft 2.

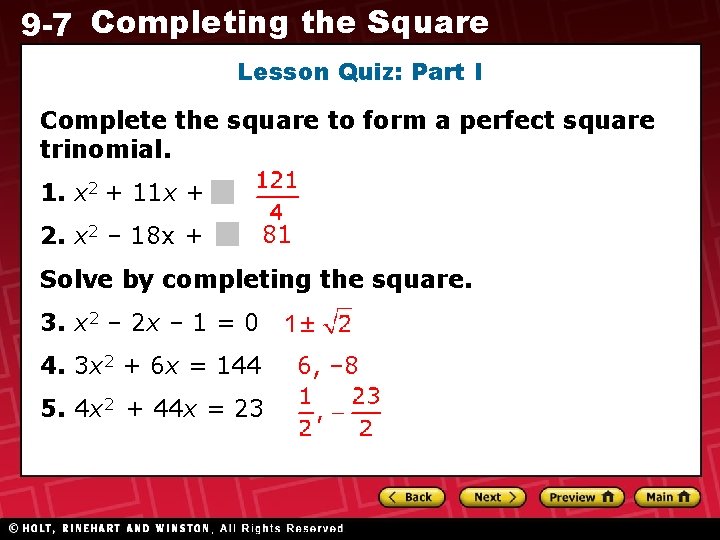
9 -7 Completing the Square Lesson Quiz: Part I Complete the square to form a perfect square trinomial. 1. x 2 + 11 x + 2. x 2 – 18 x + 81 Solve by completing the square. 3. x 2 – 2 x – 1 = 0 4. 3 x 2 + 6 x = 144 5. 4 x 2 + 44 x = 23 6, – 8

9 -7 Completing the Square Lesson Quiz: Part II 6. Dymond is painting a rectangular banner for a football game. She has enough paint to cover 120 ft 2. She wants the length of the banner to be 7 ft longer than the width. What dimensions should Dymond use for the banner? 8 feet by 15 feet
 Completing the square tutorial
Completing the square tutorial Completing the square (continued) quiz
Completing the square (continued) quiz Factoring non perfect square trinomials
Factoring non perfect square trinomials How to complete the square
How to complete the square Perfect square trinomials examples
Perfect square trinomials examples Completing the square
Completing the square 9-7 solving quadratic equations by using square roots
9-7 solving quadratic equations by using square roots How to complete the square
How to complete the square 4-6 completing the square
4-6 completing the square Completing the square (continued)
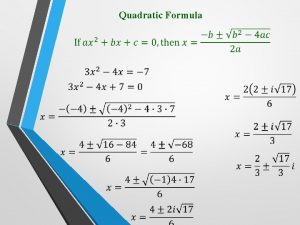
Completing the square (continued) Quadratic formula completing the square
Quadratic formula completing the square Completion of the square
Completion of the square Completing the square steps
Completing the square steps 9-8 completing the square
9-8 completing the square Dr frost maths
Dr frost maths Completing the quare
Completing the quare 9-5 completing the square
9-5 completing the square Lesson 12: completing the square
Lesson 12: completing the square Completing the square mathswatch
Completing the square mathswatch Complete the square to write 16t2 - 96t
Complete the square to write 16t2 - 96t Nat 5 parabolas
Nat 5 parabolas 2-4 completing the square
2-4 completing the square 9-5 solving quadratic equations by completing the square
9-5 solving quadratic equations by completing the square Quadratic formula examples
Quadratic formula examples Completing square program
Completing square program Completing the square
Completing the square Lesson 8-8 practice b completing the square answers
Lesson 8-8 practice b completing the square answers Completing the square
Completing the square Factorising quadratics dr frost
Factorising quadratics dr frost Completing the square formula
Completing the square formula Simplify calculator
Simplify calculator Completing the square
Completing the square When to use complete the square
When to use complete the square Completing the square examples
Completing the square examples 5-4 completing the square
5-4 completing the square How to skim effectively
How to skim effectively The selection preview
The selection preview What is a preview statement
What is a preview statement Summary thesis statement
Summary thesis statement Disadvantages of realistic job preview
Disadvantages of realistic job preview Tams dallas
Tams dallas Perintah print preview kita jalankan dari menu
Perintah print preview kita jalankan dari menu Test prep preview
Test prep preview Nnn image preview
Nnn image preview 1984 book preview
1984 book preview Chapter 21 standardized test practice answers
Chapter 21 standardized test practice answers