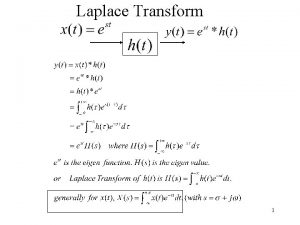Fourier Transform chapter 6 FOURIER TRANSFORM Definition of



















- Slides: 49

Fourier Transform chapter 6

FOURIER TRANSFORM: • Definition of the Fourier transforms • Relationship between Laplace Transforms and Fourier Transforms • Fourier transforms in the limit • Properties of the Fourier Transforms • Circuit applications using Fourier Transforms • Parseval’s theorem • Energy calculation in magnitude spectrum

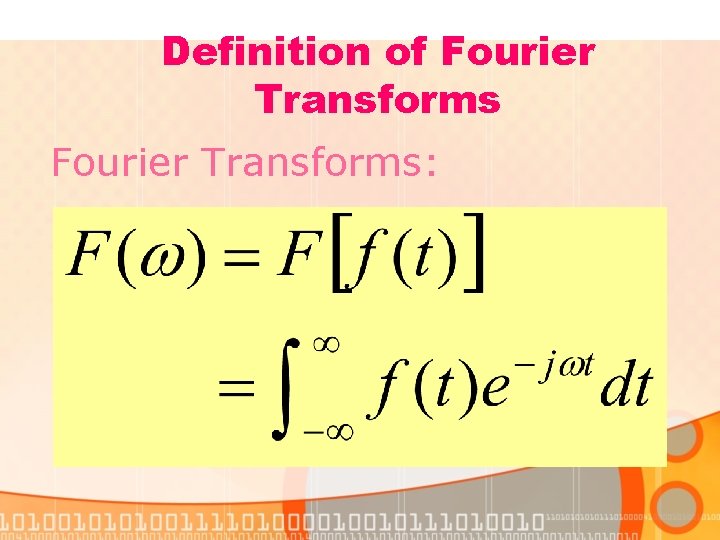
Definition of Fourier Transforms:

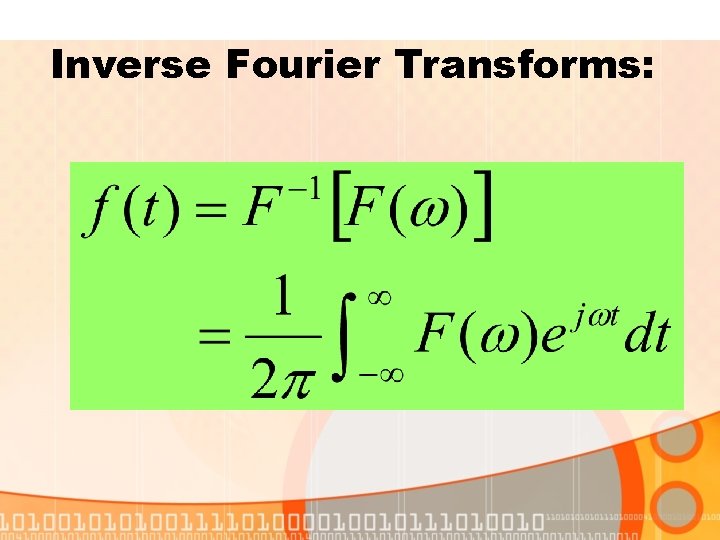
Inverse Fourier Transforms:

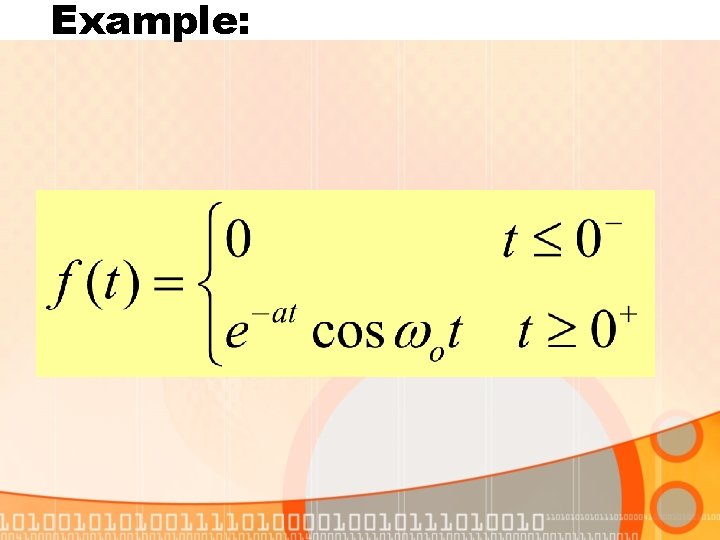
Example 1: Obtain the Fourier Transform for the function below:

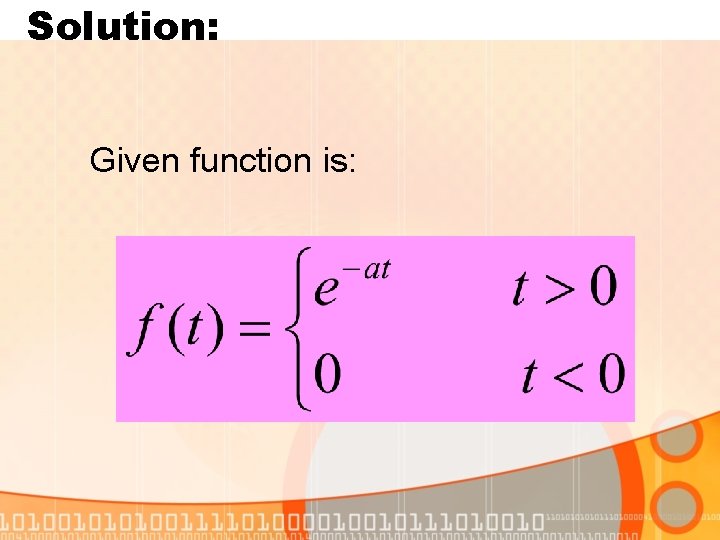
Solution: Given function is:

Fourier Transforms:

FOURIER TRANSFORM: • Definition of the Fourier transforms • Relationship between Laplace Transforms and Fourier Transforms • Fourier transforms in the limit • Properties of the Fourier Transforms • Circuit applications using Fourier Transforms • Parseval’s theorem • Energy calculation in magnitude spectrum

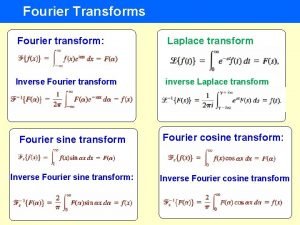
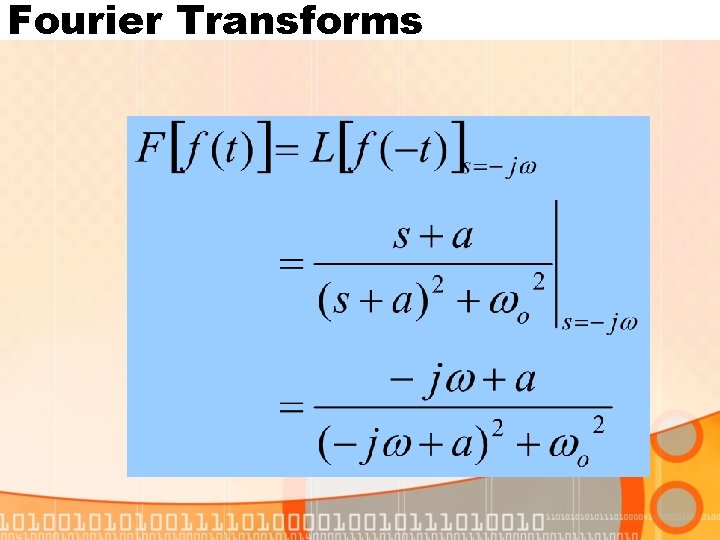
Relationship between Fourier Transforms and Laplace Transforms There are 3 rules apply to the use of Laplace transforms to find Fourier Transforms of such functions.

Rule 1: If f(t)=0 for t<=0 • Replace s=jω

Example:

Replace s=jω

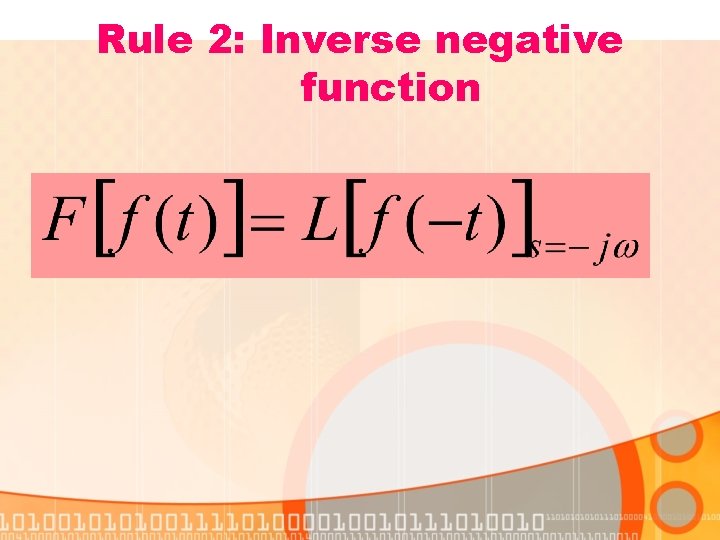
Rule 2: Inverse negative function

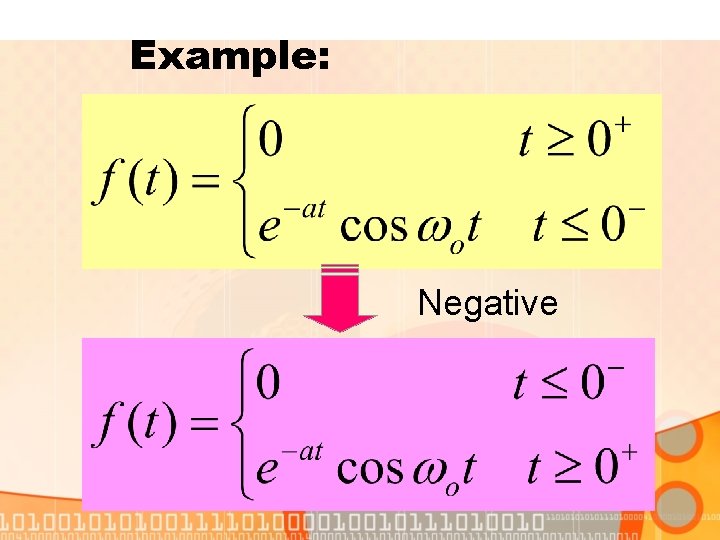
Example: Negative

Fourier Transforms

Rule 3: Add the positive and negative function

Thus,

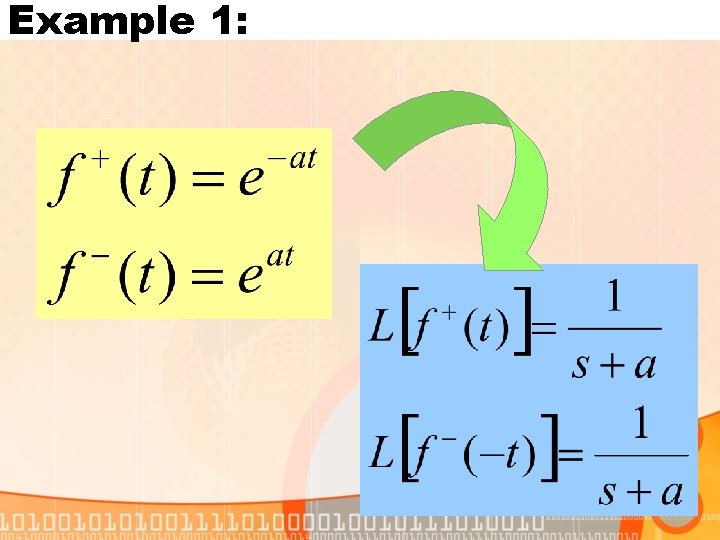
Example 1:

Fourier transforms:

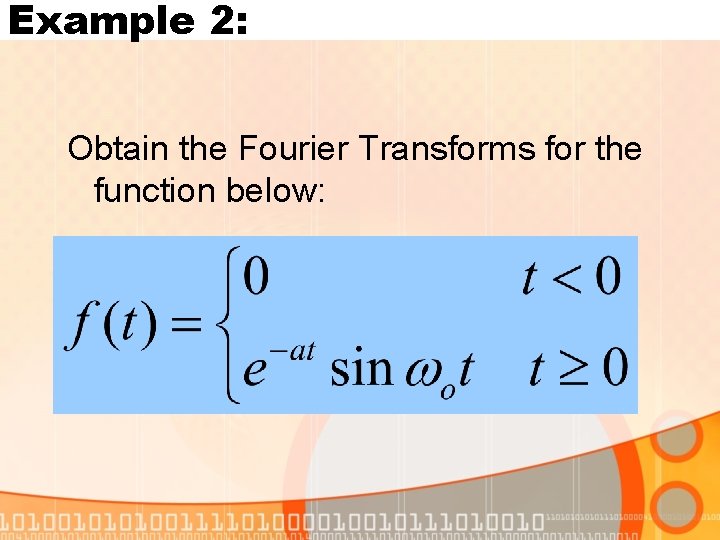
Example 2: Obtain the Fourier Transforms for the function below:

Solution:

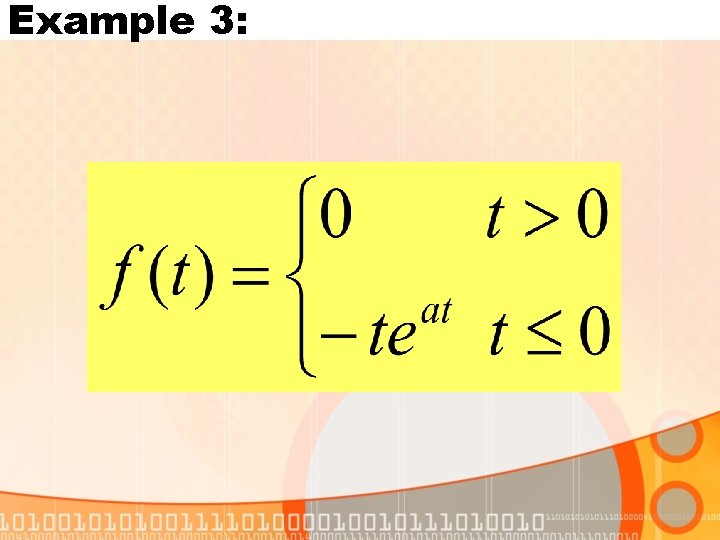
Example 3:

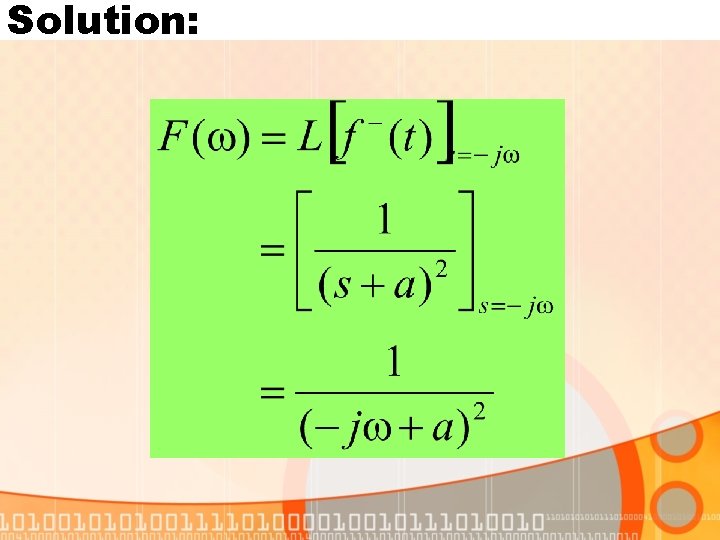
Solution:

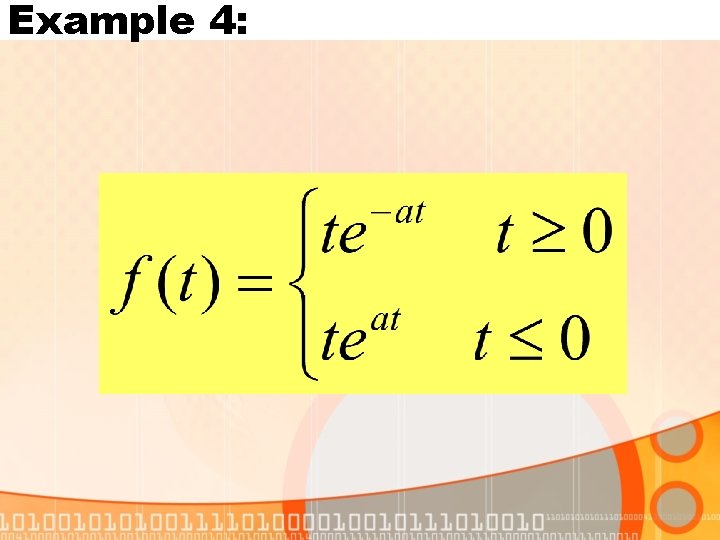
Example 4:

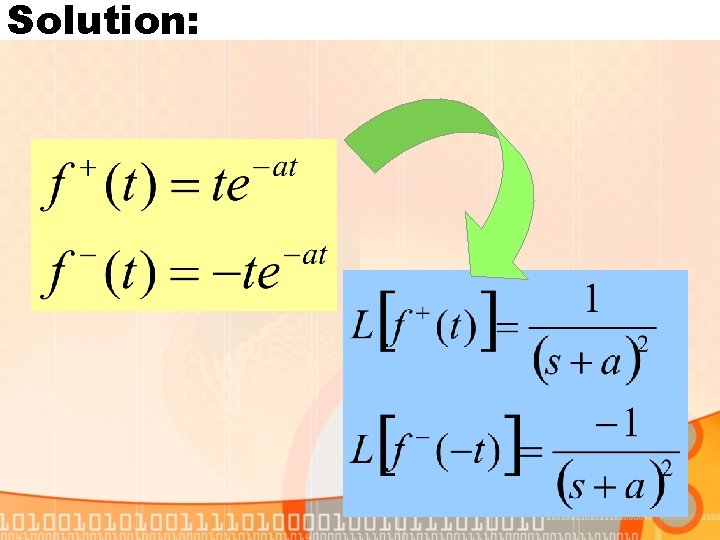
Solution:


FOURIER TRANSFORM: • Definition of the Fourier transforms • Relationship between Laplace Transforms and Fourier Transforms • Fourier transforms in the limit • Properties of the Fourier Transforms • Circuit applications using Fourier Transforms • Parseval’s theorem • Energy calculation in magnitude spectrum

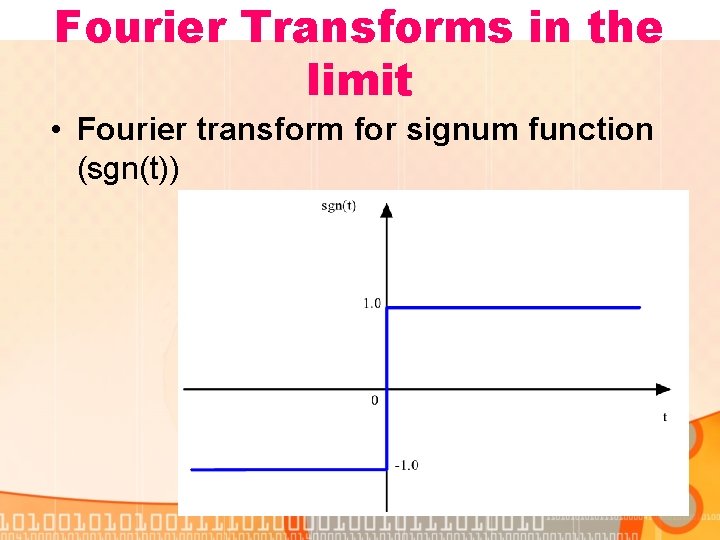
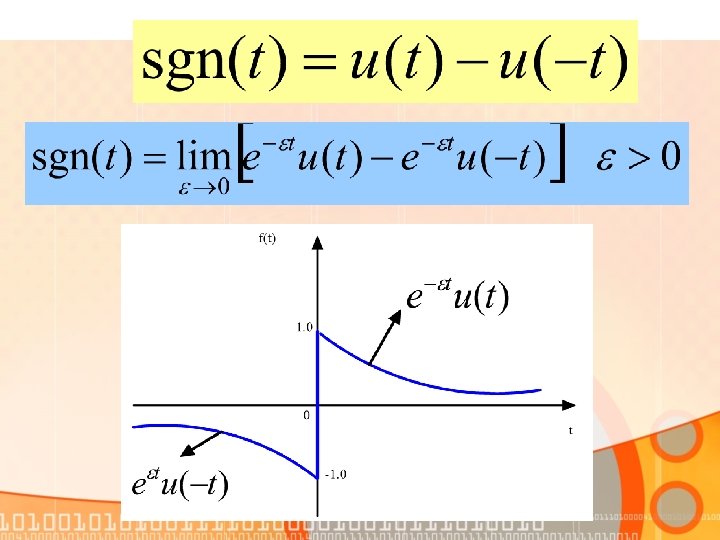
Fourier Transforms in the limit • Fourier transform for signum function (sgn(t))



assume ε→ 0,

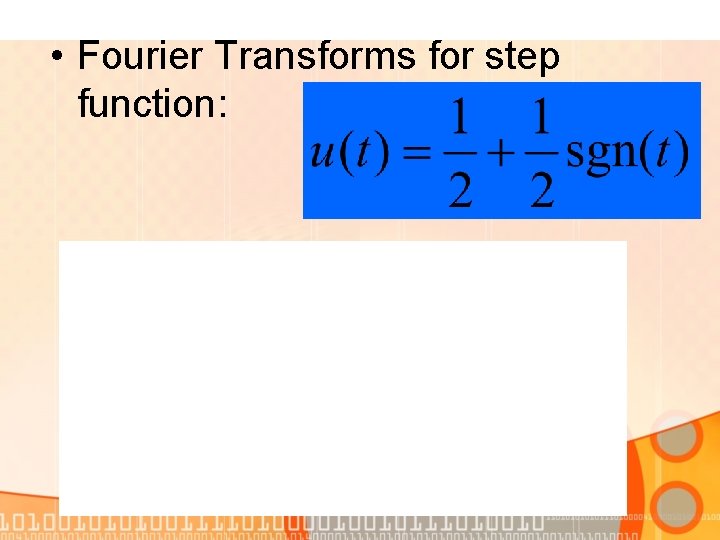
• Fourier Transforms for step function:

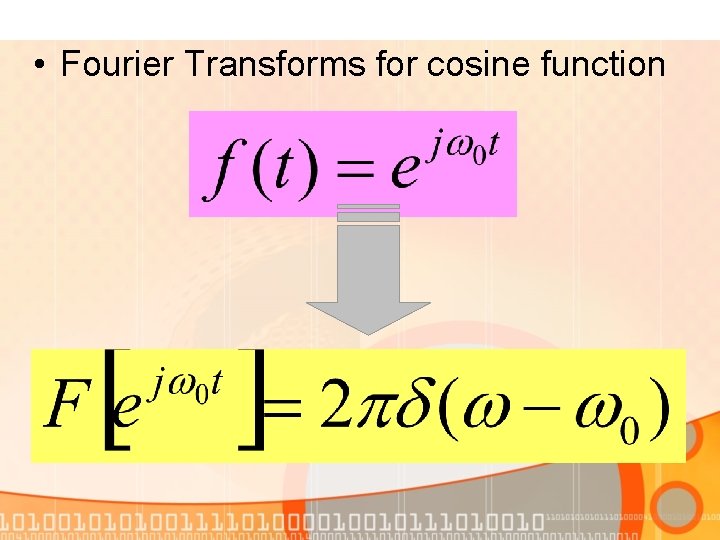
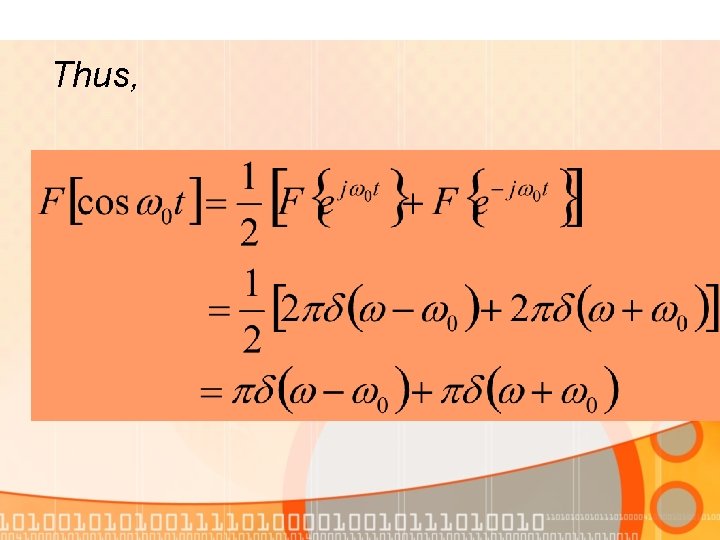
• Fourier Transforms for cosine function


Thus,

FOURIER TRANSFORM: • Definition of the Fourier transforms • Relationship between Laplace Transforms and Fourier Transforms • Fourier transforms in the limit • Properties of the Fourier Transforms • Circuit applications using Fourier Transforms • Parseval’s theorem • Energy calculation in magnitude spectrum

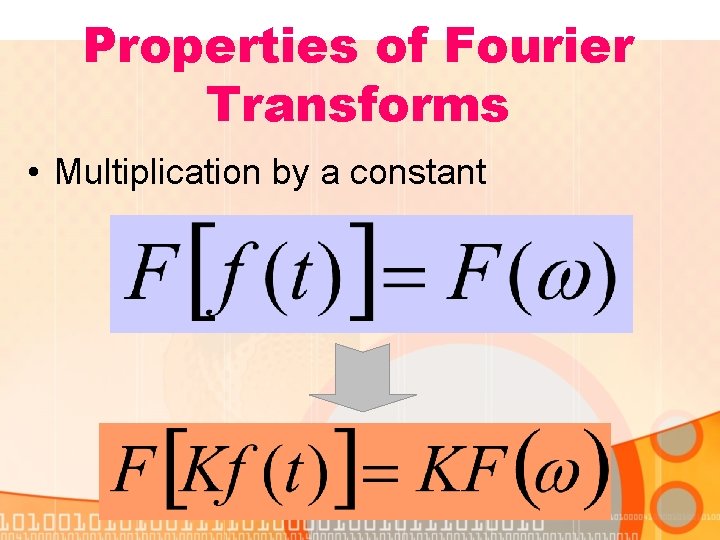
Properties of Fourier Transforms • Multiplication by a constant

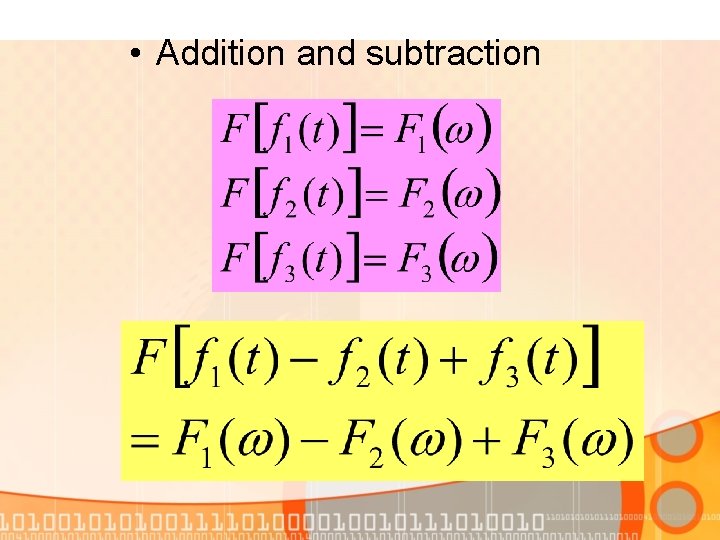
• Addition and subtraction

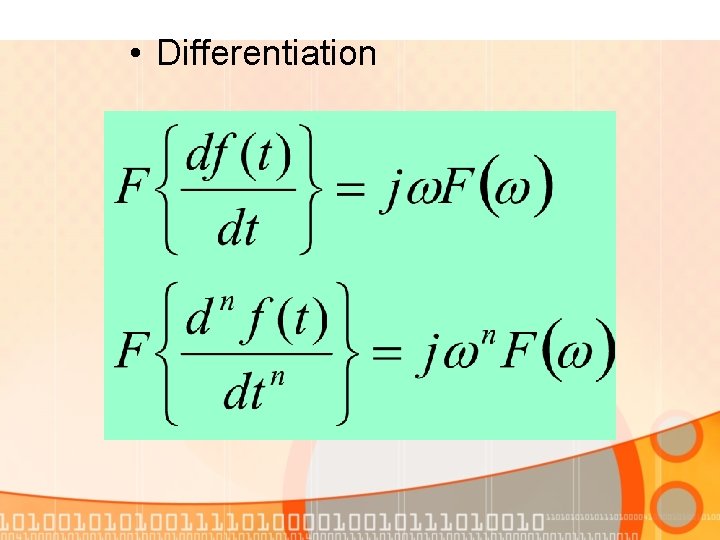
• Differentiation

• Integration

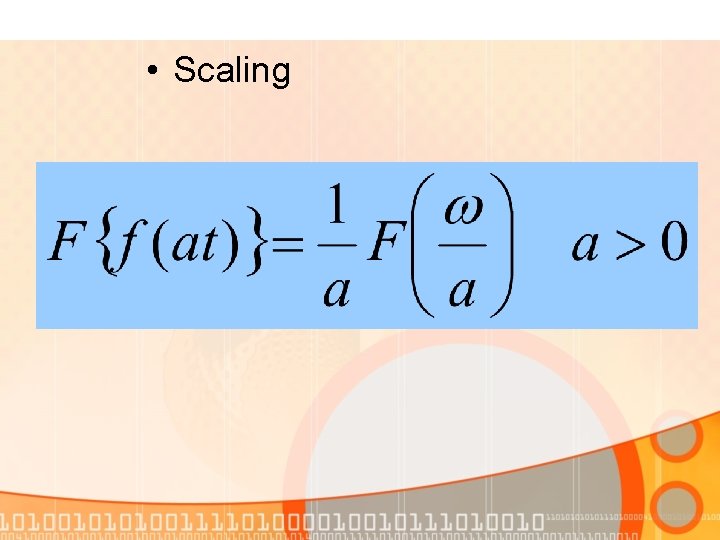
• Scaling

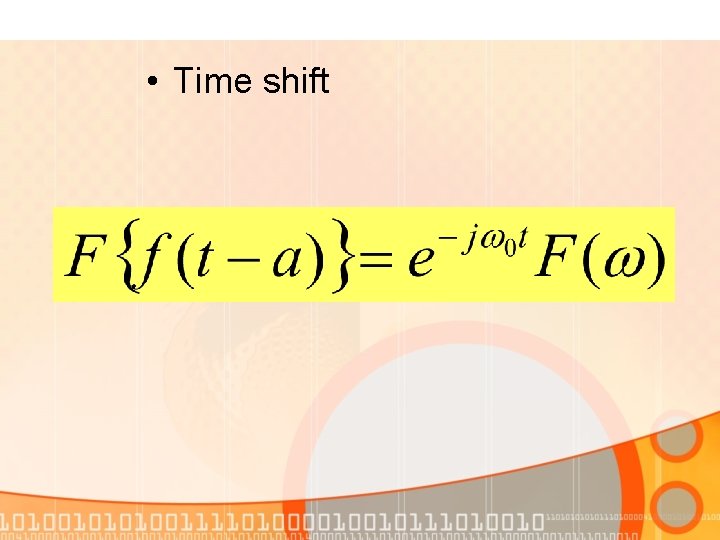
• Time shift

• Frequency shift

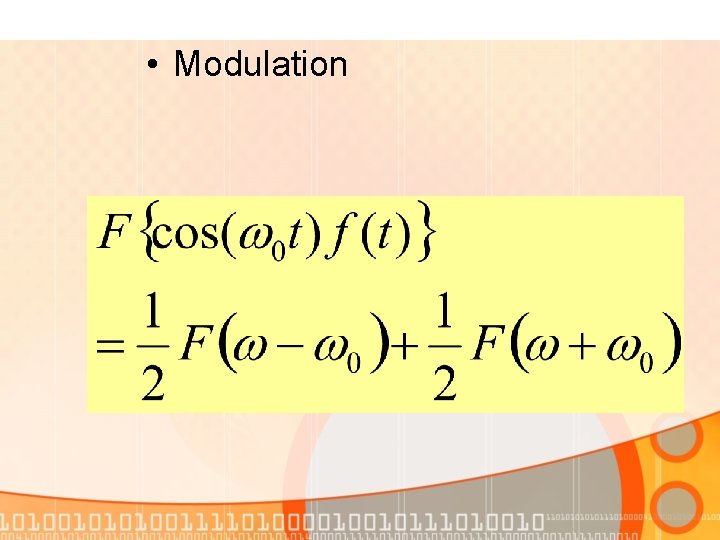
• Modulation

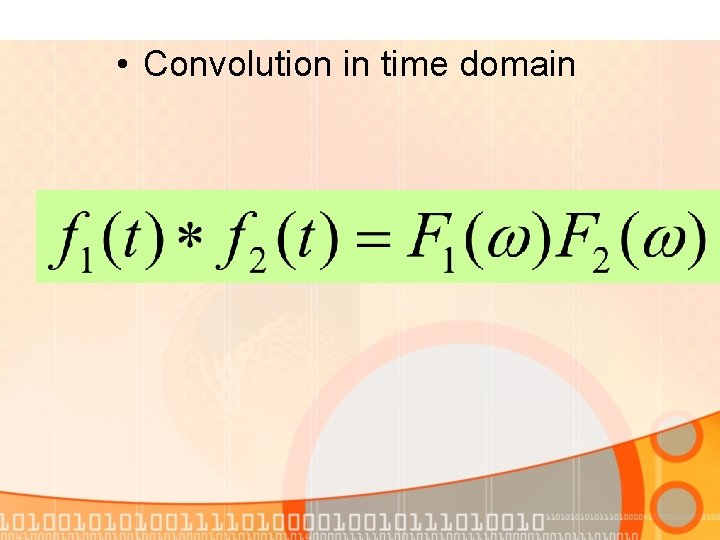
• Convolution in time domain

• Convolution in frequency domain:

Example 1: • Determine the inverse Fourier Transforms for the function below:

Solution: LAPLACE TRANSFORMS

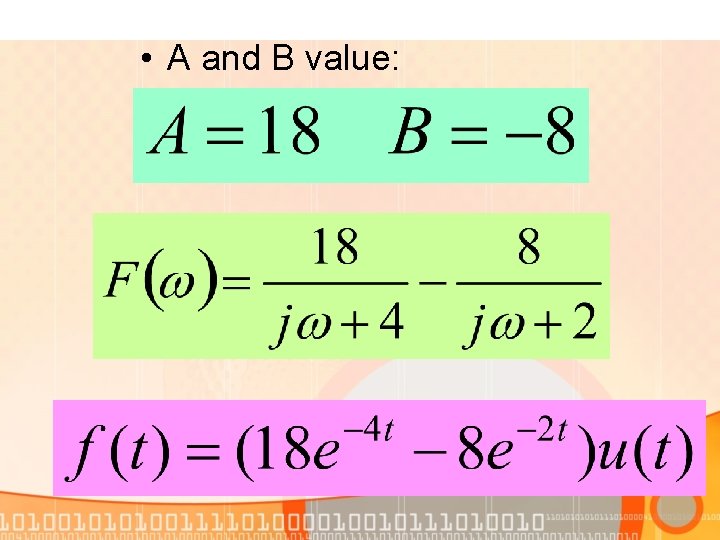
• A and B value:
 Fourier transform is defined for
Fourier transform is defined for Fourier transform definition
Fourier transform definition Transformata laplace calculator
Transformata laplace calculator Sinc to rect
Sinc to rect Inverse fourier transform of dirac delta function
Inverse fourier transform of dirac delta function Ctfs fourier
Ctfs fourier Short time fourier transform
Short time fourier transform Fourier transform table
Fourier transform table Parseval's identity for fourier transform
Parseval's identity for fourier transform Duality of fourier transform
Duality of fourier transform Synthesis equation fourier series
Synthesis equation fourier series Phase meaning
Phase meaning Forward fourier transform
Forward fourier transform Fourier transform of ramp function
Fourier transform of ramp function Fourier transform mri
Fourier transform mri Fourier transform of x
Fourier transform of x Unit step function fourier transform
Unit step function fourier transform Difference of gaussian filter
Difference of gaussian filter Multiplication property of fourier transform
Multiplication property of fourier transform Fourier transform in image processing
Fourier transform in image processing Sin(2pift)
Sin(2pift) The fourier transform and its applications
The fourier transform and its applications Inverse fourier transform formula
Inverse fourier transform formula Fourier transform
Fourier transform Stft
Stft Polar fourier series
Polar fourier series Fourier transform of product of two functions
Fourier transform of product of two functions Discrete fourier transform formula
Discrete fourier transform formula Sinc fourier transform
Sinc fourier transform Fourier series of impulse train
Fourier series of impulse train Discrete fourier transform
Discrete fourier transform Fourier transform of impulse train
Fourier transform of impulse train Fourier transform of circ function
Fourier transform of circ function Duality of fourier transform
Duality of fourier transform Parseval's equation
Parseval's equation Parseval's theorem
Parseval's theorem Fourier transform formula table
Fourier transform formula table Fourier integral representation
Fourier integral representation Duality of fourier transform
Duality of fourier transform Site:slidetodoc.com
Site:slidetodoc.com Windowed fourier transform
Windowed fourier transform Fourier transform
Fourier transform Integral of unit step function
Integral of unit step function Relation between laplace and fourier transform
Relation between laplace and fourier transform R fft
R fft Fourier transform solver
Fourier transform solver Fourier series of periodic function
Fourier series of periodic function Dft vs fft
Dft vs fft Dft
Dft Fftshift2
Fftshift2