AUGUST 2 MATH 104 Calculus I Review of









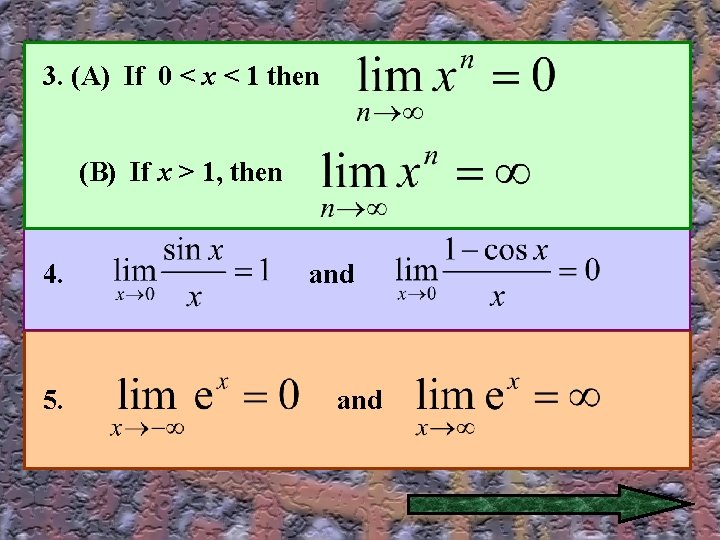

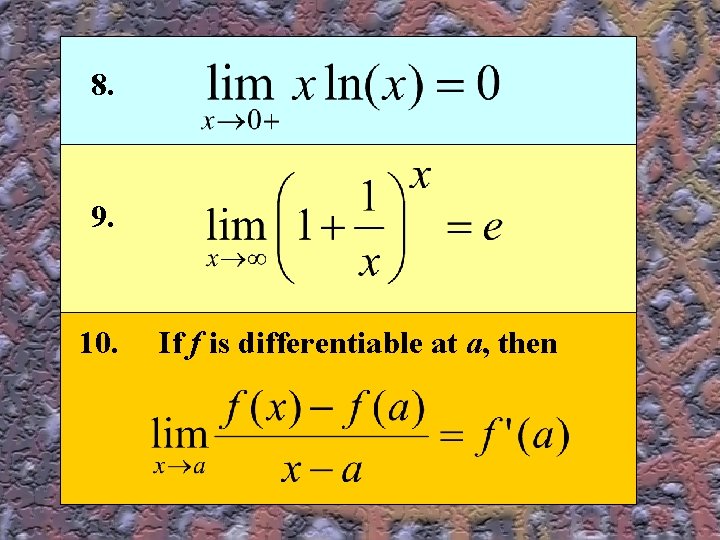
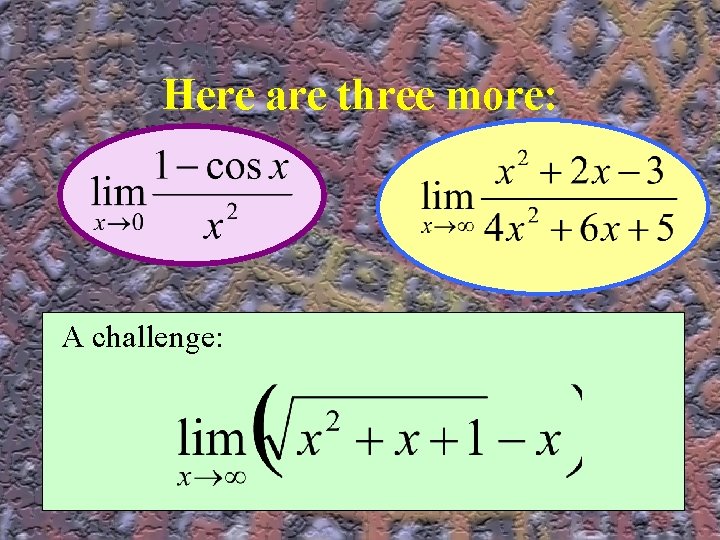
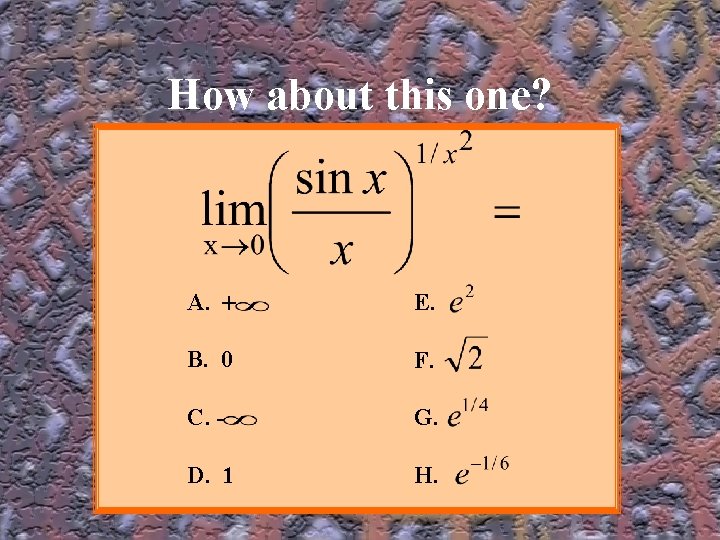
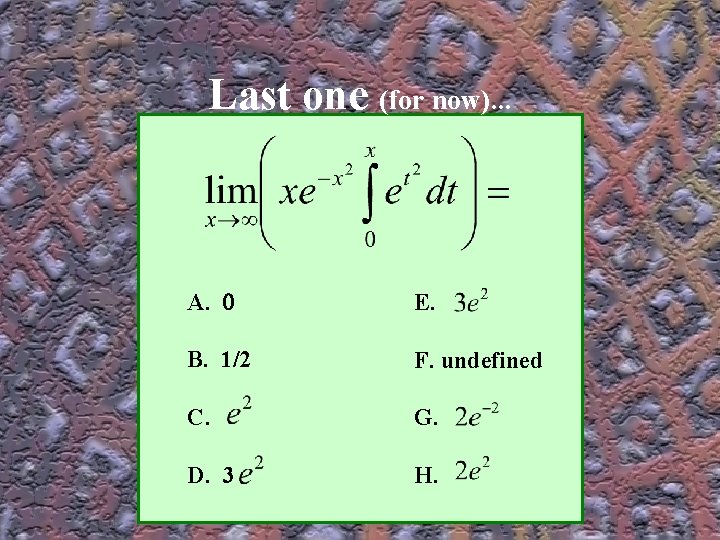

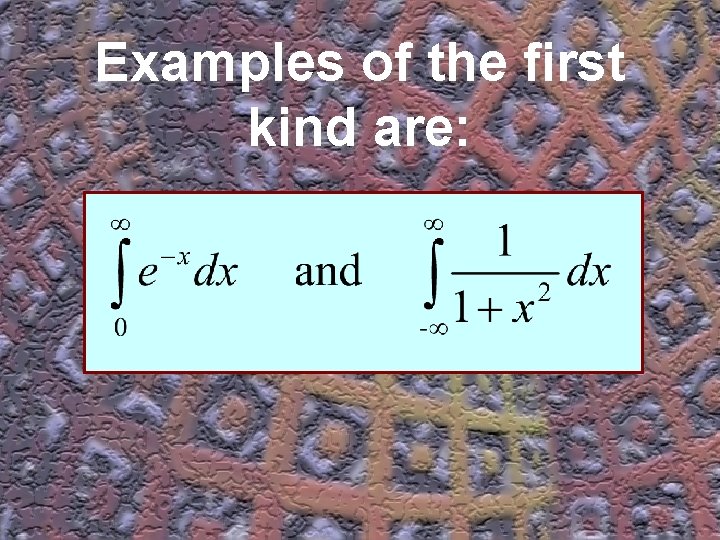
















- Slides: 50

AUGUST 2

MATH 104 Calculus I Review of previous material…. …methods and applications of integration, differential equations ………. .

Geometry of Differential Equations A differential equation of the form gives geometric information about the graph of y(x). It tells us: If the graph of y(x) goes through the point (x, y), then the slope of the graph at that point is equal to f(x, y).

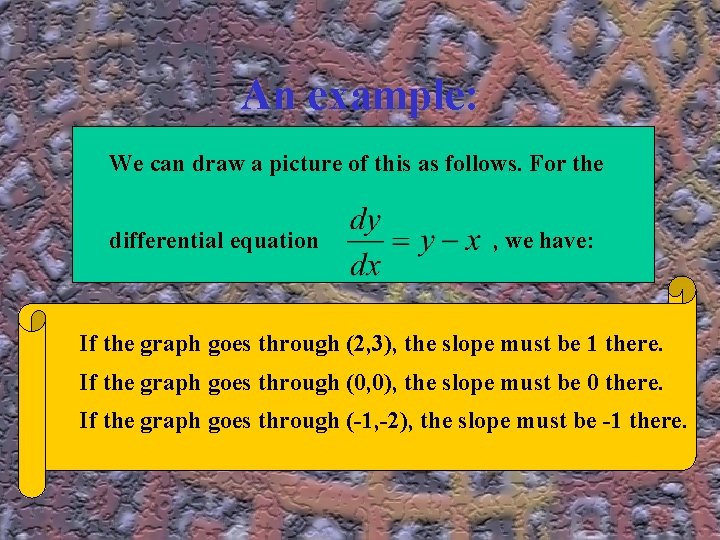
An example: We can draw a picture of this as follows. For the differential equation , we have: If the graph goes through (2, 3), the slope must be 1 there. If the graph goes through (0, 0), the slope must be 0 there. If the graph goes through (-1, -2), the slope must be -1 there.

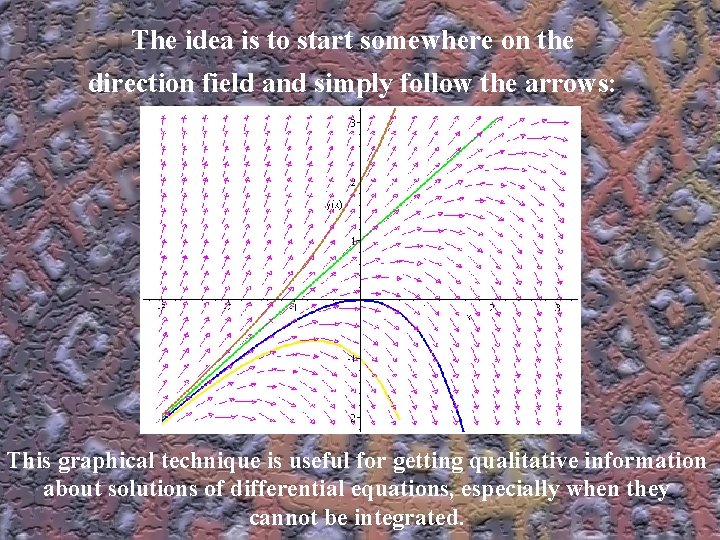
Put it on a graph. . . The slope of the arrow at any point is equal to y - x at that point. This kind of picture is called a "direction field" for the differential equation dy/dx = y - x. We can use this to solve the differential equation geometrically and recover the graph of the function.

The idea is to start somewhere on the direction field and simply follow the arrows: The idea is to start This graphical technique is useful for getting qualitative information about solutions of differential equations, especially when they cannot be integrated.

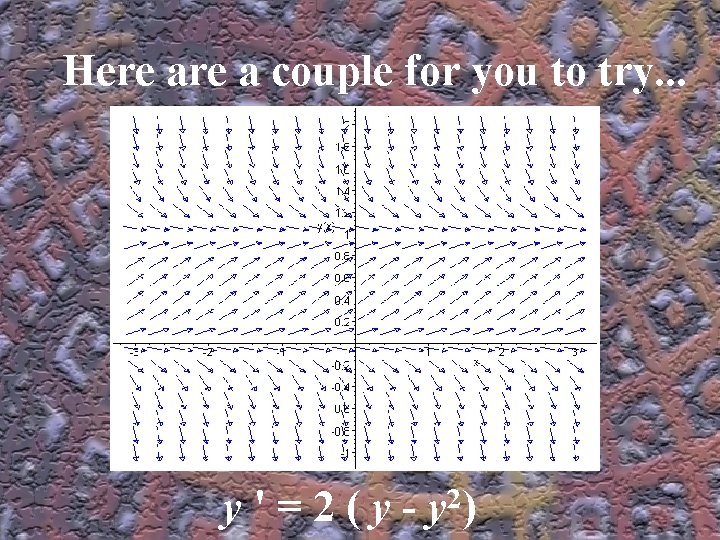
Here a couple for you to try. . . y'=2(y- 2 y)

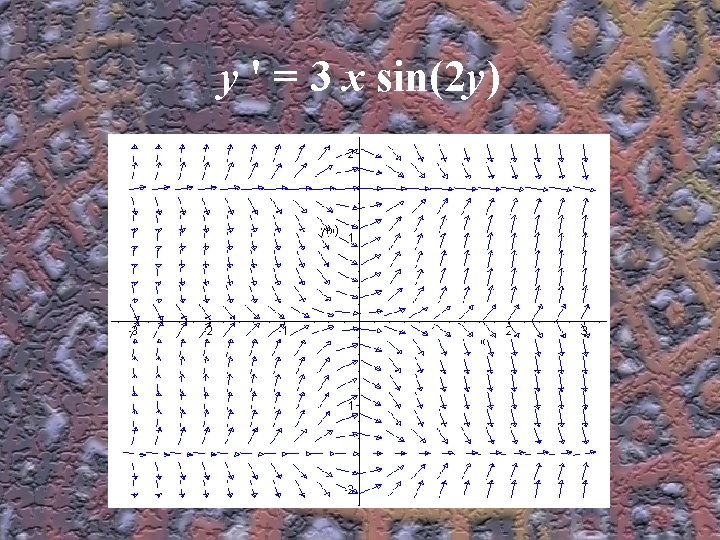
y ' = 3 x sin(2 y sin(2 )

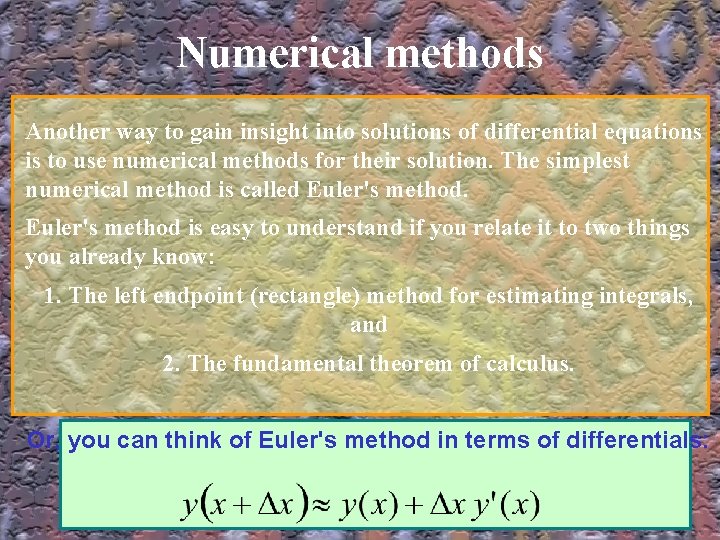
Numerical methods Another way to gain insight into solutions of differential equations is to use numerical methods for their solution. The simplest numerical method is called Euler's method is easy to understand if you relate it to two things you already know: 1. The left endpoint (rectangle) method for estimating integrals, and 2. The fundamental theorem of calculus. Or, you can think of Euler's method in terms of differentials:

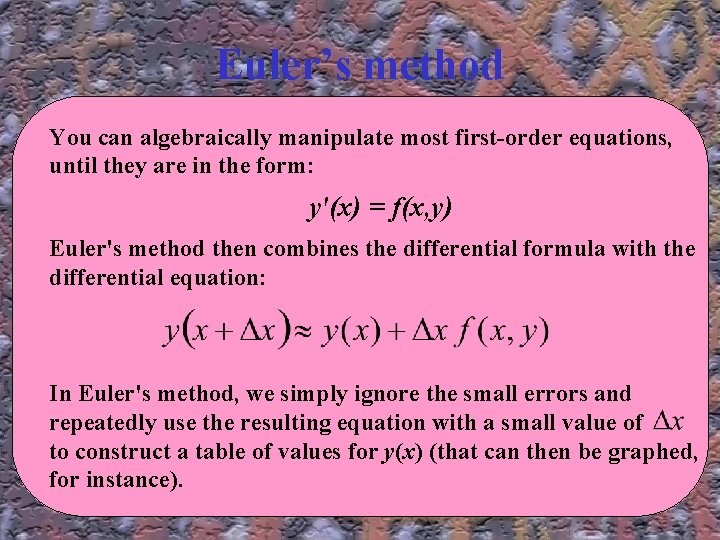
Euler’s method You can algebraically manipulate most first-order equations, until they are in the form: y'(x) = f(x, y) Euler's method then combines the differential formula with the differential equation: In Euler's method, we simply ignore the small errors and repeatedly use the resulting equation with a small value of to construct a table of values for y(x) (that can then be graphed, for instance).

An example. . . y ' = y - x, y(0) = 2 (this is the example we graphed before). We'll use Dx = 0. 1 The choice of Dx is usually dictated by the problem or the situation. The smaller Dx is the more accurate the approximated solution will be, but of course you need to do more work to cover an interval of a given length. For the first step, we can use that x=0 and y=2, therefore y ' = 2. Euler's method then tells us that: y(x + Dx) = y(x) + f(x, y) Dx y(0. 1) = 1 + (2 - 0) 0. 1 = 1. 2

Continue. . . For the second step, we have x = 0. 1, y = 2. 2, therefore y' = 2. 1. Euler's method then gives: y(0. 2) = 2. 2 + 2. 1(0. 1) = 2. 41 We continue in this manner and fill in the following table

Maple. . . Maple tells us that the exact solution of the equation y ' = y - x that has y(0) = 2 is y(x) = x + 1 + e x and so we have y(1) = 4. 71828. So the Euler method result is pretty close (within 10%). We could do better by decreasing Dx, but of course then we'd need more steps to reach x = 1. You'll get to try a couple of these on this week's homework.

L’Hospital Another useful technique for computing limits is L'Hospital's rule: Basic version: If , then provided the latter exists. This also applies if

You can use “Basic L’Hospital” for #4, 6, 8 and 10 of the top ten limit list. But for limits like #9, you need. . . Fancy L’Hospital

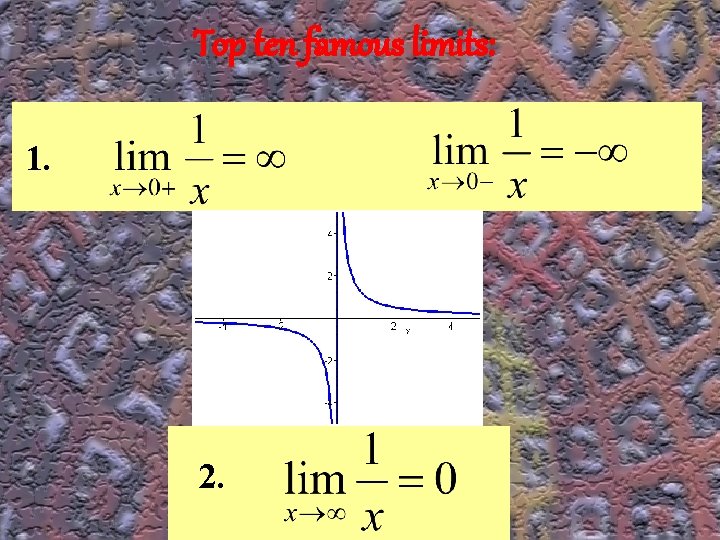
Top ten famous limits: 1. 2.
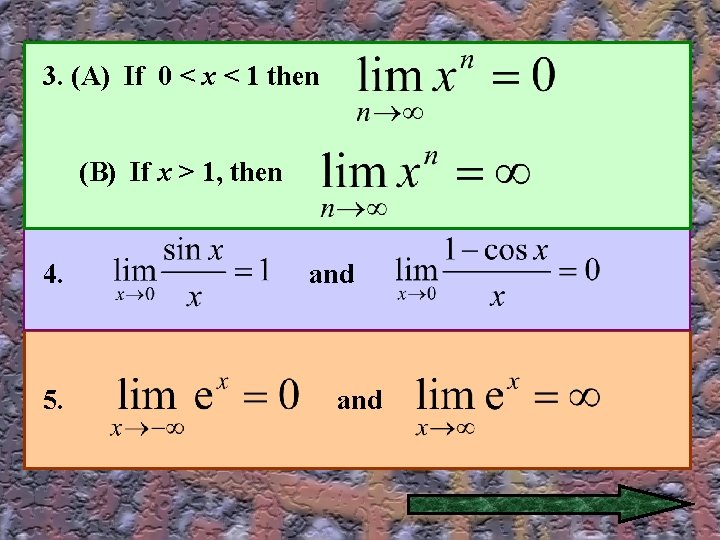
3. (A) If 0 < x < 1 then (B) If x > 1, then 4. 5. and

6. For any value of n, and for any positive value of n, 6 -10 7. does not exist!
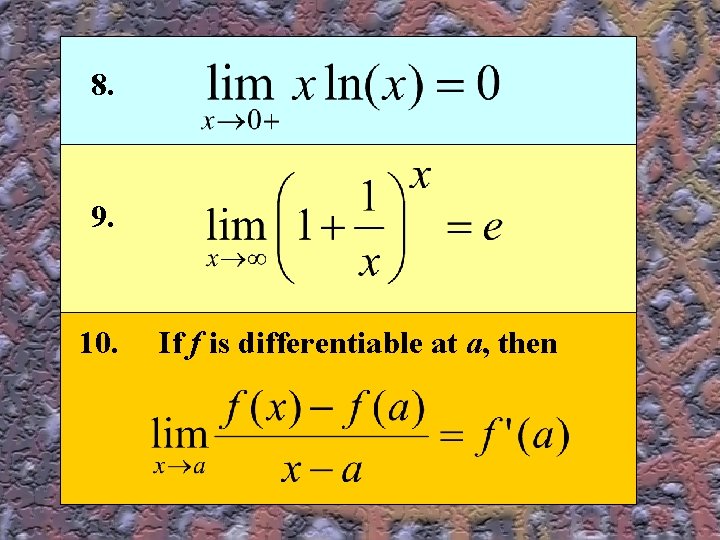
8. 9. 10. If f is differentiable at a, then
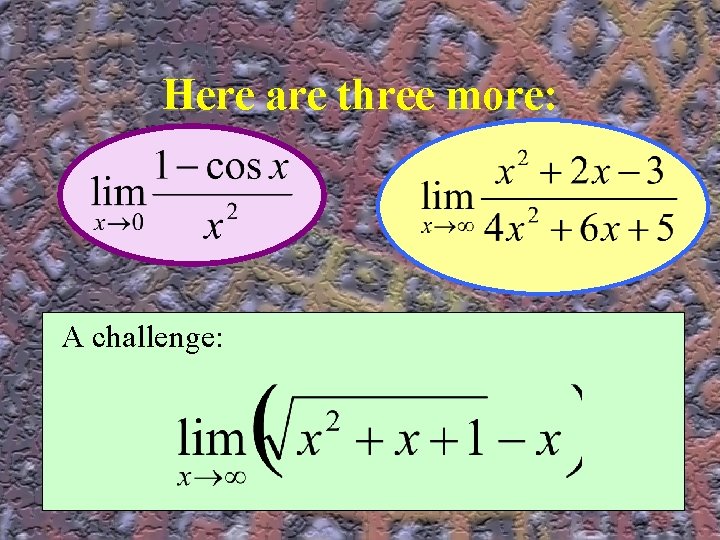
Here are three more: A challenge:
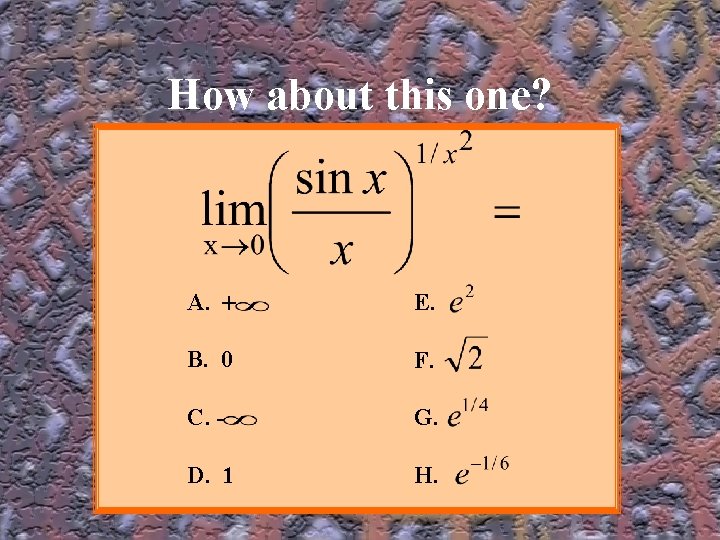
How about this one? A. + E. B. 0 F. C. - G. D. 1 H.
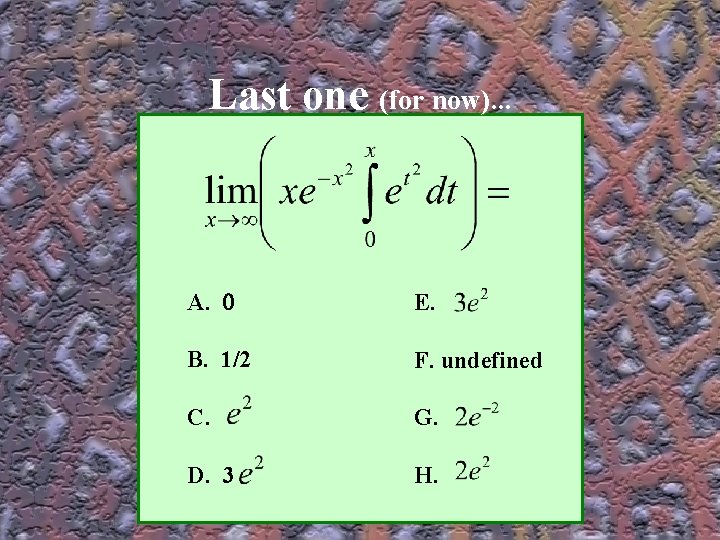
Last one (for now). . . A. 0 E. B. 1/2 F. undefined C. G. D. 3 H.

Improper integrals These are a special kind of limit. An improper integral is one where either the interval of integration is infinite, or else it includes a singularity of the function being integrated.
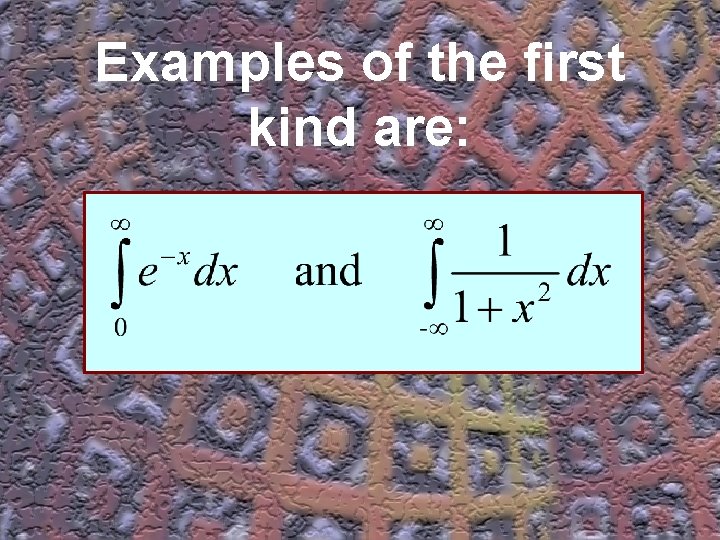
Examples of the first kind are:

Examples of the second kind: The second of these is subtle because the singularity of tan x occurs in the interior of the interval of integration rather than at one of the endpoints.

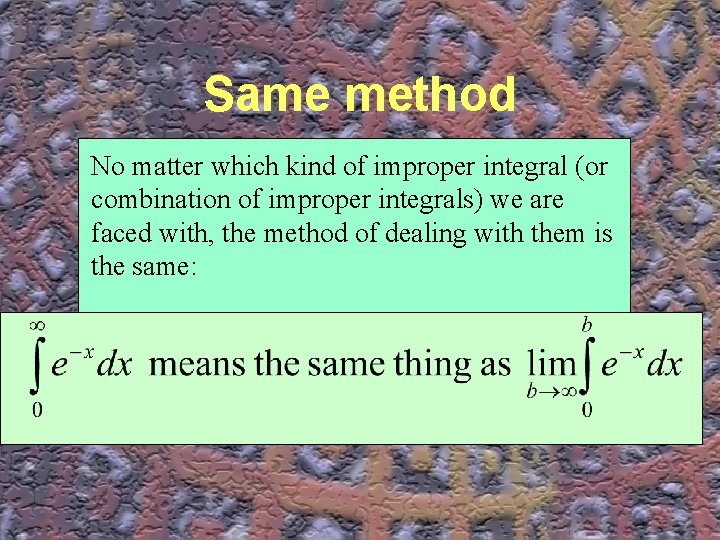
Same method No matter which kind of improper integral (or combination of improper integrals) we are faced with, the method of dealing with them is the same:

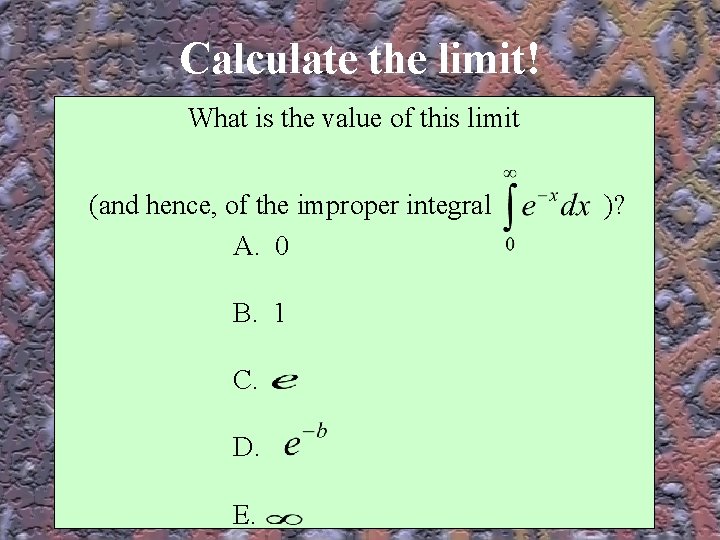
Calculate the limit! What is the value of this limit (and hence, of the improper integral A. 0 B. 1 C. D. E. )?

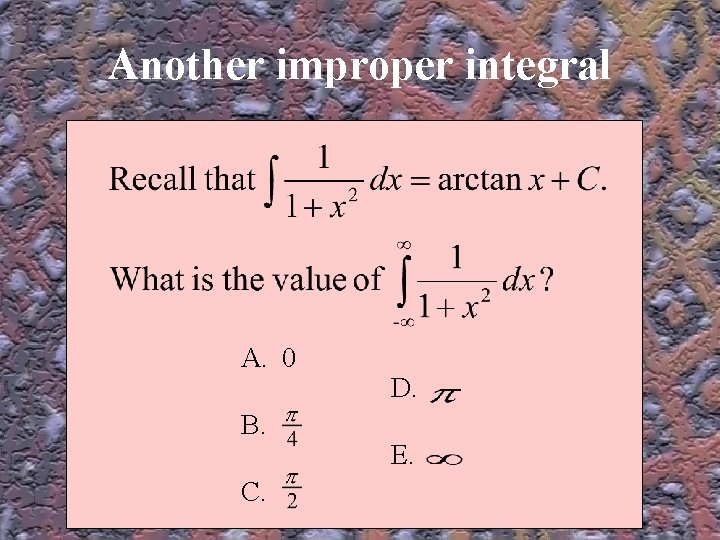
Another improper integral A. 0 B. C. D. E.

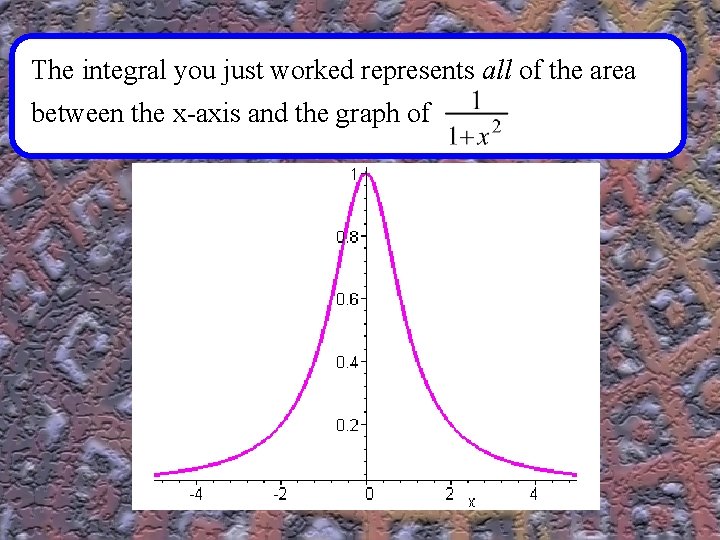
The integral you just worked represents all of the area between the x-axis and the graph of Area between the xaxis and the graph

The other type. . . For improper integrals of the other type, we make the same kind of limit definition:

Another example: What is the value of this limit, in other words, what is A. 0 B. 1 C. 2 D. E.

A divergent improper integral It is possible that the limit used to define the improper integral fails to exist or is infinite. In this case, the improper integral is said to diverge. If the limit does exist, then the improper integral converges. For example: so this improper integral diverges.

One for you: A. Converge B. Diverge

Sometimes it is possible. . . to show that an improper integral converges without actually evaluating it: So the limit of the first integral must be finite as b goes to infinity, because it increases as b does but is bounded above (by 1/3).

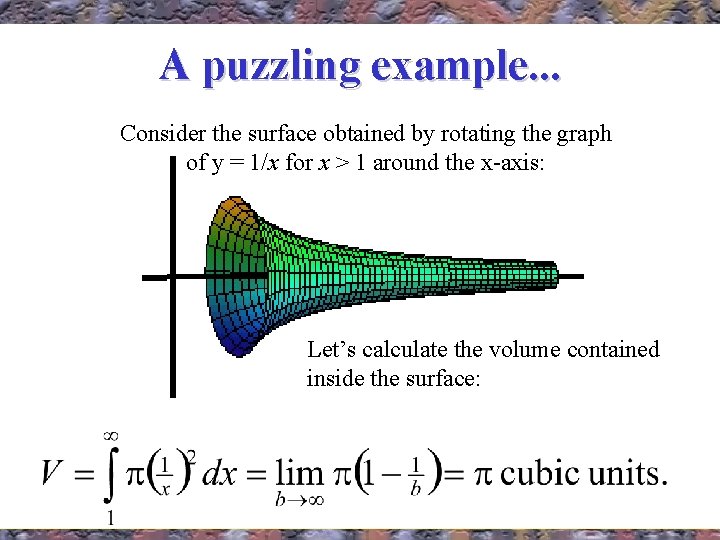
A puzzling example. . . Consider the surface obtained by rotating the graph of y = 1/x for x > 1 around the x-axis: Let’s calculate the volume contained inside the surface:

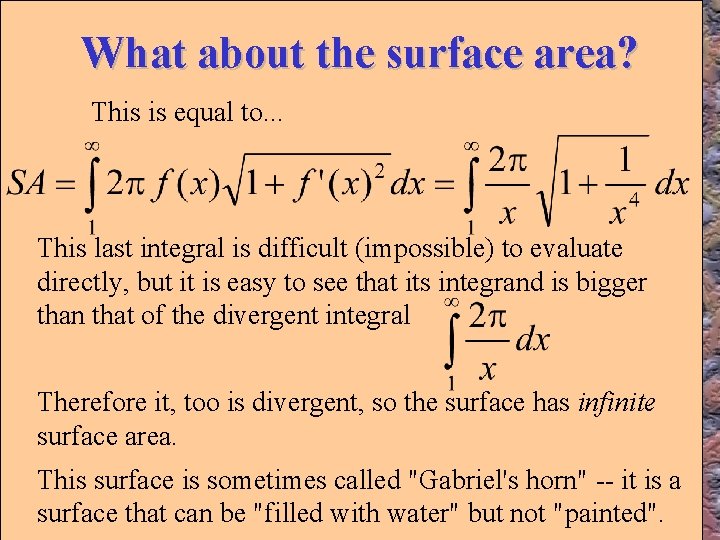
What about the surface area? This is equal to. . . This last integral is difficult (impossible) to evaluate directly, but it is easy to see that its integrand is bigger than that of the divergent integral Therefore it, too is divergent, so the surface has infinite surface area. This surface is sometimes called "Gabriel's horn" -- it is a surface that can be "filled with water" but not "painted".

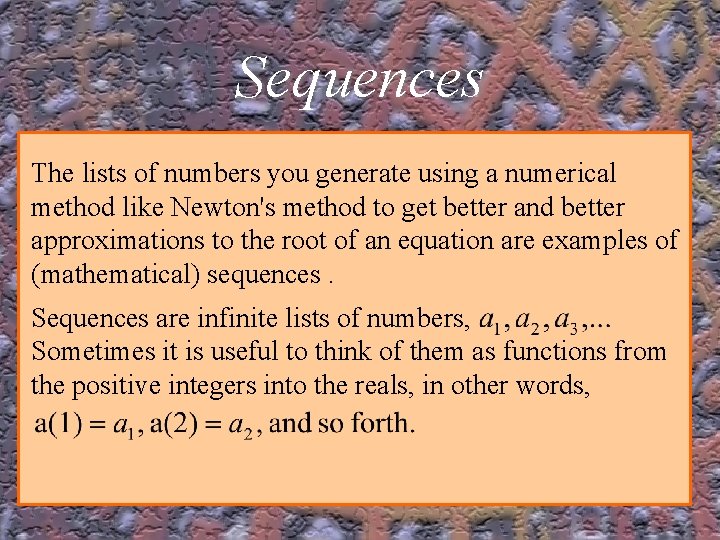
Sequences The lists of numbers you generate using a numerical method like Newton's method to get better and better approximations to the root of an equation are examples of (mathematical) sequences. Sequences are infinite lists of numbers, Sometimes it is useful to think of them as functions from the positive integers into the reals, in other words,

Convergent and Divergent The feeling we have about numerical methods like the bisection method, is that if we kept doing it more and more times, we would get numbers that are closer and closer to the actual root of the equation. In other words where r is the root. Sequences for which exists and is finite are called convergent, other sequences are called divergent

For example. . . The sequence n 1, 1/2, 1/4, 1/8, 1/16, . . , 1/2 , . . . is convergent (and converges to zero, since ), whereas: the sequence 1, 4, 9, 16, . …n 2, . . . is divergent.

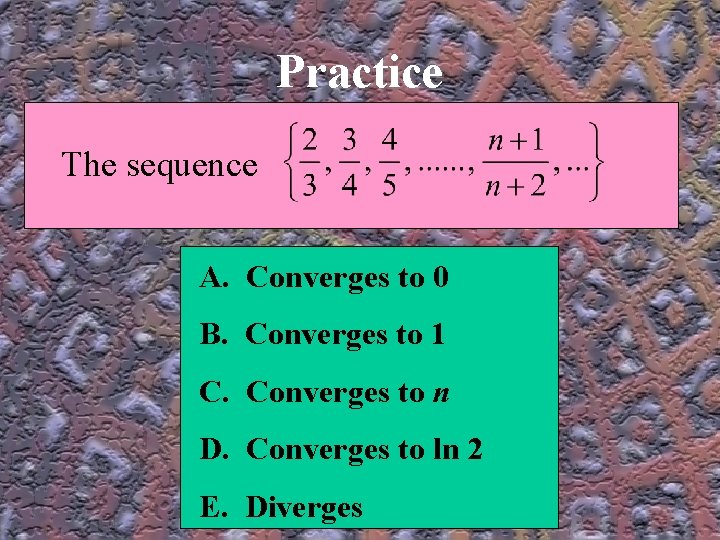
Practice The sequence A. Converges to 0 B. Converges to 1 C. Converges to n D. Converges to ln 2 E. Diverges

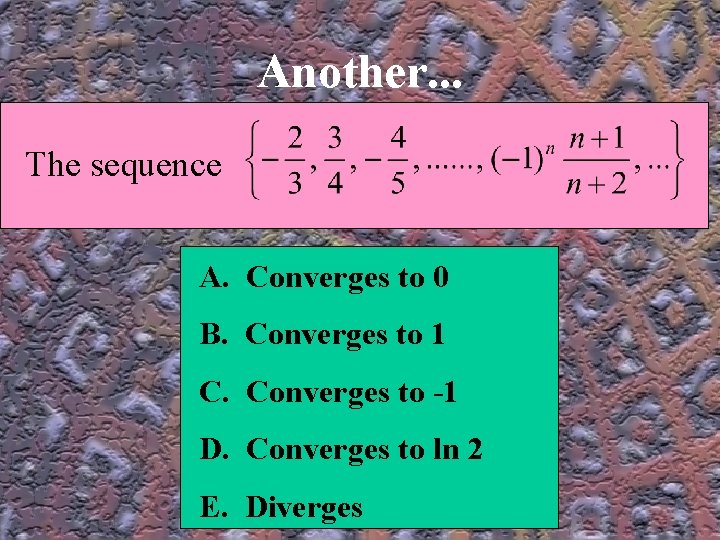
Another. . . The sequence A. Converges to 0 B. Converges to 1 C. Converges to -1 D. Converges to ln 2 E. Diverges

A powerful existence theorem It is sometimes possible to assert that a sequence is convergent even if we can't find the limit right away. We do this by using the least upper bound property of the real numbers: If a sequence has the property that a 1 <a 2<a 3<. . is called a "monotonically increasing" sequence. For such a sequence, either the sequence is bounded (all the terms are less than some fixed number) or else it increases without bound to infinity. The latter case is divergent, and the former must converge to the least upper bound of the set of numbers {a 1, a 2, . . . }. So if we find some upper bound, we are guaranteed convergence, even if we can't find the least upper bound.

Consider the sequence. . . etc. To get each term from the previous one, you add 2 and then take the square root. It is clear that this is a monotonically increasing sequence. It is convergent because all the terms are less than 2. To see this, note that if x>2, then So our terms can't be greater than 2, since adding 2 and taking the square root makes our terms bigger, not smaller. Therefore, the sequence has a limit, by theorem.

QUESTION: What is the limit?

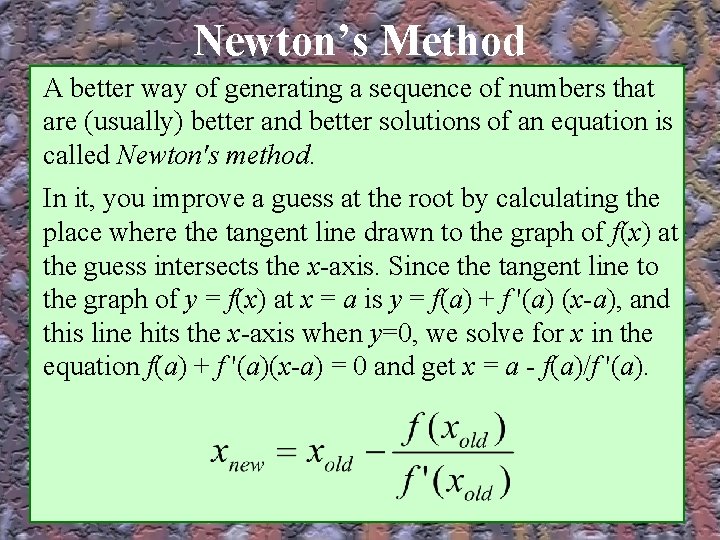
Newton’s Method A better way of generating a sequence of numbers that are (usually) better and better solutions of an equation is called Newton's method. In it, you improve a guess at the root by calculating the place where the tangent line drawn to the graph of f(x) at the guess intersects the x-axis. Since the tangent line to the graph of y = f(x) at x = a is y = f(a) + f '(a) (x-a), and this line hits the x-axis when y=0, we solve for x in the equation f(a) + f '(a)(x-a) = 0 and get x = a - f(a)/f '(a).

Let’s try it on the same function we used before, with the guess that the root x 1 = 2. Then the next guess is This is 1. 8. Let's try it again. A calculator helps:

We’re already quite close. . . with much less work than in the bisection method! One more time: And according to Maple, the root is fsolve(f(x)=0); 1. 769292354 So with not much work we have the answer to six significant figures!

Your turn… Try Newton's method out on the equation First make a reasonable guess, then iterate. Report your answer when you get two successive iterations to agree to five decimal places.

Fractals are geometric figures constructed as a limit of a sequence of geometric figures. Koch Snowflake Sierpinski Gasket Newton's method fractals

Good night! See you on Wednesdy Check the web! Turn in homework! Have a great week!
 Flipped math calculus
Flipped math calculus 30rates
30rates Calculus limits review
Calculus limits review Pre calculus unit 1 review
Pre calculus unit 1 review Apple notes
Apple notes Programa 104
Programa 104 Lativ104
Lativ104 Calculus with analytic geometry examples
Calculus with analytic geometry examples Odette building 104
Odette building 104 Fyodor urnov wife
Fyodor urnov wife Psalm 104
Psalm 104 Gg104 risk assessment
Gg104 risk assessment Cct 104
Cct 104 Aphorism 83 to 104
Aphorism 83 to 104 Cao 104
Cao 104 Cao design
Cao design Baby i'm foolish maybe i'm blind
Baby i'm foolish maybe i'm blind Csci 104 usc
Csci 104 usc How to solve conversion problems
How to solve conversion problems I 102 104
I 102 104 Daily 10/4 keller williams
Daily 10/4 keller williams Cs 104
Cs 104 104-r planned academic worksheet
104-r planned academic worksheet Tel 104
Tel 104 Lesson 1 rational numbers
Lesson 1 rational numbers Psalm 104 nbv
Psalm 104 nbv Historia de teclado
Historia de teclado 100 101 102 103 104 105 106 107 108 109 110
100 101 102 103 104 105 106 107 108 109 110 240-104
240-104 Sum 104 kg
Sum 104 kg Explain the following lines (104-110):
Explain the following lines (104-110): Prime number of 105
Prime number of 105 50000/67
50000/67 Grupo gerdau
Grupo gerdau Legge 104
Legge 104 Ps 104
Ps 104 Passivity rule opa
Passivity rule opa Mmorandum
Mmorandum Psalm 22 nbv
Psalm 22 nbv P 94
P 94 Psak 104
Psak 104 Fajar memiliki 7 kantong kelereng
Fajar memiliki 7 kantong kelereng Csci 104
Csci 104 Xkcd tommy tables
Xkcd tommy tables Math game math hit the button
Math game math hit the button Number sentence
Number sentence Daily math review 4th grade
Daily math review 4th grade Ap biology math review
Ap biology math review Ap biology math review
Ap biology math review Math 20-1 trigonometry review
Math 20-1 trigonometry review Demorgan's law proof
Demorgan's law proof