6 Lectures Theoretical Glaciology 3 Shallow Ice Approximation

6 Lectures / Theoretical Glaciology 3. Shallow Ice Approximation for Ice Sheets Contents 3. 1 Motivation 3. 2 Geometry and variables, boundary value problem 3. 3 Scaling analysis 3. 4 Limit Theory: Shallow Ice Approximation (SIA) 3. 5 Some results from climate computations 3. 6 Limitations of the SIA Kolumban Hutter, VAW-ETHZ 1

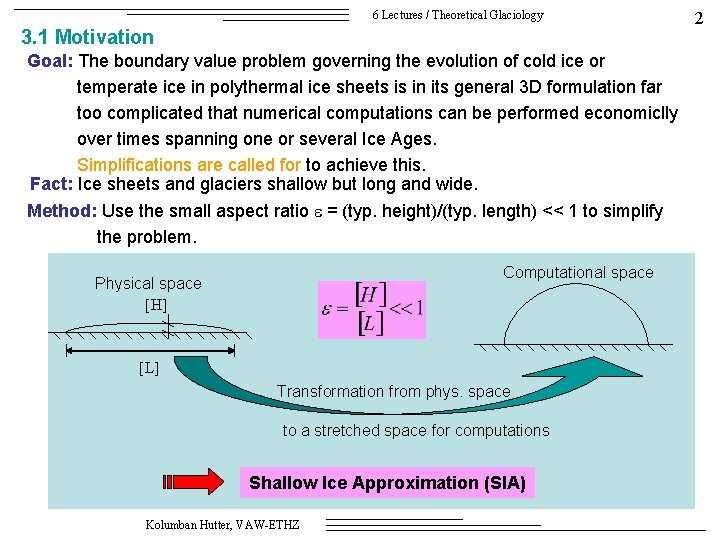
6 Lectures / Theoretical Glaciology 3. 1 Motivation Goal: The boundary value problem governing the evolution of cold ice or temperate ice in polythermal ice sheets is in its general 3 D formulation far too complicated that numerical computations can be performed economiclly over times spanning one or several Ice Ages. Simplifications are called for to achieve this. Fact: Ice sheets and glaciers shallow but long and wide. Method: Use the small aspect ratio = (typ. height)/(typ. length) << 1 to simplify the problem. Computational space Physical space [H] [L] Transformation from phys. space to a stretched space for computations Shallow Ice Approximation (SIA) Kolumban Hutter, VAW-ETHZ 2

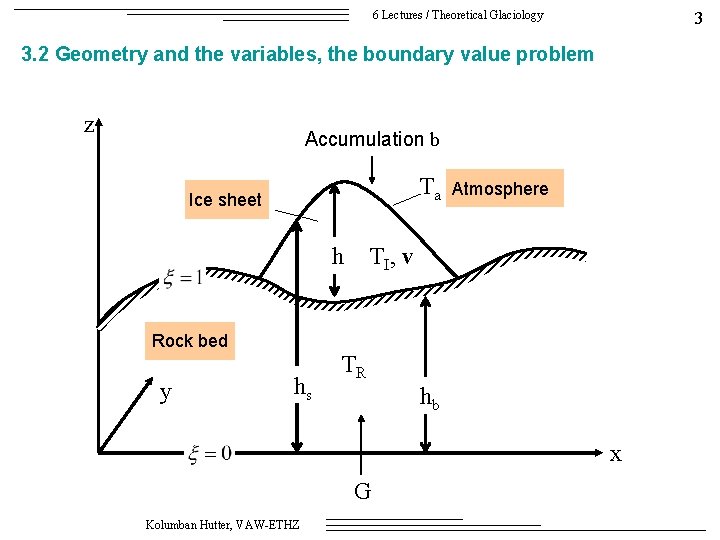
6 Lectures / Theoretical Glaciology 3 3. 2 Geometry and the variables, the boundary value problem z Accumulation b Ta Ice sheet h Rock bed y hs Atmosphere TI, v TR hb x G Kolumban Hutter, VAW-ETHZ

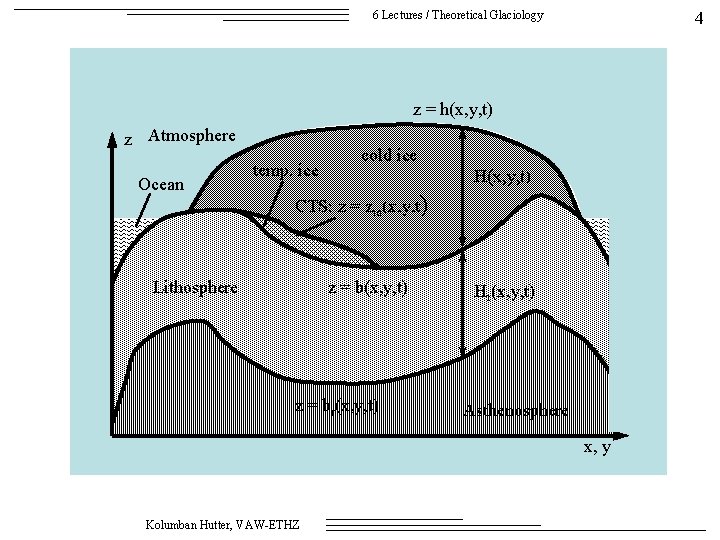
6 Lectures / Theoretical Glaciology 4 z = h(x, y, t) z Atmosphere Ocean temp. ice cold ice H(x, y, t) CTS: z = zm(x, y, t) Lithosphere z = b(x, y, t) z = br(x, y, t) Hr(x, y, t) Asthenosphere x, y Kolumban Hutter, VAW-ETHZ

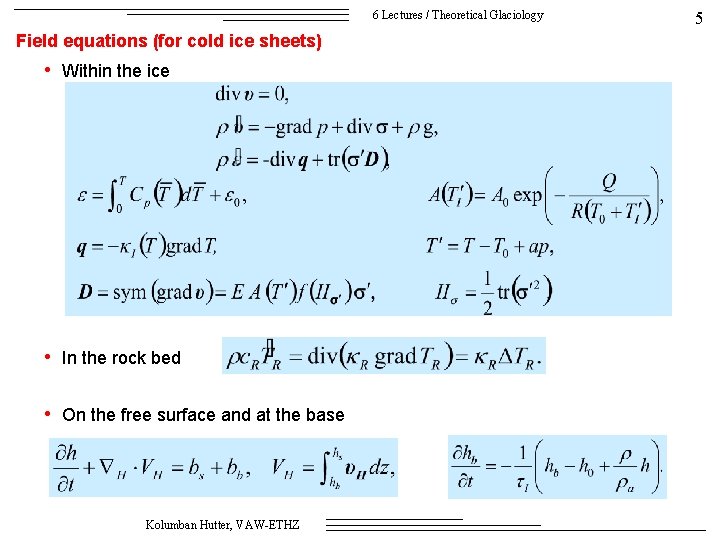
6 Lectures / Theoretical Glaciology Field equations (for cold ice sheets) • Within the ice • In the rock bed • On the free surface and at the base Kolumban Hutter, VAW-ETHZ 5

6 Lectures / Theoretical Glaciology Boundary conditions Kolumban Hutter, VAW-ETHZ 6

6 Lectures / Theoretical Glaciology 3. 3 The scaling analysis · Length horizontal [L] 105 -106 m vertical [H] 102 -103 m · Velocities horizontal [U] 102 -103 m a-1 vertical [W] 1 m a-1 · Stresses [ρg. H] 105 -106 Pa (pressure, frictional stresses) · Temperature [T] 273. 15° K (Temperature range) [ΔT] 20 -30° K · Typical stretching [D]=[a]/[H] 10 -3 -10 -1 a-1 * · Typical material stress [σ]= [ρg. H] *[a] is a typical accumulation rate Kolumban Hutter, VAW-ETHZ 104 -105 Pa 7

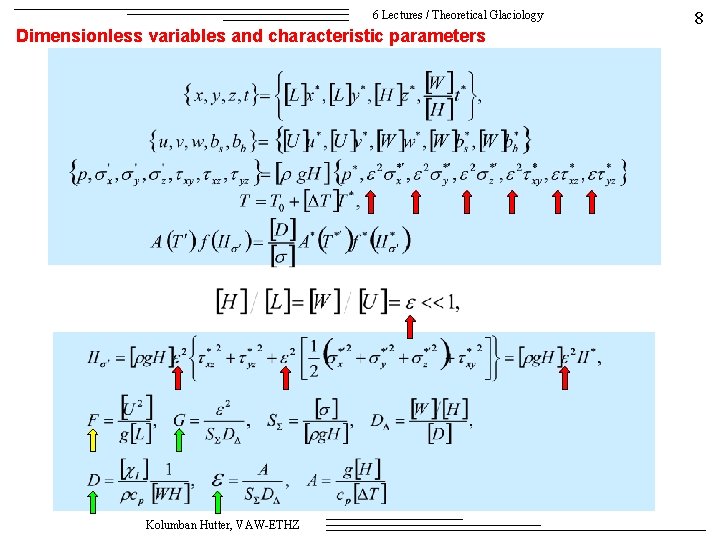
6 Lectures / Theoretical Glaciology Dimensionless variables and characteristic parameters Kolumban Hutter, VAW-ETHZ 8

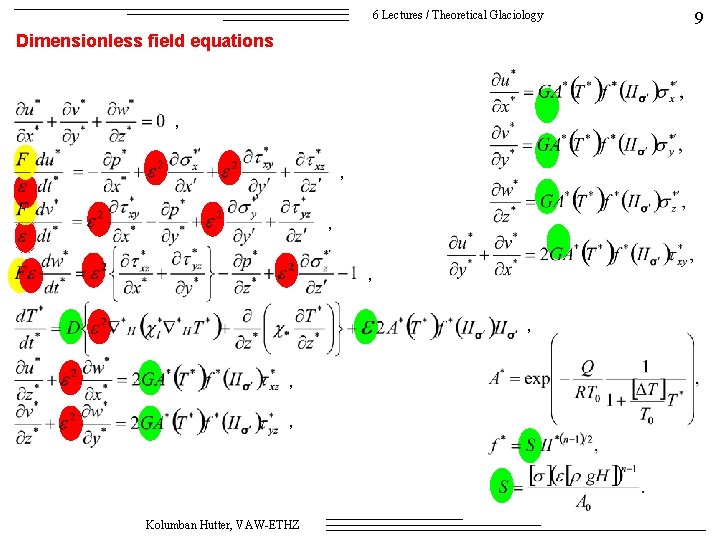
6 Lectures / Theoretical Glaciology Dimensionless field equations , , , , Kolumban Hutter, VAW-ETHZ 9

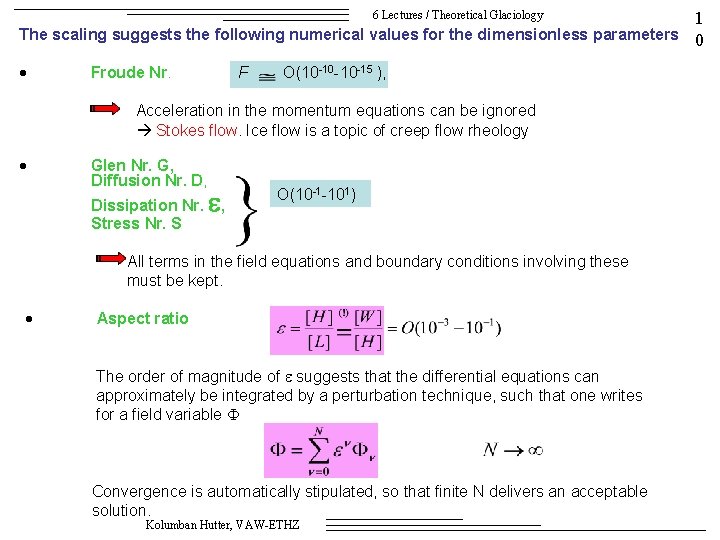
6 Lectures / Theoretical Glaciology The scaling suggests the following numerical values for the dimensionless parameters · Froude Nr. F O(10 -10 -10 -15 ), Acceleration in the momentum equations can be ignored Stokes flow. Ice flow is a topic of creep flow rheology · Glen Nr. G, Diffusion Nr. D, Dissipation Nr. Stress Nr. S , O(10 -1 -101) All terms in the field equations and boundary conditions involving these must be kept. · Aspect ratio The order of magnitude of suggests that the differential equations can approximately be integrated by a perturbation technique, such that one writes for a field variable Convergence is automatically stipulated, so that finite N delivers an acceptable solution. Kolumban Hutter, VAW-ETHZ 1 0

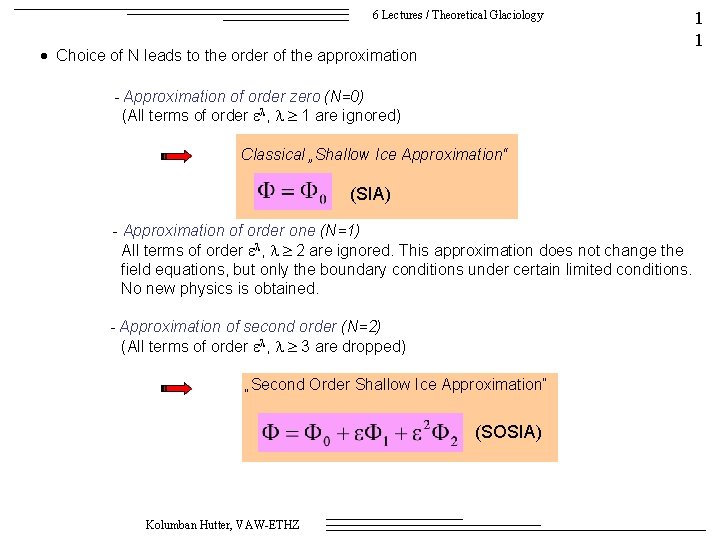
6 Lectures / Theoretical Glaciology 1 1 · Choice of N leads to the order of the approximation - Approximation of order zero (N=0) (All terms of order , 1 are ignored) Classical „Shallow Ice Approximation“ (SIA) - Approximation of order one (N=1) All terms of order , 2 are ignored. This approximation does not change the field equations, but only the boundary conditions under certain limited conditions. No new physics is obtained. - Approximation of second order (N=2) (All terms of order , 3 are dropped) „Second Order Shallow Ice Approximation“ (SOSIA) Kolumban Hutter, VAW-ETHZ

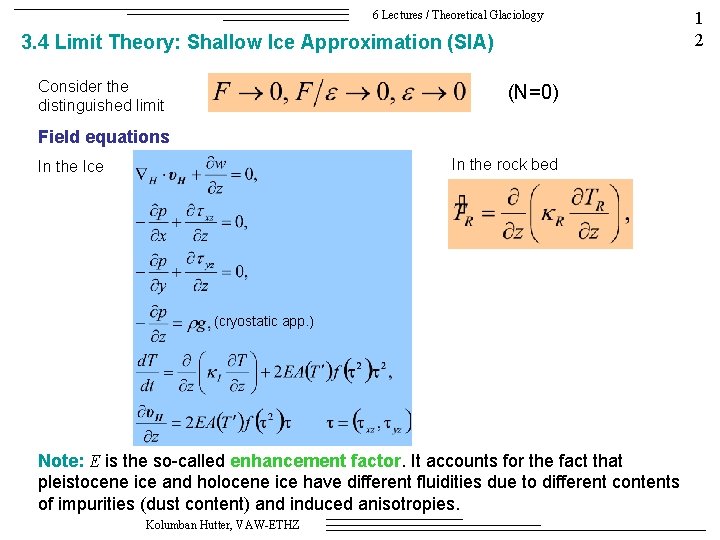
6 Lectures / Theoretical Glaciology 3. 4 Limit Theory: Shallow Ice Approximation (SIA) Consider the distinguished limit (N=0) Field equations In the rock bed In the Ice (cryostatic app. ) Note: E is the so-called enhancement factor. It accounts for the fact that pleistocene ice and holocene ice have different fluidities due to different contents of impurities (dust content) and induced anisotropies. Kolumban Hutter, VAW-ETHZ 1 2

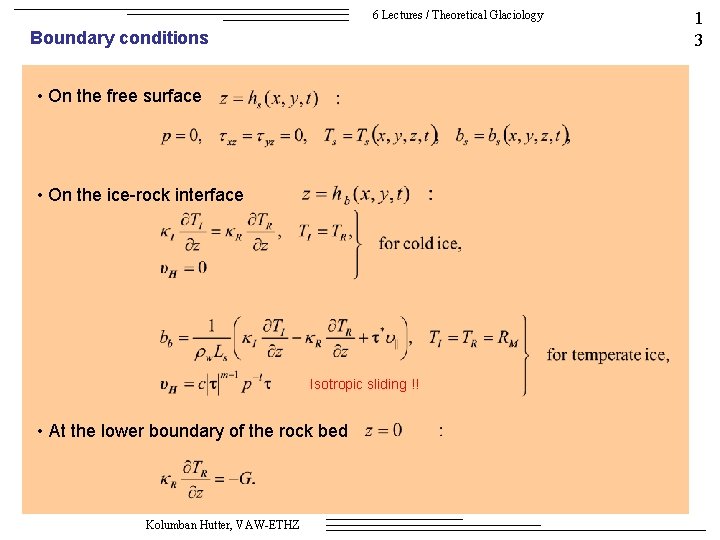
6 Lectures / Theoretical Glaciology Boundary conditions • On the free surface • On the ice-rock interface Isotropic sliding !! • At the lower boundary of the rock bed Kolumban Hutter, VAW-ETHZ 1 3

6 Lectures / Theoretical Glaciology Integration (i) The cryostatic pressure equation together with the SIA-approximated boundary condition at the free surface can be integrated. (ii) Substitution of that result into the horizontal force (momentum) balances allows determination of the shear stresses xz, yz. In this integration step the stress boundary conditions at the free surface are used. → Determination of these stresses is achievable without using material equations. (iii) With the stresses p, xz, yz determined, the right-hand sides of the material equations are now known, if one assumes that the temperature distribution is equally known, which we assume to hold. Integration of (*) in z and determination of the „corresponding constant of integration“ by the sliding condition at the base then yields the quadrature formula for the horizontal velocity components. (iv) With the continuity equation , the vertical velocity component w follows from a further integration in z and use of the basal sliding condition. Kolumban Hutter, VAW-ETHZ 1 4

6 Lectures / Theoretical Glaciology (cold ice) (temperate ice) Kolumban Hutter, VAW-ETHZ 1 5

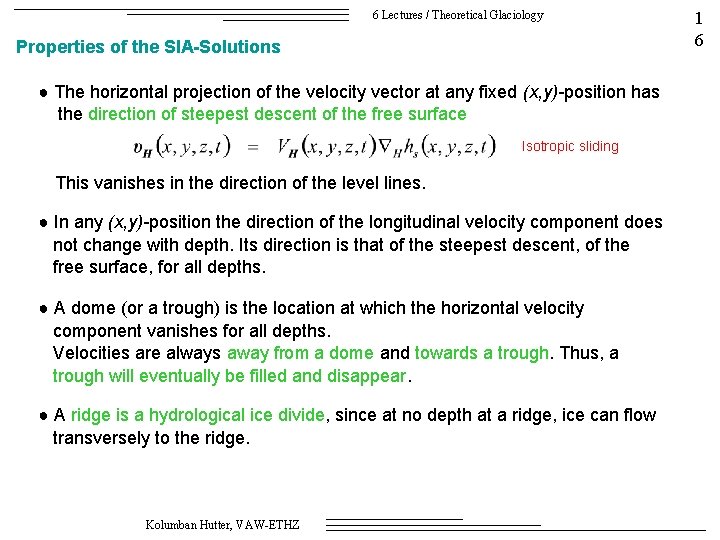
6 Lectures / Theoretical Glaciology Properties of the SIA-Solutions ● The horizontal projection of the velocity vector at any fixed (x, y)-position has the direction of steepest descent of the free surface Isotropic sliding This vanishes in the direction of the level lines. ● In any (x, y)-position the direction of the longitudinal velocity component does not change with depth. Its direction is that of the steepest descent, of the free surface, for all depths. ● A dome (or a trough) is the location at which the horizontal velocity component vanishes for all depths. Velocities are always away from a dome and towards a trough. Thus, a trough will eventually be filled and disappear. ● A ridge is a hydrological ice divide, since at no depth at a ridge, ice can flow transversely to the ridge. Kolumban Hutter, VAW-ETHZ 1 6

6 Lectures / Theoretical Glaciology Kolumban Hutter, VAW-ETHZ 1 7

6 Lectures / Theoretical Glaciology Remarks: ● The SIA-equations are used in many numerical programs by computational specialists: Calov, Greve, Huybrechts, Mac. Ayel, Ritz, . . . For whole-earth modeling there is also an SIA version in spherical coordinates, Calov, Greve ● The SIA equations have also been applied for polythermal ice masses. The software, due to Greve is known as SICOPOLIS (SImulation COde for POLythemal Ice Sheets) ● The horizontal projection of the trajectories of the ice particles can be determined in the SIA-approximation by constructing from topographic maps the orthogonal trajectories to the level lines. ● If observations contradict results of the SIA then certain assumptions of the SIA are not satisfied, e. g. , - Sliding at the base may be anisotropic, - Topography of the base may locally be steep, -. . Kolumban Hutter, VAW-ETHZ 1 8

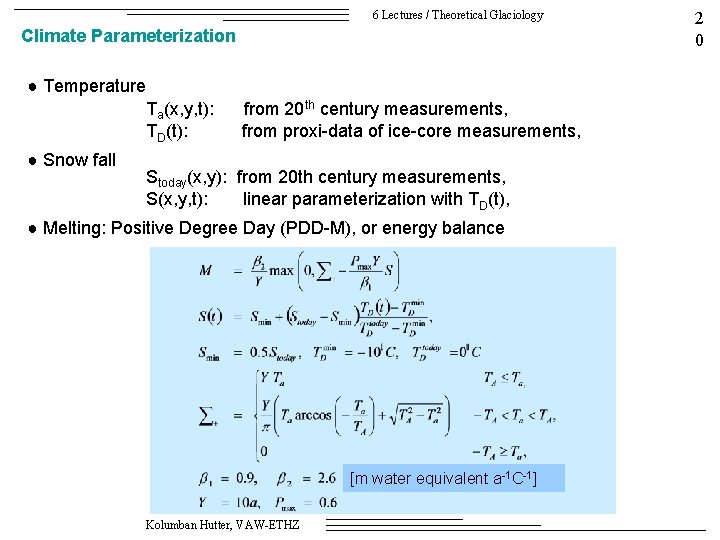
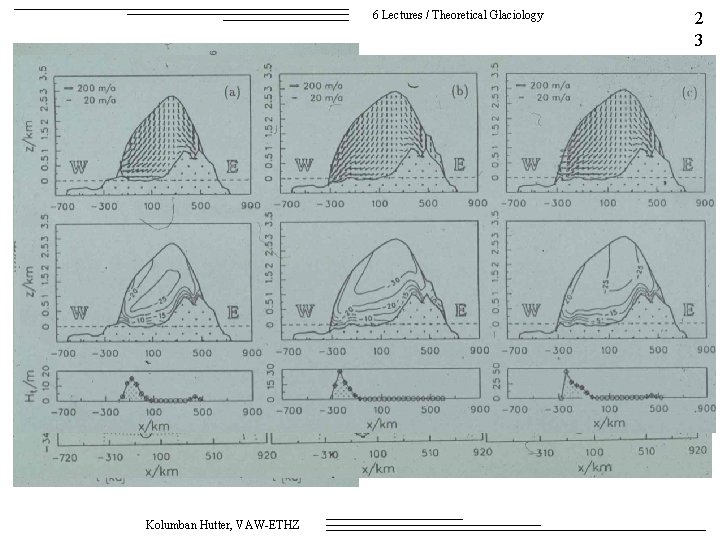
6 Lectures / Theoretical Glaciology 3. 5 Some results from climate computations Model: SICOPOLIS ● Simple parameterization of the climate input - Geothermal heat flow 5 km below the ice rock interface Qgeoth=42 -55 m. W m-2 - Relaxing Lithosophere-Asthenosphere system with relaxation time =3000 years ● Glen´s flow law with enhancement factor E - E=1, for ice younger than 11 ka (holocene ice) - E=3, for ice older than 11 ka (pleistocene ice) ● Simplified climate input for the free surface, temperature and accumulation function. Atm. Temperature at free surface: Accumulation-Ablation rate: S=snow fall, M= melting rate Kolumban Hutter, VAW-ETHZ 1 9

6 Lectures / Theoretical Glaciology Climate Parameterization ● Temperature Ta(x, y, t): from 20 th century measurements, TD(t): from proxi-data of ice-core measurements, ● Snow fall Stoday(x, y): from 20 th century measurements, S(x, y, t): linear parameterization with TD(t), ● Melting: Positive Degree Day (PDD-M), or energy balance [m water equivalent a-1 C-1] Kolumban Hutter, VAW-ETHZ 2 0

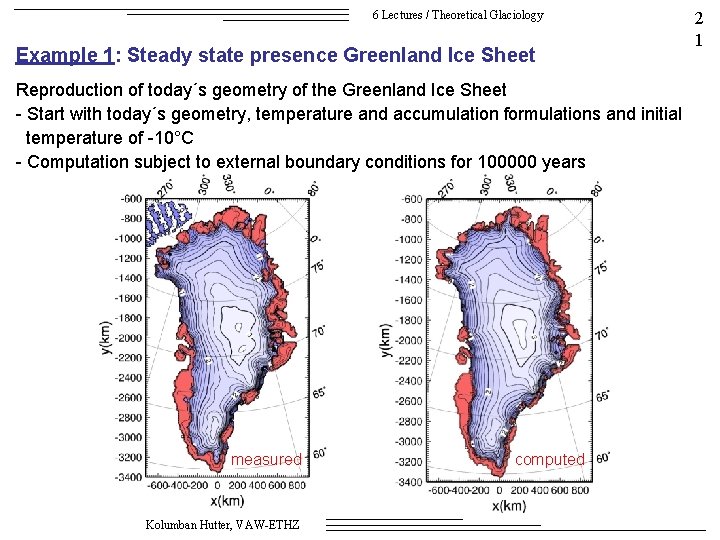
6 Lectures / Theoretical Glaciology Example 1: Steady state presence Greenland Ice Sheet Reproduction of today´s geometry of the Greenland Ice Sheet - Start with today´s geometry, temperature and accumulation formulations and initial temperature of -10°C - Computation subject to external boundary conditions for 100000 years measured Kolumban Hutter, VAW-ETHZ computed 2 1

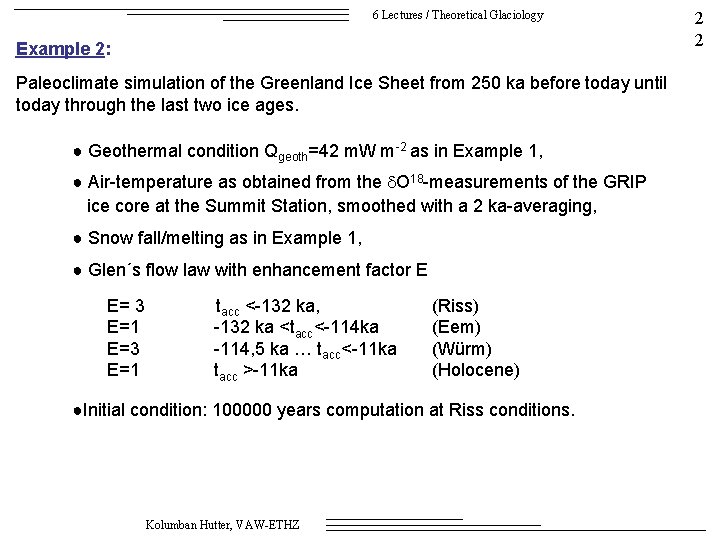
6 Lectures / Theoretical Glaciology Example 2: Paleoclimate simulation of the Greenland Ice Sheet from 250 ka before today until today through the last two ice ages. ● Geothermal condition Qgeoth=42 m. W m-2 as in Example 1, ● Air-temperature as obtained from the O 18 -measurements of the GRIP ice core at the Summit Station, smoothed with a 2 ka-averaging, ● Snow fall/melting as in Example 1, ● Glen´s flow law with enhancement factor E E= 3 tacc <-132 ka, E=1 -132 ka <tacc<-114 ka E=3 -114, 5 ka … tacc<-11 ka E=1 tacc >-11 ka (Riss) (Eem) (Würm) (Holocene) ●Initial condition: 100000 years computation at Riss conditions. Kolumban Hutter, VAW-ETHZ 2 2

6 Lectures / Theoretical Glaciology Kolumban Hutter, VAW-ETHZ 2 3

6 Lectures / Theoretical Glaciology Example 3: Antropogenically caused sudden temperature rise by 2, 4, 6° K, kept constant from today, 5000 years into the furure. Results after 5000 years ΔTmin 2°C 4°C 6°C ΔVol Sea level rise 4. 9% 13. 4% 87. 2% 0. 34 m 2. 6 m 6. 1 m Greve, 1995 Kolumban Hutter, VAW-ETHZ 2 4

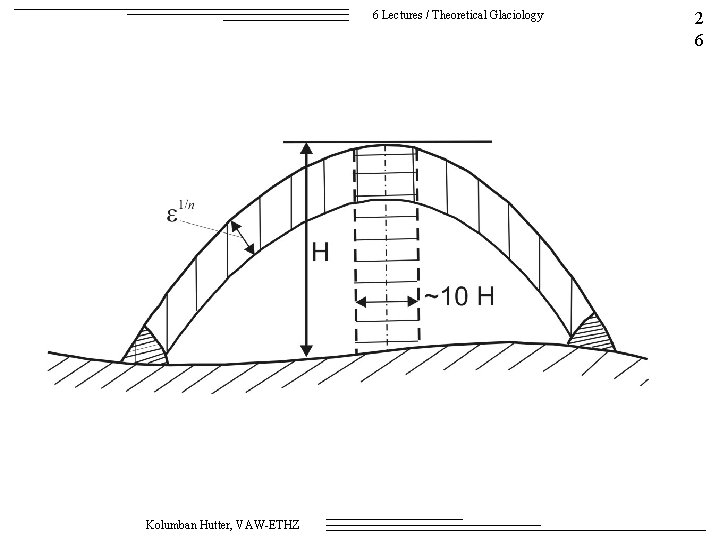
6 Lectures / Theoretical Glaciology 3. 6 Limitations of the SIA There are three regions in ice sheets, in which the SIA is physically not satisfactory and must be corrected. (i) In the vicinity of domes and, in 2 D or 3 D, ice divides the SIA is violated since vertical velocities are much larger than horizontal velocities. (ii) In a boundary layer, close to the free surface normal stresses of the (dissipative) stress deviator are not negligible. An infinite viscosity law generates a singularity and makes these stresses infinitely large. A finite viscosity law regularizes the problem, but normal stresses close to the surface are still large. (iii) Close to the margin, where surface gradients are not small. This boundary region is a region typical of contact line problems. It is a passive boundary layer and in glacier and ice sheet problems of subgrid scale. Kolumban Hutter, VAW-ETHZ 2 5

6 Lectures / Theoretical Glaciology Kolumban Hutter, VAW-ETHZ 2 6

6 Lectures / Theoretical Glaciology Evolution equation for the free surface in the SIA (i) This equation demonstrates the singularity at a dome for an infinite viscosity law, for which f(0)=0, and Hhs=0. Regularization by using a finite viscosity law helps little since the apparent viscosity at small strain rates remains large, yielding inaccuracies. (ii) Cure: (1) matched asymptotics [Wilchinsky & Chugunov, 2001], (2) go to SOSIA [Baral, Greve & Hutter 2001, Margeney et al, 2001 and others] Kolumban Hutter, VAW-ETHZ 2 7

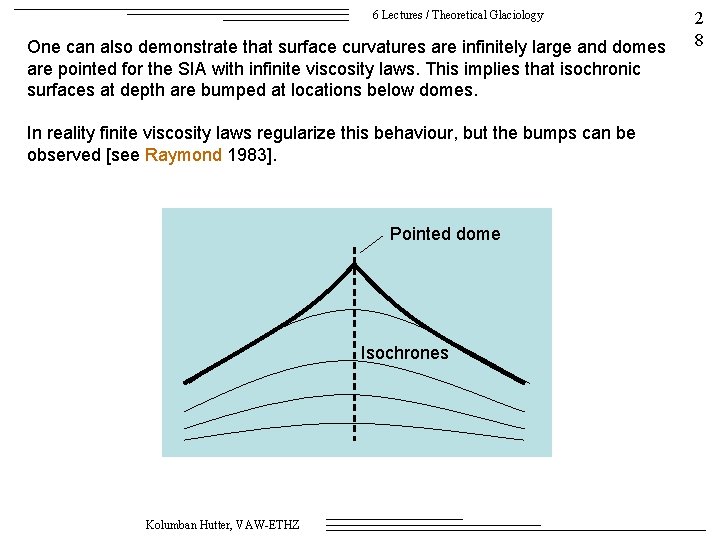
6 Lectures / Theoretical Glaciology One can also demonstrate that surface curvatures are infinitely large and domes are pointed for the SIA with infinite viscosity laws. This implies that isochronic surfaces at depth are bumped at locations below domes. In reality finite viscosity laws regularize this behaviour, but the bumps can be observed [see Raymond 1983]. Pointed dome Isochrones Kolumban Hutter, VAW-ETHZ 2 8

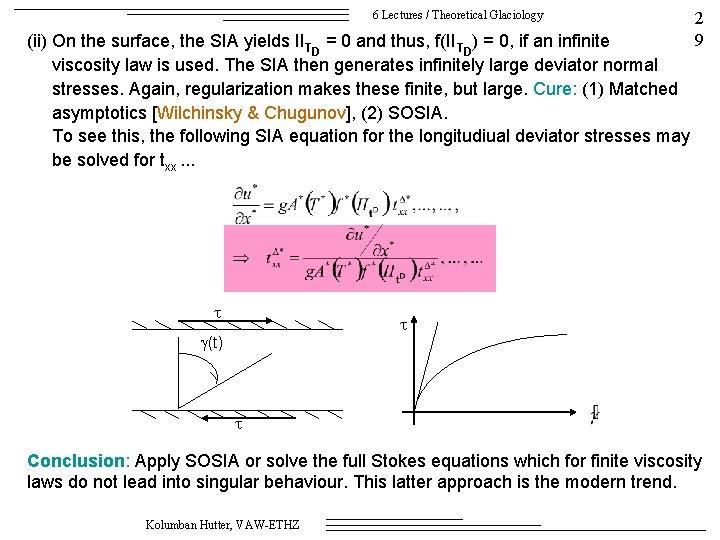
6 Lectures / Theoretical Glaciology (ii) On the surface, the SIA yields IITD = 0 and thus, f(IITD) = 0, if an infinite viscosity law is used. The SIA then generates infinitely large deviator normal stresses. Again, regularization makes these finite, but large. Cure: (1) Matched asymptotics [Wilchinsky & Chugunov], (2) SOSIA. To see this, the following SIA equation for the longitudiual deviator stresses may be solved for txx. . . 2 9 (t) Conclusion: Apply SOSIA or solve the full Stokes equations which for finite viscosity laws do not lead into singular behaviour. This latter approach is the modern trend. Kolumban Hutter, VAW-ETHZ

6 Lectures / Theoretical Glaciology Thank you Go from SIA, SSA to SOSIA, SOSSA Kolumban Hutter, VAW-ETHZ 3 0