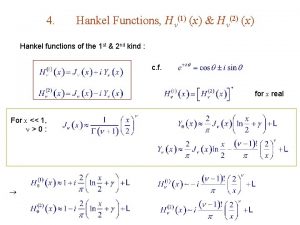
3 Laguerre Functions Laguerre ODE Rodrigues Formula Schlaefli

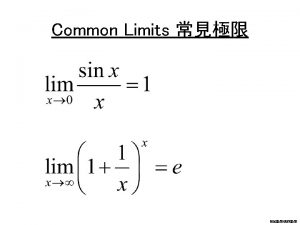
3. Laguerre Functions Laguerre ODE Rodrigues Formula : Schlaefli integral : Hermitian form Laguerre Polynomials ( n! changes scale ) C encircles x but no other singularities

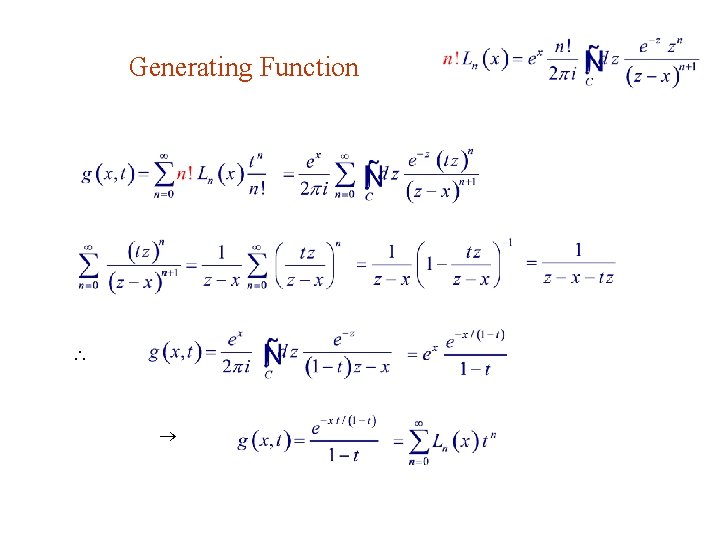
Generating Function

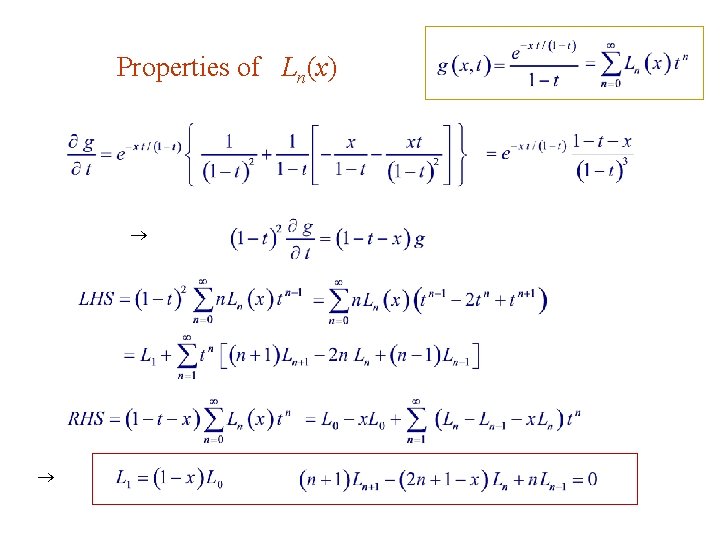
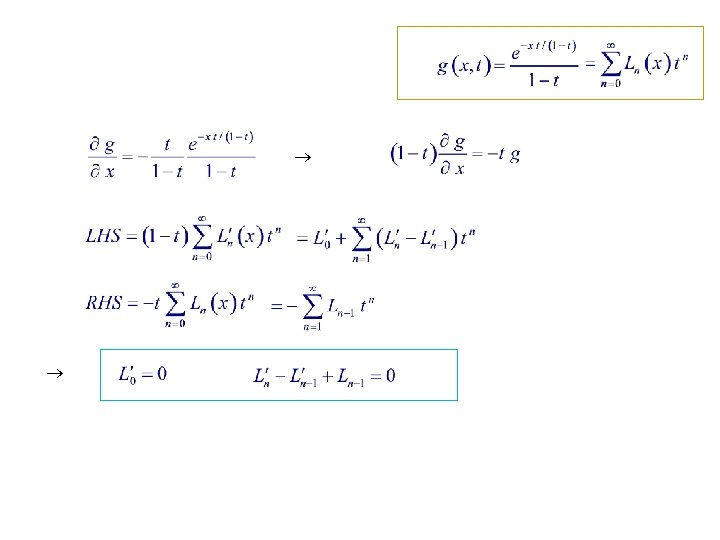
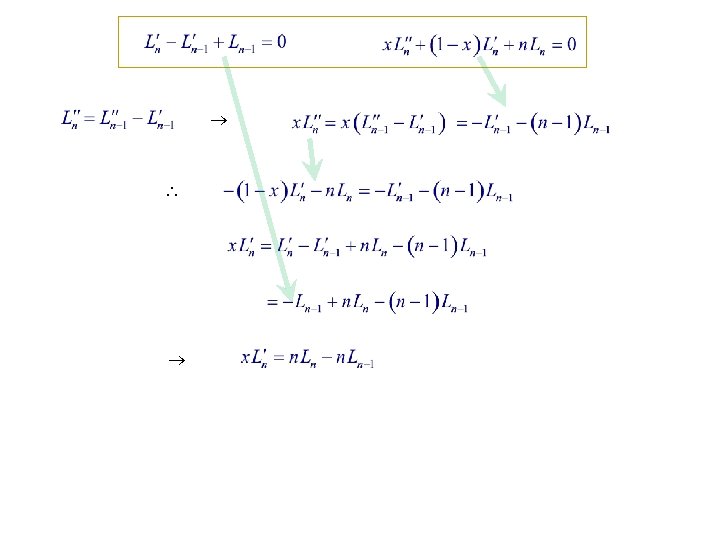
Properties of Ln(x)

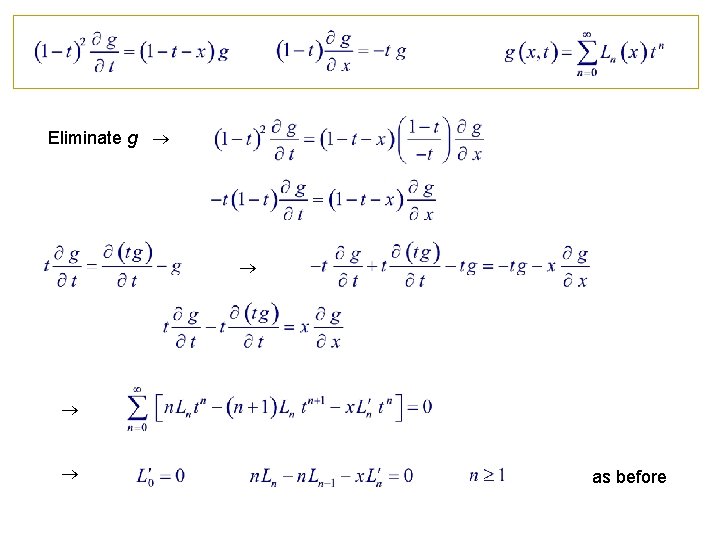
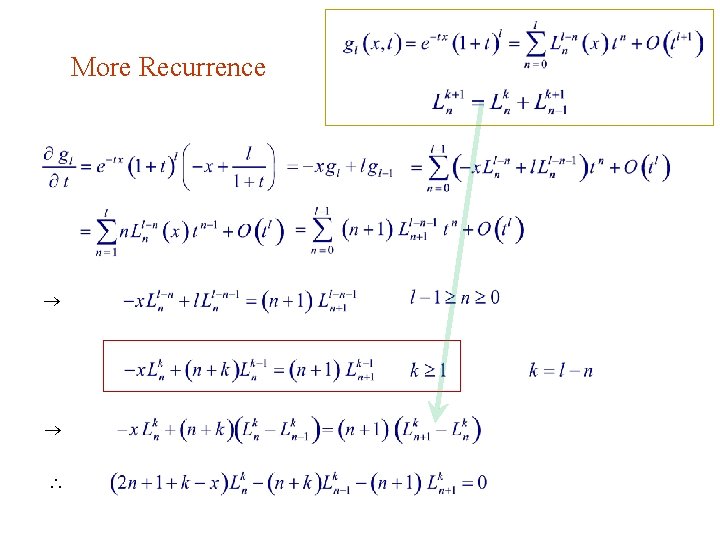
Eliminate g as before

Table & Figure. Laguerre Polynomials Ln orthogonal over [0, ] Mathematica

Power Series

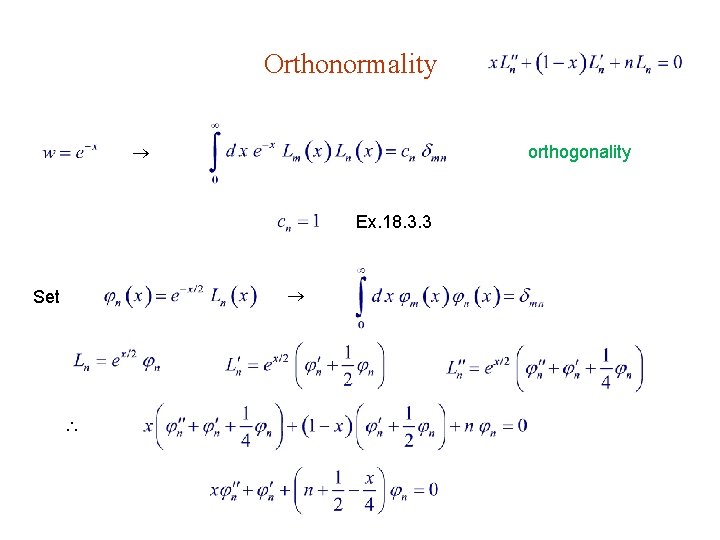
Orthonormality orthogonality Ex. 18. 3. 3 Set

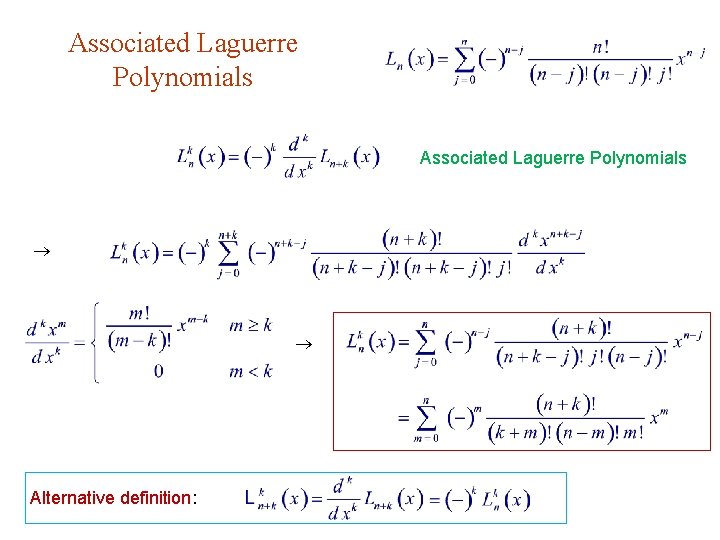
Associated Laguerre Polynomials Alternative definition:

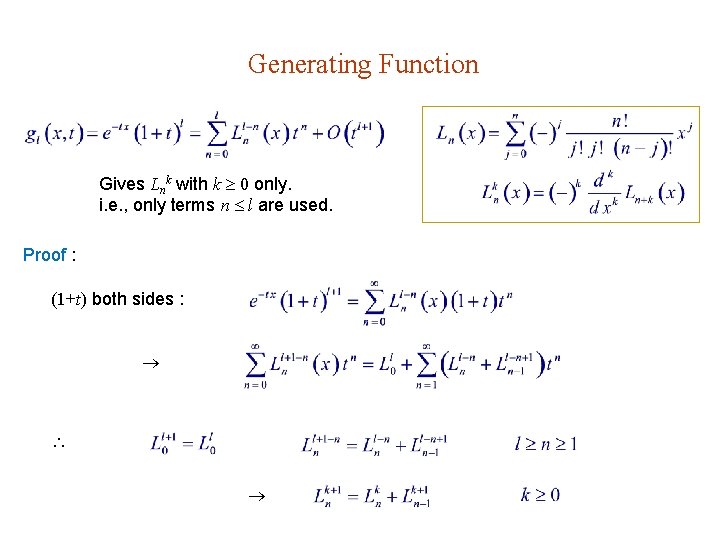
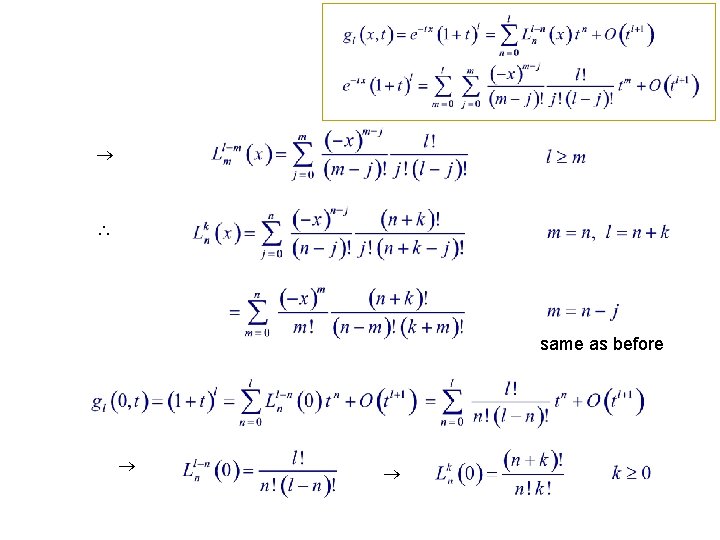
Generating Function Gives Lnk with k 0 only. i. e. , only terms n l are used. Proof : (1+t) both sides :

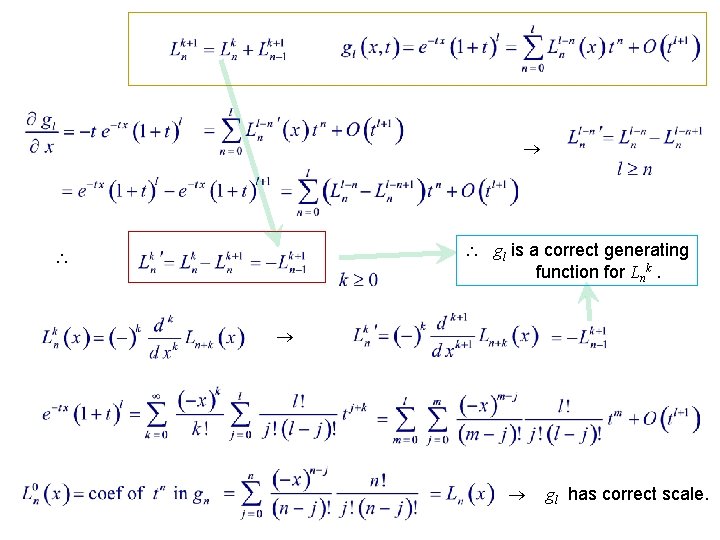
gl is a correct generating function for Lnk. gl has correct scale.

same as before

More Recurrence

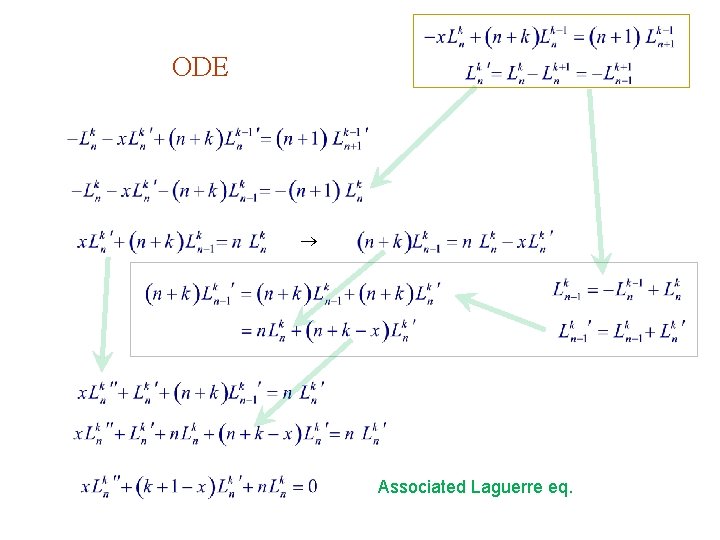
ODE Associated Laguerre eq.

Hermitian form Orthogonality obtained from

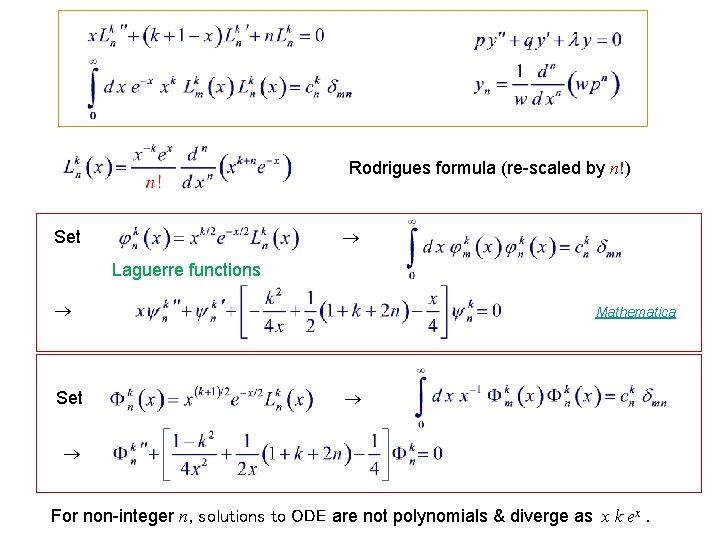
Rodrigues formula (re-scaled by n!) Set Laguerre functions Set Mathematica For non-integer n, solutions to ODE are not polynomials & diverge as x k ex.

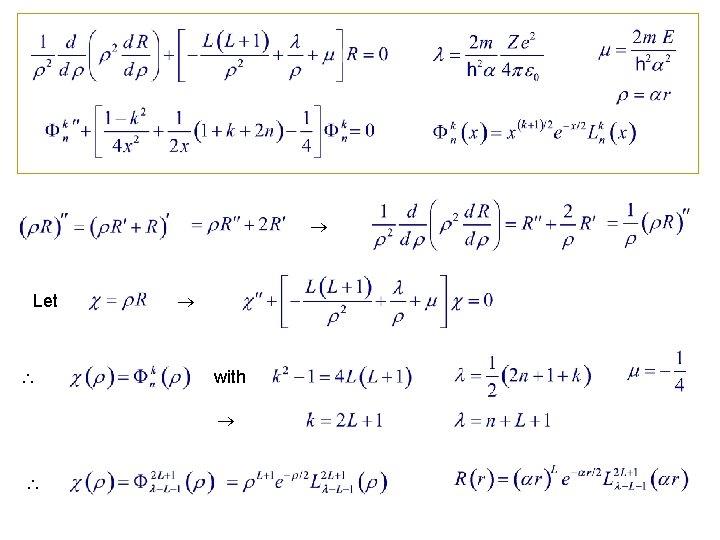
Example 18. 3. 1. The Hydrogen Atom Schrodinger eq. for H-like atom of atomic number Z. SI units B. C. for bound states : Let R(0) finite & R( ) = 0.

Let with

1 must be an integer. Integers Set Bohr radius

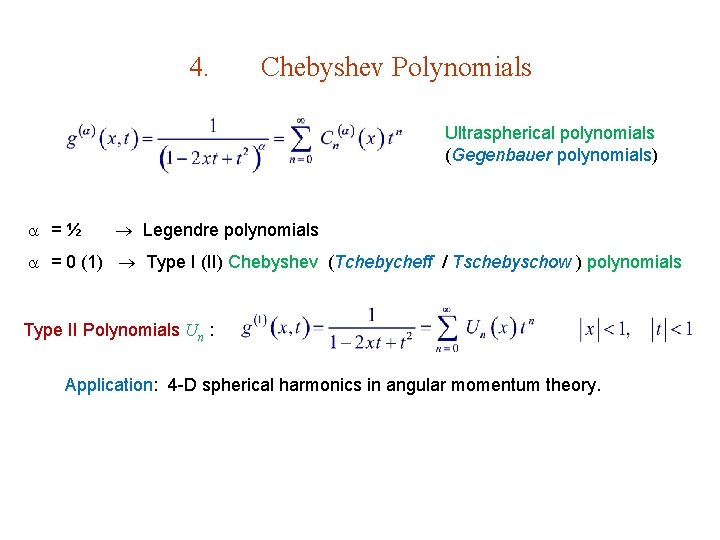
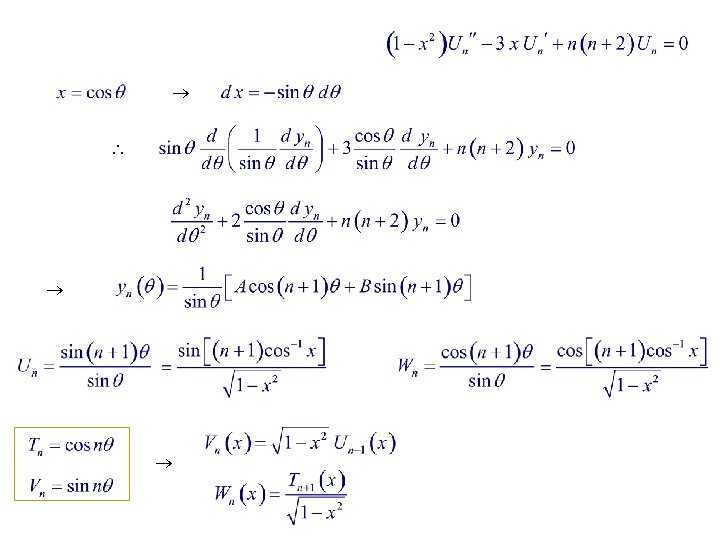
4. Chebyshev Polynomials Ultraspherical polynomials (Gegenbauer polynomials) = ½ Legendre polynomials = 0 (1) Type I (II) Chebyshev (Tchebycheff / Tschebyschow ) polynomials Type II Polynomials Un : Application: 4 -D spherical harmonics in angular momentum theory.

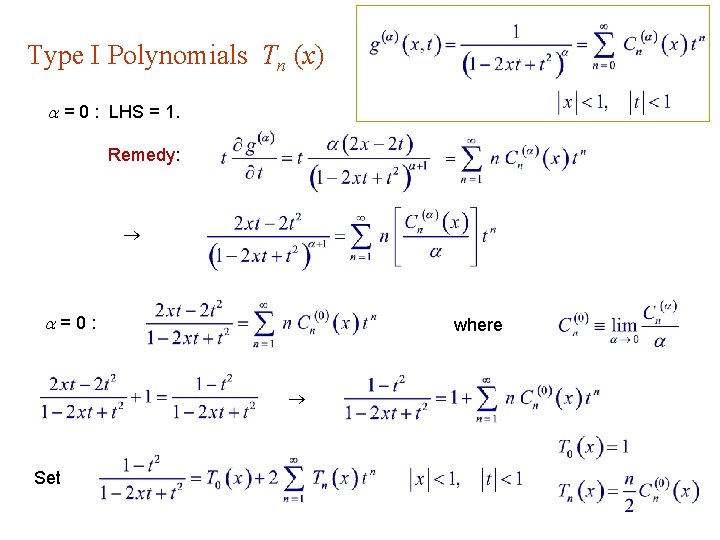
Type I Polynomials Tn (x) = 0 : LHS = 1. Remedy: = 0 : where Set

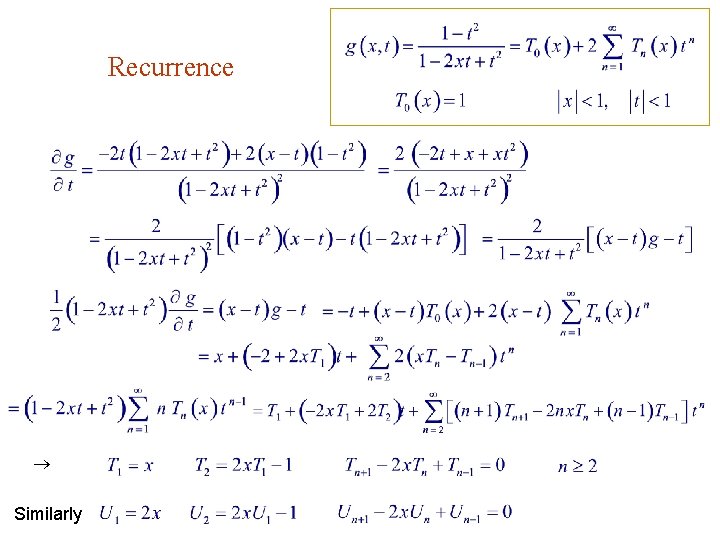
Recurrence Similarly

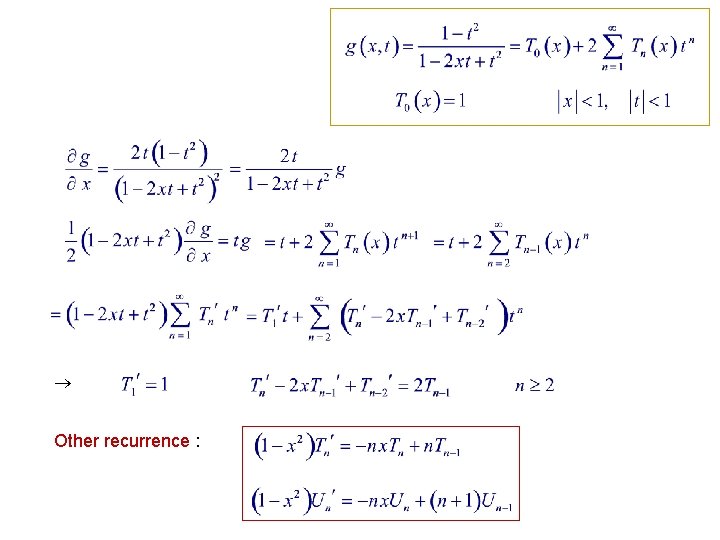
Other recurrence :

Table & Figure Mathematica

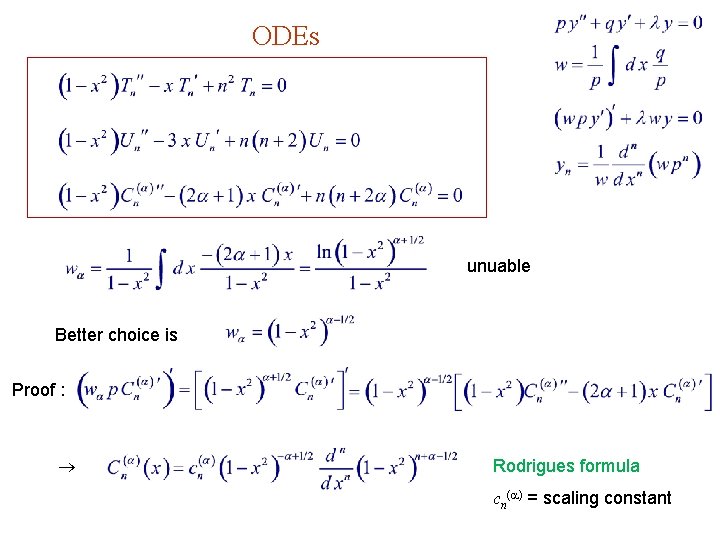
ODEs unuable Better choice is Proof : Rodrigues formula cn( ) = scaling constant

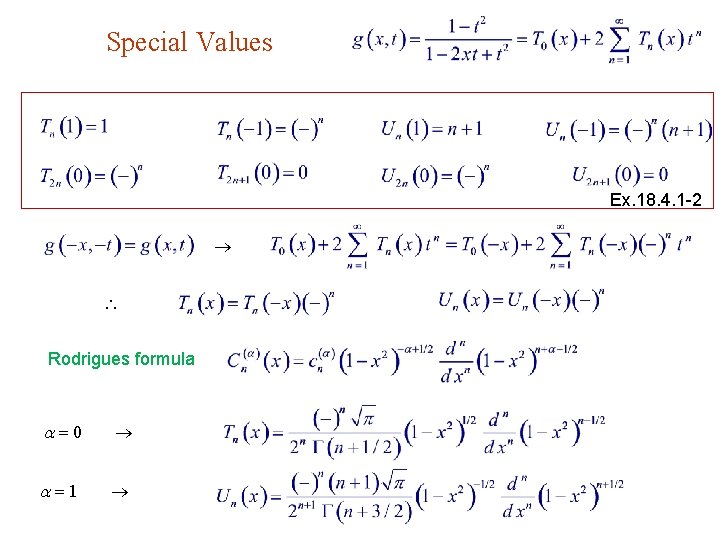
Special Values Ex. 18. 4. 1 -2 Rodrigues formula 0 1

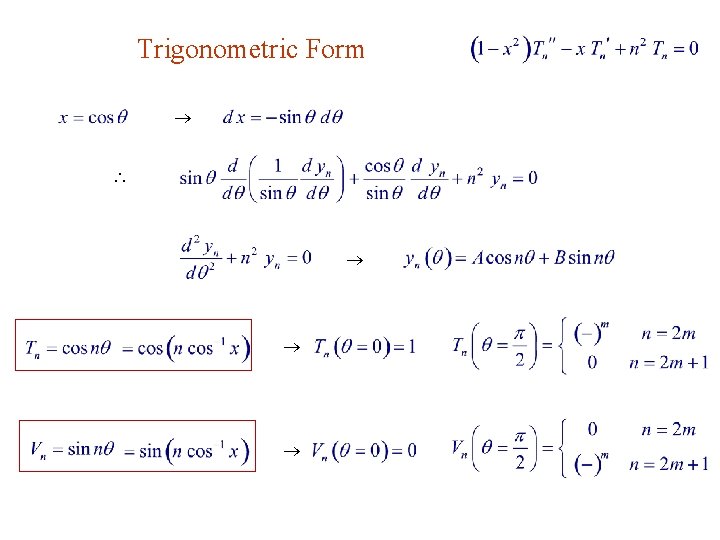
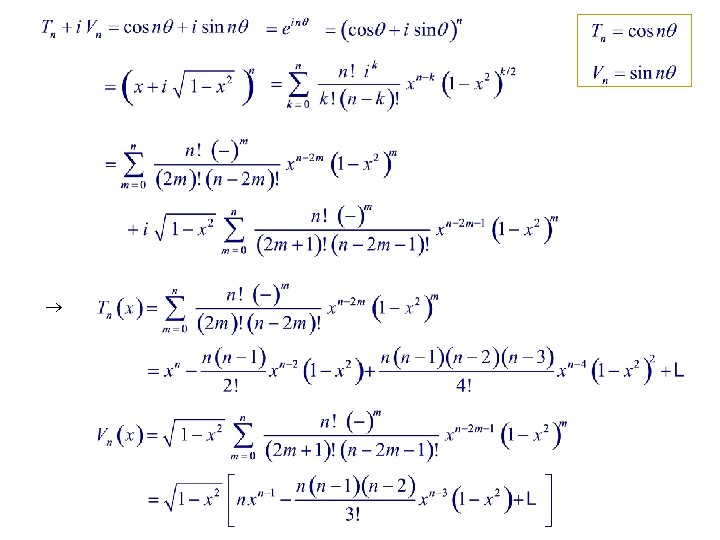
Trigonometric Form

From g( ) or ODE (Frobenius series) :

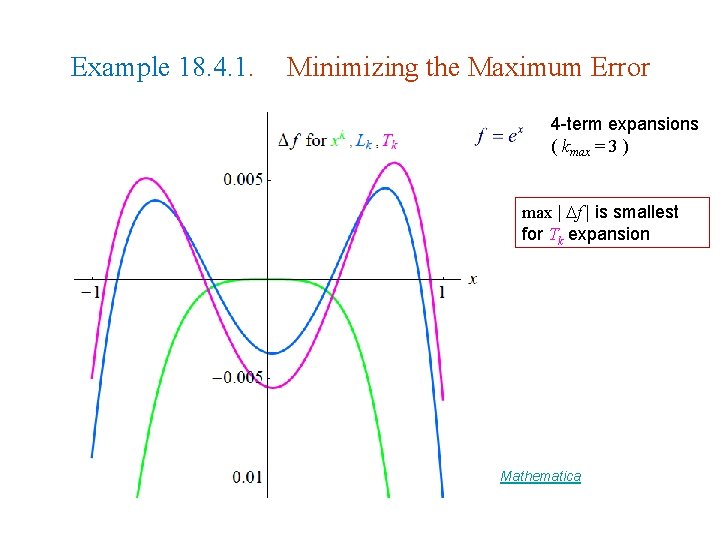
Application to Numerical Analysis Let If error decreases rapidly for m > M, then at at error satisfies the minimax principle. i. e. , max of error is minimized by spreading it into regions between points of negligible error.

Example 18. 4. 1. Minimizing the Maximum Error 4 -term expansions ( kmax = 3 ) max | f | is smallest for Tk expansion Mathematica

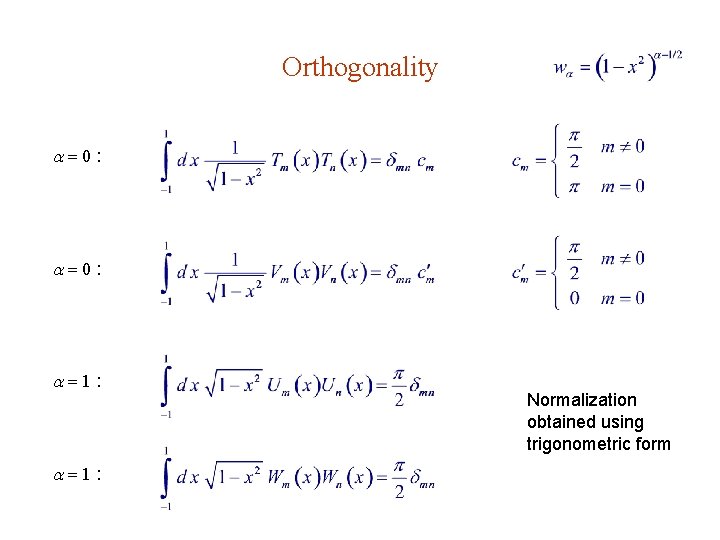
Orthogonality 0 : 1 : Normalization obtained using trigonometric form