ECO 365 Intermediate Microeconomics Lecture Notes Monopoly Market









- Slides: 24

ECO 365 – Intermediate Microeconomics Lecture Notes

Monopoly � Market environment where there is only one firm in the market � Firm faces ALL of demand � So monopoly profit = p(y)y – c(y) � Where p(y) = inverse market demand let p(y)y = r(y) revenue function � � Monopolistic problem: Choose y to Max r(y) – c(y) First order conditions are given by: MR = MC

�The same condition we got with perfect competition �But now MR does not equal P (i. e. firms not price takers) �Two effects of changing y (say increase y) on revenues � 1 -sell more so revenue increases � 2 -price decreases so revenue decreases � ∆ r (y) = p ∆y + y ∆p � ∆ r(y)/ ∆ y = MR = p + y ∆p/ ∆y or: � For price takers ∆p=0 => ∆r = p ∆y � But now P decreases as y increases so the second term matters.

�Now both 1 and 2 measure Marginal Revenue (MR) �MR= ∆r/ ∆y = p + y ∆p/ ∆y �= p(1 + (y/p)(∆p/ ∆y) �= p(y) (1 + 1/ε) �Since ε = price elasticity of demand = (p/y)(∆y/∆p) �=> can re-write optimal condition, MR = MC as: � p(y) (1 + 1/ ε(y)) = mc (y) �Or p(y) (1 - 1/| ε(y)|) = mc (y) � Since ε < 0 � Also recall that | ε | > 0 elastic � | ε | < 1 inelastic � So that if demand elastic regions | ε | > 1 � MR > 0 but if demand inelastic MR < 0

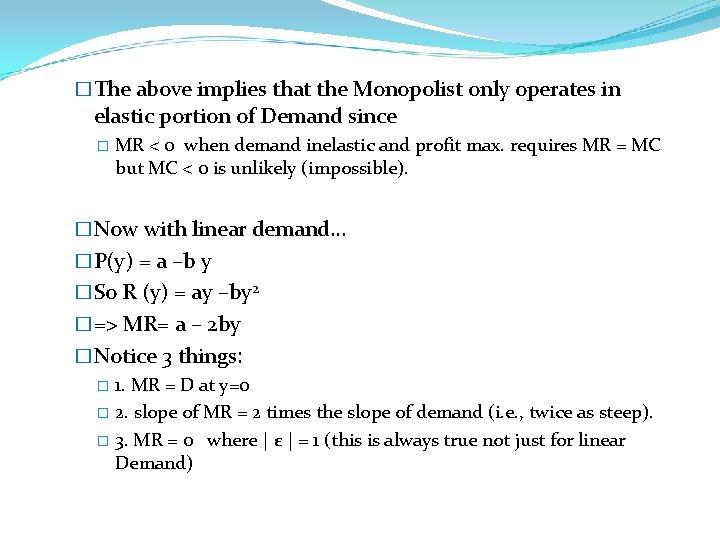
�The above implies that the Monopolist only operates in elastic portion of Demand since � MR < 0 when demand inelastic and profit max. requires MR = MC but MC < 0 is unlikely (impossible). �Now with linear demand… �P(y) = a –b y �So R (y) = ay –by 2 �=> MR= a – 2 by �Notice 3 things: � 1. MR = D at y=0 � 2. slope of MR = 2 times the slope of demand (i. e. , twice as steep). � 3. MR = 0 where | ε | = 1 (this is always true not just for linear Demand)

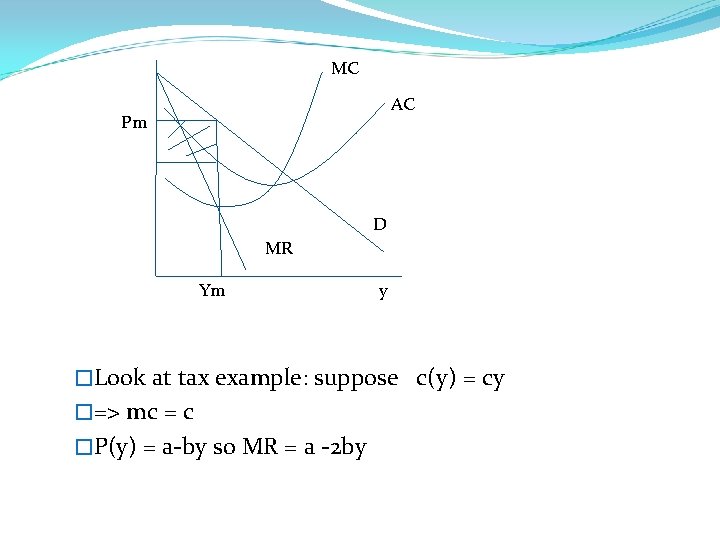
MC AC Pm D MR Ym y �Look at tax example: suppose c(y) = cy �=> mc = c �P(y) = a-by so MR = a -2 by

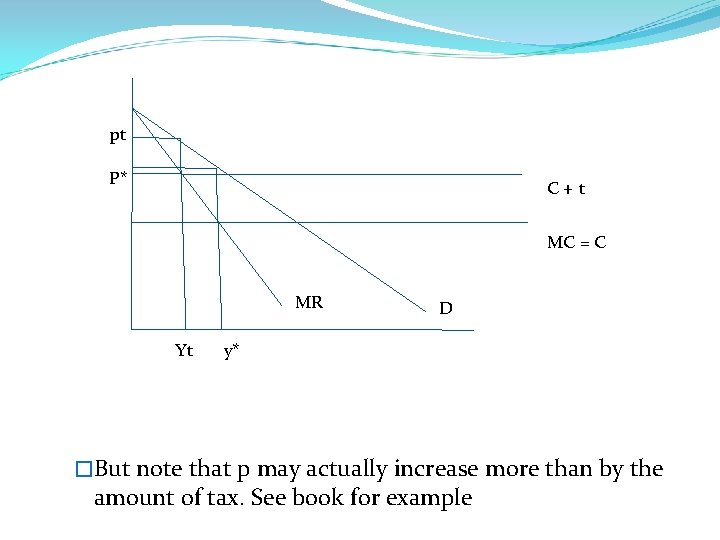
�Now suppose a tax on the monopolist = t (quantity tax) so pc = ps + t �So mc w/ tax is c + t or c(y) = (c+ t)y �=> before profit max where c = a -2 by �Or y* = a-c/2 b �Now MC = c + t = a – 2 by = MR �So y* = (a-c-t)/2 b �=> Δy/ Δ t = -1/(2 b) (why? ) �What is the impact of the tax on price, p? Recall slope of demand function = Δp/ Δy = -b, so � The tax is imposed => y changes by -1/(2 b) then � The price changes by – b, the overall impact is both of these together, � Or – b times -1/(2 b) = -1/2 � �Interpretation: if t increases by $1 => price increases by $. 50

pt P* C+t MC = C MR Yt D y* �But note that p may actually increase more than by the amount of tax. See book for example

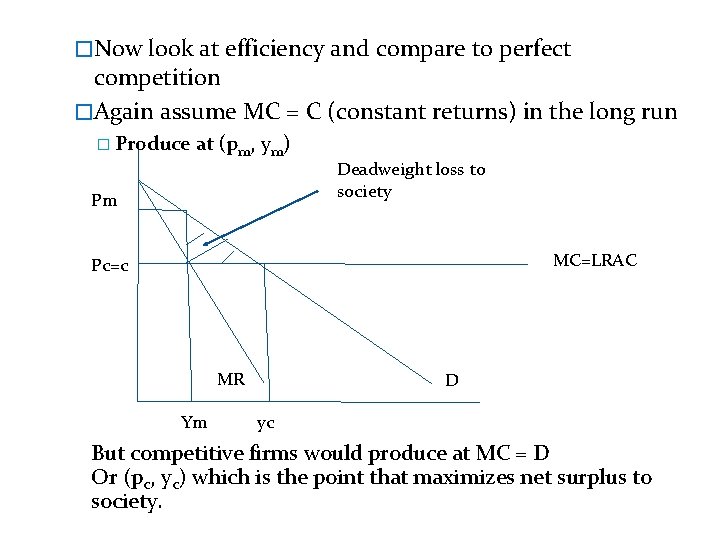
�Now look at efficiency and compare to perfect competition �Again assume MC = C (constant returns) in the long run � Produce at (pm, ym) Pm Deadweight loss to society MC=LRAC Pc=c MR Ym D yc But competitive firms would produce at MC = D Or (pc, yc) which is the point that maximizes net surplus to society.

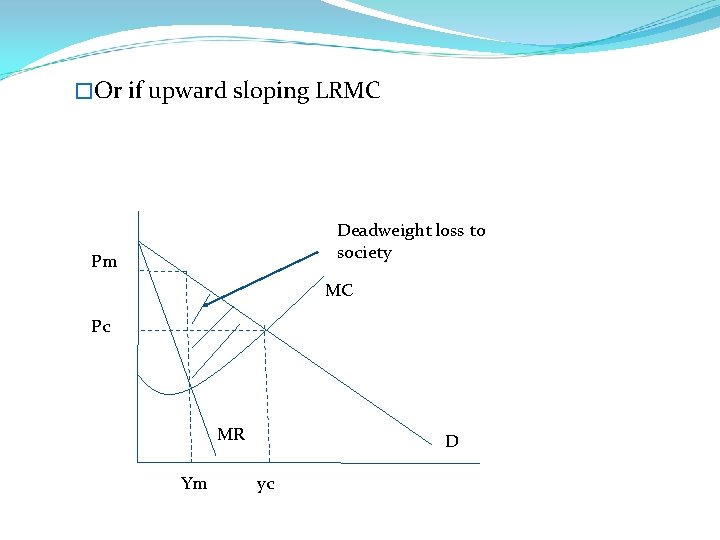
�Or if upward sloping LRMC Deadweight loss to society Pm MC Pc MR Ym D yc

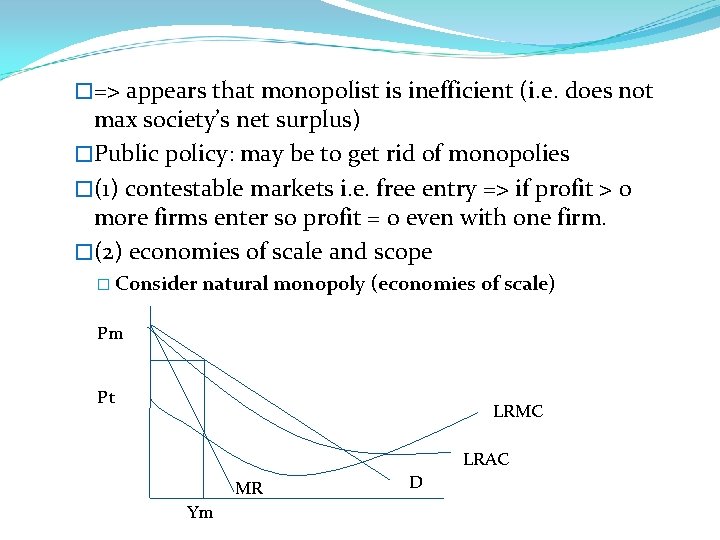
�=> appears that monopolist is inefficient (i. e. does not max society’s net surplus) �Public policy: may be to get rid of monopolies �(1) contestable markets i. e. free entry => if profit > 0 more firms enter so profit = o even with one firm. �(2) economies of scale and scope � Consider natural monopoly (economies of scale) Pm Pt LRMC LRAC MR Ym D

�Only one firm can cheaply produce given demand but � (1) if p=mc=Pso(socially optimum price) � Firms makes a loss and leaves � (2) if p=pm => deadweight loss � (3) if p=AC=pf (fair price) still a loss in profit but firm can operate � But if break up of monopoly: � Pc > Pm > Pf >Pso => competition is not more efficient due to economies of scale. � Same may to be true due to economies of scope.

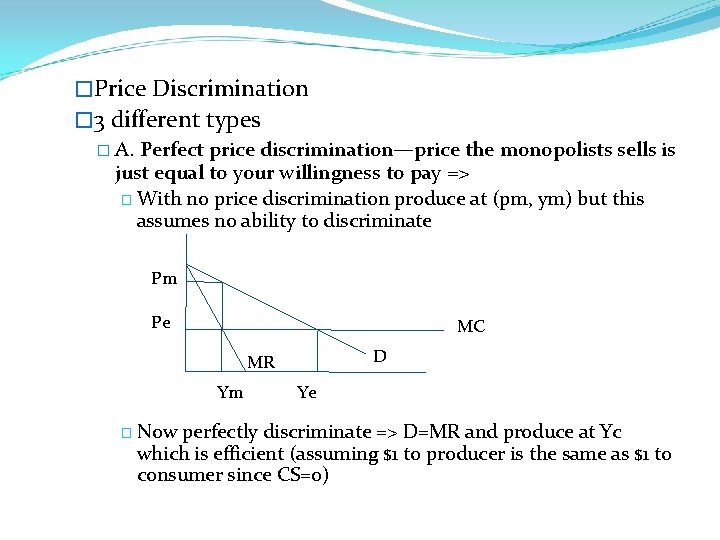
�Price Discrimination � 3 different types � A. Perfect price discrimination—price the monopolists sells is just equal to your willingness to pay => � With no price discrimination produce at (pm, ym) but this assumes no ability to discriminate Pm Pe MC D MR Ym � Now Ye perfectly discriminate => D=MR and produce at Yc which is efficient (assuming $1 to producer is the same as $1 to consumer since CS=0)

� 2 nd degree Price Discrimination � Pi= f(yi) i. e. how much you pay depends on your consumption � Examples: utilities, bulk discounts for large purchases � 3 rd degree price discrimination-different groups get different prices but individuals within a group get the same price �Most common type: Examples � 1. movie theatre discounts (kids v. adults) � 2. local ski discounts (locals v. non-locals) � More formally suppose 2 groups with different demand � => max P 1 (Y 1) Y 1 + P 2 (Y 2) Y 2 – C(Y 1 + Y 2) by: � MR 1 – MC(Y 1 + Y 2) = 0 � MR 2 – MC(Y 1 + Y 2) = 0

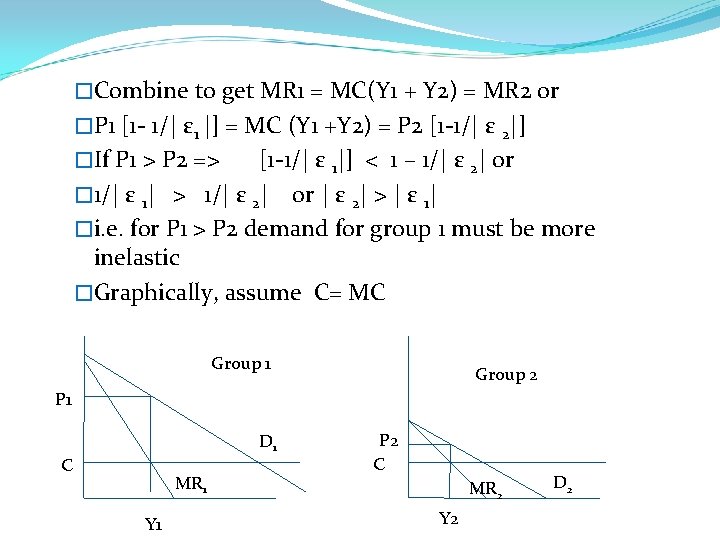
�Combine to get MR 1 = MC(Y 1 + Y 2) = MR 2 or �P 1 [1 - 1/| ε 1 |] = MC (Y 1 +Y 2) = P 2 [1 -1/| ε 2|] �If P 1 > P 2 => [1 -1/| ε 1|] < 1 – 1/| ε 2| or � 1/| ε 1| > 1/| ε 2| or | ε 2| > | ε 1| �i. e. for P 1 > P 2 demand for group 1 must be more inelastic �Graphically, assume C= MC Group 1 Group 2 P 1 D 1 C MR 1 Y 1 P 2 C MR 2 Y 2 D 2

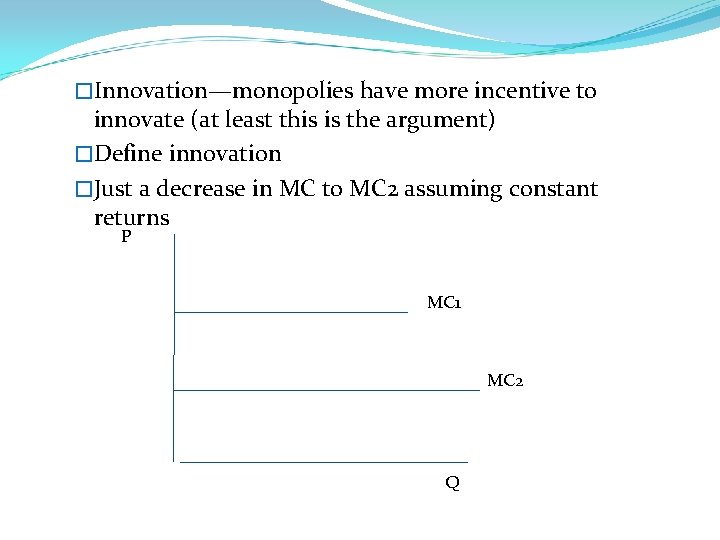
�Innovation—monopolies have more incentive to innovate (at least this is the argument) �Define innovation �Just a decrease in MC to MC 2 assuming constant returns P MC 1 MC 2 Q

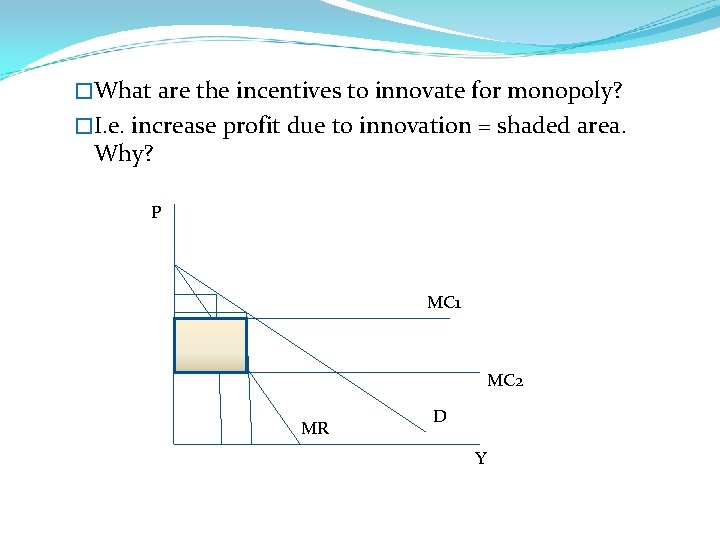
�What are the incentives to innovate for monopoly? �I. e. increase profit due to innovation = shaded area. Why? P MC 1 MC 2 MR D Y

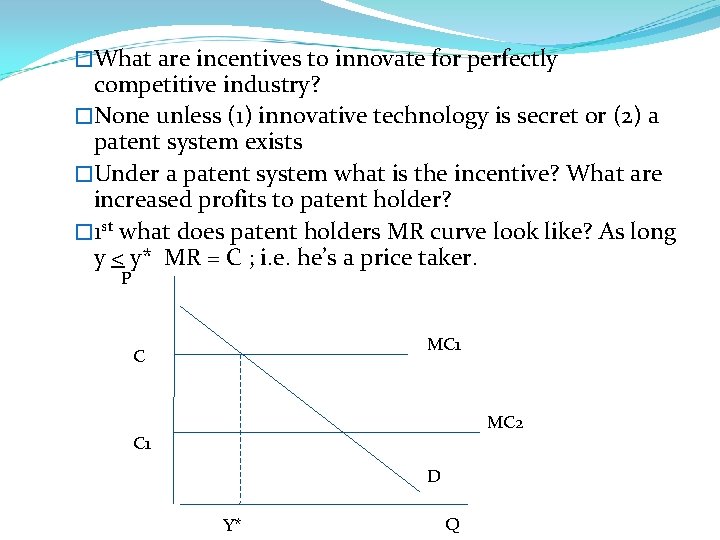
�What are incentives to innovate for perfectly competitive industry? �None unless (1) innovative technology is secret or (2) a patent system exists �Under a patent system what is the incentive? What are increased profits to patent holder? � 1 st what does patent holders MR curve look like? As long y < y* MR = C ; i. e. he’s a price taker. P MC 1 C MC 2 C 1 D Y* Q

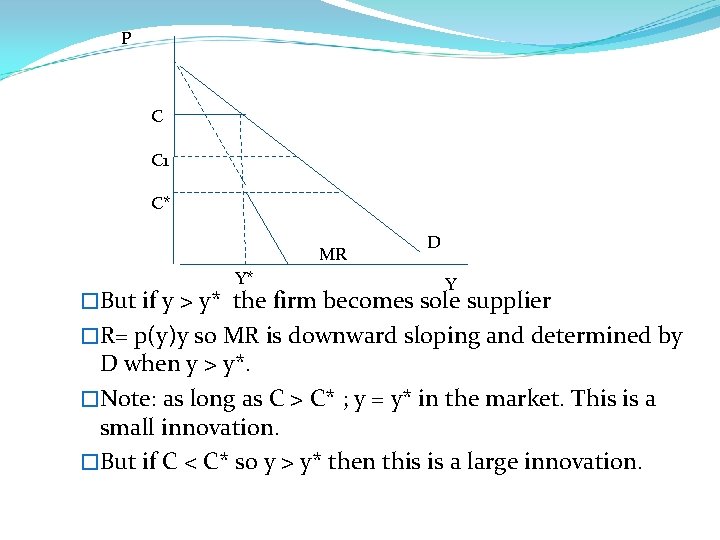
P C C 1 C* MR Y* D Y �But if y > y* the firm becomes sole supplier �R= p(y)y so MR is downward sloping and determined by D when y > y*. �Note: as long as C > C* ; y = y* in the market. This is a small innovation. �But if C < C* so y > y* then this is a large innovation.

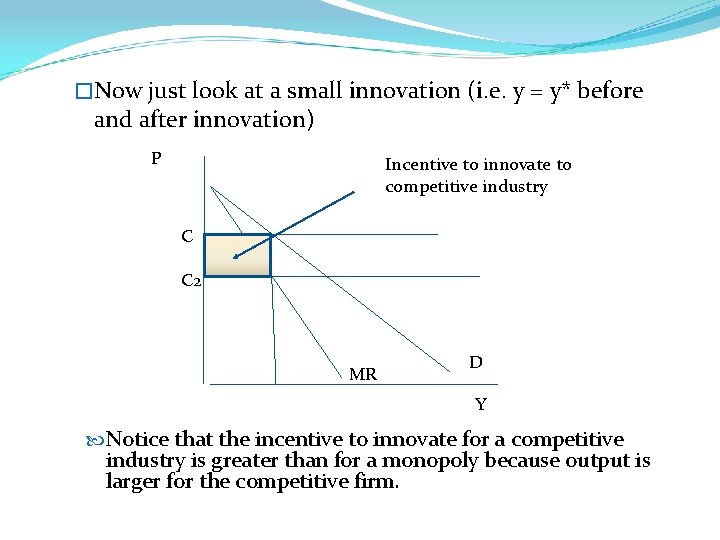
�Now just look at a small innovation (i. e. y = y* before and after innovation) P Incentive to innovate to competitive industry C C 2 MR D Y Notice that the incentive to innovate for a competitive industry is greater than for a monopoly because output is larger for the competitive firm.

�Q: What if economies of scale in innovation (i. e. small firms in competitive industry don’t have resources to innovate) �A: Firms specialize in innovating, gain patents and license to small competitive firms � Example: agriculture where innovating is done by � Universities � Seed companies � Etc.

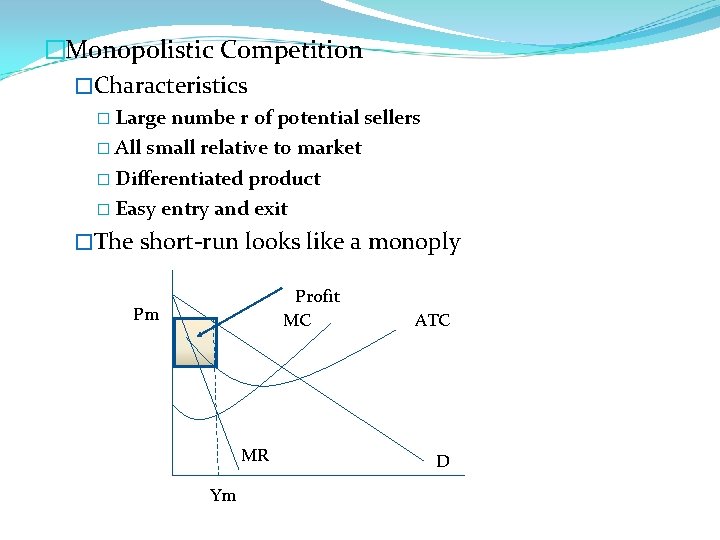
�Monopolistic Competition �Characteristics � Large numbe r of potential sellers � All small relative to market � Differentiated product � Easy entry and exit �The short-run looks like a monoply Profit MC Pm MR Ym ATC D

�Profit can also be negative or zero in the short-run. If negative => firms exit if p< avc. �Long-run equilibrium is just like for competition: �If profit > 0 => entry which drives profit down. �If profit < 0 => exit which drives profit up. �Therefore, long-run equilibrium is where profit equals zero, where no exit or entry. MC ATC Po Pc MR Qo Qc D

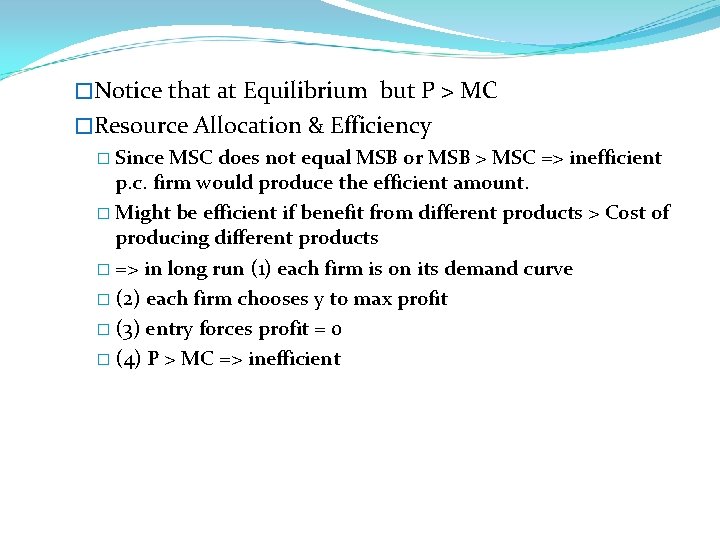
�Notice that at Equilibrium but P > MC �Resource Allocation & Efficiency � Since MSC does not equal MSB or MSB > MSC => inefficient p. c. firm would produce the efficient amount. � Might be efficient if benefit from different products > Cost of producing different products � => in long run (1) each firm is on its demand curve � (2) each firm chooses y to max profit � (3) entry forces profit = 0 � (4) P > MC => inefficient
 Intermediate microeconomics lecture notes
Intermediate microeconomics lecture notes Intermediate microeconomics notes
Intermediate microeconomics notes 01:640:244 lecture notes - lecture 15: plat, idah, farad
01:640:244 lecture notes - lecture 15: plat, idah, farad Gradiente eco
Gradiente eco Market leader market challenger market follower
Market leader market challenger market follower Market segmentation target market selection and positioning
Market segmentation target market selection and positioning Difference between monopolistic competition and oligopoly
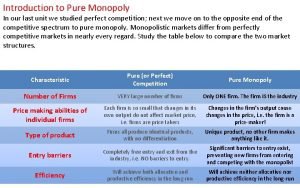
Difference between monopolistic competition and oligopoly Difference between perfect competition and monopoly
Difference between perfect competition and monopoly Difference between perfect competition and monopoly
Difference between perfect competition and monopoly Example of monopoly market
Example of monopoly market Monopoly maximize profit
Monopoly maximize profit Monopoly market examples
Monopoly market examples Characteristics of a monopoly
Characteristics of a monopoly Monopoly economics
Monopoly economics Features of monopoly market
Features of monopoly market Monopoly market
Monopoly market Migration whiz
Migration whiz Project procurement management lecture notes
Project procurement management lecture notes Theology proper lecture notes
Theology proper lecture notes Public sector accounting lecture notes
Public sector accounting lecture notes 4 p's of project management in software engineering
4 p's of project management in software engineering Electricity and magnetism lecture notes
Electricity and magnetism lecture notes Physics 111 lecture notes
Physics 111 lecture notes Physical science lecture notes
Physical science lecture notes Power system dynamics and stability lecture notes
Power system dynamics and stability lecture notes