Chapter 5 Applications of Integration Areas Volumes Work






- Slides: 27

Chapter 5. Applications of Integration Areas, Volumes, Work Sections 5. 1, 5. 2, 5. 3, 5. 4

5. 1 Area between curves

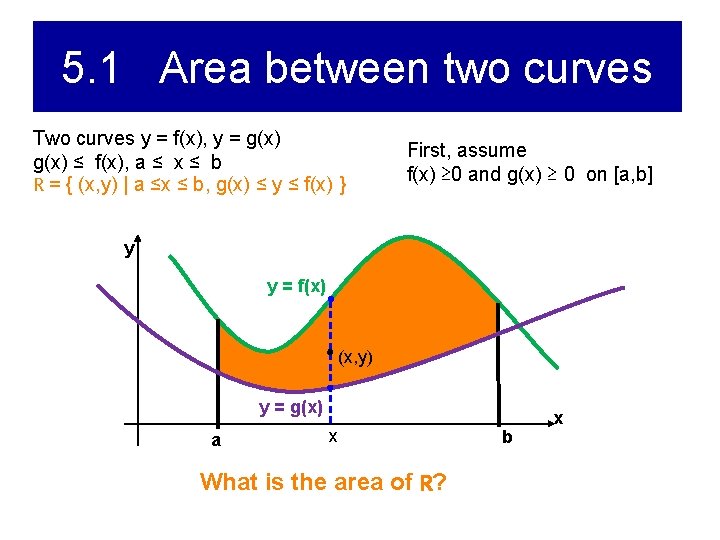
5. 1 Area between two curves Two curves y = f(x), y = g(x) ≤ f(x), a ≤ x ≤ b R = { (x, y) | a ≤x ≤ b, g(x) ≤ y ≤ f(x) } First, assume f(x) ≥ 0 and g(x) ≥ 0 on [a, b] y y = f(x) (x, y) y = g(x) a x What is the area of R? b x

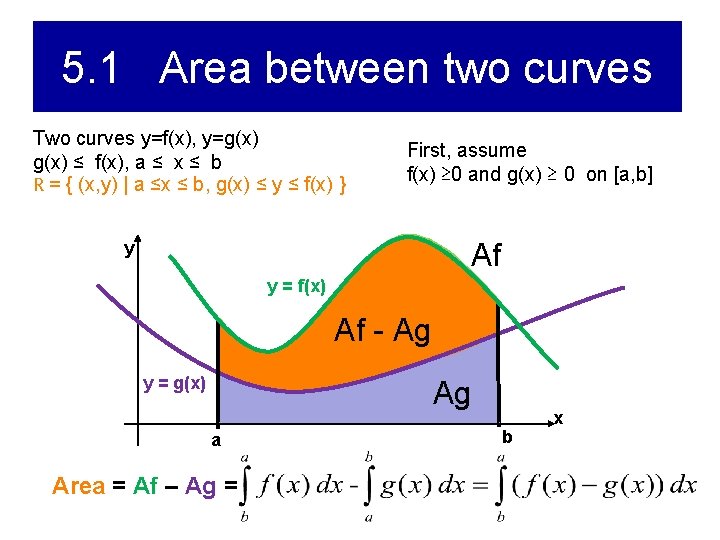
5. 1 Area between two curves Two curves y=f(x), y=g(x) ≤ f(x), a ≤ x ≤ b R = { (x, y) | a ≤x ≤ b, g(x) ≤ y ≤ f(x) } First, assume f(x) ≥ 0 and g(x) ≥ 0 on [a, b] Af y y = f(x) Af - Ag y = g(x) a Area = Af – Ag = Ag b x

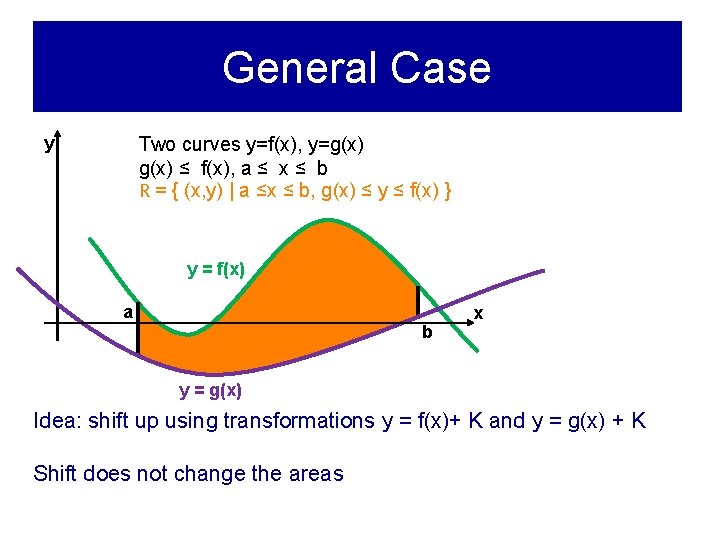
General Case y Two curves y=f(x), y=g(x) ≤ f(x), a ≤ x ≤ b R = { (x, y) | a ≤x ≤ b, g(x) ≤ y ≤ f(x) } y = f(x) a b x y = g(x) Idea: shift up using transformations y = f(x)+ K and y = g(x) + K Shift does not change the areas

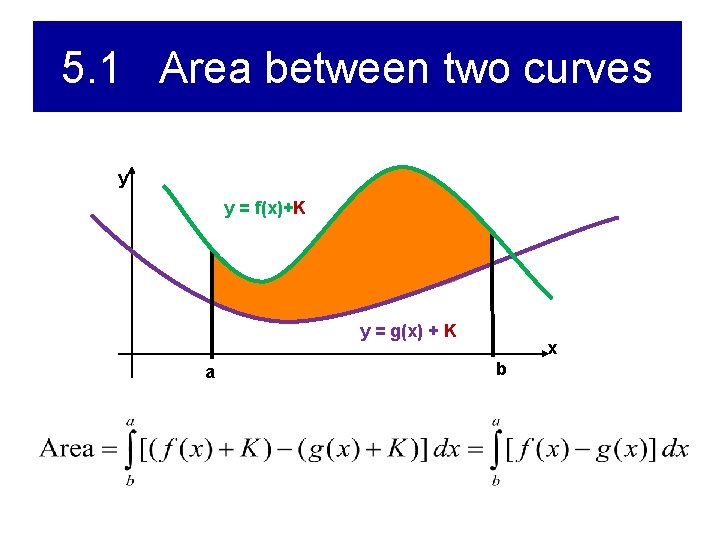
5. 1 Area between two curves y y = f(x)+K y = g(x) + K a x b

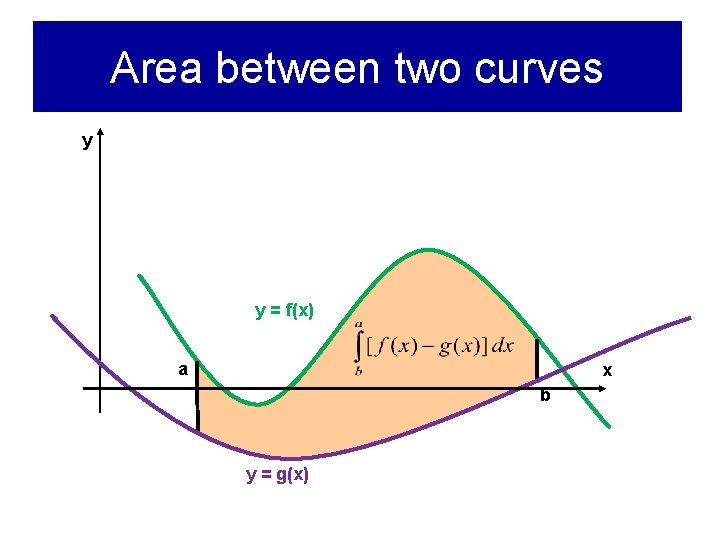
Area between two curves y y = f(x) a x b y = g(x)

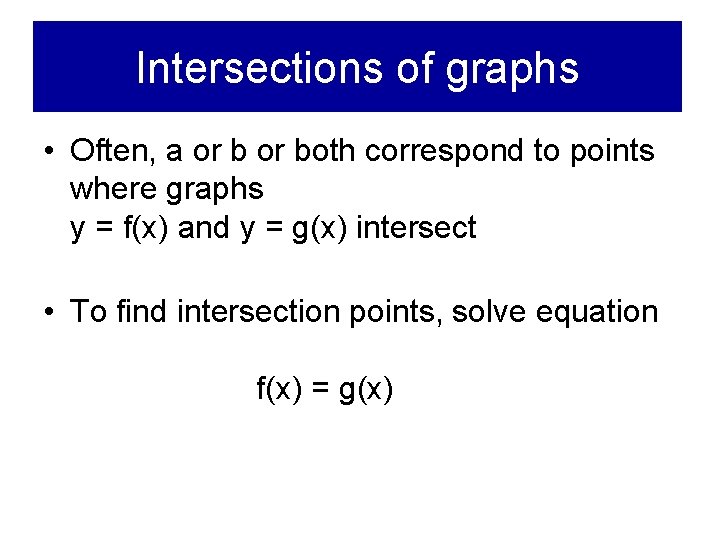
Intersections of graphs • Often, a or both correspond to points where graphs y = f(x) and y = g(x) intersect • To find intersection points, solve equation f(x) = g(x)

5. 2 Volumes

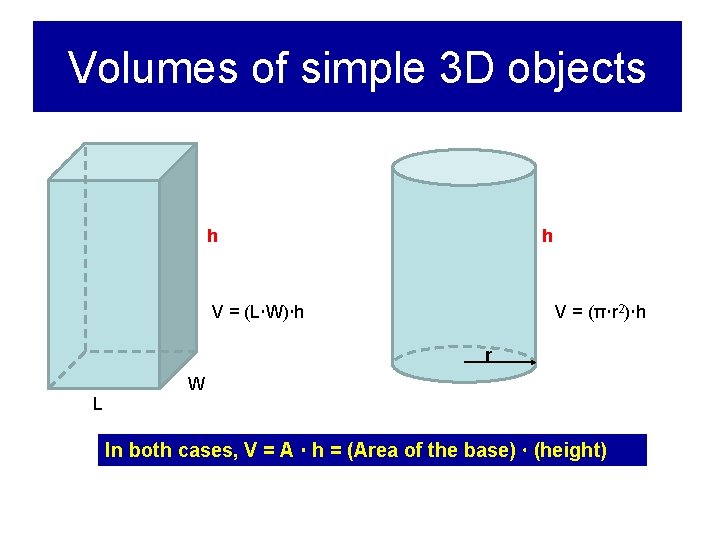
Volumes of simple 3 D objects h h V = (L W) h V = (π r 2) h r L W In both cases, V = A h = (Area of the base) (height)

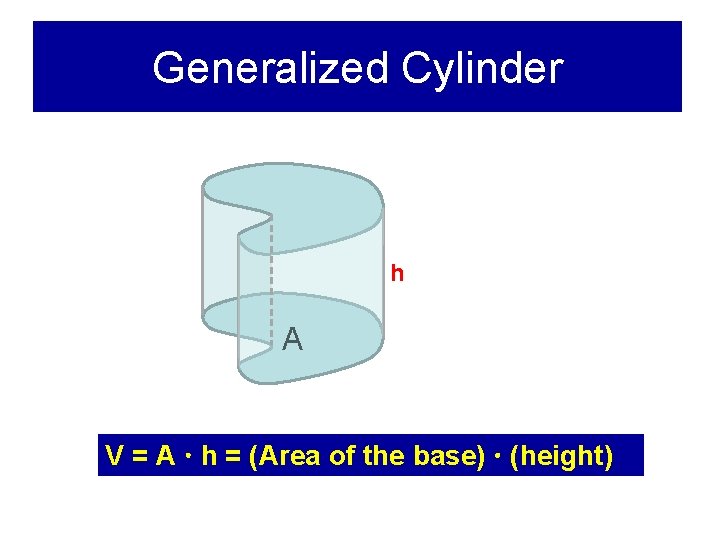
Generalized Cylinder h A V = A h = (Area of the base) (height)

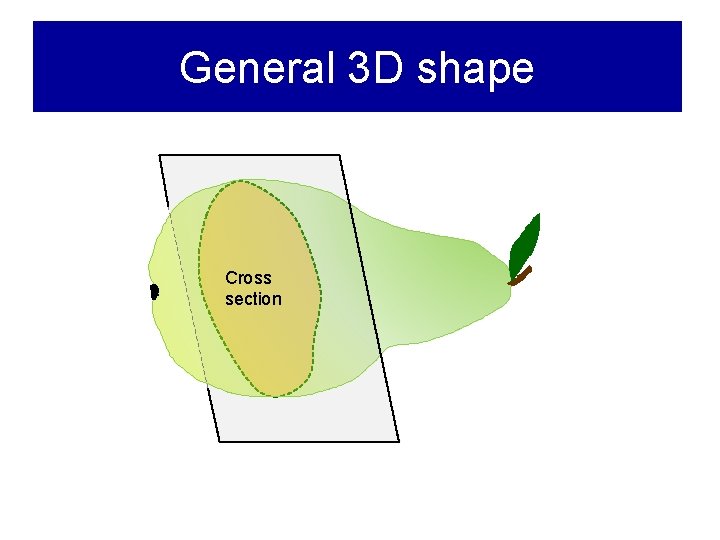
General 3 D shape Cross section

Area of a cross section A(x) x x

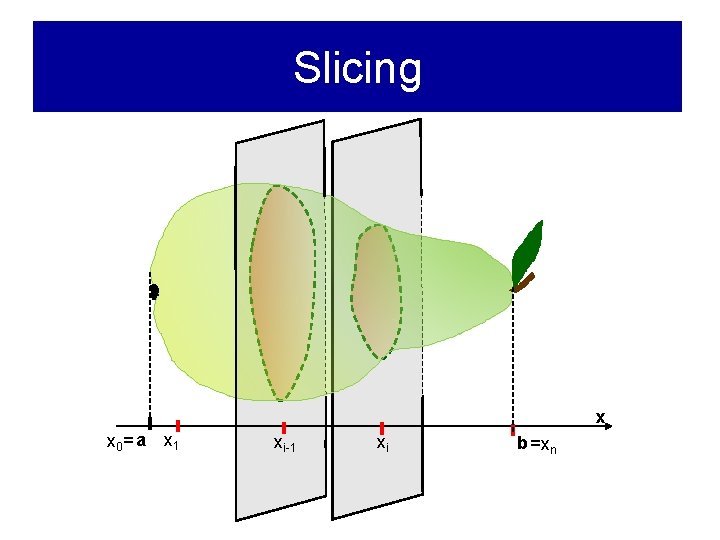
Slicing x x 0= a x 1 xi-1 xi b =xn

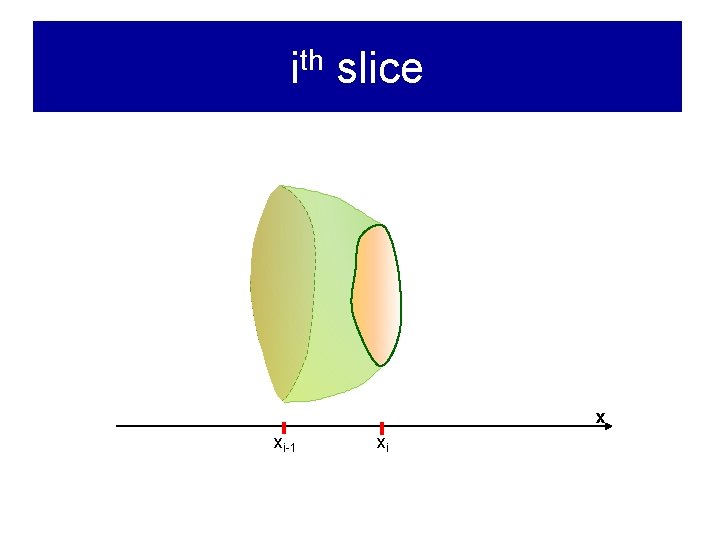
ith slice x xi-1 xi

ith slice – approximation by cylinder x xi-1 xi

ith slice – approximation by cylinder V(ith slice) ≈ V(ith cylinder) = Area of the base height = A(xi) Δx Vi A(xi) Vi ≈ A(xi) Δx x xi-1 Δx xi

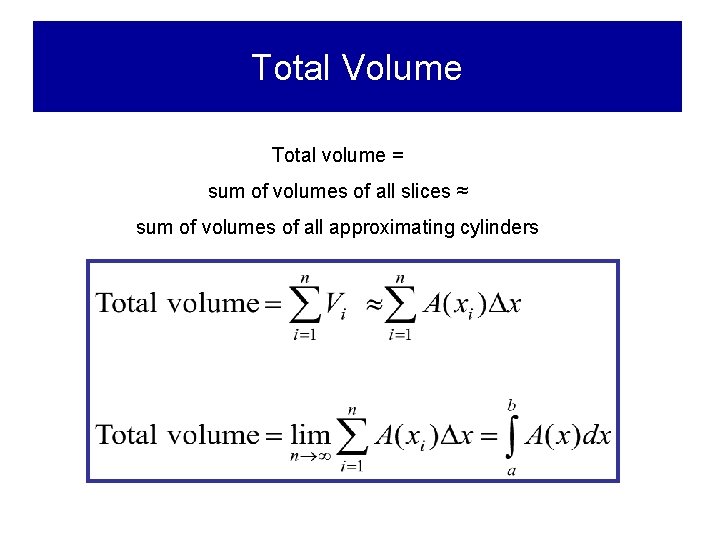
Total Volume Total volume = sum of volumes of all slices ≈ sum of volumes of all approximating cylinders

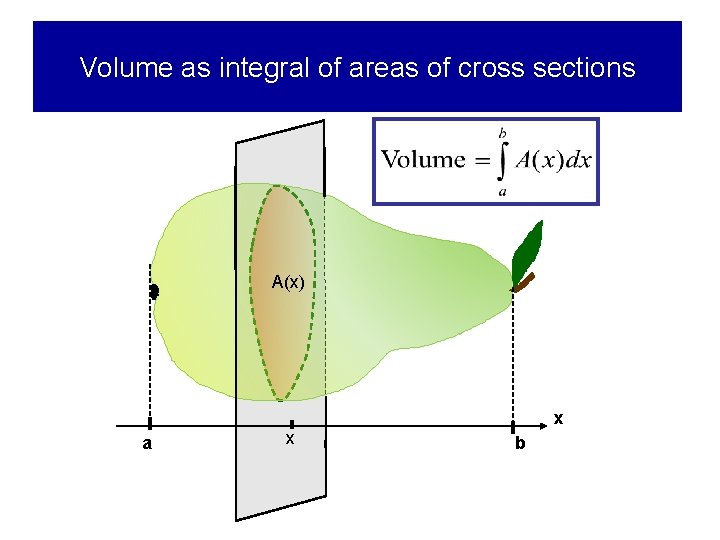
Volume as integral of areas of cross sections A(x) x a x b

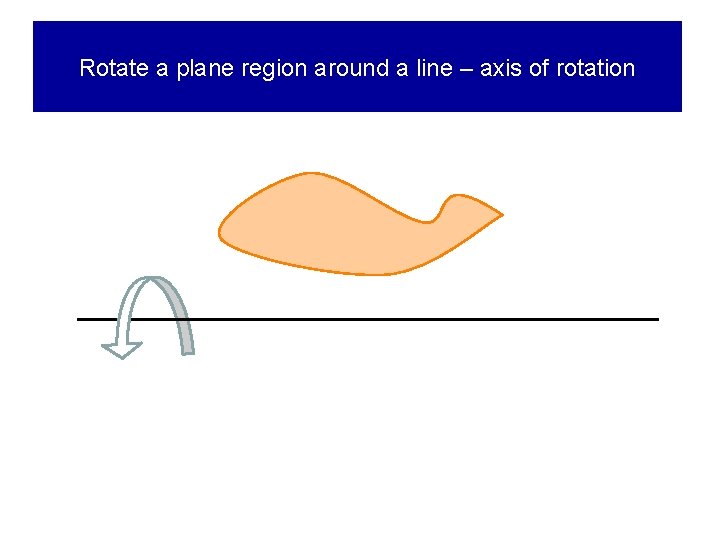
Solids of revolution

Rotate a plane region around a line – axis of rotation

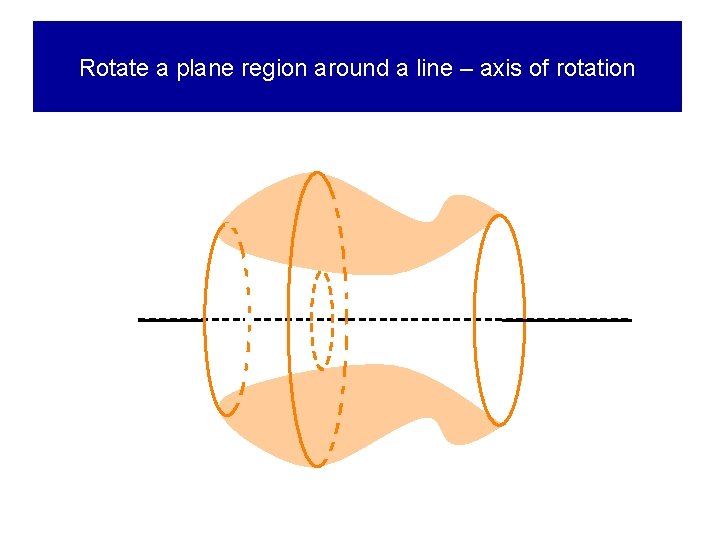
Rotate a plane region around a line – axis of rotation

Volumes of solids of revolution using “washers”

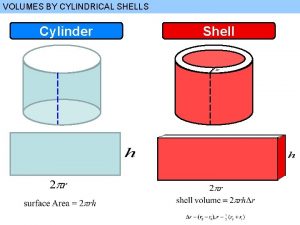
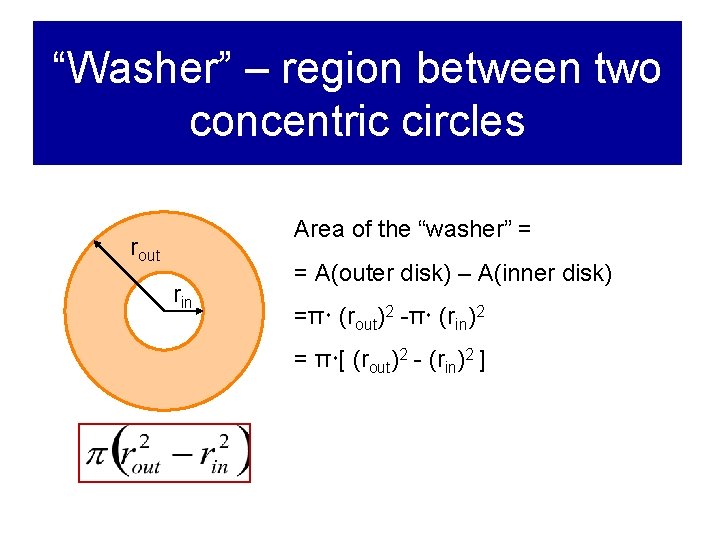
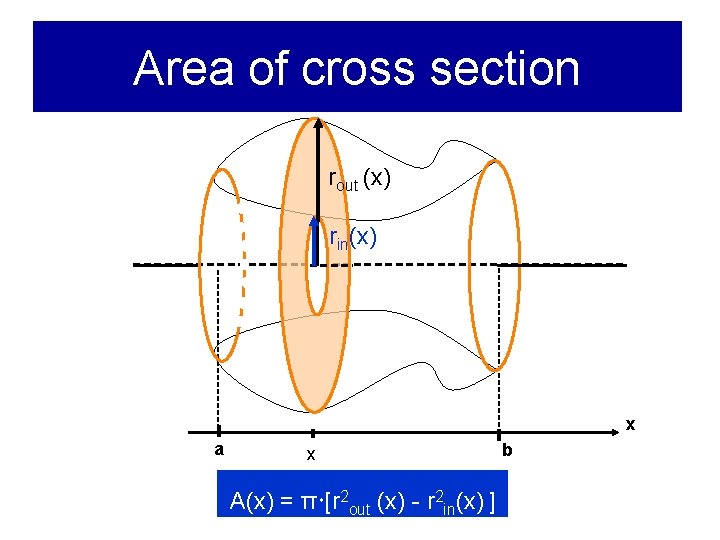
“Washer” – region between two concentric circles Area of the “washer” = rout rin = A(outer disk) – A(inner disk) =π (rout)2 -π (rin)2 = π [ (rout)2 - (rin)2 ]

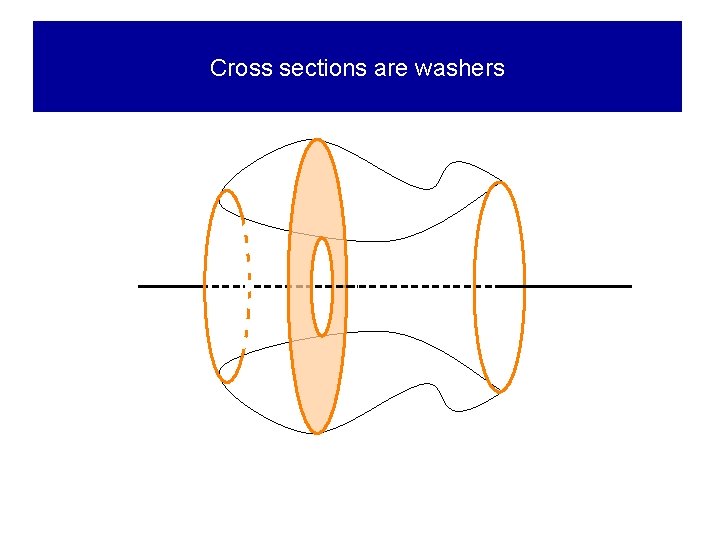
Cross sections are washers

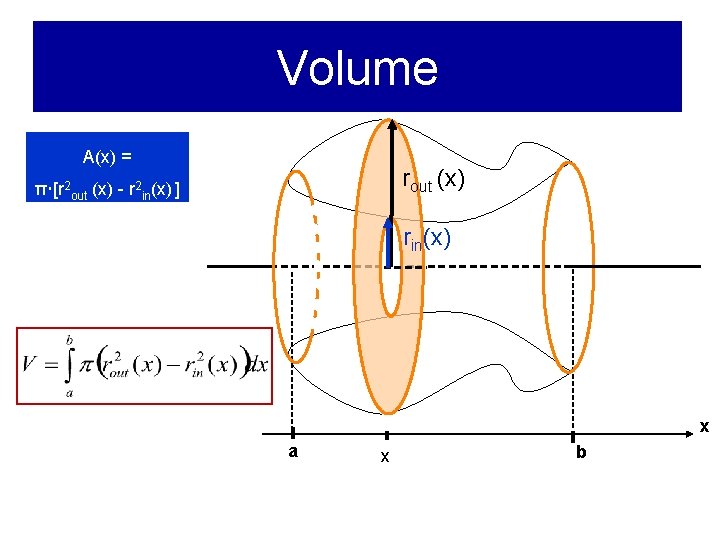
Area of cross section rout (x) rin(x) x a x A(x) = π [r 2 out (x) - r 2 in(x) ] b

Volume A(x) = rout (x) π [r 2 out (x) - r 2 in(x) ] rin(x) x a x b
 Unit 6 lesson 6 surface areas and volumes of spheres
Unit 6 lesson 6 surface areas and volumes of spheres Cone volume ratio
Cone volume ratio Further applications of integration
Further applications of integration Further applications of integration
Further applications of integration Applications of integration volume
Applications of integration volume Further applications of integration
Further applications of integration Further applications of integration
Further applications of integration Further applications of integration
Further applications of integration Further applications of integration
Further applications of integration Forward integration and backward integration
Forward integration and backward integration Vertical diversification example
Vertical diversification example What is simultaneous integration
What is simultaneous integration Chapter 4 section 1 work and machines answer key
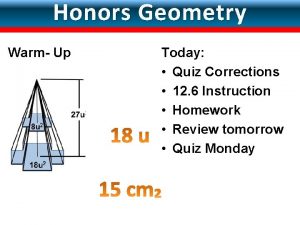
Chapter 4 section 1 work and machines answer key Practice 10-6 volumes of pyramids and cones answers
Practice 10-6 volumes of pyramids and cones answers Espirometro jaeger
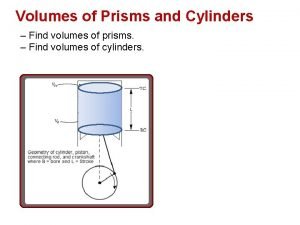
Espirometro jaeger 12-4 volumes of prisms and cylinders
12-4 volumes of prisms and cylinders Volume of pyramids and cones calculator
Volume of pyramids and cones calculator What is the volume of this prism
What is the volume of this prism Volume of solid with known cross section
Volume of solid with known cross section Hein
Hein Pediatric iv medication administration guidelines
Pediatric iv medication administration guidelines Law of combining volumes
Law of combining volumes Lesson 12-5 volumes of pyramids and cones
Lesson 12-5 volumes of pyramids and cones Ifr altitudes
Ifr altitudes Hpe cloud volumes block
Hpe cloud volumes block Law of combining volumes
Law of combining volumes Law of combining volumes
Law of combining volumes Example of law of definite proportion
Example of law of definite proportion