11 2 Zeros of Polynomial Functions Zeros of

§ 11. 2 Zeros of Polynomial Functions

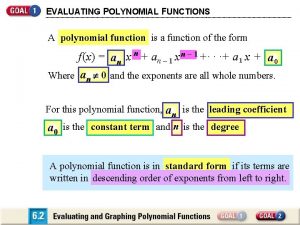
Zeros of Polynomial Functions We used a shortcut called “Synthetic Division” for long division in Chapter 6 to divide a polynomial by a binomial of the form x - c. We will use synthetic division now and a result known as the “Factor Theorem” in solving a polynomial equation. We will first review the rules in synthetic division and then will look at the Factor Theorem. Blitzer, Algebra for College Students, 6 e – Slide #2 Section 11. 2

Synthetic Division of Polynomials The method of synthetic division is just a shortcut for long division. With this process -we can save both steps and paper by writing down only what is necessary from the long division problem and by also compacting the form. Synthetic division is quick and can be extremely useful. Note that this process works only when you can express the divisor in the form x – c. Blitzer, Algebra for College Students, 6 e – Slide #3 Section 11. 2

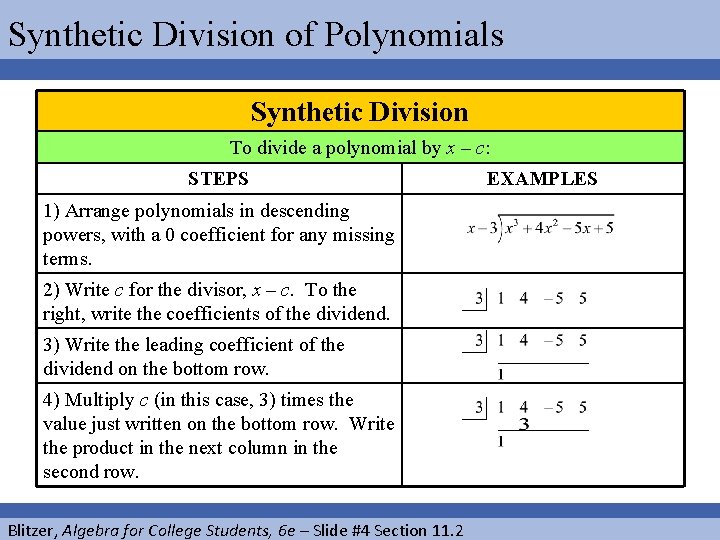
Synthetic Division of Polynomials Synthetic Division To divide a polynomial by x – c: STEPS 1) Arrange polynomials in descending powers, with a 0 coefficient for any missing terms. 2) Write c for the divisor, x – c. To the right, write the coefficients of the dividend. 3) Write the leading coefficient of the dividend on the bottom row. 4) Multiply c (in this case, 3) times the value just written on the bottom row. Write the product in the next column in the second row. Blitzer, Algebra for College Students, 6 e – Slide #4 Section 11. 2 EXAMPLES

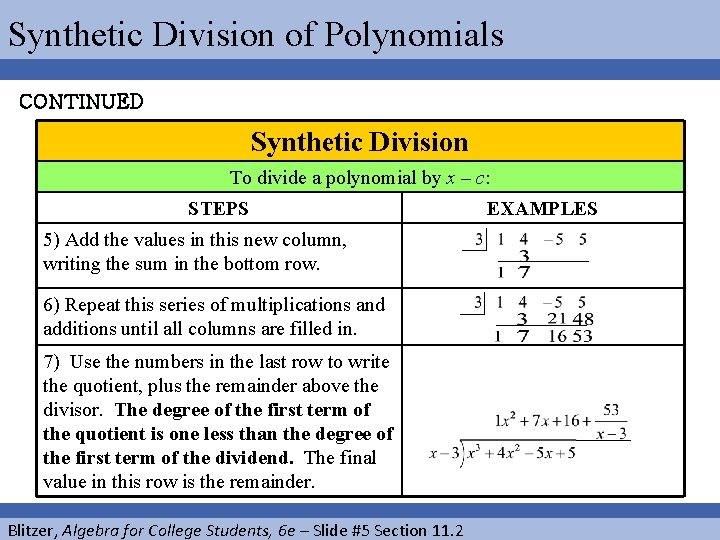
Synthetic Division of Polynomials CONTINUED Synthetic Division To divide a polynomial by x – c: STEPS 5) Add the values in this new column, writing the sum in the bottom row. 6) Repeat this series of multiplications and additions until all columns are filled in. 7) Use the numbers in the last row to write the quotient, plus the remainder above the divisor. The degree of the first term of the quotient is one less than the degree of the first term of the dividend. The final value in this row is the remainder. Blitzer, Algebra for College Students, 6 e – Slide #5 Section 11. 2 EXAMPLES

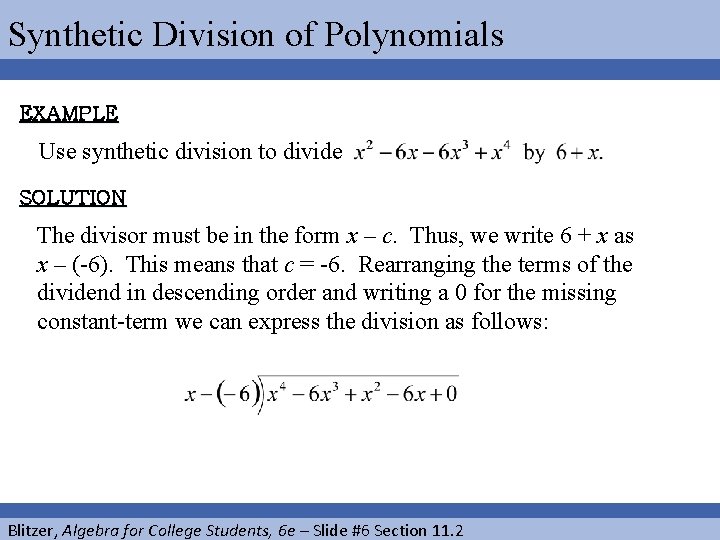
Synthetic Division of Polynomials EXAMPLE Use synthetic division to divide SOLUTION The divisor must be in the form x – c. Thus, we write 6 + x as x – (-6). This means that c = -6. Rearranging the terms of the dividend in descending order and writing a 0 for the missing constant-term we can express the division as follows: Blitzer, Algebra for College Students, 6 e – Slide #6 Section 11. 2

Synthetic Division of Polynomials CONTINUED Now we are ready to set up the problem so that we can use synthetic division. Use the coefficients of the dividend This is c in x – (-6). in descending powers of x. We begin the synthetic division process by bringing down 1. This is followed by a series of multiplications and additions. 1) Bring down 1. 2) Multiply: -6(1) = -6. Blitzer, Algebra for College Students, 6 e – Slide #7 Section 11. 2

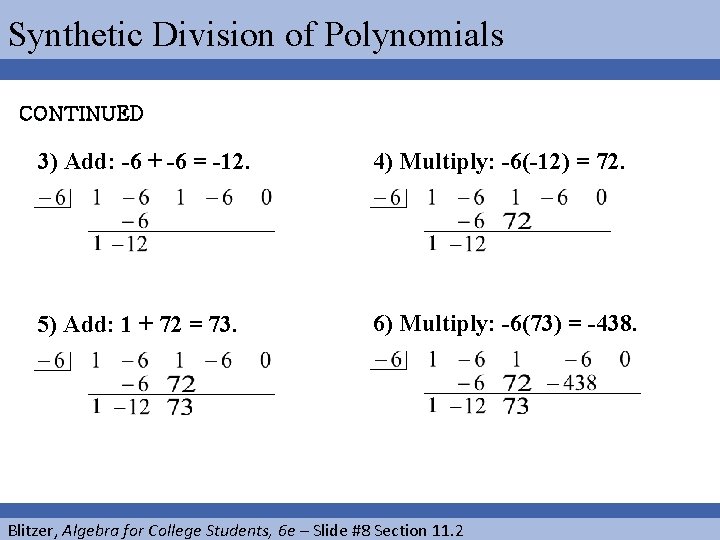
Synthetic Division of Polynomials CONTINUED 3) Add: -6 + -6 = -12. 4) Multiply: -6(-12) = 72. 5) Add: 1 + 72 = 73. 6) Multiply: -6(73) = -438. Blitzer, Algebra for College Students, 6 e – Slide #8 Section 11. 2

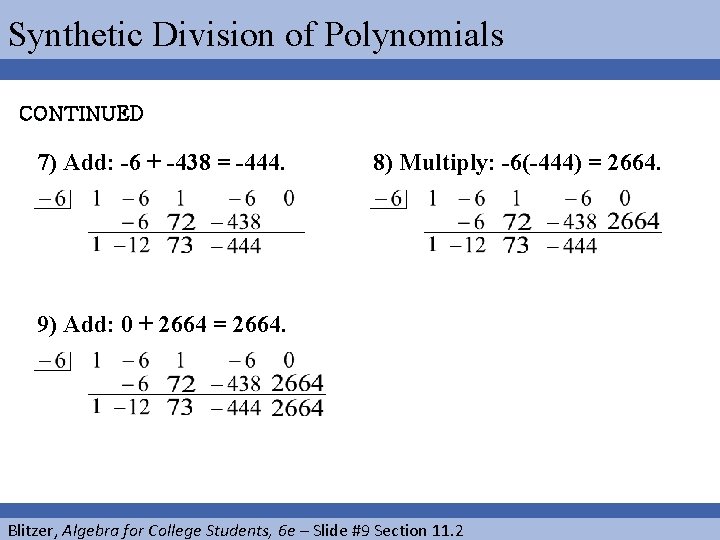
Synthetic Division of Polynomials CONTINUED 7) Add: -6 + -438 = -444. 8) Multiply: -6(-444) = 2664. 9) Add: 0 + 2664 = 2664. Blitzer, Algebra for College Students, 6 e – Slide #9 Section 11. 2

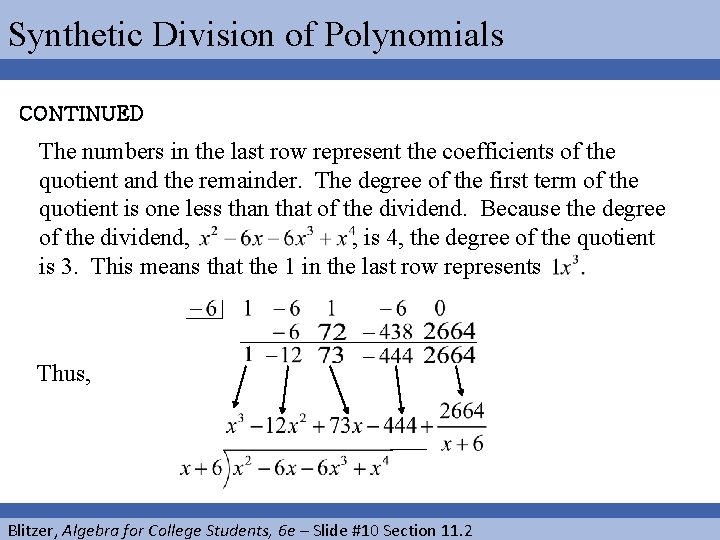
Synthetic Division of Polynomials CONTINUED The numbers in the last row represent the coefficients of the quotient and the remainder. The degree of the first term of the quotient is one less than that of the dividend. Because the degree of the dividend, , is 4, the degree of the quotient is 3. This means that the 1 in the last row represents Thus, Blitzer, Algebra for College Students, 6 e – Slide #10 Section 11. 2

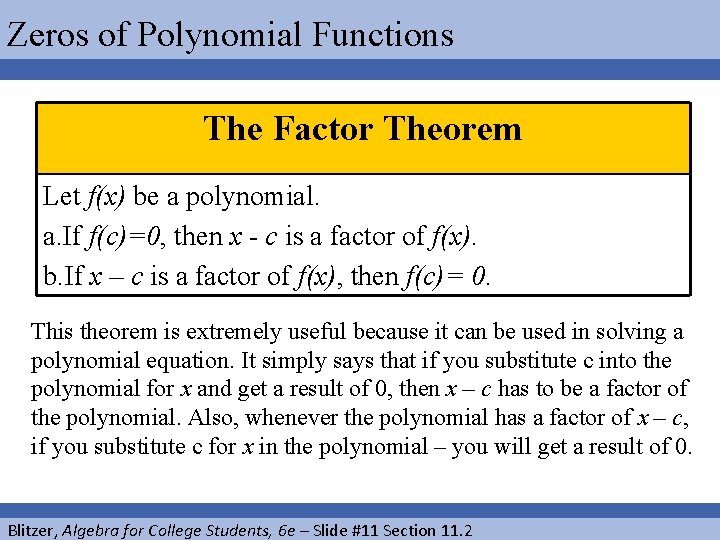
Zeros of Polynomial Functions The Factor Theorem Let f(x) be a polynomial. a. If f(c)=0, then x - c is a factor of f(x). b. If x – c is a factor of f(x), then f(c)= 0. This theorem is extremely useful because it can be used in solving a polynomial equation. It simply says that if you substitute c into the polynomial for x and get a result of 0, then x – c has to be a factor of the polynomial. Also, whenever the polynomial has a factor of x – c, if you substitute c for x in the polynomial – you will get a result of 0. Blitzer, Algebra for College Students, 6 e – Slide #11 Section 11. 2

Using the Factor Theorem EXAMPLE Solve the equation of the polynomial. given that -1 is a zero SOLUTION Since -1 is a zero of the polynomial, this means that x + 1 is a factor. We’ll use synthetic division to divide f(x) by x + 1. Proposed Solution Remainder Blitzer, Algebra for College Students, 6 e – Slide #12 Section 11. 2

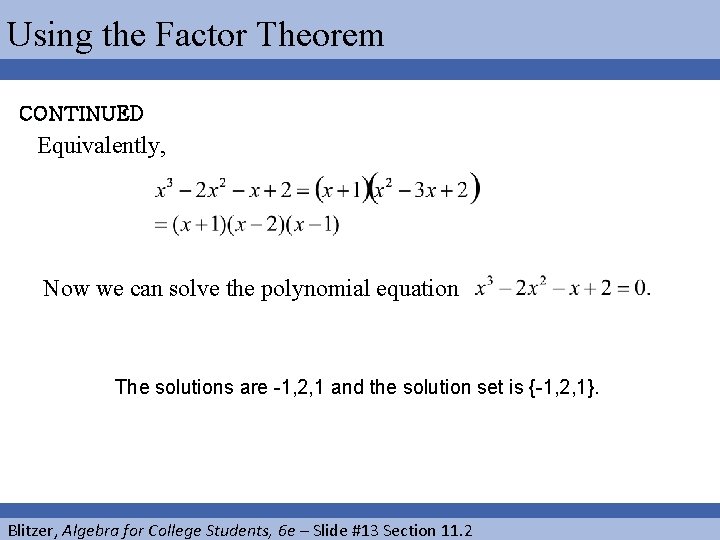
Using the Factor Theorem CONTINUED Equivalently, Now we can solve the polynomial equation The solutions are -1, 2, 1 and the solution set is {-1, 2, 1}. Blitzer, Algebra for College Students, 6 e – Slide #13 Section 11. 2

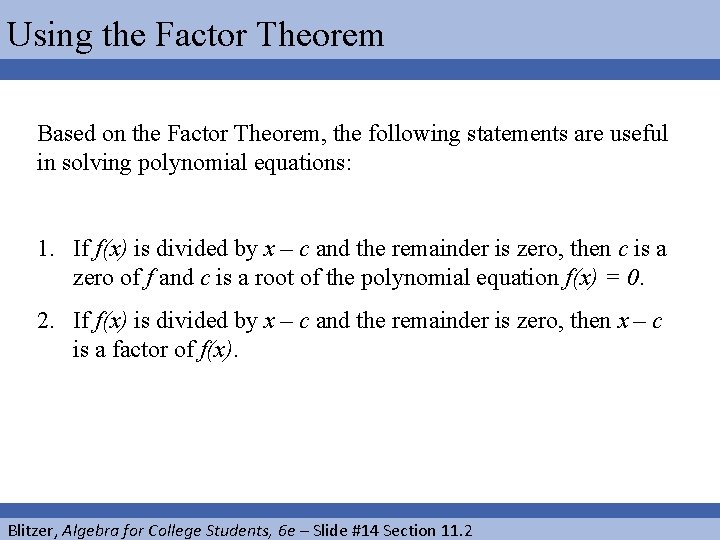
Using the Factor Theorem Based on the Factor Theorem, the following statements are useful in solving polynomial equations: 1. If f(x) is divided by x – c and the remainder is zero, then c is a zero of f and c is a root of the polynomial equation f(x) = 0. 2. If f(x) is divided by x – c and the remainder is zero, then x – c is a factor of f(x). Blitzer, Algebra for College Students, 6 e – Slide #14 Section 11. 2

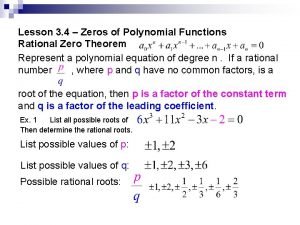
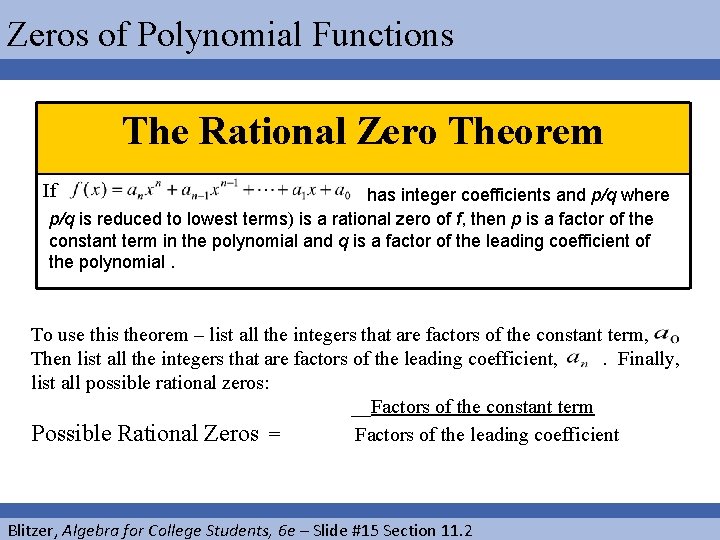
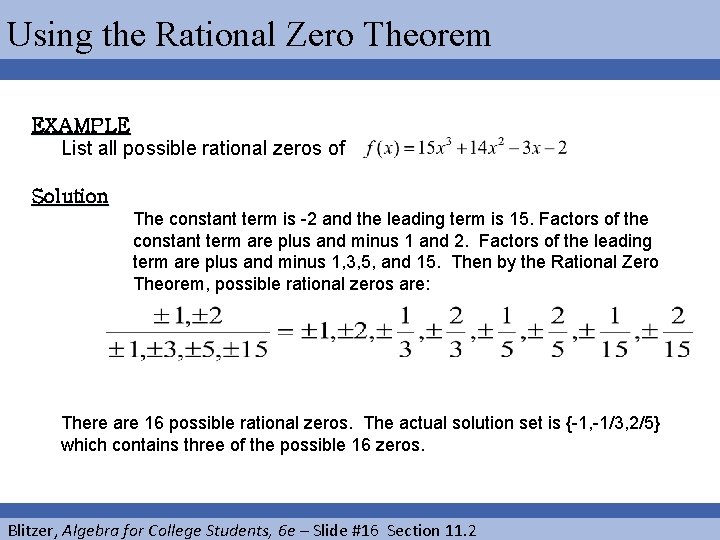
Zeros of Polynomial Functions The Rational Zero Theorem If has integer coefficients and p/q where p/q is reduced to lowest terms) is a rational zero of f, then p is a factor of the constant term in the polynomial and q is a factor of the leading coefficient of the polynomial. To use this theorem – list all the integers that are factors of the constant term, Then list all the integers that are factors of the leading coefficient, . Finally, list all possible rational zeros: __Factors of the constant term Possible Rational Zeros = Factors of the leading coefficient Blitzer, Algebra for College Students, 6 e – Slide #15 Section 11. 2

Using the Rational Zero Theorem EXAMPLE List all possible rational zeros of Solution The constant term is -2 and the leading term is 15. Factors of the constant term are plus and minus 1 and 2. Factors of the leading term are plus and minus 1, 3, 5, and 15. Then by the Rational Zero Theorem, possible rational zeros are: There are 16 possible rational zeros. The actual solution set is {-1, -1/3, 2/5} which contains three of the possible 16 zeros. Blitzer, Algebra for College Students, 6 e – Slide #16 Section 11. 2

Zeros of Polynomial Functions Properties of Polynomial Equations 1. If a polynomial equation is of degree n, then counting multiple roots separately, the equation has n roots. 2. If a + bi is a root of a polynomial equation (b is not 0), then the imaginary number a – bi is also a root. Imaginary roots, if they exist, occur in conjugate pairs. Blitzer, Algebra for College Students, 6 e – Slide #17 Section 11. 2

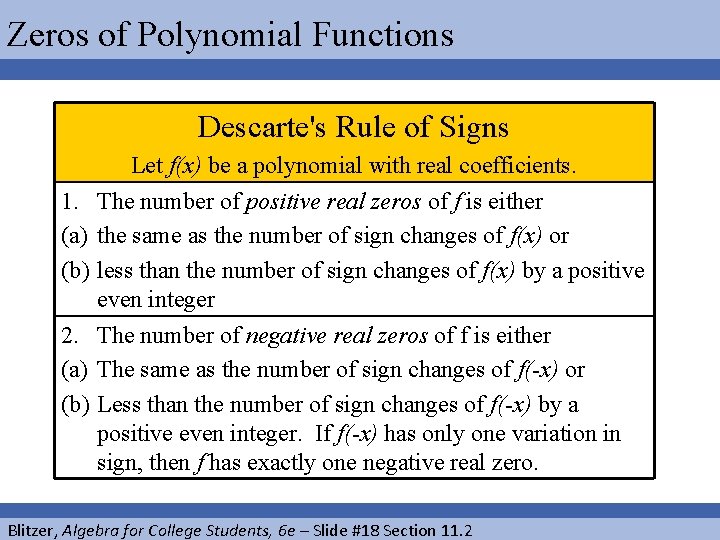
Zeros of Polynomial Functions Descarte's Rule of Signs Let f(x) be a polynomial with real coefficients. 1. The number of positive real zeros of f is either (a) the same as the number of sign changes of f(x) or (b) less than the number of sign changes of f(x) by a positive even integer 2. The number of negative real zeros of f is either (a) The same as the number of sign changes of f(-x) or (b) Less than the number of sign changes of f(-x) by a positive even integer. If f(-x) has only one variation in sign, then f has exactly one negative real zero. Blitzer, Algebra for College Students, 6 e – Slide #18 Section 11. 2

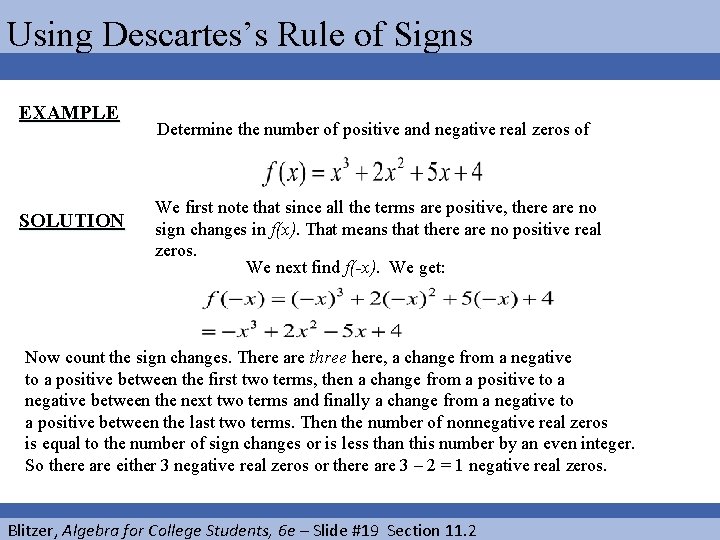
Using Descartes’s Rule of Signs EXAMPLE SOLUTION Determine the number of positive and negative real zeros of We first note that since all the terms are positive, there are no sign changes in f(x). That means that there are no positive real zeros. We next find f(-x). We get: Now count the sign changes. There are three here, a change from a negative to a positive between the first two terms, then a change from a positive to a negative between the next two terms and finally a change from a negative to a positive between the last two terms. Then the number of nonnegative real zeros is equal to the number of sign changes or is less than this number by an even integer. So there are either 3 negative real zeros or there are 3 – 2 = 1 negative real zeros. Blitzer, Algebra for College Students, 6 e – Slide #19 Section 11. 2

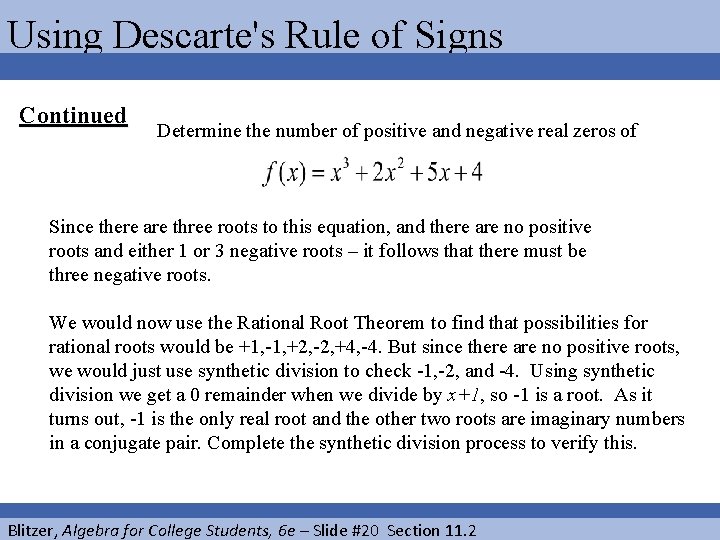
Using Descarte's Rule of Signs Continued Determine the number of positive and negative real zeros of Since there are three roots to this equation, and there are no positive roots and either 1 or 3 negative roots – it follows that there must be three negative roots. We would now use the Rational Root Theorem to find that possibilities for rational roots would be +1, -1, +2, -2, +4, -4. But since there are no positive roots, we would just use synthetic division to check -1, -2, and -4. Using synthetic division we get a 0 remainder when we divide by x+1, so -1 is a root. As it turns out, -1 is the only real root and the other two roots are imaginary numbers in a conjugate pair. Complete the synthetic division process to verify this. Blitzer, Algebra for College Students, 6 e – Slide #20 Section 11. 2