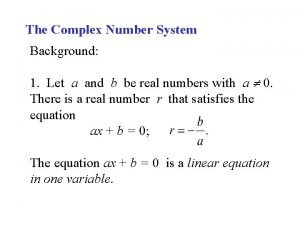
SETS OF AXIOMS AND FINITE GEOMETRIES Compiled Still

SETS OF AXIOMS AND FINITE GEOMETRIES Compiled: Still John F. Reyes

INTRODUCTION • The word “geometry” can be interpreted literally as “earth measure”. • The idea of earth measure arises from the ancient, pre-Greek development of geometry. • Practical Egyptians and Babylonian applications of geometry involved extensive measurement, and they were not complicated by formal proofs.

INTRODUCTION • For example, the properties of the right triangle were known to the extent that a rope with knots, held tightly by three men so as to form a right triangle, could be used in surveying.

INTRODUCTION • During the Greek period, the science of earth measure became more refined. • About 230 B. C. , Eratosthenes made a remarkably precise measurement of the size of the earth. • The earth-measurement aspect of geometry is useful in precise measurements made by satellites, by instruments placed on the moon, and by the U. S. Coast and Geodetic Survey in producing nautical and aeronautical charts.

INTRODUCTION • The ancient Greeks of the period 500 B. C. to 100 A. D. receive much of the credit for the development of demonstrative geometry of the sort studied at the high school level. • Greek geometry, called Euclidean geometry because of the monumental work of Euclid (300 B. C. ), includes undefined terms, axioms or postulates, and theorems.

INTRODUCTION • In Euclidean geometry, undefined terms, which are arbitrary and could easily be replaced by other terms, normally include points, lines, and planes. • Today, the words axiom and postulate are used interchangeably. • Postulate was used for an assumption confined to one particular subject (such as geometry), while axiom denoted a “universal truth, ” a more general assumption that applied to all of mathematics.

INTRODUCTION • The truth of axioms or postulates is not at issue. These statements are beginning assumptions from which logical consequences follow. • They are analogous to the rules for a game. • Theorems are statements to be proved by using the axioms, definitions, and previous theorems as reasons for the logical steps in the proof.

INTRODUCTION • The theorems of geometry are valid conclusions based on the axioms. • Example: “If the sum of the measures of the opposite angles of a quadrilateral is 180 (in degrees), then the quadrilateral can be inscribed in a circle. ” • A deductive proof begins with the hypothesis and leads through a series of logical steps to the conclusion.

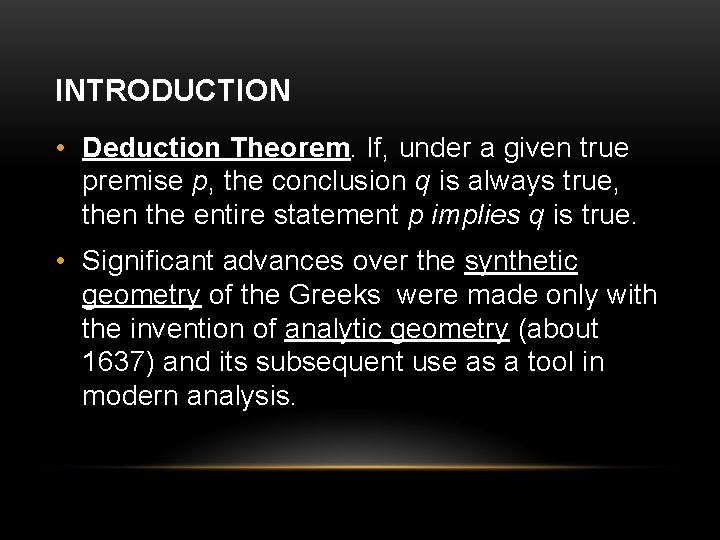
INTRODUCTION • Deduction Theorem. If, under a given true premise p, the conclusion q is always true, then the entire statement p implies q is true. • Significant advances over the synthetic geometry of the Greeks were made only with the invention of analytic geometry (about 1637) and its subsequent use as a tool in modern analysis.

INTRODUCTION • As the title Modern Geometry implies, the major emphasis is on newer geometries that have been developed since 1800. • The emergence of modern algebra, with its theory of groups, and the introduction of axiomatics into algebra paved the way for Felix Klein’s classification of geometries in 1872.

INTRODUCTION • Classification of geometries beyond Euclidean geometry: 1. Finite geometries 2. Geometry of inversion 3. Projective geometry 4. Non-Euclidean geometry

INTRODUCTION • The latter part of the nineteenth century witnessed a revival of interest in the classical geometry of the circle and the triangle. • Modern geometries differ from a traditional college geometry of a century ago, which concentrated only on a direct extension of the Euclidean geometry of the high school.

WORKSHEET 1 •

WORKSHEET 1 2. How many knots in the rope would be necessary to produce a triangle with shorter sides measuring 5 and 12 units? (2 pts. ) For numbers 3 – 8, answer true or false. (6 pts. ) 3. High school geometry owes more to the ancient Egyptians than to the ancient Greeks. 4. Euclid used the word postulate for an assumption confined to one particular subject.

WORKSHEET 1 5. The deduction theorem is used because a conclusion, not an implication, is to be proved. 6. Analytic geometry was invented before the development of finite geometries. 7. The latter part of the nineteenth century witnessed a revival of interest in the classical geometry of the circle and the triangle. 8. Traditional college geometry of a century ago included the study of more different geometries than are included today.

SETS OF AXIOMS FOR EUCLIDEAN GEOMETRY • Euclid, about 300 B. C. gave a famous set of five axioms and five postulates as follows: Axioms (or common notions) 1. Things that are equal to the same thing are also equal to one another. 2. If equals are added to equals, the wholes are equal. 3. If equals are subtracted from equals, the remainders are equal. 4. Things that coincide with one another are equal to one another. 5. The whole is greater than the part.

SETS OF AXIOMS FOR EUCLIDEAN GEOMETRY Postulates 1. A straight line can be drawn from any point to any point. 2. A finite straight line can be produced continuously in a straight line. 3. A circle may be described with any point as center and any distance as radius. 4. All right angles are equal to one another. 5. If a transversal falls on two lines in such a way that the interior angles on one side of the transversal are less than two right angles, then the lines meet on that side on which the angles are less than two right angles.

SETS OF AXIOMS FOR EUCLIDEAN GEOMETRY • Within the past hundred years in particular, mathematicians studying the foundations of mathematics have pointed out various flaws in the assumptions of Euclid. Logical problems pointed out have included: a. The need for a definite statement about the continuity of lines and circles. b. The need for a statement about the infinite extent of a straight line. c. The need to state the fact that if a straight line enters a triangle at a vertex, it must intersect the opposite side.

SETS OF AXIOMS FOR EUCLIDEAN GEOMETRY d. The need for statements about the order of points on a line. e. The need for a statement about the concept of betweenness. f. The need for a statement guaranteeing the uniqueness of a line joining two distinct points. g. The need for a more logical approach, such as that of transformations, which does not depend on the concept of superposition. Euclid assumed that a triangle can be picked up and put down in another place with all the properties remaining invariant, yet no statement to this effect was made. h. The need for a list of undefined terms.

SETS OF AXIOMS FOR EUCLIDEAN GEOMETRY • Many modern sets of axioms for Euclidean geometry have been introduced to remedy the defects in Euclid. • In general, these newer sets of axioms were more comprehensive than that of Euclid, but for this reason they appear more complex. • One of the first modern sets of axioms for Euclidean geometry was devised by Moritz Pasch in 1882.

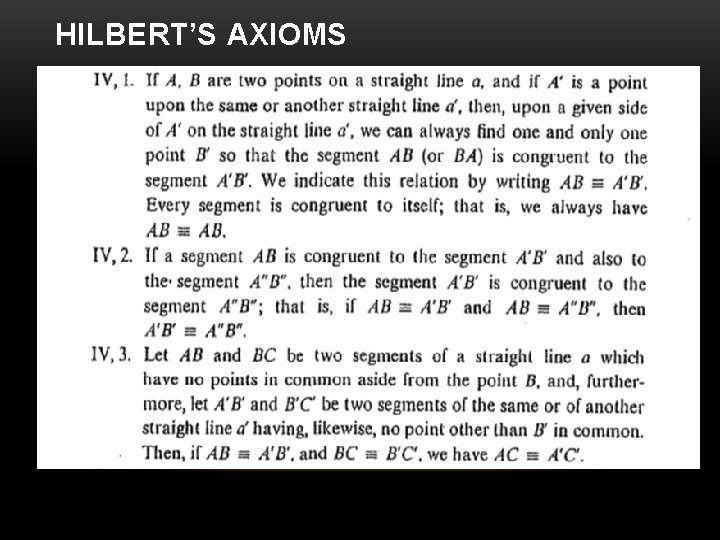
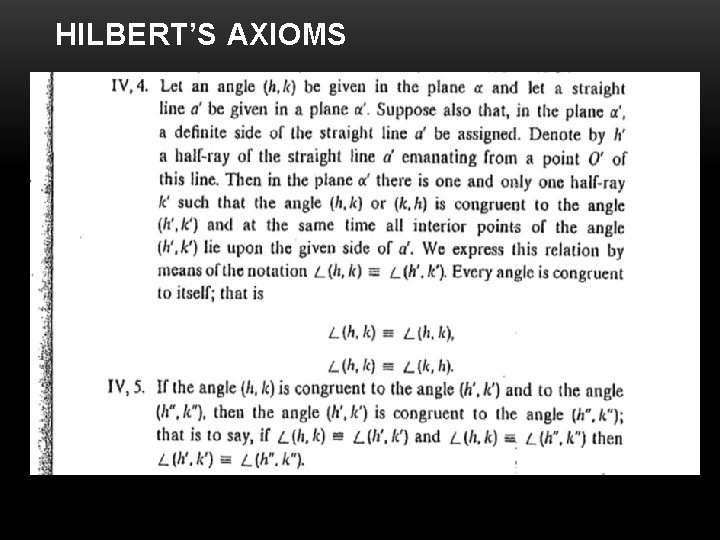
SETS OF AXIOMS FOR EUCLIDEAN GEOMETRY • Moritz Pasch is given credit for what is called Pasch’s axiom: A line entering a triangle at a vertex intersects the opposite side. • Guiseppi Peano provided another new approach in 1889. • Probably the most famous set of axioms for Euclidean geometry was given by David Hilbert and was published in English in 1902.

SETS OF AXIOMS FOR EUCLIDEAN GEOMETRY • Hilbert used six undefined terms: point, line, plane, between, congruent, and on. • He gave his axioms in five groups, and the grouping helps show the logical difficulties of Euclid were overcome. • Other sets of modern postulates for Euclidean geometry were devised. Some of these are by Oswald Veblen (1904 and 1911), Huntington (1913), Henry Forder (1927), G. D. Birkhoff (1932), and others.

HILBERT’S AXIOMS

HILBERT’S AXIOMS

HILBERT’S AXIOMS

HILBERT’S AXIOMS

HILBERT’S AXIOMS

HILBERT’S AXIOMS

SETS OF AXIOMS FOR EUCLIDEAN GEOMETRY • The axioms of Birkhoff are of special significance because they emphasize the connections between geometry and the real numbers, because they include distance and angle as undefined terms, and because they have been modified for incorporation in most recent experimental geometry courses.

BIRKHOFF’S POSTULATES

SETS OF AXIOMS FOR EUCLIDEAN GEOMETRY • Sets of axioms for Euclidean geometry, as well as for any mathematical system, should have two important properties. 1. The set should be consistent. In a consistent set of axioms, it is not possible to use them to prove a theorem that contradicts any axiom or other theorem that has already been proved. An example of an inconsistent system could be one that included both of these axioms: a. ) Two distinct points determine exactly one line; b. ) Two distinct points determine exactly two lines.

SETS OF AXIOMS FOR EUCLIDEAN GEOMETRY 2. The set should be complete. It should be impossible to add a consistent, independent axiom to the set without introducing new undefined terms. • It is often important that the set of axioms have at least one additional property so that none of the axioms can be proved from the remaining set of axioms. Set of axioms having this property are called independent sets of axioms.

PROBLEM SET 1 1. Draw a figure to explain the wording of Euclid’s fifth postulate. (5 pts. ) Draw a figure to explain the following axioms of Hilbert. (12 pts. ) 2. Order Axiom 2 3. Order Axiom 4 4. Order Axiom 5 5. Congruence Axiom 3 6. Congruence Axiom 4 7. The Axiom of Continuity

PROBLEM SET 1 For numbers 8 – 10, name the axioms of Hilbert that: 8. State Pasch’s axiom. (2 pts. ) 9. Guarantee the uniqueness of a line joining two distinct points. (2 pts. ) 10. Deal with betweenness for points on a line. (4 pts. )