Sequences and Series 1 Sequences and Series Objectives

Sequences and Series 1

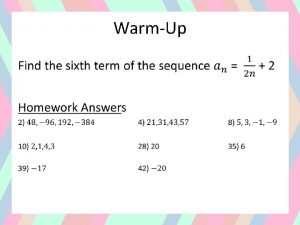
Sequences and Series Objectives: • To review concepts of sequences vs series * • To review arithmetic sequences and series • To review geometric sequences and series • To solve IB Exams questions on the topic • To use TI-84 Plus/CE in calculations 2

SEQUENCES Definitions ** • A sequence is a function. HOW is that? Spot #: 1 2 3 4 …. . Value/term: 9 1 - 7 -15 …. . • If not specified, it is understood that the domain, as a set of consecutive integers, starts with 1. D = { 1, 2, 3, 4, … } • Values in the range - terms of the sequence: R = { 9, 1, -7, -15, … } 3
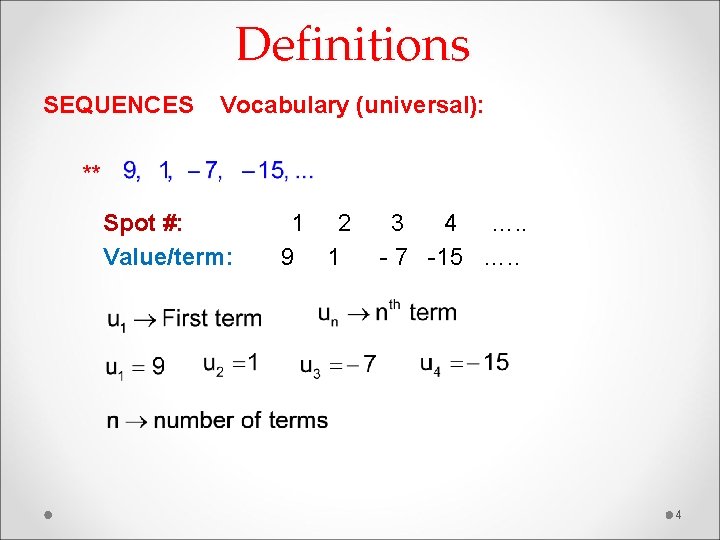
Definitions SEQUENCES Vocabulary (universal): ** Spot #: Value/term: 1 2 9 1 3 4 …. . - 7 -15 …. . 4

Definitions SERIES • A series is when the terms of sequence are added together • A series can be finite or infinite (i. e. we can add a finite number of terms or all of the infinite number of terms) Notation: 5
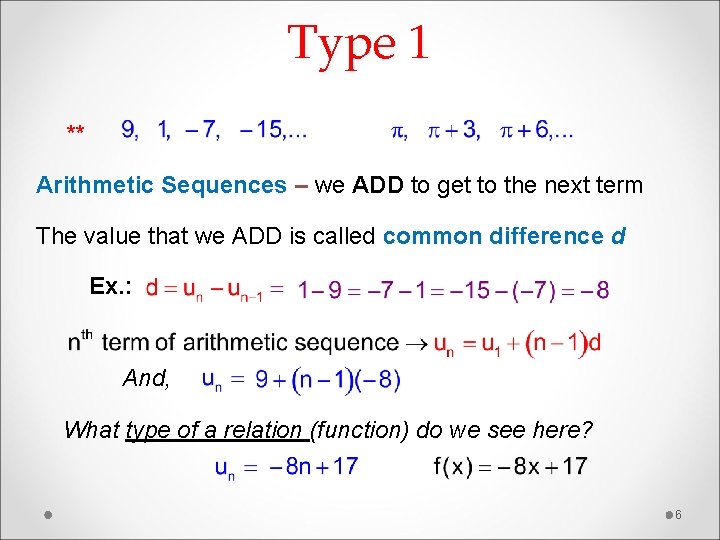
Type 1 ** Arithmetic Sequences – we ADD to get to the next term The value that we ADD is called common difference d Ex. : And, What type of a relation (function) do we see here? 6

Type 1 ** Arithmetic Series Expression formed by adding the first n terms of an arithmetic sequence. or Will only work for a finite series: In this example: 7

Arithmetic Sequences – example 1 (#6 your handout) Lets use your TI-84 …… 8

Arithmetic Sequences – example 2 9
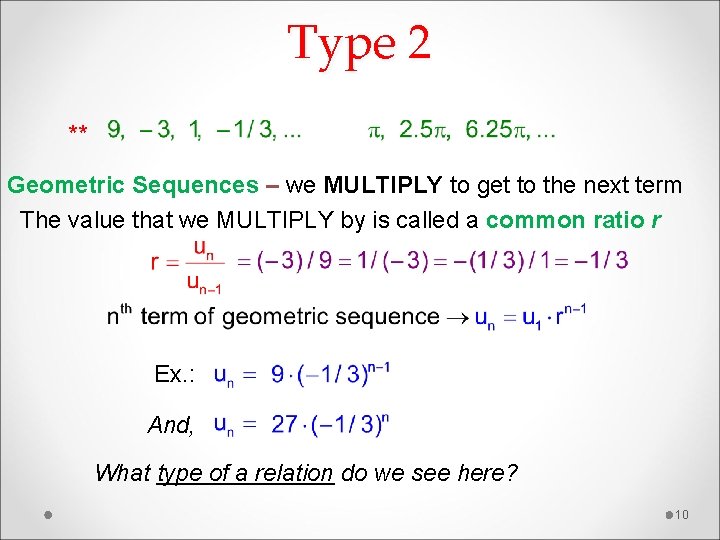
Type 2 ** Geometric Sequences – we MULTIPLY to get to the next term The value that we MULTIPLY by is called a common ratio r Ex. : And, What type of a relation do we see here? 10
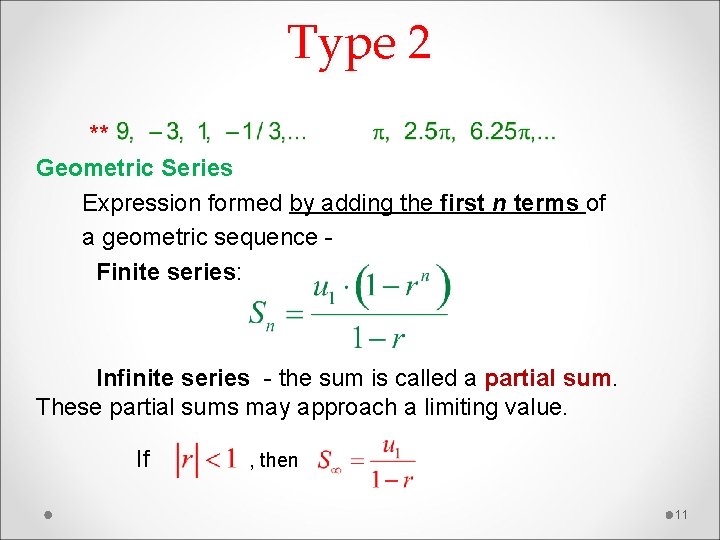
Type 2 ** Geometric Series Expression formed by adding the first n terms of a geometric sequence Finite series: Infinite series - the sum is called a partial sum. These partial sums may approach a limiting value. If , then 11

Geometric Sequences – example (#2 your handout) 12
- Slides: 12