Arithmetic Sequences Series Geometric Sequences Series Infinite Sequences

Arithmetic Sequences & Series Geometric Sequences & Series Infinite Sequences & Series “A good head and a good heart are always a formidable combination. ” -Nelson Mandela

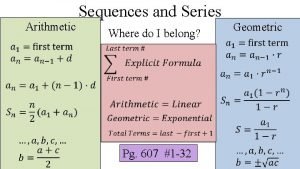
Arithmetic Sequences • Def: a sequence in which there is a common constant being added between successive terms. • The constant being added in known as the common difference (denoted d) • Ex: -5, -2, 1, … – Common difference is 3 – Next three terms would be 4, 7, 10

Arithmetic Sequence Continued • To find a specific term within an Arithmetic Sequence an = a 1 + (n – 1)d an = actual value of term a 1 = 1 st term of sequence n = what # term in the sequence d = common difference

Arithmetic Series • Def: is the sum of the terms of an arithmetic sequence • Sn refers to the nth partial sum: meaning sum of first “n” terms Sn = (a 1 + an) n/2

Arithmetic Means • Def: the terms between any two non consecutive terms of an arithmetic sequence. • Example: – 5, 16, 27; 16 is the arithmetic mean – 4, 9, 14, 19; 9 & 14 would be two AM btw 4 & 19 • To find arithmetic means, you want to find “d” – d = (an – a 1)/(n – 1)

Arithmetic Mean Example • Find 3 arithmetic means between 4 & 16 • Means: 4, _____, 16 • Find d: – an = 16, a 1 = 4, n = 5 – d = (16 – 4)/(5 – 1) which equals 3 • Therefore: 4, _7 , _10 , _13 , 16

Geometric Sequences • A Geometric Sequence is a sequence in which there is a constant that is multiplied between successive terms • The constant term if referred to as the common ratio, notated as ‘r ’ • Example: 1, 3, 9, 27, … – Common ratio is 3 – To find common ratio, divide 2 nd term by 1 st term – Next 3 terms are 81, 243, 729

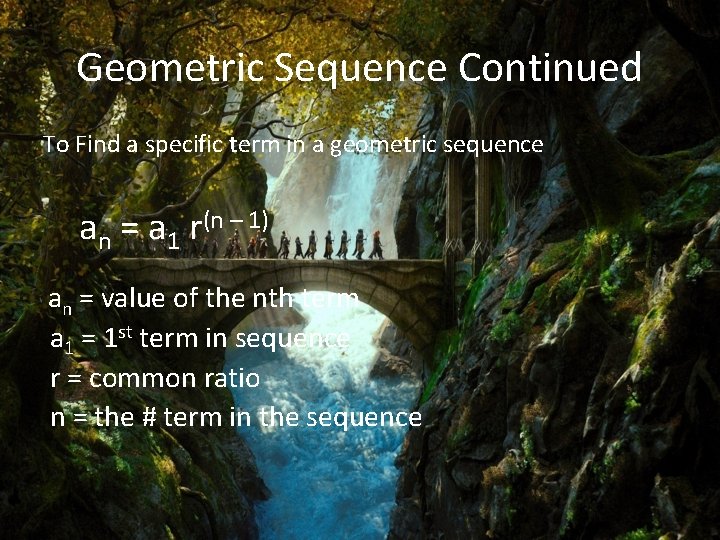
Geometric Sequence Continued To Find a specific term in a geometric sequence an = a 1 r(n – 1) an = value of the nth term a 1 = 1 st term in sequence r = common ratio n = the # term in the sequence

Geometric Series • A geometric Series is the sum of the terms of a geometric sequence

Infinite Sequence • An infinite sequence is a sequence with a an infinite number of terms • Infinite sequences have limits, meaning as the number of terms increases the sequence is heading in a specific direction • Written as:

• 3 Cases of limits ① Degree larger in the denominator means limit = 0 ② Degrees are the same in numerator & denominator means limit = ratio of leading coeffiecients ③ Degree larger in the numerator means limit = Does Not Exist (DNE)

Infinite Series • An infinite series is the indicated sum of an infinite sequence

One Ring to rule them all, One ring to find them; One ring to bring them all and in the darkness bind them Finish the task!!!!!!