Unit 10 Sequences Series By Saranya Nistala Unit

Unit 10: Sequences & Series By: Saranya Nistala

Unit Goal: I can find analyze arithmetic and geometric sequences and series. Key Concepts: Ø Define & use sequences and series Ø Analyze arithmetic sequences and series Ø Analyze Geometric sequences and series Ø Find sums of infinite geometric series Ø Use recursive rules with sequences and functions

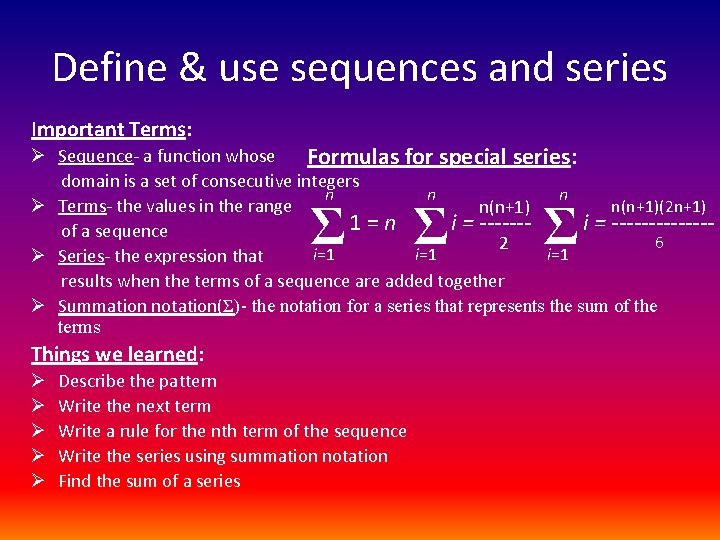
Define & use sequences and series Important Terms: Ø Sequence- a function whose Formulas for special series: domain is a set of consecutive integers n n n Ø Terms- the values in the range n(n+1)(2 n+1) n(n+1) 1=n i = -------------of a sequence 6 2 i=1 i=1 Ø Series- the expression that results when the terms of a sequence are added together Ø Summation notation(Ʃ)- the notation for a series that represents the sum of the terms Ʃ Things we learned: Ø Ø Ø Describe the pattern Write the next term Write a rule for the nth term of the sequence Write the series using summation notation Find the sum of a series Ʃ Ʃ

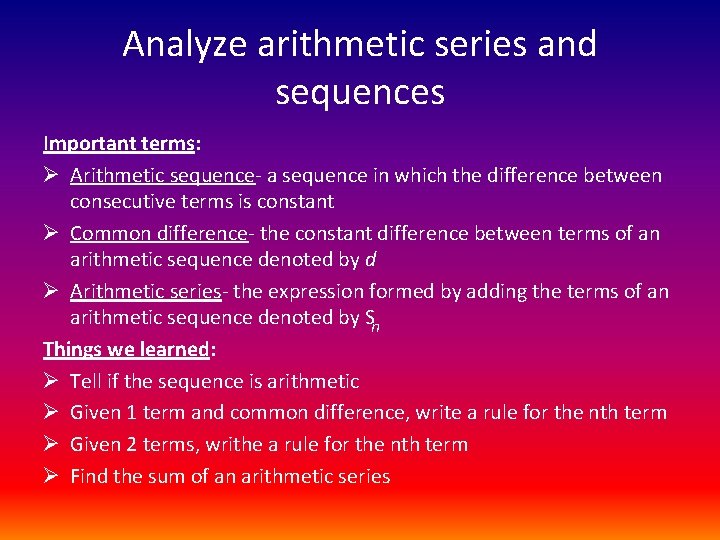
Analyze arithmetic series and sequences Important terms: Ø Arithmetic sequence- a sequence in which the difference between consecutive terms is constant Ø Common difference- the constant difference between terms of an arithmetic sequence denoted by d Ø Arithmetic series- the expression formed by adding the terms of an arithmetic sequence denoted by Sn Things we learned: Ø Tell if the sequence is arithmetic Ø Given 1 term and common difference, write a rule for the nth term Ø Given 2 terms, writhe a rule for the nth term Ø Find the sum of an arithmetic series

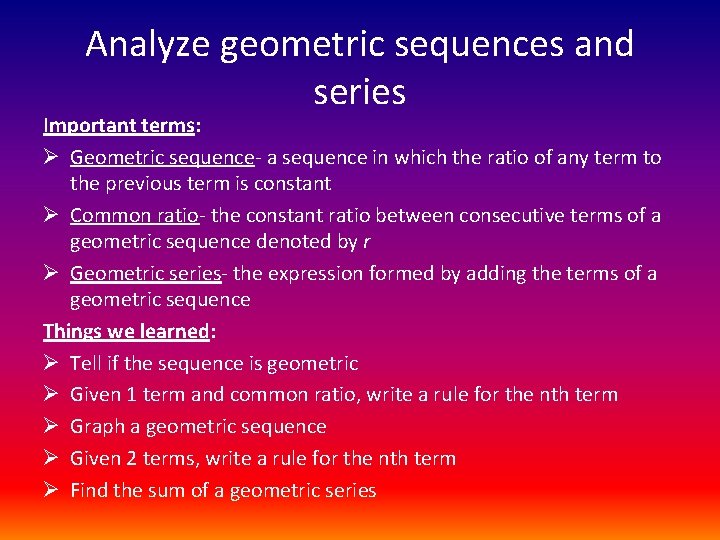
Analyze geometric sequences and series Important terms: Ø Geometric sequence- a sequence in which the ratio of any term to the previous term is constant Ø Common ratio- the constant ratio between consecutive terms of a geometric sequence denoted by r Ø Geometric series- the expression formed by adding the terms of a geometric sequence Things we learned: Ø Tell if the sequence is geometric Ø Given 1 term and common ratio, write a rule for the nth term Ø Graph a geometric sequence Ø Given 2 terms, write a rule for the nth term Ø Find the sum of a geometric series

Arithmetic and geometric sequence formulas

Find sums of infinite geometric series Important terms: Formula for infinite geometric series: Ø Partial sum- the sum Sn of the first a 1 n terms of an infinite series S = -------1 - r Things we learned: Ø Find the sum of an infinite geometric series Ø Write a recurring decimal as a fraction in lowest terms Example 1: ∞ Ʃ Solution: 6(0. 6)i-1 i=1 6 1 - 0. 6 6 0. 4 ------ = 15

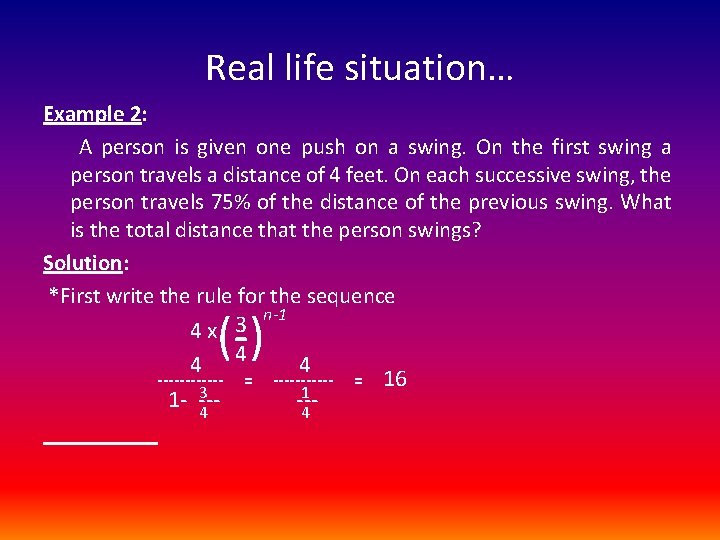
Real life situation… Example 2: A person is given one push on a swing. On the first swing a person travels a distance of 4 feet. On each successive swing, the person travels 75% of the distance of the previous swing. What is the total distance that the person swings? Solution: *First write the rule for the sequence n-1 3 4 x 4 4 4 ------ = 16 3 1 1 - ----4 4 (-)

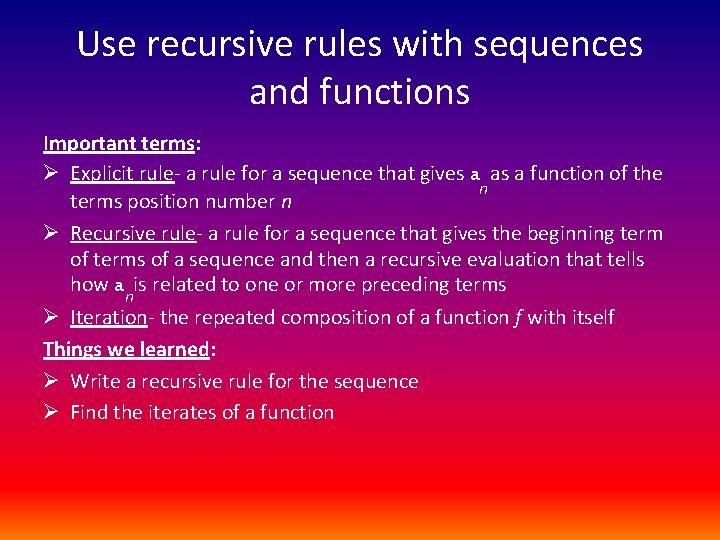
Use recursive rules with sequences and functions Important terms: Ø Explicit rule- a rule for a sequence that gives a as a function of the n terms position number n Ø Recursive rule- a rule for a sequence that gives the beginning term of terms of a sequence and then a recursive evaluation that tells how a is related to one or more preceding terms n Ø Iteration- the repeated composition of a function f with itself Things we learned: Ø Write a recursive rule for the sequence Ø Find the iterates of a function

Practice problems Example 3: Write a recursive rule for the sequence 3, 5, 2, -3, -5……. Solution: a =na - n-1 a n-2 Example 4: Find the first three iterates x 1, x 2 of the function 3 f(x) = 2 x – 5 for an initial value of x = 3. Solution: x =1 f(x )0 x =1 1, x = 2 -3, x = -1 3

Common mistakes and struggles Ø Applying the correct formula for a problem Ø When writing a recurring decimal as a fraction in lowest terms, separate non-repeating and repeating parts Ø Always use a when taking an even root Ø When solving a story problem, write the rule for the sequence first, to avoid mistakes Ø When finding the sum for an infinite geometric series, r must be less than 1

Connections to other topics ØExponential growth and decay modelsconnected to graphing geometric sequences ØStatistics- connected because both use summation notation