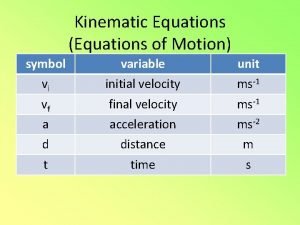
Kinematics of a particle moving in a straight

Kinematics of a particle moving in a straight line or plane

Introduction • In this chapter you will extend the knowledge of kinematics from M 1 • You will use the SUVAT equations to solve problems in 2 D where a particle has vertical and horizontal motion (commonly referred to as projectiles) • You will also see how to solve problems involving variable velocities and accelerations, and how calculus from Core Maths fits into Mechanics!

Teachings for Exercise 1 A

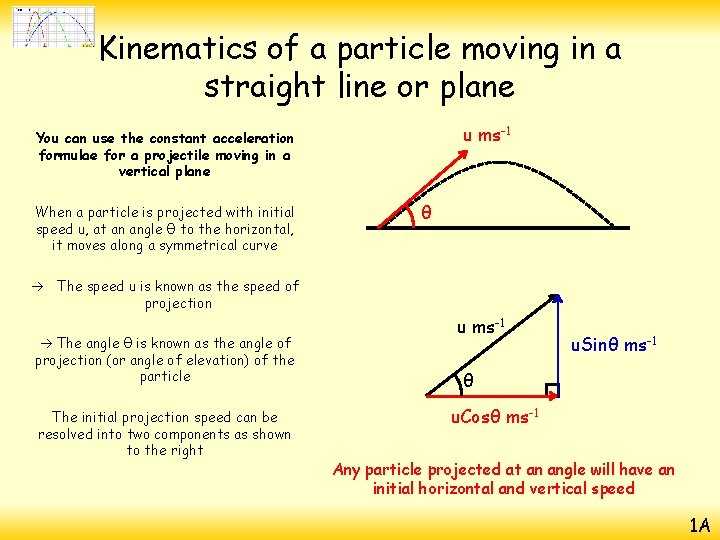
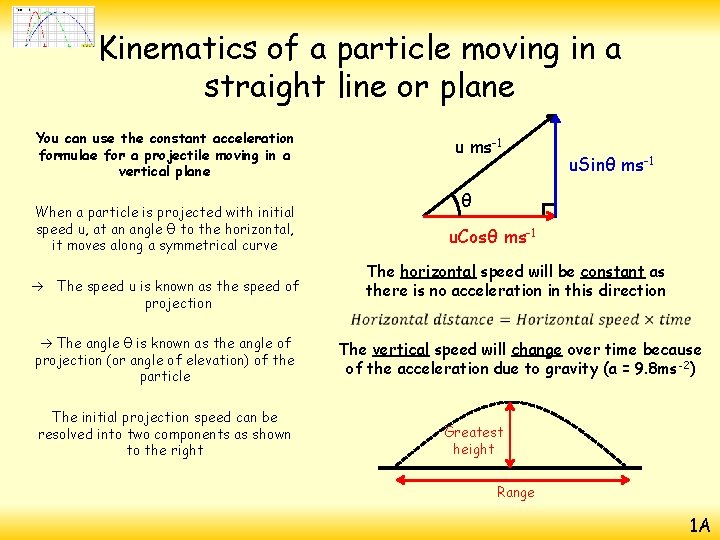
Kinematics of a particle moving in a straight line or plane u ms-1 You can use the constant acceleration formulae for a projectile moving in a vertical plane When a particle is projected with initial speed u, at an angle θ to the horizontal, it moves along a symmetrical curve θ The speed u is known as the speed of projection The angle θ is known as the angle of projection (or angle of elevation) of the particle The initial projection speed can be resolved into two components as shown to the right u ms-1 u. Sinθ ms-1 θ u. Cosθ ms-1 Any particle projected at an angle will have an initial horizontal and vertical speed 1 A

Kinematics of a particle moving in a straight line or plane You can use the constant acceleration formulae for a projectile moving in a vertical plane u ms-1 θ When a particle is projected with initial speed u, at an angle θ to the horizontal, it moves along a symmetrical curve The speed u is known as the speed of projection The angle θ is known as the angle of projection (or angle of elevation) of the particle The initial projection speed can be resolved into two components as shown to the right u. Sinθ ms-1 u. Cosθ ms-1 The horizontal speed will be constant as there is no acceleration in this direction The vertical speed will change over time because of the acceleration due to gravity (a = 9. 8 ms-2) Greatest height Range 1 A

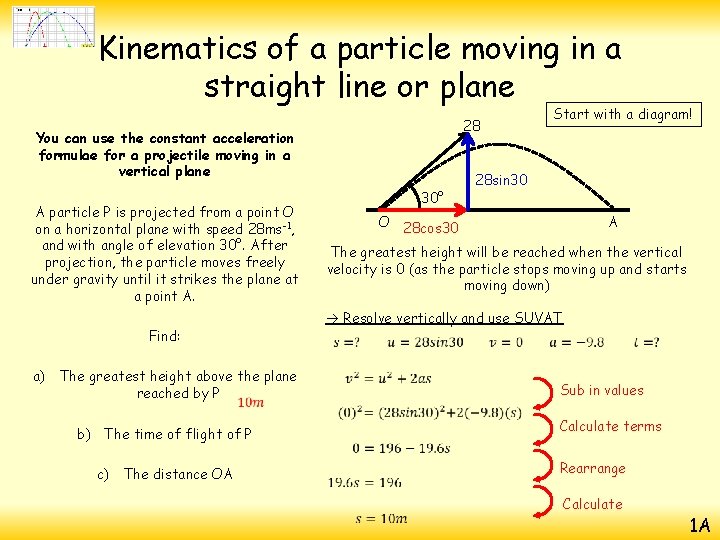
Kinematics of a particle moving in a straight line or plane You can use the constant acceleration formulae for a projectile moving in a vertical plane A particle P is projected from a point O on a horizontal plane with speed 28 ms-1, and with angle of elevation 30°. After projection, the particle moves freely under gravity until it strikes the plane at a point A. 30° 28 sin 30 O 28 cos 30 A The greatest height will be reached when the vertical velocity is 0 (as the particle stops moving up and starts moving down) Resolve vertically and use SUVAT Find: a) The greatest height above the plane reached by P Sub in values b) The time of flight of P c) The distance OA Start with a diagram! 28 Calculate terms Rearrange Calculate 1 A

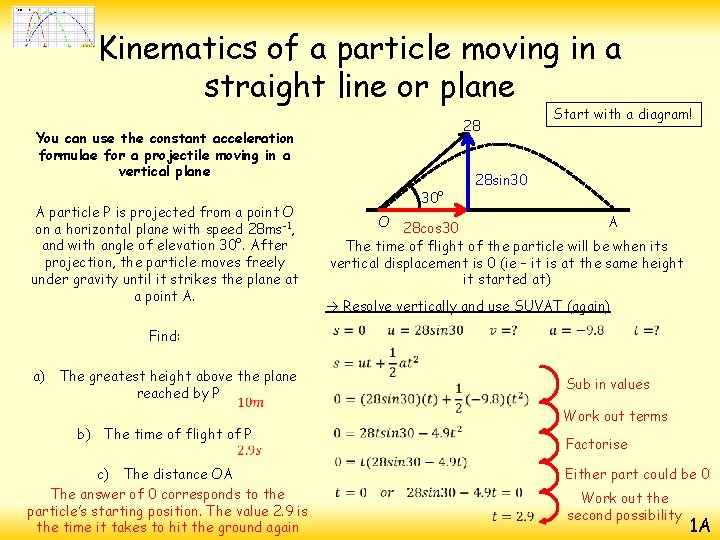
Kinematics of a particle moving in a straight line or plane You can use the constant acceleration formulae for a projectile moving in a vertical plane A particle P is projected from a point O on a horizontal plane with speed 28 ms-1, and with angle of elevation 30°. After projection, the particle moves freely under gravity until it strikes the plane at a point A. 30° Resolve vertically and use SUVAT (again) a) The greatest height above the plane reached by P c) The distance OA The answer of 0 corresponds to the particle’s starting position. The value 2. 9 is the time it takes to hit the ground again Work out terms Factorise Sub in values 28 sin 30 O 28 cos 30 A The time of flight of the particle will be when its vertical displacement is 0 (ie – it is at the same height it started at) Find: b) The time of flight of P Start with a diagram! 28 Either part could be 0 Work out the second possibility 1 A

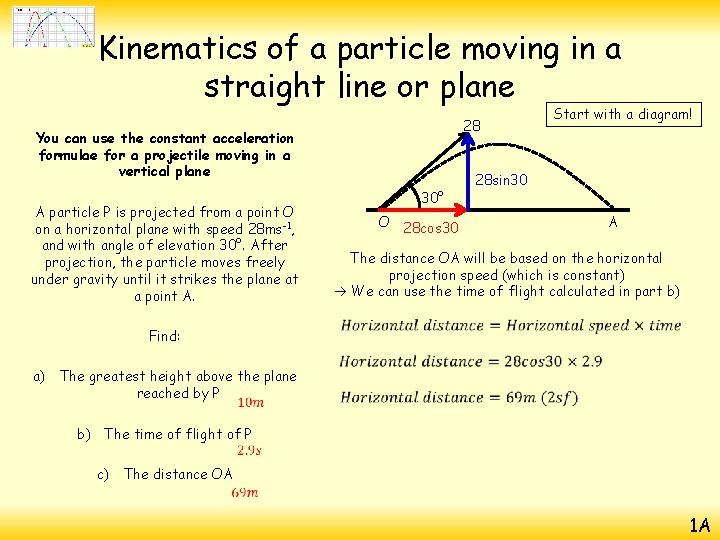
Kinematics of a particle moving in a straight line or plane 28 You can use the constant acceleration formulae for a projectile moving in a vertical plane A particle P is projected from a point O on a horizontal plane with speed 28 ms-1, and with angle of elevation 30°. After projection, the particle moves freely under gravity until it strikes the plane at a point A. 30° O 28 cos 30 Start with a diagram! 28 sin 30 A The distance OA will be based on the horizontal projection speed (which is constant) We can use the time of flight calculated in part b) Find: a) The greatest height above the plane reached by P b) The time of flight of P c) The distance OA 1 A

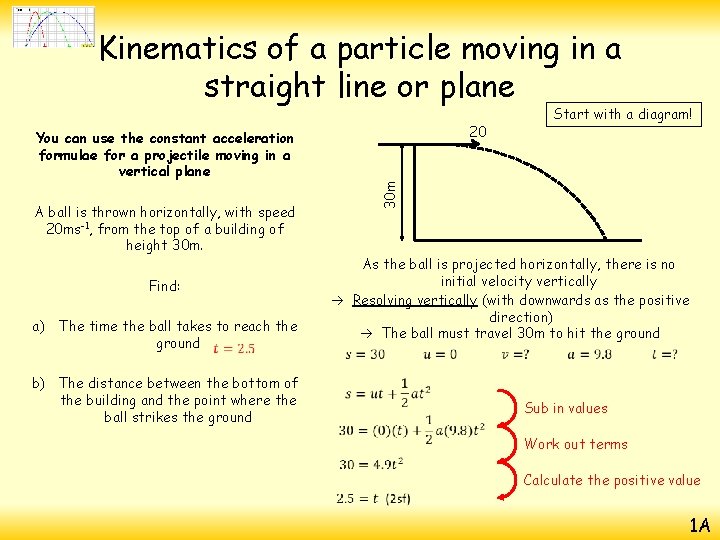
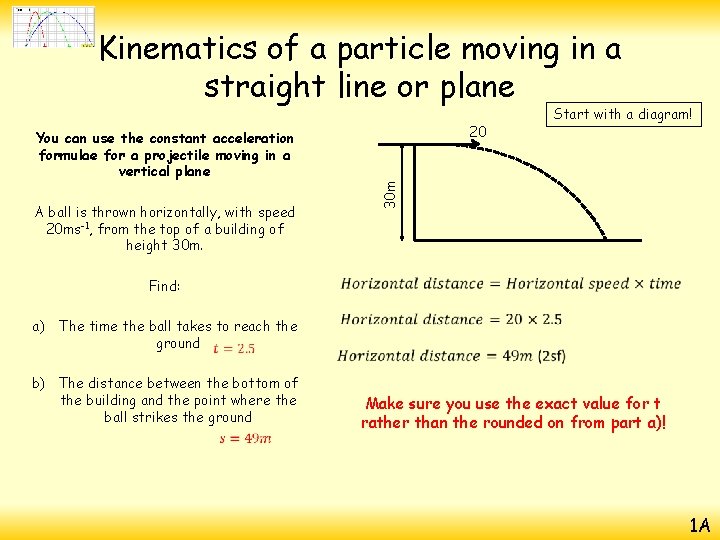
Kinematics of a particle moving in a straight line or plane 20 Find: a) The time the ball takes to reach the ground b) The distance between the bottom of the building and the point where the ball strikes the ground 30 m You can use the constant acceleration formulae for a projectile moving in a vertical plane A ball is thrown horizontally, with speed 20 ms-1, from the top of a building of height 30 m. Start with a diagram! As the ball is projected horizontally, there is no initial velocity vertically Resolving vertically (with downwards as the positive direction) The ball must travel 30 m to hit the ground Sub in values Work out terms Calculate the positive value 1 A

Kinematics of a particle moving in a straight line or plane 20 30 m You can use the constant acceleration formulae for a projectile moving in a vertical plane A ball is thrown horizontally, with speed 20 ms-1, from the top of a building of height 30 m. Find: a) The time the ball takes to reach the ground b) The distance between the bottom of the building and the point where the ball strikes the ground Start with a diagram! Make sure you use the exact value for t rather than the rounded on from part a)! 1 A

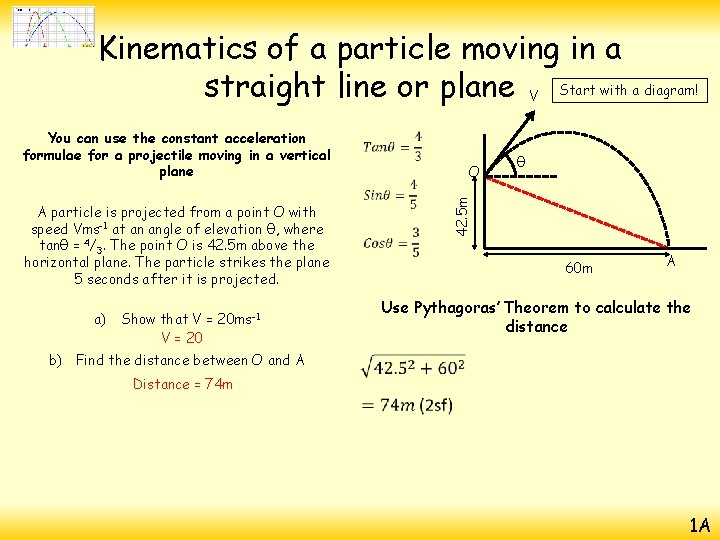
Kinematics of a particle moving in a straight line or plane V Start with a diagram! A particle is projected from a point O with speed Vms-1 at an angle of elevation θ, where tanθ = 4/3. The point O is 42. 5 m above the horizontal plane. The particle strikes the plane 5 seconds after it is projected. O 42. 5 m You can use the constant acceleration formulae for a projectile moving in a vertical plane Find the values of Sinθ and Cosθ first, by labelling a triangle where Tanθ = 4/3 (That is, opposite = 4 and adjacent = 3) Hyp 5 Use Pythagoras’ Theorem 4 Opp to find the missing side Remember Sinθ = opp/ adj/ 3 hyp and Cosθ = hyp Adj Vcosθ A Resolving vertically using the information given (taking upwards as positive) The ball will be 42. 5 m lower after 5 seconds a) Show that V = 20 ms-1 b) Find the distance between O and A Vsinθ θ Sub in values Calculate terms (use sinθ = 4/5) Add 122. 5 Divide by 4 1 A

Kinematics of a particle moving in a straight line or plane V Start with a diagram! You can use the constant acceleration formulae for a projectile moving in a vertical plane A particle is projected from a point O with speed Vms-1 at an angle of elevation θ, where tanθ = 4/3. The point O is 42. 5 m above the horizontal plane. The particle strikes the plane 5 seconds after it is projected. a) Show that V = 20 b) Find the distance between O and A 20 ms-1 Make sure you read the question – we want the distance OA, not just the horizontal distance travelled! 42. 5 m O θ Vsinθ Vcosθ 60 m A 1 A

Kinematics of a particle moving in a straight line or plane V Start with a diagram! You can use the constant acceleration formulae for a projectile moving in a vertical plane A particle is projected from a point O with speed Vms-1 at an angle of elevation θ, where tanθ = 4/3. The point O is 42. 5 m above the horizontal plane. The particle strikes the plane 5 seconds after it is projected. a) Show that V = 20 ms-1 V = 20 b) Find the distance between O and A θ 42. 5 m O 60 m A Use Pythagoras’ Theorem to calculate the distance Distance = 74 m 1 A

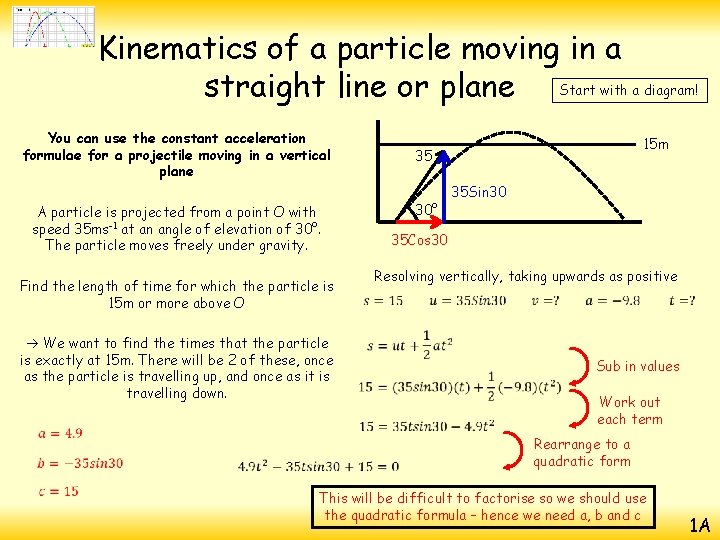
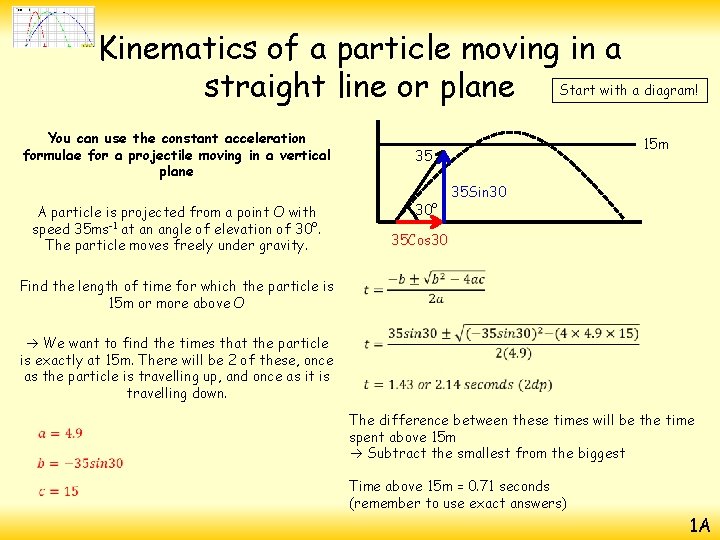
Kinematics of a particle moving in a straight line or plane Start with a diagram! You can use the constant acceleration formulae for a projectile moving in a vertical plane 30° A particle is projected from a point O with speed 35 ms-1 at an angle of elevation of 30°. The particle moves freely under gravity. Resolving vertically, taking upwards as positive 35 Sin 30 35 Cos 30 Find the length of time for which the particle is 15 m or more above O We want to find the times that the particle is exactly at 15 m. There will be 2 of these, once as the particle is travelling up, and once as it is travelling down. 15 m 35 Sub in values Work out each term Rearrange to a quadratic form This will be difficult to factorise so we should use the quadratic formula – hence we need a, b and c 1 A

Kinematics of a particle moving in a straight line or plane Start with a diagram! You can use the constant acceleration formulae for a projectile moving in a vertical plane 30° A particle is projected from a point O with speed 35 ms-1 at an angle of elevation of 30°. The particle moves freely under gravity. Find the length of time for which the particle is 15 m or more above O We want to find the times that the particle is exactly at 15 m. There will be 2 of these, once as the particle is travelling up, and once as it is travelling down. 15 m 35 35 Sin 30 35 Cos 30 The difference between these times will be the time spent above 15 m Subtract the smallest from the biggest Time above 15 m = 0. 71 seconds (remember to use exact answers) 1 A

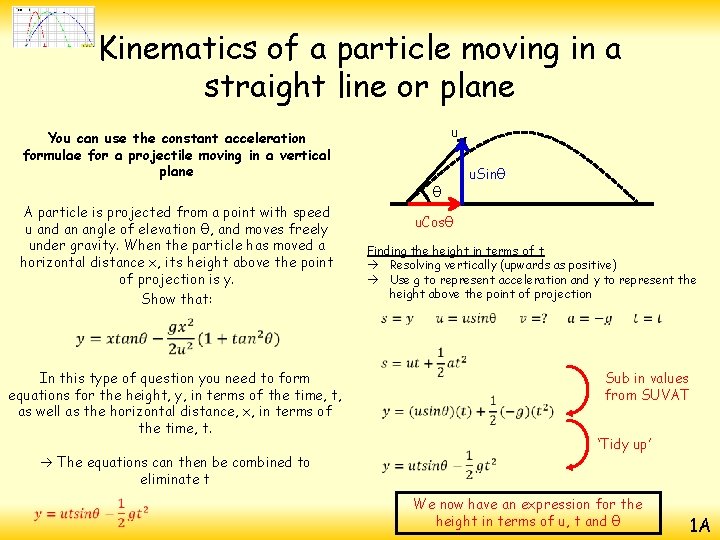
Kinematics of a particle moving in a straight line or plane u You can use the constant acceleration formulae for a projectile moving in a vertical plane θ A particle is projected from a point with speed u and an angle of elevation θ, and moves freely under gravity. When the particle has moved a horizontal distance x, its height above the point of projection is y. Show that: u. Sinθ u. Cosθ Finding the height in terms of t Resolving vertically (upwards as positive) Use g to represent acceleration and y to represent the height above the point of projection In this type of question you need to form equations for the height, y, in terms of the time, t, as well as the horizontal distance, x, in terms of the time, t. The equations can then be combined to eliminate t Sub in values from SUVAT ‘Tidy up’ We now have an expression for the height in terms of u, t and θ 1 A

Kinematics of a particle moving in a straight line or plane u You can use the constant acceleration formulae for a projectile moving in a vertical plane θ A particle is projected from a point with speed u and an angle of elevation θ, and moves freely under gravity. When the particle has moved a horizontal distance x, its height above the point of projection is y. Show that: u. Sinθ u. Cosθ Finding the horizontal distance in terms of t In this type of question you need to form equations for the height, y, in terms of the time, t, as well as the horizontal distance, x, in terms of the time, t. Sub in values (remember x is to be used to represent the horizontal distance) The equations can then be combined to eliminate t 1 A

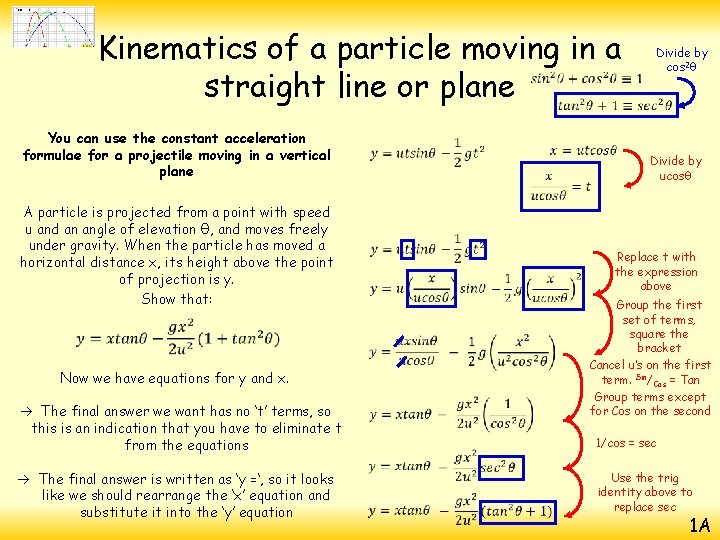
Kinematics of a particle moving in a straight line or plane Divide by cos 2θ You can use the constant acceleration formulae for a projectile moving in a vertical plane A particle is projected from a point with speed u and an angle of elevation θ, and moves freely under gravity. When the particle has moved a horizontal distance x, its height above the point of projection is y. Show that: Now we have equations for y and x. The final answer we want has no ‘t’ terms, so this is an indication that you have to eliminate t from the equations The final answer is written as ‘y =‘, so it looks like we should rearrange the ‘x’ equation and substitute it into the ‘y’ equation Divide by ucosθ Replace t with the expression above Group the first set of terms, square the bracket Cancel u’s on the first term. Sin/Cos = Tan Group terms except for Cos on the second 1/cos = sec Use the trig identity above to replace sec 1 A

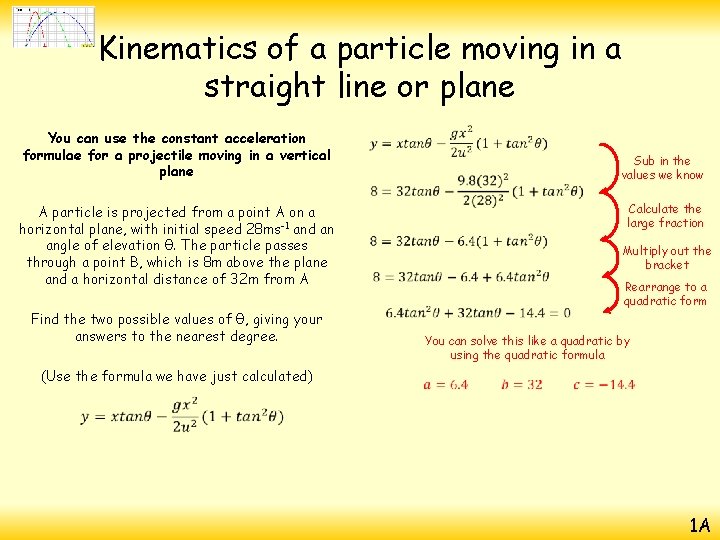
Kinematics of a particle moving in a straight line or plane You can use the constant acceleration formulae for a projectile moving in a vertical plane A particle is projected from a point A on a horizontal plane, with initial speed 28 ms-1 and an angle of elevation θ. The particle passes through a point B, which is 8 m above the plane and a horizontal distance of 32 m from A Find the two possible values of θ, giving your answers to the nearest degree. (Use the formula we have just calculated) Sub in the values we know Calculate the large fraction Multiply out the bracket Rearrange to a quadratic form You can solve this like a quadratic by using the quadratic formula 1 A

Kinematics of a particle moving in a straight line or plane You can use the constant acceleration formulae for a projectile moving in a vertical plane A particle is projected from a point A on a horizontal plane, with initial speed 28 ms-1 and an angle of elevation θ. The particle passes through a point B, which is 8 m above the plane and a horizontal distance of 32 m from A Find the two possible values of θ, giving your answers to the nearest degree. Sub in values Calculate each possibility Use inverse Tan (and round answers) There will be two possibilities here: (Use the formula we have just calculated) B If a projectile passes through point B, there are two possible angles it could have been launched through A 1 A

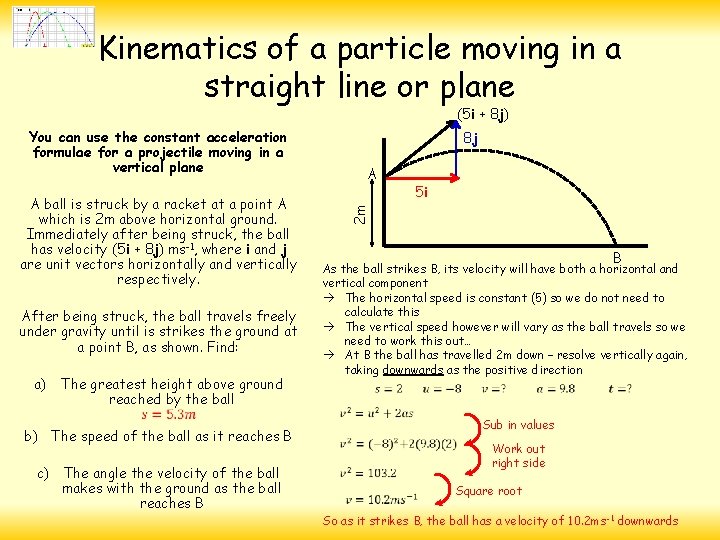
Kinematics of a particle moving in a straight line or plane (5 i + 8 j) You can use the constant acceleration formulae for a projectile moving in a vertical plane A B You can use the vectors in each direction as the initial velocities Resolve vertically to find the greatest height After being struck, the ball travels freely under gravity until is strikes the ground at a point B, as shown. Find: a) The greatest height above ground reached by the ball 5 i 2 m A ball is struck by a racket at a point A which is 2 m above horizontal ground. Immediately after being struck, the ball has velocity (5 i + 8 j) ms-1, where i and j are unit vectors horizontally and vertically respectively. 8 j Sub in values Work out terms b) The speed of the ball as it reaches B c) The angle the velocity of the ball makes with the ground as the ball reaches B Calculate s Careful – the ball starts at a height of 2 m, so this must be added on! 1 A

Kinematics of a particle moving in a straight line or plane (5 i + 8 j) You can use the constant acceleration formulae for a projectile moving in a vertical plane After being struck, the ball travels freely under gravity until is strikes the ground at a point B, as shown. Find: a) The greatest height above ground reached by the ball b) The speed of the ball as it reaches B c) The angle the velocity of the ball makes with the ground as the ball reaches B A 5 i 2 m A ball is struck by a racket at a point A which is 2 m above horizontal ground. Immediately after being struck, the ball has velocity (5 i + 8 j) ms-1, where i and j are unit vectors horizontally and vertically respectively. 8 j B As the ball strikes B, its velocity will have both a horizontal and vertical component The horizontal speed is constant (5) so we do not need to calculate this The vertical speed however will vary as the ball travels so we need to work this out… At B the ball has travelled 2 m down – resolve vertically again, taking downwards as the positive direction Sub in values Work out right side Square root So as it strikes B, the ball has a velocity of 10. 2 ms -1 downwards

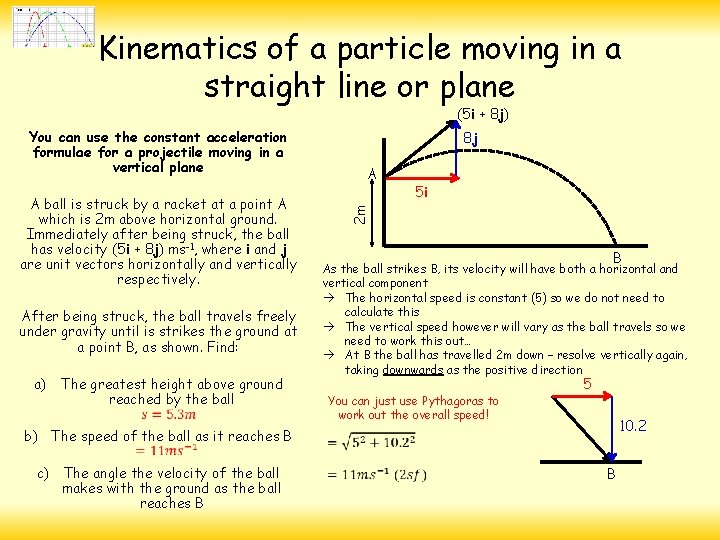
Kinematics of a particle moving in a straight line or plane (5 i + 8 j) You can use the constant acceleration formulae for a projectile moving in a vertical plane After being struck, the ball travels freely under gravity until is strikes the ground at a point B, as shown. Find: a) The greatest height above ground reached by the ball A B As the ball strikes B, its velocity will have both a horizontal and vertical component The horizontal speed is constant (5) so we do not need to calculate this The vertical speed however will vary as the ball travels so we need to work this out… At B the ball has travelled 2 m down – resolve vertically again, taking downwards as the positive direction You can just use Pythagoras to work out the overall speed! b) The speed of the ball as it reaches B c) The angle the velocity of the ball makes with the ground as the ball reaches B 5 i 2 m A ball is struck by a racket at a point A which is 2 m above horizontal ground. Immediately after being struck, the ball has velocity (5 i + 8 j) ms-1, where i and j are unit vectors horizontally and vertically respectively. 8 j 5 10. 2 B

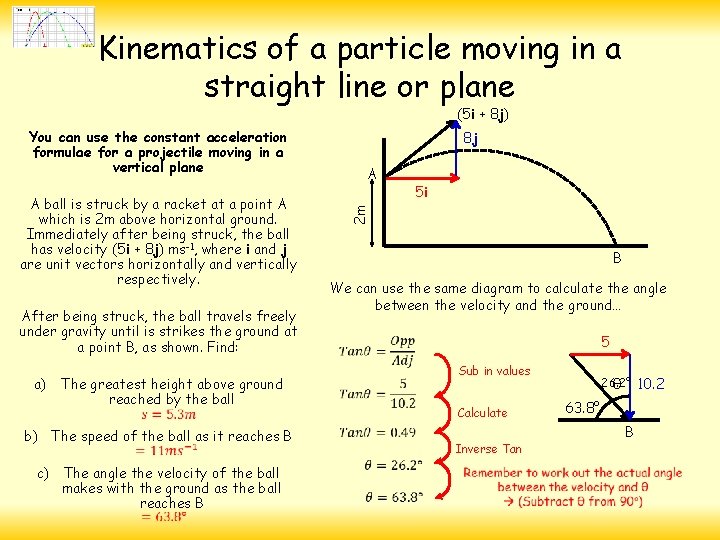
Kinematics of a particle moving in a straight line or plane (5 i + 8 j) You can use the constant acceleration formulae for a projectile moving in a vertical plane After being struck, the ball travels freely under gravity until is strikes the ground at a point B, as shown. Find: A B We can use the same diagram to calculate the angle between the velocity and the ground… b) The speed of the ball as it reaches B c) The angle the velocity of the ball makes with the ground as the ball reaches B 5 a) The greatest height above ground reached by the ball 5 i 2 m A ball is struck by a racket at a point A which is 2 m above horizontal ground. Immediately after being struck, the ball has velocity (5 i + 8 j) ms-1, where i and j are unit vectors horizontally and vertically respectively. 8 j Sub in values Calculate Inverse Tan 26. 2° θ 10. 2 63. 8° B

Teachings for Exercise 1 B

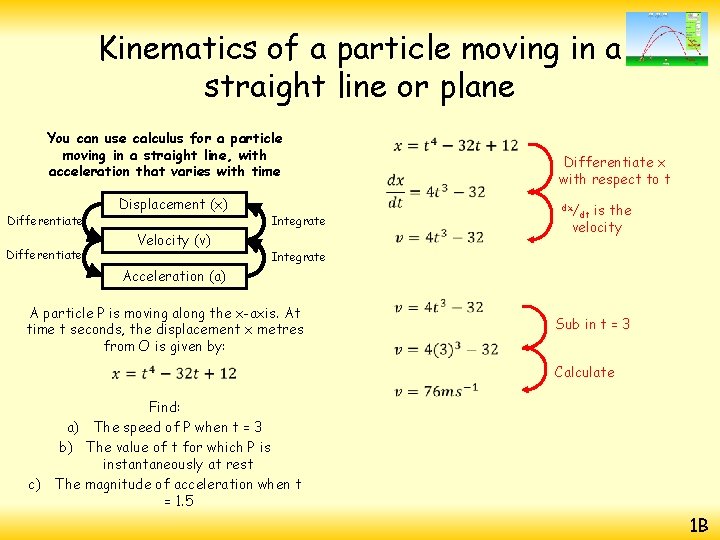
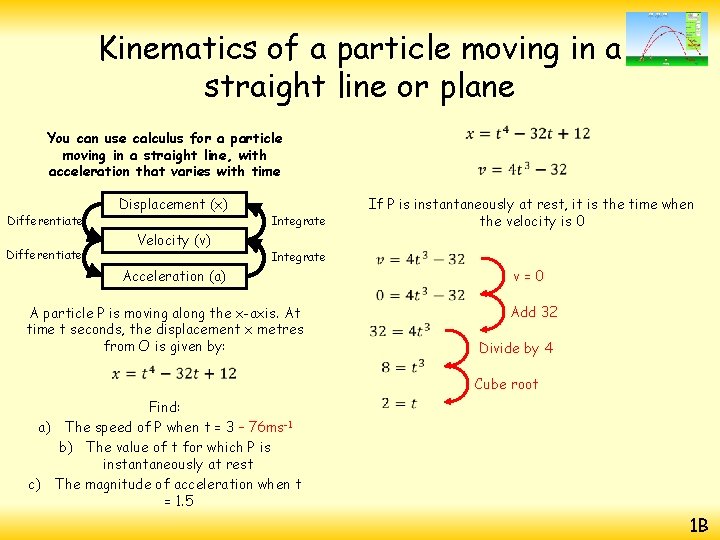
Kinematics of a particle moving in a straight line or plane You can use calculus for a particle moving in a straight line, with acceleration that varies with time Displacement (x) Differentiate Integrate The SUVAT equations can be used when acceleration is constant and a particle is moving in a straight line. If the acceleration of a particle varies, you need to use Calculus Velocity (v) Differentiate Integrate Acceleration (a) Velocity is the rate of change of displacement with respect to time Acceleration is the rate of change of velocity with respect to time Therefore, differentiating displacement gives velocity Therefore, differentiating velocity gives acceleration In reverse, use integration! 1 B

Kinematics of a particle moving in a straight line or plane You can use calculus for a particle moving in a straight line, with acceleration that varies with time Displacement (x) Differentiate Velocity (v) Differentiate Integrate Differentiate x with respect to t dx/ is the velocity dt Acceleration (a) A particle P is moving along the x-axis. At time t seconds, the displacement x metres from O is given by: c) Find: a) The speed of P when t = 3 b) The value of t for which P is instantaneously at rest The magnitude of acceleration when t = 1. 5 Sub in t = 3 Calculate 1 B

Kinematics of a particle moving in a straight line or plane You can use calculus for a particle moving in a straight line, with acceleration that varies with time Displacement (x) Differentiate Velocity (v) Differentiate Integrate If P is instantaneously at rest, it is the time when the velocity is 0 Integrate Acceleration (a) A particle P is moving along the x-axis. At time t seconds, the displacement x metres from O is given by: Find: a) The speed of P when t = 3 – 76 ms-1 b) The value of t for which P is instantaneously at rest c) The magnitude of acceleration when t = 1. 5 v=0 Add 32 Divide by 4 Cube root 1 B

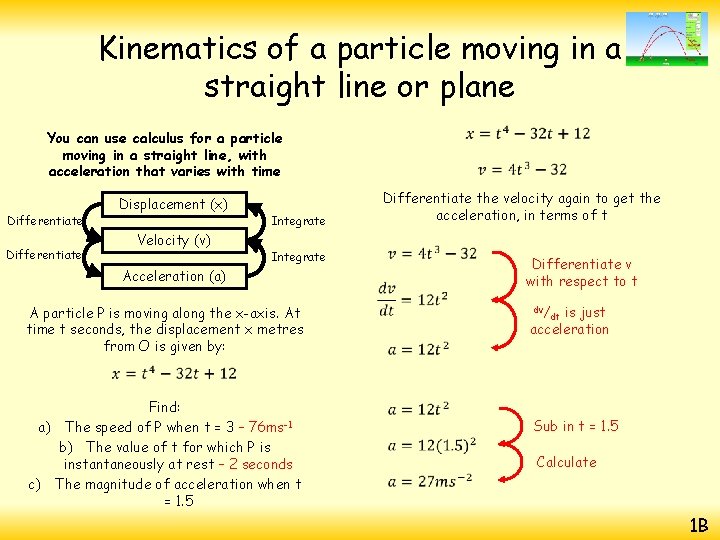
Kinematics of a particle moving in a straight line or plane You can use calculus for a particle moving in a straight line, with acceleration that varies with time Displacement (x) Differentiate Velocity (v) Differentiate Integrate Differentiate the velocity again to get the acceleration, in terms of t Integrate Acceleration (a) A particle P is moving along the x-axis. At time t seconds, the displacement x metres from O is given by: Differentiate v with respect to t dv/ is just acceleration dt Find: a) The speed of P when t = 3 – 76 ms-1 b) The value of t for which P is instantaneously at rest – 2 seconds c) The magnitude of acceleration when t = 1. 5 Sub in t = 1. 5 Calculate 1 B

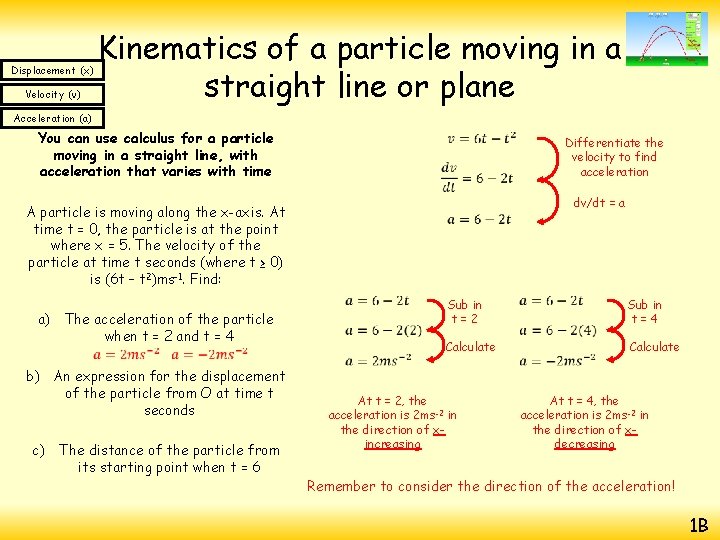
Displacement (x) Velocity (v) Kinematics of a particle moving in a straight line or plane Acceleration (a) You can use calculus for a particle moving in a straight line, with acceleration that varies with time dv/dt = a A particle is moving along the x-axis. At time t = 0, the particle is at the point where x = 5. The velocity of the particle at time t seconds (where t ≥ 0) is (6 t – t 2)ms-1. Find: a) The acceleration of the particle when t = 2 and t = 4 b) An expression for the displacement of the particle from O at time t seconds c) The distance of the particle from its starting point when t = 6 Differentiate the velocity to find acceleration Sub in t=2 Calculate At t = 2, the acceleration is 2 ms-2 in the direction of xincreasing Sub in t=4 Calculate At t = 4, the acceleration is 2 ms-2 in the direction of xdecreasing Remember to consider the direction of the acceleration! 1 B

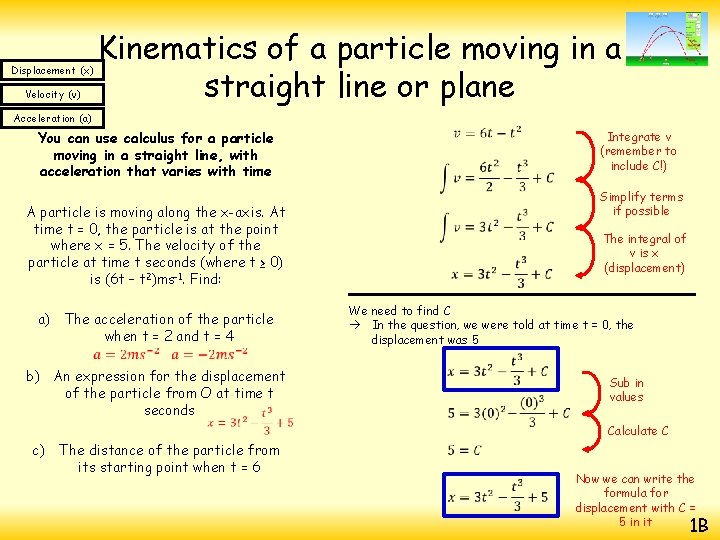
Displacement (x) Velocity (v) Kinematics of a particle moving in a straight line or plane Acceleration (a) You can use calculus for a particle moving in a straight line, with acceleration that varies with time A particle is moving along the x-axis. At time t = 0, the particle is at the point where x = 5. The velocity of the particle at time t seconds (where t ≥ 0) is (6 t – t 2)ms-1. Find: a) The acceleration of the particle when t = 2 and t = 4 b) An expression for the displacement of the particle from O at time t seconds c) The distance of the particle from its starting point when t = 6 Integrate v (remember to include C!) Simplify terms if possible The integral of v is x (displacement) We need to find C In the question, we were told at time t = 0, the displacement was 5 Sub in values Calculate C Now we can write the formula for displacement with C = 5 in it 1 B

Displacement (x) Velocity (v) Kinematics of a particle moving in a straight line or plane Acceleration (a) You can use calculus for a particle moving in a straight line, with acceleration that varies with time A particle is moving along the x-axis. At time t = 0, the particle is at the point where x = 5. The velocity of the particle at time t seconds (where t ≥ 0) is (6 t – t 2)ms-1. Find: a) The acceleration of the particle when t = 2 and t = 4 b) An expression for the displacement of the particle from O at time t seconds Sub in t = 6 Calculate x Remember to read the question! We need the particle’s displacement from its starting point. As its starting point was 5 m from O, it has moved 36 m (to get to 41 m) c) The distance of the particle from its starting point when t = 6 1 B

Displacement (x) Velocity (v) Kinematics of a particle moving in a straight line or plane Acceleration (a) You can use calculus for a particle moving in a straight line, with acceleration that varies with time A particle P is moving along a straight line. At time t = 0, the particle is at a point A and is moving with velocity 8 ms-1 towards a point B on the line, where AB = 30 m. At time t seconds, where t ≥ 0, the acceleration of P is (2 – 2 t)ms-2 in the direction AB. a) Find an expression, in terms of t, for the displacement of P from A at time t seconds b) Integrate We know at time t = 0, the velocity is 8 Use these to find the value of C Show that P does not reach B c) Find the value of t when P returns to A d) Find the total distance travelled by P in the interval between the two instances when it passes through A The integral of a is v, simplify the fraction Sub in values Calculate C We can now put the value C = 8 into our equation for velocity 1 B

Displacement (x) Velocity (v) Kinematics of a particle moving in a straight line or plane Acceleration (a) You can use calculus for a particle moving in a straight line, with acceleration that varies with time A particle P is moving along a straight line. At time t = 0, the particle is at a point A and is moving with velocity 8 ms-1 towards a point B on the line, where AB = 30 m. At time t seconds, where t ≥ 0, the acceleration of P is (2 – 2 t)ms-2 in the direction AB. a) Find an expression, in terms of t, for the displacement of P from A at time t seconds b) Show that P does not reach B c) Find the value of t when P returns to A d) Find the total distance travelled by P in the interval between the two instances when it passes through A Integrate v now to get a formula for the displacement x The integral of v is x, simplify the fraction At time t = 0, the particle is a point A We can take A as the starting displacement – ie) 0 Sub in t = 0, x=0 Calculate D So we do not need to include a constant in our formula for x 1 B

Displacement (x) Velocity (v) Kinematics of a particle moving in a straight line or plane Acceleration (a) You can use calculus for a particle moving in a straight line, with acceleration that varies with time A particle P is moving along a straight line. At time t = 0, the particle is at a point A and is moving with velocity 8 ms-1 towards a point B on the line, where AB = 30 m. At time t seconds, where t ≥ 0, the acceleration of P is (2 – 2 t)ms-2 in the direction AB. a) Find an expression, in terms of t, for the displacement of P from A at time t seconds b) c) d) Show that P does not reach B Find the value of t when P returns to A Find the total distance travelled by P in the interval between the two instances when it passes through A Show that P does not reach B Calculate the furthest distance P gets from A This will occur at the time where the velocity is instantaneously 0 Set v = 0 and start solving for t Rearrange to an easier quadratic form Factorise 2 values for t Only the positive value is possible Now calculate the displacement at time t = 2 As the maximum distance is 262/3 m, and AB is 30 m, P will never reach B Sub in t = 2 Work out the displacement 1 B

Displacement (x) Velocity (v) Kinematics of a particle moving in a straight line or plane Acceleration (a) You can use calculus for a particle moving in a straight line, with acceleration that varies with time A particle P is moving along a straight line. At time t = 0, the particle is at a point A and is moving with velocity 8 ms-1 towards a point B on the line, where AB = 30 m. At time t seconds, where t ≥ 0, the acceleration of P is (2 – 2 t)ms-2 in the direction AB. a) Find an expression, in terms of t, for the displacement of P from A at time t seconds b) Show that P does not reach B Max displacement = 26 2/3 c) Find the value of t when P returns to A d) Find the total distance travelled by P in the interval between the two instances when it passes through A Find the value of t when P returns to A This means the displacement from A will be 0 Sub in x = 0 Multiply by 3 to remove the fraction Rearrange to have t 3 as positive Factorise Either inside the bracket or outside it must be 0 This is because the particle starts at A Use the Quadratic formula to solve this part Sub in values Calculate So the particle returns to A 6. 62 seconds after leaving it! 1 B

Displacement (x) Velocity (v) Kinematics of a particle moving in a straight line or plane Acceleration (a) You can use calculus for a particle moving in a straight line, with acceleration that varies with time A particle P is moving along a straight line. At time t = 0, the particle is at a point A and is moving with velocity 8 ms-1 towards a point B on the line, where AB = 30 m. At time t seconds, where t ≥ 0, the acceleration of P is (2 – 2 t)ms-2 in the direction AB. a) Find an expression, in terms of t, for the displacement of P from A at time t seconds The particle starts at A, moves 262/3 m away and then comes back The total distance travelled in this time is therefore just given by: 2 x 262/3 = 531/3 m b) c) Show that P does not reach B Max displacement = 26 2/3 Find the value of t when P returns to A 6. 62 seconds d) Find the total distance travelled by P in the interval between the two instances when it passes through A 1 B

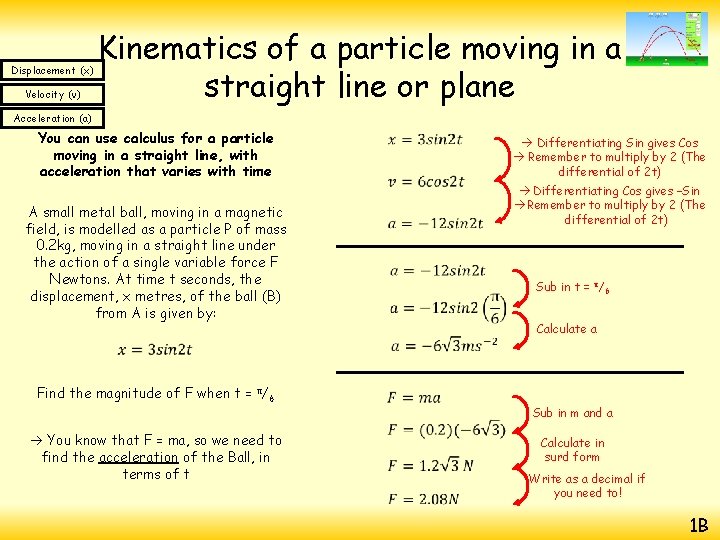
Displacement (x) Velocity (v) Kinematics of a particle moving in a straight line or plane Acceleration (a) You can use calculus for a particle moving in a straight line, with acceleration that varies with time A small metal ball, moving in a magnetic field, is modelled as a particle P of mass 0. 2 kg, moving in a straight line under the action of a single variable force F Newtons. At time t seconds, the displacement, x metres, of the ball (B) from A is given by: Find the magnitude of F when t = π/6 You know that F = ma, so we need to find the acceleration of the Ball, in terms of t Differentiating Sin gives Cos Remember to multiply by 2 (The differential of 2 t) Differentiating Cos gives –Sin Remember to multiply by 2 (The differential of 2 t) Sub in t = π/6 Calculate a Sub in m and a Calculate in surd form Write as a decimal if you need to! 1 B

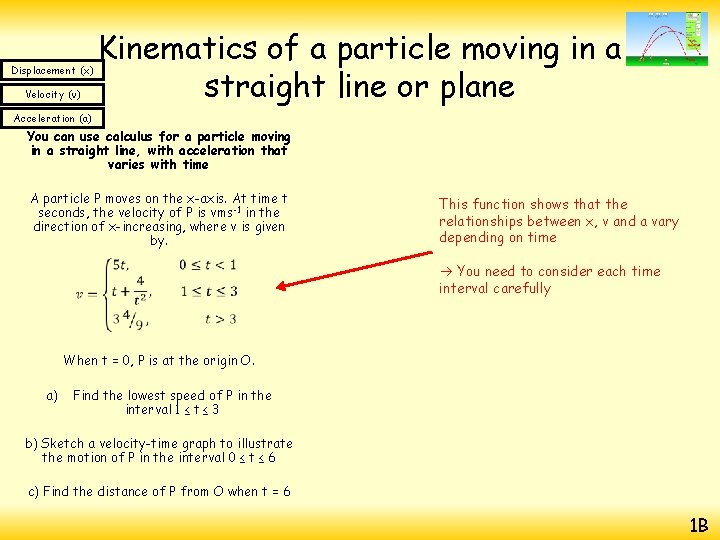
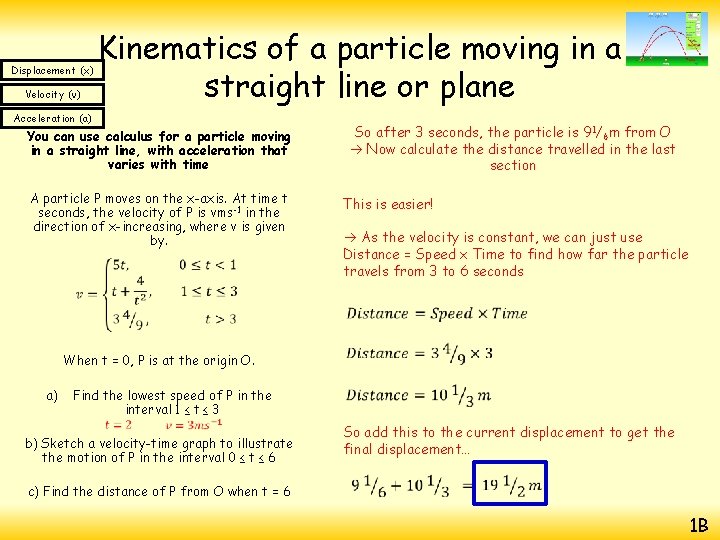
Displacement (x) Velocity (v) Kinematics of a particle moving in a straight line or plane Acceleration (a) You can use calculus for a particle moving in a straight line, with acceleration that varies with time A particle P moves on the x-axis. At time t seconds, the velocity of P is vms -1 in the direction of x-increasing, where v is given by. This function shows that the relationships between x, v and a vary depending on time You need to consider each time interval carefully When t = 0, P is at the origin O. a) Find the lowest speed of P in the interval 1 ≤ t ≤ 3 b) Sketch a velocity-time graph to illustrate the motion of P in the interval 0 ≤ t ≤ 6 c) Find the distance of P from O when t = 6 1 B

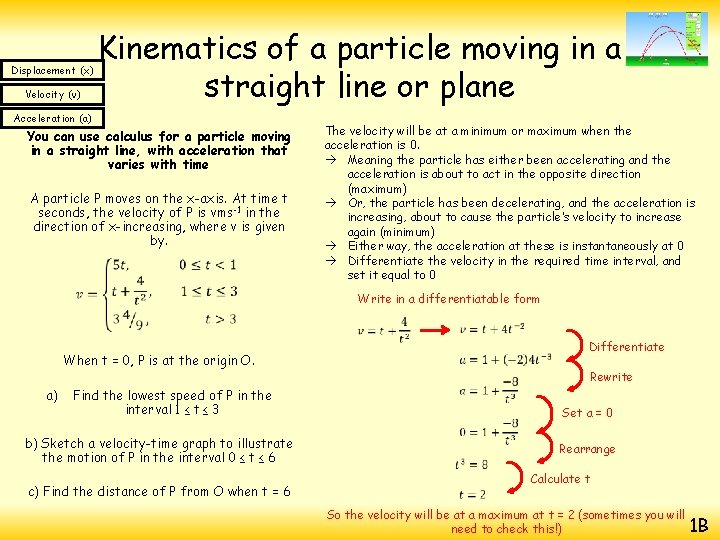
Displacement (x) Velocity (v) Kinematics of a particle moving in a straight line or plane Acceleration (a) You can use calculus for a particle moving in a straight line, with acceleration that varies with time A particle P moves on the x-axis. At time t seconds, the velocity of P is vms -1 in the direction of x-increasing, where v is given by. The velocity will be at a minimum or maximum when the acceleration is 0. Meaning the particle has either been accelerating and the acceleration is about to act in the opposite direction (maximum) Or, the particle has been decelerating, and the acceleration is increasing, about to cause the particle’s velocity to increase again (minimum) Either way, the acceleration at these is instantaneously at 0 Differentiate the velocity in the required time interval, and set it equal to 0 Write in a differentiatable form When t = 0, P is at the origin O. a) Find the lowest speed of P in the interval 1 ≤ t ≤ 3 b) Sketch a velocity-time graph to illustrate the motion of P in the interval 0 ≤ t ≤ 6 c) Find the distance of P from O when t = 6 Differentiate Rewrite Set a = 0 Rearrange Calculate t So the velocity will be at a maximum at t = 2 (sometimes you will need to check this!) 1 B

Displacement (x) Velocity (v) Kinematics of a particle moving in a straight line or plane Acceleration (a) You can use calculus for a particle moving in a straight line, with acceleration that varies with time A particle P moves on the x-axis. At time t seconds, the velocity of P is vms -1 in the direction of x-increasing, where v is given by. Sub in t = 2 Calculate When t = 0, P is at the origin O. a) Sub t = 2 into the relevant equation for velocity As this was the only value it must be the answer with more than 1 answer you could sub both in and check which gives the biggest/smallest velocity Find the lowest speed of P in the interval 1 ≤ t ≤ 3 b) Sketch a velocity-time graph to illustrate the motion of P in the interval 0 ≤ t ≤ 6 c) Find the distance of P from O when t = 6 1 B

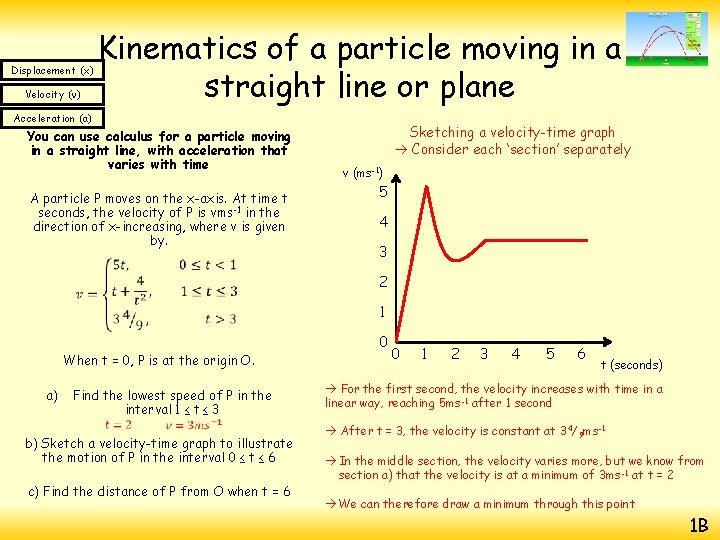
Displacement (x) Velocity (v) Kinematics of a particle moving in a straight line or plane Acceleration (a) You can use calculus for a particle moving in a straight line, with acceleration that varies with time A particle P moves on the x-axis. At time t seconds, the velocity of P is vms -1 in the direction of x-increasing, where v is given by. Sketching a velocity-time graph Consider each ‘section’ separately v (ms-1) 5 4 3 2 1 0 When t = 0, P is at the origin O. a) Find the lowest speed of P in the interval 1 ≤ t ≤ 3 b) Sketch a velocity-time graph to illustrate the motion of P in the interval 0 ≤ t ≤ 6 c) Find the distance of P from O when t = 6 0 1 2 3 4 5 6 t (seconds) For the first second, the velocity increases with time in a linear way, reaching 5 ms -1 after 1 second After t = 3, the velocity is constant at 3 4/9 ms-1 In the middle section, the velocity varies more, but we know from section a) that the velocity is at a minimum of 3 ms -1 at t = 2 We can therefore draw a minimum through this point 1 B

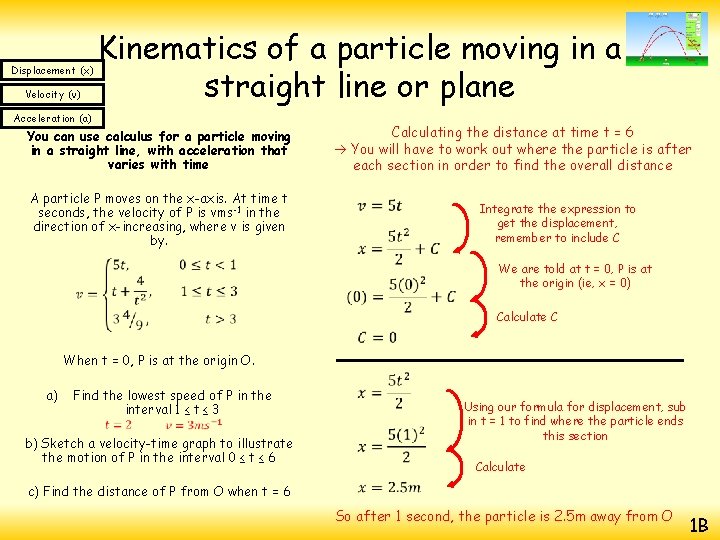
Displacement (x) Velocity (v) Kinematics of a particle moving in a straight line or plane Acceleration (a) You can use calculus for a particle moving in a straight line, with acceleration that varies with time Calculating the distance at time t = 6 You will have to work out where the particle is after each section in order to find the overall distance A particle P moves on the x-axis. At time t seconds, the velocity of P is vms -1 in the direction of x-increasing, where v is given by. Integrate the expression to get the displacement, remember to include C We are told at t = 0, P is at the origin (ie, x = 0) Calculate C When t = 0, P is at the origin O. a) Find the lowest speed of P in the interval 1 ≤ t ≤ 3 b) Sketch a velocity-time graph to illustrate the motion of P in the interval 0 ≤ t ≤ 6 c) Find the distance of P from O when t = 6 Using our formula for displacement, sub in t = 1 to find where the particle ends this section Calculate So after 1 second, the particle is 2. 5 m away from O 1 B

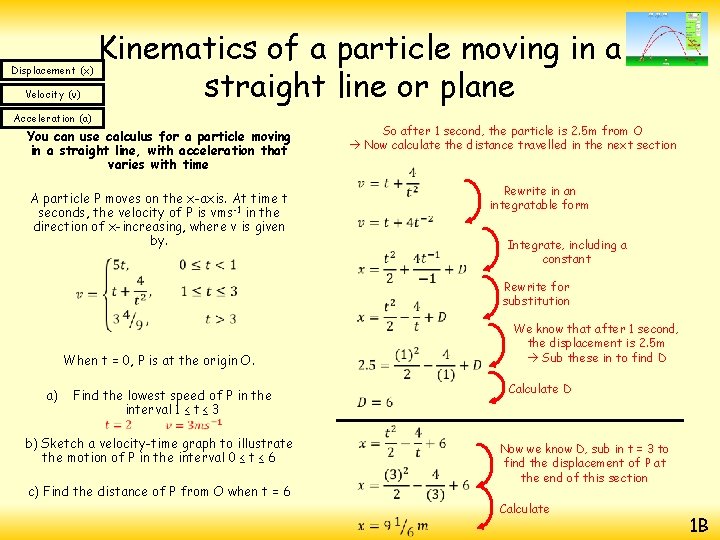
Displacement (x) Velocity (v) Kinematics of a particle moving in a straight line or plane Acceleration (a) You can use calculus for a particle moving in a straight line, with acceleration that varies with time A particle P moves on the x-axis. At time t seconds, the velocity of P is vms -1 in the direction of x-increasing, where v is given by. So after 1 second, the particle is 2. 5 m from O Now calculate the distance travelled in the next section When t = 0, P is at the origin O. a) Find the lowest speed of P in the interval 1 ≤ t ≤ 3 b) Sketch a velocity-time graph to illustrate the motion of P in the interval 0 ≤ t ≤ 6 c) Find the distance of P from O when t = 6 Rewrite in an integratable form Integrate, including a constant Rewrite for substitution We know that after 1 second, the displacement is 2. 5 m Sub these in to find D Calculate D Now we know D, sub in t = 3 to find the displacement of P at the end of this section Calculate 1 B

Displacement (x) Velocity (v) Kinematics of a particle moving in a straight line or plane Acceleration (a) So after 3 seconds, the particle is 91/6 m from O Now calculate the distance travelled in the last section You can use calculus for a particle moving in a straight line, with acceleration that varies with time A particle P moves on the x-axis. At time t seconds, the velocity of P is vms -1 in the direction of x-increasing, where v is given by. This is easier! As the velocity is constant, we can just use Distance = Speed x Time to find how far the particle travels from 3 to 6 seconds When t = 0, P is at the origin O. a) Find the lowest speed of P in the interval 1 ≤ t ≤ 3 b) Sketch a velocity-time graph to illustrate the motion of P in the interval 0 ≤ t ≤ 6 c) Find the distance of P from O when t = 6 So add this to the current displacement to get the final displacement… 1 B

Teachings for Exercise 1 C

Kinematics of a particle moving in a straight line or plane You can use calculus with vectors for a particle moving in a plane (up to now was just along a straight Differentiate line!) When a particle is moving in a plane you can describe its position (r), its velocity (v) and its acceleration (a) using vectors. When you integrate using vectors, the constant of integration (C) will also be a vector Integrate Velocity Vector (v) Differentiate The relationships between these in two dimensions are the same as they are in one dimension Sometimes dot notation is used. A single dot means differentiate once and two dots means differentiate twice. Position Vector (r) Integrate Acceleration Vector (a) Dot notation used for a single differentiation Dot notation used for a double differentiation 1 C

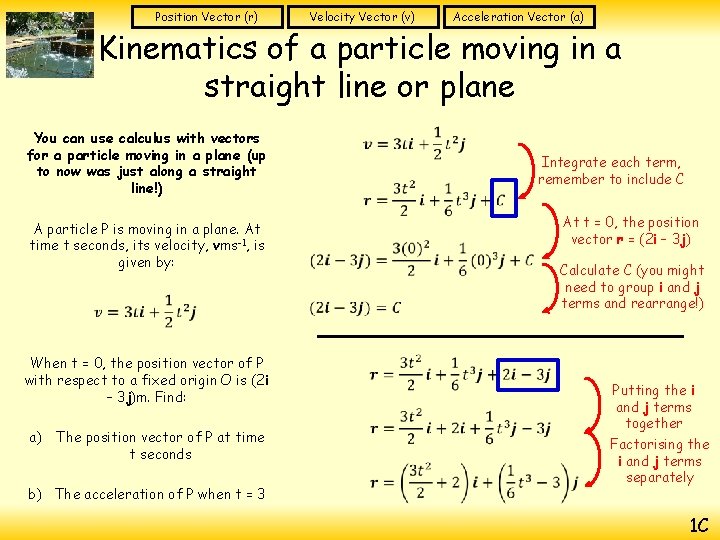
Position Vector (r) Velocity Vector (v) Acceleration Vector (a) Kinematics of a particle moving in a straight line or plane You can use calculus with vectors for a particle moving in a plane (up to now was just along a straight line!) A particle P is moving in a plane. At time t seconds, its velocity, vms-1, is given by: When t = 0, the position vector of P with respect to a fixed origin O is (2 i – 3 j)m. Find: a) The position vector of P at time t seconds b) The acceleration of P when t = 3 Integrate each term, remember to include C At t = 0, the position vector r = (2 i – 3 j) Calculate C (you might need to group i and j terms and rearrange!) Putting the i and j terms together Factorising the i and j terms separately 1 C

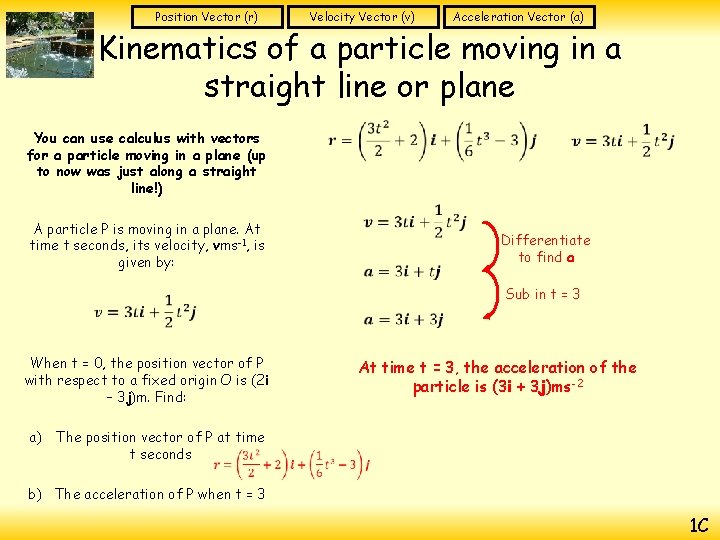
Position Vector (r) Velocity Vector (v) Acceleration Vector (a) Kinematics of a particle moving in a straight line or plane You can use calculus with vectors for a particle moving in a plane (up to now was just along a straight line!) A particle P is moving in a plane. At time t seconds, its velocity, vms-1, is given by: When t = 0, the position vector of P with respect to a fixed origin O is (2 i – 3 j)m. Find: Differentiate to find a Sub in t = 3 At time t = 3, the acceleration of the particle is (3 i + 3 j)ms-2 a) The position vector of P at time t seconds b) The acceleration of P when t = 3 1 C

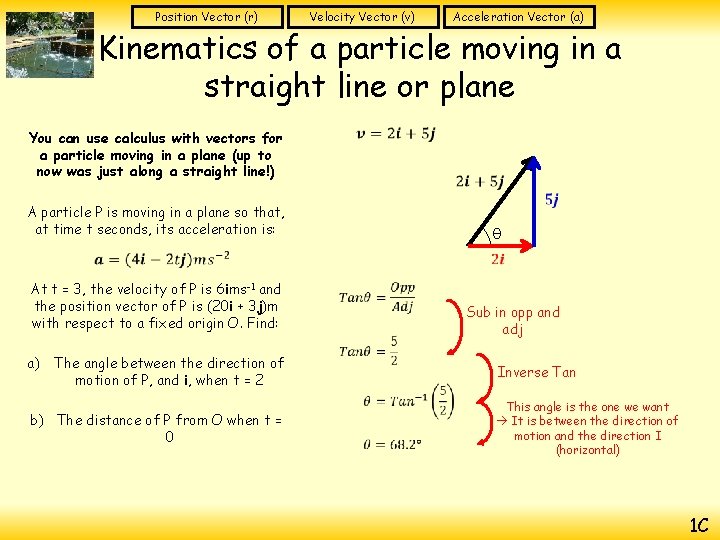
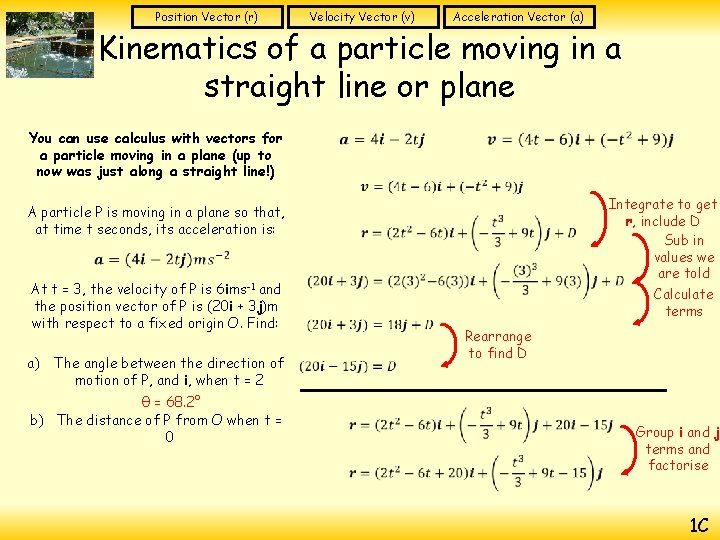
Position Vector (r) Velocity Vector (v) Acceleration Vector (a) Kinematics of a particle moving in a straight line or plane You can use calculus with vectors for a particle moving in a plane (up to now was just along a straight line!) A particle P is moving in a plane so that, at time t seconds, its acceleration is: At t = 3, the velocity of P is 6 ims-1 and the position vector of P is (20 i + 3 j)m with respect to a fixed origin O. Find: a) The angle between the direction of motion of P, and i, when t = 2 b) The distance of P from O when t = 0 Integrate to get v, include C At t = 3, we know the velocity is 6 i Calculate terms Rearrange to get the missing vector C Group i and j terms and factorise Sub in t = 2 to find the velocity vector Calculate terms 1 C

Position Vector (r) Velocity Vector (v) Acceleration Vector (a) Kinematics of a particle moving in a straight line or plane You can use calculus with vectors for a particle moving in a plane (up to now was just along a straight line!) A particle P is moving in a plane so that, at time t seconds, its acceleration is: θ At t = 3, the velocity of P is 6 ims-1 and the position vector of P is (20 i + 3 j)m with respect to a fixed origin O. Find: a) The angle between the direction of motion of P, and i, when t = 2 b) The distance of P from O when t = 0 Sub in opp and adj Inverse Tan This angle is the one we want It is between the direction of motion and the direction I (horizontal) 1 C

Position Vector (r) Velocity Vector (v) Acceleration Vector (a) Kinematics of a particle moving in a straight line or plane You can use calculus with vectors for a particle moving in a plane (up to now was just along a straight line!) A particle P is moving in a plane so that, at time t seconds, its acceleration is: 6 ims-1 At t = 3, the velocity of P is and the position vector of P is (20 i + 3 j)m with respect to a fixed origin O. Find: a) The angle between the direction of motion of P, and i, when t = 2 θ = 68. 2° b) The distance of P from O when t = 0 Integrate to get r, include D Sub in values we are told Calculate terms Rearrange to find D Group i and j terms and factorise 1 C

Position Vector (r) Velocity Vector (v) Acceleration Vector (a) Kinematics of a particle moving in a straight line or plane You can use calculus with vectors for a particle moving in a plane (up to now was just along a straight line!) A particle P is moving in a plane so that, at time t seconds, its acceleration is: At t = 3, the velocity of P is 6 ims-1 and the position vector of P is (20 i + 3 j)m with respect to a fixed origin O. Find: a) The angle between the direction of motion of P, and i, when t = 2 θ = 68. 2° b) The distance of P from O when t = 0 Sub in t=0 Calculate r The initial position vector r, is just the value of the constant of integration! 1 C

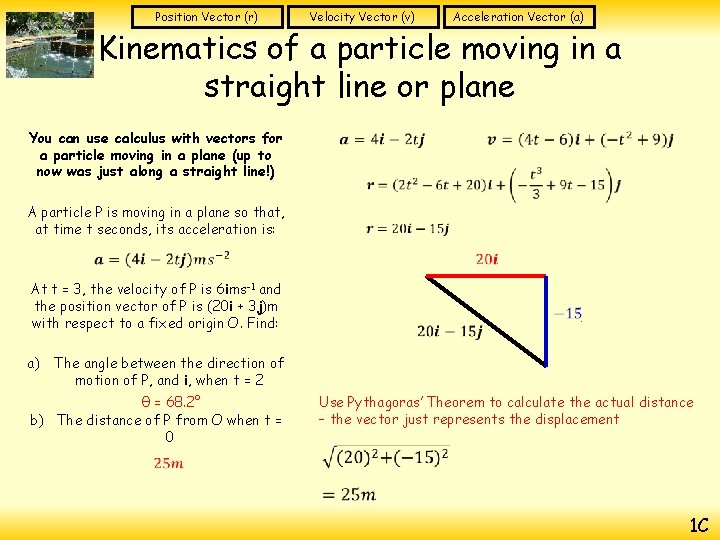
Position Vector (r) Velocity Vector (v) Acceleration Vector (a) Kinematics of a particle moving in a straight line or plane You can use calculus with vectors for a particle moving in a plane (up to now was just along a straight line!) A particle P is moving in a plane so that, at time t seconds, its acceleration is: At t = 3, the velocity of P is 6 ims-1 and the position vector of P is (20 i + 3 j)m with respect to a fixed origin O. Find: a) The angle between the direction of motion of P, and i, when t = 2 θ = 68. 2° b) The distance of P from O when t = 0 Use Pythagoras’ Theorem to calculate the actual distance – the vector just represents the displacement 1 C

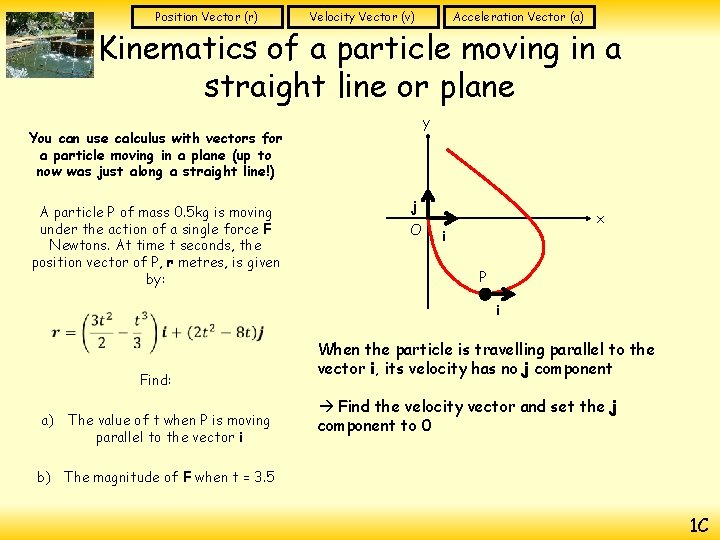
Position Vector (r) Velocity Vector (v) Acceleration Vector (a) Kinematics of a particle moving in a straight line or plane y You can use calculus with vectors for a particle moving in a plane (up to now was just along a straight line!) A particle P of mass 0. 5 kg is moving under the action of a single force F Newtons. At time t seconds, the position vector of P, r metres, is given by: j O x i P i Find: a) The value of t when P is moving parallel to the vector i When the particle is travelling parallel to the vector i, its velocity has no j component Find the velocity vector and set the j component to 0 b) The magnitude of F when t = 3. 5 1 C

Position Vector (r) Velocity Vector (v) Acceleration Vector (a) Kinematics of a particle moving in a straight line or plane You can use calculus with vectors for a particle moving in a plane (up to now was just along a straight line!) A particle P of mass 0. 5 kg is moving under the action of a single force F Newtons. At time t seconds, the position vector of P, r metres, is given by: Differentiate to get the velocity vector We want the j term of the velocity to be equal to 0 Solve for t Find: a) The value of t when P is moving parallel to the vector i b) The magnitude of F when t = 3. 5 1 C

Position Vector (r) Velocity Vector (v) Acceleration Vector (a) Kinematics of a particle moving in a straight line or plane You can use calculus with vectors for a particle moving in a plane (up to now was just along a straight line!) We can use the formula F = ma to find the force, but first we will need to find the acceleration at the appropriate time! A particle P of mass 0. 5 kg is moving under the action of a single force F Newtons. At time t seconds, the position vector of P, r metres, is given by: Differentiate to get the acceleration vector Sub in t = 3. 5 Calculate a Find: a) The value of t when P is moving parallel to the vector i b) The magnitude of F when t = 3. 5 Sub in m and a Calculate F in terms of i and j Use Pythagoras’ to find the magnitude Calculate F 1 C

Position Vector (r) Velocity Vector (v) Acceleration Vector (a) Kinematics of a particle moving in a straight line or plane You can use calculus with vectors for a particle moving in a plane (up to now was just along a straight line!) The velocity of a particle at time t seconds is given by: Integrate to find r, include C Sub in the values given Find C When t = 0, the position vector of P with respect to a fixed origin is (2 i – 4 j)m a) Find the position vector of P after t seconds Sub in the value of C we found Group i and j terms and factorise 1 C

Position Vector (r) Velocity Vector (v) Acceleration Vector (a) Kinematics of a particle moving in a straight line or plane You can use calculus with vectors for a particle moving in a plane (up to now was just along a straight line!) The velocity of a particle at time t seconds is given by: When t = 0, the position vector of P with respect to a fixed origin is (2 i – 4 j)m a) Find the position vector of P after t seconds A second particle Q moves with constant velocity (8 i + 4 j)ms-1. When t = 0, the position vector of Q with respect to the origin O is 2 i m. b) Prove that P and Q collide If the particles collide, there must be a time for which their i and j components are both the same Find the time for which either the i’s or j’s are equal, and check if it works for the other component Start by finding an equation for the position vector of Q in terms of t Integrate to find r, include D Sub in the values given Find D Sub in the value of D we have Group i and j terms and factorise 1 C

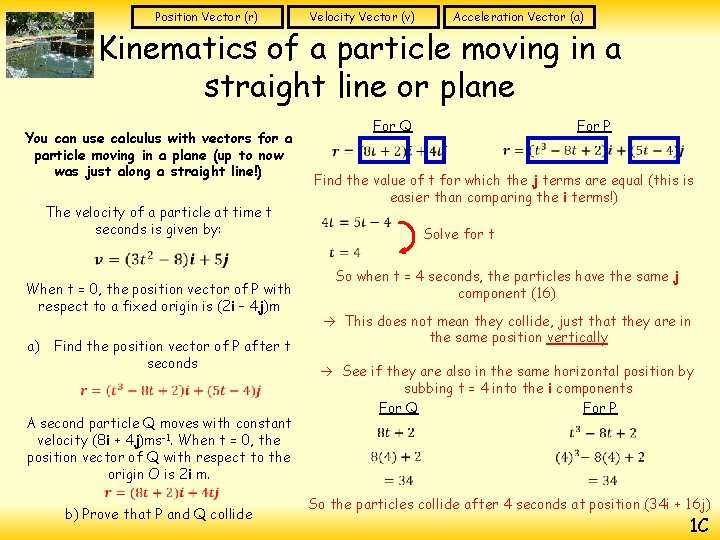
Position Vector (r) Velocity Vector (v) Acceleration Vector (a) Kinematics of a particle moving in a straight line or plane You can use calculus with vectors for a particle moving in a plane (up to now was just along a straight line!) The velocity of a particle at time t seconds is given by: When t = 0, the position vector of P with respect to a fixed origin is (2 i – 4 j)m a) Find the position vector of P after t seconds A second particle Q moves with constant velocity (8 i + 4 j)ms-1. When t = 0, the position vector of Q with respect to the origin O is 2 i m. b) Prove that P and Q collide For Q For P Find the value of t for which the j terms are equal (this is easier than comparing the i terms!) Solve for t So when t = 4 seconds, the particles have the same j component (16) This does not mean they collide, just that they are in the same position vertically See if they are also in the same horizontal position by subbing t = 4 into the i components For Q For P So the particles collide after 4 seconds at position (34 i + 16 j) 1 C

Summary • We have learnt how to solve problems involving projectiles • We have used calculus to solve problems involving displacements, velocities and accelerations that vary with time • We have also seen how vectors are used to model movement in a 2 D plane!