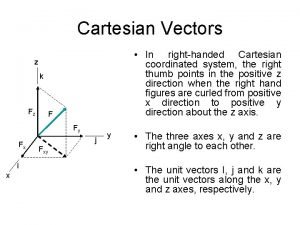
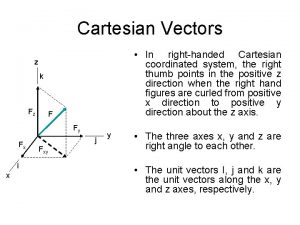
COORDINATE SYSTEMS TRANSFORMATION 1 6 CARTESIAN COORDINATES 1















- Slides: 48

COORDINATE SYSTEMS & TRANSFORMATION 1. 6 CARTESIAN COORDINATES 1. 7 CIRCULAR CYLINDRICAL COORDINATES 1. 8 SPHERICAL COORDINATES 1. 9 TRANSFORMATION BETWEEN COORDINATE SYSTEMS

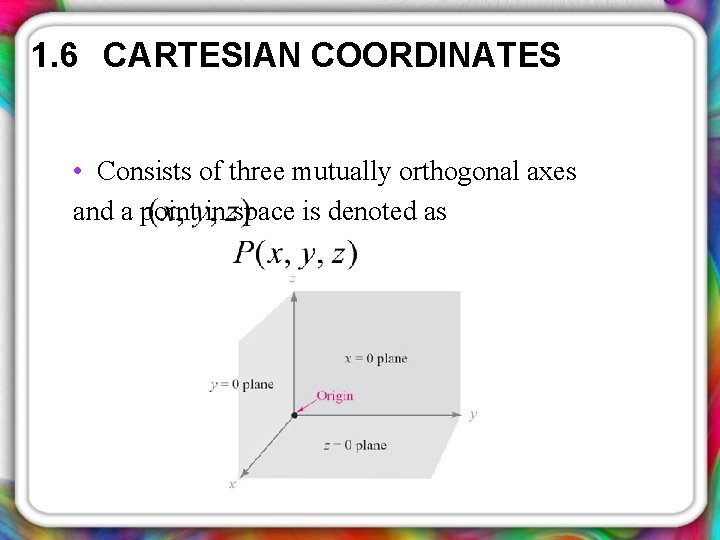
1. 6 CARTESIAN COORDINATES • Consists of three mutually orthogonal axes and a point in space is denoted as

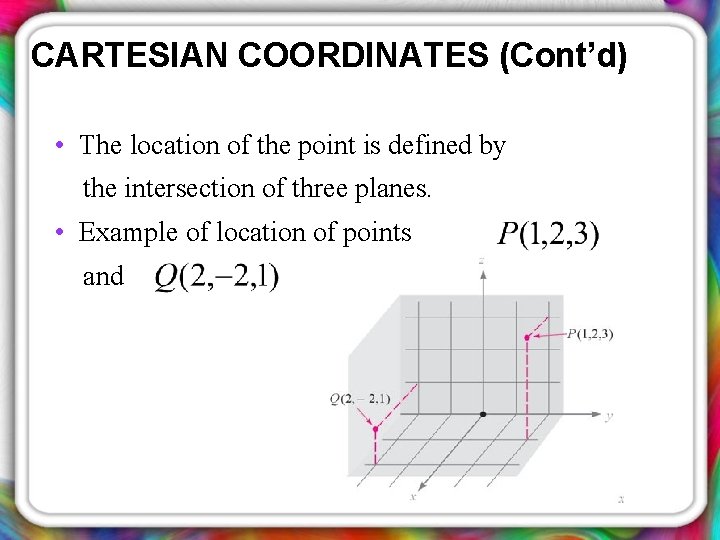
CARTESIAN COORDINATES (Cont’d) • The location of the point is defined by the intersection of three planes. • Example of location of points and

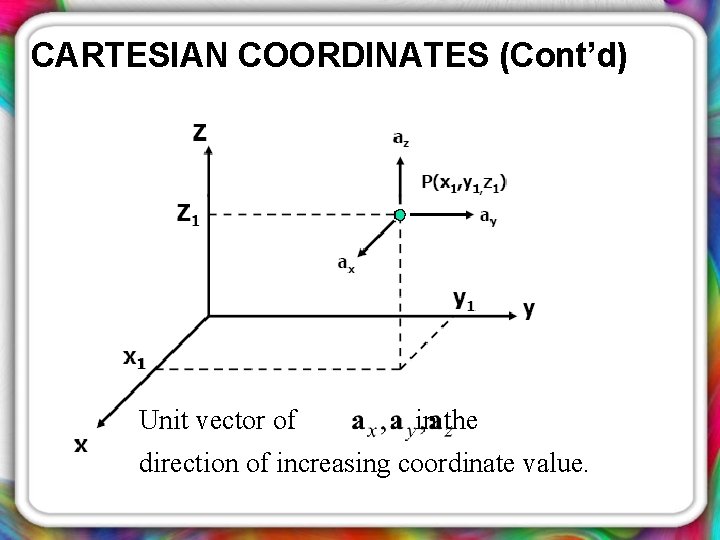
CARTESIAN COORDINATES (Cont’d) Unit vector of in the direction of increasing coordinate value.

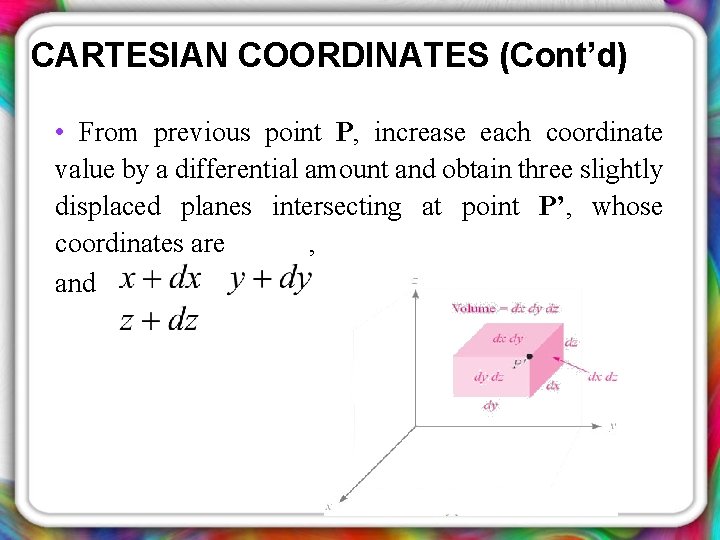
CARTESIAN COORDINATES (Cont’d) • From previous point P, increase each coordinate value by a differential amount and obtain three slightly displaced planes intersecting at point P’, whose coordinates are , and

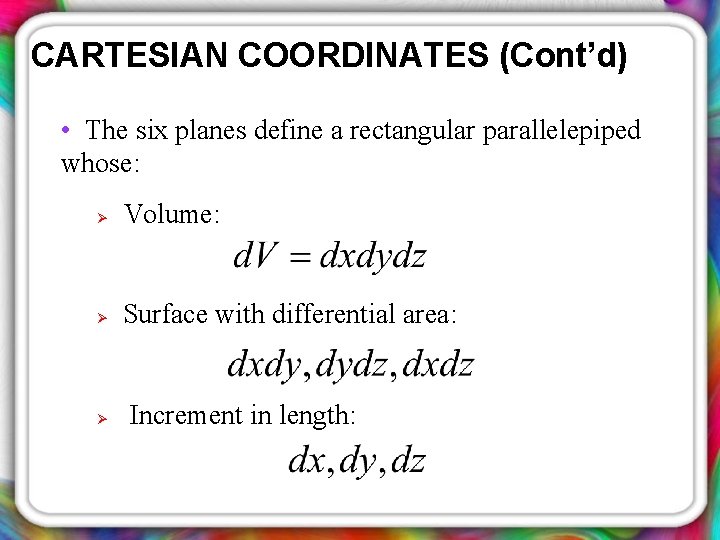
CARTESIAN COORDINATES (Cont’d) • The six planes define a rectangular parallelepiped whose: Ø Volume: Ø Surface with differential area: Ø Increment in length:

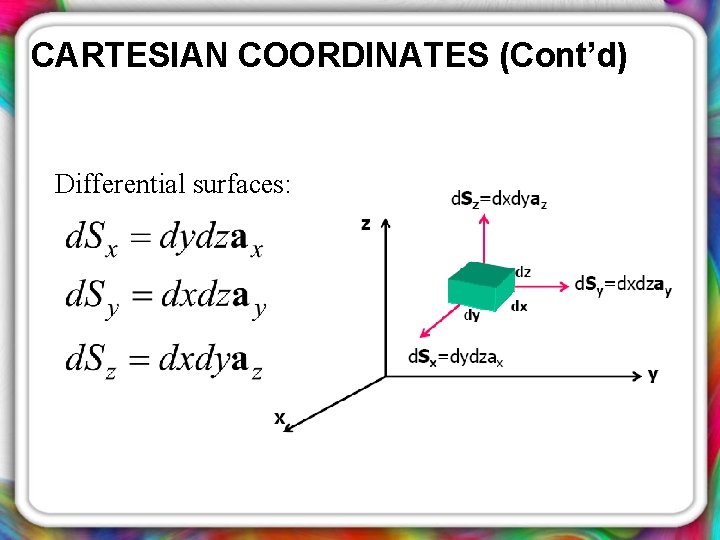
CARTESIAN COORDINATES (Cont’d) Differential surfaces:

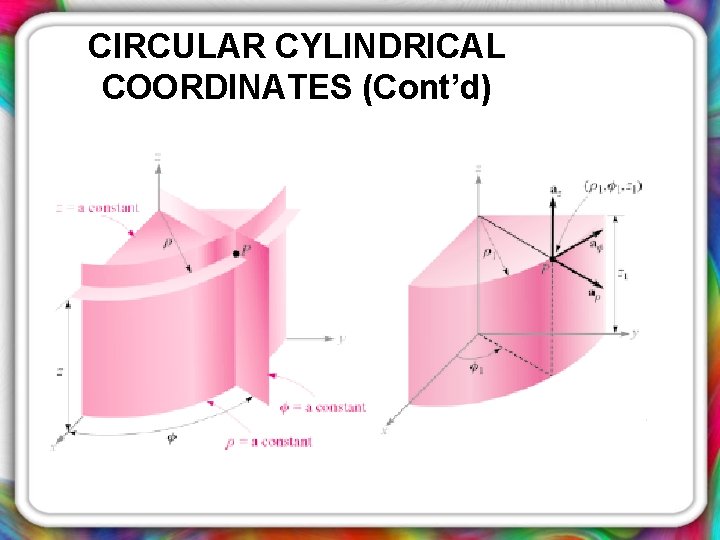
1. 7 CIRCULAR CYLINDRICAL COORDINATES • Form by three surfaces or planes: Ø Plane of z (constant value of z) Ø Cylinder centered on the z axis with radius of Plane perpendicular to x-y plane and rotate about the z axis by angle of Ø • Unit vector of in the direction of increasing coordinate value.

CIRCULAR CYLINDRICAL COORDINATES (Cont’d)

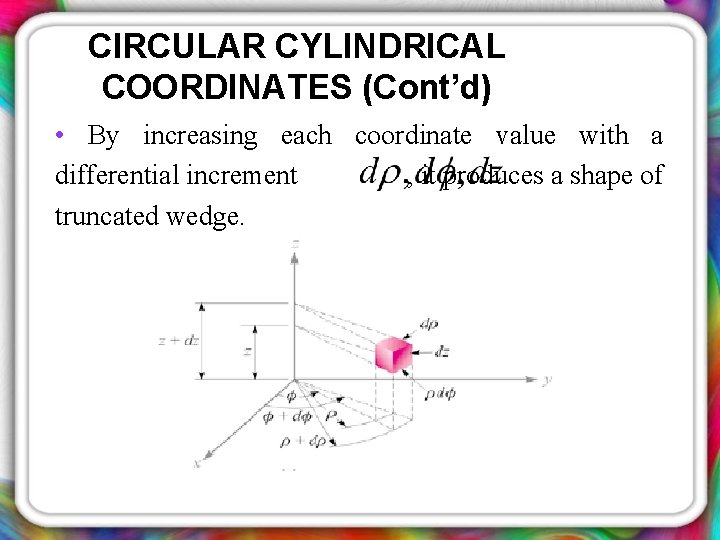
CIRCULAR CYLINDRICAL COORDINATES (Cont’d) • By increasing each coordinate value with a differential increment , it produces a shape of truncated wedge.

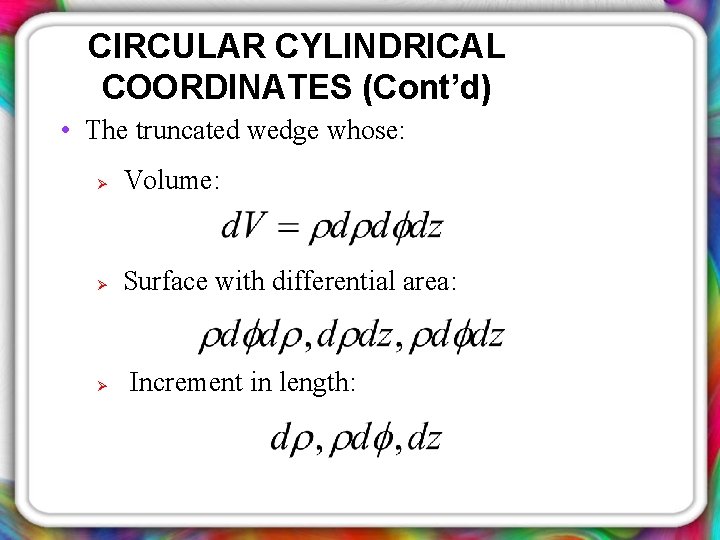
CIRCULAR CYLINDRICAL COORDINATES (Cont’d) • The truncated wedge whose: Ø Volume: Ø Surface with differential area: Ø Increment in length:

CIRCULAR CYLINDRICAL COORDINATES (Cont’d) Remember ? ! Increment in length for direction: is not increment in length!

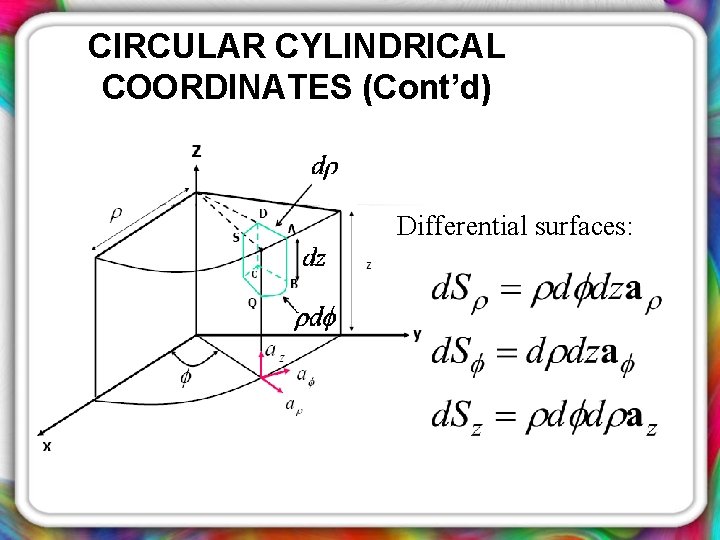
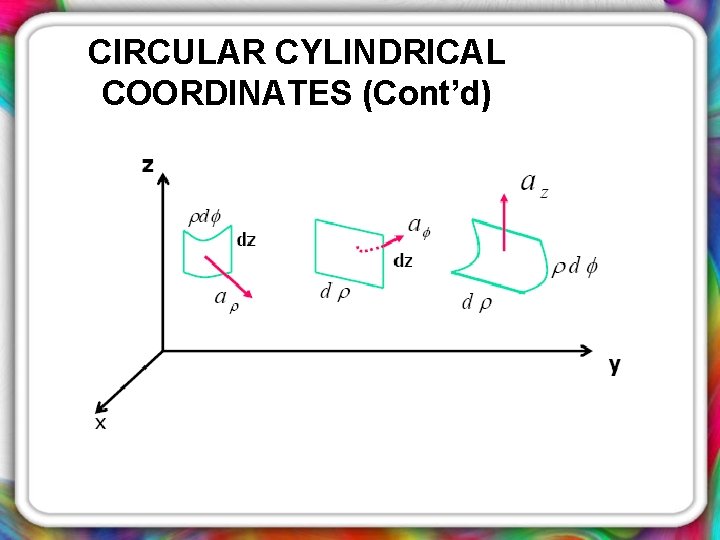
CIRCULAR CYLINDRICAL COORDINATES (Cont’d) Differential surfaces:

CIRCULAR CYLINDRICAL COORDINATES (Cont’d)

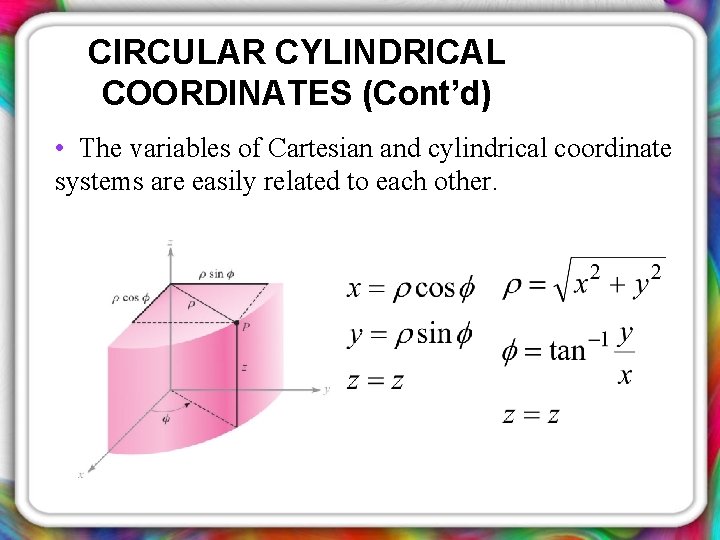
CIRCULAR CYLINDRICAL COORDINATES (Cont’d) • The variables of Cartesian and cylindrical coordinate systems are easily related to each other.

EXAMPLE 5 A cylinder with radius of Determine: and length of (i) The volume enclosed. (ii) The surface area of that volume. .

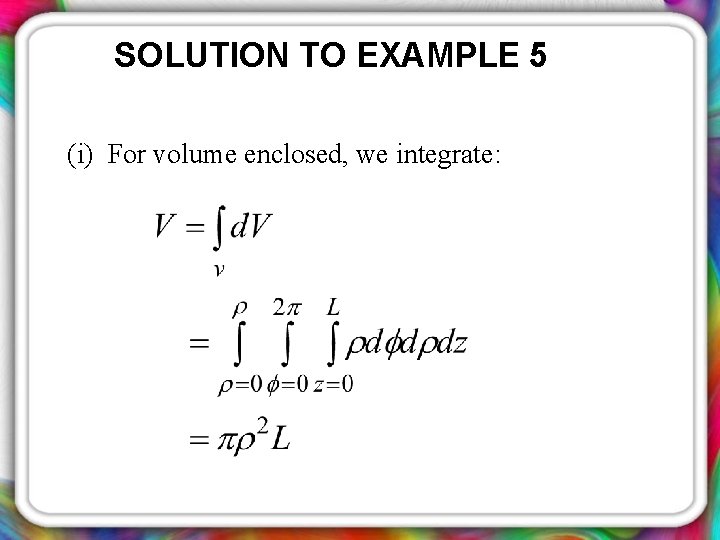
SOLUTION TO EXAMPLE 5 (i) For volume enclosed, we integrate:

SOLUTION TO EXAMPLE 5 (Cont’d) (ii) For surface area of the volume:

EXAMPLE 6 The surfaces define a closed surface. Find: (a) The enclosed volume. (b) The total area of the enclosing surface. (a) The length of the longest straight (b) line that lies entirely within the (c) volume.

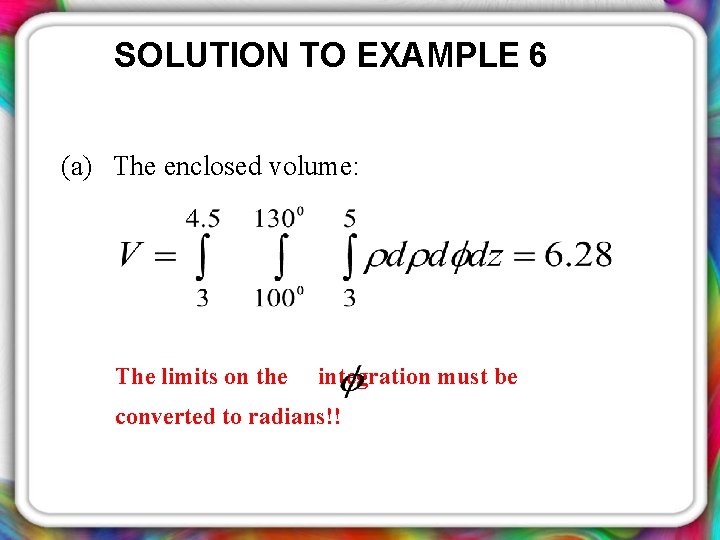
SOLUTION TO EXAMPLE 6 (a) The enclosed volume: The limits on the integration must be converted to radians!!

SOLUTION TO EXAMPLE 6 (Cont’d) (b) The total area of the enclosed surface:

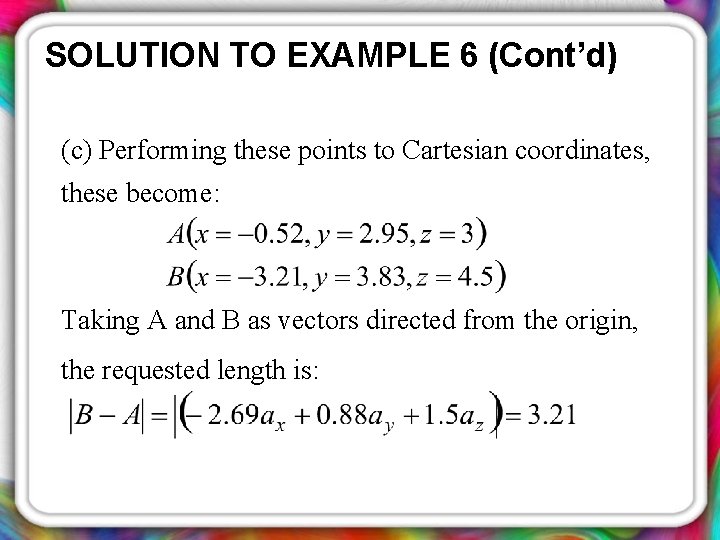
SOLUTION TO EXAMPLE 6 (Cont’d) (c) Performing these points to Cartesian coordinates, these become: Taking A and B as vectors directed from the origin, the requested length is:

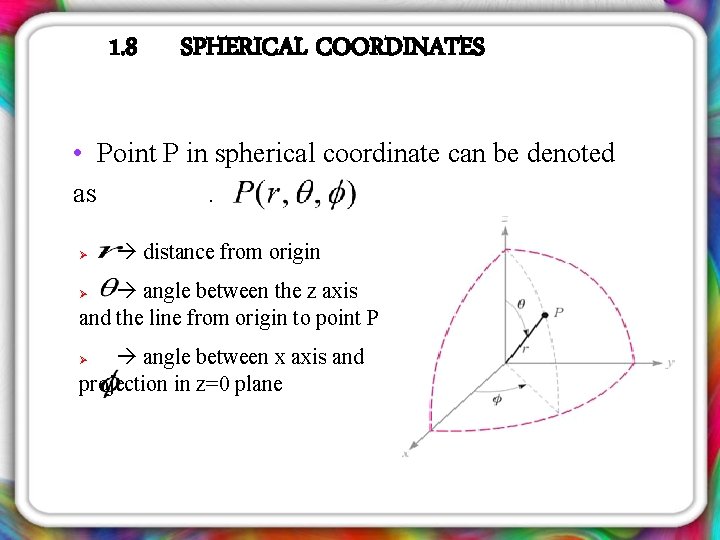
1. 8 SPHERICAL COORDINATES • Point P in spherical coordinate can be denoted as. Ø distance from origin angle between the z axis and the line from origin to point P Ø angle between x axis and projection in z=0 plane Ø

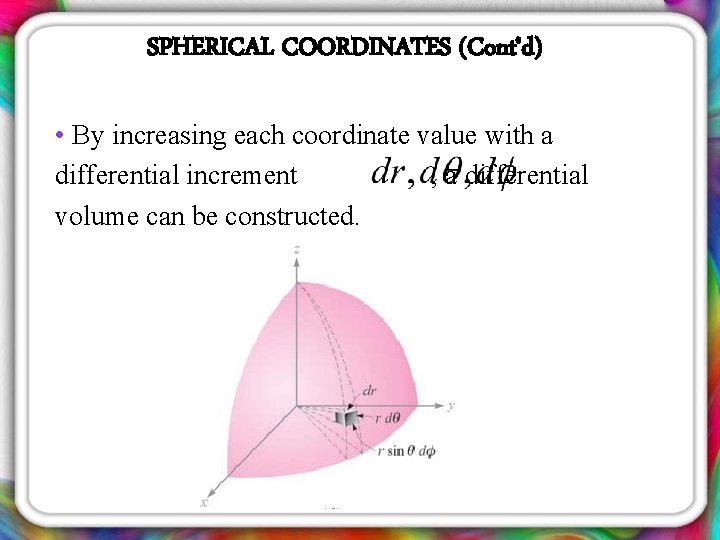
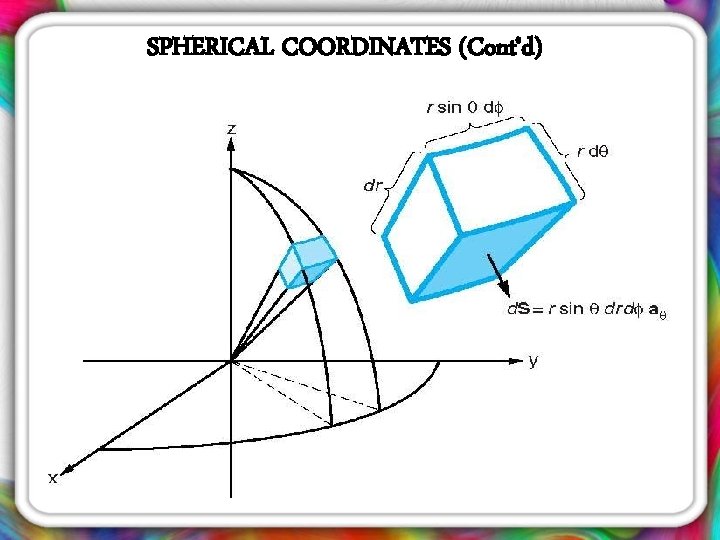
SPHERICAL COORDINATES (Cont’d) • By increasing each coordinate value with a differential increment , a differential volume can be constructed.

SPHERICAL COORDINATES (Cont’d)

SPHERICAL COORDINATES (Cont’d) • However, the increment of length is different from the differential increment previously, where: Ø distance between two radius Ø distance between two angles Ø distance between two radial planes at angles

SPHERICAL COORDINATES (Cont’d) • It has: Ø Volume: Ø Surface with differential area: Ø Increment in length:

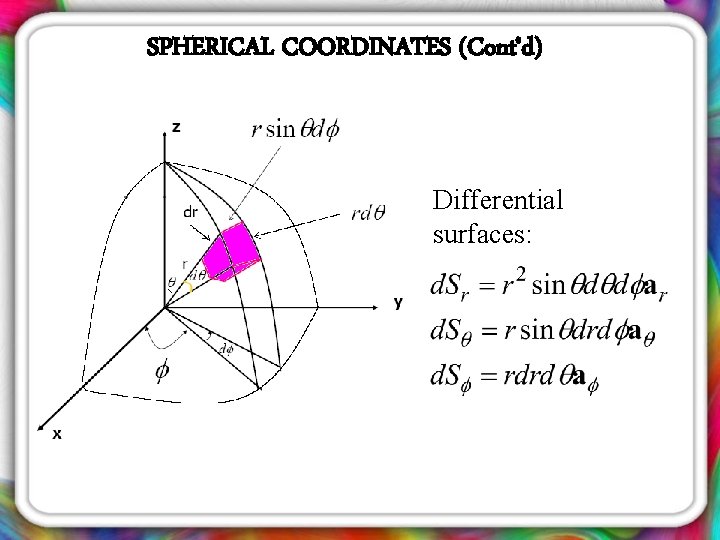
SPHERICAL COORDINATES (Cont’d) Differential surfaces:

SPHERICAL COORDINATES (Cont’d)

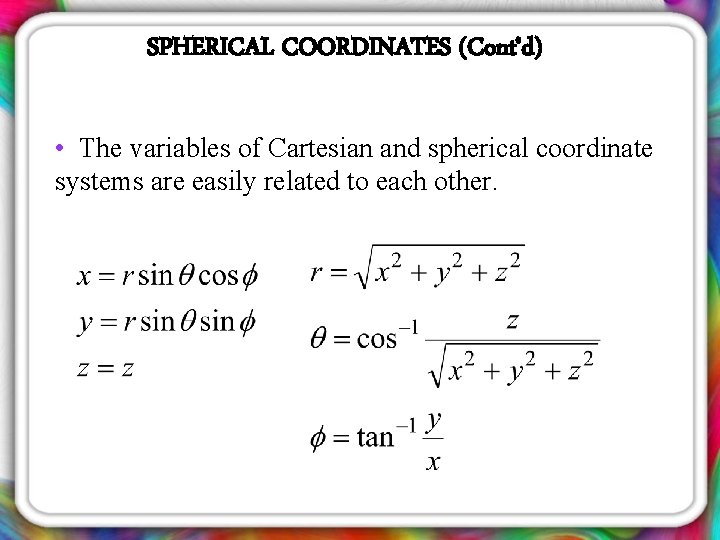
SPHERICAL COORDINATES (Cont’d) • The variables of Cartesian and spherical coordinate systems are easily related to each other.

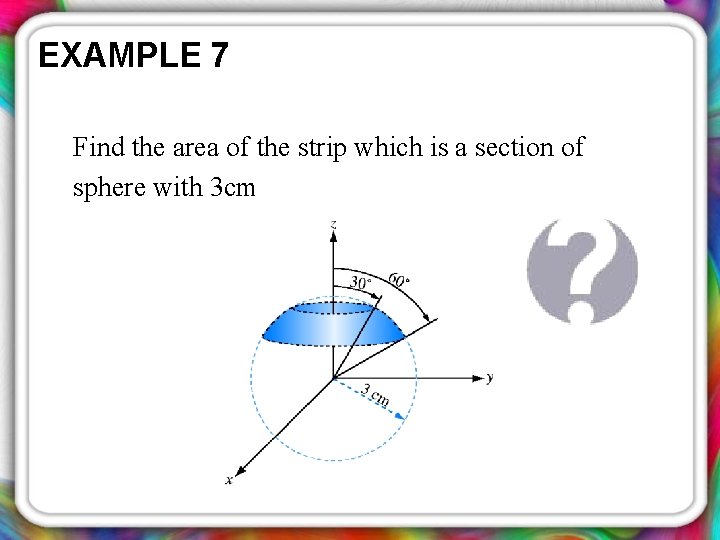
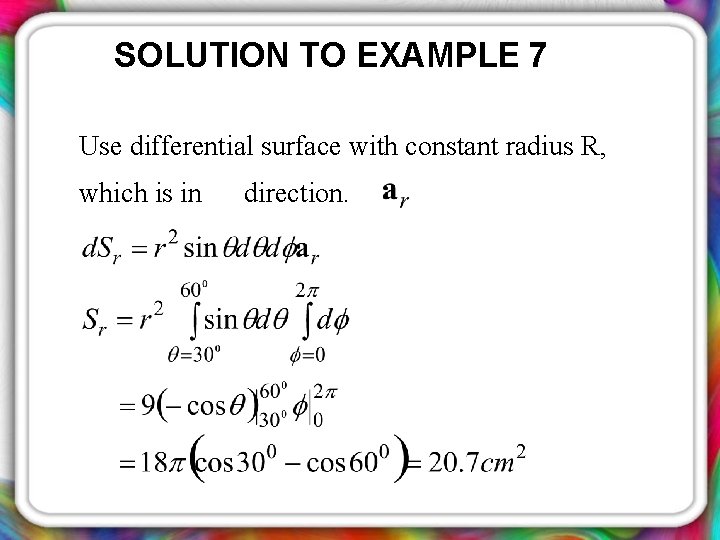
EXAMPLE 7 Find the area of the strip which is a section of sphere with 3 cm

SOLUTION TO EXAMPLE 7 Use differential surface with constant radius R, which is in direction.

VERY IMPORTANT!! Cartesian Cylindrical Spherical Differential in length Differential Surface Differential Volume

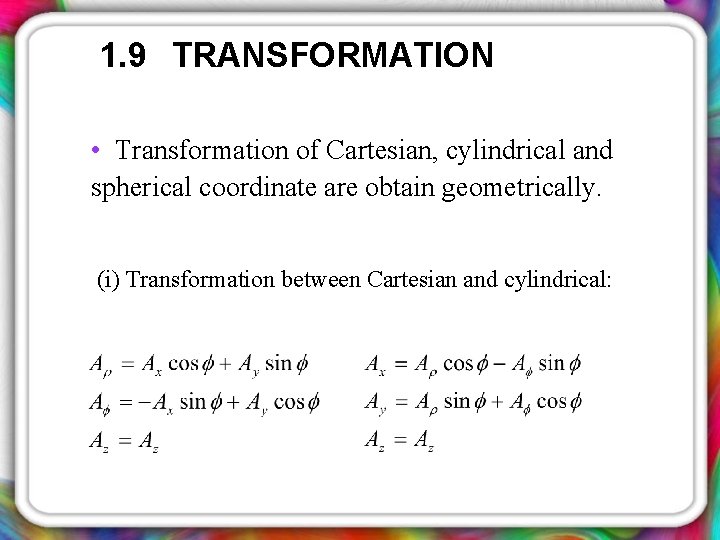
1. 9 TRANSFORMATION • Transformation of Cartesian, cylindrical and spherical coordinate are obtain geometrically. (i) Transformation between Cartesian and cylindrical:

TRANSFORMATION (Cont’d) (ii) Transformation between Cartesian and spherical:

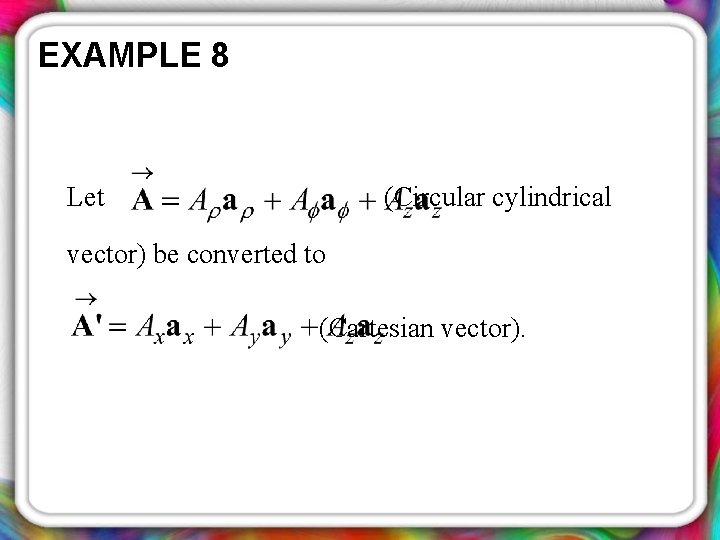
EXAMPLE 8 Let (Circular cylindrical vector) be converted to (Cartesian vector).

SOLUTION TO EXAMPLE 8 For Cartesian vector: Let Cartesian vector component, So,

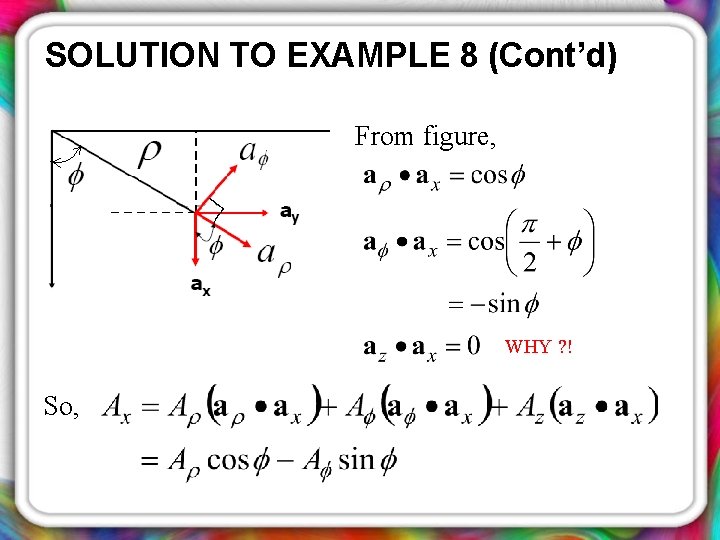
SOLUTION TO EXAMPLE 8 (Cont’d) From figure, WHY ? ! So,

SOLUTION TO EXAMPLE 8 (Cont’d) For: And,

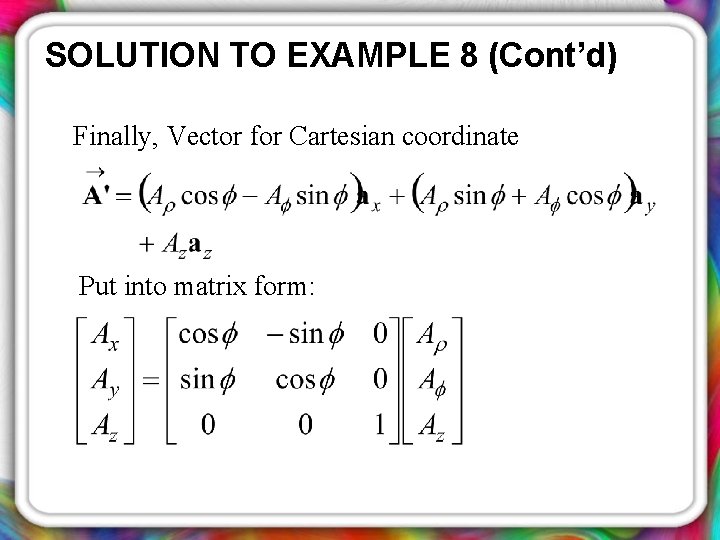
SOLUTION TO EXAMPLE 8 (Cont’d) Finally, Vector for Cartesian coordinate Put into matrix form:

IMPORTANT!! From previous example, we can conclude that the transformation emphasizing on the dot product, where:

EXAMPLE 9 Given in Cartesian coordinates, Evaluate: (i) Point P in Cylindrical coordinates (ii)Vector at P in Cartesian coordinates system (iii)Vector in Cylindrical coordinates system (iv)Vector at P in Cylindrical coordinates system

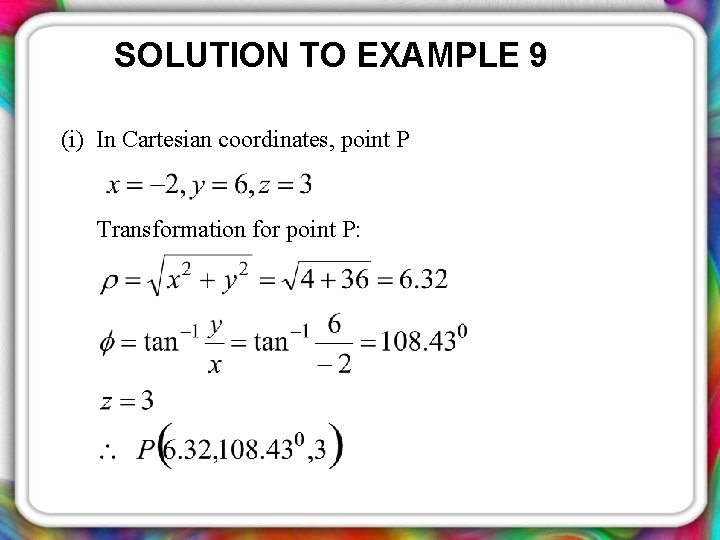
SOLUTION TO EXAMPLE 9 (i) In Cartesian coordinates, point P Transformation for point P:

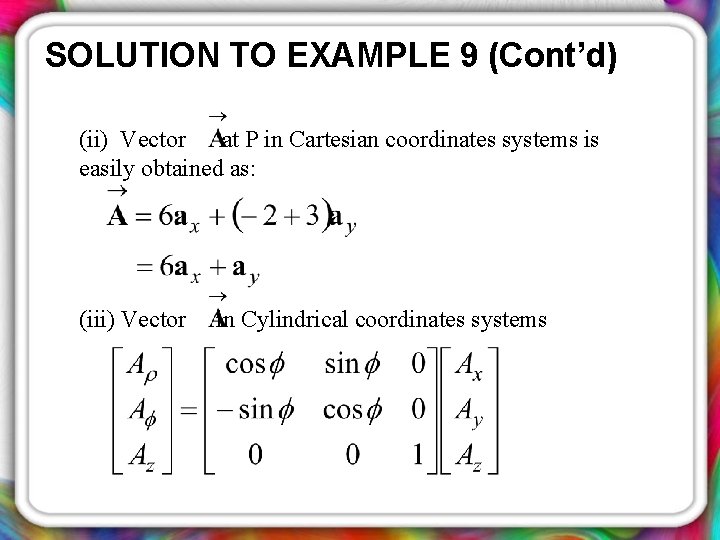
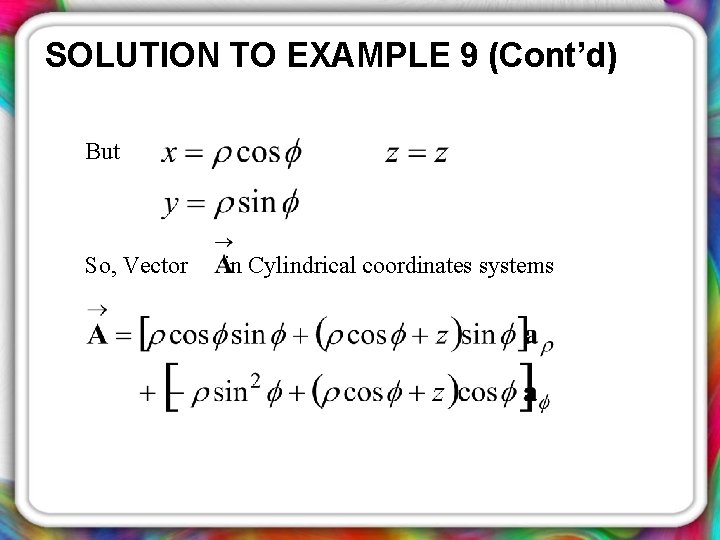
SOLUTION TO EXAMPLE 9 (Cont’d) (ii) Vector at P in Cartesian coordinates systems is easily obtained as: (iii) Vector in Cylindrical coordinates systems

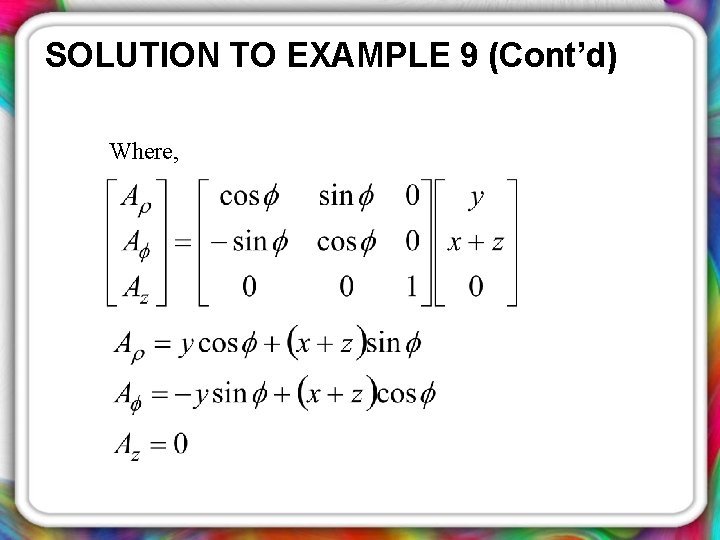
SOLUTION TO EXAMPLE 9 (Cont’d) Where,

SOLUTION TO EXAMPLE 9 (Cont’d) But So, Vector in Cylindrical coordinates systems

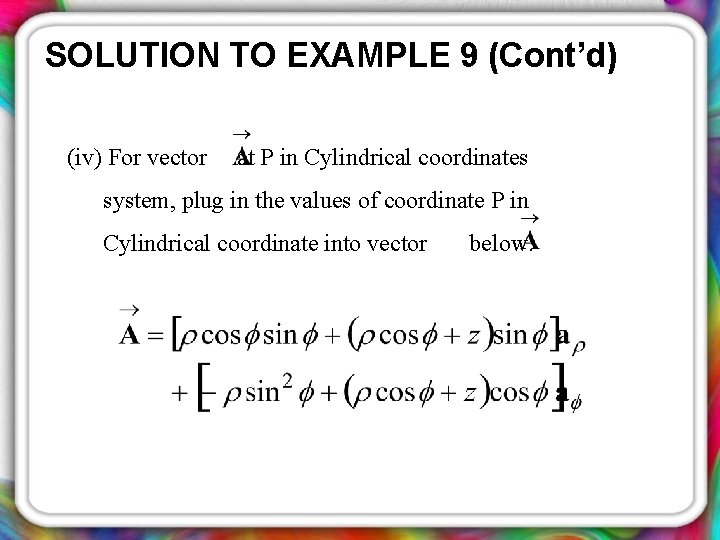
SOLUTION TO EXAMPLE 9 (Cont’d) (iv) For vector at P in Cylindrical coordinates system, plug in the values of coordinate P in Cylindrical coordinate into vector below:

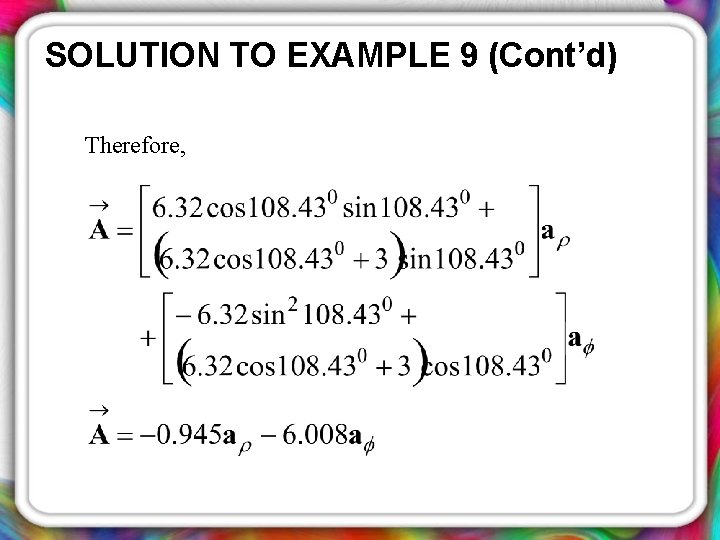
SOLUTION TO EXAMPLE 9 (Cont’d) Therefore,
 Transformation between two cartesian coordinate systems
Transformation between two cartesian coordinate systems Dot product properties
Dot product properties Graphing cylindrical coordinates
Graphing cylindrical coordinates Cylindrical coordinates to cartesian
Cylindrical coordinates to cartesian What is robot anatomy
What is robot anatomy Consecutive coordinates in surveying
Consecutive coordinates in surveying Rectangular coords to polar coords
Rectangular coords to polar coords Difference between pre indexing and post indexing
Difference between pre indexing and post indexing Coordinate covalent bond vs coordinate ionic
Coordinate covalent bond vs coordinate ionic Ncat download
Ncat download Identify the transformation from abc to a'b'c'
Identify the transformation from abc to a'b'c' 2d conformal transformation
2d conformal transformation Transformation in the coordinate plane
Transformation in the coordinate plane Rotating coordinate systems
Rotating coordinate systems Empiricists vs rationalists
Empiricists vs rationalists Cartesian mind body dualism
Cartesian mind body dualism Rectangular
Rectangular Horizontal axis
Horizontal axis Cartesian equations
Cartesian equations Draw this
Draw this Dualism mind body problem
Dualism mind body problem Cartesian product example
Cartesian product example Cartesian plane named after
Cartesian plane named after Statics hibbeler
Statics hibbeler Nested quantifiers
Nested quantifiers What is cartesian form
What is cartesian form Cartesian plane activities
Cartesian plane activities Modulus form
Modulus form Cartesian product of fuzzy sets examples
Cartesian product of fuzzy sets examples Multiplying phasors
Multiplying phasors Cartesian space trajectory planning
Cartesian space trajectory planning Cartesian product example
Cartesian product example Addition and subtraction
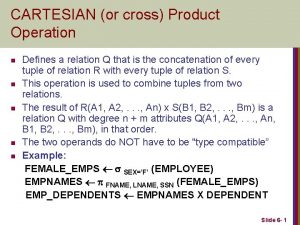
Addition and subtraction Cartesian product operation
Cartesian product operation Cartesian mind body dualism
Cartesian mind body dualism 카테시안 조인
카테시안 조인 Definition of power set
Definition of power set Descartes certainty
Descartes certainty Polar plane graph
Polar plane graph Cartesian product of sets venn diagram
Cartesian product of sets venn diagram Cartesian product example
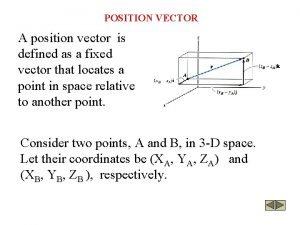
Cartesian product example Position vector
Position vector Cartesian product and relations
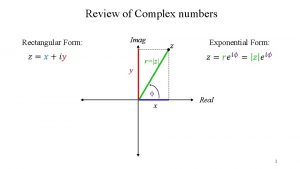
Cartesian product and relations Cartesian form of complex numbers
Cartesian form of complex numbers Cartesian space vs joint space
Cartesian space vs joint space Contoh cartesian product
Contoh cartesian product Haskell guards
Haskell guards Cartesian vectors statics
Cartesian vectors statics Koordinat cartesian
Koordinat cartesian