730 Lecture 14 Todays lecture 6182021 730 Lecture

730 Lecture 14 Today’s lecture: 6/18/2021 730 Lecture 14 1

Confidence Intervals Two methods • Inverting tests • Finding pivotal quantities 6/18/2021 730 Lecture 14 2

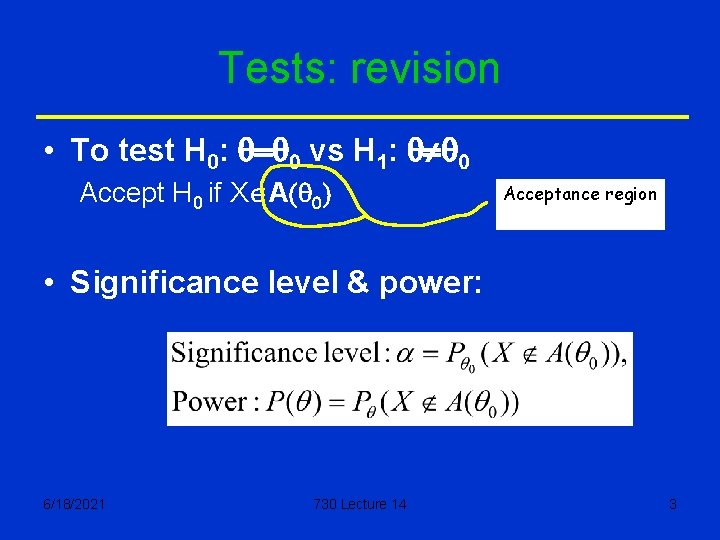
Tests: revision • To test H 0: q=q 0 vs H 1: q¹q 0 Accept H 0 if XÎA(q 0) Acceptance region • Significance level & power: 6/18/2021 730 Lecture 14 3

Power functions Testing q=1: 6/18/2021 730 Lecture 14 4

Confidence intervals • If L(X), R(X) are statistics such that P(L<q<R)=1 -a. . • then [L, R] is a 100(1 -a)% confidence interval for q with coverage probability 1 -a 6/18/2021 730 Lecture 14 5

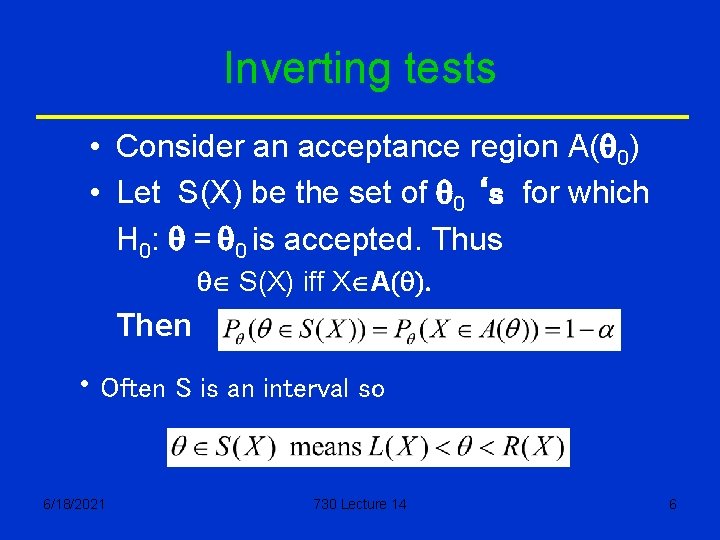
Inverting tests • Consider an acceptance region A(q 0) • Let S(X) be the set of q 0 ‘s for which H 0: q = q 0 is accepted. Thus qÎ S(X) iff XÎA(q). Then • Often S is an interval so 6/18/2021 730 Lecture 14 6

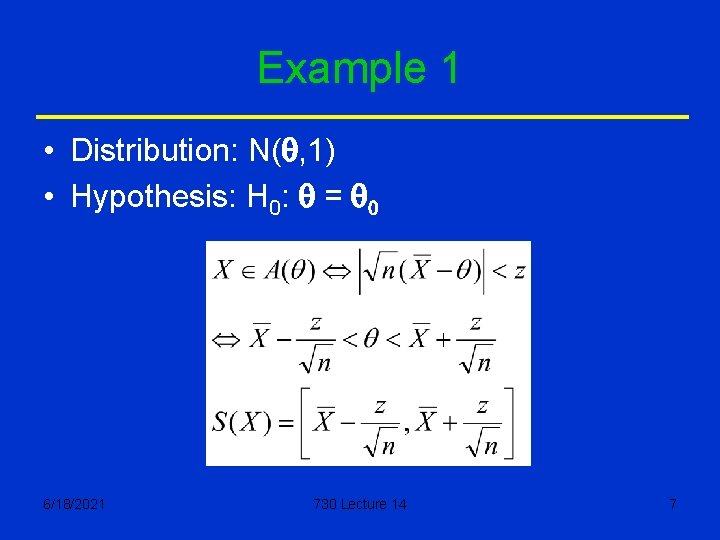
Example 1 • Distribution: N(q, 1) • Hypothesis: H 0: q = q 0 6/18/2021 730 Lecture 14 7

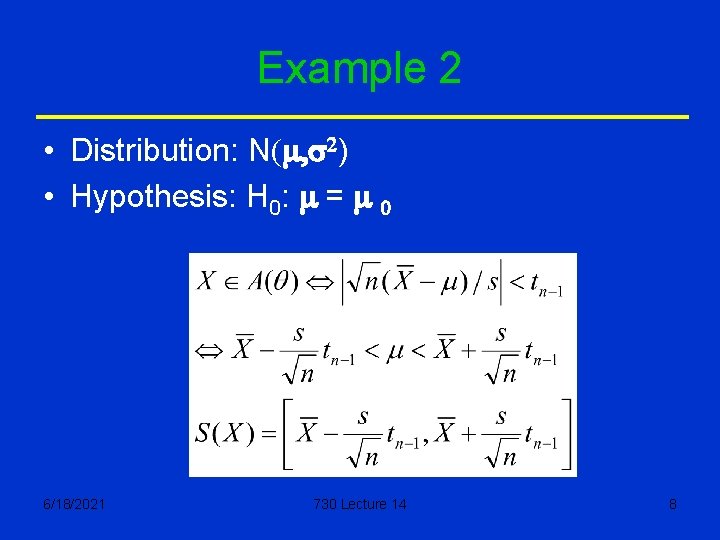
Example 2 • Distribution: N(m, s 2) • Hypothesis: H 0: m = m 0 6/18/2021 730 Lecture 14 8

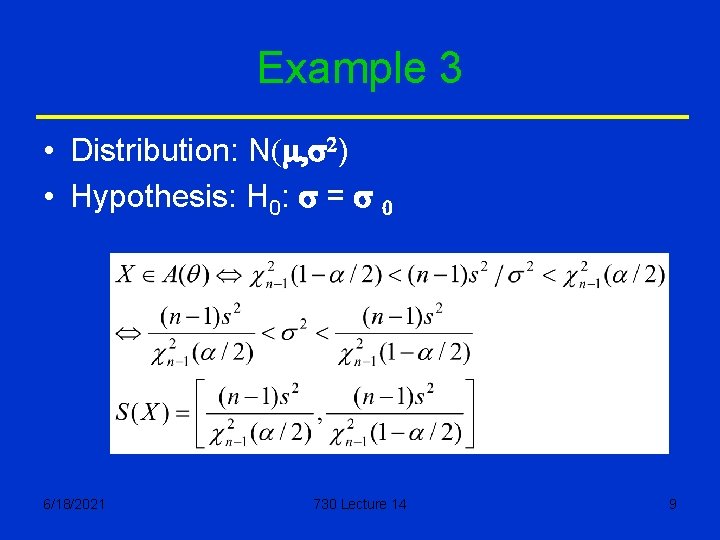
Example 3 • Distribution: N(m, s 2) • Hypothesis: H 0: s = s 0 6/18/2021 730 Lecture 14 9

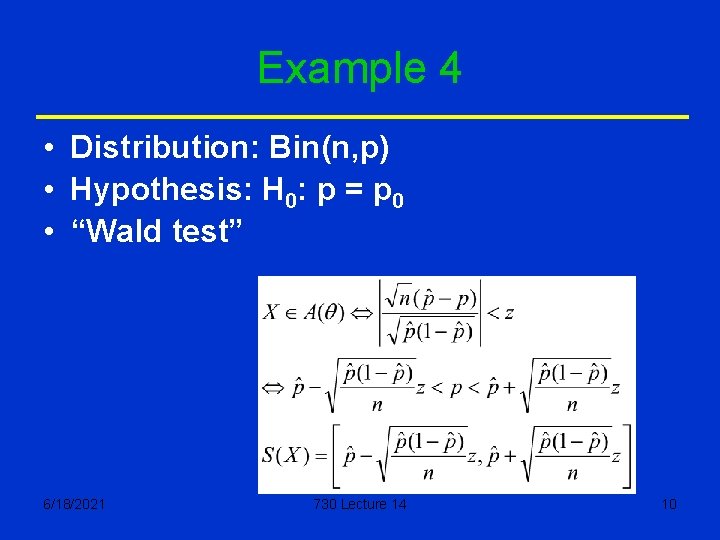
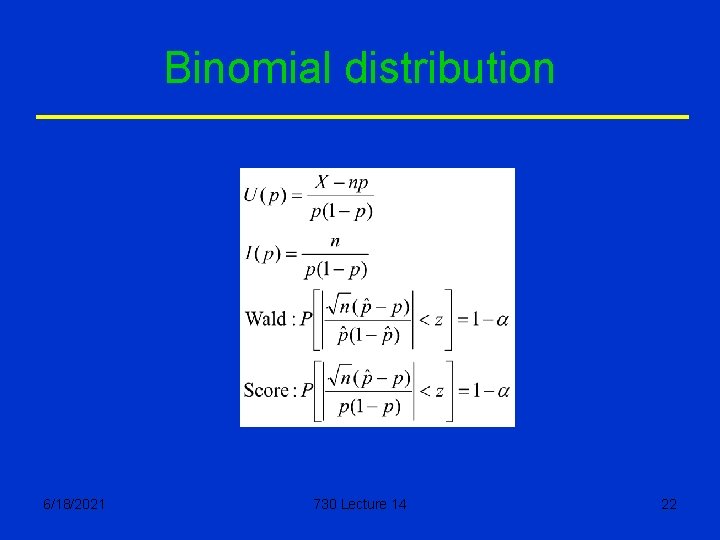
Example 4 • Distribution: Bin(n, p) • Hypothesis: H 0: p = p 0 • “Wald test” 6/18/2021 730 Lecture 14 10

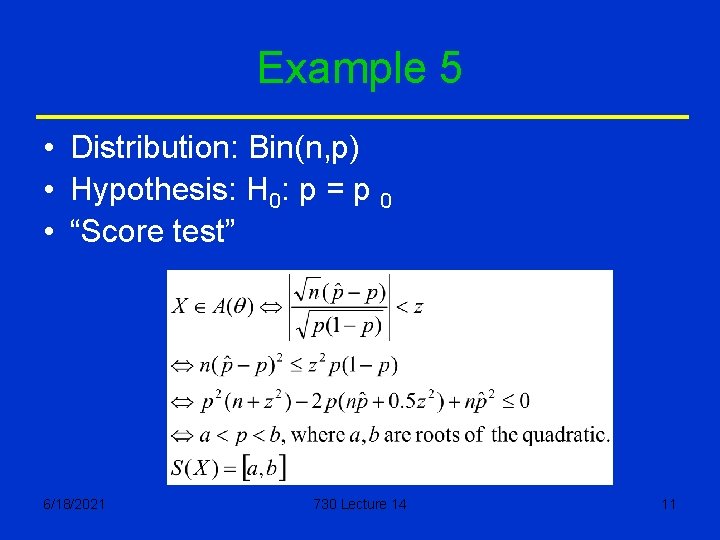
Example 5 • Distribution: Bin(n, p) • Hypothesis: H 0: p = p 0 • “Score test” 6/18/2021 730 Lecture 14 11

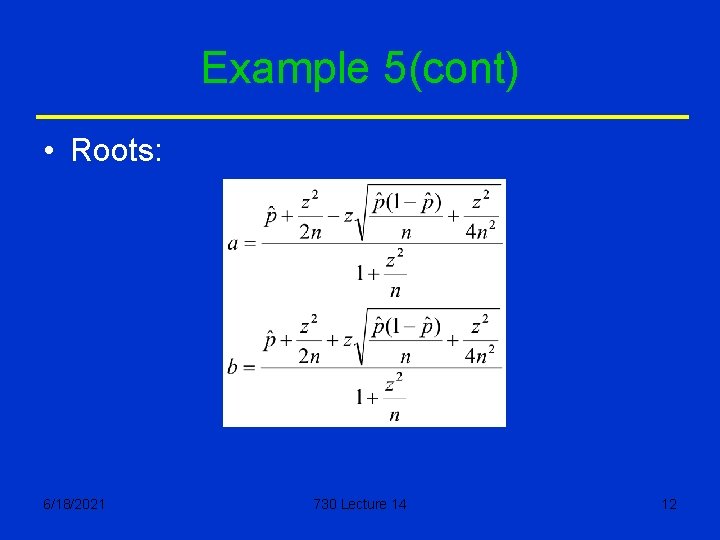
Example 5(cont) • Roots: 6/18/2021 730 Lecture 14 12

Example 6 Distribution: Bin(n, p) Hypothesis: H 0: p = p 0 “Exact test” Put x. L=largest integer such that Put x. R=smallest integer such that 6/18/2021 730 Lecture 14 13

Example 6 (cont) A “conservative” test 6/18/2021 730 Lecture 14 14

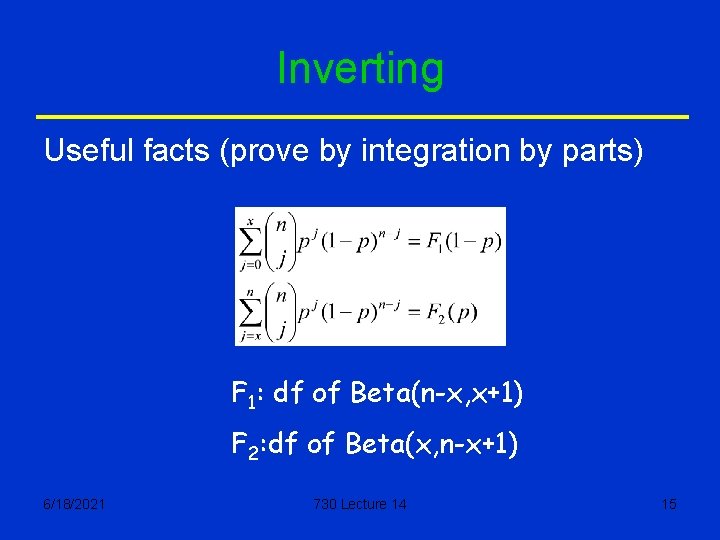
Inverting Useful facts (prove by integration by parts) F 1: df of Beta(n-x, x+1) F 2: df of Beta(x, n-x+1) 6/18/2021 730 Lecture 14 15

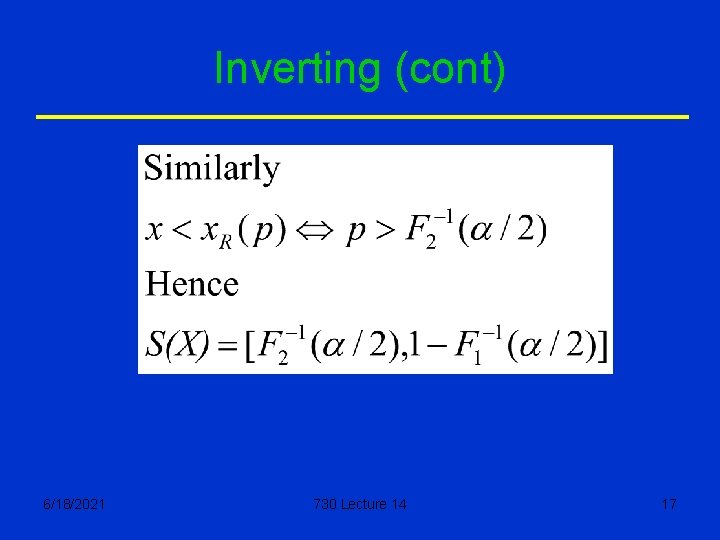
Inverting (cont) 6/18/2021 730 Lecture 14 16

Inverting (cont) 6/18/2021 730 Lecture 14 17

Using pivotal quantities • A pivotal quantity is a function S(X, q) of the data X and the parameters q whose distribution does not depend on q. • We invert the pivot to get the interval. 6/18/2021 730 Lecture 14 18

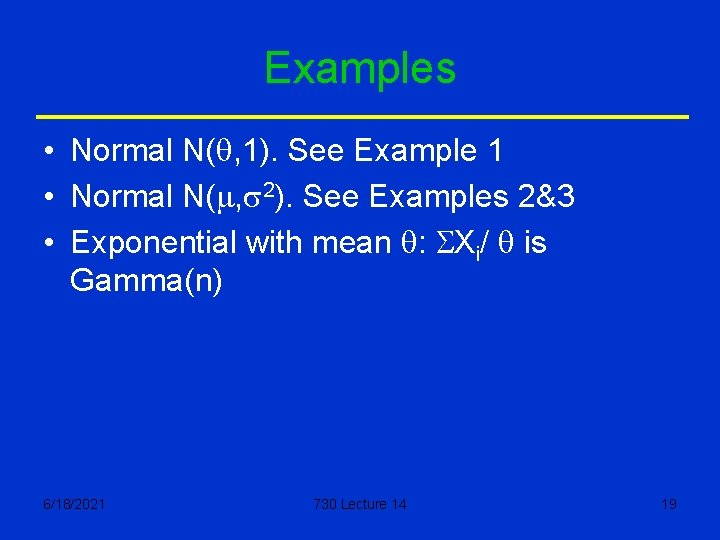
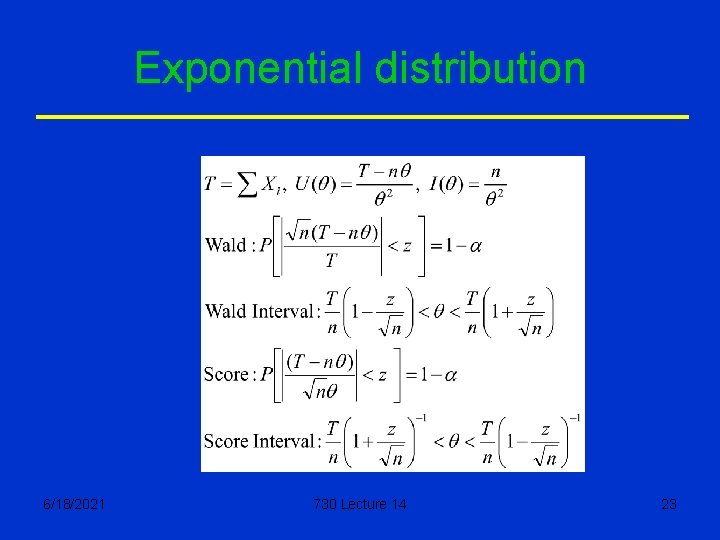
Examples • Normal N(q, 1). See Example 1 • Normal N(m, s 2). See Examples 2&3 • Exponential with mean q: SXi/ q is Gamma(n) 6/18/2021 730 Lecture 14 19

Inverting: Exponential each area a/2 g 1 6/18/2021 g 2 730 Lecture 14 20

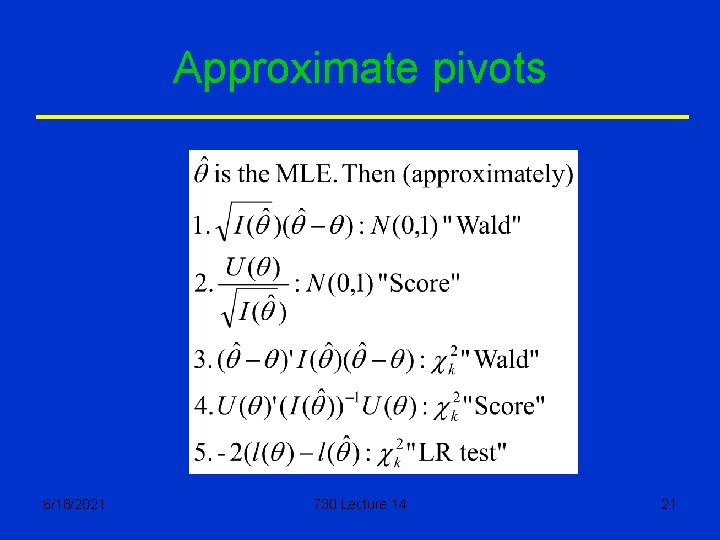
Approximate pivots 6/18/2021 730 Lecture 14 21

Binomial distribution 6/18/2021 730 Lecture 14 22

Exponential distribution 6/18/2021 730 Lecture 14 23

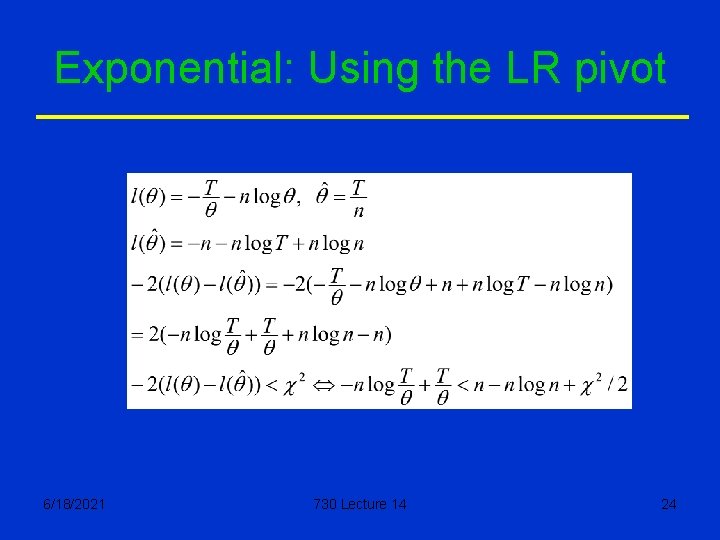
Exponential: Using the LR pivot 6/18/2021 730 Lecture 14 24

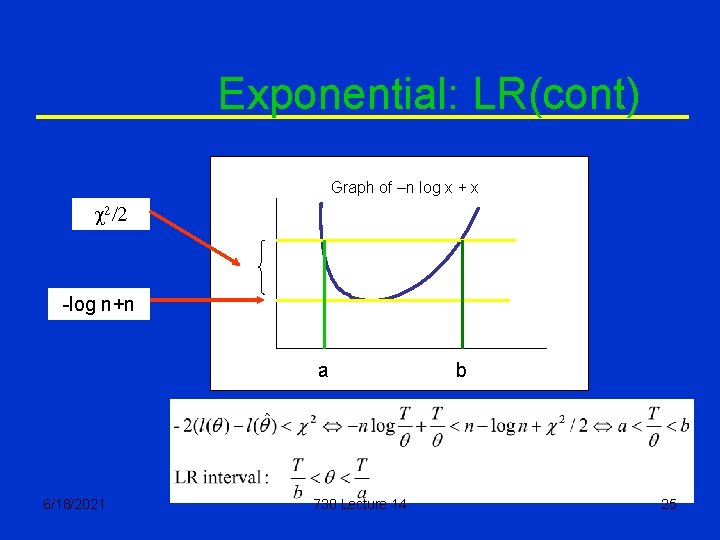
Exponential: LR(cont) Graph of –n log x + x c 2/2 -log n+n a 6/18/2021 730 Lecture 14 b 25

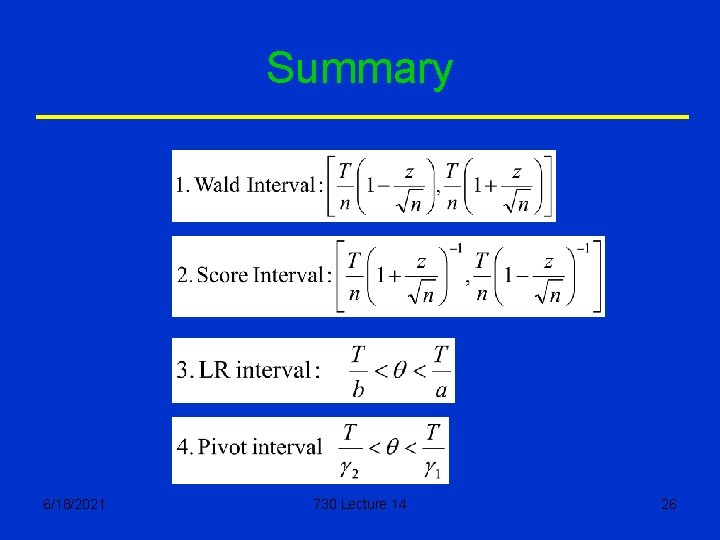
Summary 6/18/2021 730 Lecture 14 26

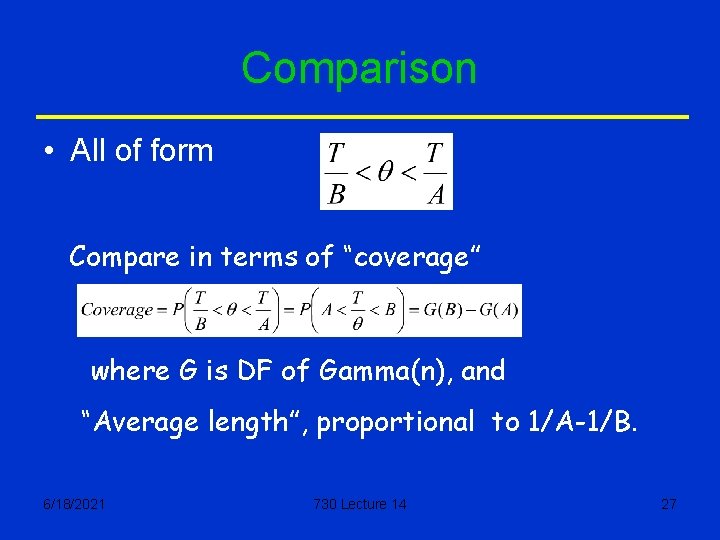
Comparison • All of form Compare in terms of “coverage” where G is DF of Gamma(n), and “Average length”, proportional to 1/A-1/B. 6/18/2021 730 Lecture 14 27

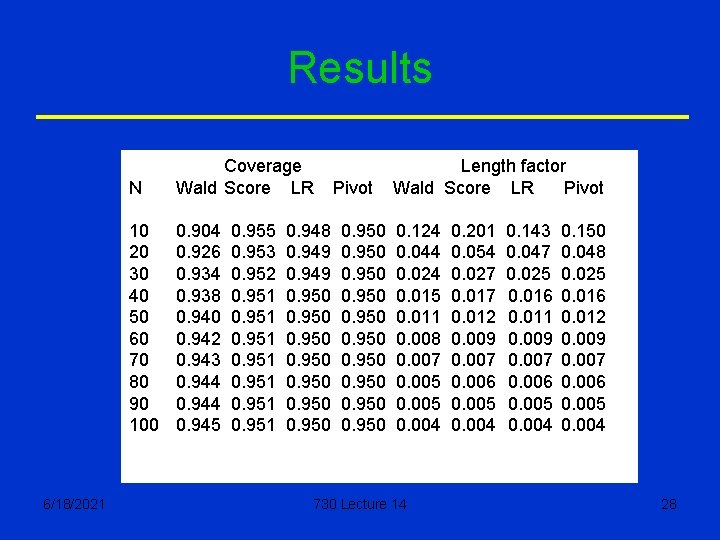
Results 6/18/2021 N Coverage Wald Score LR 10 20 30 40 50 60 70 80 90 100 0. 904 0. 926 0. 934 0. 938 0. 940 0. 942 0. 943 0. 944 0. 945 0. 953 0. 952 0. 951 0. 948 0. 949 0. 950 0. 950 Pivot 0. 950 0. 950 Length factor Wald Score LR Pivot 0. 124 0. 044 0. 024 0. 015 0. 011 0. 008 0. 007 0. 005 0. 004 730 Lecture 14 0. 201 0. 054 0. 027 0. 012 0. 009 0. 007 0. 006 0. 005 0. 004 0. 143 0. 047 0. 025 0. 016 0. 011 0. 009 0. 007 0. 006 0. 005 0. 004 0. 150 0. 048 0. 025 0. 016 0. 012 0. 009 0. 007 0. 006 0. 005 0. 004 28