NavierStokes Equation n Newtonian Fluid u Constant Density







- Slides: 24

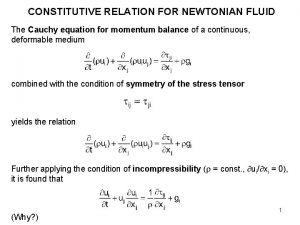
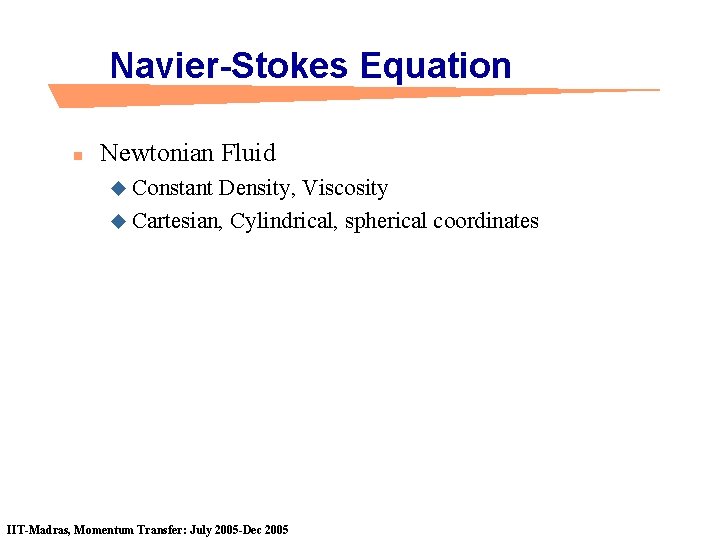
Navier-Stokes Equation n Newtonian Fluid u Constant Density, Viscosity u Cartesian, Cylindrical, spherical coordinates IIT-Madras, Momentum Transfer: July 2005 -Dec 2005

Cartesian Coordinates IIT-Madras, Momentum Transfer: July 2005 -Dec 2005

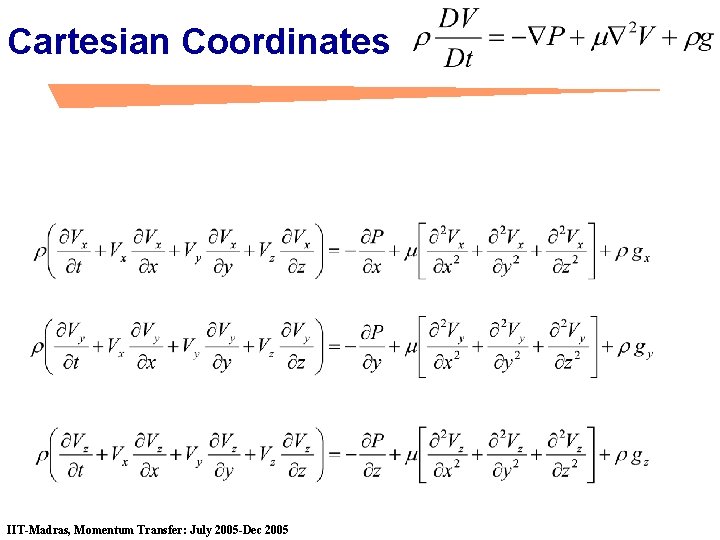
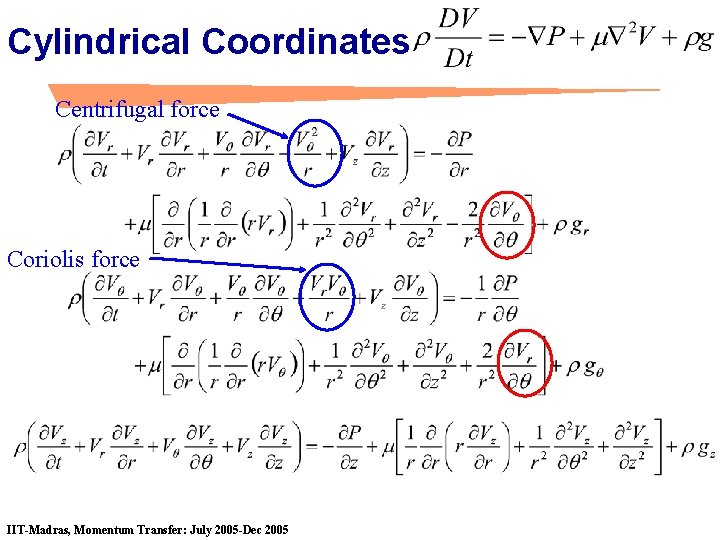
Cylindrical Coordinates Centrifugal force Coriolis force IIT-Madras, Momentum Transfer: July 2005 -Dec 2005

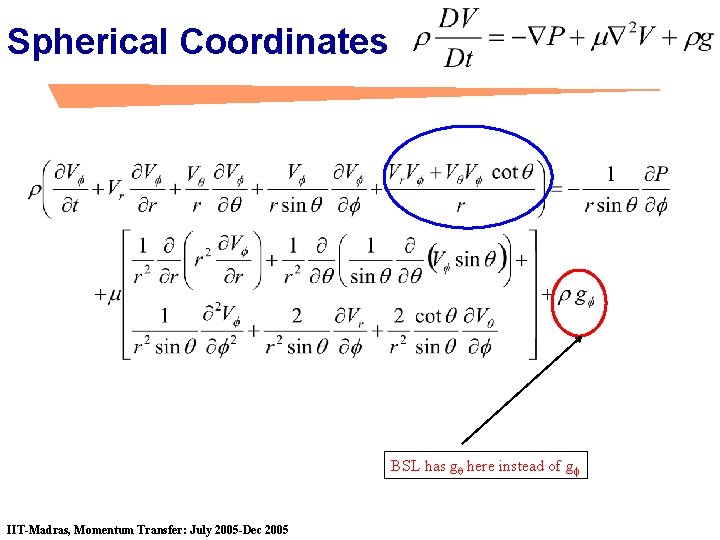
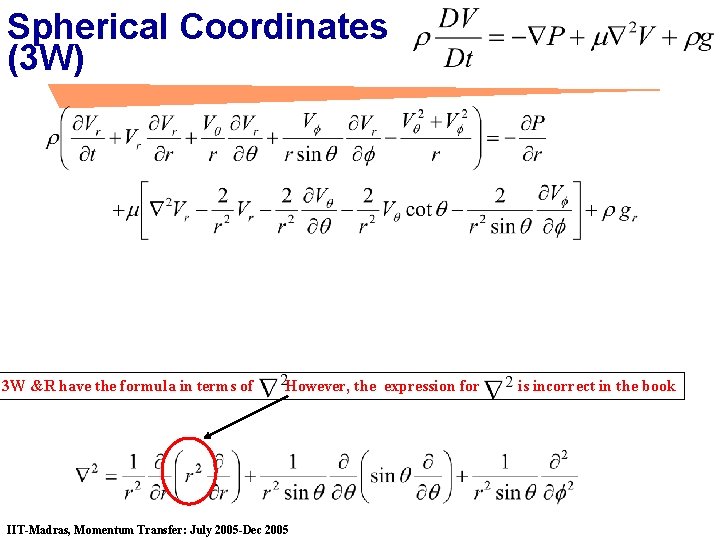
Spherical Coordinates IIT-Madras, Momentum Transfer: July 2005 -Dec 2005

Spherical Coordinates BSL has gq here instead of gf IIT-Madras, Momentum Transfer: July 2005 -Dec 2005

Spherical Coordinates (3 W) 3 W &R have the formula in terms of However, the expression for IIT-Madras, Momentum Transfer: July 2005 -Dec 2005 is incorrect in the book

Continuity IIT-Madras, Momentum Transfer: July 2005 -Dec 2005

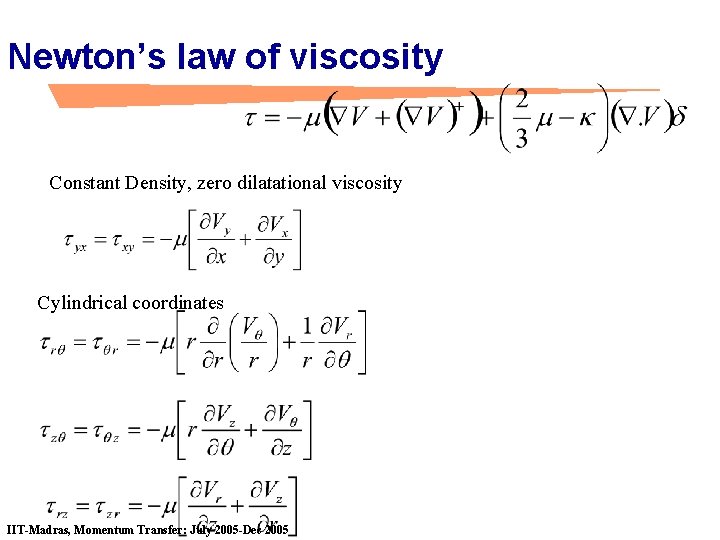
Newton’s law of viscosity Constant Density, zero dilatational viscosity Cylindrical coordinates IIT-Madras, Momentum Transfer: July 2005 -Dec 2005

Newton’s law of viscosity Spherical coordinates: Constant Density, zero dilatational viscosity IIT-Madras, Momentum Transfer: July 2005 -Dec 2005

N-S Equation: Examples • ODE vs PDE • Spherical and cylindrical coordinates • Eqn for pipe flow (Hagen Poiseulle) • Flow between rotating cylinders (not solved in class) • Thin film flow with temp variation (not solved in class, steps were discussed briefly. BSL ‘worked out’ example) • Radial flow between circular plates (BSL 3 B. 10) IIT-Madras, Momentum Transfer: July 2005 -Dec 2005

Example problems 1. Pressure driven steady state flow of fluid between two infinite parallel plates inside a circular tube 2. Steady state Couvette flow of a fluid between two infinite parallel plates with top plate moving at a known velocity between two circular plates of finite radius, with the top plate rotating at a known angular velocity between two circular cylinders with outer cylinder rotating at a known angular velocity (end effects are negligible) between a cone and plate (stationary plate and cone is rotating at a known angular velocity). Angle of cone is very small (almost a parallel plate with almost zero gap) 3. Coutte Poisseuille flow between two parallel plates IIT-Madras, Momentum Transfer: July 2005 -Dec 2005

N-S Equation: Examples • PDE • Please refer to the book “Applied Mathematical Methods for Chemical Engineers” by Norman W Loney (CRC press), pages 330 to 342 for “worked out” examples for Momentum Transfer problems involving PDE. • Either multi dimensional or time dependent (however multidimensional and time dependent cases are not discussed in detail) • Steady state in Rectangular channel: pressure driven , coutte flow • Plan suddenly moving with constant velocity (or stress) from time t=0 (Stokes problem) • Sudden pressure gradient in a cylindrical tube (unsteady flow , converging to Hagen-Poisseuille’s flow (Bessel functions) • Flow between two (non rotating) cylinders, caused by boundary movement (coutte flow). Unsteady vs steady (not discussed in class or covered in tutorial) IIT-Madras, Momentum Transfer: July 2005 -Dec 2005

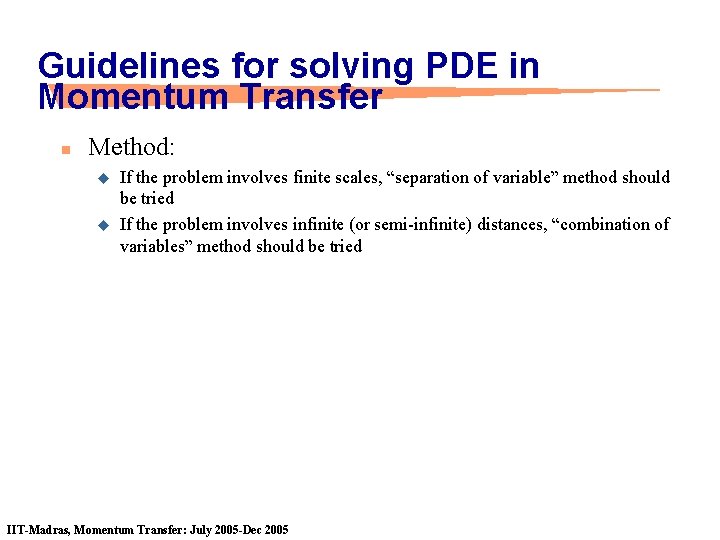
Guidelines for solving PDE in Momentum Transfer n Method: u u If the problem involves finite scales, “separation of variable” method should be tried If the problem involves infinite (or semi-infinite) distances, “combination of variables” method should be tried IIT-Madras, Momentum Transfer: July 2005 -Dec 2005

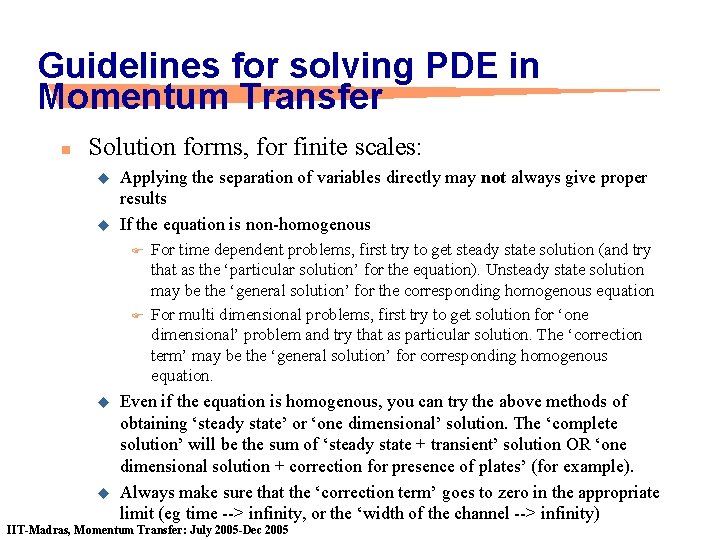
Guidelines for solving PDE in Momentum Transfer n Solution forms, for finite scales: u u Applying the separation of variables directly may not always give proper results If the equation is non-homogenous F F u u For time dependent problems, first try to get steady state solution (and try that as the ‘particular solution’ for the equation). Unsteady state solution may be the ‘general solution’ for the corresponding homogenous equation For multi dimensional problems, first try to get solution for ‘one dimensional’ problem and try that as particular solution. The ‘correction term’ may be the ‘general solution’ for corresponding homogenous equation. Even if the equation is homogenous, you can try the above methods of obtaining ‘steady state’ or ‘one dimensional’ solution. The ‘complete solution’ will be the sum of ‘steady state + transient’ solution OR ‘one dimensional solution + correction for presence of plates’ (for example). Always make sure that the ‘correction term’ goes to zero in the appropriate limit (eg time --> infinity, or the ‘width of the channel --> infinity) IIT-Madras, Momentum Transfer: July 2005 -Dec 2005

Guidelines for solving PDE in Momentum Transfer n Other relevant Information: u u u Problems in Cartesian coordinates tend to give Cosine/ Sine series solution. In cylindrical coordinates, Bessel functions. In spherical coordinates, Legendre functions When you attempt a ‘complete solution’ as ‘steady state+ transient’ (OR ‘one dimensional + correction’), make sure that you also translate the boundary conditions correctly While solving for the ‘transient’ or ‘correction’ terms, you may encounter a situation where you have to choose an arbitrary constant (either positive or negative or zero). Usually the constant will not be zero. Choose the constant as positive or negative, depending on the boundary conditions (otherwise, you will proceed only to realize that it will not work). IIT-Madras, Momentum Transfer: July 2005 -Dec 2005

Stoke’s first problem (Please refer to BSL for solution) IIT-Madras, Momentum Transfer: July 2005 -Dec 2005

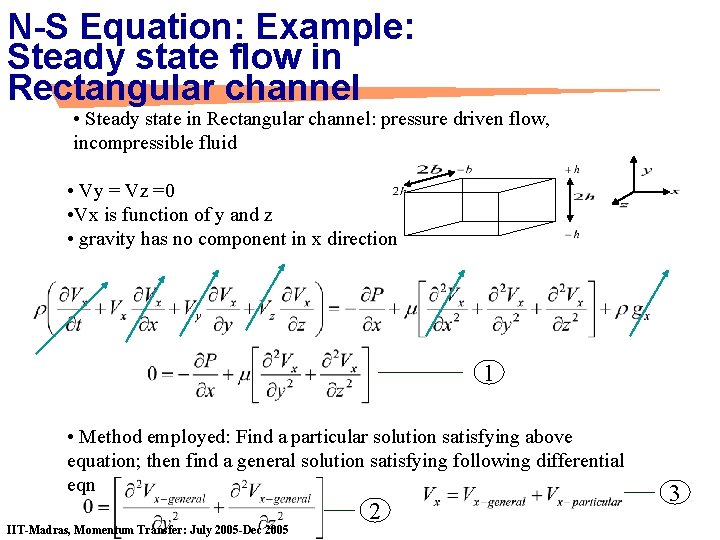
N-S Equation: Example: Steady state flow in Rectangular channel • Steady state in Rectangular channel: pressure driven flow, incompressible fluid • Vy = Vz =0 • Vx is function of y and z • gravity has no component in x direction 1 • Method employed: Find a particular solution satisfying above equation; then find a general solution satisfying following differential eqn IIT-Madras, Momentum Transfer: July 2005 -Dec 2005 2 3

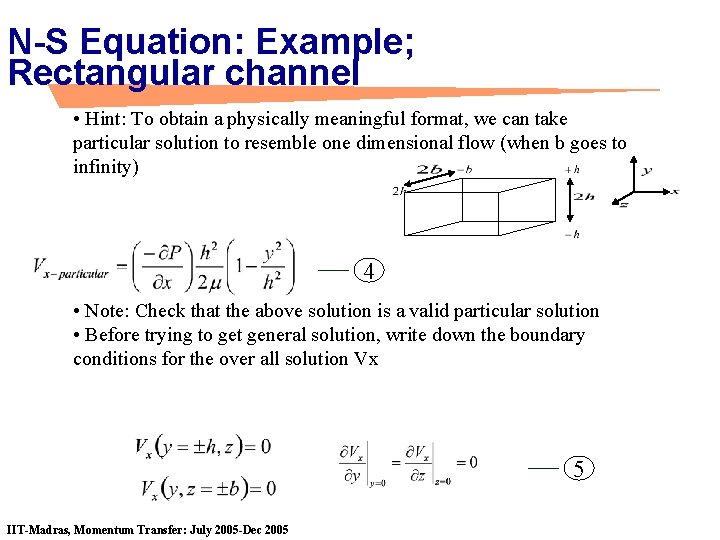
N-S Equation: Example; Rectangular channel • Hint: To obtain a physically meaningful format, we can take particular solution to resemble one dimensional flow (when b goes to infinity) 4 • Note: Check that the above solution is a valid particular solution • Before trying to get general solution, write down the boundary conditions for the over all solution Vx 5 IIT-Madras, Momentum Transfer: July 2005 -Dec 2005

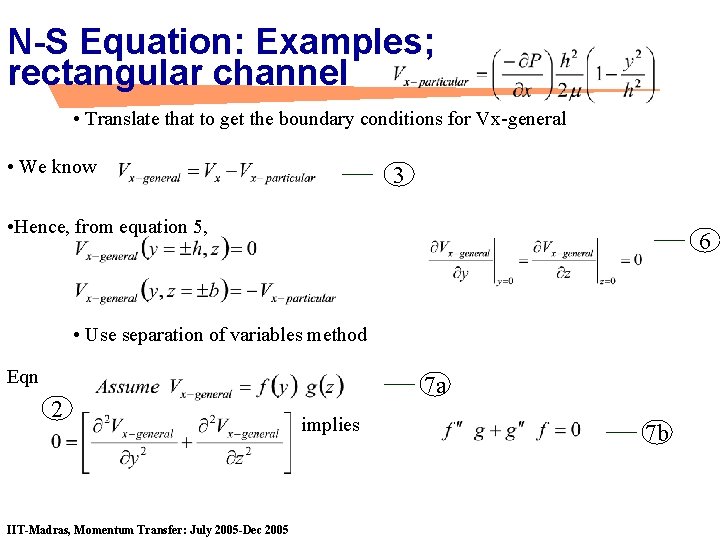
N-S Equation: Examples; rectangular channel • Translate that to get the boundary conditions for Vx-general • We know 3 • Hence, from equation 5, 6 • Use separation of variables method Eqn 2 IIT-Madras, Momentum Transfer: July 2005 -Dec 2005 7 a implies 7 b

N-S Equation: Examples; rectangular channel implies • Since LHS is only a function of y and RHS is fn of z, both must be equal to a constant • We say 8 • Note: Why do we say , why not happen if you try that? Or if we say ? ? What will • In any case, the chosen constant leads to 9 From 6 implies IIT-Madras, Momentum Transfer: July 2005 -Dec 2005 and implies

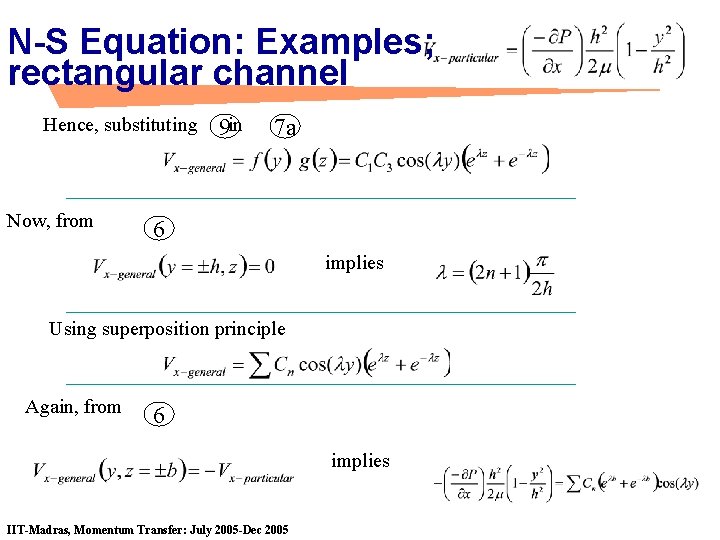
N-S Equation: Examples; rectangular channel Hence, substituting Now, from 9 in 7 a 6 implies Using superposition principle Again, from 6 implies IIT-Madras, Momentum Transfer: July 2005 -Dec 2005

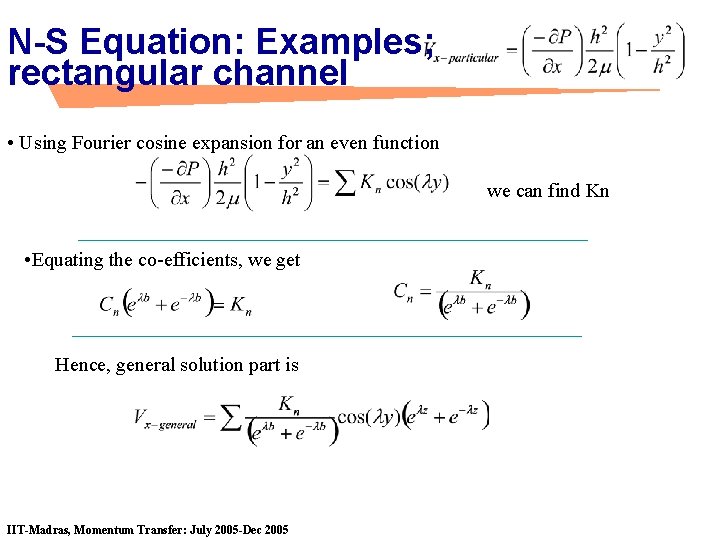
N-S Equation: Examples; rectangular channel • Using Fourier cosine expansion for an even function we can find Kn • Equating the co-efficients, we get Hence, general solution part is IIT-Madras, Momentum Transfer: July 2005 -Dec 2005

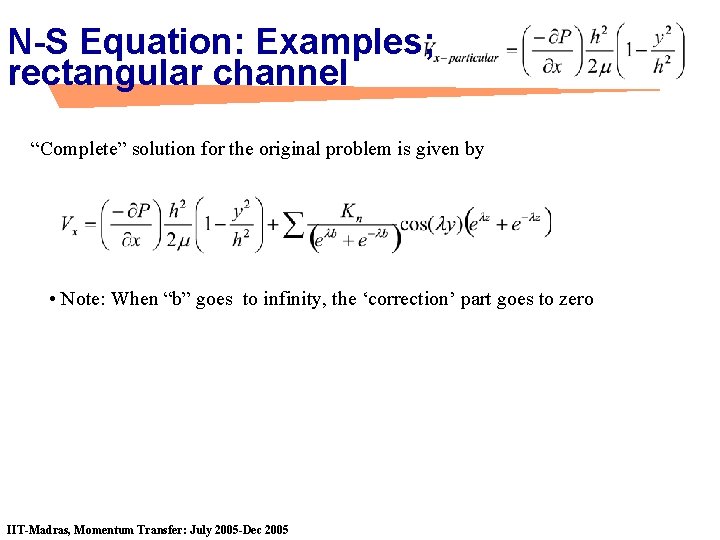
N-S Equation: Examples; rectangular channel “Complete” solution for the original problem is given by • Note: When “b” goes to infinity, the ‘correction’ part goes to zero IIT-Madras, Momentum Transfer: July 2005 -Dec 2005

N-S Equation: Other examples • To determine the velocity profile in a rectangular channel, where the top plate is moving at a constant velocity of V-zero, under steady state conditions • Try out a solution of the form “V-parallel-plate + V-correction” • Use separation of variable techniques, to determine V-correction • What happens if you try separation of variable in the first place? • To determine the unsteady state solution for a flow in a cylindrical pipe, caused by sudden application of pressure • Try a solution of the form ‘Steady state + Transient’, just like the one we saw for flow between parallel plates • You will get Bessel Equations. Just like we represented functions in rectangular coordinates by sine and cosine functions, we can represent functions in cylindrical co ordinates by Bessel functions, because they are orthogonal. IIT-Madras, Momentum Transfer: July 2005 -Dec 2005
 Newtonian
Newtonian Newtonian fluid formula
Newtonian fluid formula Anthropometry
Anthropometry Relatividad de newton
Relatividad de newton Impulse = mv-mu
Impulse = mv-mu Modified newtonian dynamics
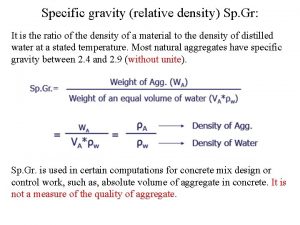
Modified newtonian dynamics Define specific gravity in fluid mechanics
Define specific gravity in fluid mechanics Linear and planar densities
Linear and planar densities Crude population density vs physiological density
Crude population density vs physiological density Linear density of fcc 110
Linear density of fcc 110 High density low density
High density low density Arithmetic density ap human geography
Arithmetic density ap human geography Fluid density definition
Fluid density definition Mass density in fluid mechanics
Mass density in fluid mechanics Drilling fluid density
Drilling fluid density Fluid density
Fluid density Viscoseal
Viscoseal Fluid statics deals with fluid at rest
Fluid statics deals with fluid at rest Fluid statics deals with
Fluid statics deals with Transcellular fluid compartment
Transcellular fluid compartment Bioimpedância
Bioimpedância Hypoosmotic
Hypoosmotic Fluid mechanics chapter 4
Fluid mechanics chapter 4 Extracellular fluid and interstitial fluid
Extracellular fluid and interstitial fluid Bulging flanks ascites
Bulging flanks ascites