Newtonian Relativity A reference frame in which Newtons






- Slides: 22

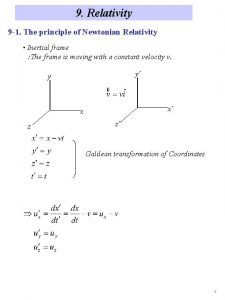
Newtonian Relativity ØA reference frame in which Newton’s laws are valid is called an inertial frame ØNewtonian principle of relativity or Galilean invariance n n If Newton’s laws are valid in one reference frame, then they are also valid in a reference frame moving at uniform velocity relative to the first system Thus this moving frame is also an inertial frame 1

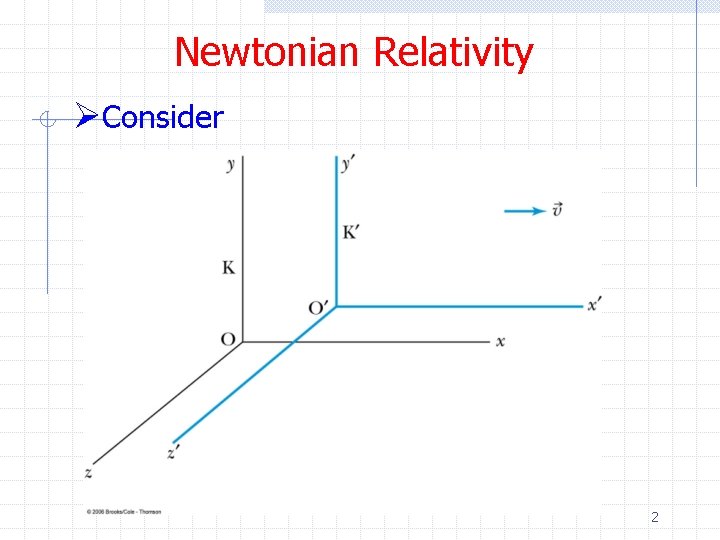
Newtonian Relativity ØConsider 2

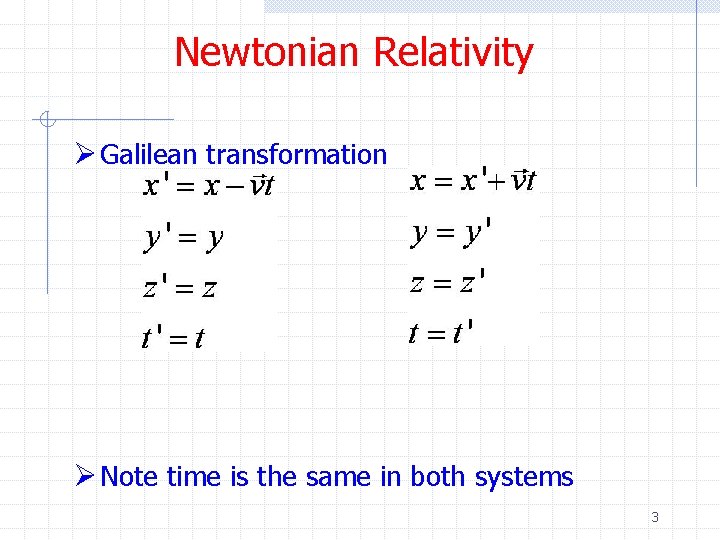
Newtonian Relativity Ø Galilean transformation Ø Note time is the same in both systems 3

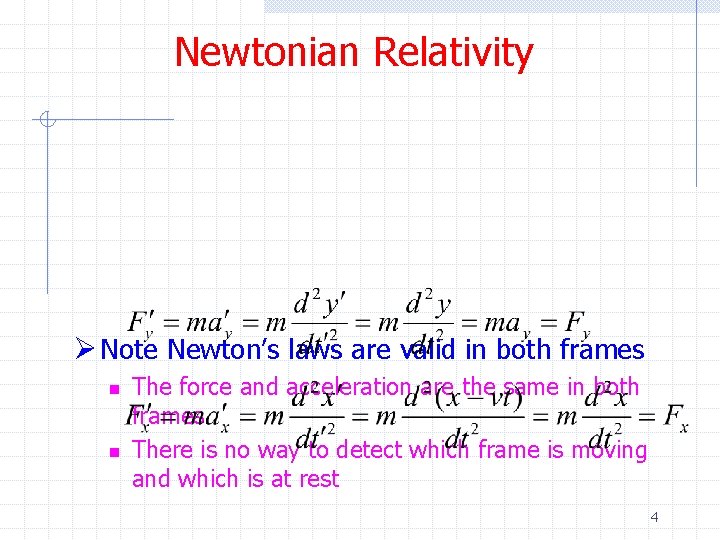
Newtonian Relativity Ø Note Newton’s laws are valid in both frames n n The force and acceleration are the same in both frames There is no way to detect which frame is moving and which is at rest 4

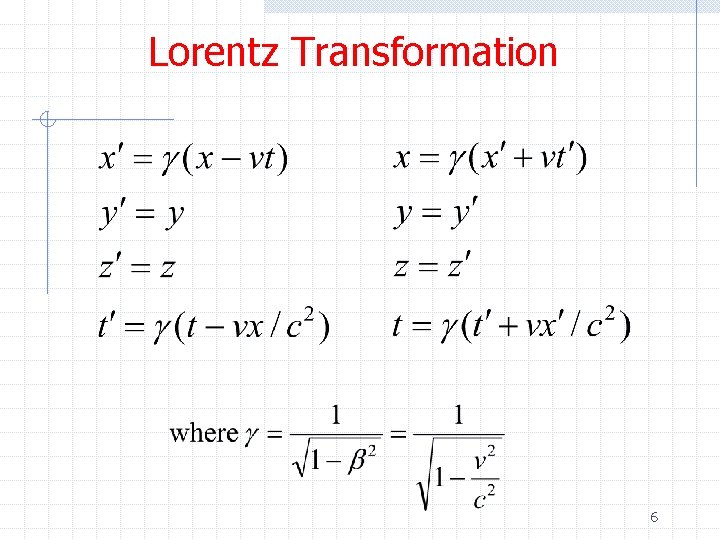
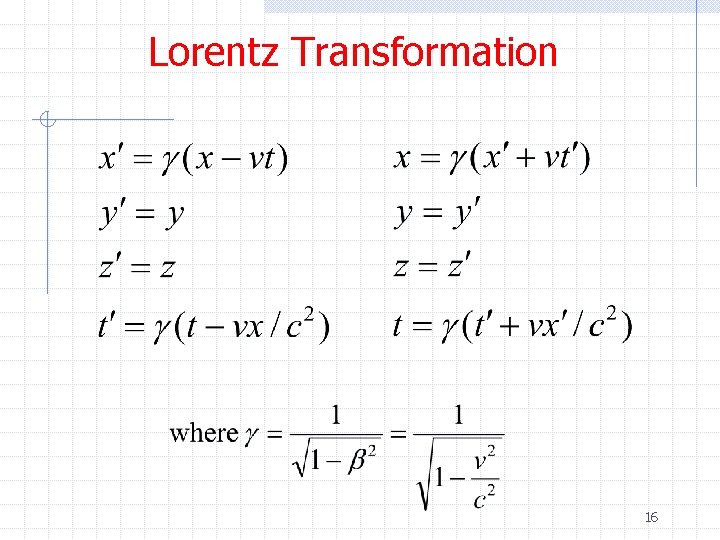
Lorentz Transformation ØWe immediately see the Galilean transformation is inconsistent with Einstein’s postulates n If the velocity of light = c in frame K, the velocity of light = c – V in frame K’ ØThe Lorentz transformation satisfies Einstein’s postulates and also reduces to the Galilean transformation at low velocities ØA derivation is given in Thornton and Rex p 30 -31 5

Lorentz Transformation 6

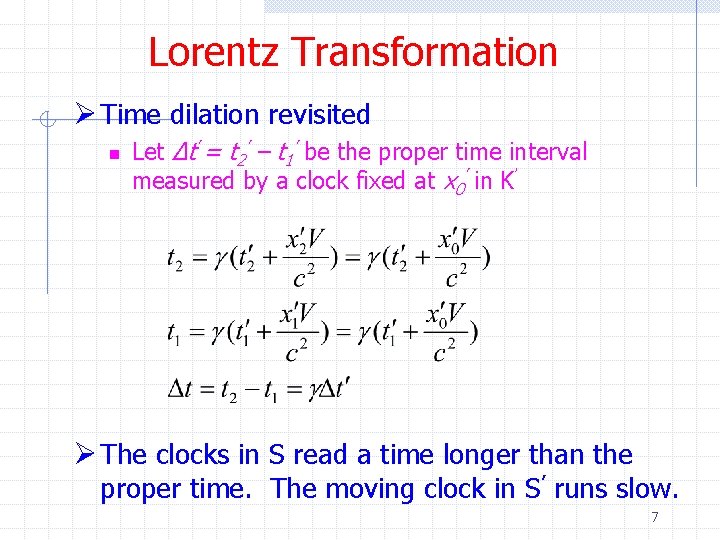
Lorentz Transformation Ø Time dilation revisited n Let Δt’ = t 2’ – t 1’ be the proper time interval measured by a clock fixed at x 0’ in K’ Ø The clocks in S read a time longer than the proper time. The moving clock in S’ runs slow. 7

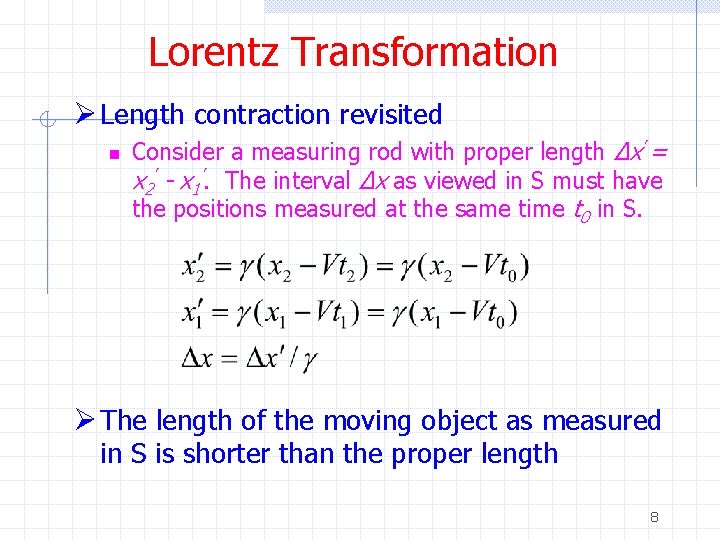
Lorentz Transformation Ø Length contraction revisited n Consider a measuring rod with proper length Δx’ = x 2’ - x 1’. The interval Δx as viewed in S must have the positions measured at the same time t 0 in S. Ø The length of the moving object as measured in S is shorter than the proper length 8

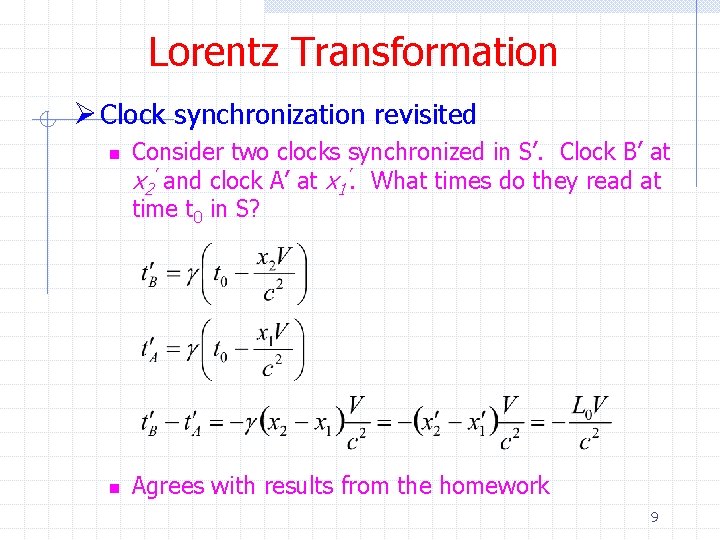
Lorentz Transformation Ø Clock synchronization revisited n n Consider two clocks synchronized in S’. Clock B’ at x 2’ and clock A’ at x 1’. What times do they read at time t 0 in S? Agrees with results from the homework 9

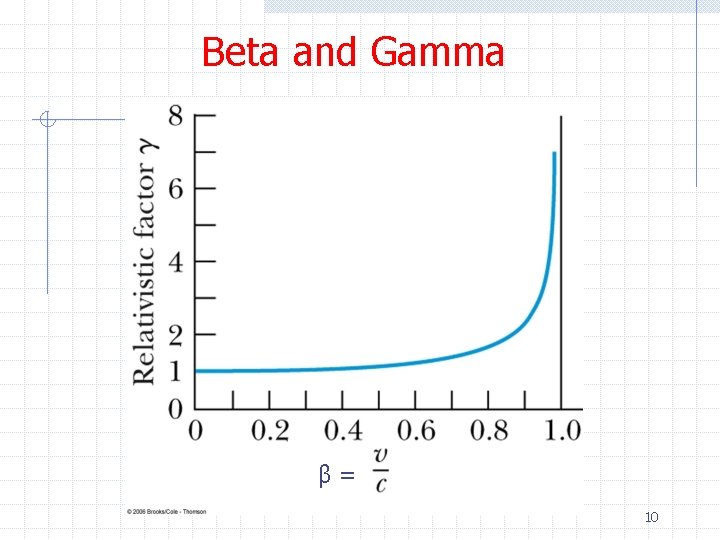
Beta and Gamma β= 10

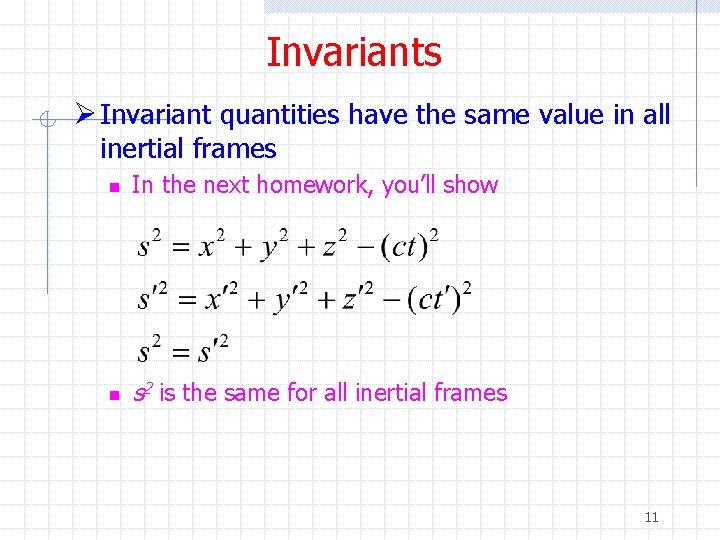
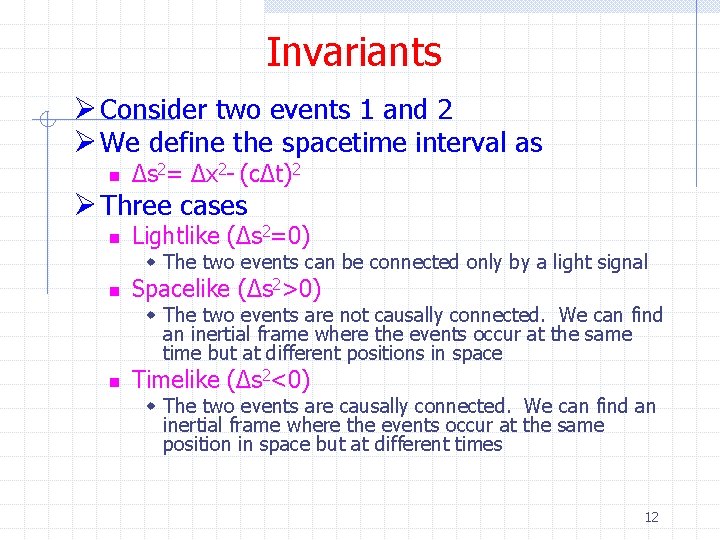
Invariants Ø Invariant quantities have the same value in all inertial frames n In the next homework, you’ll show n s 2 is the same for all inertial frames 11

Invariants Ø Consider two events 1 and 2 Ø We define the spacetime interval as n Δs 2= Δx 2 - (cΔt)2 Ø Three cases n Lightlike (Δs 2=0) w The two events can be connected only by a light signal n Spacelike (Δs 2>0) w The two events are not causally connected. We can find an inertial frame where the events occur at the same time but at different positions in space n Timelike (Δs 2<0) w The two events are causally connected. We can find an inertial frame where the events occur at the same position in space but at different times 12

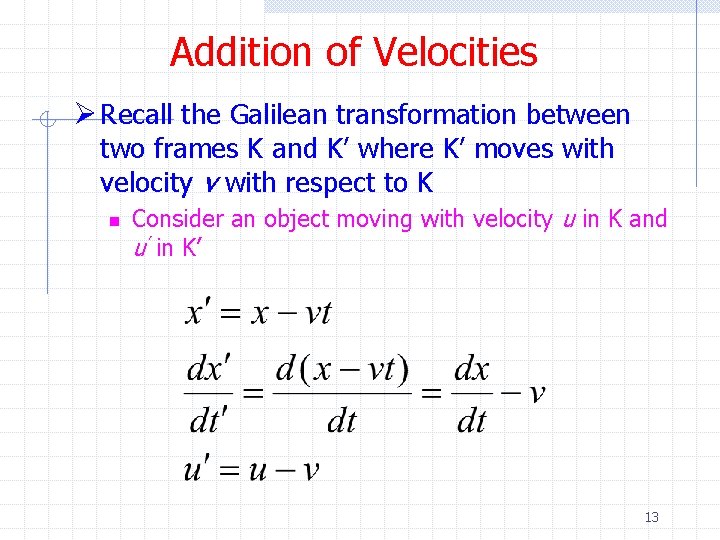
Addition of Velocities Ø Recall the Galilean transformation between two frames K and K’ where K’ moves with velocity v with respect to K n Consider an object moving with velocity u in K and u’ in K’ 13

Galilean Transformation Ø Note time is the same in both systems 14

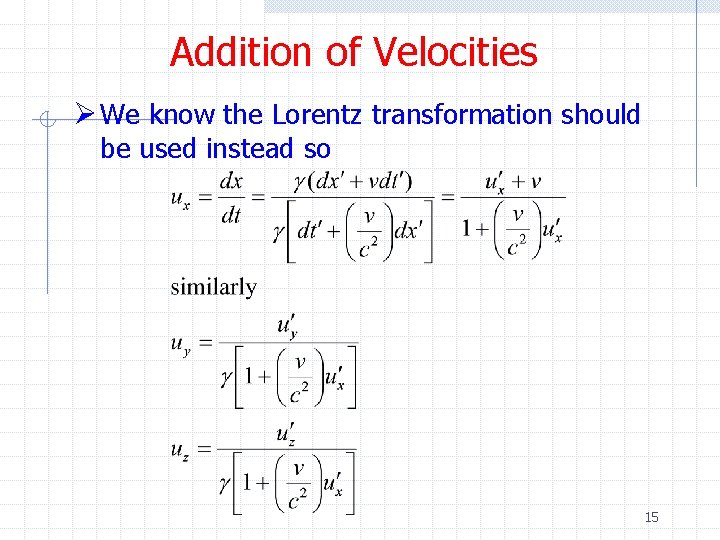
Addition of Velocities Ø We know the Lorentz transformation should be used instead so 15

Lorentz Transformation 16

Addition of Velocities Ø Swapping primed and unprimed variables and letting v go to –v 17

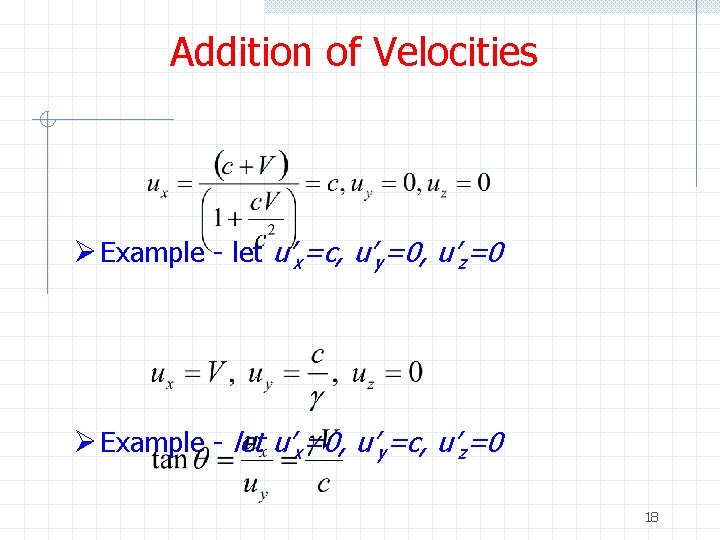
Addition of Velocities Ø Example - let u’x=c, u’y=0, u’z=0 Ø Example - let u’x=0, u’y=c, u’z=0 18

Addition of Velocities ØA rocket blasts off from the earth at v=0. 90 c ØA second rocket follows in the same direction at velocity 0. 98 c ØWhat is the relative velocity of the rockets using a Galilean transformation ØWhat is the relative velocity of the rockets using a Lorentz transformation? 19

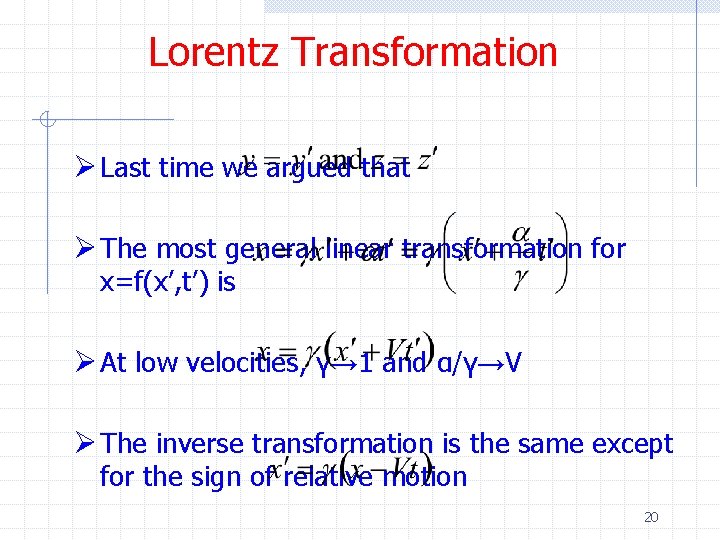
Lorentz Transformation Ø Last time we argued that Ø The most general linear transformation for x=f(x’, t’) is Ø At low velocities, γ→ 1 and α/γ→V Ø The inverse transformation is the same except for the sign of relative motion 20

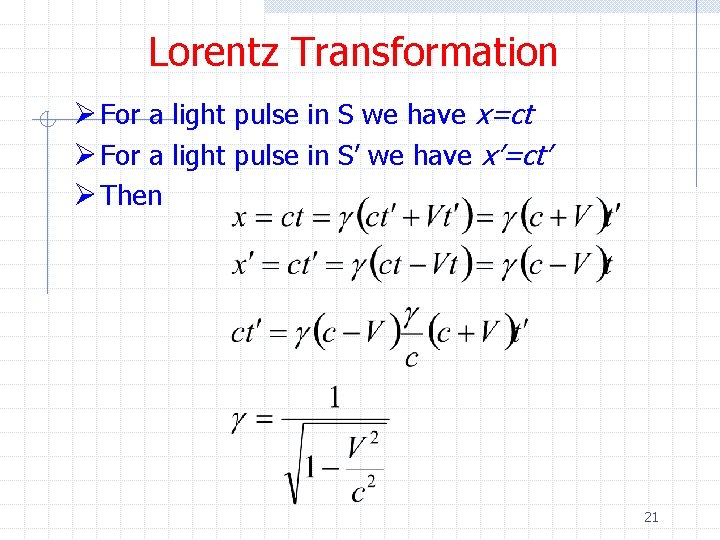
Lorentz Transformation Ø For a light pulse in S we have x=ct Ø For a light pulse in S’ we have x’=ct’ Ø Then 21

Lorentz Transformation Ø For the t transformation 22
 Relativity newton
Relativity newton Indeed
Indeed Special relativity equations
Special relativity equations Special vs general relativity
Special vs general relativity L
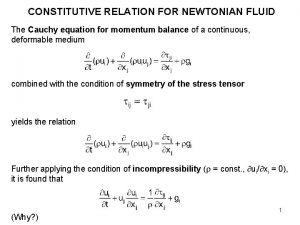
L Constitutive equation in fluid mechanics
Constitutive equation in fluid mechanics Mv mu
Mv mu Newtonian fluid formula
Newtonian fluid formula Modified newtonian dynamics
Modified newtonian dynamics Frame by frame animation programs
Frame by frame animation programs Pengertian animasi frame by frame
Pengertian animasi frame by frame Risanuri hidayat
Risanuri hidayat Frame relay frame format
Frame relay frame format Reference frame theory
Reference frame theory Frame of reference communication
Frame of reference communication Competitive frame of reference of coca-cola
Competitive frame of reference of coca-cola Contoh frame of reference dan field of experience
Contoh frame of reference dan field of experience Contoh field of experience
Contoh field of experience Frame of reference units
Frame of reference units Chapter 10 crafting the brand positioning
Chapter 10 crafting the brand positioning Frame of reference jacqui and aaron schiff
Frame of reference jacqui and aaron schiff Inertial and non inertial frame of reference
Inertial and non inertial frame of reference Inertial and non inertial frame of reference
Inertial and non inertial frame of reference