Electricity and magnetism dr in Marek Ossowski Institute

Electricity and magnetism dr inż. Marek Ossowski Institute of Electrical Engineering Systems I-12 Phone 42 631 25 15 mail: marekjerzyossowski@gmail. com marek. ossowski@p. lodz. pl Consultation hours: Thuesday, 11 – 12 room 14

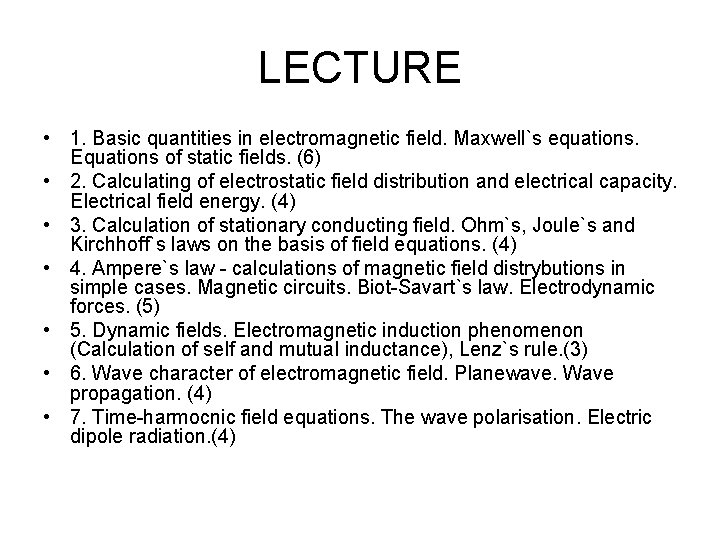
LECTURE • 1. Basic quantities in electromagnetic field. Maxwell`s equations. Equations of static fields. (6) • 2. Calculating of electrostatic field distribution and electrical capacity. Electrical field energy. (4) • 3. Calculation of stationary conducting field. Ohm`s, Joule`s and Kirchhoff`s laws on the basis of field equations. (4) • 4. Ampere`s law - calculations of magnetic field distrybutions in simple cases. Magnetic circuits. Biot-Savart`s law. Electrodynamic forces. (5) • 5. Dynamic fields. Electromagnetic induction phenomenon (Calculation of self and mutual inductance), Lenz`s rule. (3) • 6. Wave character of electromagnetic field. Planewave. Wave propagation. (4) • 7. Time-harmocnic field equations. The wave polarisation. Electric dipole radiation. (4)

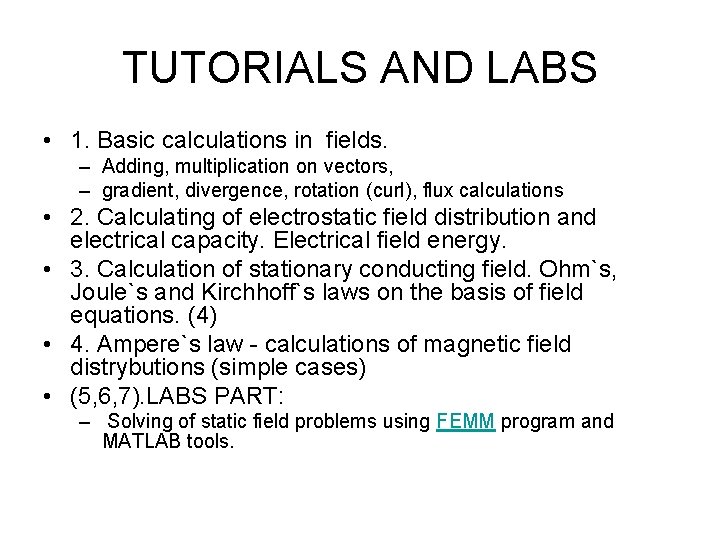
TUTORIALS AND LABS • 1. Basic calculations in fields. – Adding, multiplication on vectors, – gradient, divergence, rotation (curl), flux calculations • 2. Calculating of electrostatic field distribution and electrical capacity. Electrical field energy. • 3. Calculation of stationary conducting field. Ohm`s, Joule`s and Kirchhoff`s laws on the basis of field equations. (4) • 4. Ampere`s law - calculations of magnetic field distrybutions (simple cases) • (5, 6, 7). LABS PART: – Solving of static field problems using FEMM program and MATLAB tools.

Literature: • 1. Maciej Krakowski, Elektrotechnika Teoretyczna, tom II, Teoria pola. • 2. Andrzej Kuczyński, Podstawy Teorii Pola Elektromagnetycznego, part I. • 3. Richard P. Feynman, The Feynman Lectures on Physics, vol. 2. • 4. Fundamentals of Electromagnetics using MATLAB, Karl E. Lonngren

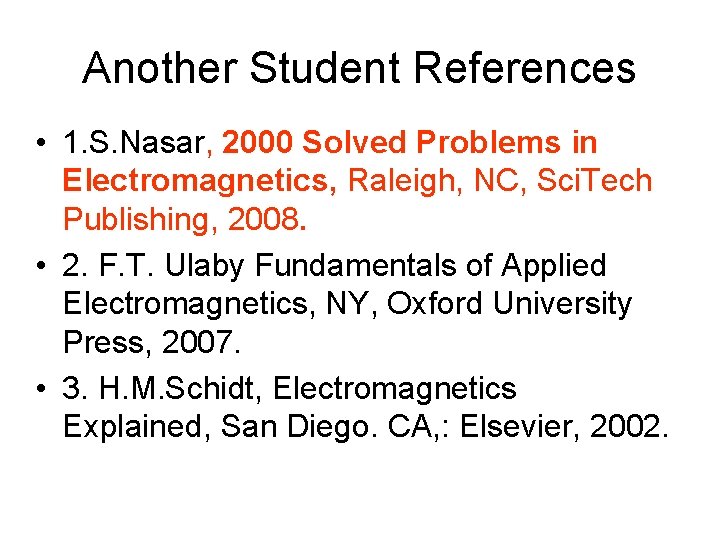
Another Student References • 1. S. Nasar, 2000 Solved Problems in Electromagnetics, Raleigh, NC, Sci. Tech Publishing, 2008. • 2. F. T. Ulaby Fundamentals of Applied Electromagnetics, NY, Oxford University Press, 2007. • 3. H. M. Schidt, Electromagnetics Explained, San Diego. CA, : Elsevier, 2002.

The object of our interest is electromagnetism – - the branch of classic physics. We will talk about electromagnetic phenomena around the charges and around the wires with the flowing current. We use notions „electromagnetic field”, „field of temperature”, „forces field”. What does the notion „field” mean? We know from physics, that – e. g. gravitation field - can do the work, if the mass will be introduced in the field area, so the feature of the field is having energy. If the physical field possesses energy, we can say it is the form of matter existing. In mathematics we can describe any physical phenomenon as a function determined in space. For each point in considered area we can find the function value. It can be scalar (like temperature) or vector (like the force, velocity). We say about spatial distribution of any physical value.

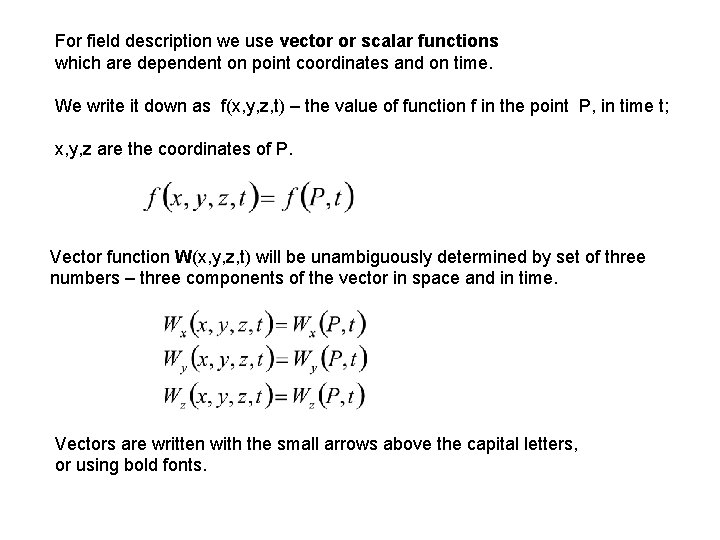
For field description we use vector or scalar functions which are dependent on point coordinates and on time. We write it down as f(x, y, z, t) – the value of function f in the point P, in time t; x, y, z are the coordinates of P. Vector function W(x, y, z, t) will be unambiguously determined by set of three numbers – three components of the vector in space and in time. Vectors are written with the small arrows above the capital letters, or using bold fonts.

Coordinate systems In 3 D space every point can be unambiguously described by three numbers called point coordinates. This ordered set of three numbers depends on chosen system of coordinates. The correct choice of coordinate system can make the field problem much simpler because its mathematical description will be easier.

Remarks: • • • Coordinate system- system which uses one or more numbers to uniqely determine the position of the points or other geometic elements on a manifold such as Euklidean space. Vector notation allows us the convenience of visualizing problems with or without the specification of coordinate systems. There are many orthogonal coordinate systems and one generalized coordinate system. ORTHOGONAL >> implies that every point in particular coordinate system can be defined as the intersection of threee mutually perpendicular surfaces in that coordinate system. In field theory we use (the most often) one of three systems:

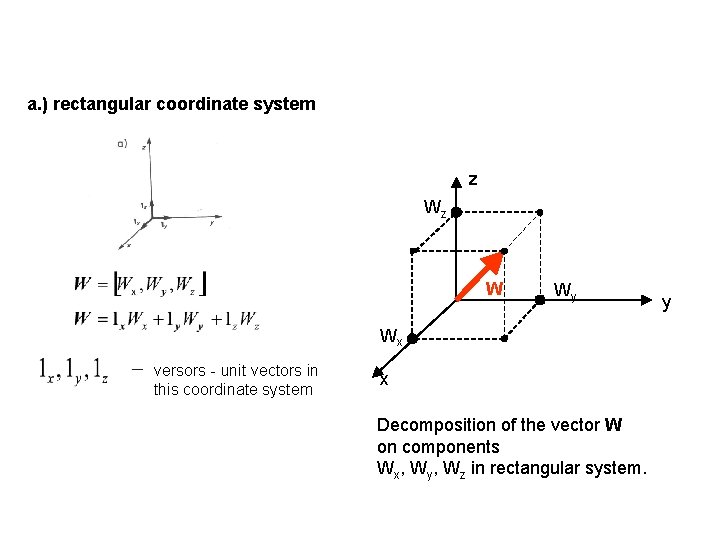
a. ) rectangular coordinate system z Wz W Wy Wx versors - unit vectors in this coordinate system x Decomposition of the vector W on components Wx, Wy, Wz in rectangular system. y

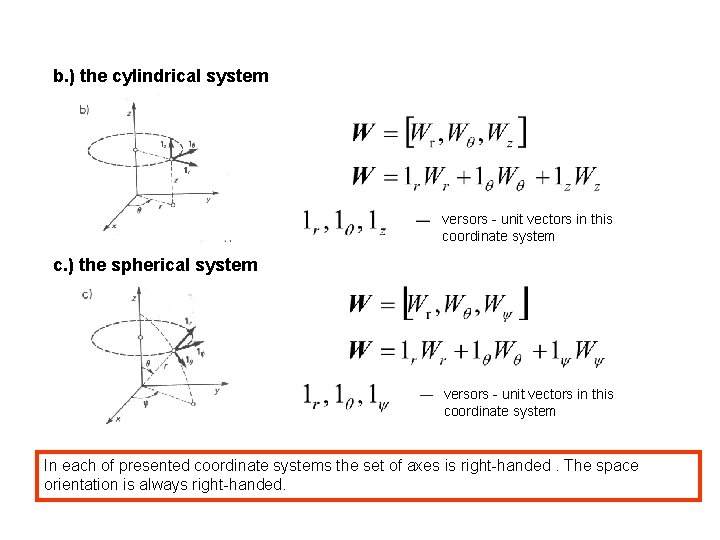
b. ) the cylindrical system versors - unit vectors in this coordinate system c. ) the spherical system versors - unit vectors in this coordinate system In each of presented coordinate systems the set of axes is right-handed. The space orientation is always right-handed.

Right-hand rule When we turn the screw its rotation creates the plane. The direction of the movement of the screw itself is always perpendicular to this plane. 1 z , 1ψ 1 y, 1θ 1 x , 1 r

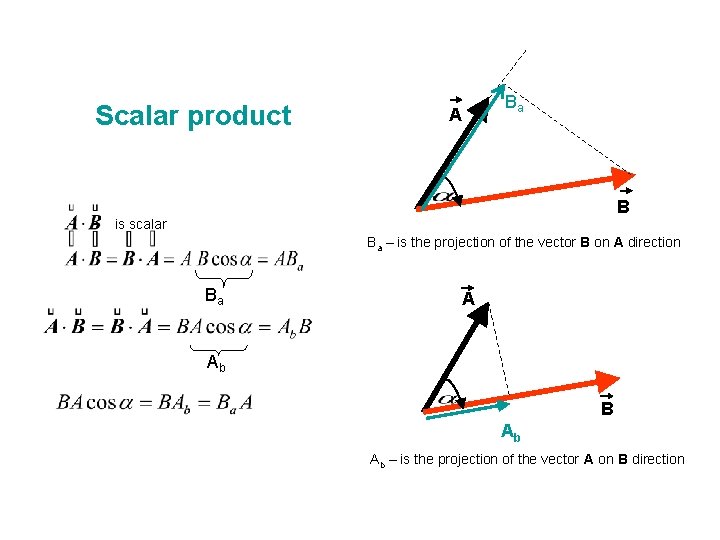
Scalar product - Ba A B is scalar Ba – is the projection of the vector B on A direction Ba A Ab B Ab Ab – is the projection of the vector A on B direction

: The measure of a vector (its value or lenght) is: Conclusion 1: Scalar product of two identical vectors equals the square of their lenght (their values) Conclusion 2: Scalar product of two parallel vectors equals the product of their numerical values. Scalar product of two perpendicular (orthogonal) vectors equals zero.

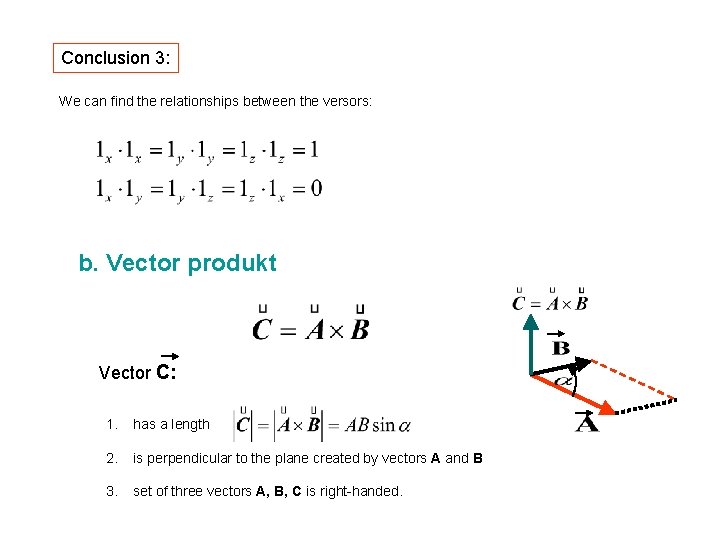
Conclusion 3: We can find the relationships between the versors: b. Vector produkt Vector C: 1. has a length 2. is perpendicular to the plane created by vectors A and B 3. set of three vectors A, B, C is right-handed.

Right-hand rule When we turn the screw its rotation creates the plane. The direction of the movement of the screw itself is always perpendicular to this plane. This rule is also called corkscrew rule. For vector product: If we turn the screw from first vector to the other (A to B), the movement of the screw indicates the vector product direction (C).

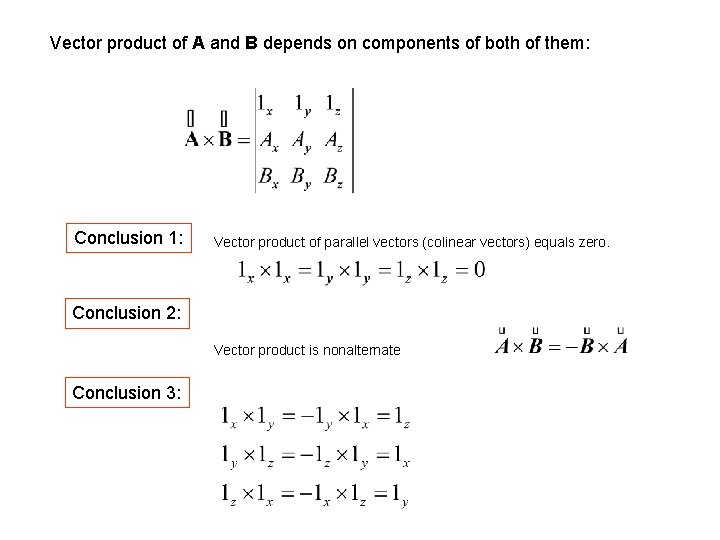
Vector product of A and B depends on components of both of them: Conclusion 1: Vector product of parallel vectors (colinear vectors) equals zero. Conclusion 2: Vector product is nonalternate Conclusion 3:

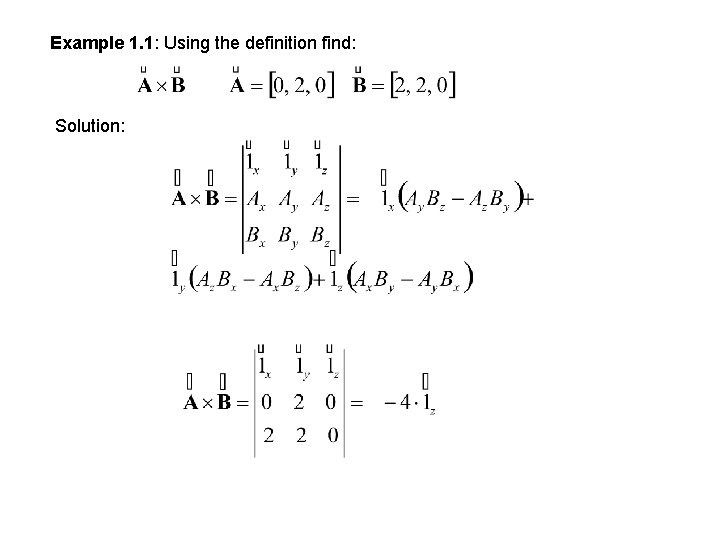
Example 1. 1: Using the definition find: Solution:

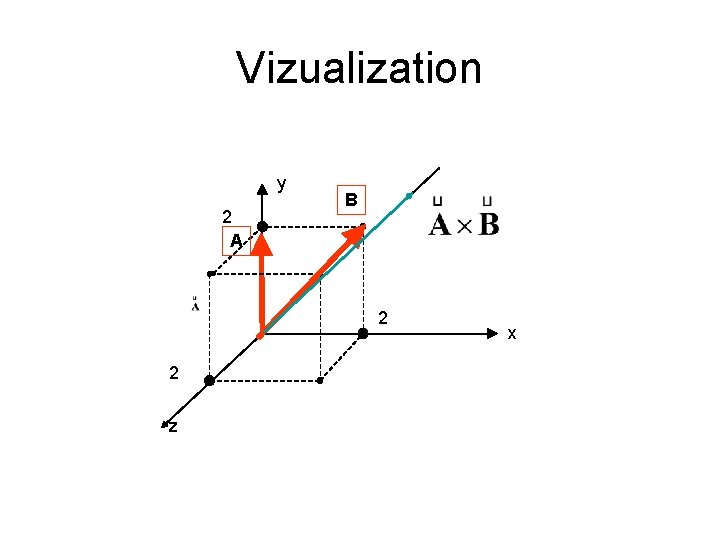
Vizualization y 2 A B 2 2 z x

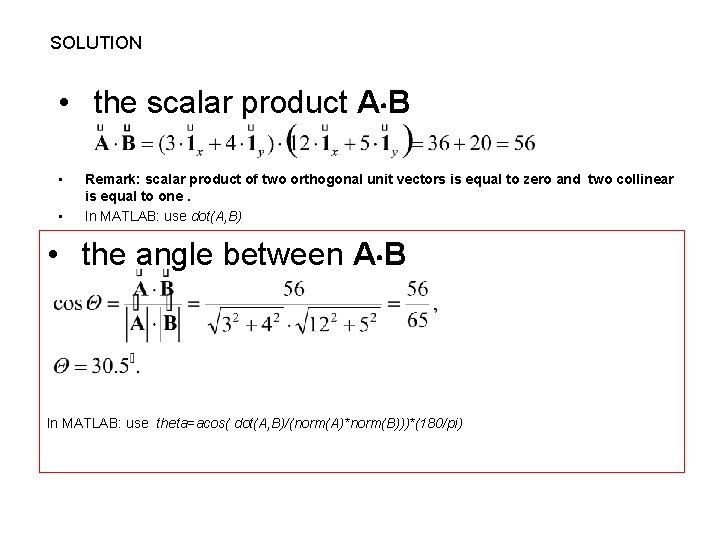
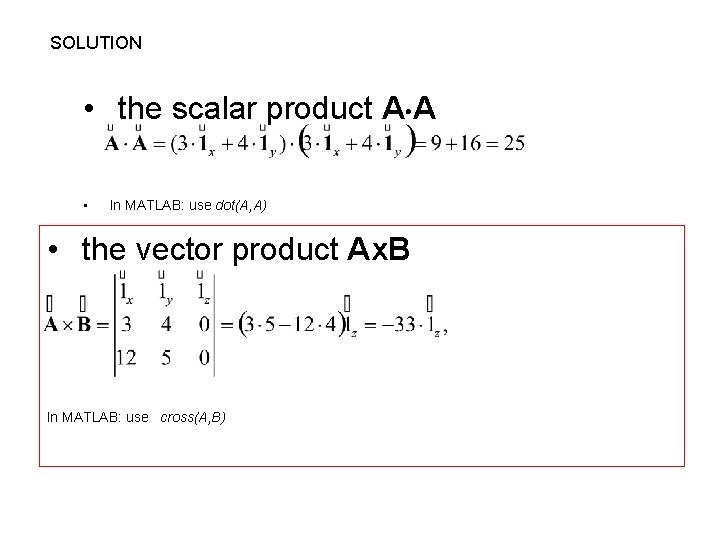
Example 1. 2: Given the two vectors in Cartesian coordinates: evaluate the following quantities: • • • the scalar product A B the angle between the two vectors the scalar product A A the vector product Ax. B Remark: The MATLAB notation for the vectors is:

SOLUTION • the scalar product A B • • Remark: scalar product of two orthogonal unit vectors is equal to zero and two collinear is equal to one. In MATLAB: use dot(A, B) • the angle between A B In MATLAB: use theta=acos( dot(A, B)/(norm(A)*norm(B)))*(180/pi)

SOLUTION • the scalar product A A • In MATLAB: use dot(A, A) • the vector product Ax. B In MATLAB: use cross(A, B)

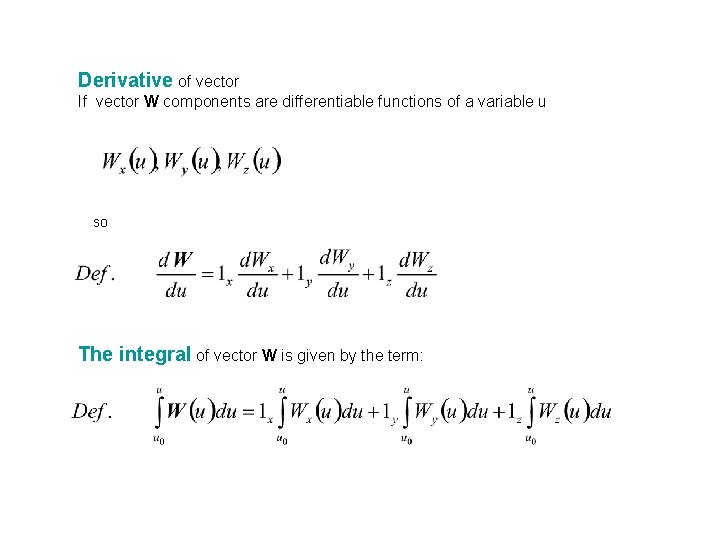
Derivative of vector If vector W components are differentiable functions of a variable u so The integral of vector W is given by the term:

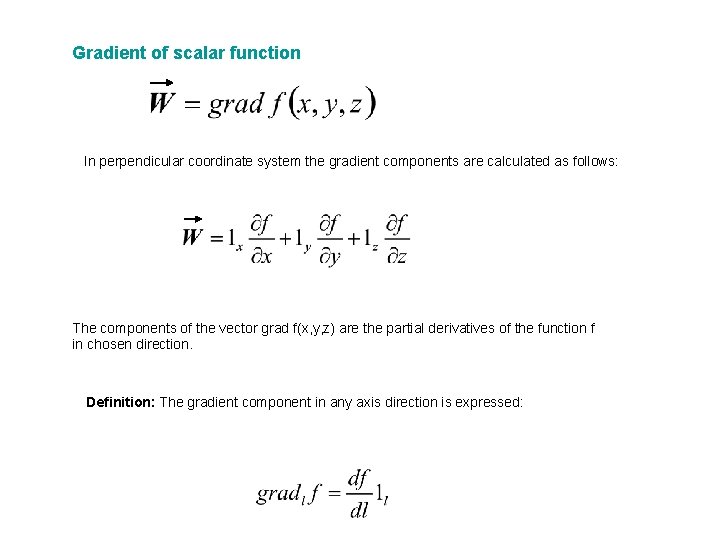
Gradient of scalar function In perpendicular coordinate system the gradient components are calculated as follows: The components of the vector grad f(x, y, z) are the partial derivatives of the function f in chosen direction. Definition: The gradient component in any axis direction is expressed:

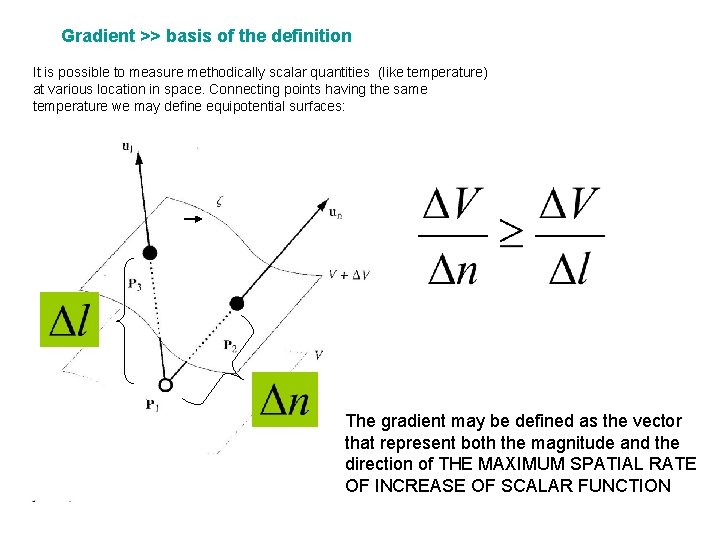
Gradient >> basis of the definition It is possible to measure methodically scalar quantities (like temperature) at various location in space. Connecting points having the same temperature we may define equipotential surfaces: The gradient may be defined as the vector that represent both the magnitude and the direction of THE MAXIMUM SPATIAL RATE OF INCREASE OF SCALAR FUNCTION

The surface on which scalar function f(x, y, z) has a constant value is called equiscalar surface Conclusion 1: Components of the vector W=grad f(x, y, z) in direction of any axis laying on the equiskalar surface is always equal zero Conclusion 2: Vector W=grad f(x, y, z) is perpendicular to the equiscalar surface. Conclusion 3: Vector W=grad f(x, y, z) is directed to increasing values of the function f(x, y, z). . Vector W= - grad f(x, y, z) is directed to decreasing values of the function f(x, y, z).

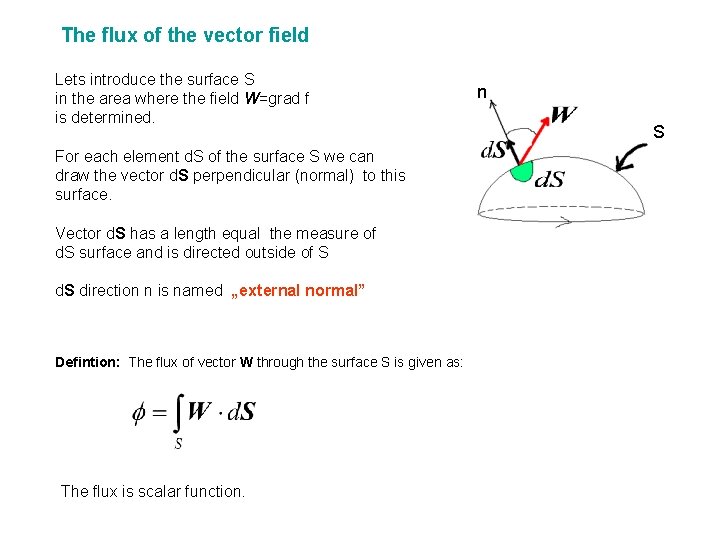
The flux of the vector field Lets introduce the surface S in the area where the field W=grad f is determined. For each element d. S of the surface S we can draw the vector d. S perpendicular (normal) to this surface. Vector d. S has a length equal the measure of d. S surface and is directed outside of S direction n is named „external normal” Defintion: The flux of vector W through the surface S is given as: The flux is scalar function. n S

Scalar product of two perpendicular vectors =0, so the flux of the vector tangential to the surface =0 (because the angle between W i d. S equals 90º) Conclusion 1: The flux of the vector W is unequal 0, when the vector has the normal projection. Conclusion 2: The flux directed outside from the surface S is positive, The flux directed into the surface S is negative. It results from the assumpted direction of vector d. S. n n S S

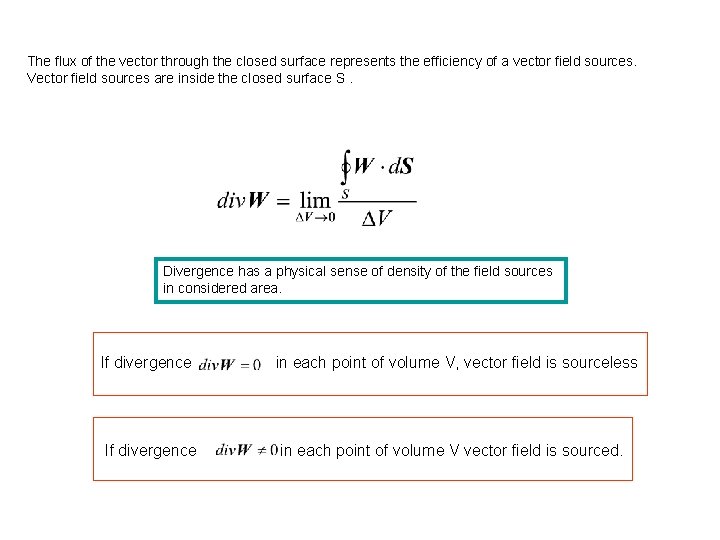
Divergence (1) in out • The divergence operator is useful in determining if there is a source or a sink at location in space where vector field exists.

Divergence (2) This operation transforms wektor field W(x, y, z) into scalar spatial function v(x, y, z) If vector W components are differentiable functions of a variables x, y, z in considered area the divergence can be calculated like that: Def: Divergence of vector W is the term:

The flux of the vector through the closed surface represents the efficiency of a vector field sources. Vector field sources are inside the closed surface S. Divergence has a physical sense of density of the field sources in considered area. If divergence in each point of volume V, vector field is sourceless If divergence in each point of volume V vector field is sourced.

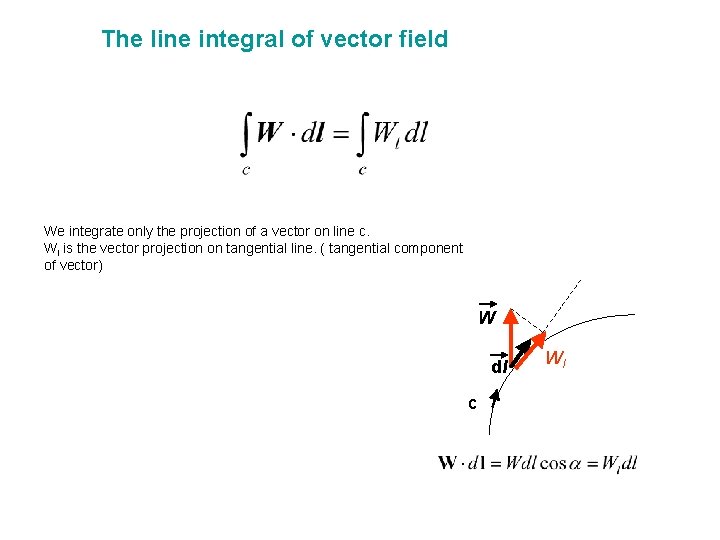
The line integral of vector field We integrate only the projection of a vector on line c. Wl is the vector projection on tangential line. ( tangential component of vector) W dl c Wl

Def. The line integral of the vector along the close line is called circulation of the vector Vector rotation Operation „rotation” transforms vector W to the another vector

Let`s extend this determinant with respect of the first line: A field is rotational if rot W≠ 0. A field is unrotational if rot. W=0.

Rotation (curl) • Small padding wheel in a flowing river – In the middle not rotate since velocity on either side of the center will be the same – Near the edge of river rotation is present since velocity will be less than in a region furter from edge – The curl operation determines both the sense and magnitude of the rotation

REMARK: Operator nabla Nabla is formal vector, it means, it has the components but it hasn`t the lenght Using operator nabla we can write vector operatior shorter.