Atomic Physics The Dirac equation for a oneelectron





- Slides: 12

Atomic Physics The Dirac equation for a one-electron atom Part 1

Controlling the Quantum World: http: //www. nap. edu/catalog. php? record_id=11705 Summary Atomic, molecular, and optical (AMO) science demonstrates powerfully the ties of fundamental physics to society. Its very name reflects three of 20 th century physics’ greatest advances: the establishment of the atom as a building block of matter; the development of quantum mechanics, which made it possible to understand the inner workings of atoms and molecules; and the invention of the laser. Navigation by the stars gave way to navigation by clocks, which in turn has given way to today’s navigation by atomic clocks. Laser surgery has replaced the knife for the most delicate operations. Our nation’s defense depends on rapid deployment using global positioning satellites, laser-guided weapons, and secure communication, all derived directly from fundamental advances in AMO science. Homeland security relies on a multitude of screening technologies based on AMO research to detect toxins in the air and hidden weapons in luggage or on persons, to name a few. New drugs are now designed with the aid of x-ray scattering to determine their structure at the molecular level using AMO-based precision measurement techniques. And the global economy depends critically on high-speed telecommunication by laser light sent over thin optical fibers encircling the globe. 1 These advances, made possible by the scientists in this field, touched many areas of societal importance in the past century, and AMO scientists have been rewarded with numerous Nobel prizes over the past decade, including the 2005 prize in physics.

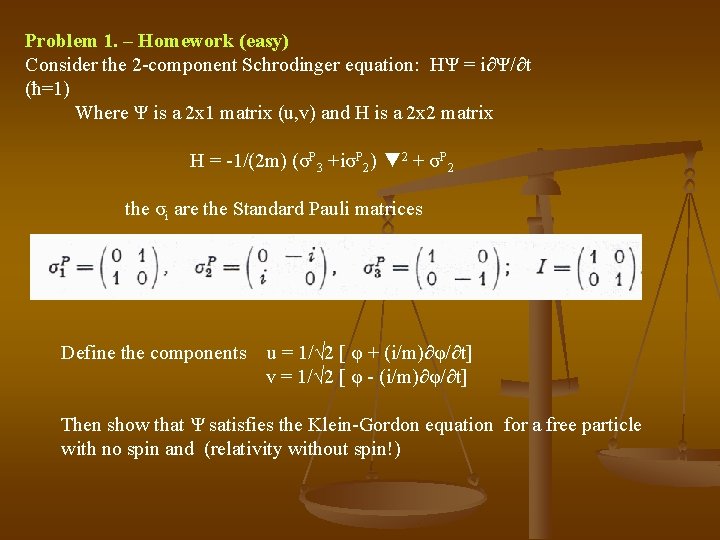
Problem 1. – Homework (easy) Consider the 2 -component Schrodinger equation: HΨ = i∂Ψ/∂t (ħ=1) Where Ψ is a 2 x 1 matrix (u, v) and H is a 2 x 2 matrix H = -1/(2 m) (σP 3 +iσP 2) ▼ 2 + σP 2 the σi are the Standard Pauli matrices Define the components u = 1/√ 2 [ φ + (i/m)∂φ/∂t] v = 1/√ 2 [ φ - (i/m)∂φ/∂t] Then show that Ψ satisfies the Klein-Gordon equation for a free particle with no spin and (relativity without spin!)

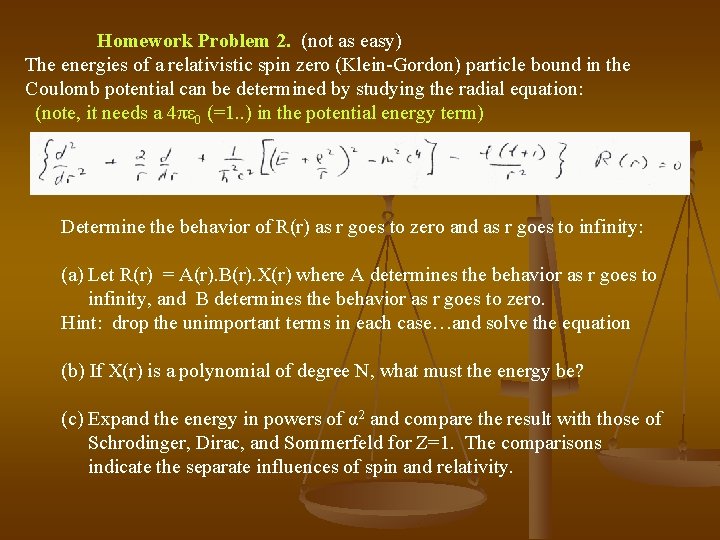
Homework Problem 2. (not as easy) The energies of a relativistic spin zero (Klein-Gordon) particle bound in the Coulomb potential can be determined by studying the radial equation: (note, it needs a 4πε 0 (=1. . ) in the potential energy term) Determine the behavior of R(r) as r goes to zero and as r goes to infinity: (a) Let R(r) = A(r). B(r). X(r) where A determines the behavior as r goes to infinity, and B determines the behavior as r goes to zero. Hint: drop the unimportant terms in each case…and solve the equation (b) If X(r) is a polynomial of degree N, what must the energy be? (c) Expand the energy in powers of α 2 and compare the result with those of Schrodinger, Dirac, and Sommerfeld for Z=1. The comparisons indicate the separate influences of spin and relativity.

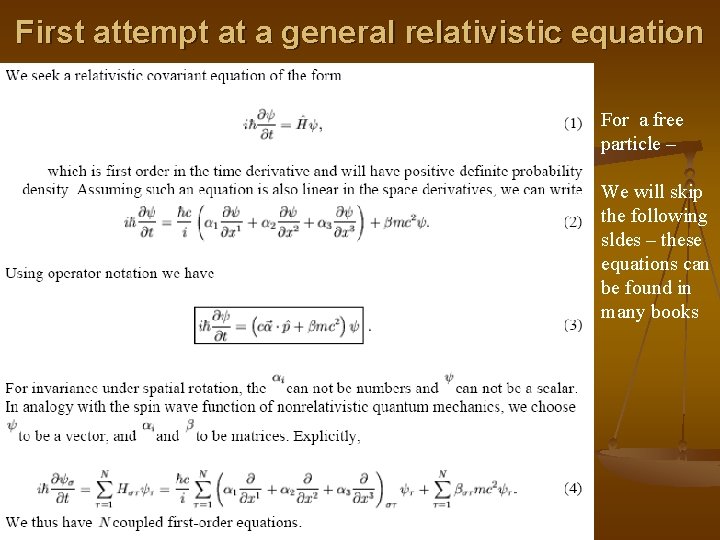
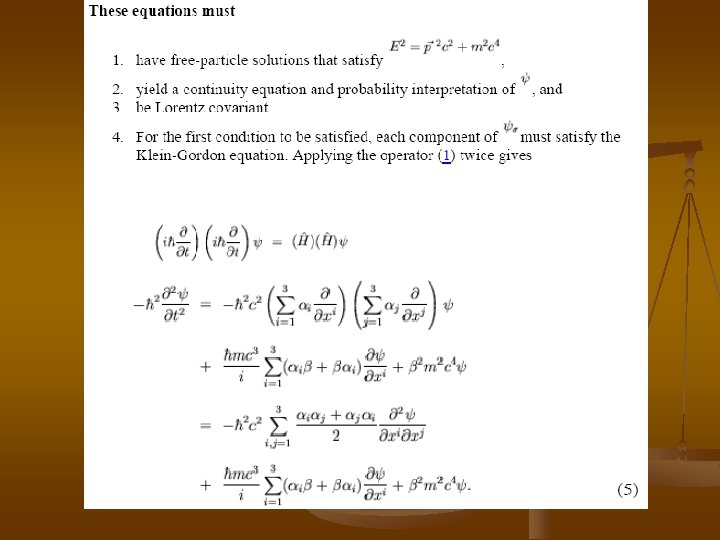
First attempt at a general relativistic equation For a free particle – We will skip the following sldes – these equations can be found in many books




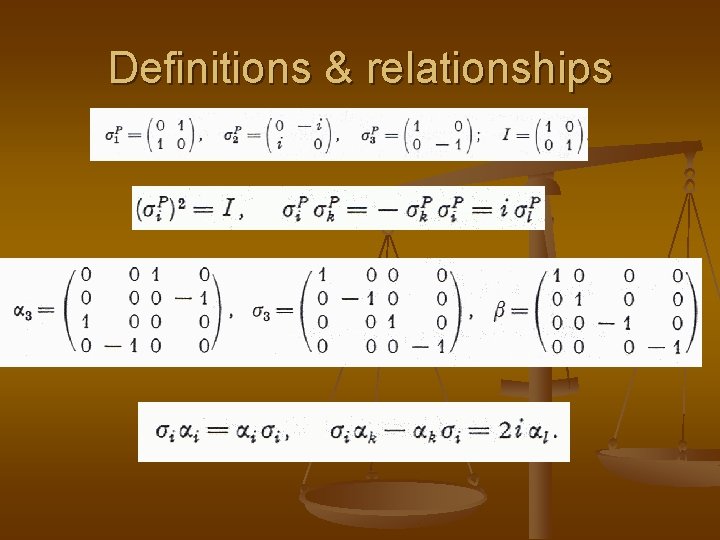
Definitions & relationships

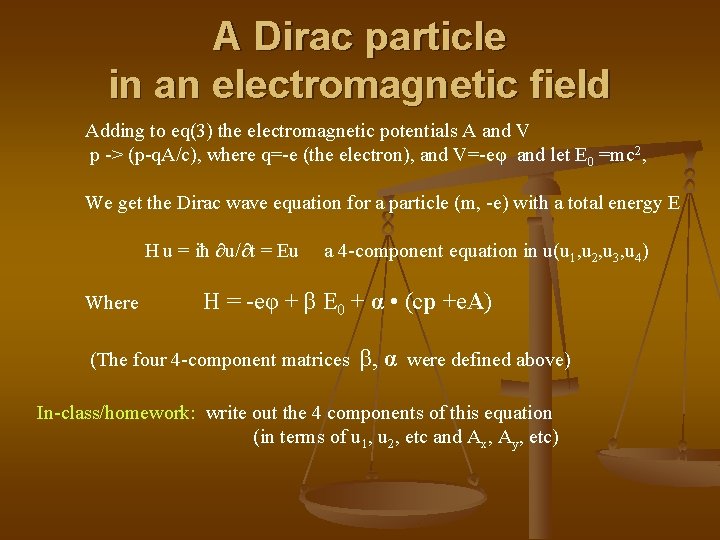
A Dirac particle in an electromagnetic field Adding to eq(3) the electromagnetic potentials A and V p -> (p-q. A/c), where q=-e (the electron), and V=-eφ and let E 0 =mc 2, We get the Dirac wave equation for a particle (m, -e) with a total energy E H u = iħ ∂u/∂t = Eu Where a 4 -component equation in u(u 1, u 2, u 3, u 4) H = -eφ + β E 0 + α • (cp +e. A) (The four 4 -component matrices β, α were defined above) In-class/homework: write out the 4 components of this equation (in terms of u 1, u 2, etc and Ax, Ay, etc)

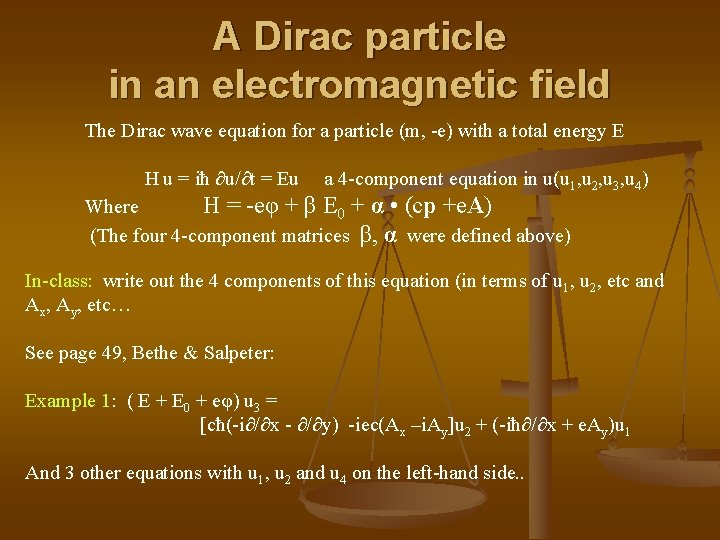
A Dirac particle in an electromagnetic field The Dirac wave equation for a particle (m, -e) with a total energy E H u = iħ ∂u/∂t = Eu a 4 -component equation in u(u 1, u 2, u 3, u 4) Where H = -eφ + β E 0 + α • (cp +e. A) (The four 4 -component matrices β, α were defined above) In-class: write out the 4 components of this equation (in terms of u 1, u 2, etc and Ax, Ay, etc… See page 49, Bethe & Salpeter: Example 1: ( E + E 0 + eφ) u 3 = [cħ(-i∂/∂x - ∂/∂y) -iec(Ax –i. Ay]u 2 + (-iħ∂/∂x + e. Ay)u 1 And 3 other equations with u 1, u 2 and u 4 on the left-hand side. .

Comments on the Dirac equation 1. – Not obviously Lorentz invariant – ie covariant – we will show this below. 2 (a) Define the scalar product of 2 Dirac wavefunction vectors <v*u> = ∫ d 3 r vρ*(r)uρ(r) where ρ is a “repeated index” 1 to 4 2 (b) Usual Normalization = <u*u> = 1 3. Similarly, an eigenvalue is calculated as: <M> = ∫ d 3 r uρ*(r)Mρσuσ(r) We will (a) show that the Dirac equation can be written in covariant form, (b) introduce the Pauli approximation (reduction to a 2 component form), (c) solve the equation exactly for a central Coulomb 1/r potential
 Dirac equation
Dirac equation Time independent schrodinger equation
Time independent schrodinger equation Is atomic mass and relative atomic mass the same
Is atomic mass and relative atomic mass the same Atomic trends
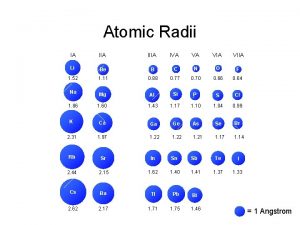
Atomic trends Ionic radius trend
Ionic radius trend Atomic weight of oxygen
Atomic weight of oxygen Atomic mass and atomic number difference
Atomic mass and atomic number difference Atomic number vs atomic radius
Atomic number vs atomic radius Inverse fourier transform of dirac delta function
Inverse fourier transform of dirac delta function Equazione di dirac
Equazione di dirac Conjugate matrix
Conjugate matrix Dirac delta fonksiyonu
Dirac delta fonksiyonu Experimento de la lamina de oro de ernest rutherford
Experimento de la lamina de oro de ernest rutherford