3 3 Rules for Differentiation AKA Shortcuts Review






- Slides: 18

3. 3 Rules for Differentiation AKA “Shortcuts”

Review from 3. 2 • 4 places derivatives do not exist: ▫ ▫ Corner Cusp Vertical tangent (where derivative is undefined) Discontinuity (jump, hole, vertical asymptote, infinite oscillation) • In other words, a function is differentiable everywhere in its domain if its graph is smooth and continuous.

3. 2 Intermediate Value Theorem for Derivatives • If a and b are any two points in an interval on which f is differentiable, then f’ takes on every value between f’(a) and f’(b).

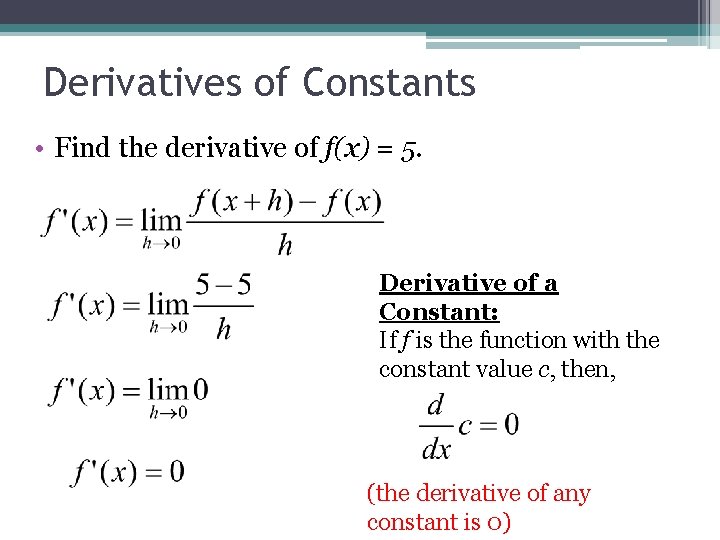
Derivatives of Constants • Find the derivative of f(x) = 5. Derivative of a Constant: If f is the function with the constant value c, then, (the derivative of any constant is 0)

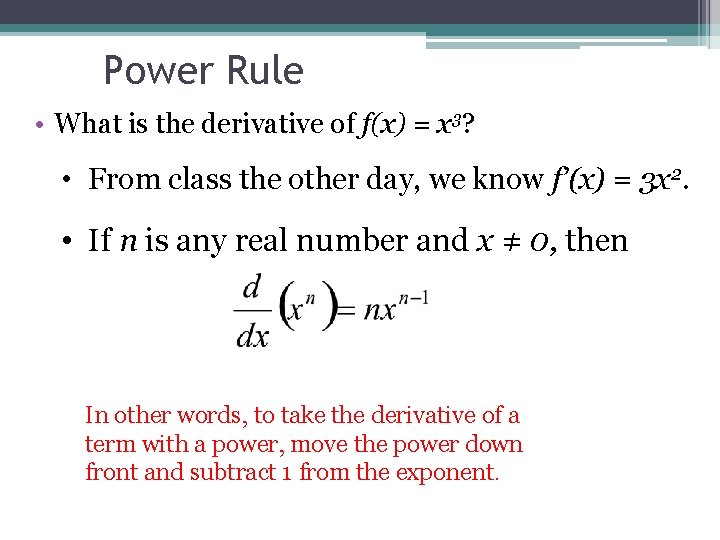
Power Rule • What is the derivative of f(x) = x 3? • From class the other day, we know f’(x) = 3 x 2. • If n is any real number and x ≠ 0, then In other words, to take the derivative of a term with a power, move the power down front and subtract 1 from the exponent.

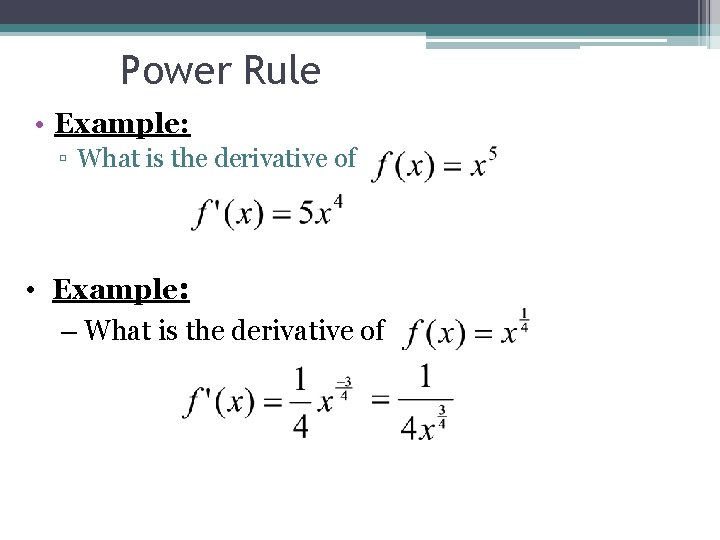
Power Rule • Example: ▫ What is the derivative of • Example: – What is the derivative of

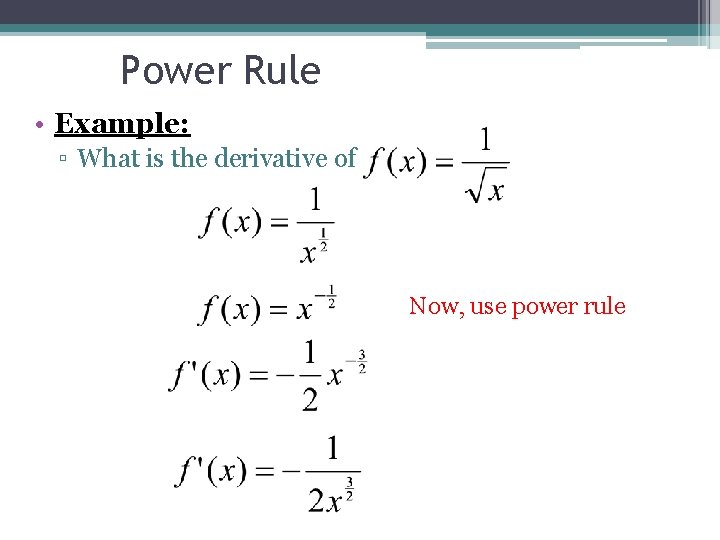
Power Rule • Example: ▫ What is the derivative of Now, use power rule

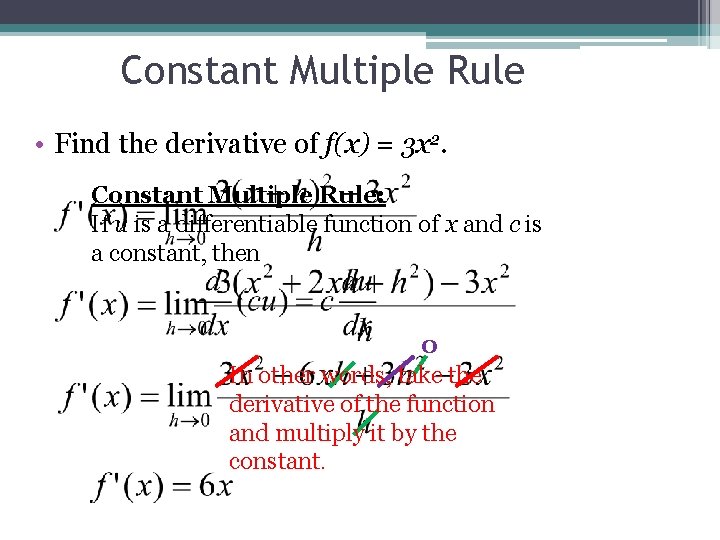
Constant Multiple Rule • Find the derivative of f(x) = 3 x 2. Constant Multiple Rule: If u is a differentiable function of x and c is a constant, then 0 In other words, take the derivative of the function and multiply it by the constant.

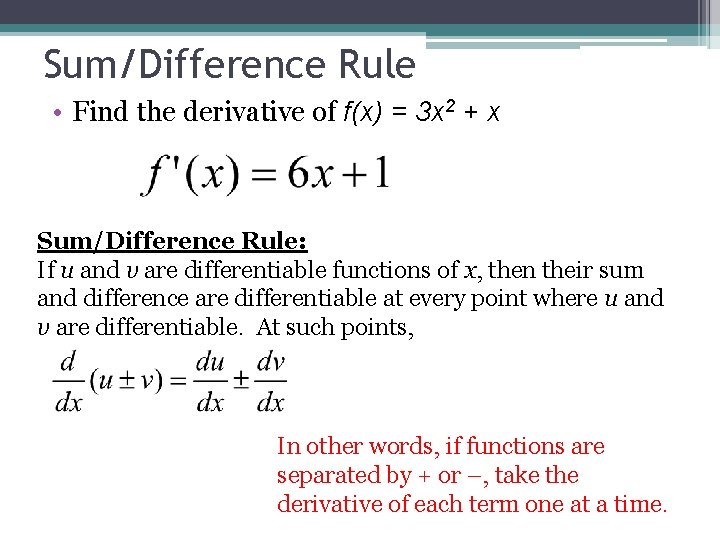
Sum/Difference Rule • Find the derivative of f(x) = 3 x 2 + x Sum/Difference Rule: If u and v are differentiable functions of x, then their sum and difference are differentiable at every point where u and v are differentiable. At such points, In other words, if functions are separated by + or –, take the derivative of each term one at a time.

Example • Find where horizontal tangent occurs for the function f(x) = 3 x 3 + 4 x 2 – 1. A horizontal tangent occurs when the slope (derivative) equals 0.

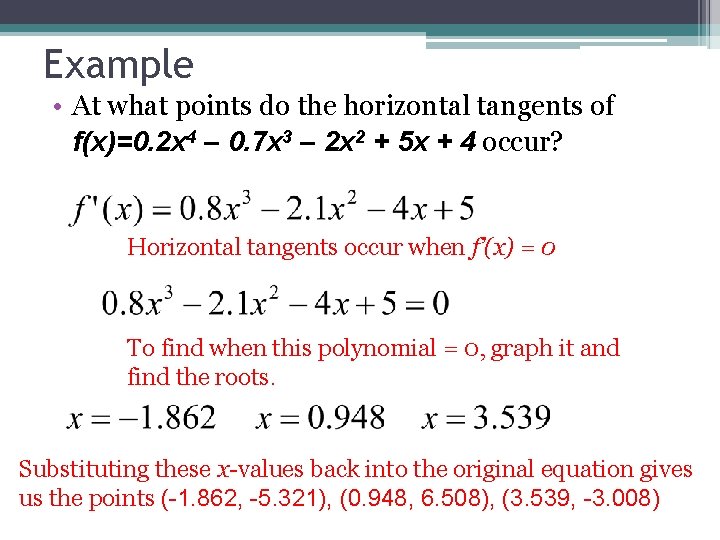
Example • At what points do the horizontal tangents of f(x)=0. 2 x 4 – 0. 7 x 3 – 2 x 2 + 5 x + 4 occur? Horizontal tangents occur when f’(x) = 0 To find when this polynomial = 0, graph it and find the roots. Substituting these x-values back into the original equation gives us the points (-1. 862, -5. 321), (0. 948, 6. 508), (3. 539, -3. 008)

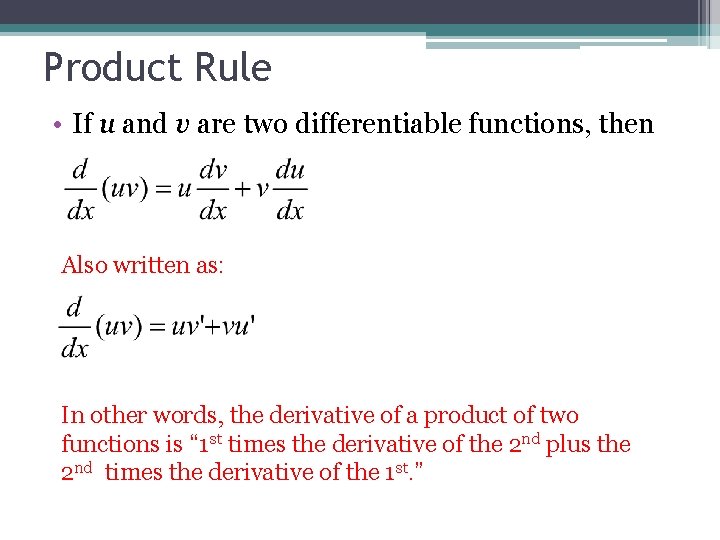
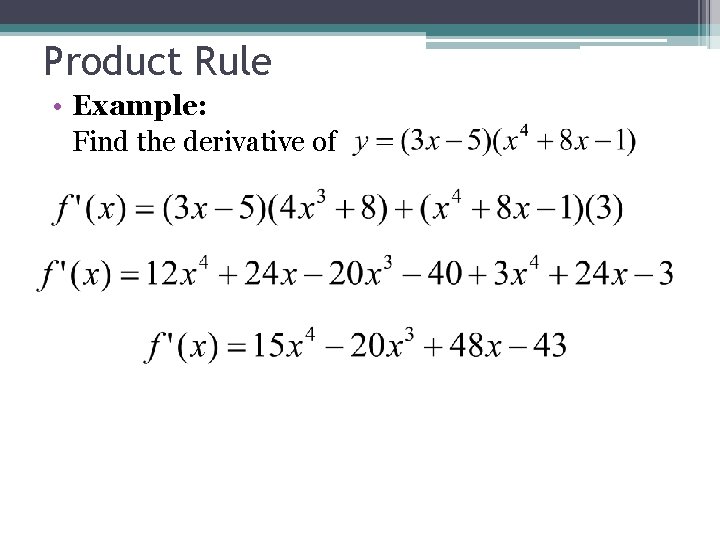
Product Rule • If u and v are two differentiable functions, then Also written as: In other words, the derivative of a product of two functions is “ 1 st times the derivative of the 2 nd plus the 2 nd times the derivative of the 1 st. ”

Product Rule • Example: Find the derivative of

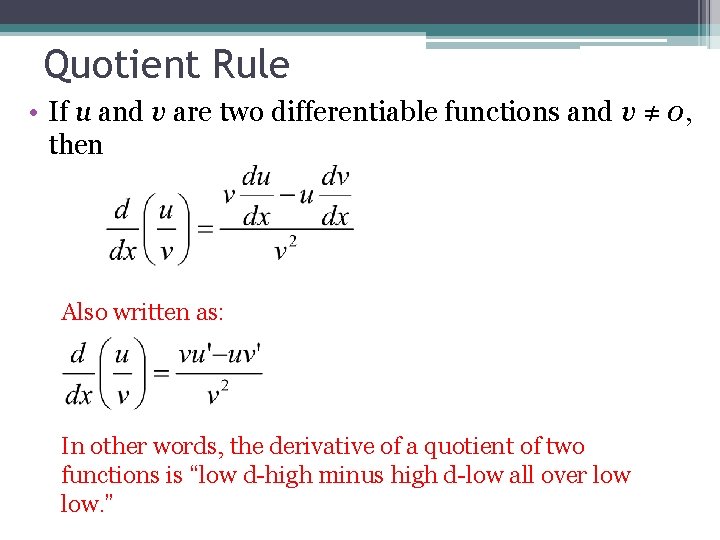
Quotient Rule • If u and v are two differentiable functions and v ≠ 0, then Also written as: In other words, the derivative of a quotient of two functions is “low d-high minus high d-low all over low. ”

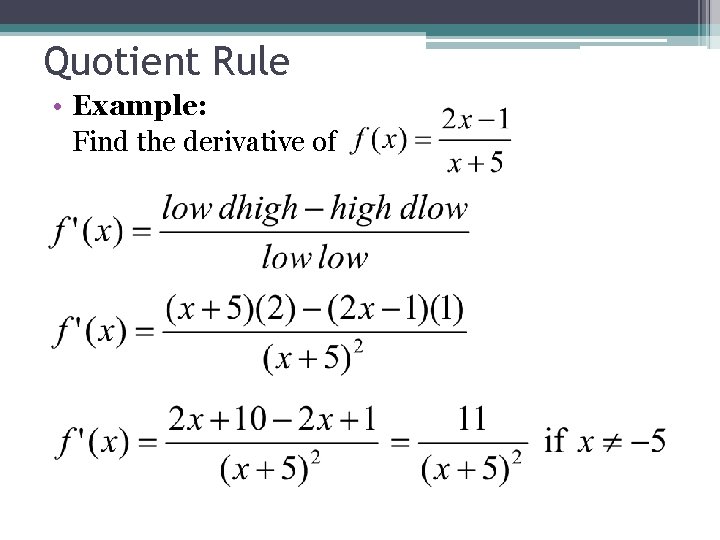
Quotient Rule • Example: Find the derivative of

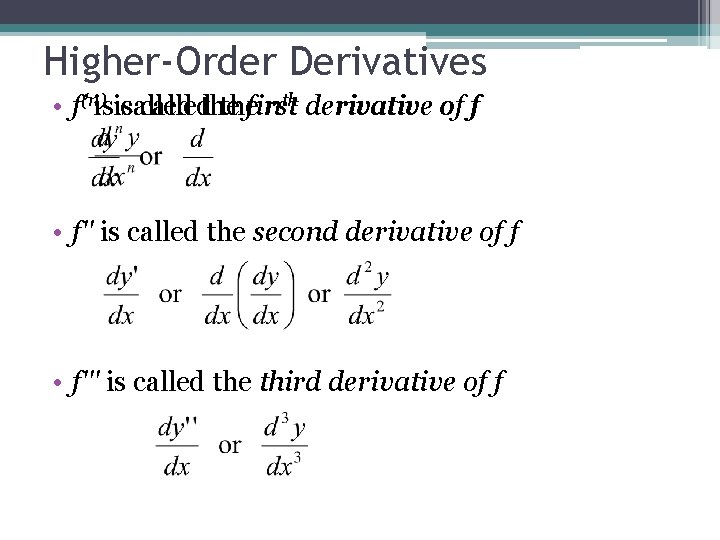
Higher-Order Derivatives • f’ f(n)isiscalledthe thefirst nth derivative of f • f'' is called the second derivative of f • f''' is called the third derivative of f

Higher-Order Derivatives • Example Find the first four derivatives of

Friday Classwork: • Section 3. 3 ▫ (#1 -9 odd, 15 -23 odd, 25, 27, 29, 33 -37 odd, 46)
 Differentiation shortcuts
Differentiation shortcuts Ukcat test dates 2020
Ukcat test dates 2020 Incrementing shortcuts
Incrementing shortcuts Congruent triangle shortcuts
Congruent triangle shortcuts Colab.google.con
Colab.google.con Polynomial shortcuts
Polynomial shortcuts Relfexive property
Relfexive property Gedit find and replace
Gedit find and replace Emotional shortcuts
Emotional shortcuts Keyboard shortcuts instead of mouse
Keyboard shortcuts instead of mouse Exponent shortcuts
Exponent shortcuts Screencastify meaning
Screencastify meaning Shortcuts used in judging others
Shortcuts used in judging others Rules of implicit differentiation
Rules of implicit differentiation General power rule derivative
General power rule derivative Chain rule of differentiation
Chain rule of differentiation Basic rule of rates
Basic rule of rates Basic differentiation rules homework
Basic differentiation rules homework Derivative 數學
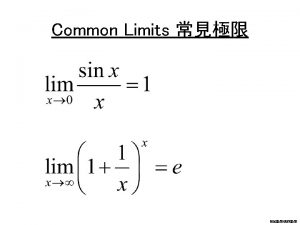
Derivative 數學