Lecture 5 Elementary Matrix Methid For find Inverse









- Slides: 28

Lecture 5 Elementary Matrix Methid For find Inverse

1. 5 Elementary Matrices and a Method for Finding A-1

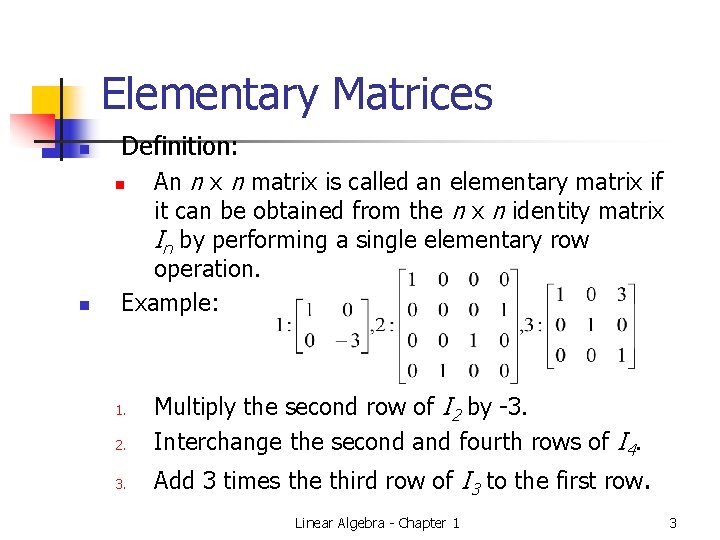
Elementary Matrices n n Definition: n An n x n matrix is called an elementary matrix if it can be obtained from the n x n identity matrix In by performing a single elementary row operation. Example: 2. Multiply the second row of I 2 by -3. Interchange the second and fourth rows of I 4. 3. Add 3 times the third row of I 3 to the first row. 1. Linear Algebra - Chapter 1 3

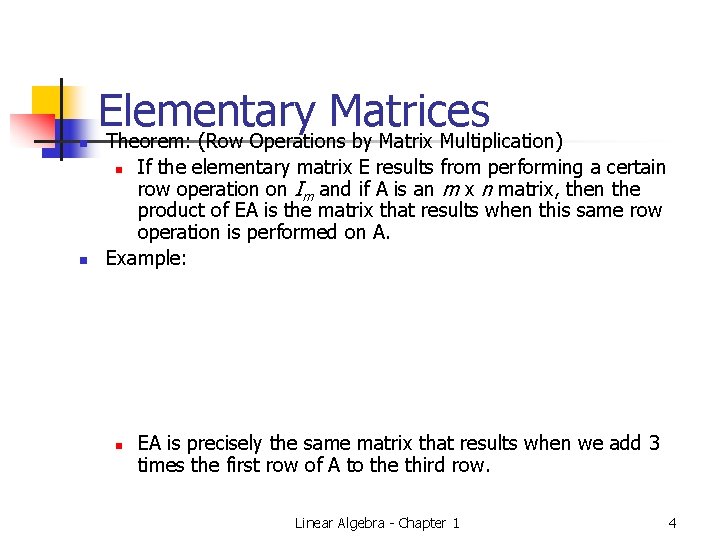
n Elementary Matrices Theorem: (Row Operations by Matrix Multiplication) If the elementary matrix E results from performing a certain row operation on Im and if A is an m x n matrix, then the product of EA is the matrix that results when this same row operation is performed on A. Example: n n n EA is precisely the same matrix that results when we add 3 times the first row of A to the third row. Linear Algebra - Chapter 1 4

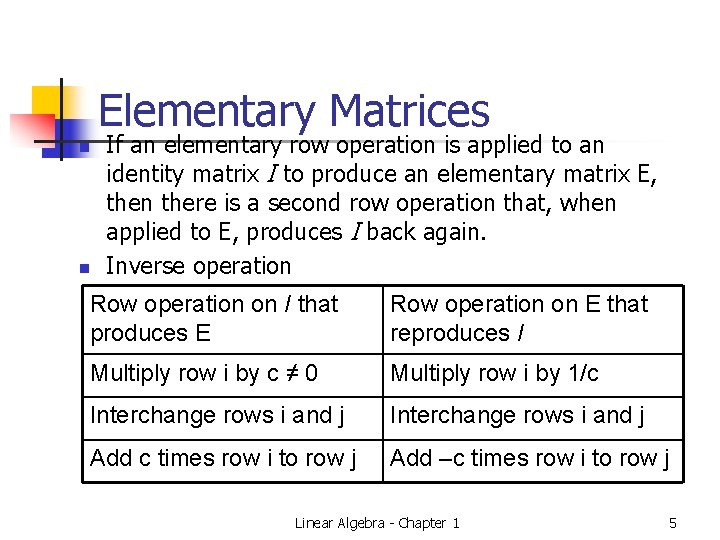
Elementary Matrices n n If an elementary row operation is applied to an identity matrix I to produce an elementary matrix E, then there is a second row operation that, when applied to E, produces I back again. Inverse operation Row operation on I that produces E Row operation on E that reproduces I Multiply row i by c ≠ 0 Multiply row i by 1/c Interchange rows i and j Add c times row i to row j Add –c times row i to row j Linear Algebra - Chapter 1 5

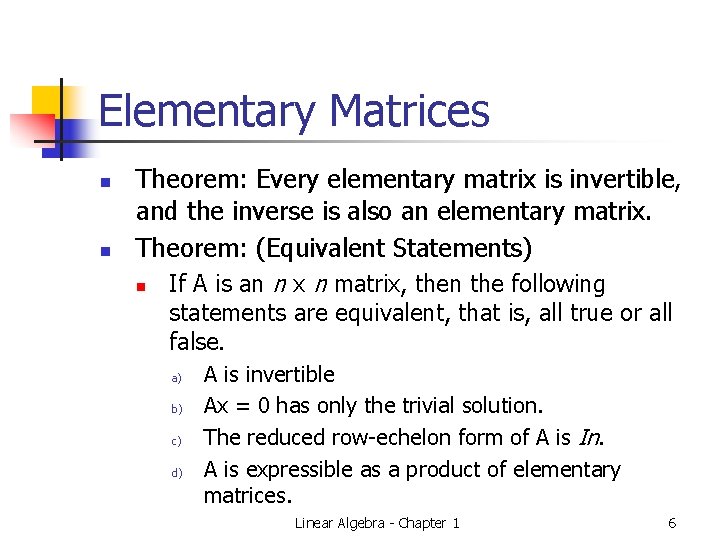
Elementary Matrices n n Theorem: Every elementary matrix is invertible, and the inverse is also an elementary matrix. Theorem: (Equivalent Statements) n If A is an n x n matrix, then the following statements are equivalent, that is, all true or all false. a) b) c) d) A is invertible Ax = 0 has only the trivial solution. The reduced row-echelon form of A is In. A is expressible as a product of elementary matrices. Linear Algebra - Chapter 1 6

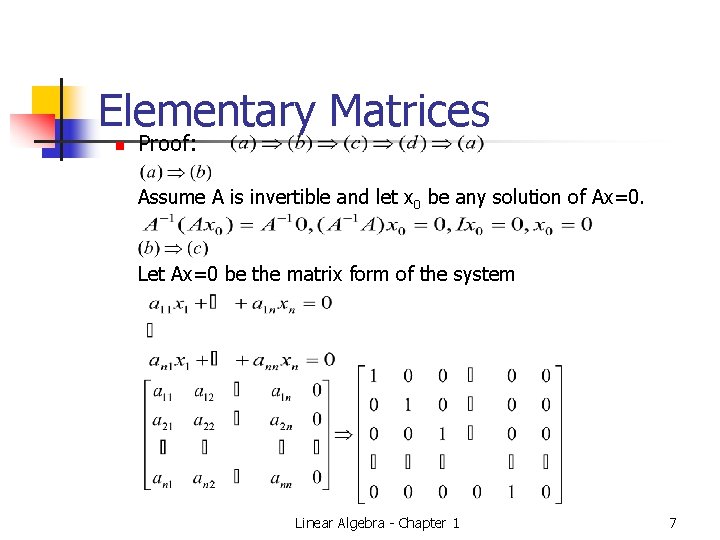
Elementary Matrices n Proof: Assume A is invertible and let x 0 be any solution of Ax=0. Let Ax=0 be the matrix form of the system Linear Algebra - Chapter 1 7

Elementary Matrices Assumed that the reduced row-echelon form of A is In by a finite sequence of elementary row operations, such that: By theorem, E 1, …, En are invertible. Multiplying both sides of equation on the left we obtain: This equation expresses A as a product of elementary matrices. If A is a product of elementary matrices, then the matrix A is a product of invertible matrices, and hence is invertible. n n Matrices that can be obtained from one another by a finite sequence of elementary row operations are said to be row equivalent. An n x n matrix A is invertible if and only if it is row equivalent to the n x n identity. Linear matrix. Algebra - Chapter 1 8

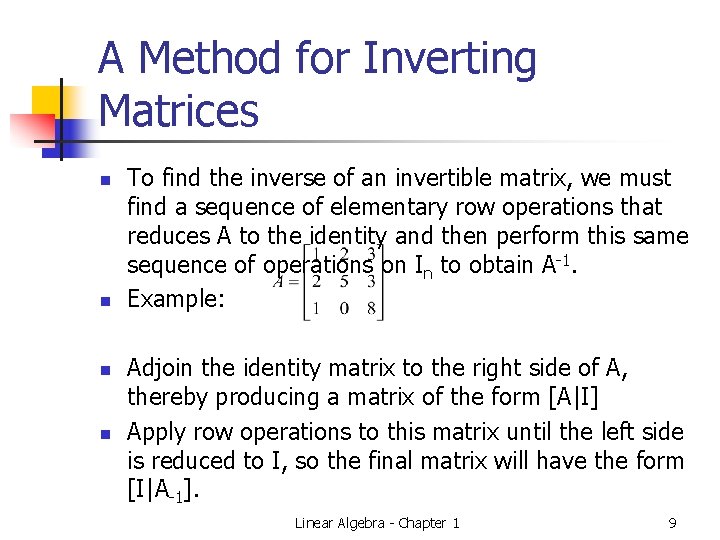
A Method for Inverting Matrices n n To find the inverse of an invertible matrix, we must find a sequence of elementary row operations that reduces A to the identity and then perform this same sequence of operations on In to obtain A-1. Example: Adjoin the identity matrix to the right side of A, thereby producing a matrix of the form [A|I] Apply row operations to this matrix until the left side is reduced to I, so the final matrix will have the form [I|A-1]. Linear Algebra - Chapter 1 9

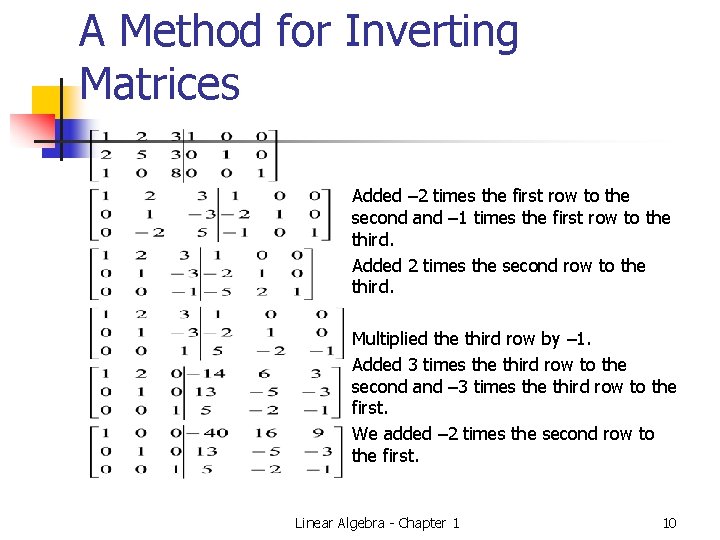
A Method for Inverting Matrices Added – 2 times the first row to the second and – 1 times the first row to the third. Added 2 times the second row to the third. Multiplied the third row by – 1. Added 3 times the third row to the second and – 3 times the third row to the first. We added – 2 times the second row to the first. Linear Algebra - Chapter 1 10

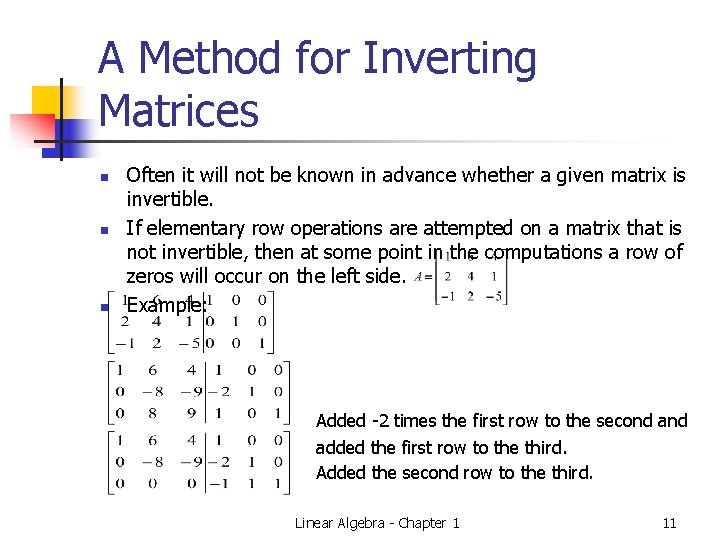
A Method for Inverting Matrices n n n Often it will not be known in advance whether a given matrix is invertible. If elementary row operations are attempted on a matrix that is not invertible, then at some point in the computations a row of zeros will occur on the left side. Example: Added -2 times the first row to the second added the first row to the third. Added the second row to the third. Linear Algebra - Chapter 1 11

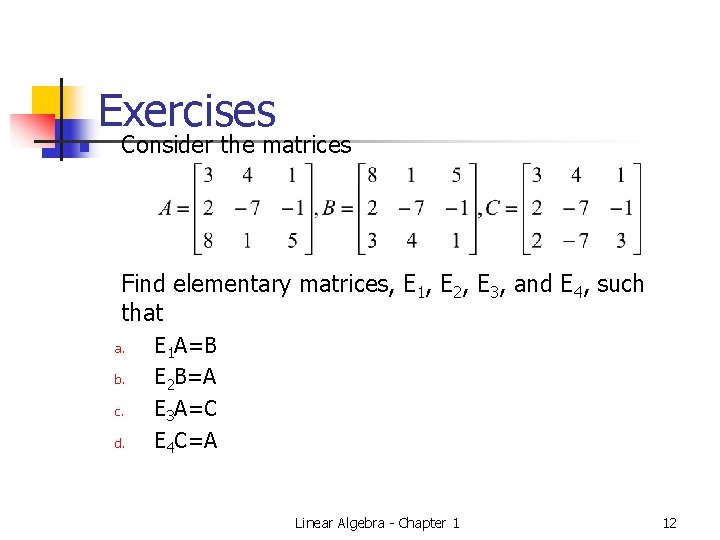
Exercises n Consider the matrices Find elementary matrices, E 1, E 2, E 3, and E 4, such that a. b. c. d. E 1 A=B E 2 B=A E 3 A=C E 4 C=A Linear Algebra - Chapter 1 12

Exercises n Express the matrix: in the form A = E F G R, where E, F, G are elementary matrices, and R is in row-echelon form. Linear Algebra - Chapter 1 13

1. 6 Further Results on Systems of Equations and Invertibility

Linear Systems n n Theorem: Solving Linear Systems by Matrix Inversion: If A is an invertible n x n matrix, then for each n x 1 matrix b, the system of equations Ax = b has exactly one solution, namely, x = A-1 b. Linear systems with a common coefficient matrix. Ax=b 1, Ax=b 2, Ax=b 3, . . . , Ax=bk If A is invertible, then the solutions x 1=A-1 b 1, x 2=A-1 b 2, x 3=A-1 b 3, . . . , xk=A-1 bk This can be efficiently done using Gauss-Jordan Elimination on [A|b 1|b 2|. . . |bk] Linear Algebra - Chapter 1 15

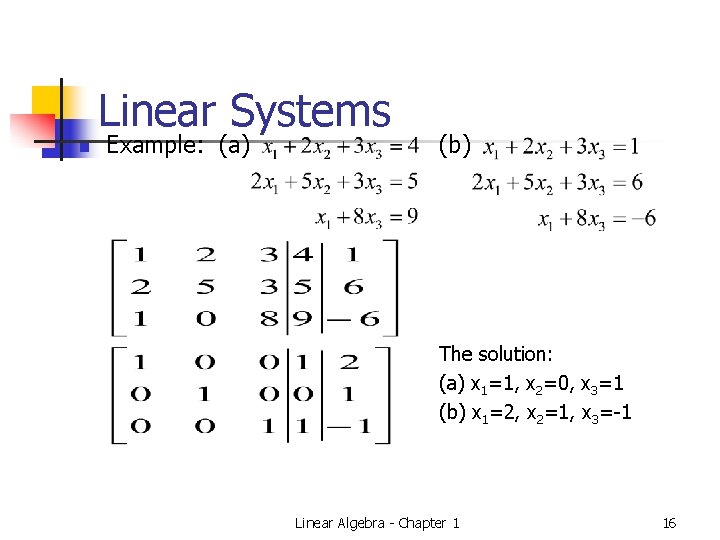
Linear Systems n Example: (a) (b) The solution: (a) x 1=1, x 2=0, x 3=1 (b) x 1=2, x 2=1, x 3=-1 Linear Algebra - Chapter 1 16

Properties of Invertible Matrices n Theorem: Let A be a square matrix. a) b) n If B is a square matrix satisfying BA=I, then B=A-1. If B is a square matrix satisfying AB=I, then B=A-1. Theorem: Equivalent Statements a) b) c) d) e) f) A is invertible Ax=0 has only the trivial solutions The reduced row-echelon form of A is In A is expresssible as a product of elementary matrices Ax=b is consistent for every n x 1 matrix b Ax=b has exactly one solution for every n x 1 matrix b Linear Algebra - Chapter 1 17

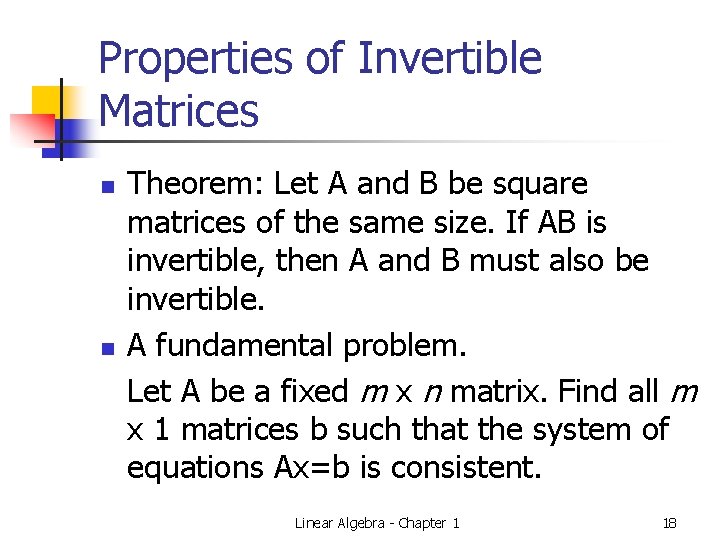
Properties of Invertible Matrices n n Theorem: Let A and B be square matrices of the same size. If AB is invertible, then A and B must also be invertible. A fundamental problem. Let A be a fixed m x n matrix. Find all m x 1 matrices b such that the system of equations Ax=b is consistent. Linear Algebra - Chapter 1 18

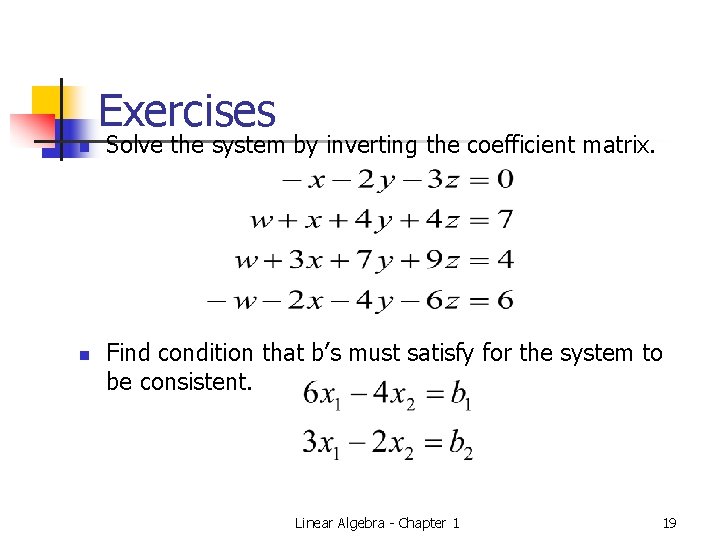
Exercises n n Solve the system by inverting the coefficient matrix. Find condition that b’s must satisfy for the system to be consistent. Linear Algebra - Chapter 1 19

1. 7 Diagonal, Triangular, and Symmetric Matrices

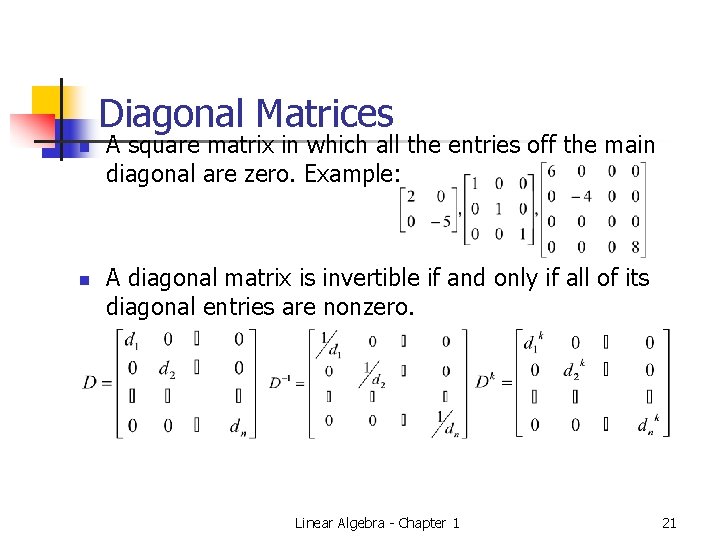
Diagonal Matrices n n A square matrix in which all the entries off the main diagonal are zero. Example: A diagonal matrix is invertible if and only if all of its diagonal entries are nonzero. Linear Algebra - Chapter 1 21

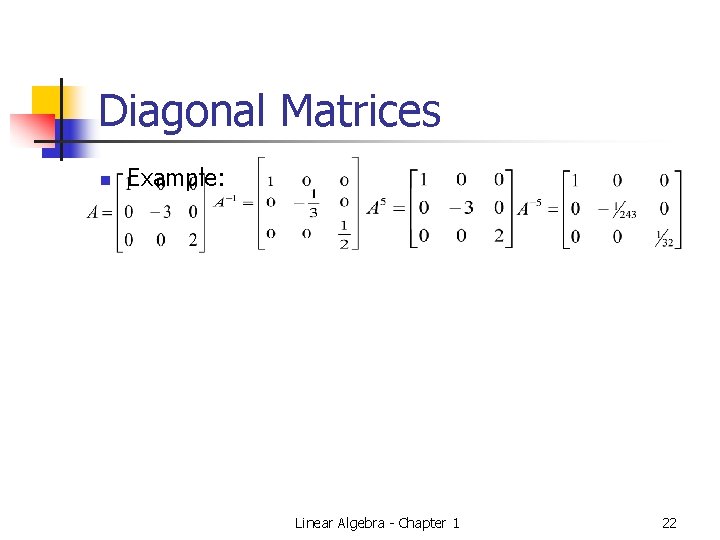
Diagonal Matrices n Example: Linear Algebra - Chapter 1 22

Triangular Matrices n n n Lower triangular = a square matrix in which all the entries above the main diagonal are zero. Upper triangular = a square matrix in which all the entries under the main diagonal are zero. Triangular = a matrix that is either upper triangular or lower triangular. Linear Algebra - Chapter 1 23

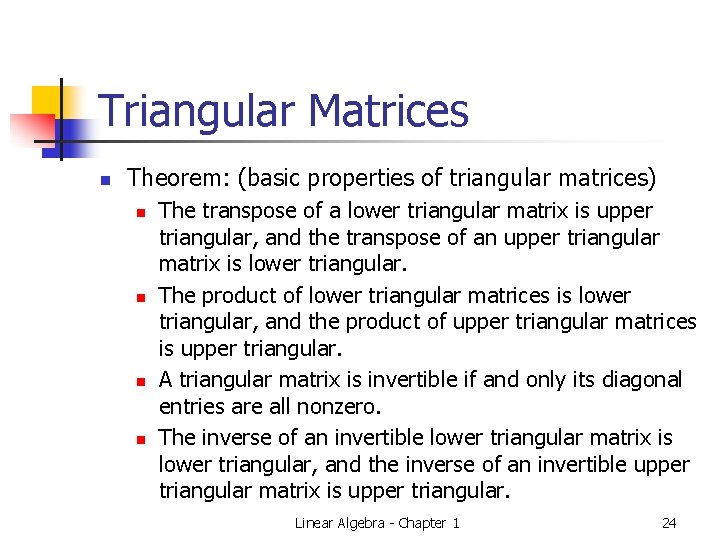
Triangular Matrices n Theorem: (basic properties of triangular matrices) n n The transpose of a lower triangular matrix is upper triangular, and the transpose of an upper triangular matrix is lower triangular. The product of lower triangular matrices is lower triangular, and the product of upper triangular matrices is upper triangular. A triangular matrix is invertible if and only its diagonal entries are all nonzero. The inverse of an invertible lower triangular matrix is lower triangular, and the inverse of an invertible upper triangular matrix is upper triangular. Linear Algebra - Chapter 1 24

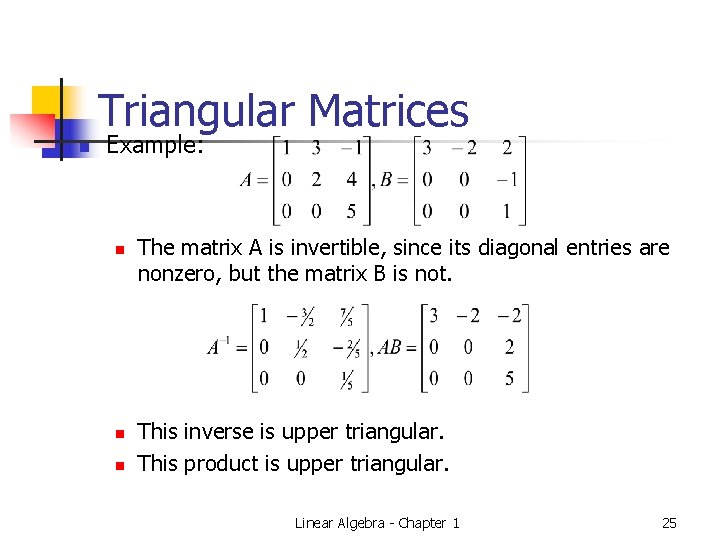
Triangular Matrices n Example: n n n The matrix A is invertible, since its diagonal entries are nonzero, but the matrix B is not. This inverse is upper triangular. This product is upper triangular. Linear Algebra - Chapter 1 25

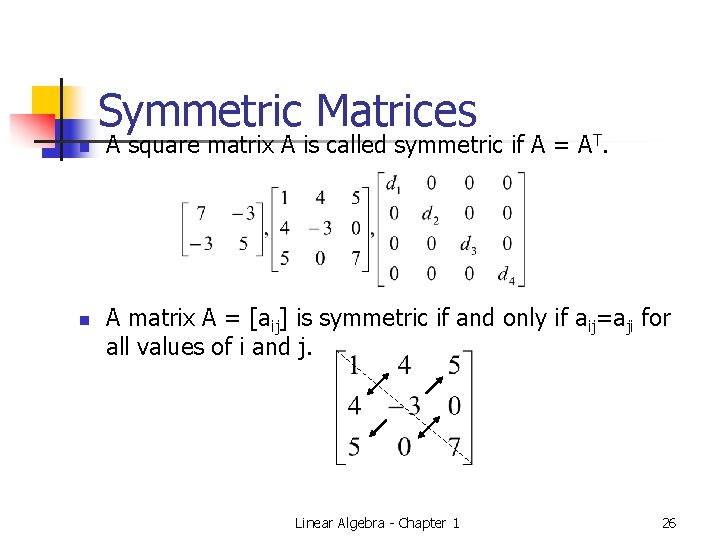
Symmetric Matrices n n A square matrix A is called symmetric if A = AT. A matrix A = [aij] is symmetric if and only if aij=aji for all values of i and j. Linear Algebra - Chapter 1 26

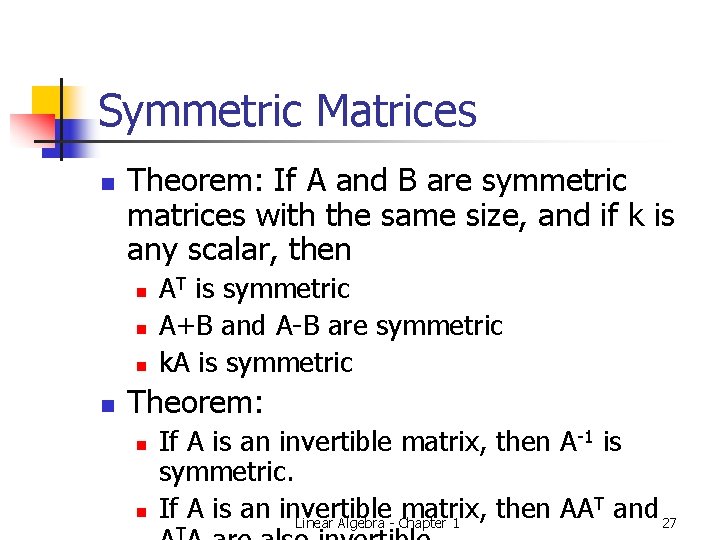
Symmetric Matrices n Theorem: If A and B are symmetric matrices with the same size, and if k is any scalar, then n n AT is symmetric A+B and A-B are symmetric k. A is symmetric Theorem: n n If A is an invertible matrix, then A-1 is symmetric. T and If A is an invertible matrix, then AA Linear Algebra - Chapter 1 27

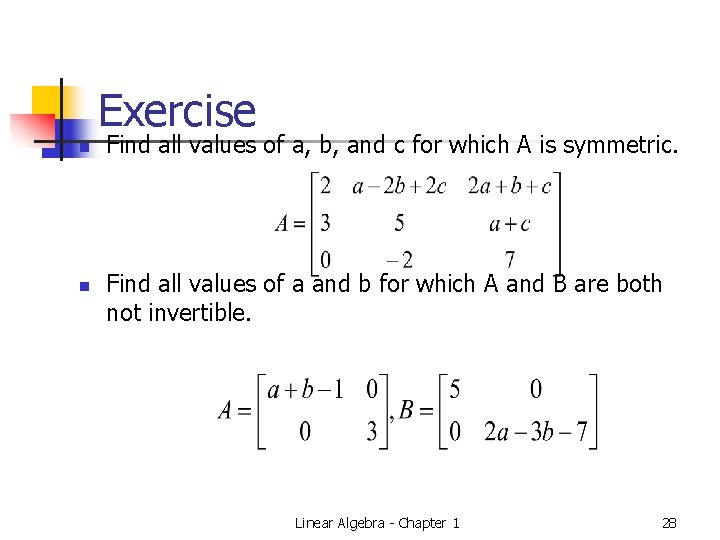
Exercise n n Find all values of a, b, and c for which A is symmetric. Find all values of a and b for which A and B are both not invertible. Linear Algebra - Chapter 1 28
 Elementary matrix examples
Elementary matrix examples Identity matrix inverse
Identity matrix inverse A inverse matrix
A inverse matrix Matrix inverse formula 3x3
Matrix inverse formula 3x3 A inverse matrix
A inverse matrix 01:640:244 lecture notes - lecture 15: plat, idah, farad
01:640:244 lecture notes - lecture 15: plat, idah, farad Direct stiffness method truss
Direct stiffness method truss Division de matrices 3x3
Division de matrices 3x3 3x2 matrix
3x2 matrix Matrix inverse gaussian elimination
Matrix inverse gaussian elimination Elementary matrix
Elementary matrix Matrix
Matrix Identity matrix
Identity matrix Site:slidetodoc.com
Site:slidetodoc.com Matrix of cofactors
Matrix of cofactors Inverse of a matrix using adjoint
Inverse of a matrix using adjoint Inverse matrix code
Inverse matrix code Use inverse matrix to solve system of equations
Use inverse matrix to solve system of equations A homogeneous transformation matrix is in the order of
A homogeneous transformation matrix is in the order of Linear algebra chapter 2
Linear algebra chapter 2 Inverse of a matrix definition
Inverse of a matrix definition The inverse of matrix a is written as a-1 so that aa-1=?
The inverse of matrix a is written as a-1 so that aa-1=? Simulink inverse matrix
Simulink inverse matrix Matrix inverse worksheet
Matrix inverse worksheet Elementary scaling matrix
Elementary scaling matrix Find an inverse of 101 modulo 4620
Find an inverse of 101 modulo 4620 Trigonometric function
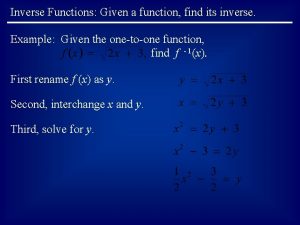
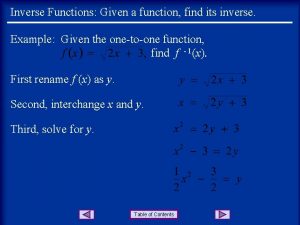
Trigonometric function How to find the inverse of a function
How to find the inverse of a function Inverse of log
Inverse of log