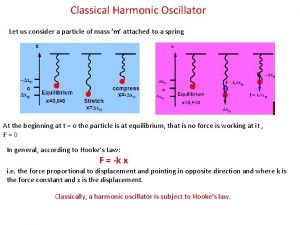
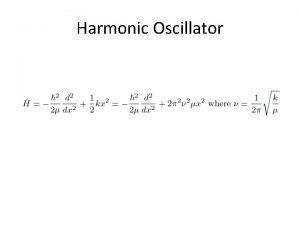
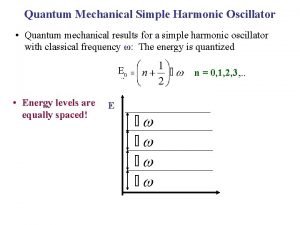
Energy of the Simple Harmonic Oscillator The Total

Energy of the Simple Harmonic Oscillator

The Total Mechanical Energy (PE + KE) Is Constant KINETIC ENERGY: • KE = ½ mv 2 • Remember v = -ωAsin(ωt+ϕ) • KE = ½ mω2 A 2 sin 2(ωt+ϕ)

The Total Mechanical Energy (PE + KE) Is Constant POTENTIAL ENERGY: • PE = ½ kx 2 • Remember x = Acos(ωt+ϕ) • PE = ½ k. A 2 cos 2(ωt+ϕ)

The Total Mechanical Energy (PE + KE) Is Constant Etot = KE + PE • Etot = ½k. A 2(sin 2(ωt+ϕ) + cos 2(ωt+ϕ)) • Remember: • ω2 = k/m • sin 2θ + cos 2θ = 1 • Therefore Etot = ½k. A 2

• Note that PE is small when KE is large and vice versa • The sum of PE and KE is constant and the sum = ½ k. A 2 • Both PE and KE are always positive • PE and KE vs time is shown on the left • The variations of PE and KE with the displacement x are shown on the right

Velocity as a function of position for a Simple Harmonic Oscillator

The Simple Pendulum

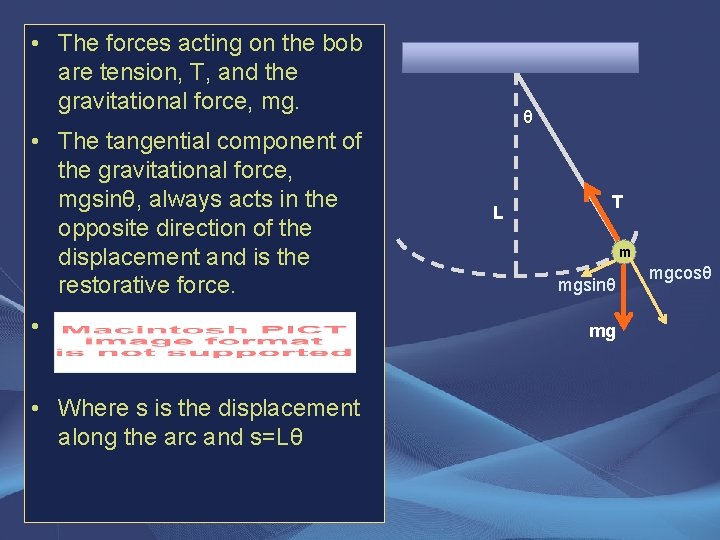
• The forces acting on the bob are tension, T, and the gravitational force, mg. • The tangential component of the gravitational force, mgsinθ, always acts in the opposite direction of the displacement and is the restorative force. • • Where s is the displacement along the arc and s=Lθ θ L T m mgsinθ mg mgcosθ

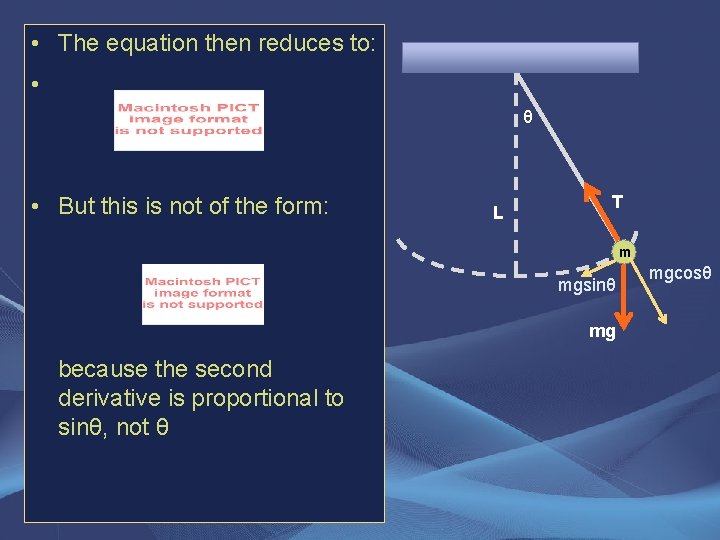
• The equation then reduces to: • θ • But this is not of the form: L T m mgsinθ mg because the second derivative is proportional to sinθ, not θ mgcosθ

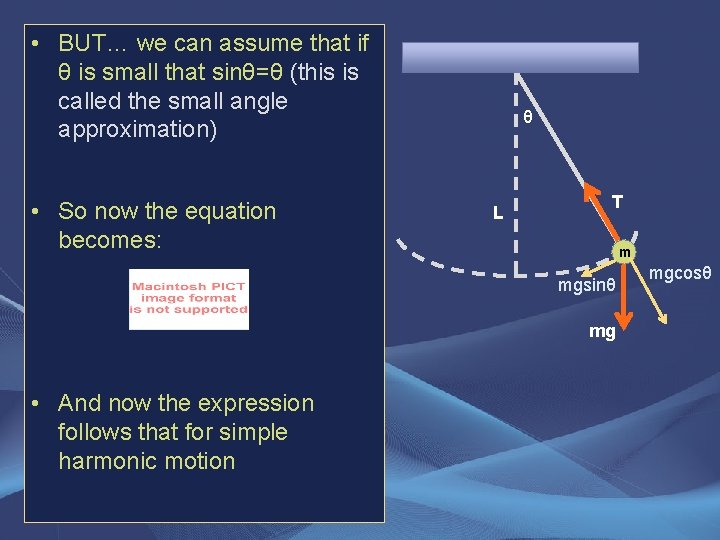
• BUT… we can assume that if θ is small that sinθ=θ (this is called the small angle approximation) • So now the equation becomes: θ L T m mgsinθ mg • And now the expression follows that for simple harmonic motion mgcosθ

SHM: The Pendulum • From this equation θ can be written as: • θ = θmaxcos(ωt+Φ) • Θmax is the maximum angular displacement • ω, the angular frequency, is: • because this follows the function • The Period, T of the motion would be:

Damped Oscillations • In many cases dissipative forces (like friction) act on an object. • The Mechanical Energy diminishes with time and the motion is damped • The retarding force can be expressed as: R = -bv (b is a constant, the damping coefficient) • The restoring force can be expressed as F = -kx

Damped Oscillations • When we do the sum of the forces: • The solution to this equation follows the form:

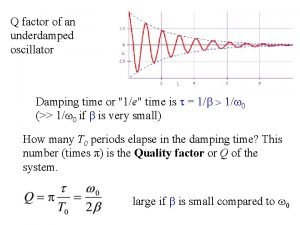
Damped Oscillations • When the retarding force < the restoring force, the oscillatory character is preserved but the amplitude decreases • The amplitude decays exponentially with time

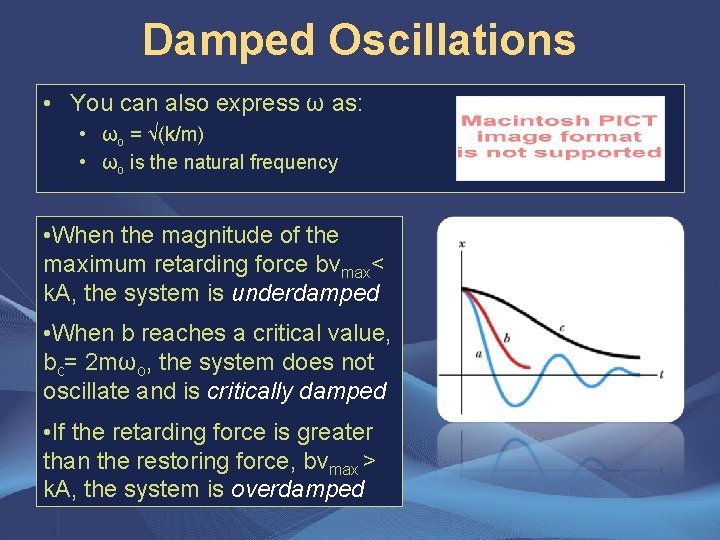
Damped Oscillations • You can also express ω as: • ωo = √(k/m) • ωo is the natural frequency • When the magnitude of the maximum retarding force bvmax< k. A, the system is underdamped • When b reaches a critical value, bc= 2 mωo, the system does not oscillate and is critically damped • If the retarding force is greater than the restoring force, bvmax > k. A, the system is overdamped

Forced Oscillations • The amplitude will remain constant if the energy input per cycle equals the energy lost due to damping • This type of motion is called a force oscillation • Then the sum of the forces becomes:

Forced Oscillations • The solution to this equation follows the form:

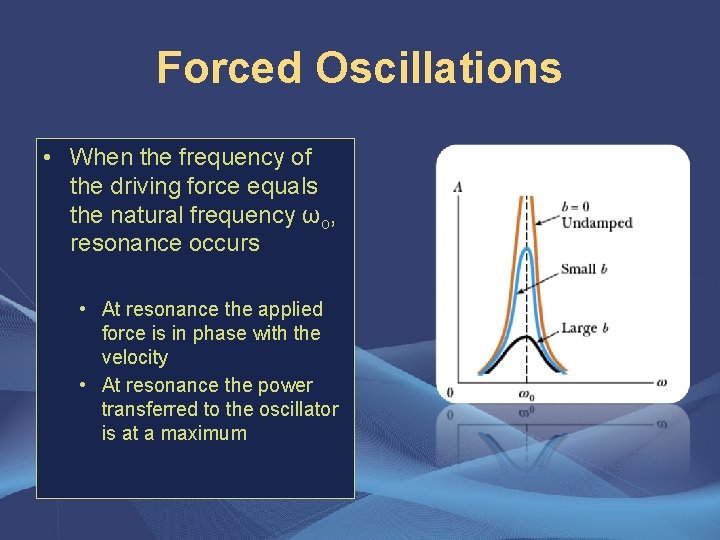
Forced Oscillations • When the frequency of the driving force equals the natural frequency ωo, resonance occurs • At resonance the applied force is in phase with the velocity • At resonance the power transferred to the oscillator is at a maximum