Constructing a triangle given SAS How could we

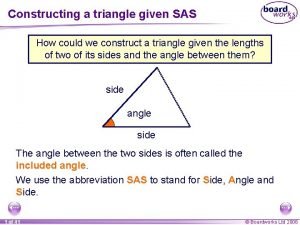
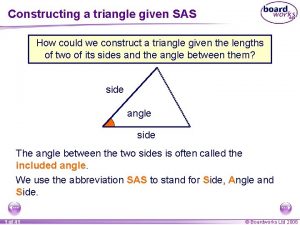
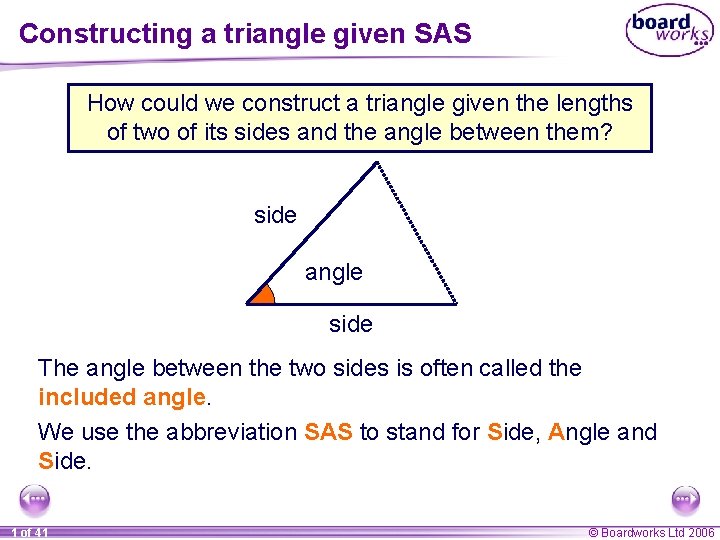
Constructing a triangle given SAS How could we construct a triangle given the lengths of two of its sides and the angle between them? side angle side The angle between the two sides is often called the included angle. We use the abbreviation SAS to stand for Side, Angle and Side. 1 of 41 © Boardworks Ltd 2006

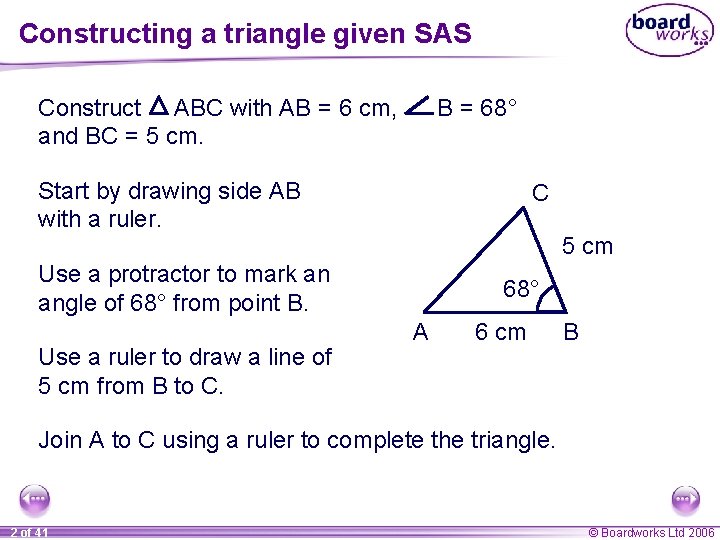
Constructing a triangle given SAS Construct ABC with AB = 6 cm, and BC = 5 cm. B = 68° Start by drawing side AB with a ruler. C 5 cm Use a protractor to mark an angle of 68° from point B. Use a ruler to draw a line of 5 cm from B to C. 68° A 6 cm B Join A to C using a ruler to complete the triangle. 2 of 41 © Boardworks Ltd 2006

Constructing a triangle given ASA How could we construct a triangle given two angles and the length of the side between them? angle side The side between the two angles is often called the included side. We use the abbreviation ASA to stand for Angle, Side and Angle. 3 of 41 © Boardworks Ltd 2006

Constructing a triangle given ASA For example, construct and B = 115°. ABC with AB = 10 cm, A = 35° C Start by drawing side AB with a ruler. Use a protractor to mark an angle of 35° from point A. Use a ruler to draw a long line from A. Use a protractor to mark an angle of 115° from point B. 35° A 10 cm 115° B Use a ruler to draw a line from B to meet the other line at point C. 4 of 41 © Boardworks Ltd 2006

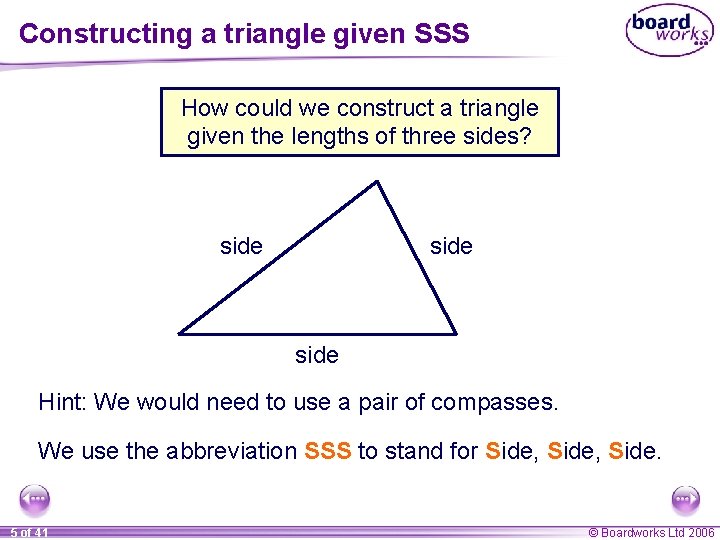
Constructing a triangle given SSS How could we construct a triangle given the lengths of three sides? side Hint: We would need to use a pair of compasses. We use the abbreviation SSS to stand for Side, Side. 5 of 41 © Boardworks Ltd 2006

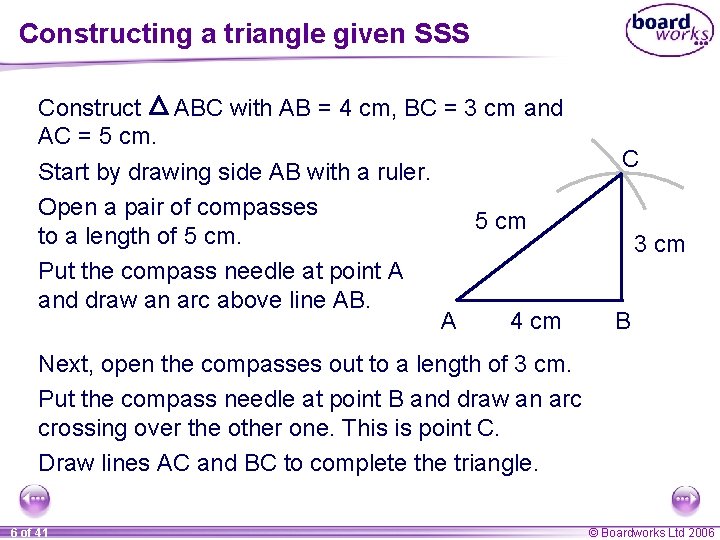
Constructing a triangle given SSS Construct ABC with AB = 4 cm, BC = 3 cm and AC = 5 cm. Start by drawing side AB with a ruler. Open a pair of compasses 5 cm to a length of 5 cm. Put the compass needle at point A and draw an arc above line AB. A 4 cm C 3 cm B Next, open the compasses out to a length of 3 cm. Put the compass needle at point B and draw an arc crossing over the other one. This is point C. Draw lines AC and BC to complete the triangle. 6 of 41 © Boardworks Ltd 2006

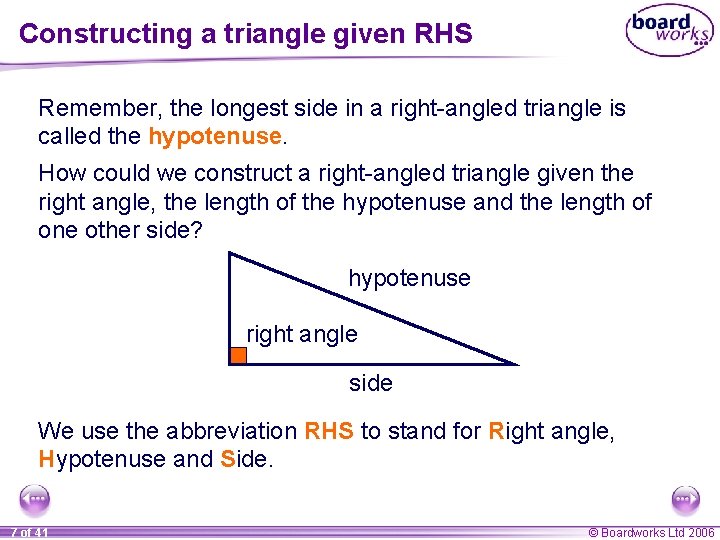
Constructing a triangle given RHS Remember, the longest side in a right-angled triangle is called the hypotenuse. How could we construct a right-angled triangle given the right angle, the length of the hypotenuse and the length of one other side? hypotenuse right angle side We use the abbreviation RHS to stand for Right angle, Hypotenuse and Side. 7 of 41 © Boardworks Ltd 2006

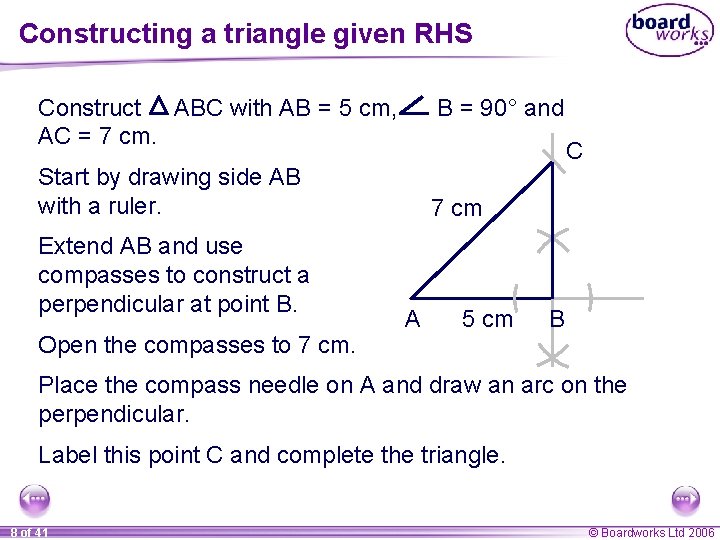
Constructing a triangle given RHS Construct ABC with AB = 5 cm, AC = 7 cm. B = 90° and C Start by drawing side AB with a ruler. Extend AB and use compasses to construct a perpendicular at point B. Open the compasses to 7 cm A 5 cm B Place the compass needle on A and draw an arc on the perpendicular. Label this point C and complete the triangle. 8 of 41 © Boardworks Ltd 2006

Constructing triangles 9 of 41 © Boardworks Ltd 2006

Contents S 6 Construction and loci A S 6. 1 Drawing lines and angles A S 6. 2 Constructing triangles A S 6. 3 Constructing lines and angles A S 6. 4 Constructing nets A N 6. 5 Loci 10 of 41 © Boardworks Ltd 2006

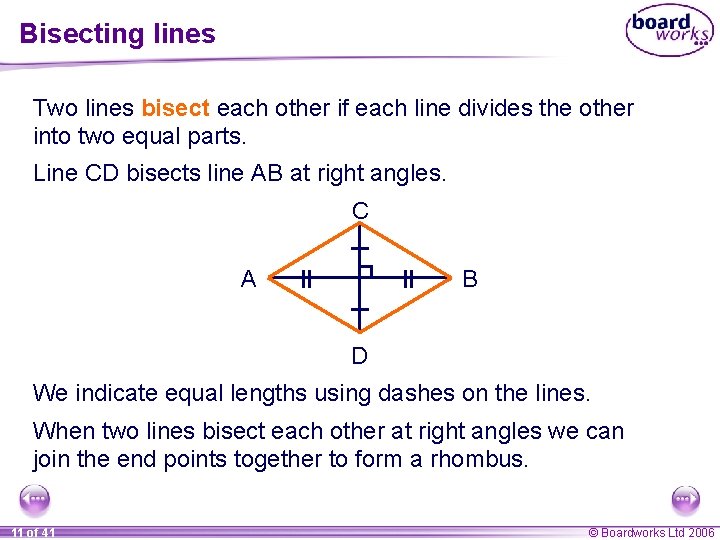
Bisecting lines Two lines bisect each other if each line divides the other into two equal parts. Line CD bisects line AB at right angles. C A B D We indicate equal lengths using dashes on the lines. When two lines bisect each other at right angles we can join the end points together to form a rhombus. 11 of 41 © Boardworks Ltd 2006

Bisecting angles A line bisects an angle if it divides it into two equal angles. In the diagram below line BD bisects ABC. A D B 12 of 41 C © Boardworks Ltd 2006

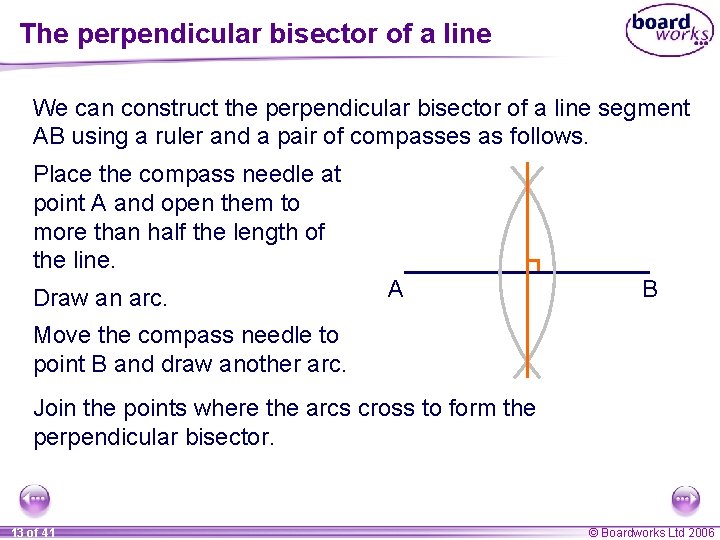
The perpendicular bisector of a line We can construct the perpendicular bisector of a line segment AB using a ruler and a pair of compasses as follows. Place the compass needle at point A and open them to more than half the length of the line. Draw an arc. A B Move the compass needle to point B and draw another arc. Join the points where the arcs cross to form the perpendicular bisector. 13 of 41 © Boardworks Ltd 2006

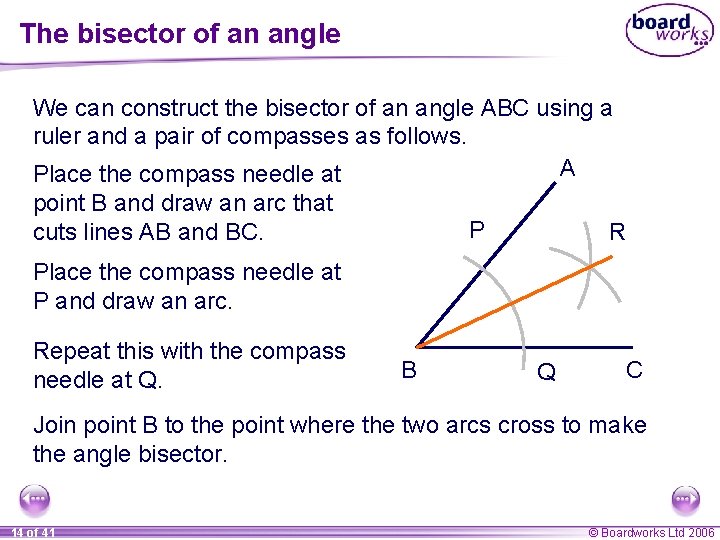
The bisector of an angle We can construct the bisector of an angle ABC using a ruler and a pair of compasses as follows. A Place the compass needle at point B and draw an arc that P cuts lines AB and BC. R Place the compass needle at P and draw an arc. Repeat this with the compass needle at Q. B Q C Join point B to the point where the two arcs cross to make the angle bisector. 14 of 41 © Boardworks Ltd 2006

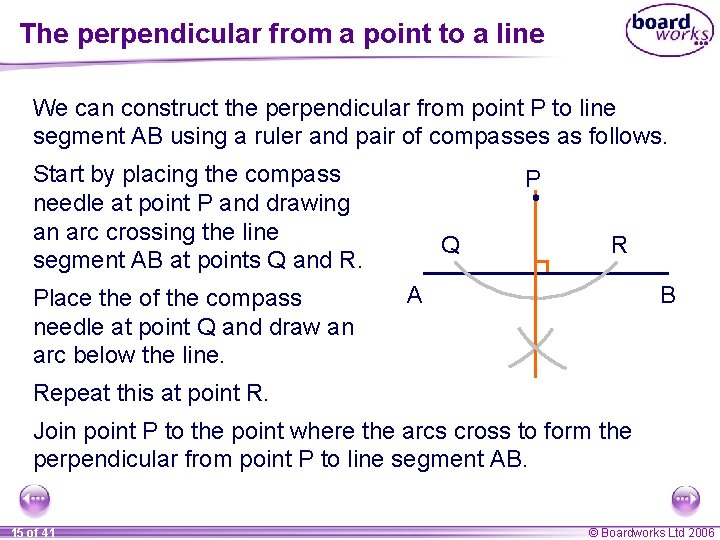
The perpendicular from a point to a line We can construct the perpendicular from point P to line segment AB using a ruler and pair of compasses as follows. Start by placing the compass needle at point P and drawing an arc crossing the line segment AB at points Q and R. Place the of the compass needle at point Q and draw an arc below the line. P Q R A B Repeat this at point R. Join point P to the point where the arcs cross to form the perpendicular from point P to line segment AB. 15 of 41 © Boardworks Ltd 2006

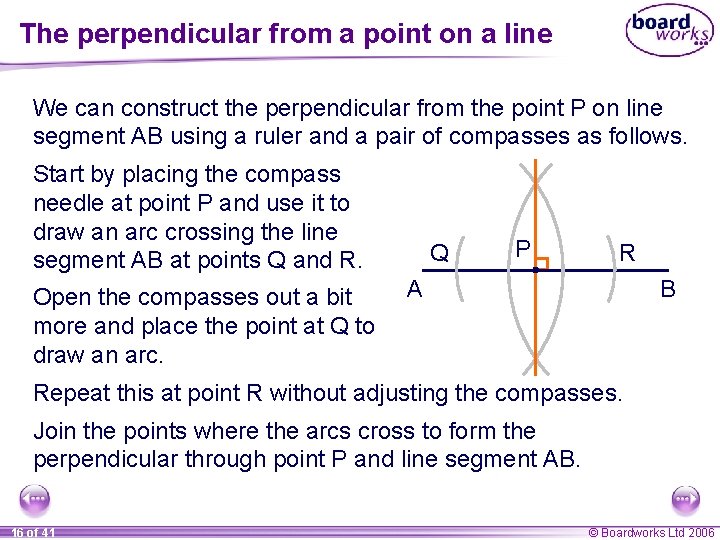
The perpendicular from a point on a line We can construct the perpendicular from the point P on line segment AB using a ruler and a pair of compasses as follows. Start by placing the compass needle at point P and use it to draw an arc crossing the line segment AB at points Q and R. Open the compasses out a bit more and place the point at Q to draw an arc. Q P R A B Repeat this at point R without adjusting the compasses. Join the points where the arcs cross to form the perpendicular through point P and line segment AB. 16 of 41 © Boardworks Ltd 2006

Contents S 6 Construction and loci A S 6. 1 Drawing lines and angles A S 6. 2 Constructing triangles A S 6. 3 Constructing lines and angles A S 6. 4 Constructing nets A N 6. 5 Loci 17 of 41 © Boardworks Ltd 2006

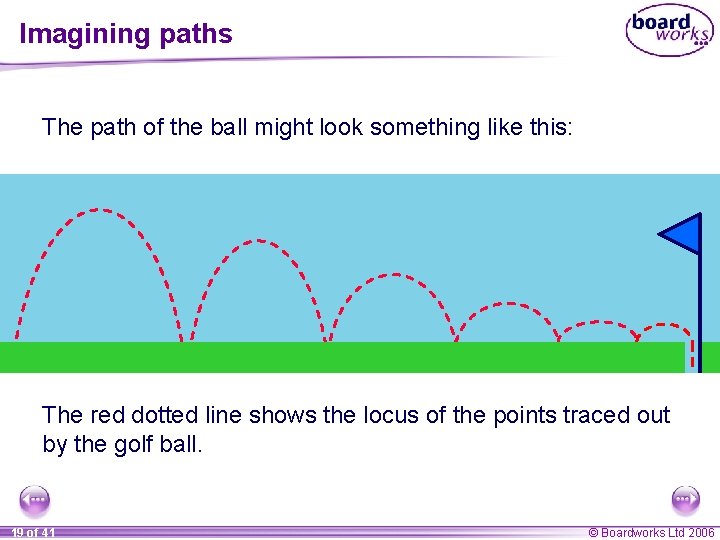
Imagining paths A locus is a set of points that satisfy a rule or set of rules. The plural of locus is loci. We can think of a locus as a path traced out by a moving point. For example: Imagine the path of a golf ball as it is driven on to the green. The ball bounces several times and drops into the hole. 18 of 41 © Boardworks Ltd 2006

Imagining paths The path of the ball might look something like this: The red dotted line shows the locus of the points traced out by the golf ball. 19 of 41 © Boardworks Ltd 2006

Imagining paths A helicopter takes off and rises to a height of 30 m. It accelerates forward and rises to 1000 m where it levels out then continues on its way. Can you imagine the path traced out by the helicopter? How could you represent the path in two dimensions? What about in three dimensions? 20 of 41 © Boardworks Ltd 2006

Imagining paths A nervous woman paces up and down in one of the capsules on the Millennium Eye as she ‘enjoys’ the view. Can you imagine the path traced out by the woman? How could you represent the path in two dimensions? What about in three dimensions? 21 of 41 © Boardworks Ltd 2006

Imagining paths A man has been out for the evening, has locked himself out of his flat and has to climb the spiral fire escape. Can you imagine the path traced out by the man? How could you represent the path in two dimensions? What about in three dimensions? 22 of 41 © Boardworks Ltd 2006

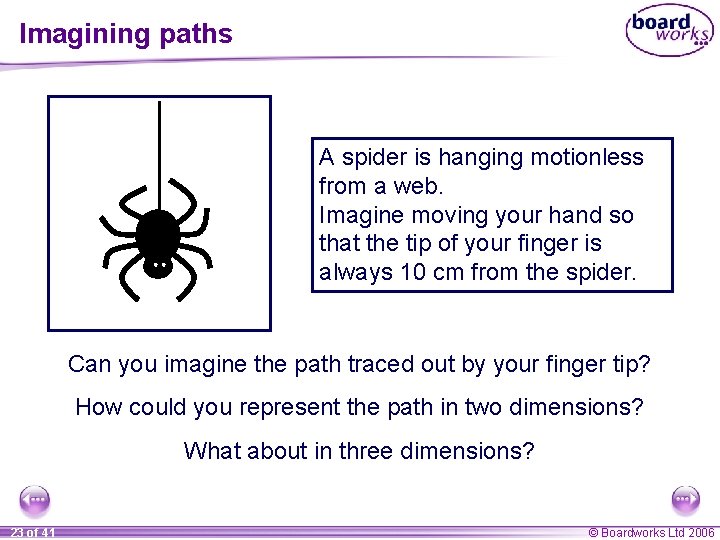
Imagining paths A spider is hanging motionless from a web. Imagine moving your hand so that the tip of your finger is always 10 cm from the spider. Can you imagine the path traced out by your finger tip? How could you represent the path in two dimensions? What about in three dimensions? 23 of 41 © Boardworks Ltd 2006

Walking turtle 24 of 41 © Boardworks Ltd 2006

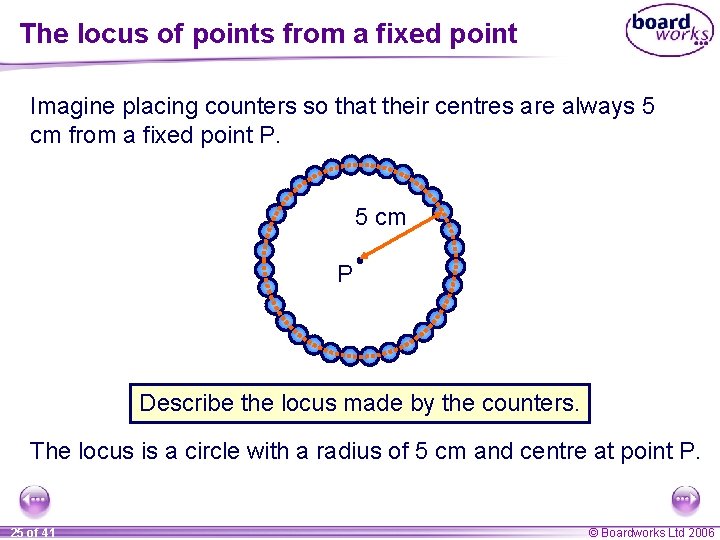
The locus of points from a fixed point Imagine placing counters so that their centres are always 5 cm from a fixed point P. 5 cm P Describe the locus made by the counters. The locus is a circle with a radius of 5 cm and centre at point P. 25 of 41 © Boardworks Ltd 2006

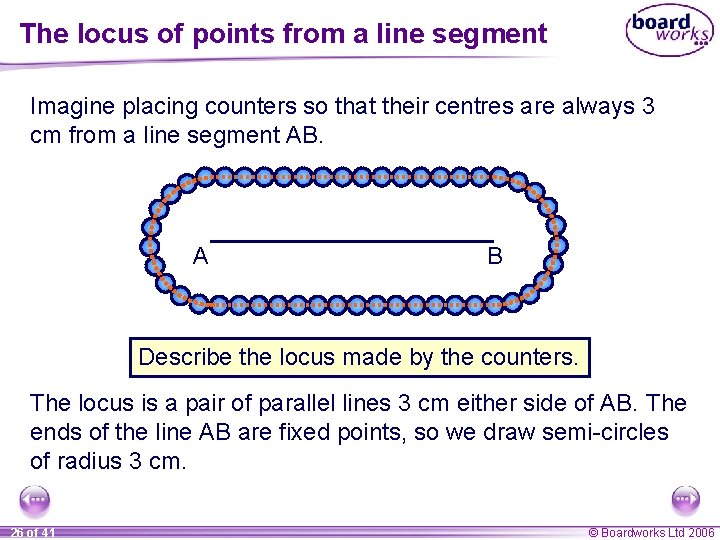
The locus of points from a line segment Imagine placing counters so that their centres are always 3 cm from a line segment AB. A B Describe the locus made by the counters. The locus is a pair of parallel lines 3 cm either side of AB. The ends of the line AB are fixed points, so we draw semi-circles of radius 3 cm. 26 of 41 © Boardworks Ltd 2006

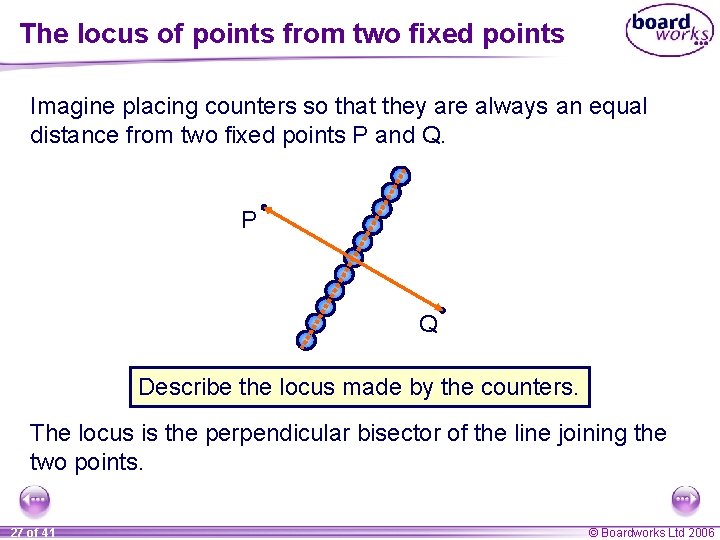
The locus of points from two fixed points Imagine placing counters so that they are always an equal distance from two fixed points P and Q. P Q Describe the locus made by the counters. The locus is the perpendicular bisector of the line joining the two points. 27 of 41 © Boardworks Ltd 2006

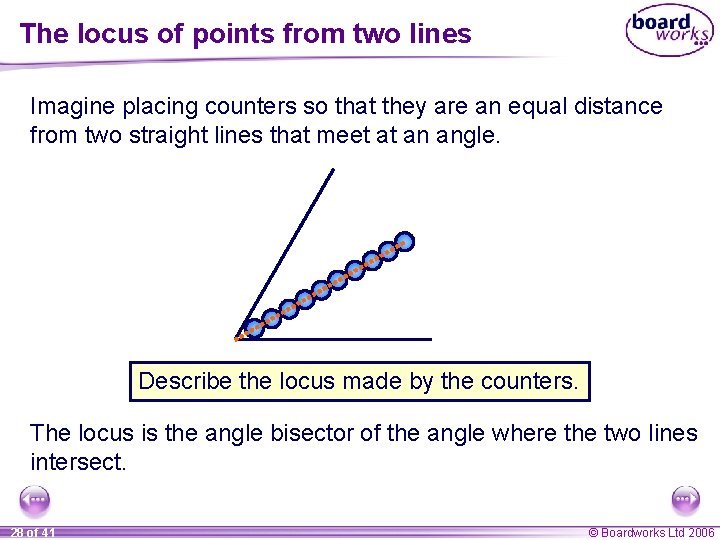
The locus of points from two lines Imagine placing counters so that they are an equal distance from two straight lines that meet at an angle. Describe the locus made by the counters. The locus is the angle bisector of the angle where the two lines intersect. 28 of 41 © Boardworks Ltd 2006

Rolling shapes along lines 29 of 41 © Boardworks Ltd 2006