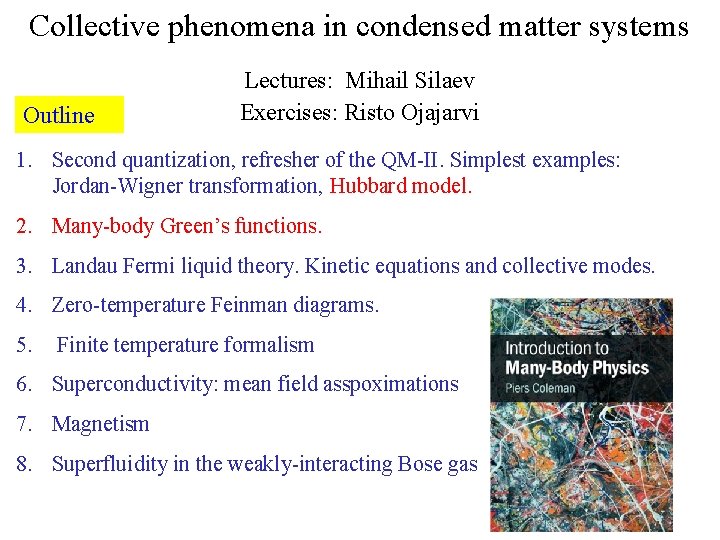
Collective phenomena in condensed matter systems Outline Lectures

Collective phenomena in condensed matter systems Outline Lectures: Mihail Silaev Exercises: Risto Ojajarvi 1. Second quantization, refresher of the QM-II. Simplest examples: Jordan-Wigner transformation, Hubbard model. 2. Many-body Green’s functions. 3. Landau Fermi liquid theory. Kinetic equations and collective modes. 4. Zero-temperature Feinman diagrams. 5. Finite temperature formalism 6. Superconductivity: mean field asspoximations 7. Magnetism 8. Superfluidity in the weakly-interacting Bose gas

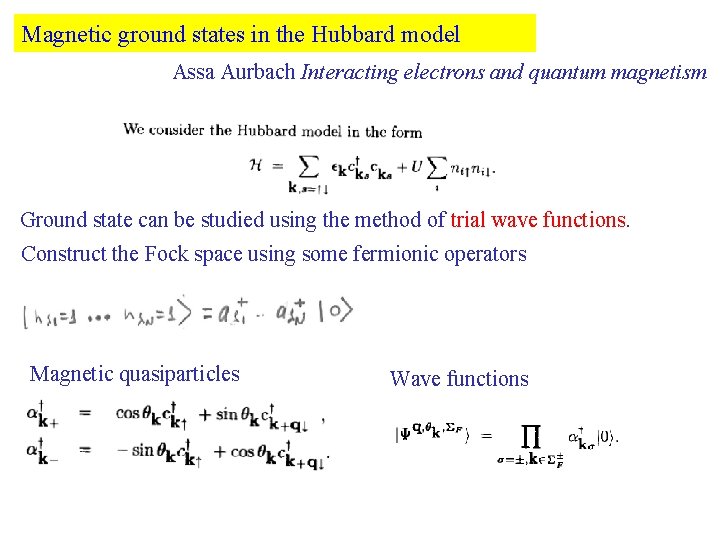
Magnetic ground states in the Hubbard model Assa Aurbach Interacting electrons and quantum magnetism Ground state can be studied using the method of trial wave functions. Construct the Fock space using some fermionic operators Magnetic quasiparticles Wave functions

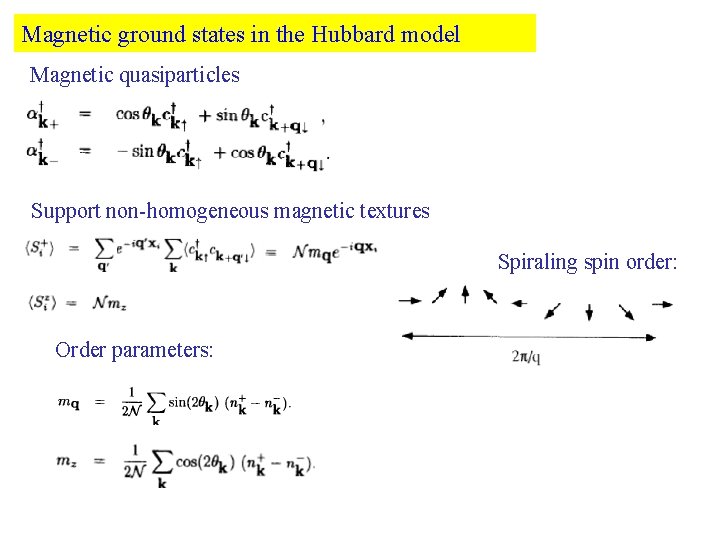
Magnetic ground states in the Hubbard model Magnetic quasiparticles Support non-homogeneous magnetic textures Spiraling spin order: Order parameters:

Calculate the average of the interaction term using the Wick’s theorem Now all variables are independent, we can minimize by k first

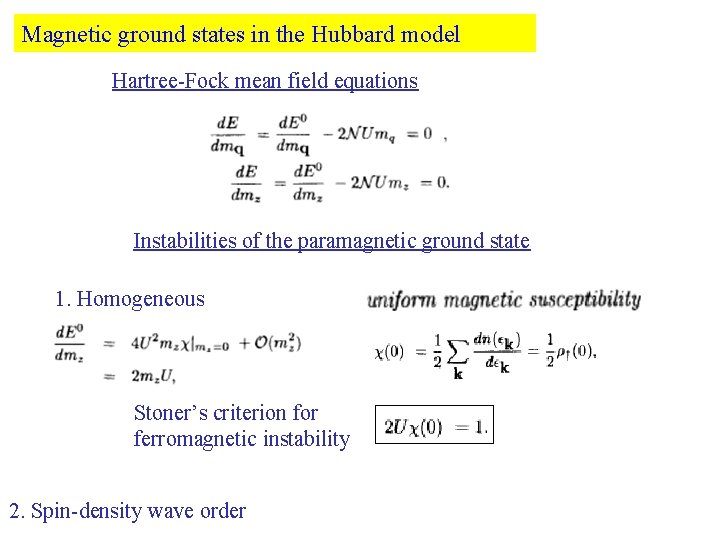
Magnetic ground states in the Hubbard model Hartree-Fock mean field equations Instabilities of the paramagnetic ground state 1. Homogeneous Stoner’s criterion for ferromagnetic instability 2. Spin-density wave order

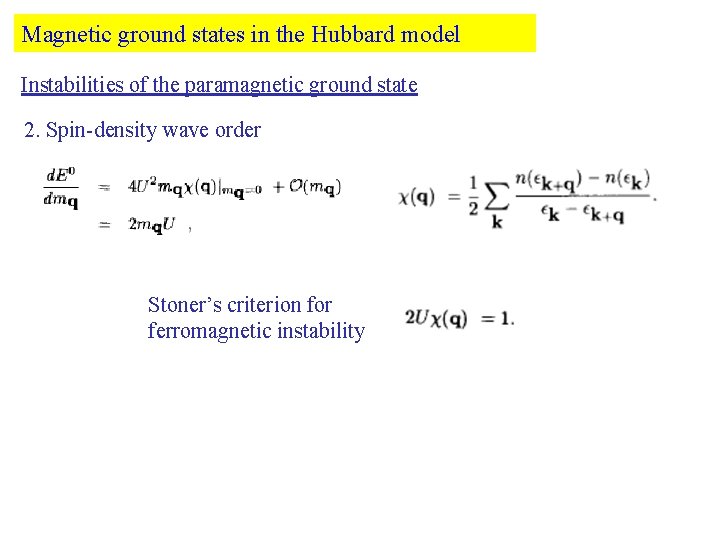
Magnetic ground states in the Hubbard model Instabilities of the paramagnetic ground state 2. Spin-density wave order Stoner’s criterion for ferromagnetic instability

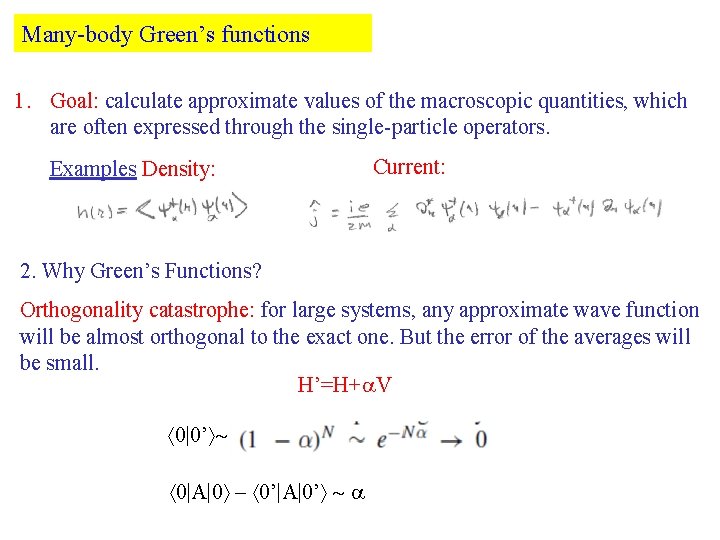
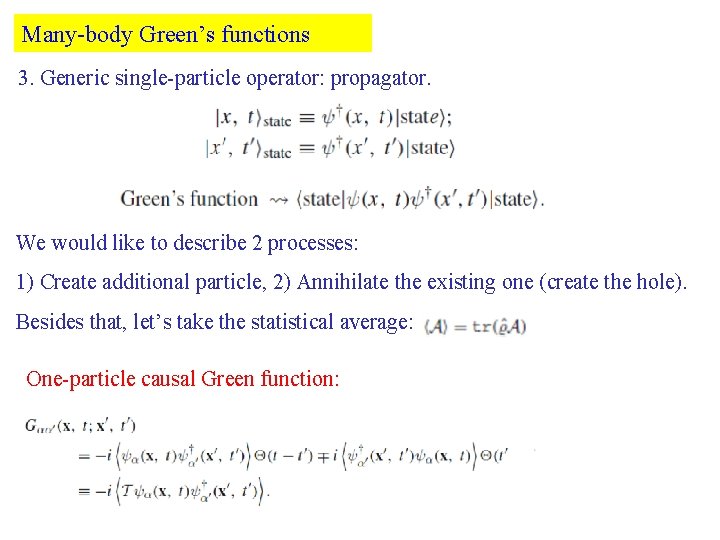
Many-body Green’s functions 1. Goal: calculate approximate values of the macroscopic quantities, which are often expressed through the single-particle operators. Examples Density: Current: 2. Why Green’s Functions? Orthogonality catastrophe: for large systems, any approximate wave function will be almost orthogonal to the exact one. But the error of the averages will be small. H’=H+ V 0|0’ 0|A|0 – 0’|A|0’

Many-body Green’s functions 3. Generic single-particle operator: propagator. We would like to describe 2 processes: 1) Create additional particle, 2) Annihilate the existing one (create the hole). Besides that, let’s take the statistical average: One-particle causal Green function:

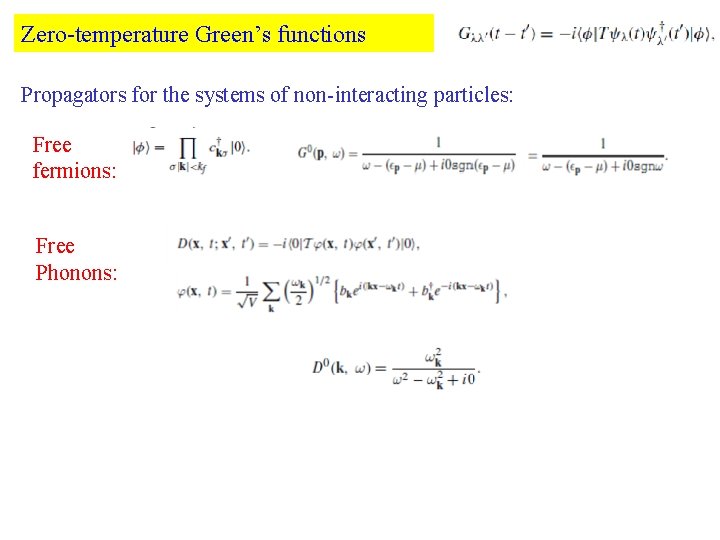
Zero-temperature Green’s functions Average over the grand canonical ensemble: Limit :

Zero-temperature Green’s functions Propagators for the systems of non-interacting particles: Free fermions: Free Phonons:

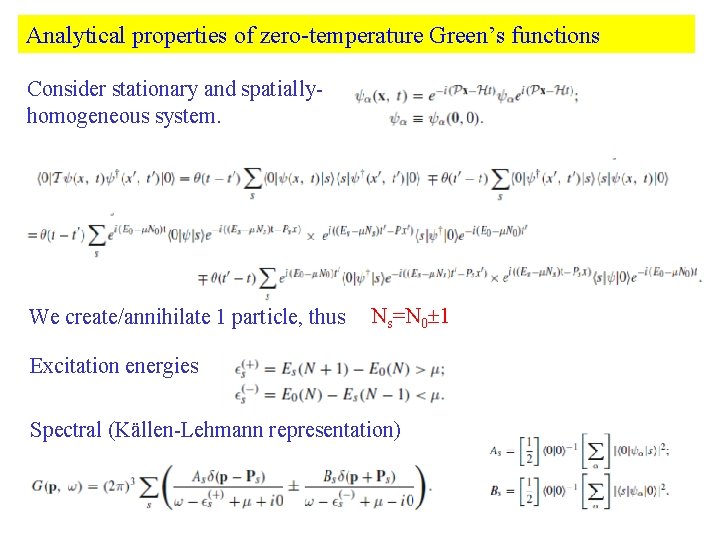
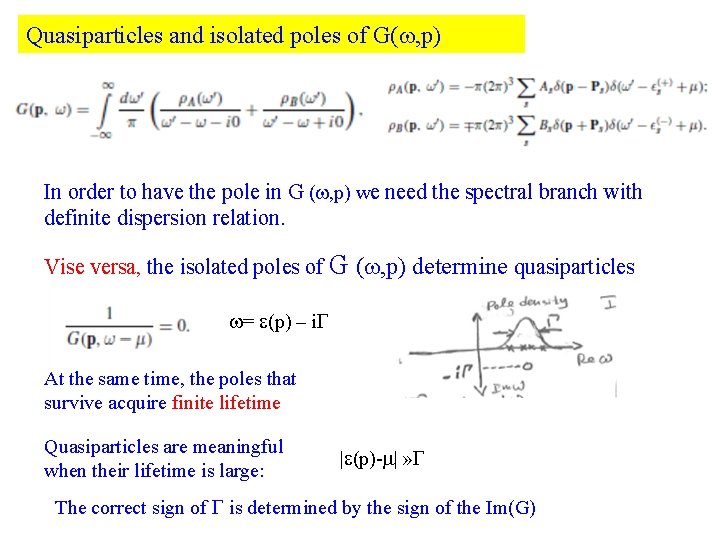
Analytical properties of zero-temperature Green’s functions Consider stationary and spatiallyhomogeneous system. We create/annihilate 1 particle, thus Ns=N 0 1 Excitation energies Spectral (Källen-Lehmann representation)

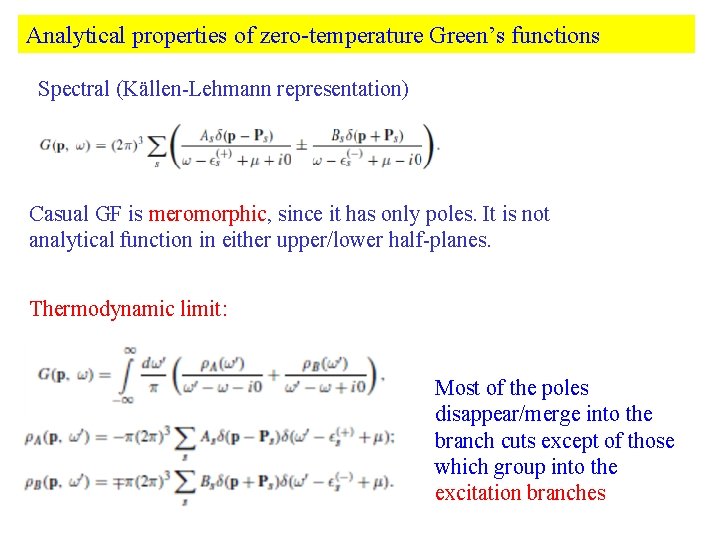
Analytical properties of zero-temperature Green’s functions Spectral (Källen-Lehmann representation) Casual GF is meromorphic, since it has only poles. It is not analytical function in either upper/lower half-planes. Thermodynamic limit: Most of the poles disappear/merge into the branch cuts except of those which group into the excitation branches

Quasiparticles and isolated poles of G( , p) In order to have the pole in G ( , p) we need the spectral branch with definite dispersion relation. Vise versa, the isolated poles of G ( , p) determine quasiparticles = (p) – i At the same time, the poles that survive acquire finite lifetime Quasiparticles are meaningful when their lifetime is large: | (p)- | » The correct sign of is determined by the sign of the Im(G)

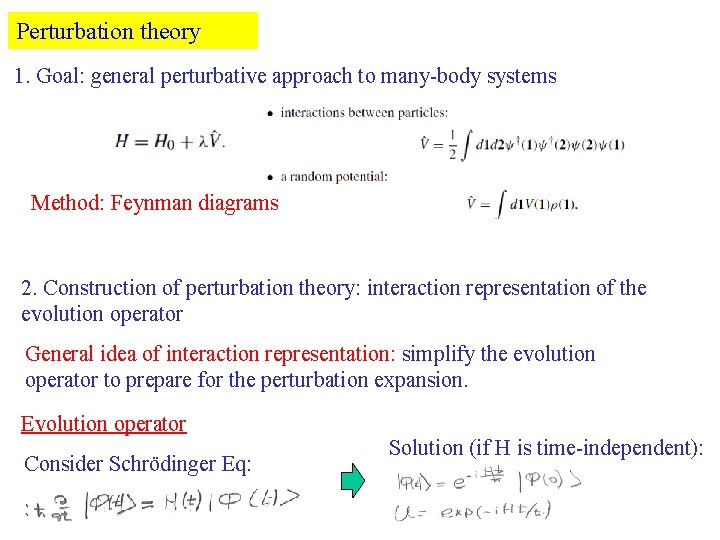
Perturbation theory 1. Goal: general perturbative approach to many-body systems Method: Feynman diagrams 2. Construction of perturbation theory: interaction representation of the evolution operator General idea of interaction representation: simplify the evolution operator to prepare for the perturbation expansion. Evolution operator Consider Schrödinger Eq: Solution (if H is time-independent):

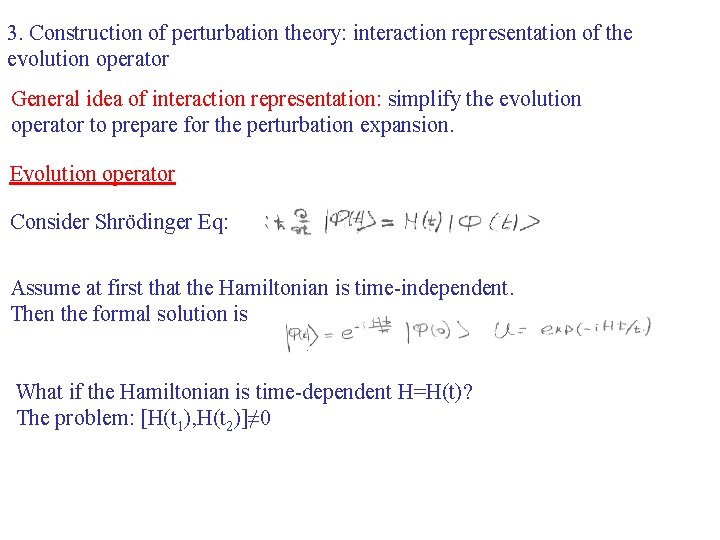
3. Construction of perturbation theory: interaction representation of the evolution operator General idea of interaction representation: simplify the evolution operator to prepare for the perturbation expansion. Evolution operator Consider Shrödinger Eq: Assume at first that the Hamiltonian is time-independent. Then the formal solution is What if the Hamiltonian is time-dependent H=H(t)? The problem: [H(t 1), H(t 2)]≠ 0

Evolution operator What if H=H(t)? The problem: [H(t 1), H(t 2)]≠ 0 Let’s use iterations Rewrite SE as follows Here the operators are time-orders: with larger times are on the left Simpler form of the evolution operator (check!)

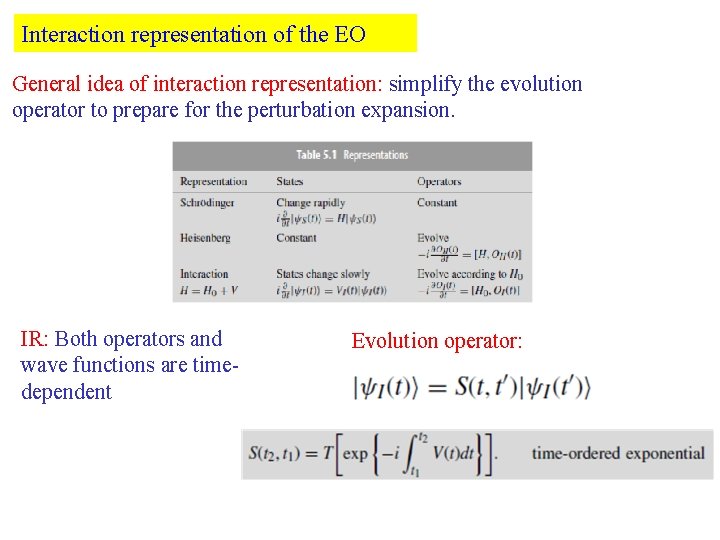
Interaction representation of the EO General idea of interaction representation: simplify the evolution operator to prepare for the perturbation expansion. IR: Both operators and wave functions are timedependent Evolution operator:

Example: single-particle potential scattering