Chapter 11 Angular Momentum Sect 11 1 Vector

Chapter 11: Angular Momentum

Sect. 11. 1: Vector Product & Torque Recall Ch. 7: Scalar Product of Two Vectors • If A & B are vectors, their Scalar Product is defined as: A B ≡ AB cosθ • In terms of vector components & unit vectors i, j, k are along the x, y, z axes: A = Axi + Ayj + Azk B = Bxi + Byj + Bzk • Using i i = j j = k k = 1, i j= i k = j k = 0 gives A B = Ax. Bx + Ay. By + Az. Bz • Dot Product clearly a SCALAR.

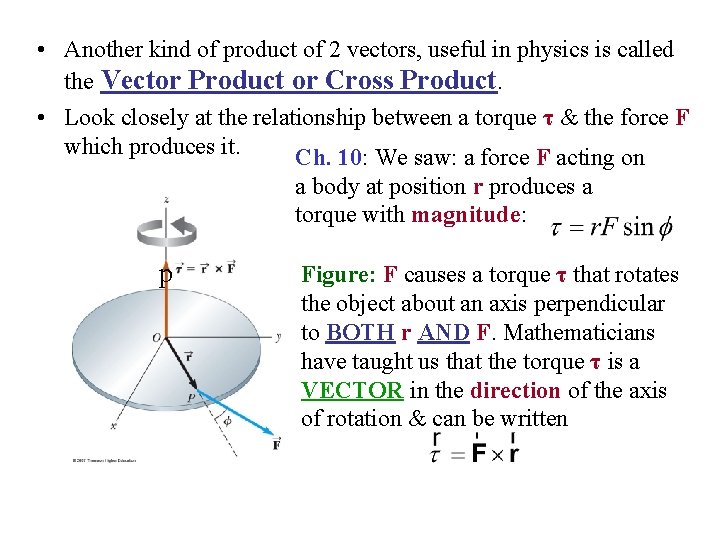
• Another kind of product of 2 vectors, useful in physics is called the Vector Product or Cross Product. • Look closely at the relationship between a torque τ & the force F which produces it. Ch. 10: We saw: a force F acting on a body at position r produces a torque with magnitude: p Figure: F causes a torque τ that rotates the object about an axis perpendicular to BOTH r AND F. Mathematicians have taught us that the torque τ is a VECTOR in the direction of the axis of rotation & can be written

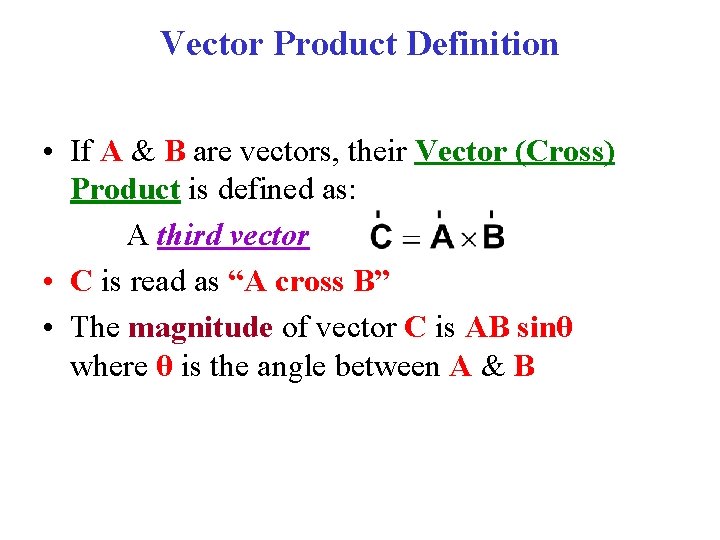
Vector Product Definition • If A & B are vectors, their Vector (Cross) Product is defined as: A third vector • C is read as “A cross B” • The magnitude of vector C is AB sinθ where θ is the angle between A & B

Vector Product • The magnitude of C, which is AB sinθ is equal to the area of the parallelogram formed by A and B. • The direction of C is perpendicular to the plane formed by A and B • The best way to determine this direction is to use the right-hand rule

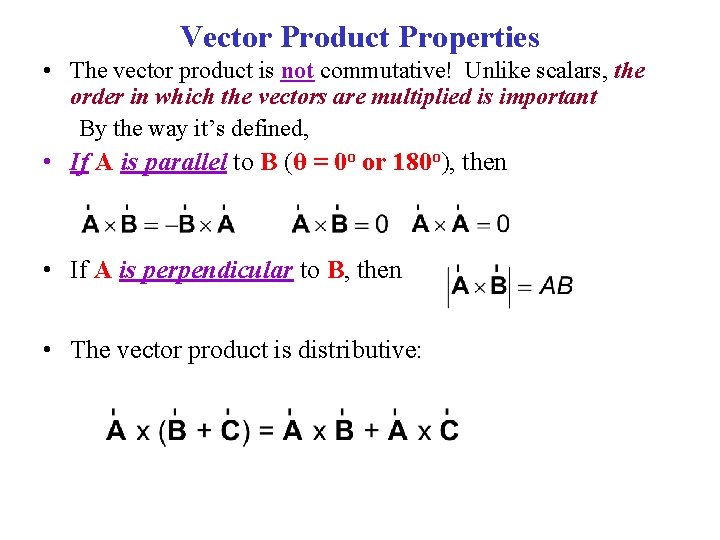
Vector Product Properties • The vector product is not commutative! Unlike scalars, the order in which the vectors are multiplied is important By the way it’s defined, • If A is parallel to B (θ = 0 o or 180 o), then • If A is perpendicular to B, then • The vector product is distributive:

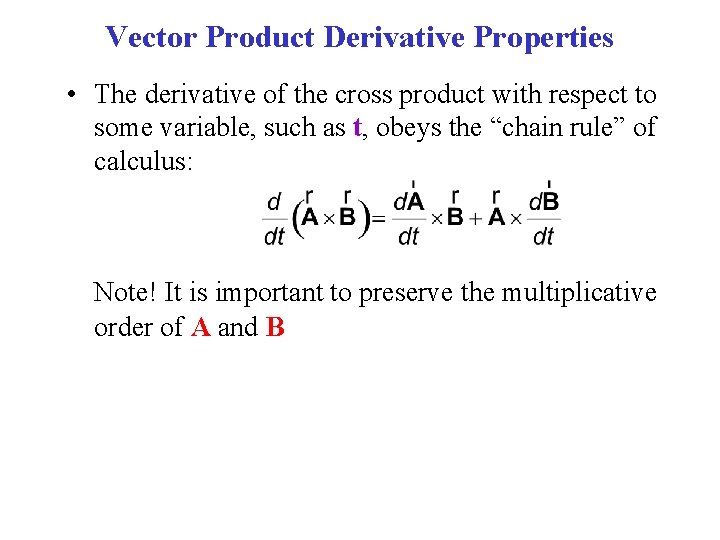
Vector Product Derivative Properties • The derivative of the cross product with respect to some variable, such as t, obeys the “chain rule” of calculus: Note! It is important to preserve the multiplicative order of A and B

Vector Products of Unit Vectors Contrast with scalar products of unit vectors Signs are interchangeable in cross products

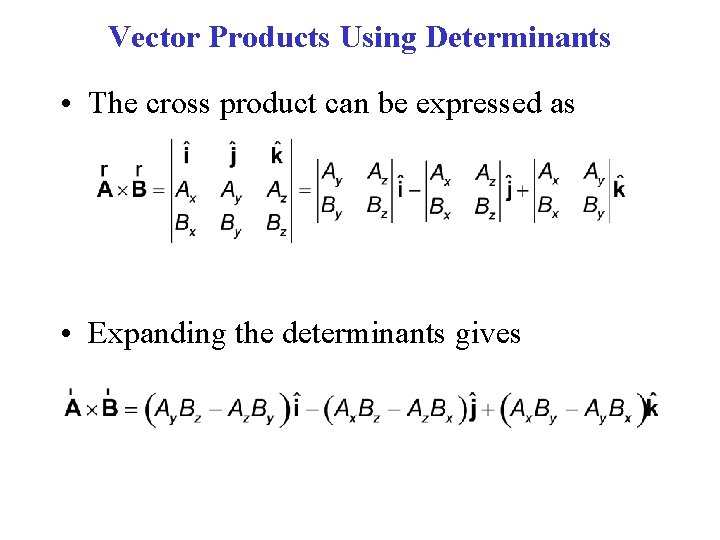
Vector Products Using Determinants • The cross product can be expressed as • Expanding the determinants gives

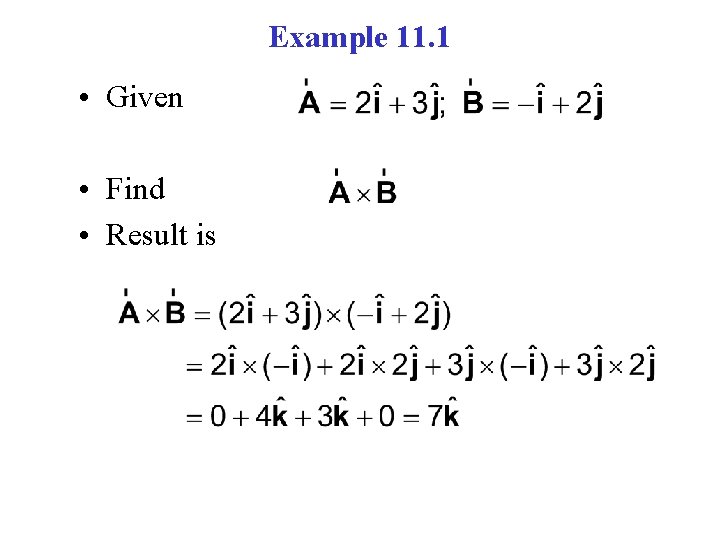
Example 11. 1 • Given • Find • Result is

Example 11. 2: Torque Vector • Given the force F and position r: • Find the torque τ produced • Result is

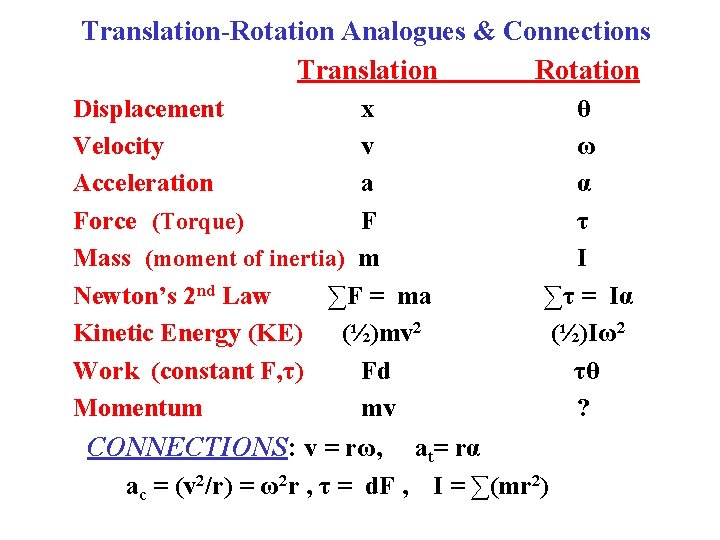
Translation-Rotation Analogues & Connections Translation Rotation Displacement x Velocity v Acceleration a Force (Torque) F Mass (moment of inertia) m Newton’s 2 nd Law ∑F = ma Kinetic Energy (KE) (½)mv 2 Work (constant F, τ) Fd Momentum mv CONNECTIONS: v = rω, θ ω α τ I ∑τ = Iα (½)Iω2 τθ ? at= rα ac = (v 2/r) = ω2 r , τ = d. F , I = ∑(mr 2)

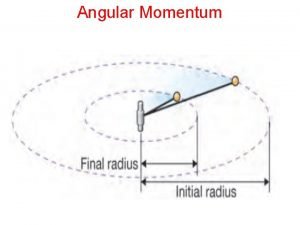
Sect. 11. 2: Angular Momentum Consider a rigid, vertical pole through a frozen ice pond, as in the figure. As a skater moves in a straight line past it, she grabs & holds the pole & suddenly she is going in a circle around the pole. We can analyze this motion by using the concept of Angular Momentum

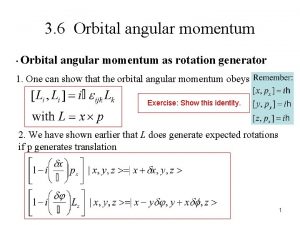
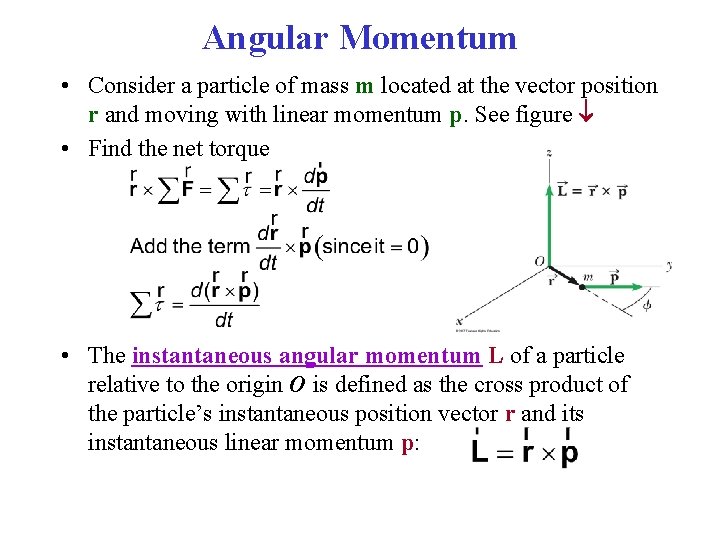
Angular Momentum • Consider a particle of mass m located at the vector position r and moving with linear momentum p. See figure • Find the net torque • The instantaneous angular momentum L of a particle relative to the origin O is defined as the cross product of the particle’s instantaneous position vector r and its instantaneous linear momentum p:

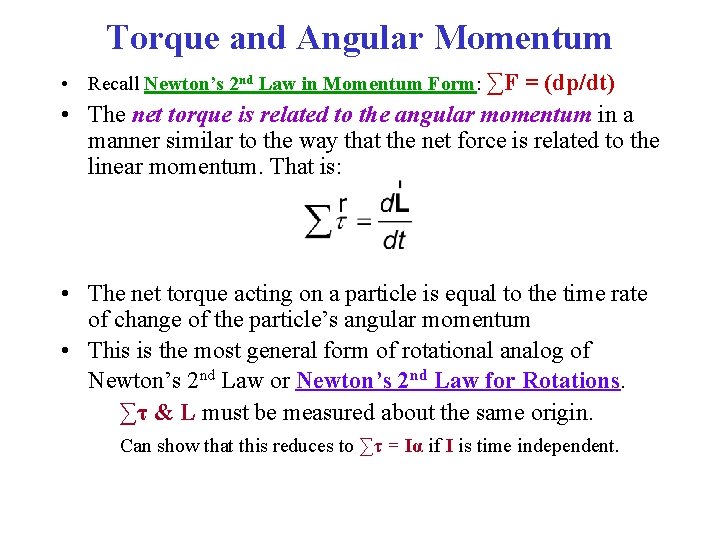
Torque and Angular Momentum • Recall Newton’s 2 nd Law in Momentum Form: ∑F = (dp/dt) • The net torque is related to the angular momentum in a manner similar to the way that the net force is related to the linear momentum. That is: • The net torque acting on a particle is equal to the time rate of change of the particle’s angular momentum • This is the most general form of rotational analog of Newton’s 2 nd Law or Newton’s 2 nd Law for Rotations. ∑τ & L must be measured about the same origin. Can show that this reduces to ∑τ = Iα if I is time independent.

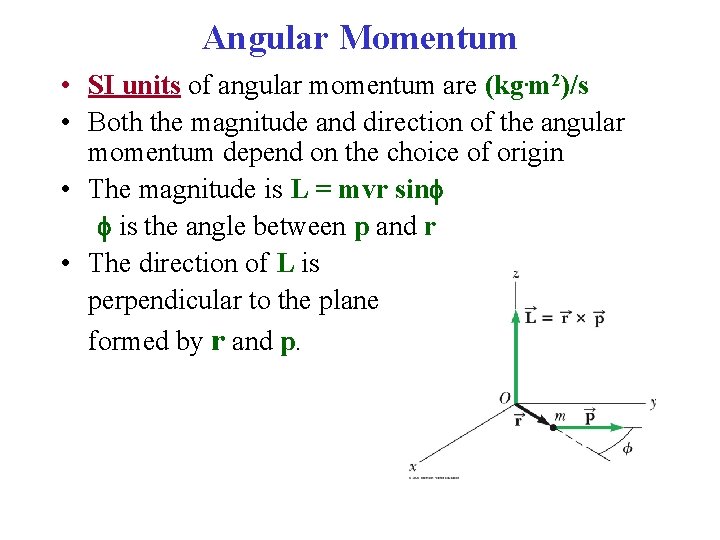
Angular Momentum • SI units of angular momentum are (kg. m 2)/s • Both the magnitude and direction of the angular momentum depend on the choice of origin • The magnitude is L = mvr sin is the angle between p and r • The direction of L is perpendicular to the plane formed by r and p.

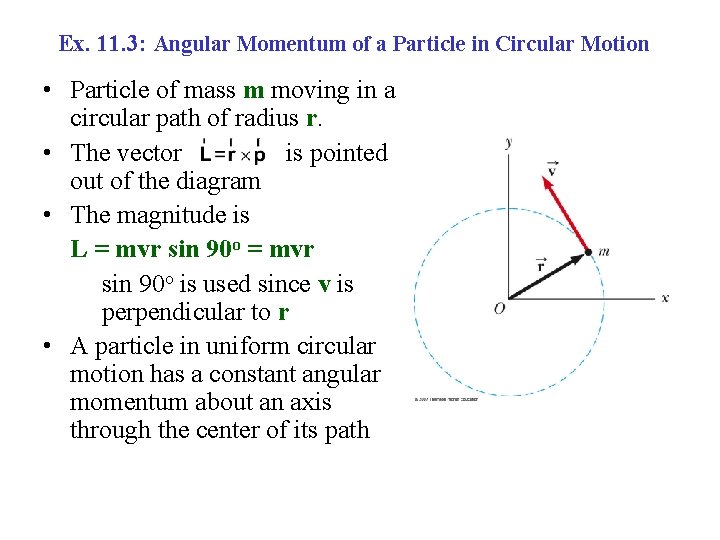
Ex. 11. 3: Angular Momentum of a Particle in Circular Motion • Particle of mass m moving in a circular path of radius r. • The vector is pointed out of the diagram • The magnitude is L = mvr sin 90 o is used since v is perpendicular to r • A particle in uniform circular motion has a constant angular momentum about an axis through the center of its path

Angular Momentum of a Particle System • Angular Momentum of a System of Particles = vector sum of the angular momenta of the each particle: • Differentiating with respect to time gives Newton’s 2 nd Law for Rotations in a many particle system: • System has internal forces & external forces. Can show: All torques coming from internal forces add to zero. So, ∑τi becomes ∑τext where now the sum includes external torques only. • Therefore, Newton’s 2 nd Law for Rotations in a many particle system becomes: – The net external torque acting on a system about some axis passing through an origin in an inertial frame equals the time rate of change of the total angular momentum of the system about that origin

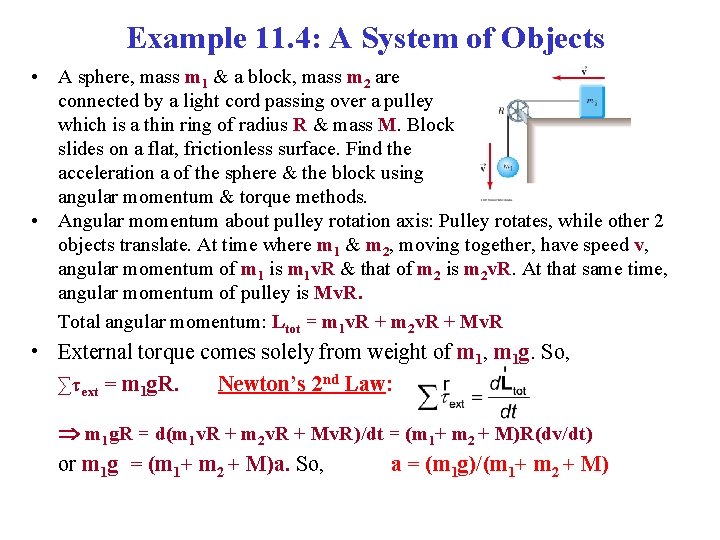
Example 11. 4: A System of Objects • A sphere, mass m 1 & a block, mass m 2 are connected by a light cord passing over a pulley which is a thin ring of radius R & mass M. Block slides on a flat, frictionless surface. Find the acceleration a of the sphere & the block using angular momentum & torque methods. • Angular momentum about pulley rotation axis: Pulley rotates, while other 2 objects translate. At time where m 1 & m 2, moving together, have speed v, angular momentum of m 1 is m 1 v. R & that of m 2 is m 2 v. R. At that same time, angular momentum of pulley is Mv. R. Total angular momentum: Ltot = m 1 v. R + m 2 v. R + Mv. R • External torque comes solely from weight of m 1, m 1 g. So, ∑τext = m 1 g. R. Newton’s 2 nd Law: m 1 g. R = d(m 1 v. R + m 2 v. R + Mv. R)/dt = (m 1+ m 2 + M)R(dv/dt) or m 1 g = (m 1+ m 2 + M)a. So, a = (m 1 g)/(m 1+ m 2 + M)