THEORY OF SOLAR MAGNETIC FLUX ROPES CMEs DYNAMICS











- Slides: 42

THEORY OF SOLAR MAGNETIC FLUX ROPES: CMEs DYNAMICS • James Chen • Plasma Physics Division, Naval Research Laboratory George Mason University 21 Feb 2008

SOLAR ERUPTIONS • Canonical parameters of solar eruptions: – KE, photons, particles – Mass g – Speed ~100 - 2000 km/s erg • Space Weather: CMEs are the solar drivers of large geomagnetic storms – Physical mechanism: CMEs lead to strong-B solar wind (SW) structures (i. e. , strong southward IMF) impinging on the magnetosphere – CMEs are magnetically dominated structures

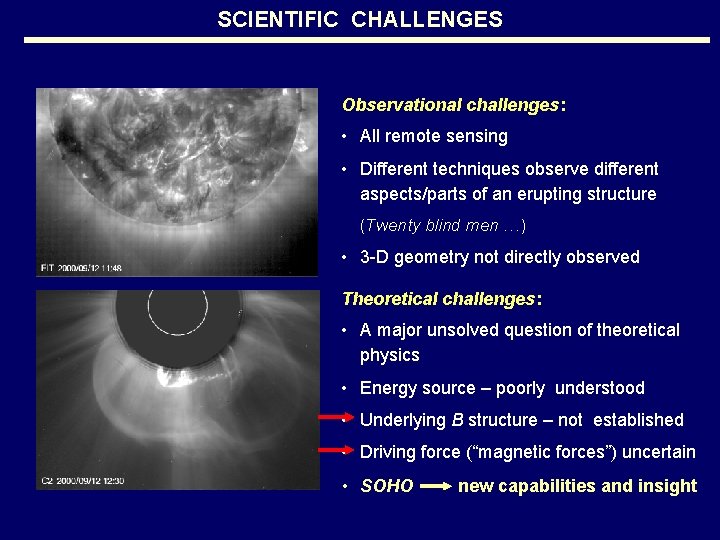
SCIENTIFIC CHALLENGES Observational challenges: • All remote sensing • Different techniques observe different aspects/parts of an erupting structure (Twenty blind men …) • 3 -D geometry not directly observed Theoretical challenges: • A major unsolved question of theoretical physics • Energy source – poorly understood • Underlying B structure – not established • Driving force (“magnetic forces”) uncertain • SOHO new capabilities and insight

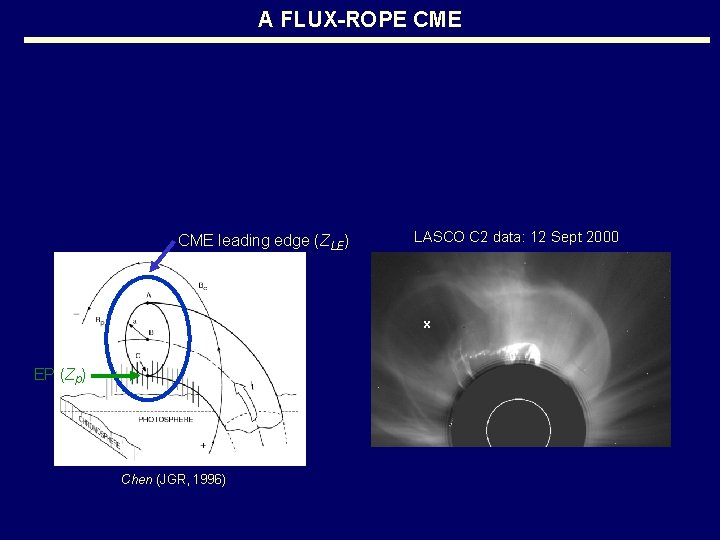
A FLUX-ROPE CME leading edge (ZLE) LASCO C 2 data: 12 Sept 2000 x EP (Zp) Chen (JGR, 1996)

OBSERVATIONAL EVIDENCE (No prominence included) • Good quantitative agreement with a flux rope viewed end-on – No evidence of reconnection (Chen et al. 1997) • Other examples of flux-rope CMEs (Wood et al. 1999; Dere et al. , 1999; Wu et al. 1999; Plunkett et al. 2000; Yurchyshyn 2000; Chen et al. 2000; Krall et al. 2001)

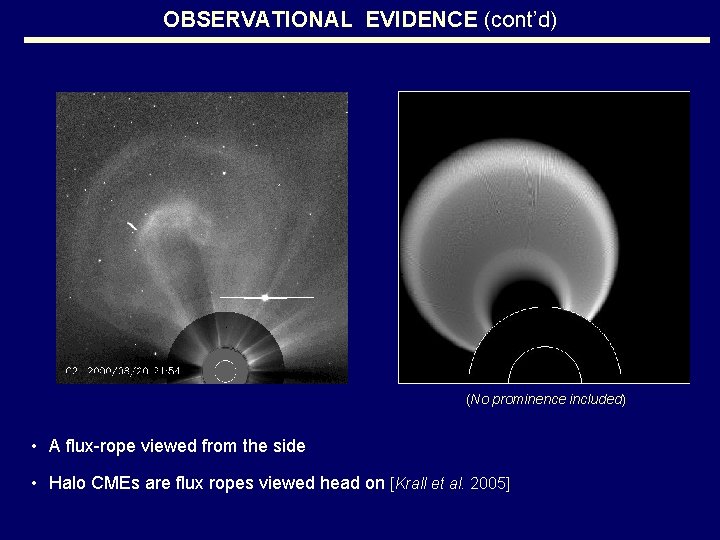
OBSERVATIONAL EVIDENCE (cont’d) (No prominence included) • A flux-rope viewed from the side • Halo CMEs are flux ropes viewed head on [Krall et al. 2005]

3 -D GEOMETRY OF CMEs • “Coronal transients” (1970’s: OSO-7, Skylab) • “Thin” flux tubes FOV: 1. 7 – 6 Rs (Mouschovias and Poland 1978; Anzer 1978) • Halo CMEs (Solwind) (Howard et al. 1982) – Fully 3 -D in extent • CME morphology (SMM): (Illing and Hundhausen 1986) – A CME consists of 3 -parts: a bright frontal rim, cavity, and a core – Conceptual structure: rotational symmetry (e. g. , ice cream cone, light bulb) (Hundhausen 1999) • SOHO data: 3 -D flux ropes (Chen et al. 1997) – 3 -part morphology is only part of a CME (Illing and Hundhausen (1986) SMM (1980 -1981; 1984 -1989)

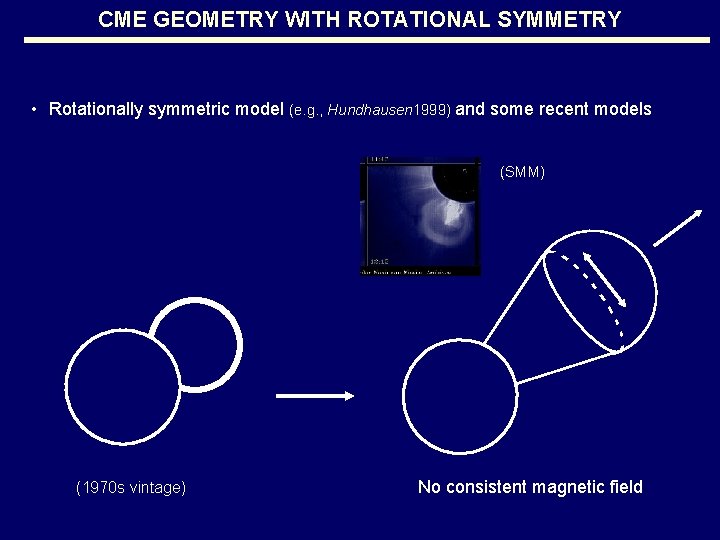
CME GEOMETRY WITH ROTATIONAL SYMMETRY • Rotationally symmetric model (e. g. , Hundhausen 1999) and some recent models (SMM) (1970 s vintage) No consistent magnetic field

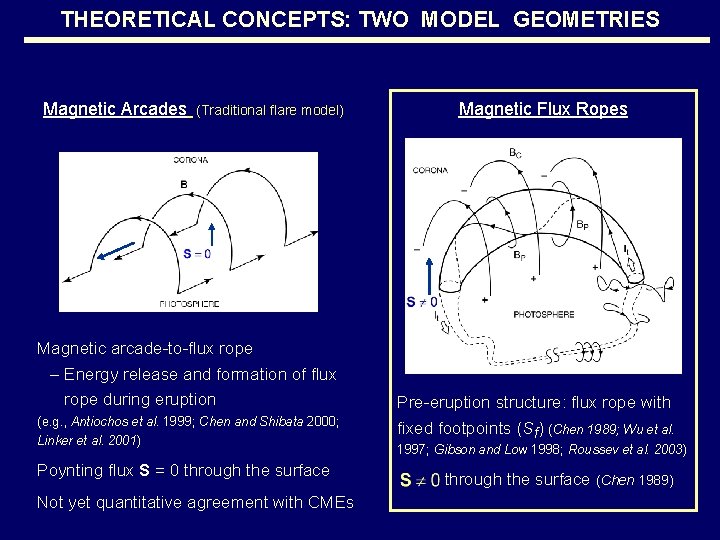
THEORETICAL CONCEPTS: TWO MODEL GEOMETRIES Magnetic Arcades (Traditional flare model) Magnetic arcade-to-flux rope – Energy release and formation of flux rope during eruption (e. g. , Antiochos et al. 1999; Chen and Shibata 2000; Linker et al. 2001) Poynting flux S = 0 through the surface Not yet quantitative agreement with CMEs Magnetic Flux Ropes Pre-eruption structure: flux rope with fixed footpoints (Sf) (Chen 1989; Wu et al. 1997; Gibson and Low 1998; Roussev et al. 2003) through the surface (Chen 1989)

GENERAL 1 D FLUX ROPE • Consider a 1 -D straight flux rope – Embedded in a background plasma of pressure pa – MHD equilibrium: – r = a : minor radius • Momentum equation: pa • Requirements for B: • Toroidal (locally axial) field: Bt(r) Poloidal (locally azimuthal) field: Bp(r)

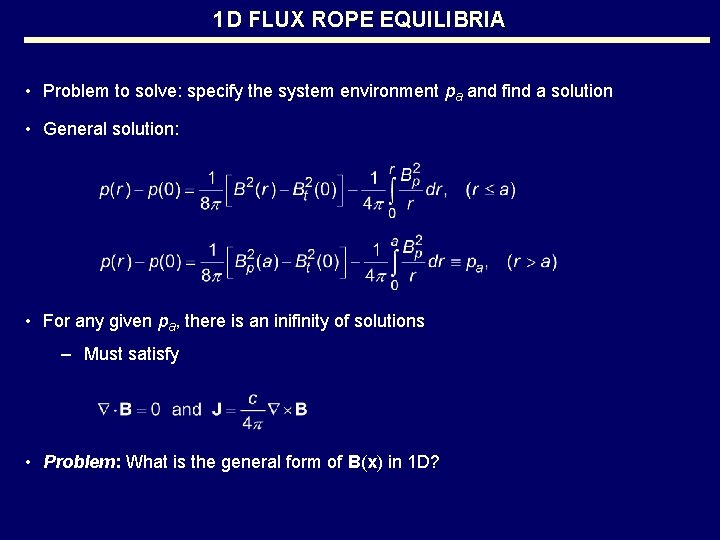
1 D FLUX ROPE EQUILIBRIA • Problem to solve: specify the system environment pa and find a solution • General solution: • For any given pa, there is an inifinity of solutions – Must satisfy • Problem: What is the general form of B(x) in 1 D?

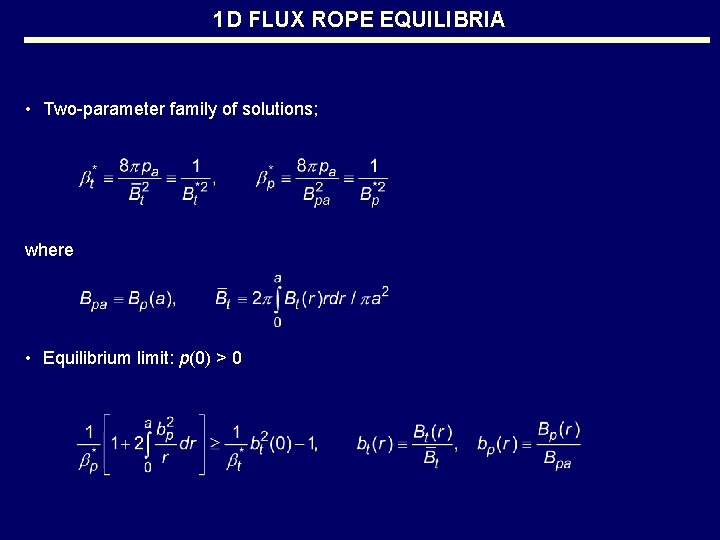
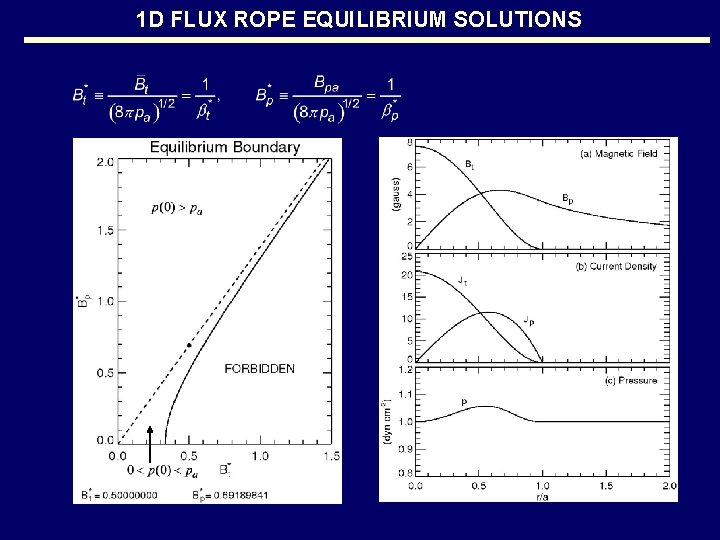
1 D FLUX ROPE EQUILIBRIA • Two-parameter family of solutions; where • Equilibrium limit: p(0) > 0

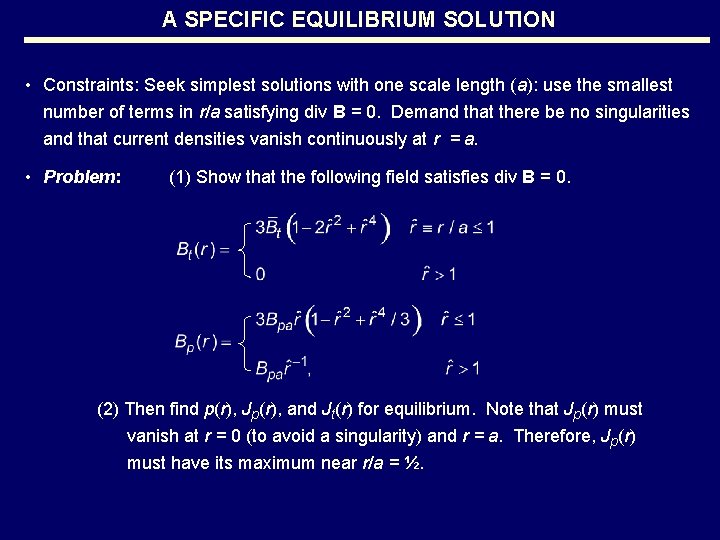
A SPECIFIC EQUILIBRIUM SOLUTION • Constraints: Seek simplest solutions with one scale length (a): use the smallest number of terms in r/a satisfying div B = 0. Demand that there be no singularities and that current densities vanish continuously at r = a. • Problem: (1) Show that the following field satisfies div B = 0. (2) Then find p(r), Jp(r), and Jt(r) for equilibrium. Note that Jp(r) must vanish at r = 0 (to avoid a singularity) and r = a. Therefore, Jp(r) must have its maximum near r/a = ½.

1 D FLUX ROPE EQUILIBRIUM SOLUTIONS

EQUILIBRIUM BOUNDARY • Problem: (1) Derive the equilibrium condition for given pa. Show that the equilibrium boundary asymptotes to the straight line (2) What is the physical meaning of the asymptote?

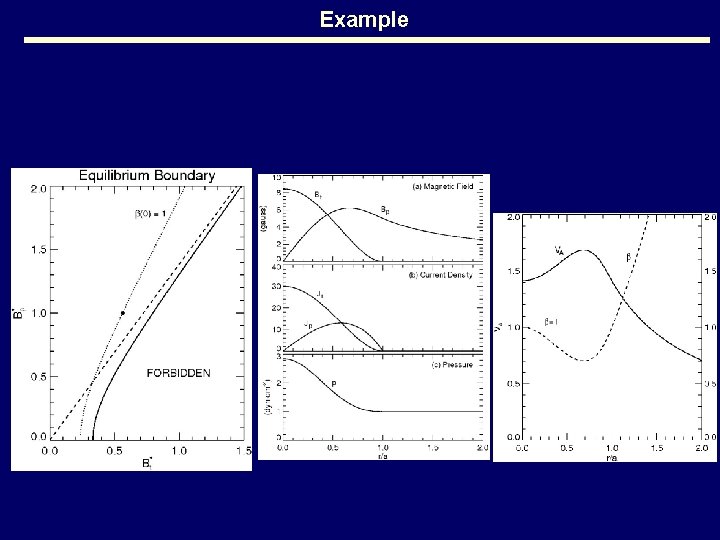
A SPECIFIC EQUILIBRIUM SOLUTION • Problem: (1) Find representative solutions to verify the general characteristics. Plot the results for different regimes. (2) Calculate the local Alfven speed inside and outside the current channel based on the total magnetic field. Express the results in terms of the ion thermal speed, assuming constant temperature. (3) Show boundary curve on the plot

Example

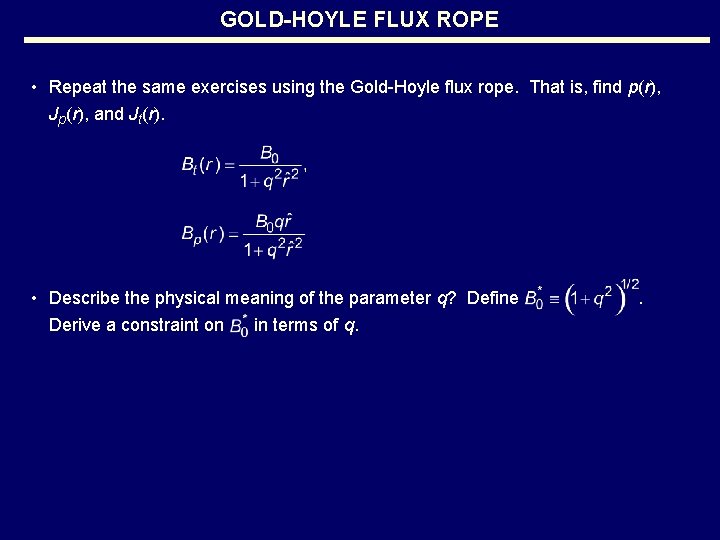
GOLD-HOYLE FLUX ROPE • Repeat the same exercises using the Gold-Hoyle flux rope. That is, find p(r), Jp(r), and Jt(r). • Describe the physical meaning of the parameter q? Define Derive a constraint on in terms of q. .

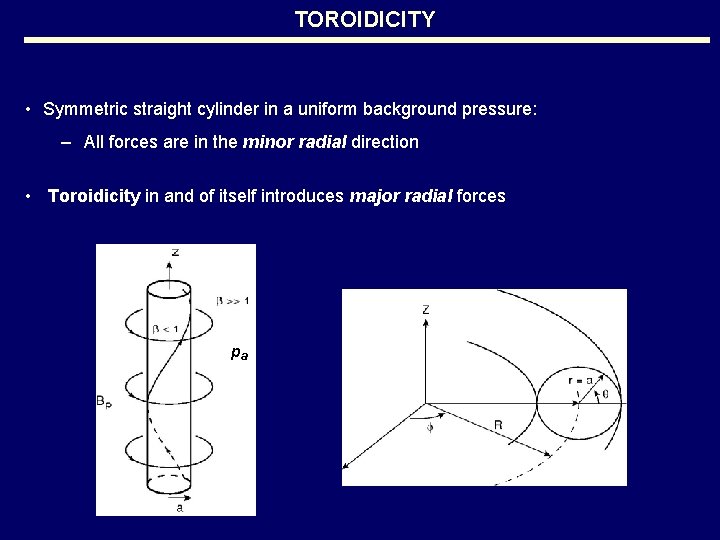
TOROIDICITY • Symmetric straight cylinder in a uniform background pressure: – All forces are in the minor radial direction • Toroidicity in and of itself introduces major radial forces pa

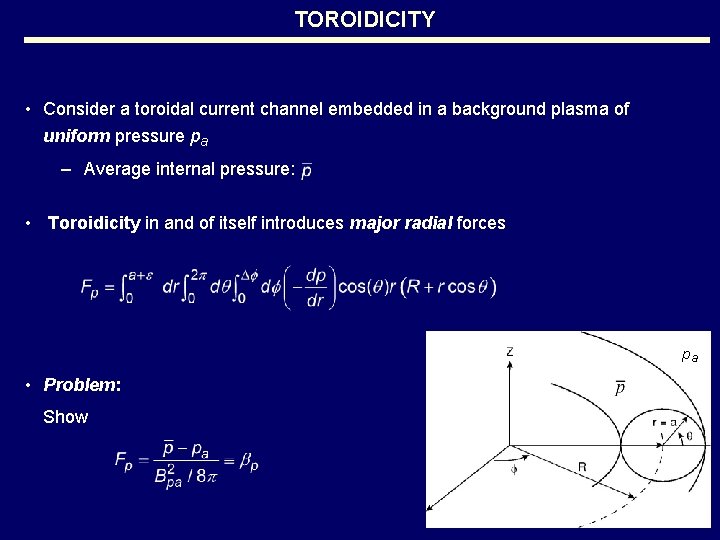
TOROIDICITY • Consider a toroidal current channel embedded in a background plasma of uniform pressure pa – Average internal pressure: • Toroidicity in and of itself introduces major radial forces pa • Problem: Show

LORENTZ (HOOP) FORCE • Self-force in the presence of major radial curvature; Biot-Savart law • Consider an axisymmetric toroidal current-carrying plasma Shafranov (1966, p. 117); Landau and Lifshitz (1984, p. 124); Garren and Chen (Phys. Plasmas 1, 3425, 1994); Miyamoto (Plasma Physics for Nuclear Fusion, 1989) R

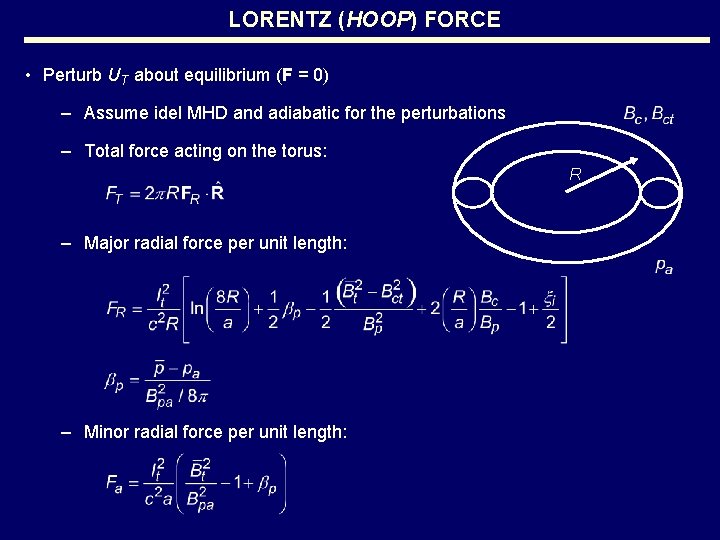
LORENTZ (HOOP) FORCE • Perturb UT about equilibrium (F = 0) – Assume idel MHD and adiabatic for the perturbations – Total force acting on the torus: R – Major radial force per unit length: – Minor radial force per unit length:

APPLICATION TO SOLAR FLUX ROPES • Solar flux ropes: – Non-axisymmetric – R/a is not uniform • Eruptive phenomena: – Shafranov’s derivation is for equilibrium (FR = 0 and Fa = 0) – CMEs are highly dynamic – Drag and gravity • Application to CMEs: Assumptions

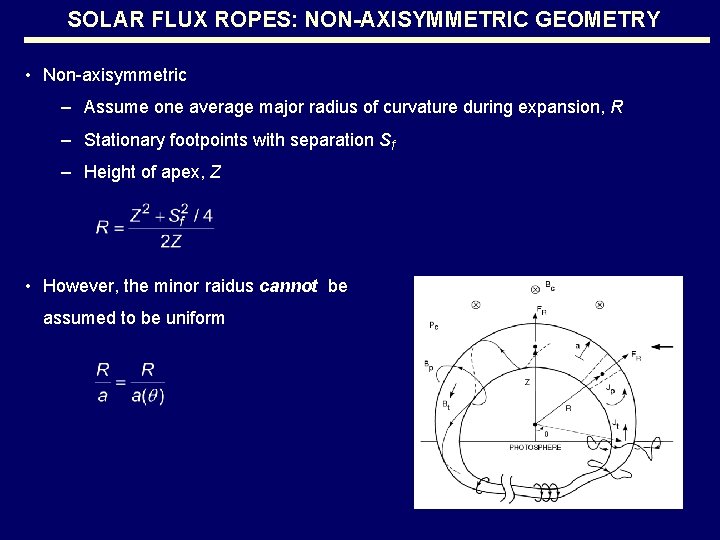
SOLAR FLUX ROPES: NON-AXISYMMETRIC GEOMETRY • Non-axisymmetric – Assume one average major radius of curvature during expansion, R – Stationary footpoints with separation Sf – Height of apex, Z • However, the minor raidus cannot be assumed to be uniform

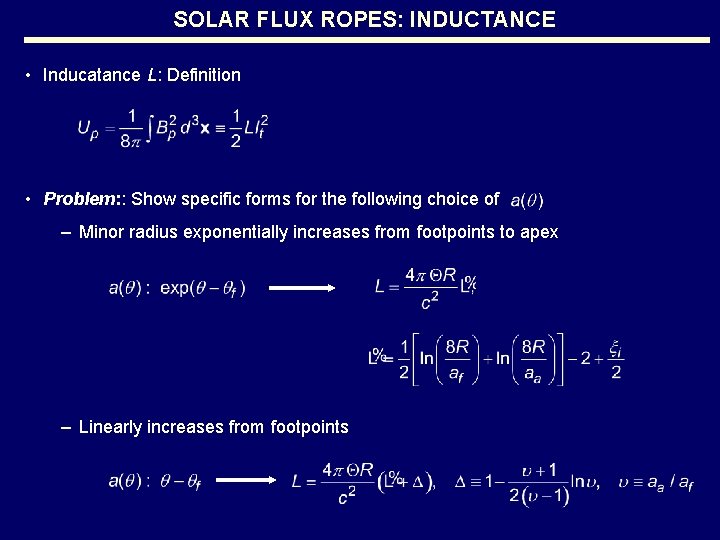
SOLAR FLUX ROPES: INDUCTANCE • Inducatance L: Definition • Problem: : Show specific forms for the following choice of – Minor radius exponentially increases from footpoints to apex – Linearly increases from footpoints

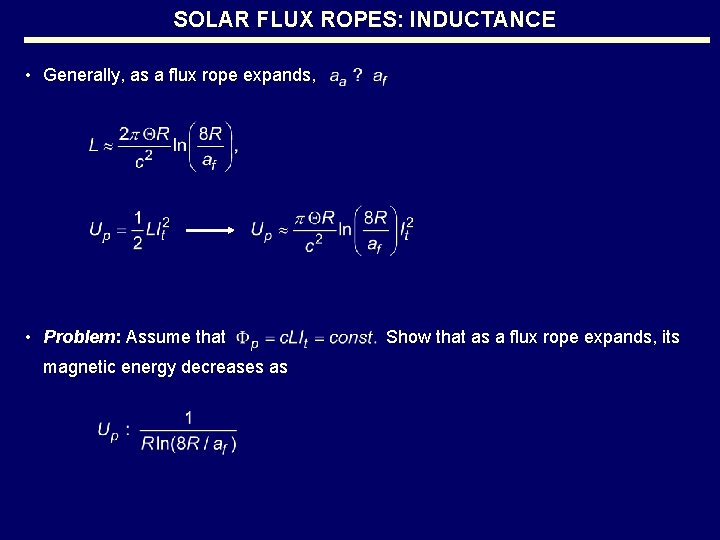
SOLAR FLUX ROPES: INDUCTANCE • Generally, as a flux rope expands, • Problem: Assume that magnetic energy decreases as Show that as a flux rope expands, its

SOLAR FLUX ROPES: INTERACTION WITH CORONA • Dynamically, the most important interaction is momentum coupling (i. e. , forces) – Drag: Flow around flux rope is not laminar (high magnetic Reynolds’ number) – Gravity:

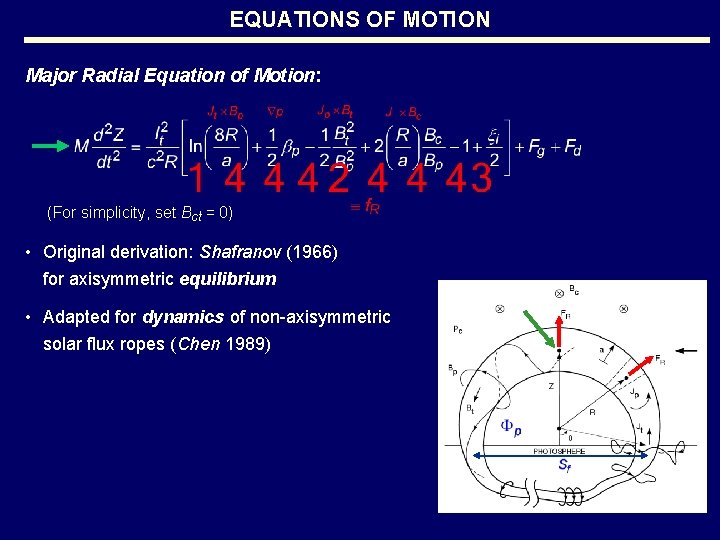
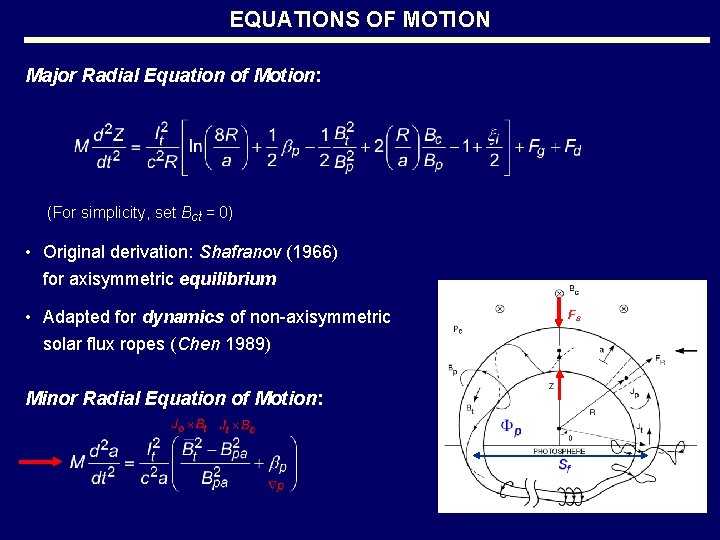
EQUATIONS OF MOTION Major Radial Equation of Motion: (For simplicity, set Bct = 0) • Original derivation: Shafranov (1966) for axisymmetric equilibrium • Adapted for dynamics of non-axisymmetric solar flux ropes (Chen 1989)

EQUATIONS OF MOTION Major Radial Equation of Motion: (For simplicity, set Bct = 0) • Original derivation: Shafranov (1966) for axisymmetric equilibrium • Adapted for dynamics of non-axisymmetric solar flux ropes (Chen 1989) Minor Radial Equation of Motion: Fa

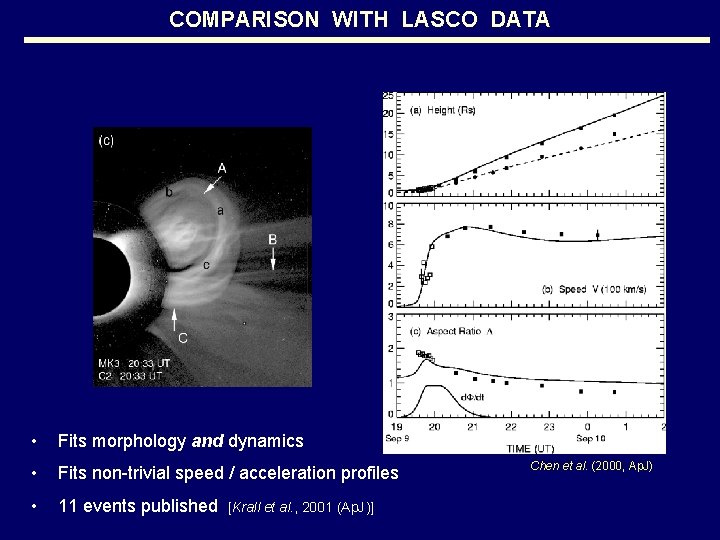
COMPARISON WITH LASCO DATA • Fits morphology and dynamics • Fits non-trivial speed / acceleration profiles • 11 events published [Krall et al. , 2001 (Ap. J)] Chen et al. (2000, Ap. J)

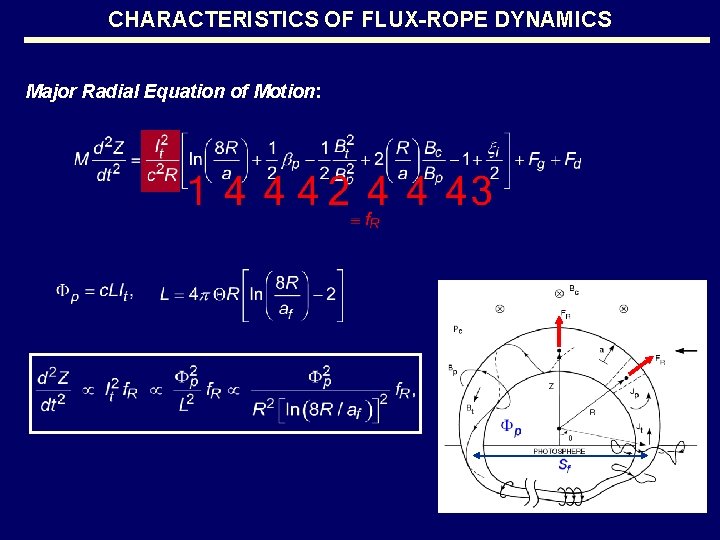
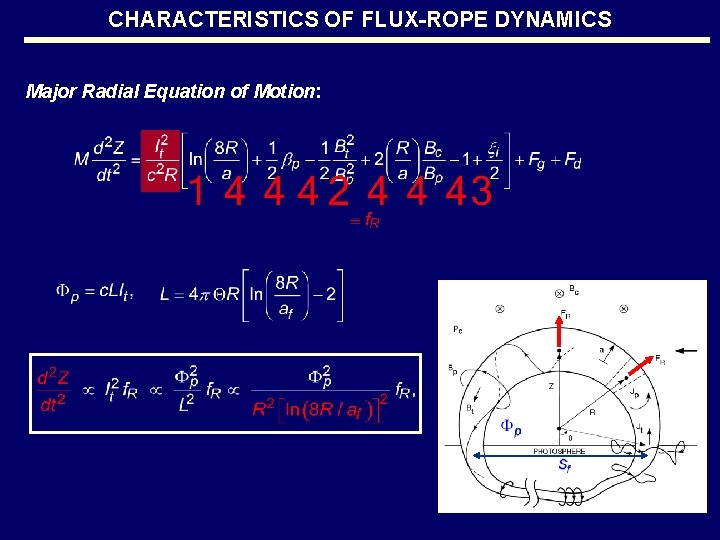
CHARACTERISTICS OF FLUX-ROPE DYNAMICS Major Radial Equation of Motion:

CHARACTERISTICS OF FLUX-ROPE DYNAMICS Major Radial Equation of Motion:

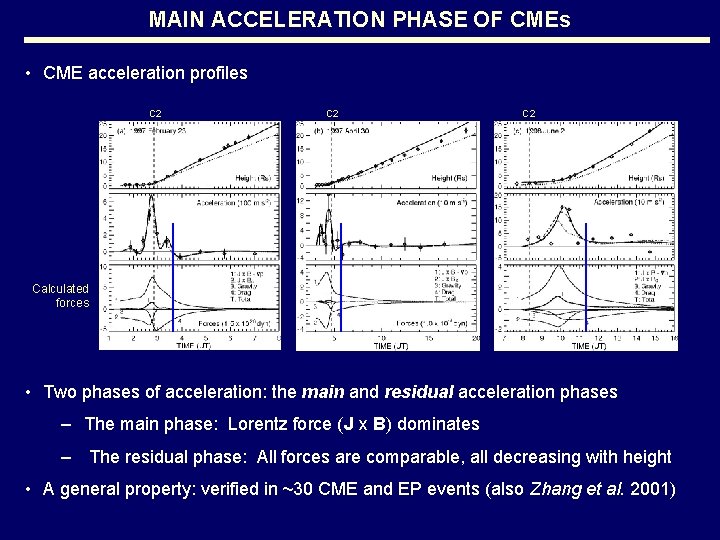
MAIN ACCELERATION PHASE OF CMEs • CME acceleration profiles C 2 C 2 Calculated forces • Two phases of acceleration: the main and residual acceleration phases – The main phase: Lorentz force (J x B) dominates – The residual phase: All forces are comparable, all decreasing with height • A general property: verified in ~30 CME and EP events (also Zhang et al. 2001)

PHYSICS OF THE MAIN ACCELERATION PHASE • Basic length scale of the equation of motion Two critical heights: Z* and Zm • Z*: Curvature is maximum (i. e. , R is minimum) at apex height Z* (analytic result from geometry) • k. R(R) is maximum at • Depends only on the 3 -D toroidal geometry with fixed

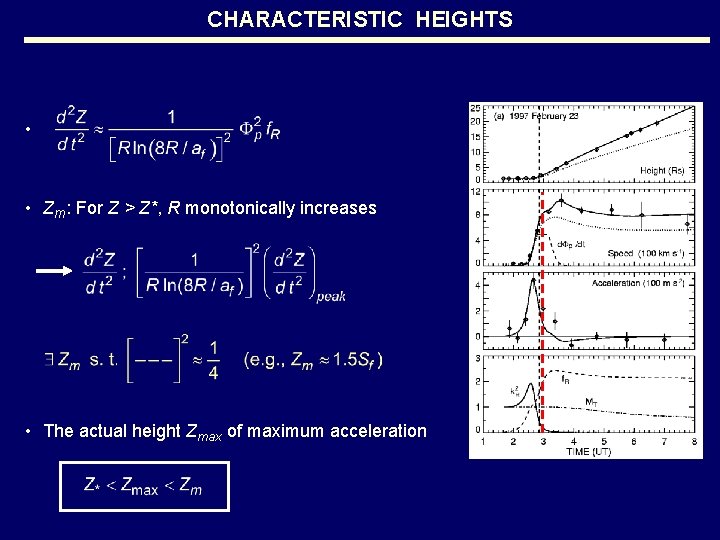
CHARACTERISTIC HEIGHTS • • Zm: For Z > Z*, R monotonically increases • The actual height Zmax of maximum acceleration

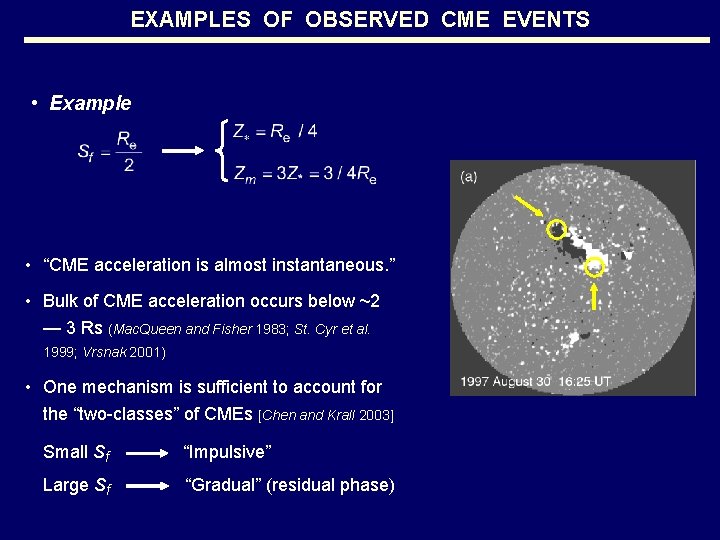
EXAMPLES OF OBSERVED CME EVENTS • Example • “CME acceleration is almost instantaneous. ” • Bulk of CME acceleration occurs below ~2 — 3 Rs (Mac. Queen and Fisher 1983; St. Cyr et al. 1999; Vrsnak 2001) • One mechanism is sufficient to account for the “two-classes” of CMEs [Chen and Krall 2003] Small Sf “Impulsive” Large Sf “Gradual” (residual phase)

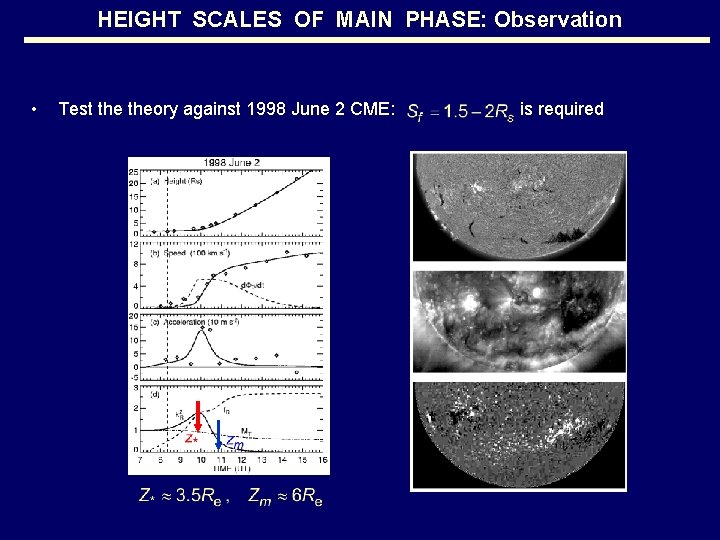
HEIGHT SCALES OF MAIN PHASE: Observation • Test theory against 1998 June 2 CME: is required

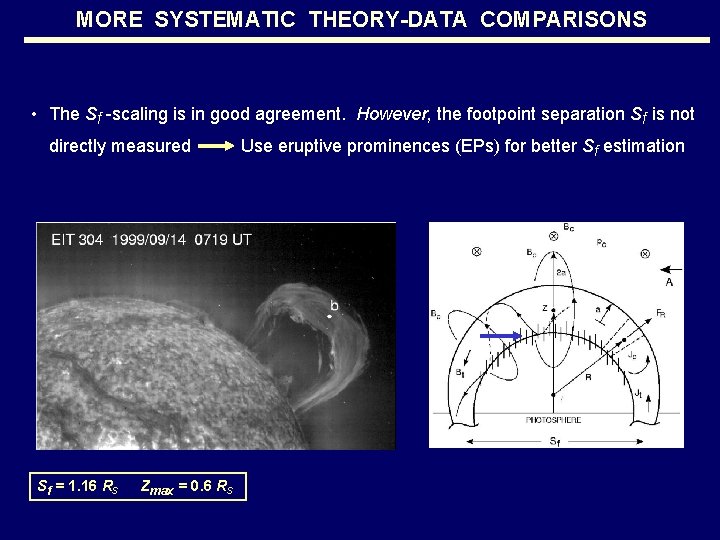
MORE SYSTEMATIC THEORY-DATA COMPARISONS • The Sf -scaling is in good agreement. However, the footpoint separation Sf is not directly measured Sf = 1. 16 RS Zmax = 0. 6 RS Use eruptive prominences (EPs) for better Sf estimation

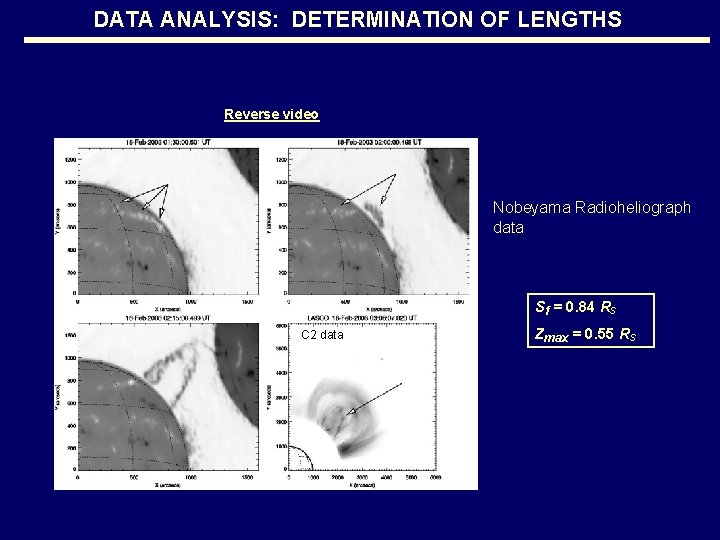
DATA ANALYSIS: DETERMINATION OF LENGTHS Reverse video Nobeyama Radioheliograph data Sf = 0. 84 RS C 2 data Zmax = 0. 55 RS

GEOMETRICAL ASSUMPTIONS • The scaling law: most directly given in quantities of flux rope (Z, Sf, Zmax, Z*) • Relationship between CME, prominence, and flux rope quantities – CME leading edge (LE): ZLE = Z + 2 aa – Prominence LE: Z = Zp – a – Prominence footpoints: Sp = Sf – 2 af • Observed quantities: – Sp, Zpmax • Calculate: – Sf (Sp, af ) – Zmax (Zpmax, aa )

COMPARISON WITH DATA • CMEs: Use magnetic neutral line or H filament to estimate Sf ; ZLE = Z + 2 a EPs: Sf = Sp + 2 af Zp = Z – a EP data CME data 4 CMEs (LASCO) 13 EPs (radio, H ) • A quantitative test of the accelerating force and the geometrical assumptions • A quantitative challenge to all CME models Chen et al. , Ap. J (2006)

MORE PROPERTIES OF EQUATIONS OF MOTION • Show that during expansion the poloidal field (Jt. Bp) loses energy to the kinetic energy of the flux rope and toroidal field. • Some CME models invoke buoyancy as the driving force. Assuming Fg is the driving force, calculate the terminal speed of the expanding flux rope if the drag is force is taken into account. How fast can buoyancy alone drive a flux rope? • Consider the momentum equation. Assuming that only Jx. B and pressure gradient force are present, what is the characteristic speed of a magnetized plasma structure of relevant dimension D? What is the characteristic time? • Normalize the MHD momentum equation to the characteristic speed and time for plasma motions in the photosphere and the corona. Can the equations of motion distinguish between the two disparate mediums?
 Coercivity
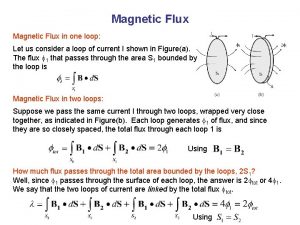
Coercivity Unit of magnetic flux is weber
Unit of magnetic flux is weber How to find magnetic flux
How to find magnetic flux Radial magnetic field
Radial magnetic field Express magnetic flux mathematically
Express magnetic flux mathematically Force of magnetic field formula
Force of magnetic field formula Magnetic flux units
Magnetic flux units Magnetic field unit
Magnetic field unit Magnetic flux
Magnetic flux Flux magnetic
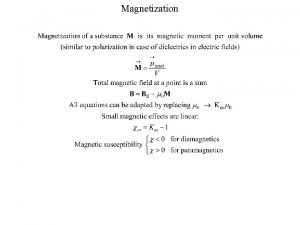
Flux magnetic Magnetization formula
Magnetization formula F=bil triangle
F=bil triangle Magnetic flux
Magnetic flux Magnetic flux = ba
Magnetic flux = ba Ampere maxwell law
Ampere maxwell law Magnetic flux density vector
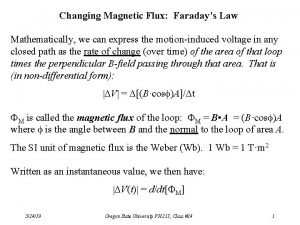
Magnetic flux density vector Change in flux equation
Change in flux equation Faraday equation
Faraday equation The annual average solar flux in tucson
The annual average solar flux in tucson Use of rope
Use of rope Diy low ropes course
Diy low ropes course Types of ropes used in the fire service
Types of ropes used in the fire service Ropes acronym
Ropes acronym Indications for suspension therapy
Indications for suspension therapy Muscles labeled
Muscles labeled Usu ropes course
Usu ropes course Tools tackles
Tools tackles Gravity ropes lubbock
Gravity ropes lubbock Magnetic moment and magnetic field relation
Magnetic moment and magnetic field relation Magnetic field and magnetic force
Magnetic field and magnetic force Solar dynamics water heater
Solar dynamics water heater Technology portfolio
Technology portfolio Solar panels wholesale
Solar panels wholesale Solar energy is free. solar is inexhaustible
Solar energy is free. solar is inexhaustible Human systems dynamics theory
Human systems dynamics theory Solar nebula
Solar nebula Astronomy
Astronomy Flux and luminosity
Flux and luminosity The flux method of labor turnover denotes:
The flux method of labor turnover denotes: Heat flux adalah
Heat flux adalah Flux de données clients
Flux de données clients Torroid
Torroid Flux of a vector field
Flux of a vector field