TESTS FOR CONVERGENCE AND DIVERGENCE Section 8 3


![Investigating Convergence Does the integral converge? Evaluate numerically: Try graphing: in [0, 20] by Investigating Convergence Does the integral converge? Evaluate numerically: Try graphing: in [0, 20] by](https://slidetodoc.com/presentation_image_h/01c86ff2affc43c5759d57defaaec514/image-5.jpg)


- Slides: 14

TESTS FOR CONVERGENCE AND DIVERGENCE Section 8. 3 b

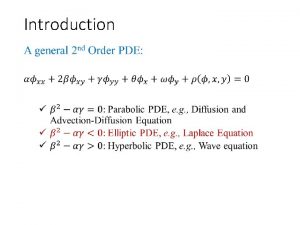
Sometimes we cannot evaluate an improper integral directly In these cases, we first try to determine whether it converges or diverges. Diverges? ? ? . . . End of story, we’re done. Converges? ? ? . . . Use numerical methods to Approximate the value of the integral.

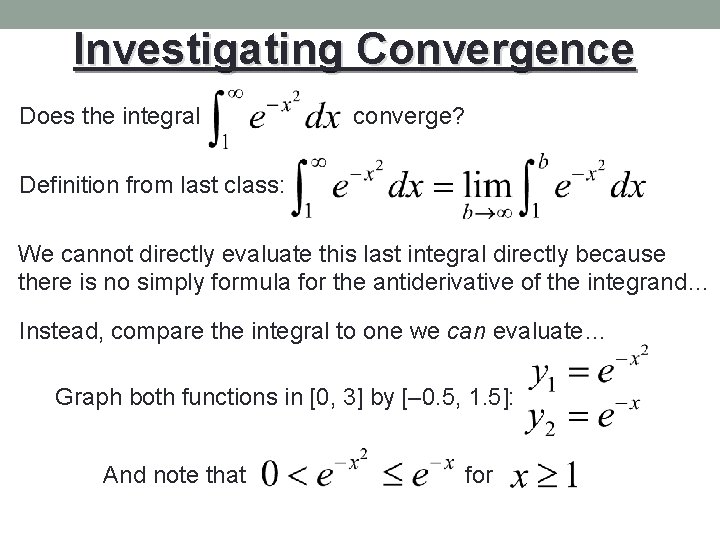
Investigating Convergence Does the integral converge? Definition from last class: We cannot directly evaluate this last integral directly because there is no simply formula for the antiderivative of the integrand… Instead, compare the integral to one we can evaluate… Graph both functions in [0, 3] by [– 0. 5, 1. 5]: And note that for

Investigating Convergence Does the integral converge? Thus, for any Because the integral of the larger function converges, the integral of the smaller function converges as well. However, this does not tell us the value of the improper integral, except that it is positive and less than 0. 368…
![Investigating Convergence Does the integral converge Evaluate numerically Try graphing in 0 20 by Investigating Convergence Does the integral converge? Evaluate numerically: Try graphing: in [0, 20] by](https://slidetodoc.com/presentation_image_h/01c86ff2affc43c5759d57defaaec514/image-5.jpg)
Investigating Convergence Does the integral converge? Evaluate numerically: Try graphing: in [0, 20] by [– 0. 1, 0. 3] What does the graph suggest as ?

Direct Comparison Test Let and be continuous on for all 1. converges if 2. diverges if and let. Then converges. diverges.

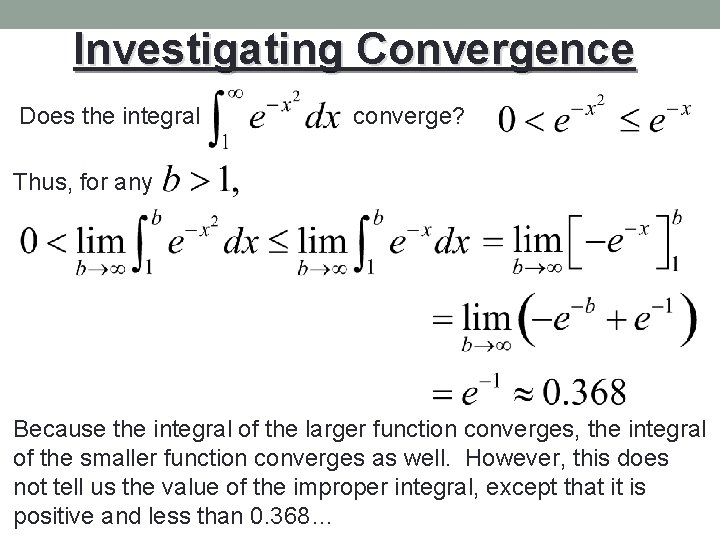
Investigating Convergence Does the integral Compare this integrand to converge? : on So because the integral of this “larger” function converges, the original integral converges as well!

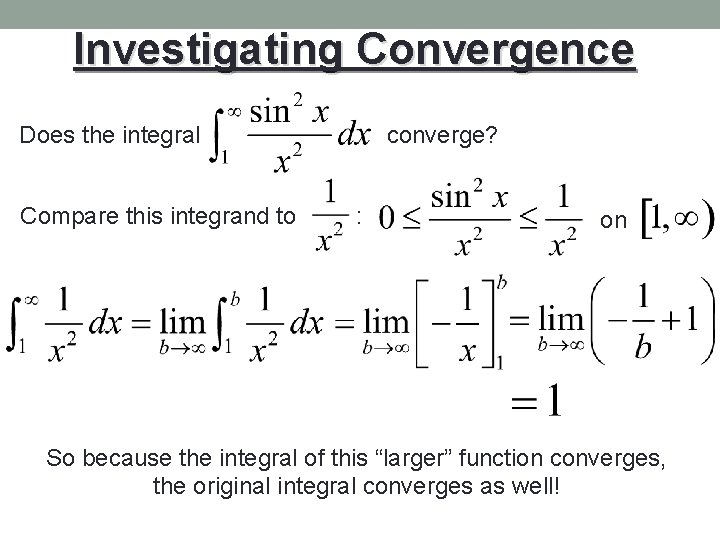
Investigating Convergence Does the integral Compare this integrand to converge? : on So because the integral of this “smaller” function diverges, the original integral diverges as well!

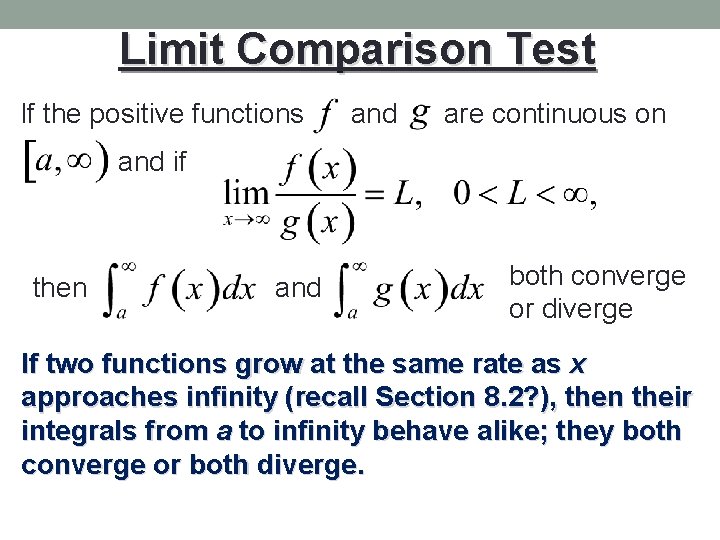
Limit Comparison Test If the positive functions and are continuous on and if then and both converge or diverge If two functions grow at the same rate as x approaches infinity (recall Section 8. 2? ), then their integrals from a to infinity behave alike; they both converge or both diverge.

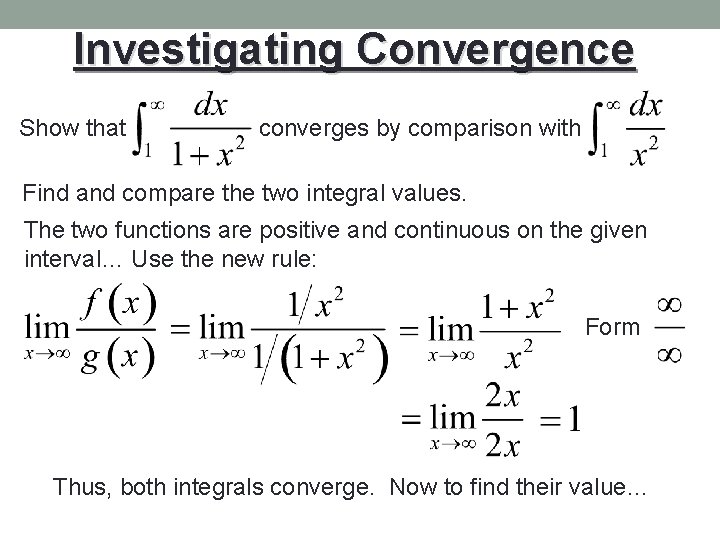
Investigating Convergence Show that converges by comparison with Find and compare the two integral values. The two functions are positive and continuous on the given interval… Use the new rule: Form Thus, both integrals converge. Now to find their value…

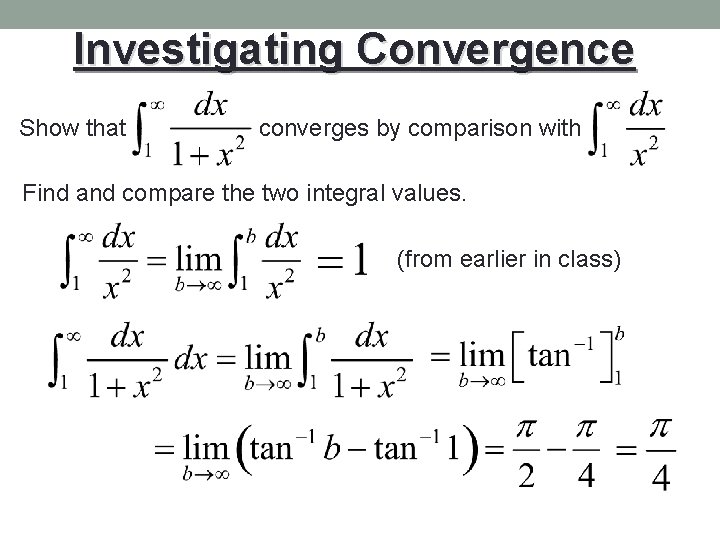
Investigating Convergence Show that converges by comparison with Find and compare the two integral values. (from earlier in class)

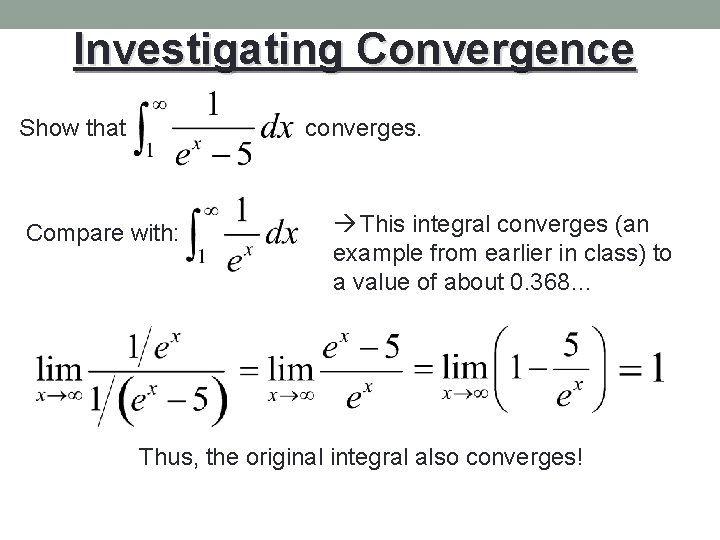
Investigating Convergence Show that converges. Compare with: This integral converges (an example from earlier in class) to a value of about 0. 368… Thus, the original integral also converges!

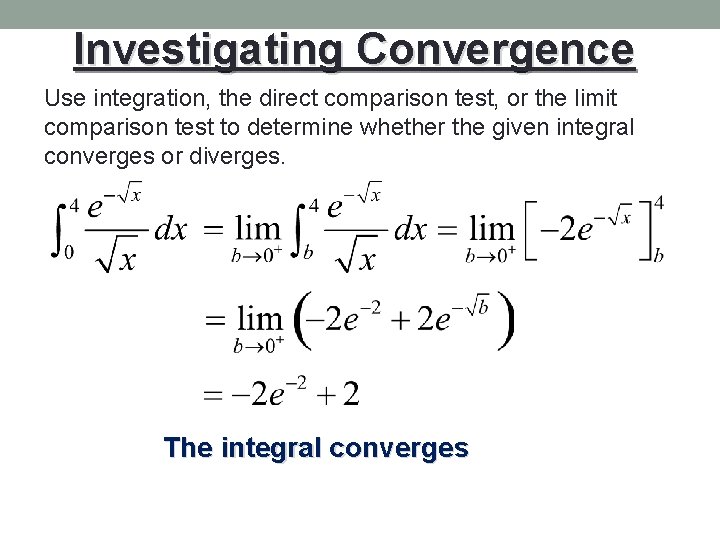
Investigating Convergence Use integration, the direct comparison test, or the limit comparison test to determine whether the given integral converges or diverges. The integral converges

Investigating Convergence Use integration, the direct comparison test, or the limit comparison test to determine whether the given integral converges or diverges. Compare to : on Since this integral diverges, the given integral diverges
 Temporal summation vs spatial summation
Temporal summation vs spatial summation Neuronal pool
Neuronal pool What is convergence in ihrm
What is convergence in ihrm Absolute convergence meaning
Absolute convergence meaning Ace different tests help iq still
Ace different tests help iq still Dissimilarities between green’s and stokes’ theorems.
Dissimilarities between green’s and stokes’ theorems. What are the phases of handwriting examination
What are the phases of handwriting examination Divergence of darkness
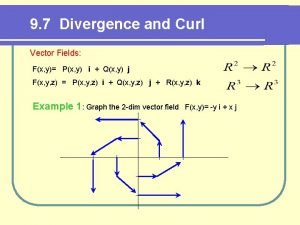
Divergence of darkness Divergence and curl of a vector field
Divergence and curl of a vector field Focus divergence tagline
Focus divergence tagline Yellow tail wine blue ocean strategy
Yellow tail wine blue ocean strategy Divergence of f
Divergence of f Pearson's measure of skewness
Pearson's measure of skewness Sel saraf divergence
Sel saraf divergence Heel of switch in railway
Heel of switch in railway