7 6 EXPONENTIAL FUNCTIONS Function Rule An equation












- Slides: 23

7. 6 EXPONENTIAL FUNCTIONS: Function Rule: An equation that describes a function. Exponent: A number that shows repeated multiplication.

GOAL:

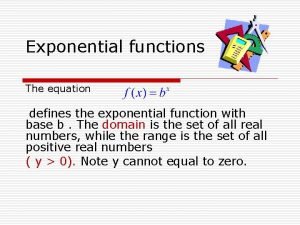
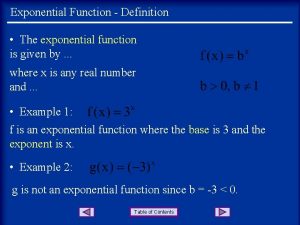
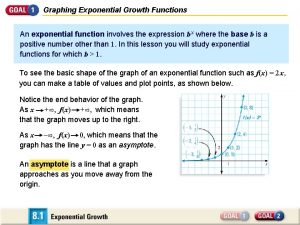
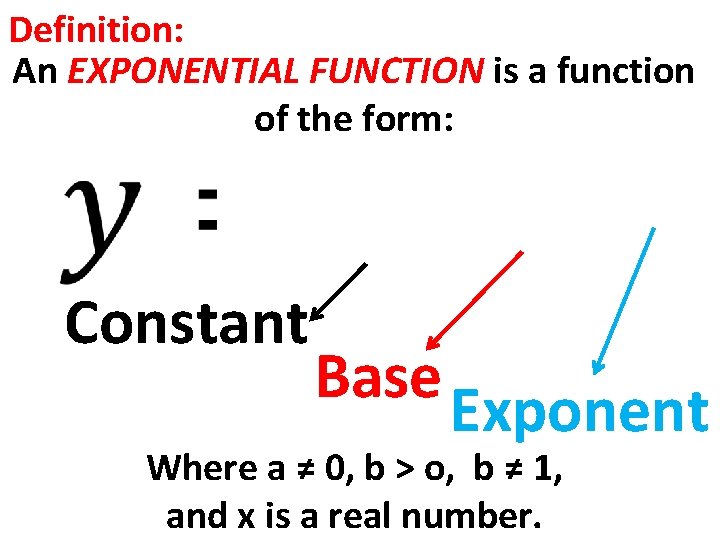
Definition: An EXPONENTIAL FUNCTION is a function of the form: Constant Base Exponent Where a ≠ 0, b > o, b ≠ 1, and x is a real number.

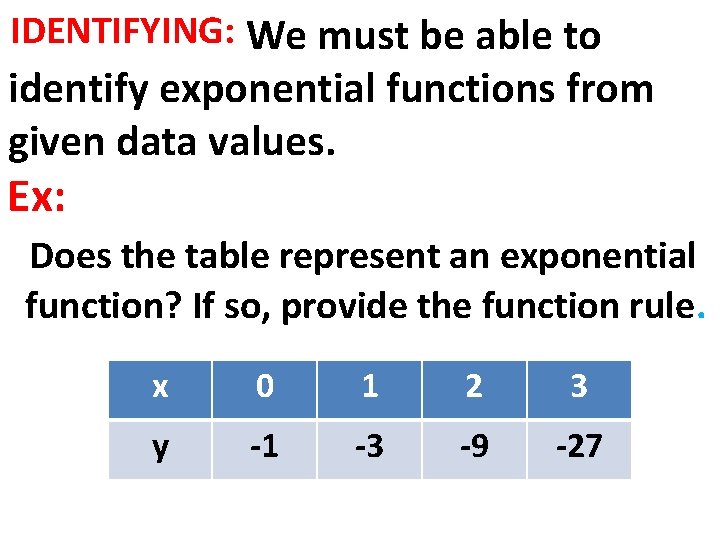
IDENTIFYING: We must be able to identify exponential functions from given data values. Ex: Does the table represent an exponential function? If so, provide the function rule. x 0 1 2 3 y -1 -3 -9 -27

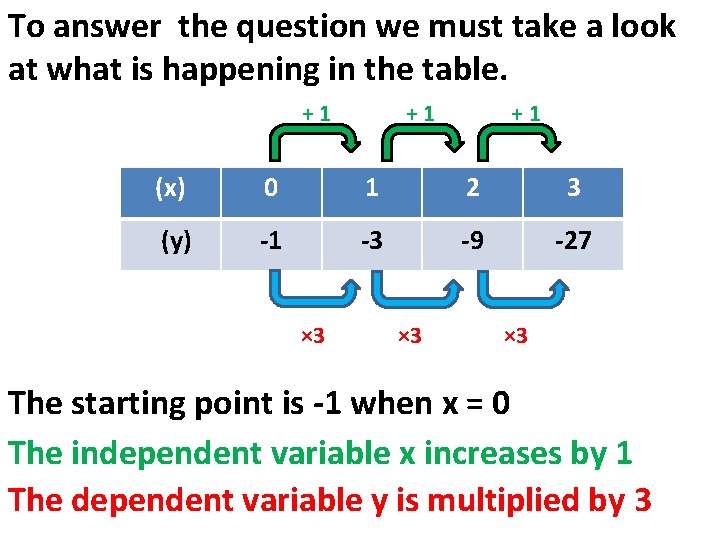
To answer the question we must take a look at what is happening in the table. +1 +1 +1 (x) 0 1 2 3 (y) -1 -3 -9 -27 × 3 × 3 The starting point is -1 when x = 0 The independent variable x increases by 1 The dependent variable y is multiplied by 3

Taking the info to consideration, we can see that the equation for the problem is: +1 (x) (y) 0 -1 +1 1 -3 × 3 +1 2 -9 × 3 3 - 27 x y=a∙b × 3 Here the difference of × 3 becomes the base. Notice: we begin with -1 when x = 0 or a = -1 x y=a∙b y= x -1∙ 3

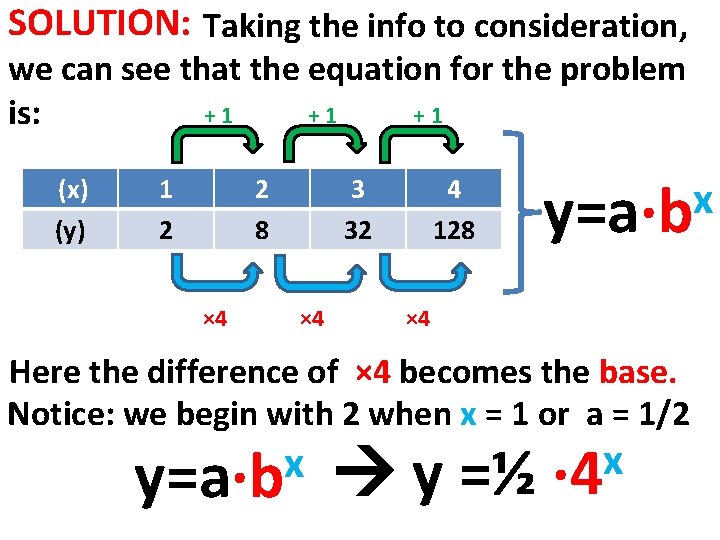
YOU TRY IT: Does the table represent an exponential function? If so, provide the function rule. x 1 2 3 4 y 2 8 32 128

SOLUTION: Taking the info to consideration, we can see that the equation for the problem +1 +1 +1 is: (x) (y) 1 2 2 8 × 4 3 32 × 4 4 128 x y=a∙b × 4 Here the difference of × 4 becomes the base. Notice: we begin with 2 when x = 1 or a = 1/2 x y=a∙b y =½ x ∙ 4

Summary: Linear Functions: y = mx + b The difference in the independent variable (y) is in form of addition or subtraction. Exponential Equations: y = abx The difference in the independent variable (y) is multiplication

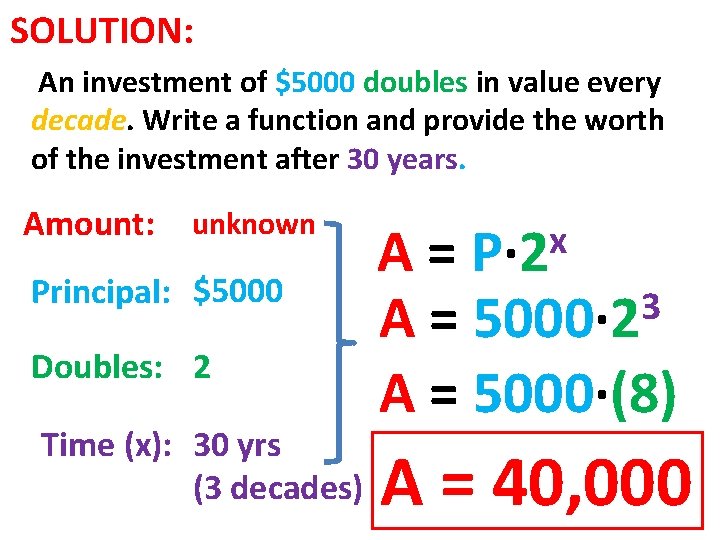
EVALUATING: We must be able to evaluate exponential functions. Ex: An investment of $5000 doubles in value every decade. Write a function and provide the worth of the investment after 30 years.

EVALUATING: To provide the solution we must know the following formula: A= x P∙ 2 A = total P = Principal (starting amount) 2 = doubles x = time

SOLUTION: An investment of $5000 doubles in value every decade. Write a function and provide the worth of the investment after 30 years. Amount: unknown Principal: $5000 Doubles: 2 Time (x): 30 yrs (3 decades) x P∙ 2 A= 3 A = 5000∙ 2 A = 5000∙(8) A = 40, 000

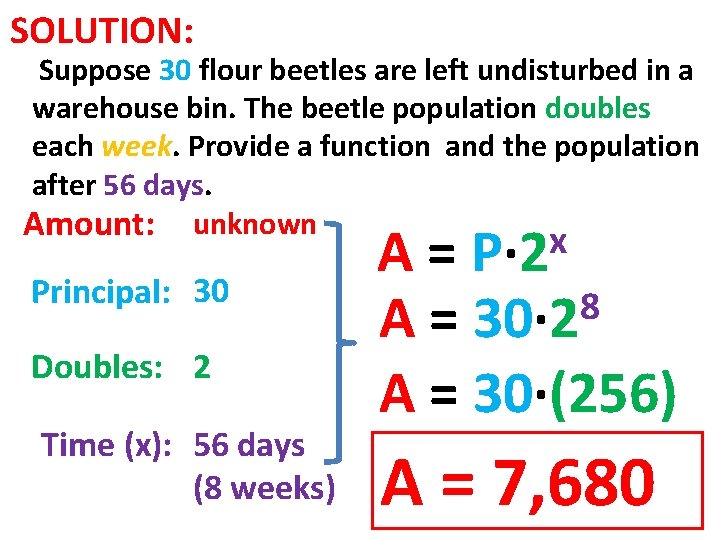
YOU TRY IT: Suppose 30 flour beetles are left undisturbed in a warehouse bin. The beetle population doubles each week. Provide a function and the population after 56 days.

SOLUTION: Suppose 30 flour beetles are left undisturbed in a warehouse bin. The beetle population doubles each week. Provide a function and the population after 56 days. Amount: unknown Principal: 30 Doubles: 2 Time (x): 56 days (8 weeks) x P∙ 2 A= 8 A = 30∙ 2 A = 30∙(256) A = 7, 680

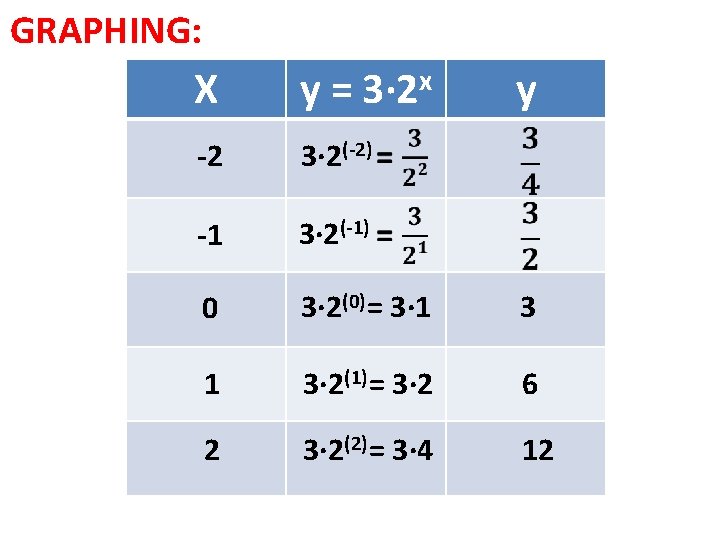
GRAPHING: To provide the graph of the equation we can go back to basics and create a table. Ex: What is the graph of y = 3∙ 2 x?

GRAPHING: X y = 3∙ 2 x y -2 3∙ 2(-2) -1 3∙ 2(-1) 0 3∙ 2(0) = 3∙ 1 3∙ 2(1) = 3∙ 2 6 2 3∙ 2(2) = 3∙ 4 12

GRAPHING: X y -2 -1 0 3 1 6 2 12 This graph grows fast = Exponential Growth

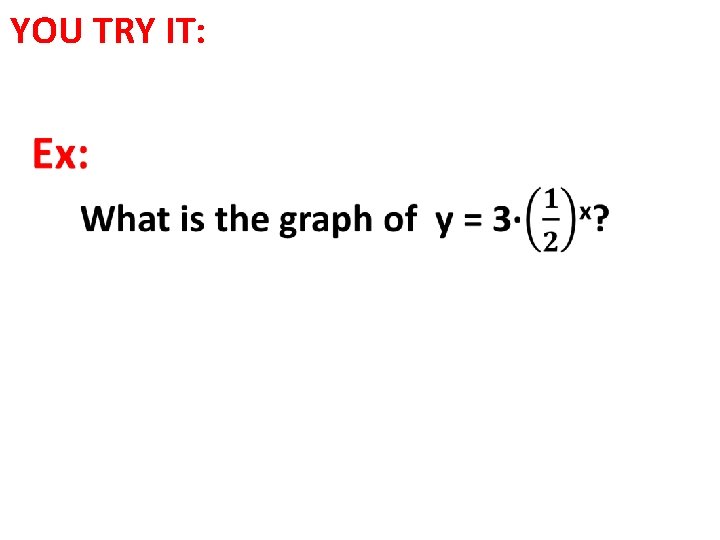
YOU TRY IT:

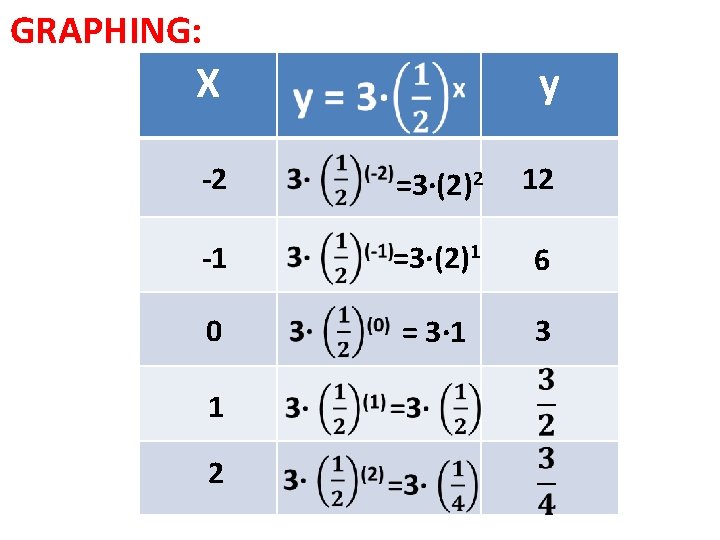
GRAPHING: X y -2 =3∙(2)2 12 -1 =3∙(2)1 6 0 = 3∙ 1 3 1 2

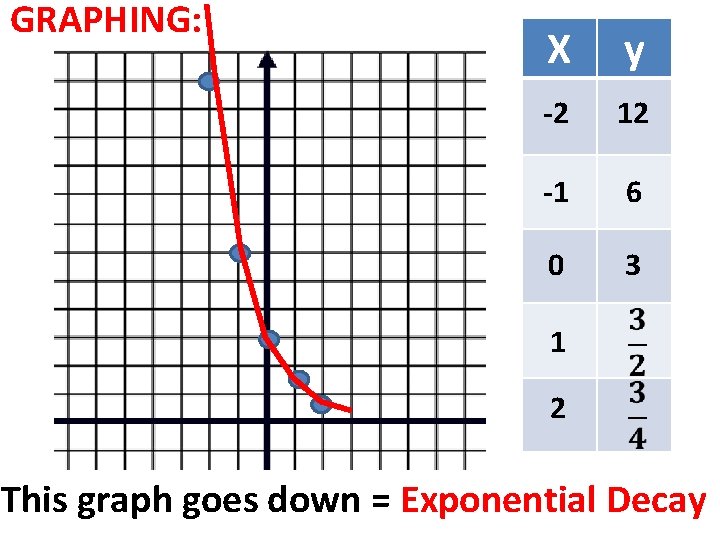
GRAPHING: X y -2 12 -1 6 0 3 1 2 This graph goes down = Exponential Decay

VIDEOS: Exponential Functions Growth https: //www. khanacademy. org/math/trigonometry/expo nential_and_logarithmic_func/exp_growth_decay/v/expo nential-growth-functions Graphing https: //www. khanacademy. org/math/trigonometry/expo nential_and_logarithmic_func/exp_growth_decay/v/graph ing-exponential-functions

VIDEOS: Exponential Functions Decay https: //www. khanacademy. org/math/trigonometry/expo nential_and_logarithmic_func/exp_growth_decay/v/wordproblem-solving--exponential-growth-and-decay

CLASSWORK: Page 450 -451: Problems: As many as needed to master the concept.
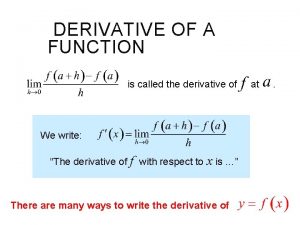
 Derivative formula
Derivative formula What is the parent function for exponential functions
What is the parent function for exponential functions Exponential math meaning
Exponential math meaning The inverse of exponential function is a composite function
The inverse of exponential function is a composite function F(x)
F(x) Integration of exponential function
Integration of exponential function Logarithm formula
Logarithm formula Integral power rule
Integral power rule Integration of exponential
Integration of exponential Common ratio of exponential functions
Common ratio of exponential functions Unit 8 review logarithms
Unit 8 review logarithms 7-1 graphing exponential functions
7-1 graphing exponential functions Unit 5: exponential and logarithmic functions answers
Unit 5: exponential and logarithmic functions answers Example of exponential equations
Example of exponential equations Table for quadratic function
Table for quadratic function Explicit and recursive formula
Explicit and recursive formula Exponential growth and decay algebra 1
Exponential growth and decay algebra 1 Domain of exponential functions
Domain of exponential functions 6-2 lesson quiz exponential functions
6-2 lesson quiz exponential functions Definition of
Definition of Exponential function transformation
Exponential function transformation 7-1 exponential functions growth and decay
7-1 exponential functions growth and decay 6-3 lesson quiz exponential growth and decay
6-3 lesson quiz exponential growth and decay Find inverse of exponential function
Find inverse of exponential function