3 1 Radians Angles are measured in degrees

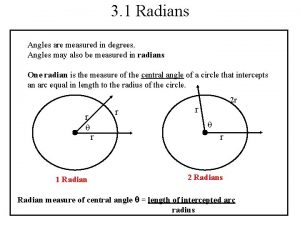
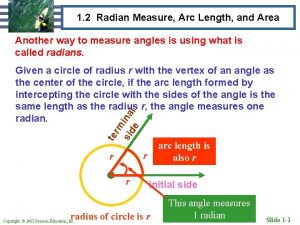
3. 1 Radians Angles are measured in degrees. Angles may also be measured in radians One radian is the measure of the central angle of a circle that intercepts an arc equal in length to the radius of the circle. r r 1 Radian 2 r r 2 Radians Radian measure of central angle = length of intercepted arc radius

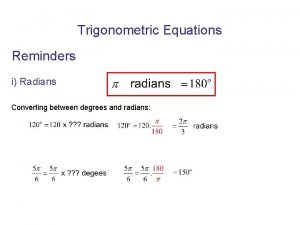
Degrees and Radians 90 degrees/ radians 2 180 degrees radians 0 degrees/ 0 radians 360 degrees/ 2 radians r 270 degrees/ 3 radians 2 The measure of the arc of a whole circle is 360° The length of the arc surrounding the whole circle is 2 r (the circumference) The radian measure of a whole circle central angle is: length of intercepted arc = 2 r radius So, 360° = 2 radians r = 2

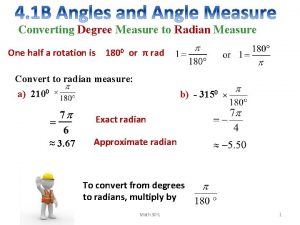
Converting radians = 180 degrees, so. . . To convert from degrees to radians, multiply degrees by radians 180° Example: Convert 120 degrees to radians 120° • radians = 120 radians = 2 radians 180° 3 To convert from radians to degrees, multiply by 180° radians Example: Convert 5 radians to degrees 6 5 • 180° = 5(180) = 150° 6 6

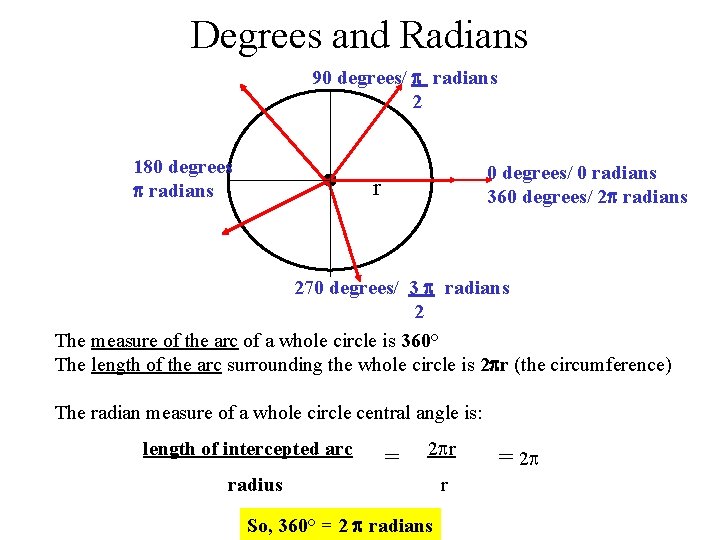
3. 2 Arc Length of a Circle Radian measure of central angle = length of intercepted arc radius Length of intercepted arc = (radius)( in radians) Example 1: A circle has radius of 18. 2 cm. Find the length of the arc intercepted by a central angle of 144º Convert 144º to radians. (144) ( /180) = 4 radians 5 Length of arc = (18. 2)(4 /5) = 45. 74 cm 144º 18. 2

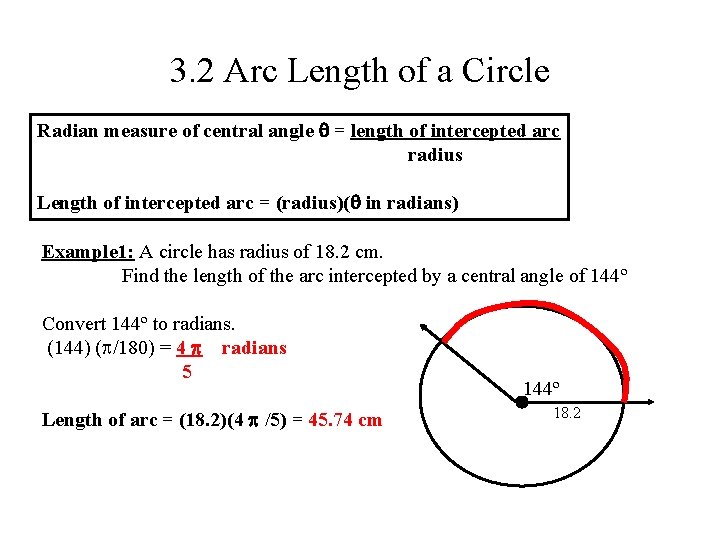
Example 2 USING LATITUDES TO FIND THE DISTANCE BETWEEN TWO CITIES • Reno, Nevada is approximately due north of Los Angeles. The latitude of Reno is 40° N, while that of Los Angeles is 34° N. The radius of Earth is about 6400 km. Find the north-south distance between the two cities. The central angle between Reno and Los Angeles is 40° – 34° = 6°. Convert 6° to radians: 3. 2 -5

Example 2 USING LATITUDES TO FIND THE DISTANCE BETWEEN TWO CITIES (continued) • Use s = rθ to find the north-south distance between the two cities. The north-south distance between Reno and Los Angeles is about 670 km. 3. 2 -6

Example 3 FINDING A LENGTH USING s = rθ A rope is being wound around a drum with radius. 8725 ft. How much rope will be wound around the drum if the drum is rotated through an angle of 39. 72°? The length of rope wound around the drum is the arc length for a circle of radius. 8725 ft and a central angle of 39. 72°. 3. 2 -7

Example 3 FINDING A LENGTH USING s = rθ (continued) • Use s = rθ to find the arc length, which is the length of the rope. Remember to convert 39. 72° to radians The length of the rope wound around the drum is about. 6049 ft. 3. 2 -8

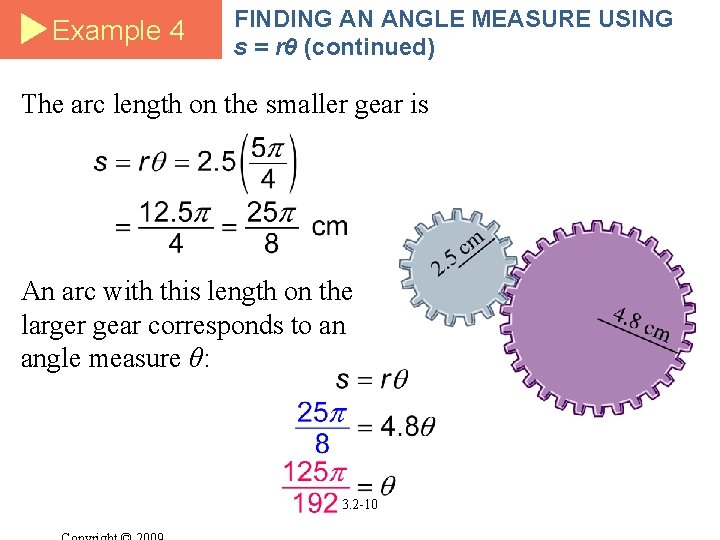
Example 4 FINDING AN ANGLE MEASURE USING s = rθ • Two gears are adjusted so that the smaller gear drives the larger one. If the smaller gear rotates through an angle of 225°, through how many degrees will the larger gear rotate? First find the radian measure of the angle, and then find the arc length on the smaller gear that determines the motion of the larger gear. 3. 2 -9

Example 4 FINDING AN ANGLE MEASURE USING s = rθ (continued) The arc length on the smaller gear is An arc with this length on the larger gear corresponds to an angle measure θ: 3. 2 -10

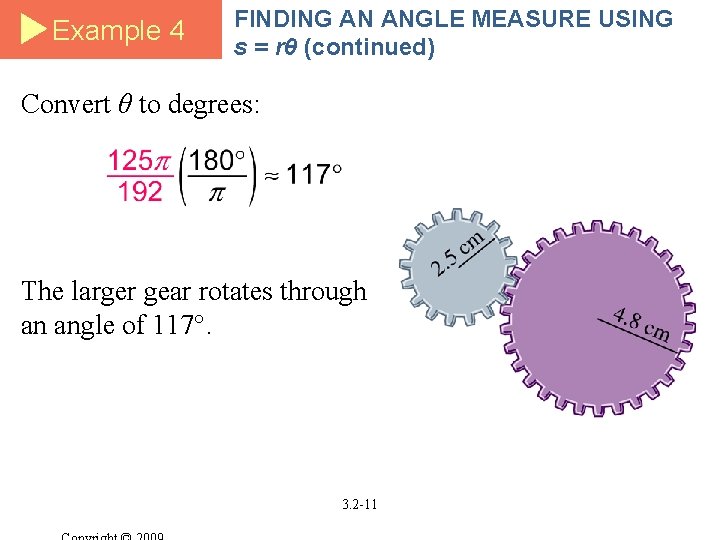
Example 4 FINDING AN ANGLE MEASURE USING s = rθ (continued) Convert θ to degrees: The larger gear rotates through an angle of 117°. 3. 2 -11

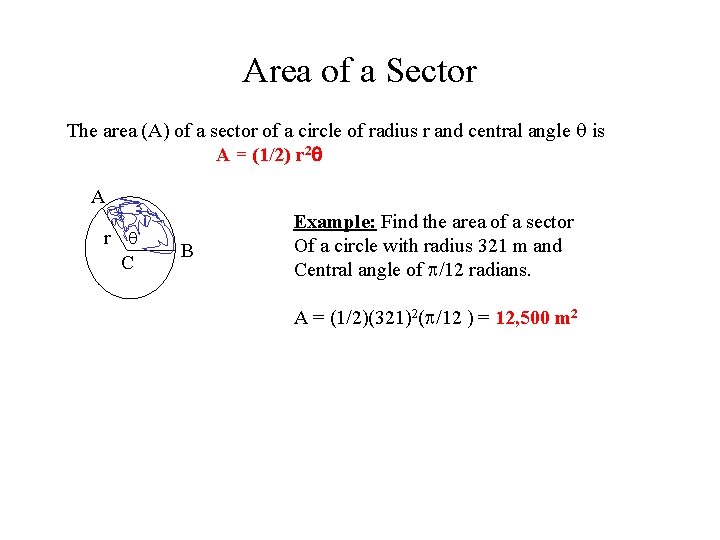
Area of a Sector The area (A) of a sector of a circle of radius r and central angle is A = (1/2) r 2 A r C B Example: Find the area of a sector Of a circle with radius 321 m and Central angle of /12 radians. A = (1/2)(321)2( /12 ) = 12, 500 m 2

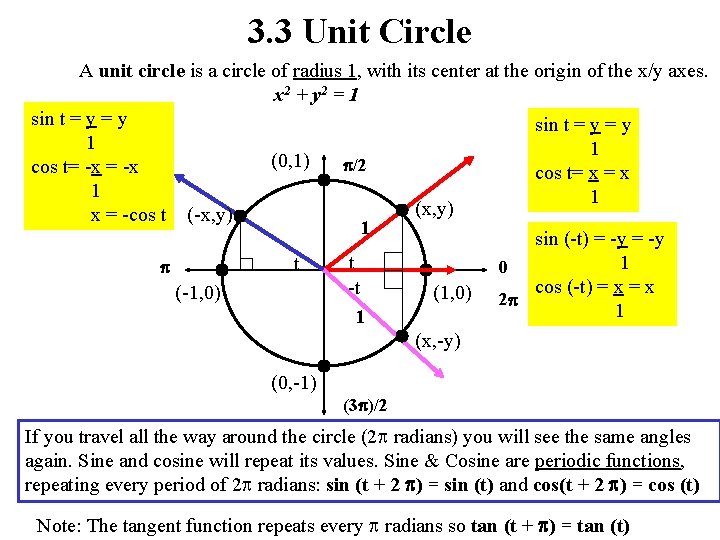
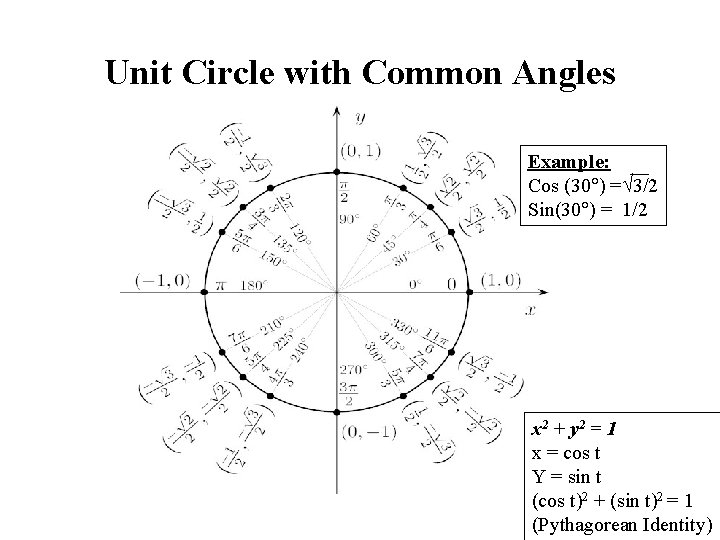
3. 3 Unit Circle A unit circle is a circle of radius 1, with its center at the origin of the x/y axes. x 2 + y 2 = 1 sin t = y = y 1 1 (0, 1) /2 cos t= -x cos t= x 1 1 (x, y) x = -cos t (-x, y) 1 sin (-t) = -y t 1 t 0 -t (-1, 0) (1, 0) 2 cos (-t) = x 1 1 (x, -y) (0, -1) (3 )/2 If you travel all the way around the circle (2 radians) you will see the same angles again. Sine and cosine will repeat its values. Sine & Cosine are periodic functions, repeating every period of 2 radians: sin (t + 2 ) = sin (t) and cos(t + 2 ) = cos (t) Note: The tangent function repeats every radians so tan (t + ) = tan (t)

Unit Circle with Common Angles Example: Cos (30 ) = 3/2 Sin(30 ) = 1/2 x 2 + y 2 = 1 x = cos t Y = sin t (cos t)2 + (sin t)2 = 1 (Pythagorean Identity)

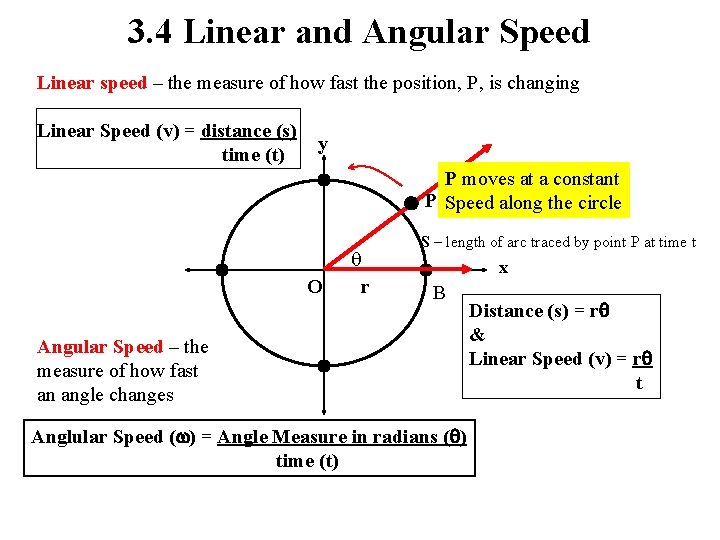
3. 4 Linear and Angular Speed Linear speed – the measure of how fast the position, P, is changing Linear Speed (v) = distance (s) time (t) y P moves at a constant P Speed along the circle O r S – length of arc traced by point P at time t x B Angular Speed – the measure of how fast an angle changes Anglular Speed ( ) = Angle Measure in radians ( ) time (t) Distance (s) = r & Linear Speed (v) = r t

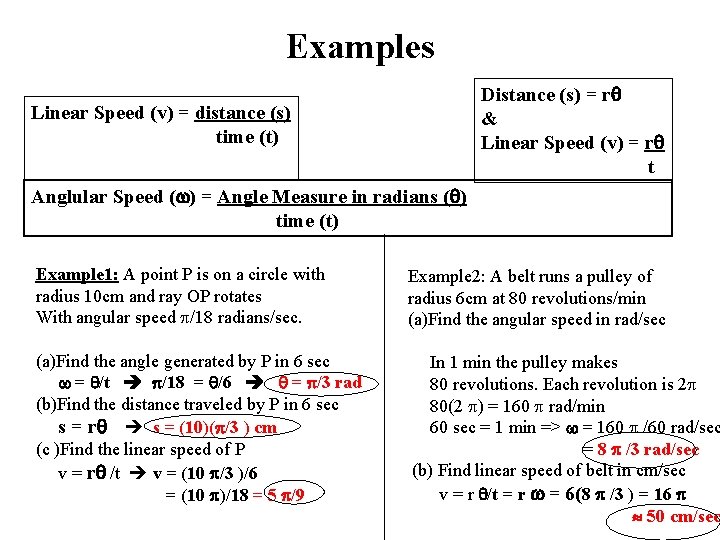
Examples Distance (s) = r & Linear Speed (v) = r t Linear Speed (v) = distance (s) time (t) Anglular Speed ( ) = Angle Measure in radians ( ) time (t) Example 1: A point P is on a circle with radius 10 cm and ray OP rotates With angular speed /18 radians/sec. Example 2: A belt runs a pulley of radius 6 cm at 80 revolutions/min (a)Find the angular speed in rad/sec (a)Find the angle generated by P in 6 sec = /t /18 = /6 = /3 rad (b)Find the distance traveled by P in 6 sec s = r s = (10)( /3 ) cm (c )Find the linear speed of P v = r /t v = (10 /3 )/6 = (10 )/18 = 5 /9 In 1 min the pulley makes 80 revolutions. Each revolution is 2 80(2 ) = 160 rad/min 60 sec = 1 min => = 160 /60 rad/sec = 8 /3 rad/sec (b) Find linear speed of belt in cm/sec v = r /t = r = 6(8 /3 ) = 16 50 cm/sec